Measurements of the Effective Stress Coefficient for Elastic Moduli of Sandstone in Quasi-Static Regime Using Semiconductor Strain Gauges
Abstract
1. Introduction
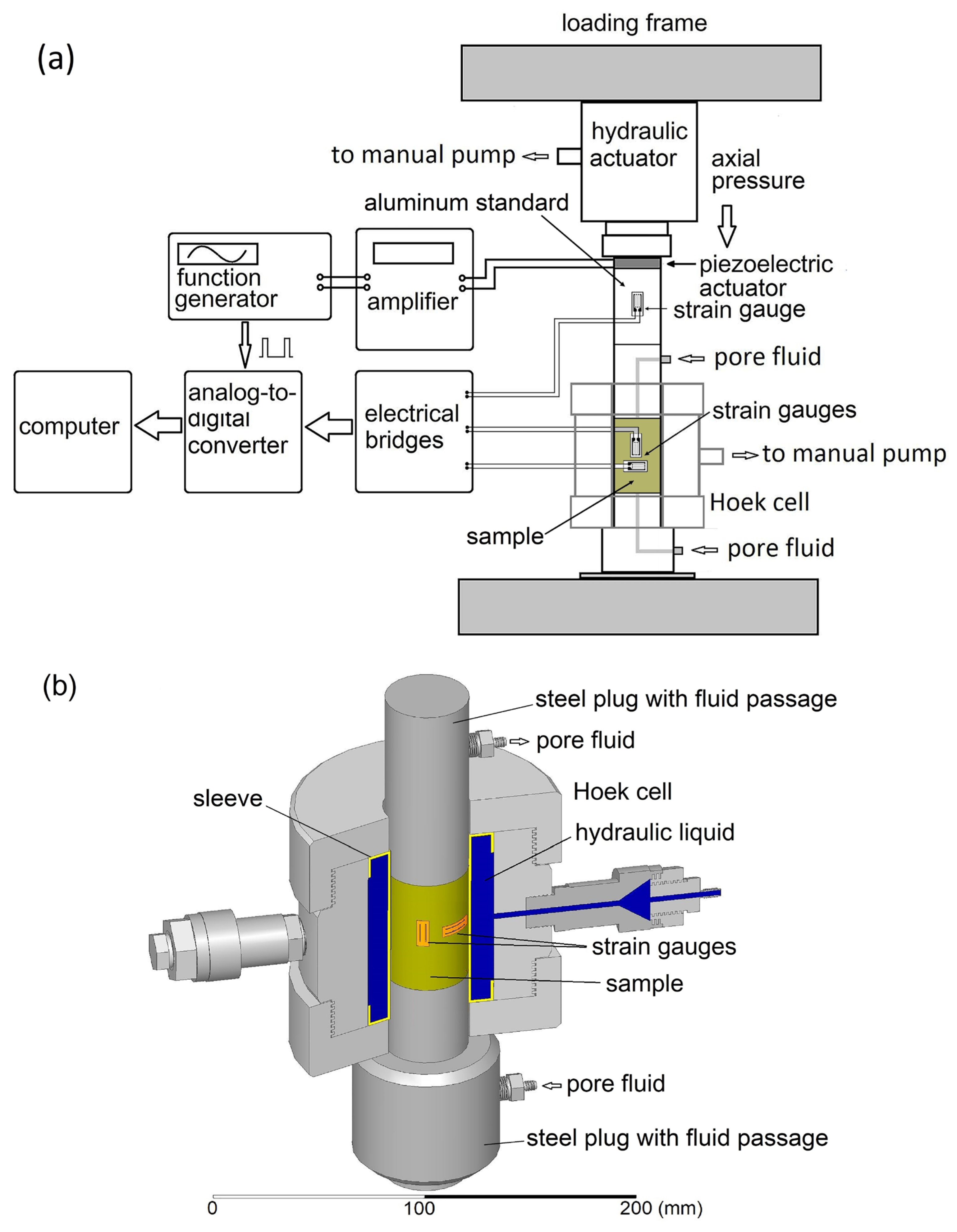
2. Experimental Setup
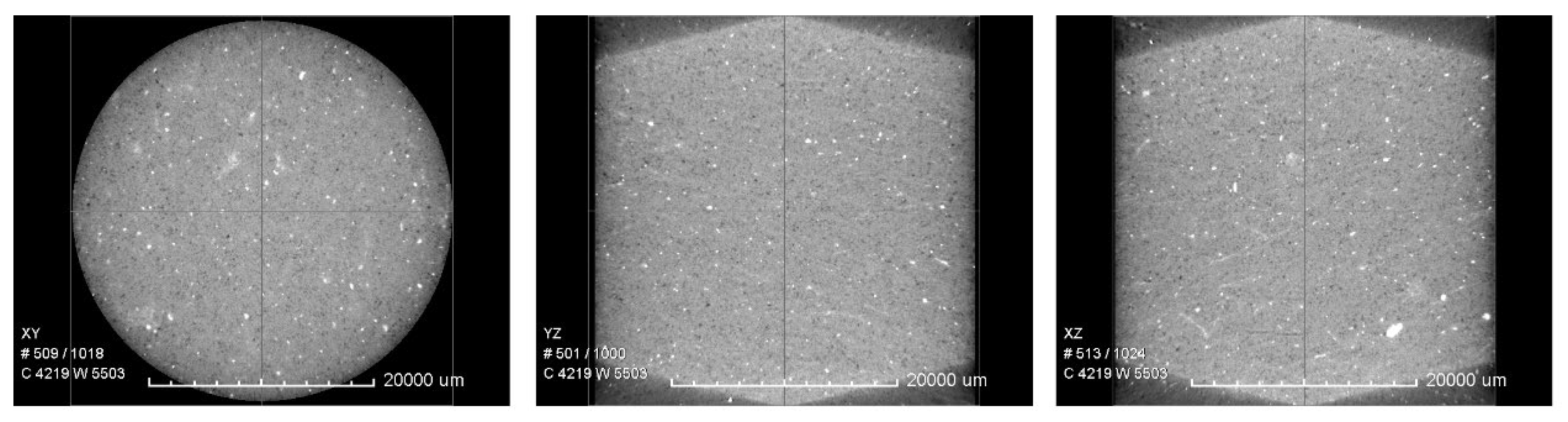
3. Sample Description and Experimental Procedure
| Mineral | Content (Volume), % | Bulk Modulus, GPa |
|---|---|---|
| Quartz | 72 | 36.6 [39] |
| K-feldspar | 2 | 57 [40] |
| Micrite | 4 | 71 [41] |
| Illite | 5 | 21 [42] |
| Kaolinite | 12 | 11 [43] |
| Calcite | 4 | 76.8 [39] |
4. Results and Discussion
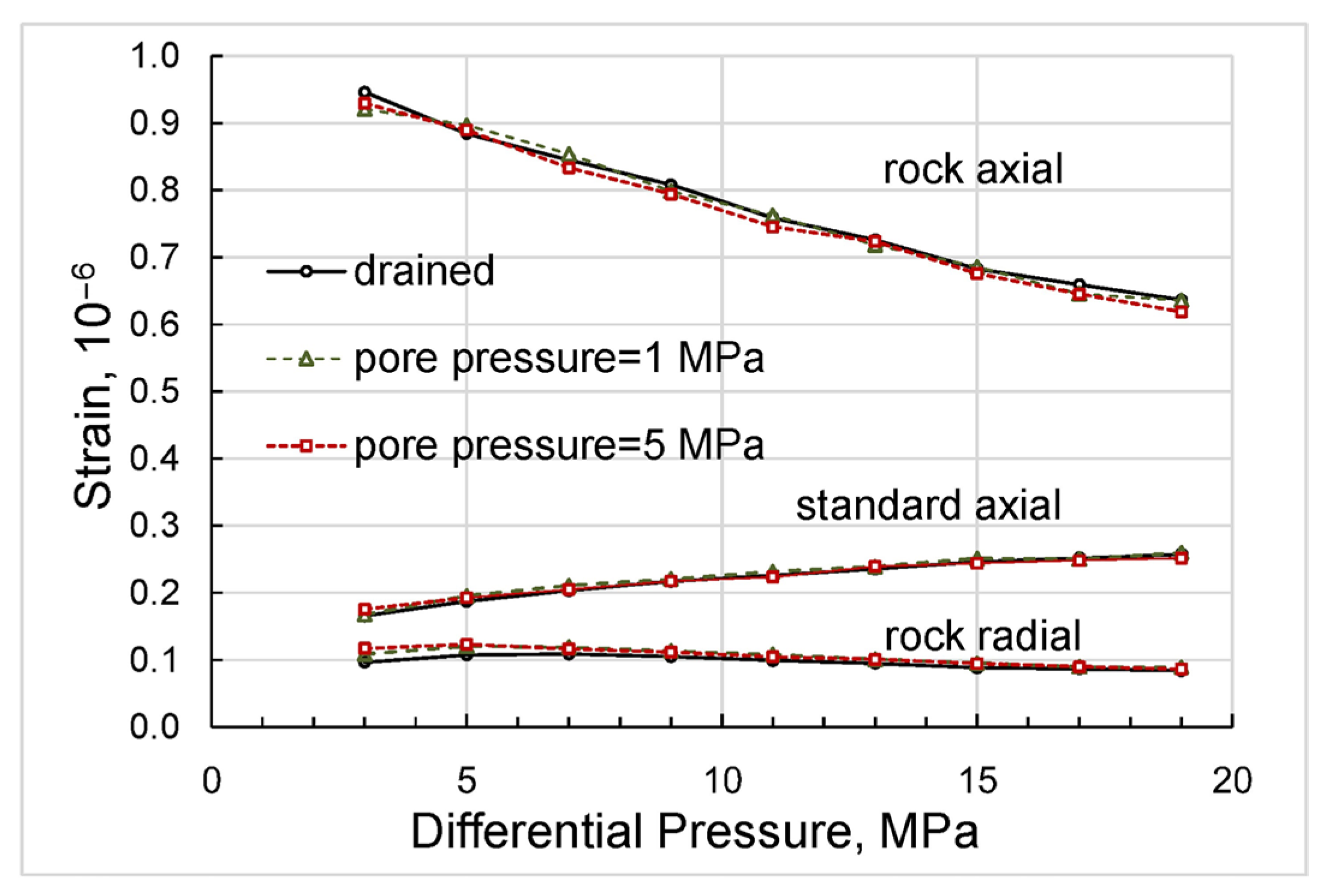
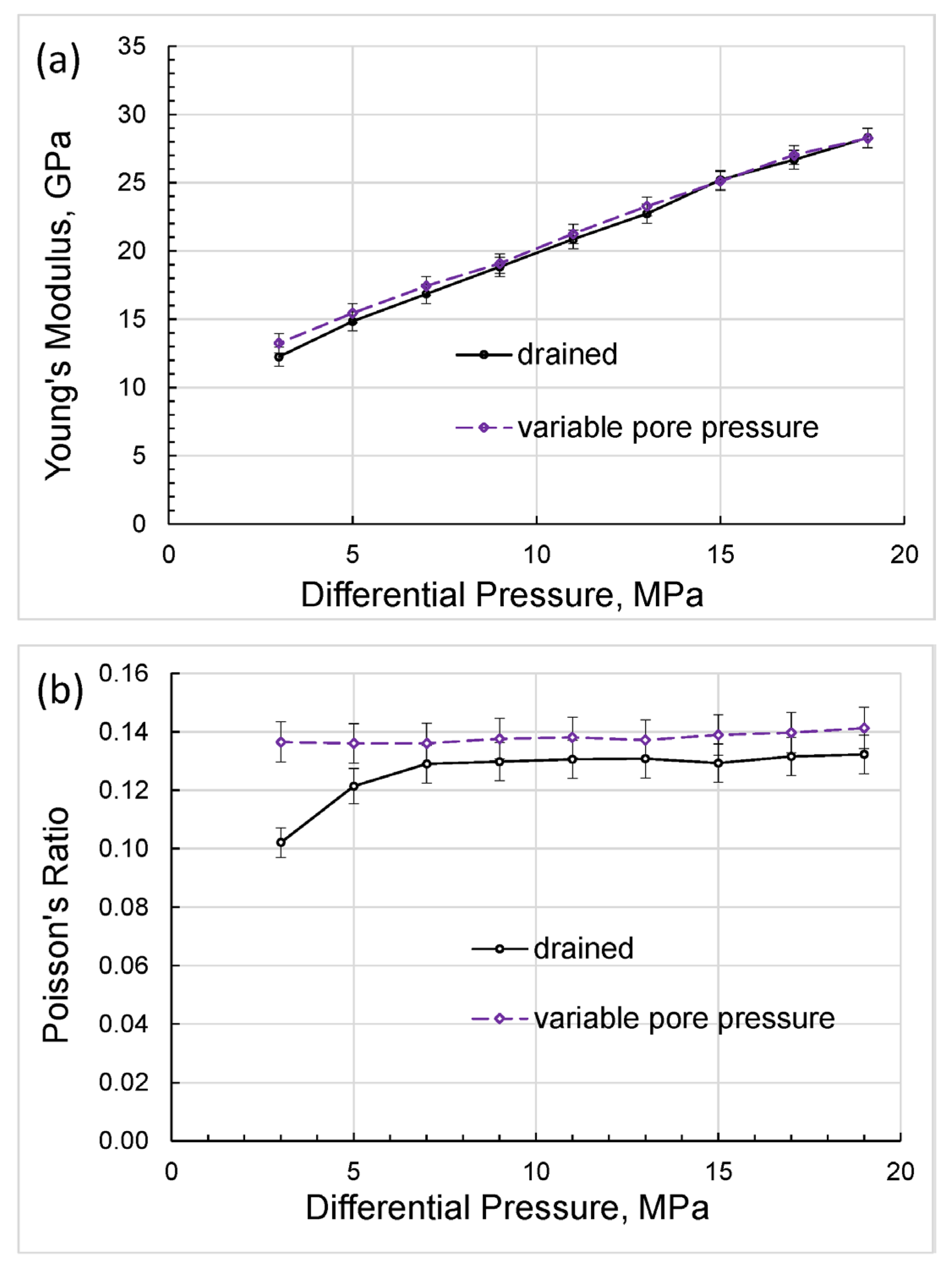
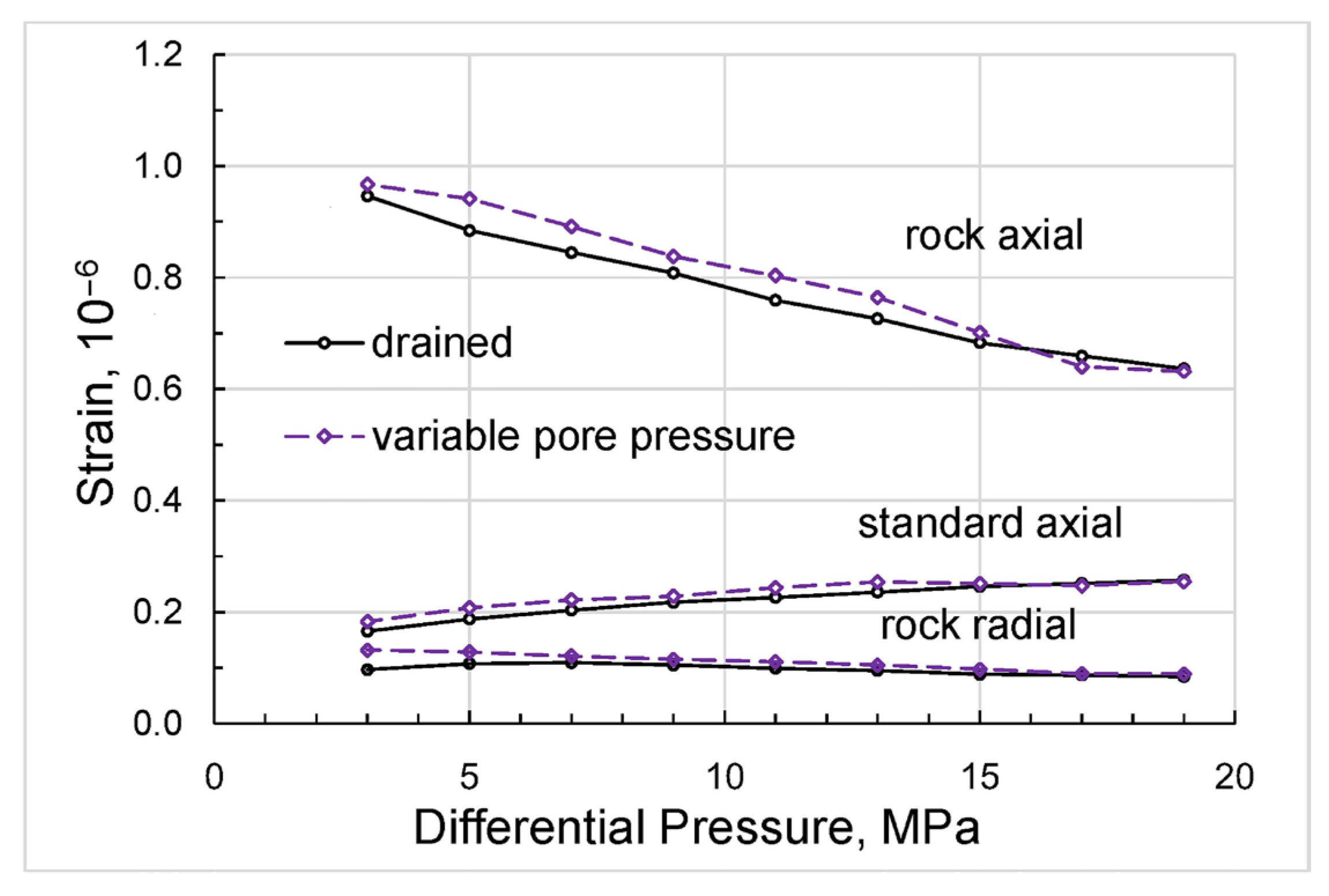
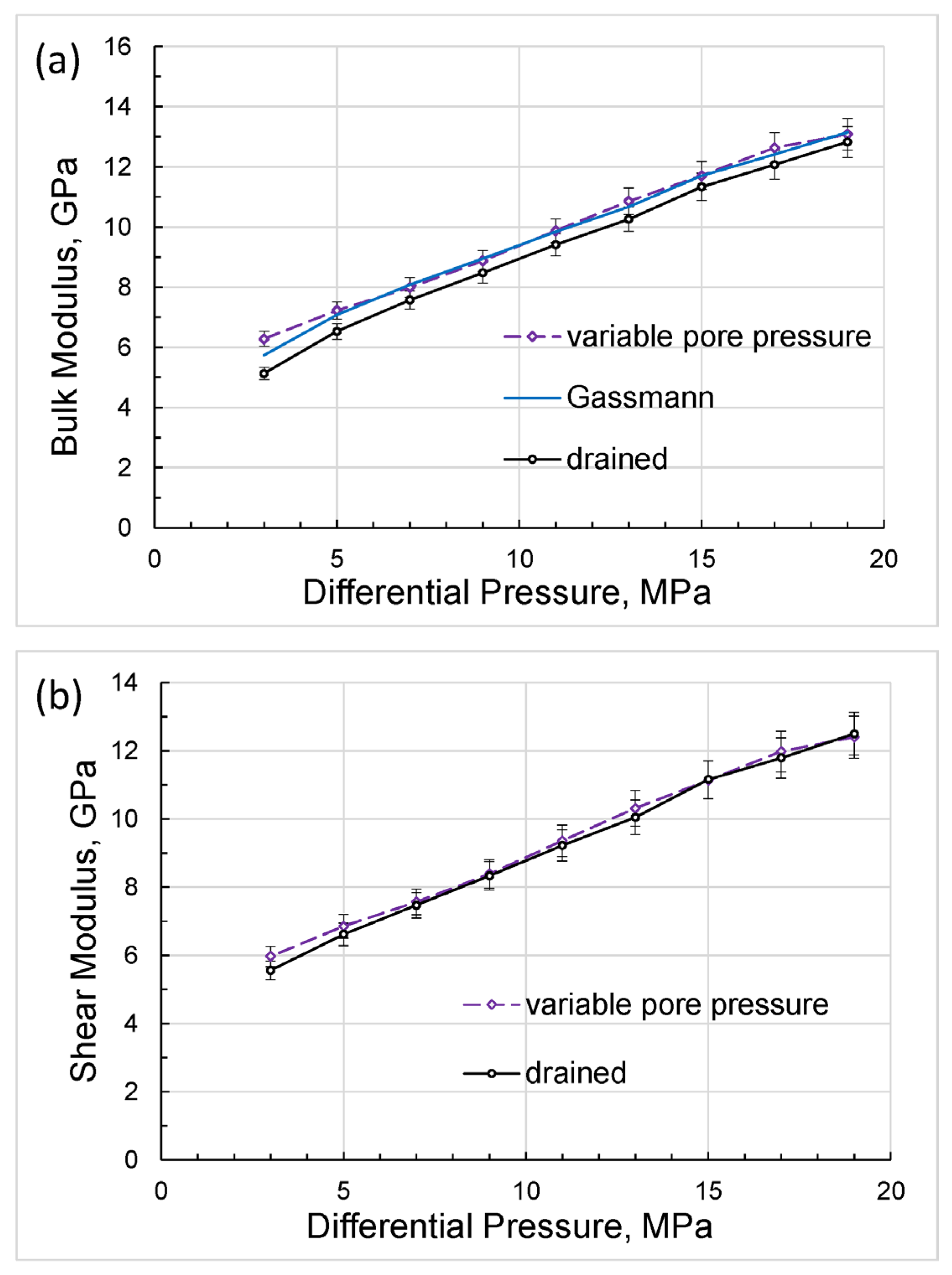
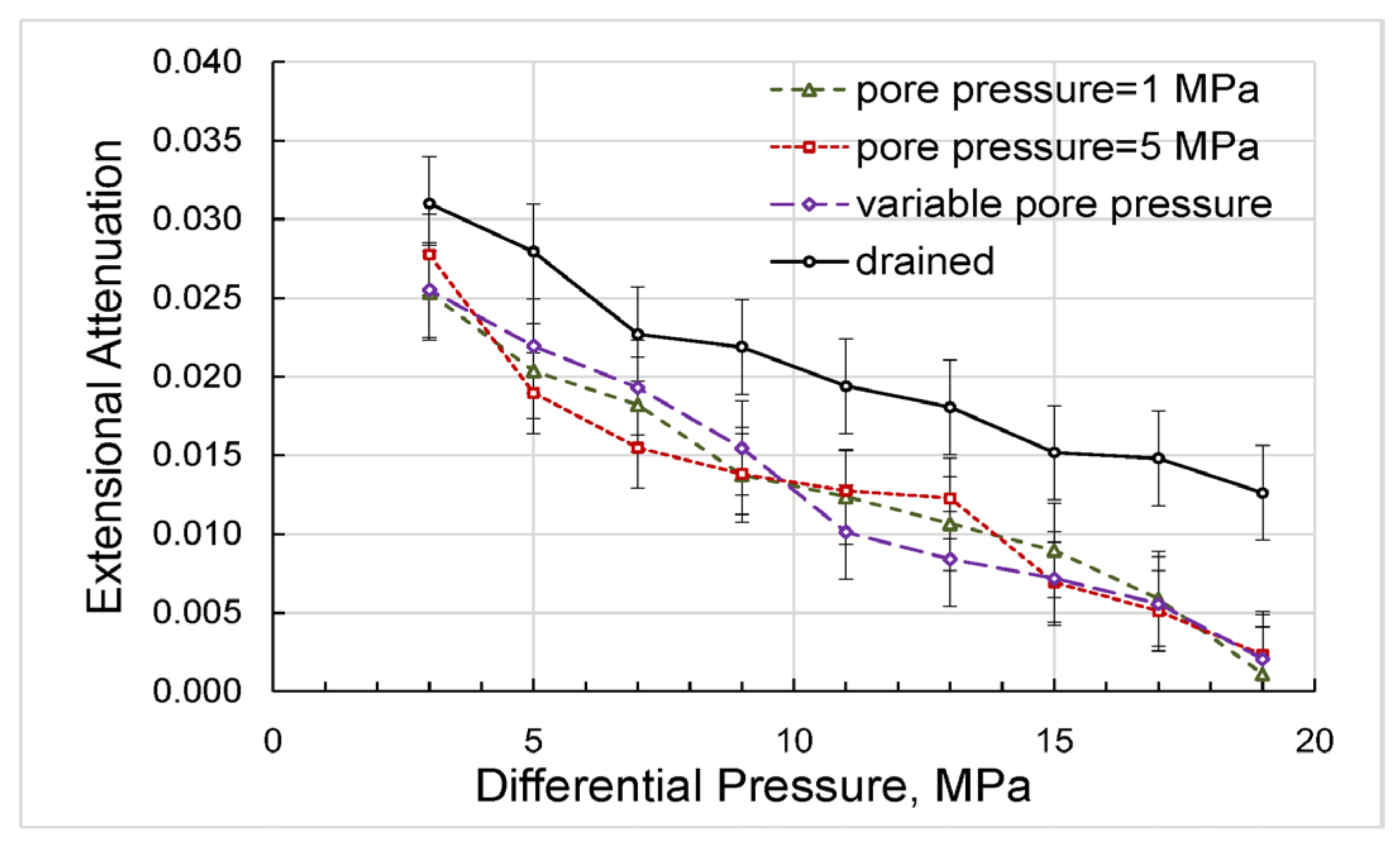
4.1. Measurements with Variable Confining Pressure
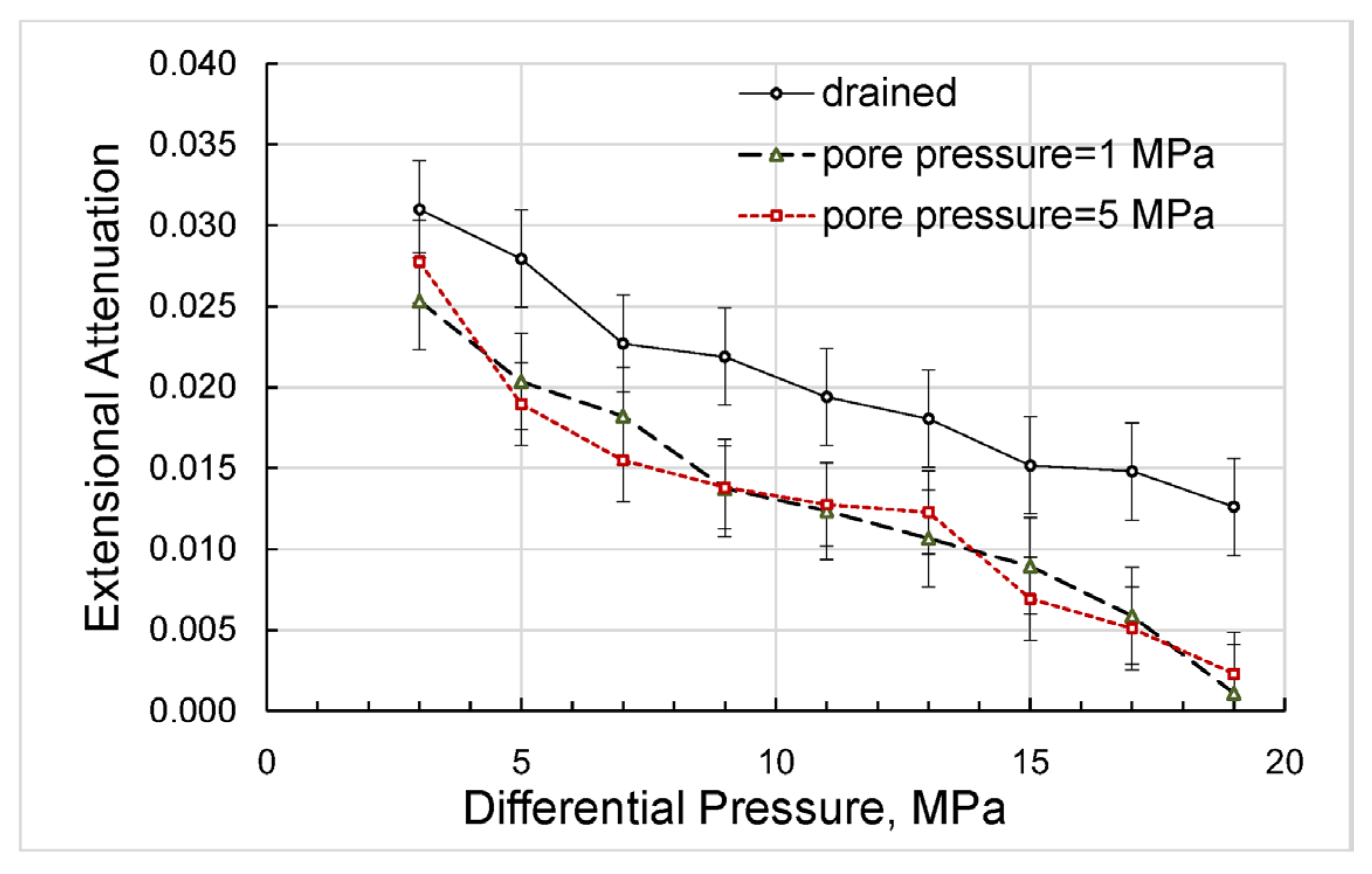
4.2. Measurements with Variable Pore Pressure
4.3. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Terzaghi, K. Theoretical Soil Mechanics; Wiley: New York, NY, USA, 1943. [Google Scholar] [CrossRef]
- Skempton, A.W. Effective stress in soils, concrete and rock. Select. Pap. Soil. Mech. 1960, 1032, 4–16. [Google Scholar] [CrossRef]
- Landrø, M. Discrimination between pressure and fluid saturation changes from time lapse seismic data. Geophysics 2001, 66, 836–844. [Google Scholar] [CrossRef]
- Vasco, D.W. Seismic imaging of reservoir flow properties: Time-lapse pressure changes. Geophysics 2004, 69, 511–521. [Google Scholar] [CrossRef][Green Version]
- Chen, S.; Zhao, Z.; Chen, Y.; Yang, Q. On the effective stress coefficient of saturated fractured rocks. Comput. Geotech. 2020, 123, 103564. [Google Scholar] [CrossRef]
- Avseth, P.; Mukerji, T.; Mavko, G. Quantitative Seismic Interpretation: Applying Rock Physics Tools to Reduce Interpretation Risk; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar] [CrossRef]
- Nur, A.; Byerlee, J.D. An exact effective stress law for elastic deformation of rock with fluids. J. Geophys. Res. 1971, 76, 6414–6419. [Google Scholar] [CrossRef]
- Carroll, M.M. An effective stress law for anisotropic elastic deformation. J. Geophys. Res. 1979, 84, 7510–7512. [Google Scholar] [CrossRef]
- Thompson, M.; Willis, J.R. A reformation of the equations of anisotropic poroelasticity. J. Appl. Mech. 1991, 58, 612–616. [Google Scholar] [CrossRef]
- Detournay, E.; Cheng, A.H.D. Fundamentals of poroelasticity. In Comprehensive Rock Engineering: Principles, Practice and Projects, Volume 2, Analysis and Design Method; Fairhurst, C., Ed.; Pergamon Press: Oxford, UK, 1993; pp. 113–171. [Google Scholar] [CrossRef]
- Cheng, A.H.-D. Material coefficients of anisotropic poroelasticity. Int. J. Rock Mech. Min. Sci. 1997, 34, 199–205. [Google Scholar] [CrossRef]
- Biot, M.A.; Willis, D.G. The elastic coefficients of the theory of consolidation. J. Appl. Mech. 1957, 24, 594–601. [Google Scholar] [CrossRef]
- Geertsma, J. The effect of fluid pressure decline on volumetric changes of porous rocks. Petrol. Trans. AIME 1957, 210, 331–340. [Google Scholar] [CrossRef]
- Gardner, G.H.F.; Wyllie, M.R.J.; Droschak, D.M. Hysteresis in the velocity-pressure characteristics of rocks. Geophysics 1965, 30, 111–116. [Google Scholar] [CrossRef]
- Zimmerman, R.W.; Somerton, W.H.; King, M.S. Compressibility of porous rocks. J. Geophys. Res. 1986, 91, 12765–12777. [Google Scholar] [CrossRef]
- Berryman, J.G. Effective stress for transport properties of inhomogeneous porous rock. J. Geophys. Res. 1992, 97, 17409–17424. [Google Scholar] [CrossRef]
- Gurevich, B. A simple derivation of the effective-stress coefficient for seismic velocities in porous rocks. Geophysics 2004, 69, 393–397. [Google Scholar] [CrossRef]
- Glubokovskikh, S.; Gurevich, B. Effect of micro-inhomogeneity on the effective-stress coefficients and undrained bulk modulus of a poroelastic medium: A double spherical shell model. Geophys. Prospect. 2014, 63, 656–668. [Google Scholar] [CrossRef]
- Todd, T.; Simmons, G. Effect of pore pressure on the velocity of compression waves in low-porosity rocks. J. Geophys. Res. 1972, 77, 3731–3743. [Google Scholar] [CrossRef]
- Christensen, N.I.; Wang, H.F. The Influence of pore pressure and confining pressure on dynamic elastic properties of Berea sandstone. Geophysics 1985, 50, 207–213. [Google Scholar] [CrossRef]
- Prasad, M.; Manghnani, M. Effects of pore and differential pressure on compression wave velocity and quality factor in Berea and Michigan sandstones. Geophysics 1997, 62, 1163–1176. [Google Scholar] [CrossRef]
- Vasquez, G.F.; Vargas, E.A., Jr.; Ribeiro, C.J.B.; Leao, M.; Justen, J.C.R. Experimental determination of the effective pressure coefficients for Brazilian limestones and sandstones. Rev. Bras. Geof. 2009, 27, 43–53. [Google Scholar] [CrossRef][Green Version]
- Tan, W.; Müller, T.M.; Ba, J.; Mikhaltsevitch, V.; Cao, C. Drained-to-undrained transition of bulk modulus in fluid-saturated porous rock induced by dead volume variation. Geophys. Prospect. 2020, 68, 2494–2503. [Google Scholar] [CrossRef]
- Robin, P.-Y.F. Note on effective pressure. J. Geophys. Res. 1973, 78, 2434–2437. [Google Scholar] [CrossRef]
- Carroll, M.M.; Katsube, N. The role of Terzaghi effective stress in linearly elastic deformation. J. Energy Resour. Technol. 1983, 105, 509–511. [Google Scholar] [CrossRef]
- Zimmerman, R.W. Compressibility of Sandstones; Elsevier: Amsterdam, The Netherlands, 1991. [Google Scholar]
- Ciz, R.; Siggins, A.F.; Gurevich, B.; Dvorkin, J. Influence of microheterogeneity on effective stress law for elastic properties of rocks. Geophysics 2008, 73, E7–E14. [Google Scholar] [CrossRef][Green Version]
- Pride, S.R. Relationships between seismic and hydrological properties. In Hydrogeophysics; Rubin, Y., Hubbard, S., Eds.; Springer: Dordrecht, The Netherlands, 2005; pp. 253–291. [Google Scholar] [CrossRef]
- Gassmann, F. Über die elastizität poröser medien. Vierteljahrsschr. Naturforsch. Ges. Zür. 1951, 96, 1–23. [Google Scholar]
- Sevostianov, I. Gassmann equation and replacement relations in micromechanics: A review. Int. J. Eng. Sci. 2020, 154, 103344. [Google Scholar] [CrossRef]
- Njiekak, G.; Schmitt, D.R. Effective-stress coefficient for seismic velocities in carbonate rocks: Effects of pore characteristics and fluid types. Pure Appl. Geophys. 2019, 176, 1467–1485. [Google Scholar] [CrossRef]
- Mikhaltsevitch, V.; Lebedev, M.; Chavez, R.; Vargas, E.A., Jr.; Vasquez, G.F. A laboratory forced-oscillation apparatus for measurements of elastic and anelastic properties of rocks at seismic frequencies. Front. Earth Sci. 2021, 9, 654205. [Google Scholar] [CrossRef]
- Gordon, R.B.; Davis, L.A. Velocity and attenuation of seismic waves in imperfectly elastic rock. J. Geophys. Res. 1968, 73, 3917–3935. [Google Scholar] [CrossRef]
- Mavko, G. Frictional attenuation: An inherent amplitude dependence. J. Geophys. Res. Solid Earth 1979, 84, 4769–4776. [Google Scholar] [CrossRef]
- Winkler, K.; Nur, A.; Gladwin, M. Friction and seismic attenuation in rocks. Nature 1979, 277, 528–531. [Google Scholar] [CrossRef]
- Nourifard, N.; Lebedev, M. Research note: The effect of strain amplitude produced by ultrasonic waves on its velocity. Geophys. Prospect. 2019, 67, 715–722. [Google Scholar] [CrossRef]
- Jong, J.T. Sedimentary History, Diagenesis and Organic Facies of the Triassic Mungaroo Formation, Barrow Sub-Basin, WA. Master’s Thesis, University of Adelaide, Roseworthy, Australia, 1996. Available online: https://hdl.handle.net/2440/98557 (accessed on 6 January 2024).
- Murphy, W.F.; Winkler, K.W.; Kleinberg, R.L. Frame modulus reduction in sedimentary rocks: The effect of adsorption on grain contacts. Geophys. Res. Lett. 1984, 11, 805–808. [Google Scholar] [CrossRef]
- Mavko, G.; Mukerji, T.; Dvorkin, J. The Rock Physics Handbook; Cambridge University Press: Cambridge, UK, 2020. [Google Scholar] [CrossRef]
- Angel, R.J.; Hazen, R.M.; McCormick, T.C.; Prewitt, C.T.; Smyth, J.R. Comparative compressibility of end-member feldspars. Phys. Chem. Miner. 1988, 15, 313–318. [Google Scholar] [CrossRef]
- Fournier, F.; Leonide, P.; Biscarrat, K.; Gallois, A.; Borgomano, J.; Foubert, A. Elastic properties of microporous cemented grainstones. Geophysics 2011, 76, E211–E226. [Google Scholar] [CrossRef]
- Dvorkin, J.; Walls, J.; Davalos, G. Velocity-porosity-mineralogy model for unconventional shale and its applications to digital rock physics. Front. Earth Sci. 2021, 8, 613716. [Google Scholar] [CrossRef]
- Vanorio, T.; Prasad, M.; Nur, A. Elastic properties of dry clay mineral aggregates, suspensions and sandstones. Geophys. J. Int. 2003, 155, 319–326. [Google Scholar] [CrossRef]
- Prak, D.J.L.; Lee, B.G.; Cowart, J.S.; Trulove, P.C. Density, viscosity, speed of sound, bulk modulus, surface tension, and flash point of binary mixtures of butylbenzene + linear alkanes (n-decane, n-dodecane, n-tetradecane, n-hexadecane, or n-heptadecane) at 0.1 MPa. J. Chem. Eng. Data 2017, 62, 169–187. [Google Scholar] [CrossRef]
- Adam, L.; Batzle, M.; Brevik, I. Gassmann’s fluid substitution and shear modulus variability in carbonates at laboratory seismic and ultrasonic frequencies. Geophysics 2006, 71, F173–F183. [Google Scholar] [CrossRef]
- Adam, L.; Batzle, M.; Lewallen, K.T.; van Wijk, K. Seismic wave attenuation in carbonates. J. Geophys. Res. Solid Earth 2009, 114, B06208. [Google Scholar] [CrossRef]
- Mikhaltsevitch, V.; Lebedev, M.; Chavez, R.; Pervukhina, M.; Glubokovskikh, S.; Vargas, E.A., Jr. The dead volume effect on the elastic moduli measurements using the forced-oscillation method. Geophys. Prospect. 2022, 70, 547–557. [Google Scholar] [CrossRef]
- Pimienta, L.; Borgomano, J.V.M.; Fortin, J.; Gueguen, Y. Modelling the drained/undrained transition: Effect of the measuring method and the boundary conditions. Geophys. Prospect. 2016, 64, 1098–1111. [Google Scholar] [CrossRef]







Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mikhaltsevitch, V.; Lebedev, M. Measurements of the Effective Stress Coefficient for Elastic Moduli of Sandstone in Quasi-Static Regime Using Semiconductor Strain Gauges. Sensors 2024, 24, 1122. https://doi.org/10.3390/s24041122
Mikhaltsevitch V, Lebedev M. Measurements of the Effective Stress Coefficient for Elastic Moduli of Sandstone in Quasi-Static Regime Using Semiconductor Strain Gauges. Sensors. 2024; 24(4):1122. https://doi.org/10.3390/s24041122
Chicago/Turabian StyleMikhaltsevitch, Vassily, and Maxim Lebedev. 2024. "Measurements of the Effective Stress Coefficient for Elastic Moduli of Sandstone in Quasi-Static Regime Using Semiconductor Strain Gauges" Sensors 24, no. 4: 1122. https://doi.org/10.3390/s24041122
APA StyleMikhaltsevitch, V., & Lebedev, M. (2024). Measurements of the Effective Stress Coefficient for Elastic Moduli of Sandstone in Quasi-Static Regime Using Semiconductor Strain Gauges. Sensors, 24(4), 1122. https://doi.org/10.3390/s24041122





