An Improved Theory for Designing and Numerically Calibrating Circular Touch Mode Capacitive Pressure Sensors
Abstract
1. Introduction
2. More Refined Analytical Solution to the Sensitive Element of the Sensor
3. Pressure–Capacitance Relationship Derivation of the Sensing Element of the Sensor
4. Results and Discussion
4.1. Design and Numerical Calibration Based on Analytical Solutions
4.2. The Effect of Changing Initially Parallel Gap g on C–q Relationships
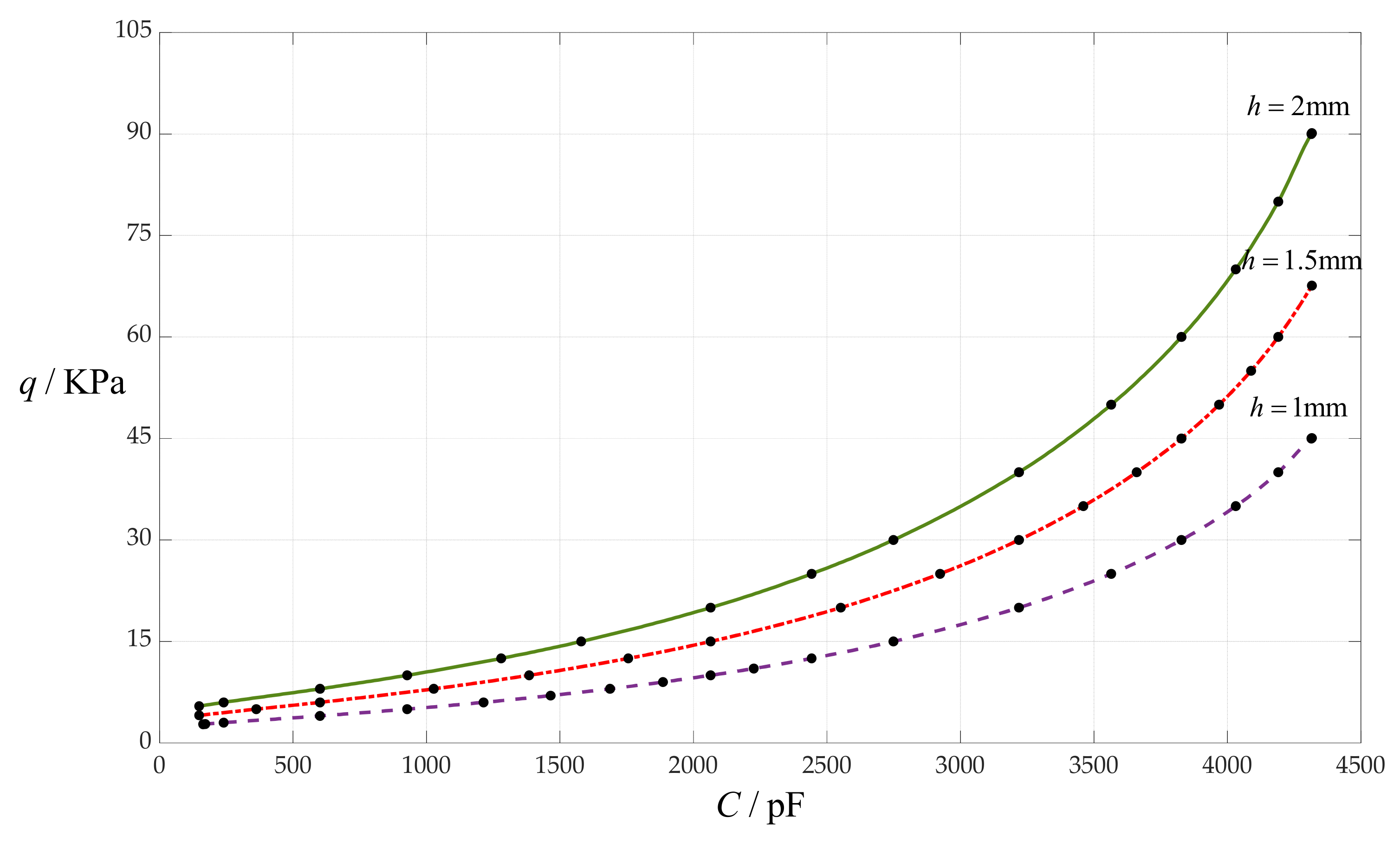
4.3. The Effect of Changing Circular Membrane Thickness h on C–q Relationships
4.4. The Effect of Changing Young’s Modulus of Elasticity E on C–q Relationships
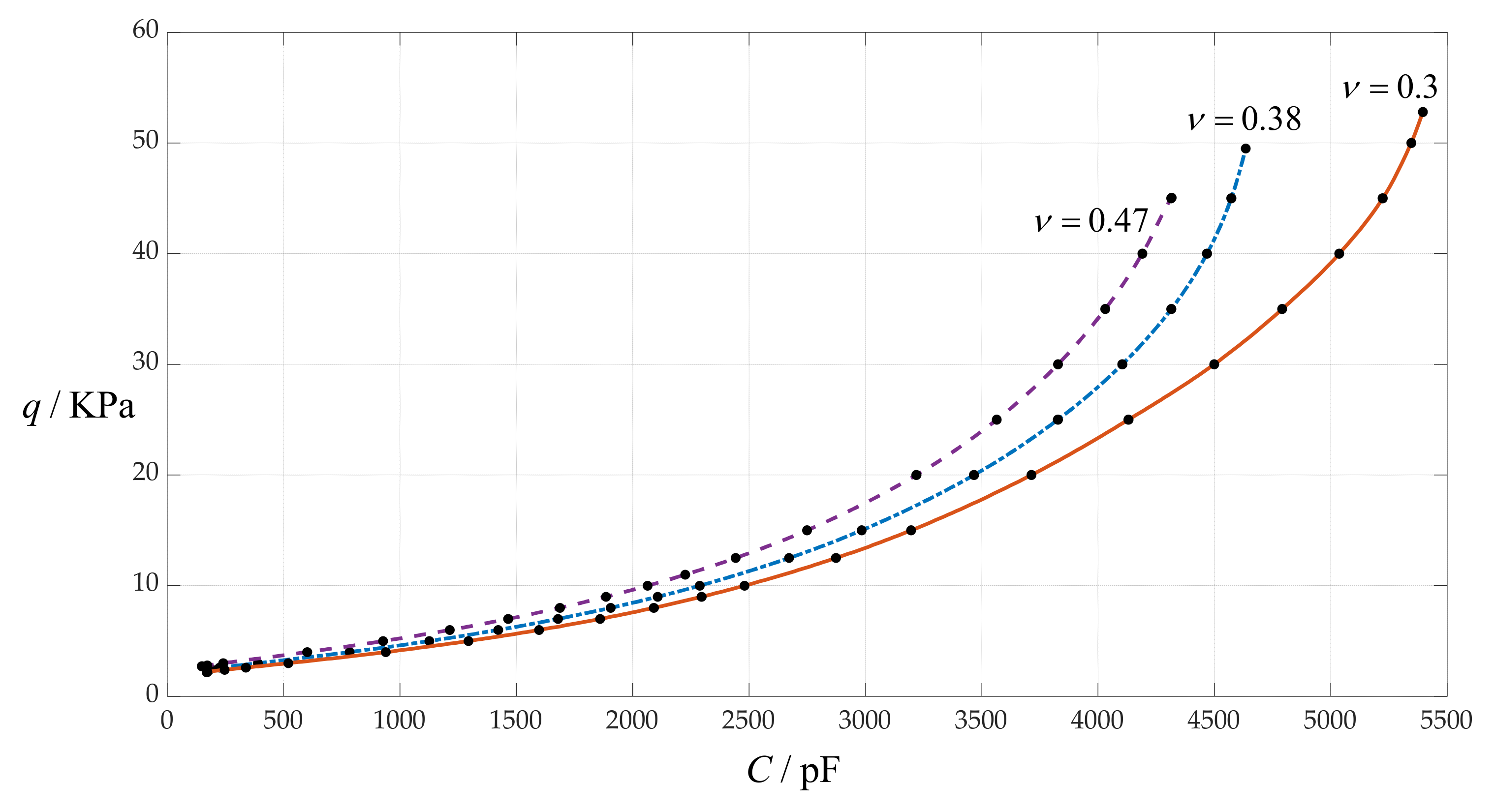
4.5. The Effect of Changing Poisson’s Ratio v on C–q Relationships
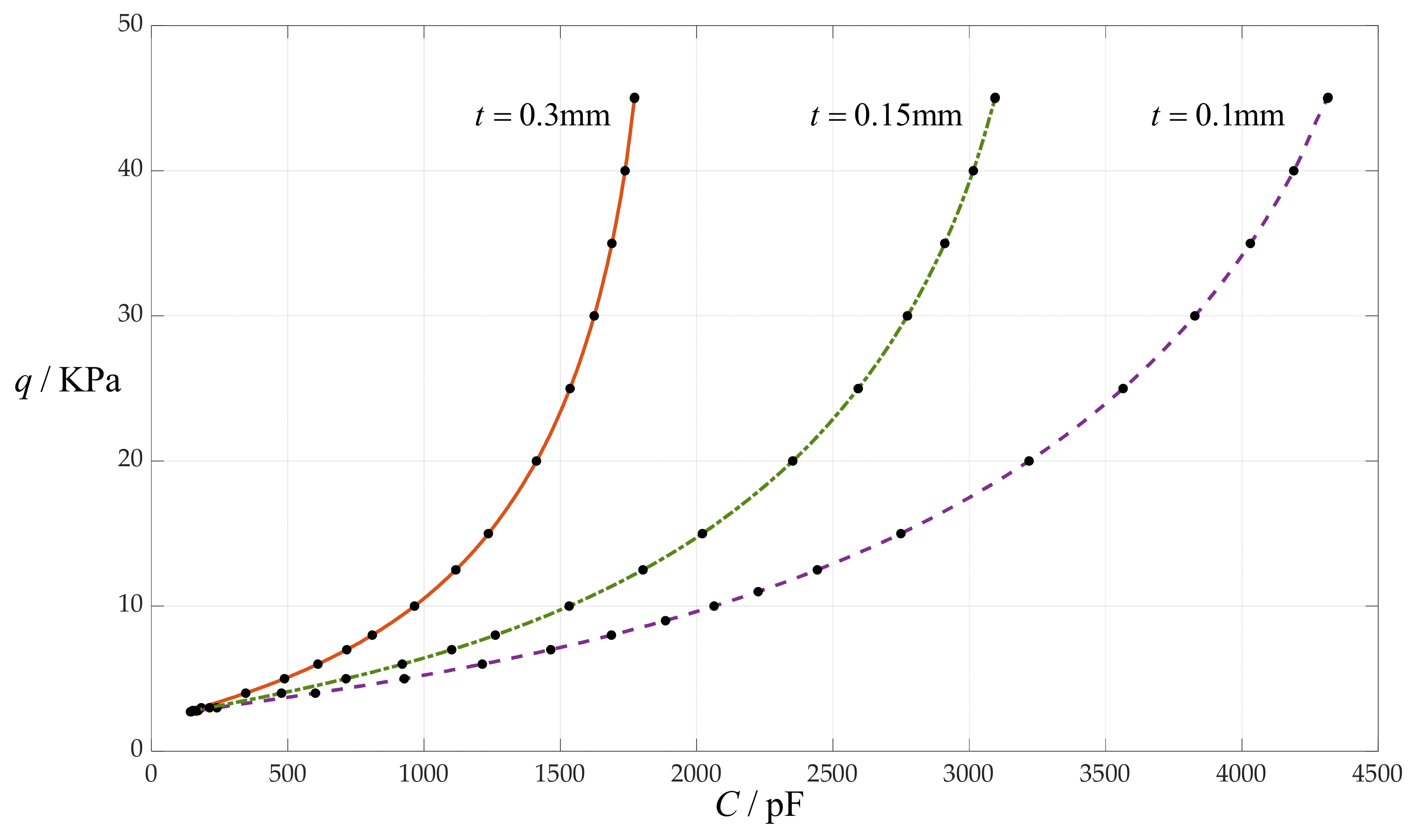
4.6. The Effect of Changing Insulator Layer Thickness t on C–q Relationships
4.7. The Effect of Changing Circular Membrane Radius a on C–q Relationships
4.8. The Effect of Changing Number of Parallel Capacitors n on C–q Relationships
5. Concluding Remarks
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
References
- Bernardo, P.; Iulianelli, A.; Macedonio, F.; Drioli, E. Membrane technologies for space engineering. J. Membr. Sci. 2021, 626, 119177. [Google Scholar] [CrossRef]
- Suresh, K.; Katara, N. Design and development of circular ceramic membrane for wastewater treatment. Mater. Today Proc. 2021, 43, 2176–2181. [Google Scholar] [CrossRef]
- Tai, Y.; Zhou, K.; Chen, N. Dynamic Properties of Microresonators with the Bionic Structure of Tympanic Membrane. Sensors 2020, 20, 6958. [Google Scholar] [CrossRef]
- Li, X.; Sun, J.Y.; Zhao, Z.H.; Li, S.Z.; He, X.T. A new solution to well-known Hencky problem: Improvement of in-plane equilibrium equation. Mathematics 2020, 8, 653. [Google Scholar] [CrossRef]
- Plaut, R.H. Linearly elastic annular and circular membranes under radial, transverse, and torsional loading. Part I: Large unwrinkled axisymmetric deformations. Acta Mech. 2009, 202, 79–99. [Google Scholar] [CrossRef]
- Lim, T.C. Large deflection of circular auxetic membranes under uniform load. J. Mater. Technol. 2016, 138, 041011. [Google Scholar] [CrossRef]
- Yang, X.Y.; Yu, L.X.; Long, R. Contact mechanics of inflated circular membrane under large deformation: Analytical solutions. Int. J. Solids Struct. 2021, 233, 111222. [Google Scholar] [CrossRef]
- Ma, Y.; Wang, G.R.; Chen, Y.L.; Long, D.; Guan, Y.C.; Liu, L.Q.; Zhang, Z. Extended Hencky solution for the blister test of nanomembrane. Extreme Mech. Lett. 2018, 22, 69–78. [Google Scholar] [CrossRef]
- Seide, P. Large deflections of rectangular membranes under uniform pressure. Int. J. Nonlin. Mech. 1977, 12, 397–407. [Google Scholar] [CrossRef]
- Nguyen, T.N.; Hien, T.D.; Nguyen-Thoi, T.; Lee, J. A unified adaptive approach for membrane structures: Form finding and large deflection isogeometric analysis. Comput. Methods Appl. Mech. Eng. 2020, 369, 113239. [Google Scholar] [CrossRef]
- Zhang, Q.; Li, F.Y.; Li, X.; He, X.T.; Sun, J.Y. Polymer conductive membrane-based circular capacitive pressure sensors from non-touch mode of operation to touch mode of operation: An analytical solution-based method for design and numerical calibration. Polymers 2022, 14, 3850. [Google Scholar] [CrossRef]
- Wang, J.; Lou, Y.; Wang, B.; Sun, Q.; Zhou, M.; Li, X. Highly sensitive, breathable, and flexible pressure sensor based on electrospun membrane with assistance of AgNW/TPU as composite dielectric layer. Sensors 2020, 20, 2459. [Google Scholar] [CrossRef]
- Liu, T.; Wang, X.H.; Qiu, X.M.; Zhang, X.H. Theoretical study on the parameter sensitivity over the mechanical states of inflatable membrane antenna. Aerosp. Sci. Technol. 2020, 102, 105843. [Google Scholar] [CrossRef]
- Yashaswini, P.R.; Mamatha, N.; Srikanth, P.C. Circular diaphragm-based MOEMS pressure sensor using ring resonator. Int. J. Inf. Technol. 2020, 13, 213–220. [Google Scholar] [CrossRef]
- Jindal, S.K.; Varma, M.A.; Thukral, D. Comprehensive assessment of MEMS double touch mode capacitive pressure sensor on utilization of SiC film as primary sensing element: Mathematical modelling and numerical simulation. Microelectron. J. 2018, 73, 30–36. [Google Scholar] [CrossRef]
- Gabbi, R.; Rasia, L.A.; Müller, D.C.D.M.; Beltrán, J.R.; Silva, J.A.G.D.; Reimbold, M.M.P. Practical Approach Design Piezoresistive Pressure Sensor in Circular Diaphragm. J. Mater. Sci. Eng. B 2019, 9, 85–91. [Google Scholar]
- Shu, J.F.; Yang, R.R.; Chang, Y.Q.; Guo, X.Q.; Yang, X. A flexible metal thin film strain sensor with micro/nano structure for large deformation and high sensitivity strain measurement. J. Alloys Compd. 2021, 879, 160466. [Google Scholar] [CrossRef]
- Zhang, D.Z.; Jiang, C.X.; Tong, J.; Zong, X.Q.; Hu, W. Flexible Strain Sensor Based on Layer-by-Layer Self-Assembled Graphene/Polymer Nanocomposite Membrane and Its Sensing Properties. J. Electron. Mater. 2018, 47, 2263–2270. [Google Scholar] [CrossRef]
- Han, X.D.; Li, G.; Xu, M.H.; Ke, X.; Chen, H.Y.; Feng, Y.J.; Yan, H.P.; Li, D.T. Differential MEMS capacitance diaphragm vacuum gauge with high sensitivity and wide range. Vacuum 2021, 191, 110367. [Google Scholar] [CrossRef]
- Wang, Q.; Ko, W.H. Si-to-Si fusion bonded touch mode capacitive pressure sensors. Mechatronics 1998, 8, 467–484. [Google Scholar] [CrossRef]
- Ko, W.H.; Wang, Q. Touch mode capacitive pressure sensors. Sens. Actuator A-Phys. 1999, 75, 242–251. [Google Scholar] [CrossRef]
- Chien, W.Z.; Wang, Z.Z.; Xu, Y.G.; Chen, S.L. The symmetrical deformation of circular membrane under the action of uniformly distributed loads in its portion. Appl. Math. Mech. 1981, 2, 653–668. [Google Scholar]
- Lian, Y.S.; Sun, J.Y.; Yang, Z.X. Closed-form solution of well-known Hencky problem without small-rotation-angle assumption. ZAMM Z. Angew. Math. Mech. 2016, 96, 1434–1441. [Google Scholar] [CrossRef]
- Lian, Y.S.; Sun, J.Y.; Zhao, Z.H.; He, X.T.; Zheng, Z.L. A revisit of the boundary value problem for Föppl–Hencky membranes: Improvement of geometric equations. Mathematics 2020, 8, 631. [Google Scholar] [CrossRef]
- Sun, J.Y.; Wu, J.; Li, X.; He, X.T. An exact in-plane equilibrium equation for transversely loaded large deflection membranes and its application to the Föppl-Hencky membrane problem. Mathematics 2023, 11, 3329. [Google Scholar] [CrossRef]
- Xu, D.W.; Liechti, K.M. Analytical and experimental study of a circular membrane in Hertzian contact with a rigid substrate. Int. J. Solids Struct. 2010, 47, 969–977. [Google Scholar] [CrossRef]
- Wang, T.F.; He, X.T.; Li, Y.H. Closed-form solution of a peripherally fixed circular membrane under uniformly-distributed transverse loads and deflection restrictions. Math. Probl. Eng. 2018, 2018, 9589010. [Google Scholar] [CrossRef]
- Lian, Y.S.; Sun, J.Y.; Dong, J.; Zheng, Z.L.; Yang, Z.X. Closed-form solution of axisymmetric deformation of prestressed Föppl-Hencky membrane under constrained deflecting. Struct. Eng. Mech. 2019, 69, 693–698. [Google Scholar]
- Li, F.Y.; Li, X.; Zhang, Q.; He, X.T.; Sun, J.Y. A refined closed-form solution for laterally loaded circular membranes in frictionless contact with rigid flat plates: Simultaneous improvement of out-of-plane equilibrium equation and geometric equation. Mathematics 2022, 10, 3025. [Google Scholar] [CrossRef]
- Föppl, A. Vorlesungen über technische Mechanik: Bd. Die wichtigsten Lehren der höheren Elastizitätstheorie. BG Teubnerv. 1907, 5, 132–144. [Google Scholar]
- Hencky, H. On the stress state in circular plates with vanishing bending stiffness. Z. Angew. Math. Phys. 1915, 63, 311–317. [Google Scholar]
- Long, R.; Hui, C.Y. Axisymmetric membrane in adhesive contact with rigid substrates: Analytical solutions under large deformation. Int. J. Solids Struct. 2012, 49, 672–683. [Google Scholar] [CrossRef]
- Plaut, R.H. Effect of pressure on pull-off of flat cylindrical punch adhered to circular membrane. J. Adhes. 2022, 98, 1438–1460. [Google Scholar] [CrossRef]
- Plaut, R.H. Effect of pressure on pull-off of flat 1-D rectangular punch adhered to membrane. J. Adhes. 2022, 98, 1480–1500. [Google Scholar] [CrossRef]
- Napolitanno, M.J.; Chudnovsky, A.; Moet, A. The constrained blister test for the energy of interfacial adhesion. J. Adhes. Sci. Technol. 1988, 2, 311–323. [Google Scholar] [CrossRef]
- Chang, Y.S.; Lai, Y.H.; Dillard, D.A. The constrained blister—A nearly constant strain-energy release rate test for adhesives. J. Adhes. 1989, 27, 197–211. [Google Scholar] [CrossRef]
- Lai, Y.H.; Dillard, D.A. A study of the fracture efficiency parameter of blister tests for films and coatings. J. Adhes. Sci. Technol. 1994, 8, 663–678. [Google Scholar] [CrossRef]
- Plaut, R.H.; White, S.A.; Dillard, D.A. Effect of work of adhesion on contact of a pressurized blister with a flat surface. Int. J. Adhes. Adhes. 2003, 23, 207–214. [Google Scholar] [CrossRef]
- Zhu, T.T.; Li, G.X.; Müftü, S. Revisiting the constrained blister test to measure thin film adhesion. J. Appl. Mech. T ASME 2017, 84, 071005. [Google Scholar] [CrossRef]
- Zhu, T.T.; Müftü, S.; Wan, K.T. One-dimensional constrained blister test to measure thin film adhesion. J. Appl. Mech. T ASME 2018, 85, 054501. [Google Scholar] [CrossRef]
- Molla-Alipour, M.; Ganji, B.A. Analytical analysis of mems capacitive pressure sensor with circular diaphragm under dynamic load using differential transformation method (DTM). Acta Mech. Solida Sin. 2015, 28, 400–408. [Google Scholar] [CrossRef]
- Lee, H.Y.; Choi, B. Theoretical and experimental investigation of the trapped air effect on air-sealed capacitive pressure sensor. Sens. Actuator A 2015, 221, 104–114. [Google Scholar] [CrossRef]
- Mishra, R.B.; Khan, S.M.; Shaikh, S.F. Low-cost foil/paper based touch mode pressure sensing element as artificial skin module for prosthetic hand. In Proceedings of the 2020 3rd IEEE International Conference on Soft Robotics (RoboSoft), New Haven, CT, USA, 15 May–15 July 2020; pp. 194–200. [Google Scholar]
- Meng, G.Q.; Ko, W.H. Modeling of circular diaphragm and spreadsheet solution programming for touch mode capacitive sensors. Sens. Actuator A 1999, 75, 45–52. [Google Scholar] [CrossRef]
- Daigle, M.; Corcos, J.; Wu, K. An analytical solution to circular touch mode capacitor. IEEE Sens. J. 2007, 7, 502–505. [Google Scholar] [CrossRef]
- Han, J.Y.; Shannon, M.A. Smooth contact capacitive pressure sensors in touch- and peeling-mode operation. IEEE Sens. J. 2009, 9, 199–206. [Google Scholar] [CrossRef]
- Fragiacomo, G.; Ansbæk, T.; Pedersen, T.; Hansen, O.; Thomsen, E.V. Analysis of small deflection touch mode behavior in capacitive pressure sensors. Sens. Actuator A 2010, 161, 114–119. [Google Scholar] [CrossRef]
- Kang, M.C.; Rim, C.S.; Pak, Y.T.; KimInstitute, W.M. A simple analysis to improve linearity of touch mode capacitive pressure sensor by modifying shape of fixed electrode. Sens. Actuator A 2017, 263, 300–304. [Google Scholar] [CrossRef]
- Berger, C.; Phillips, R.; Pasternak, I.; Sobieski, J.; Strupinski, W.; Vijayaraghavan, A. Touch-mode capacitive pressure sensor with graphene-polymer heterostructure membrane. 2D Mater. 2018, 5, 015025. [Google Scholar] [CrossRef]
- Kumar, G.A.A.U.; Jindal, S.K.; Sreekanth, P.K. Capacitance response of concave well substrate MEMS double touch mode capacitive pressure sensor: Robust design, theoretical modeling, numerical simulation and performance comparison. Silicon 2022, 14, 9659–9667. [Google Scholar] [CrossRef]
- Meng, G.Q.; Ko, W.H. A sensitivity enhanced touch mode capacitive pressure sensor with double cavities. Microsyst. Technol. 2023, 29, 755–762. [Google Scholar]











| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C1/pF | C2/pF | C/pF |
|---|---|---|---|---|---|---|---|---|
| 2.718 | 0.000 | - | - | - | 0.332 | 0.000 | 140.669 | 140.669 |
| 2.720 | 0.820 | 0.04591 | 0.04591 | 0.15227 | 0.338 | 0.505 | 148.023 | 148.528 |
| 2.800 | 5.035 | 0.04658 | 0.04122 | 0.14939 | 0.344 | 19.038 | 152.636 | 171.674 |
| 3.000 | 9.723 | 0.04820 | 0.04237 | 0.14748 | 0.360 | 71.006 | 169.488 | 240.494 |
| 4.000 | 21.580 | 0.05517 | 0.04810 | 0.14555 | 0.426 | 349.756 | 251.497 | 601.253 |
| 5.000 | 28.450 | 0.06093 | 0.05306 | 0.14529 | 0.484 | 607.885 | 319.743 | 927.629 |
| 6.000 | 33.339 | 0.06596 | 0.05742 | 0.14528 | 0.535 | 834.737 | 379.005 | 1213.742 |
| 7.000 | 37.095 | 0.07049 | 0.06136 | 0.14533 | 0.582 | 1033.427 | 431.535 | 1464.962 |
| 8.000 | 40.115 | 0.07465 | 0.06496 | 0.14539 | 0.626 | 1208.567 | 478.694 | 1687.260 |
| 9.000 | 42.621 | 0.07852 | 0.06830 | 0.14545 | 0.667 | 1364.256 | 521.413 | 1885.668 |
| 10.000 | 44.748 | 0.08215 | 0.07142 | 0.14552 | 0.707 | 1503.819 | 560.370 | 2064.189 |
| 11.000 | 46.586 | 0.08560 | 0.07437 | 0.14557 | 0.745 | 1629.896 | 596.086 | 2225.982 |
| 12.500 | 48.932 | 0.09047 | 0.07852 | 0.14564 | 0.799 | 1798.190 | 644.450 | 2442.639 |
| 15.000 | 52.060 | 0.09796 | 0.08486 | 0.14574 | 0.884 | 2035.489 | 713.531 | 2749.021 |
| 20.000 | 56.533 | 0.11131 | 0.09600 | 0.14586 | 1.040 | 2400.217 | 818.943 | 3219.159 |
| 25.000 | 59.654 | 0.12317 | 0.10579 | 0.14594 | 1.182 | 2672.563 | 892.028 | 3564.591 |
| 30.000 | 62.002 | 0.13403 | 0.114660 | 0.14598 | 1.315 | 2887.090 | 940.485 | 3827.574 |
| 35.000 | 63.856 | 0.14416 | 0.12287 | 0.14601 | 1.441 | 3062.374 | 968.835 | 4031.210 |
| 40.000 | 65.372 | 0.15372 | 0.13056 | 0.14602 | 1.562 | 3209.466 | 980.887 | 4190.353 |
| 45.000 | 66.642 | 0.16283 | 0.13787 | 0.14603 | 1.679 | 3335.431 | 978.746 | 4314.177 |
| 45.060 | 66.6562 | 0.16294 | 0.13795 | 0.14603 | 1.680 | 3336.835 | 979.311 | 4316.146 |
| Functions | C/pF | q/KPa | Analytical Expressions | Average Fitting Error Squares |
|---|---|---|---|---|
| Function 1 | 140.669~4316.146 | 2.718~45.06 | q = 5.727382 × 10−20C6 − 6.342403 × 10−16C5 + 2.743908 × 10−12C4 − 5.418370 × 10−9C3 + 5.581685 × 10−6 C2 + 3.712548 × 10−4C + 2.592449 | 0.0028814 |
| Function 2 | 140.669~2225.982 | 2.718~11 | q = 3.766098 × 10−3C + 1.928101 | 0.1255083 |
| Function 3 | 140.669~4316.146 | 2.718~45.06 | q = 9.115659 × 10−3C − 3.585634 | 25.5704600 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF |
|---|---|---|---|---|---|---|
| 0.3512 | 0.000 | 0.01164 | 0.01058 | 0.07784 | 0.076 | 313.512 |
| 0.3514 | 0.895 | 0.01179 | 0.01064 | 0.07639 | 0.080 | 324.196 |
| 0.3530 | 2.093 | 0.01181 | 0.01063 | 0.07591 | 0.081 | 340.354 |
| 0.4000 | 11.378 | 0.01252 | 0.01113 | 0.07383 | 0.087 | 464.029 |
| 0.5000 | 20.431 | 0.01384 | 0.01227 | 0.07316 | 0.098 | 808.403 |
| 1.0000 | 39.389 | 0.01847 | 0.01648 | 0.07311 | 0.138 | 2099.409 |
| 2.5000 | 56.215 | 0.02655 | 0.02385 | 0.07349 | 0.211 | 3810.873 |
| 5.0000 | 65.407 | 0.03497 | 0.03139 | 0.07371 | 0.293 | 4655.640 |
| 7.5000 | 69.776 | 0.04121 | 0.03689 | 0.07381 | 0.356 | 4917.764 |
| 10.0000 | 72.503 | 0.04642 | 0.04143 | 0.07387 | 0.412 | 5050.278 |
| 20.0000 | 78.000 | 0.06256 | 0.05528 | 0.07396 | 0.593 | 5327.927 |
| 40.0000 | 82.233 | 0.08647 | 0.07544 | 0.07399 | 0.880 | 5599.954 |
| 60.0000 | 84.221 | 0.10623 | 0.09201 | 0.07399 | 1.128 | 5751.129 |
| 80.0000 | 85.440 | 0.12406 | 0.10705 | 0.07397 | 1.356 | 5851.602 |
| 100.0000 | 86.285 | 0.14074 | 0.12126 | 0.07395 | 1.572 | 5924.701 |
| 110.0000 | 86.621 | 0.14877 | 0.12816 | 0.07394 | 1.677 | 5954.636 |
| 110.2000 | 86.627 | 0.14893 | 0.12830 | 0.07394 | 1.680 | 5955.198 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF |
|---|---|---|---|---|---|---|
| 8.702 | 0.000 | 0.09818 | 0.08636 | 0.23015 | 0.796 | 116.259 |
| 8.710 | 1.202 | 0.09905 | 0.08662 | 0.22651 | 0.805 | 130.806 |
| 9.100 | 6.357 | 0.10156 | 0.08779 | 0.22163 | 0.830 | 147.293 |
| 9.500 | 9.180 | 0.10393 | 0.08938 | 0.21996 | 0.854 | 190.312 |
| 10.000 | 11.898 | 0.10674 | 0.09142 | 0.21878 | 0.884 | 246.070 |
| 12.500 | 20.863 | 0.11919 | 0.10109 | 0.21672 | 1.020 | 526.564 |
| 15.000 | 26.686 | 0.12996 | 0.10973 | 0.21622 | 1.167 | 791.823 |
| 17.500 | 31.033 | 0.13965 | 0.11755 | 0.21606 | 1.256 | 1037.767 |
| 20.000 | 34.479 | 0.14856 | 0.12473 | 0.21602 | 1.363 | 1266.945 |
| 22.500 | 37.311 | 0.15689 | 0.13142 | 0.21602 | 1.464 | 1483.482 |
| 25.000 | 39.701 | 0.16476 | 0.13770 | 0.21603 | 1.561 | 1692.108 |
| 26.000 | 40.558 | 0.16779 | 0.14011 | 0.21604 | 1.599 | 1774.515 |
| 27.000 | 41.368 | 0.17077 | 0.14248 | 0.21605 | 1.636 | 1856.934 |
| 27.500 | 41.756 | 0.17224 | 0.14365 | 0.21365 | 1.650 | 1898.278 |
| 28.000 | 42.134 | 0.17370 | 0.14480 | 0.21606 | 1.673 | 1939.797 |
| 28.100 | 42.208 | 0.17399 | 0.14503 | 0.21606 | 1.677 | 1948.130 |
| 28.150 | 42.245 | 0.17413 | 0.14514 | 0.21606 | 1.679 | 1952.296 |
| 28.170 | 42.260 | 0.17419 | 0.14519 | 0.21606 | 1.680 | 1953.975 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF |
|---|---|---|---|---|---|---|
| 4.08 | 0.820 | 0.04591 | 0.04591 | 0.15227 | 0.338 | 148.528 |
| 5.00 | 14.759 | 0.05071 | 0.04436 | 0.14631 | 0.383 | 362.457 |
| 6.00 | 21.580 | 0.05517 | 0.04810 | 0.14555 | 0.426 | 601.253 |
| 8.00 | 30.243 | 0.06267 | 0.05457 | 0.14527 | 0.501 | 1027.261 |
| 10.00 | 35.939 | 0.06903 | 0.06009 | 0.14531 | 0.566 | 1384.715 |
| 12.50 | 41.000 | 0.07597 | 0.06610 | 0.14542 | 0.640 | 1755.832 |
| 15.00 | 44.748 | 0.08215 | 0.07142 | 0.14552 | 0.707 | 2064.189 |
| 20.00 | 50.067 | 0.09305 | 0.08071 | 0.14568 | 0.828 | 2551.568 |
| 25.00 | 53.761 | 0.10262 | 0.08877 | 0.14579 | 0.938 | 2923.610 |
| 30.00 | 56.533 | 0.11131 | 0.09600 | 0.14586 | 1.040 | 3219.159 |
| 35.00 | 58.719 | 0.11935 | 0.10265 | 0.14591 | 1.136 | 3460.166 |
| 40.00 | 60.505 | 0.12689 | 0.10883 | 0.14595 | 1.227 | 3659.999 |
| 45.00 | 62.002 | 0.13403 | 0.11466 | 0.14598 | 1.315 | 3827.574 |
| 50.00 | 63.282 | 0.14085 | 0.12019 | 0.14600 | 1.400 | 3968.910 |
| 55.00 | 64.393 | 0.14740 | 0.12548 | 0.14601 | 1.482 | 4088.528 |
| 60.00 | 65.372 | 0.15372 | 0.13056 | 0.14602 | 1.562 | 4189.975 |
| 67.59 | 66.656 | 0.16294 | 0.13795 | 0.14603 | 1.680 | 4316.146 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF |
|---|---|---|---|---|---|---|
| 5.44 | 0.820 | 0.04591 | 0.04591 | 0.15227 | 0.338 | 148.528 |
| 6.00 | 9.723 | 0.04820 | 0.04237 | 0.14748 | 0.360 | 240.494 |
| 8.00 | 21.580 | 0.05517 | 0.04810 | 0.14555 | 0.426 | 601.253 |
| 10.00 | 28.450 | 0.06093 | 0.05306 | 0.14529 | 0.484 | 927.629 |
| 12.50 | 34.364 | 0.06713 | 0.05844 | 0.14529 | 0.547 | 1279.574 |
| 15.00 | 38.681 | 0.07261 | 0.06319 | 0.14536 | 0.604 | 1579.385 |
| 20.00 | 44.748 | 0.08215 | 0.07142 | 0.14552 | 0.707 | 2064.189 |
| 25.00 | 48.932 | 0.09047 | 0.07852 | 0.14564 | 0.799 | 2442.639 |
| 30.00 | 52.060 | 0.09796 | 0.08486 | 0.14574 | 0.884 | 2749.021 |
| 40.00 | 56.533 | 0.11131 | 0.09600 | 0.14586 | 1.040 | 3219.159 |
| 50.00 | 59.654 | 0.12317 | 0.10579 | 0.14594 | 1.182 | 3564.591 |
| 60.00 | 62.002 | 0.13403 | 0.11466 | 0.14598 | 1.315 | 3827.574 |
| 70.00 | 63.856 | 0.14416 | 0.12287 | 0.14601 | 1.441 | 4031.210 |
| 80.00 | 65.372 | 0.15372 | 0.13056 | 0.14602 | 1.562 | 4190.353 |
| 90.00 | 66.642 | 0.16283 | 0.13787 | 0.14603 | 1.679 | 4314.177 |
| 90.12 | 66.656 | 0.16294 | 0.13795 | 0.14603 | 1.680 | 4316.146 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF |
|---|---|---|---|---|---|---|
| 1.735 | 0.897 | 0.04591 | 0.04097 | 0.15221 | 0.215 | 155.165 |
| 1.800 | 5.720 | 0.04676 | 0.04134 | 0.14904 | 0.221 | 178.632 |
| 2.000 | 12.010 | 0.04925 | 0.04318 | 0.14686 | 0.236 | 290.047 |
| 2.500 | 20.893 | 0.05467 | 0.04767 | 0.14559 | 0.269 | 573.373 |
| 3.000 | 26.684 | 0.05932 | 0.05166 | 0.14532 | 0.298 | 835.366 |
| 4.000 | 34.451 | 0.06724 | 0.05853 | 0.14529 | 0.349 | 1285.266 |
| 5.000 | 39.671 | 0.07400 | 0.06440 | 0.14538 | 0.395 | 1653.415 |
| 6.000 | 43.528 | 0.08003 | 0.06960 | 0.14548 | 0.436 | 1960.710 |
| 8.000 | 48.994 | 0.09061 | 0.07864 | 0.14564 | 0.510 | 2448.583 |
| 10.000 | 52.785 | 0.09989 | 0.08648 | 0.14576 | 0.578 | 2822.750 |
| 12.500 | 56.236 | 0.11030 | 0.09517 | 0.14585 | 0.655 | 3186.919 |
| 15.000 | 58.828 | 0.11978 | 0.10300 | 0.14592 | 0.728 | 3472.338 |
| 20.000 | 62.547 | 0.13685 | 0.11695 | 0.14599 | 0.861 | 3888.116 |
| 25.000 | 65.148 | 0.15222 | 0.12936 | 0.14602 | 0.984 | 4167.118 |
| 30.000 | 67.105 | 0.16644 | 0.14075 | 0.14603 | 1.100 | 4356.798 |
| 40.000 | 69.913 | 0.19252 | 0.16148 | 0.14601 | 1.317 | 4580.665 |
| 50.000 | 71.873 | 0.21648 | 0.18040 | 0.14598 | 1.520 | 4705.456 |
| 55.000 | 72.657 | 0.22788 | 0.18938 | 0.14596 | 1.617 | 4751.286 |
| 58.000 | 73.081 | 0.23457 | 0.19465 | 0.14594 | 1.675 | 4775.727 |
| 58.200 | 73.108 | 0.23501 | 0.19500 | 0.14594 | 1.680 | 4777.261 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF |
|---|---|---|---|---|---|---|
| 0.868 | 1.744 | 0.04598 | 0.04095 | 0.15113 | 0.108 | 158.298 |
| 0.900 | 5.720 | 0.04676 | 0.04134 | 0.14904 | 0.110 | 178.632 |
| 1.000 | 12.010 | 0.04925 | 0.04318 | 0.14686 | 0.118 | 290.047 |
| 2.000 | 34.451 | 0.06724 | 0.05853 | 0.14529 | 0.175 | 1285.266 |
| 3.000 | 43.528 | 0.08003 | 0.06960 | 0.14548 | 0.436 | 1960.710 |
| 4.000 | 48.994 | 0.09061 | 0.07864 | 0.14564 | 0.255 | 2448.583 |
| 6.000 | 55.628 | 0.10831 | 0.09351 | 0.14584 | 0.320 | 3121.327 |
| 8.000 | 59.701 | 0.12337 | 0.10595 | 0.14594 | 0.378 | 3569.879 |
| 10.000 | 62.547 | 0.13685 | 0.11695 | 0.14599 | 0.431 | 3888.116 |
| 15.000 | 67.105 | 0.16644 | 0.14075 | 0.14603 | 0.550 | 4356.798 |
| 20.000 | 69.913 | 0.19252 | 0.16148 | 0.14601 | 0.659 | 4580.665 |
| 25.000 | 71.873 | 0.21648 | 0.18040 | 0.14598 | 0.760 | 4705.456 |
| 30.000 | 73.347 | 0.23897 | 0.19812 | 0.14593 | 0.856 | 4790.991 |
| 40.000 | 75.457 | 0.28089 | 0.23116 | 0.14583 | 1.039 | 4915.433 |
| 50.000 | 76.932 | 0.31991 | 0.26202 | 0.14573 | 1.211 | 5009.968 |
| 60.000 | 78.045 | 0.35681 | 0.29135 | 0.14562 | 1.375 | 5087.531 |
| 70.000 | 78.930 | 0.39202 | 0.31948 | 0.14553 | 1.533 | 5153.463 |
| 79.000 | 79.591 | 0.42249 | 0.34395 | 0.14544 | 1.671 | 5205.312 |
| 79.500 | 79.625 | 0.42415 | 0.34529 | 0.14544 | 1.680 | 5208.021 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF |
|---|---|---|---|---|---|---|
| 2.392 | 0.379 | 0.04067 | 0.03586 | 0.15323 | 0.292 | 174.459 |
| 2.400 | 1.657 | 0.04073 | 0.03579 | 0.15215 | 0.293 | 180.222 |
| 2.500 | 6.282 | 0.04154 | 0.03614 | 0.14935 | 0.301 | 186.514 |
| 2.600 | 8.915 | 0.04234 | 0.03668 | 0.14831 | 0.308 | 224.696 |
| 3.000 | 15.811 | 0.04530 | 0.03893 | 0.14669 | 0.336 | 389.251 |
| 4.000 | 25.829 | 0.05153 | 0.04407 | 0.14584 | 0.397 | 783.394 |
| 5.000 | 32.123 | 0.05675 | 0.04848 | 0.14573 | 0.450 | 1126.314 |
| 6.000 | 36.673 | 0.06134 | 0.05237 | 0.14575 | 0.497 | 1421.968 |
| 7.000 | 40.190 | 0.06549 | 0.05588 | 0.14581 | 0.541 | 1679.219 |
| 8.000 | 43.027 | 0.06932 | 0.05910 | 0.14587 | 0.582 | 1905.663 |
| 9.000 | 45.385 | 0.07290 | 0.06209 | 0.14593 | 0.621 | 2107.173 |
| 10.000 | 47.390 | 0.07626 | 0.06489 | 0.14598 | 0.658 | 2288.223 |
| 12.500 | 51.338 | 0.08399 | 0.07126 | 0.14609 | 0.745 | 2672.042 |
| 15.000 | 54.293 | 0.09098 | 0.07696 | 0.14616 | 0.825 | 2983.817 |
| 20.000 | 58.520 | 0.10348 | 0.08701 | 0.14626 | 0.973 | 3466.839 |
| 25.000 | 61.470 | 0.11465 | 0.09586 | 0.14632 | 1.108 | 3827.439 |
| 30.000 | 63.689 | 0.12491 | 0.10390 | 0.14635 | 1.235 | 4104.314 |
| 35.000 | 65.441 | 0.13451 | 0.11136 | 0.14636 | 1.356 | 4315.009 |
| 40.000 | 66.872 | 0.14360 | 0.11838 | 0.14637 | 1.472 | 4468.178 |
| 45.000 | 68.071 | 0.15229 | 0.12506 | 0.14637 | 1.583 | 4572.718 |
| 49.500 | 68.999 | 0.15981 | 0.13082 | 0.14636 | 1.680 | 4634.432 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF |
|---|---|---|---|---|---|---|
| 2.173 | 0.792 | 0.03714 | 0.03235 | 0.15333 | 0.262 | 169.126 |
| 2.200 | 3.304 | 0.03735 | 0.03231 | 0.15146 | 0.264 | 172.501 |
| 2.400 | 9.935 | 0.03893 | 0.03323 | 0.14846 | 0.279 | 246.287 |
| 2.600 | 13.912 | 0.04041 | 0.03431 | 0.14748 | 0.293 | 337.604 |
| 3.000 | 19.641 | 0.04311 | 0.03642 | 0.14666 | 0.319 | 520.458 |
| 4.000 | 28.820 | 0.04886 | 0.04111 | 0.14616 | 0.376 | 938.829 |
| 5.000 | 34.754 | 0.05372 | 0.04513 | 0.14611 | 0.425 | 1294.335 |
| 6.000 | 39.075 | 0.05801 | 0.04869 | 0.14615 | 0.470 | 1597.681 |
| 7.000 | 42.427 | 0.06191 | 0.05190 | 0.14620 | 0.512 | 1860.274 |
| 8.000 | 45.135 | 0.06552 | 0.05485 | 0.14626 | 0.551 | 2090.900 |
| 9.000 | 47.389 | 0.06889 | 0.05759 | 0.14631 | 0.588 | 2296.061 |
| 10.000 | 49.306 | 0.07207 | 0.06016 | 0.14635 | 0.623 | 2480.588 |
| 12.500 | 53.084 | 0.07939 | 0.06601 | 0.14644 | 0.706 | 2873.643 |
| 15.000 | 55.914 | 0.08603 | 0.07125 | 0.14651 | 0.783 | 3196.845 |
| 20.000 | 59.962 | 0.09796 | 0.08050 | 0.14659 | 0.925 | 3713.862 |
| 25.000 | 62.787 | 0.10865 | 0.08868 | 0.14663 | 1.056 | 4130.838 |
| 30.000 | 64.912 | 0.11850 | 0.09612 | 0.14665 | 1.178 | 4499.176 |
| 35.000 | 66.588 | 0.12774 | 0.10304 | 0.14666 | 1.295 | 4791.569 |
| 40.000 | 67.956 | 0.13651 | 0.10957 | 0.14666 | 1.407 | 5036.617 |
| 45.000 | 69.103 | 0.14490 | 0.11579 | 0.14666 | 1.515 | 5223.193 |
| 50.000 | 70.082 | 0.15297 | 0.12176 | 0.14665 | 1.621 | 5346.050 |
| 52.800 | 70.572 | 0.15738 | 0.12500 | 0.14664 | 1.680 | 5379.050 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF |
|---|---|---|---|---|---|---|
| 2.72 | 0.820 | 0.04591 | 0.04591 | 0.15227 | 0.338 | 146.585 |
| 2.80 | 5.035 | 0.04658 | 0.04122 | 0.14939 | 0.344 | 162.993 |
| 3.00 | 9.723 | 0.04820 | 0.04237 | 0.14748 | 0.360 | 213.413 |
| 4.00 | 21.580 | 0.05517 | 0.04810 | 0.14555 | 0.426 | 477.139 |
| 5.00 | 28.450 | 0.06093 | 0.05306 | 0.14529 | 0.484 | 713.705 |
| 6.00 | 33.339 | 0.06596 | 0.05742 | 0.14528 | 0.535 | 920.432 |
| 7.00 | 37.095 | 0.07049 | 0.06136 | 0.14533 | 0.582 | 1101.607 |
| 8.00 | 40.115 | 0.07465 | 0.06496 | 0.14539 | 0.626 | 1261.671 |
| 10.00 | 44.748 | 0.08215 | 0.07142 | 0.14552 | 0.707 | 1532.428 |
| 12.50 | 48.932 | 0.09047 | 0.07852 | 0.14564 | 0.799 | 1803.162 |
| 15.00 | 52.060 | 0.09796 | 0.08486 | 0.14574 | 0.884 | 2021.169 |
| 20.00 | 56.533 | 0.11131 | 0.09600 | 0.14586 | 1.040 | 2352.714 |
| 25.00 | 59.654 | 0.12317 | 0.10579 | 0.14594 | 1.182 | 2592.964 |
| 30.00 | 62.002 | 0.13403 | 0.11466 | 0.14598 | 1.315 | 2773.046 |
| 35.00 | 63.856 | 0.14416 | 0.12287 | 0.14601 | 1.441 | 2910.032 |
| 40.00 | 65.372 | 0.15372 | 0.13056 | 0.14602 | 1.562 | 3014.646 |
| 45.00 | 66.642 | 0.16283 | 0.13787 | 0.14603 | 1.679 | 3094.516 |
| 45.06 | 66.656 | 0.16294 | 0.13795 | 0.14603 | 1.680 | 3095.387 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF |
|---|---|---|---|---|---|---|
| 2.72 | 0.820 | 0.04591 | 0.04591 | 0.15227 | 0.338 | 143.284 |
| 2.80 | 5.035 | 0.04658 | 0.04122 | 0.14939 | 0.344 | 151.505 |
| 3.00 | 9.723 | 0.04820 | 0.04237 | 0.14748 | 0.360 | 182.955 |
| 4.00 | 21.580 | 0.05517 | 0.04810 | 0.14555 | 0.426 | 345.800 |
| 5.00 | 28.450 | 0.06093 | 0.05306 | 0.14529 | 0.484 | 488.127 |
| 6.00 | 33.339 | 0.06596 | 0.05742 | 0.14528 | 0.535 | 610.728 |
| 7.00 | 37.095 | 0.07049 | 0.06136 | 0.14533 | 0.582 | 716.981 |
| 8.00 | 40.115 | 0.07465 | 0.06496 | 0.14539 | 0.626 | 809.911 |
| 10.00 | 44.748 | 0.08215 | 0.07142 | 0.14552 | 0.707 | 964.911 |
| 12.50 | 48.932 | 0.09047 | 0.07852 | 0.14564 | 0.799 | 1116.742 |
| 15.00 | 52.060 | 0.09796 | 0.08486 | 0.14574 | 0.884 | 1236.315 |
| 20.00 | 56.533 | 0.11131 | 0.09600 | 0.14586 | 1.040 | 1412.682 |
| 25.00 | 59.654 | 0.12317 | 0.10579 | 0.14594 | 1.182 | 1535.543 |
| 30.00 | 62.002 | 0.13403 | 0.11466 | 0.14598 | 1.315 | 1624.285 |
| 35.00 | 63.856 | 0.14416 | 0.12287 | 0.14601 | 1.441 | 1689.360 |
| 40.00 | 65.372 | 0.15372 | 0.13056 | 0.14602 | 1.562 | 1737.354 |
| 45.00 | 66.642 | 0.16283 | 0.13787 | 0.14603 | 1.679 | 1772.129 |
| 45.06 | 66.656 | 0.16294 | 0.13795 | 0.14603 | 1.680 | 1772.506 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF |
|---|---|---|---|---|---|---|
| 19.46 | 0.556 | 0.12009 | 0.10423 | 0.25143 | 1.010 | 55.810 |
| 20.00 | 2.959 | 0.12217 | 0.10497 | 0.24678 | 1.030 | 66.442 |
| 22.00 | 6.667 | 0.12873 | 0.10930 | 0.24272 | 1.100 | 107.551 |
| 24.00 | 9.077 | 0.13467 | 0.11367 | 0.24125 | 1.166 | 148.419 |
| 26.00 | 10.982 | 0.14022 | 0.11788 | 0.24048 | 1.229 | 189.159 |
| 28.00 | 12.583 | 0.14545 | 0.12192 | 0.24002 | 1.290 | 229.332 |
| 30.00 | 13.969 | 0.15043 | 0.12579 | 0.23973 | 1.348 | 268.756 |
| 32.00 | 15.194 | 0.15519 | 0.12952 | 0.23954 | 1.405 | 307.370 |
| 34.00 | 16.289 | 0.15977 | 0.13311 | 0.23940 | 1.460 | 345.185 |
| 36.00 | 17.280 | 0.16419 | 0.13658 | 0.23931 | 1.514 | 382.246 |
| 38.00 | 18.184 | 0.16847 | 0.13994 | 0.23925 | 1.566 | 418.632 |
| 40.00 | 19.013 | 0.17263 | 0.14319 | 0.23920 | 1.618 | 454.440 |
| 42.49 | 19.957 | 0.17764 | 0.14712 | 0.23917 | 1.680 | 498.372 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF |
|---|---|---|---|---|---|---|
| 6.48 | 0.570 | 0.07034 | 0.06226 | 0.18993 | 0.543 | 90.433 |
| 7.00 | 6.811 | 0.07326 | 0.06385 | 0.18428 | 0.571 | 138.438 |
| 8.00 | 12.066 | 0.07829 | 0.06770 | 0.18222 | 0.622 | 236.738 |
| 9.00 | 15.696 | 0.08281 | 0.07137 | 0.18150 | 0.669 | 334.167 |
| 10.00 | 18.556 | 0.08698 | 0.07481 | 0.18117 | 0.712 | 427.901 |
| 12.50 | 23.862 | 0.09628 | 0.08259 | 0.18090 | 0.813 | 642.502 |
| 15.00 | 27.662 | 0.10447 | 0.08946 | 0.18088 | 0.904 | 830.354 |
| 17.50 | 30.588 | 0.11191 | 0.09568 | 0.18093 | 0.988 | 995.958 |
| 20.00 | 32.944 | 0.11879 | 0.10140 | 0.18098 | 1.068 | 1143.713 |
| 25.00 | 36.556 | 0.13132 | 0.11175 | 0.18109 | 1.216 | 1399.378 |
| 30.00 | 39.245 | 0.14269 | 0.12102 | 0.18117 | 1.354 | 1618.568 |
| 35.00 | 41.354 | 0.15321 | 0.12951 | 0.18122 | 1.484 | 1816.202 |
| 40.00 | 43.070 | 0.16308 | 0.13742 | 0.18127 | 1.608 | 2005.539 |
| 42.50 | 43.818 | 0.16782 | 0.14120 | 0.18128 | 1.668 | 2092.215 |
| 43.02 | 43.965 | 0.16879 | 0.14197 | 0.18128 | 1.680 | 2112.884 |
| q/KPa | b/mm | b0 | c0 | d0 | σm/MPa | C/pF | ||
|---|---|---|---|---|---|---|---|---|
| n = 10 | n = 20 | n = 30 | ||||||
| 2.72 | 0.082 | 0.04591 | 0.04591 | 0.15227 | 0.338 | 120.276 | 240.553 | 360.829 |
| 2.80 | 0.503 | 0.04658 | 0.04122 | 0.14939 | 0.344 | 129.318 | 258.635 | 387.953 |
| 3.00 | 0.972 | 0.04820 | 0.04237 | 0.14748 | 0.360 | 144.877 | 289.755 | 434.632 |
| 4.00 | 2.158 | 0.05517 | 0.04810 | 0.14555 | 0.426 | 220.875 | 441.749 | 662.624 |
| 5.00 | 2.845 | 0.06093 | 0.05306 | 0.14529 | 0.484 | 280.673 | 561.346 | 842.019 |
| 6.00 | 3.334 | 0.06596 | 0.05742 | 0.14528 | 0.535 | 328.477 | 656.955 | 985.432 |
| 7.00 | 3.709 | 0.07049 | 0.06136 | 0.14533 | 0.582 | 367.438 | 734.877 | 1102.315 |
| 8.00 | 4.012 | 0.07465 | 0.06496 | 0.14539 | 0.626 | 399.742 | 799.484 | 1199.226 |
| 10.00 | 4.475 | 0.08215 | 0.07142 | 0.14552 | 0.707 | 450.097 | 900.194 | 1350.292 |
| 12.50 | 4.893 | 0.09047 | 0.07852 | 0.14564 | 0.799 | 495.312 | 990.624 | 1485.936 |
| 15.00 | 5.206 | 0.09796 | 0.08486 | 0.14574 | 0.884 | 528.158 | 1056.316 | 1584.474 |
| 20.00 | 5.653 | 0.11131 | 0.09600 | 0.14586 | 1.040 | 572.318 | 1144.636 | 1716.954 |
| 25.00 | 5.965 | 0.12317 | 0.10579 | 0.14594 | 1.182 | 600.135 | 1200.271 | 1800.406 |
| 30.00 | 6.200 | 0.13403 | 0.11466 | 0.14598 | 1.315 | 618.720 | 1237.440 | 1856.160 |
| 35.00 | 6.386 | 0.14416 | 0.12287 | 0.14601 | 1.441 | 631.493 | 1262.985 | 1894.478 |
| 40.00 | 6.537 | 0.15372 | 0.13056 | 0.14602 | 1.562 | 640.340 | 1280.680 | 1921.020 |
| 45.00 | 6.664 | 0.16283 | 0.13787 | 0.14603 | 1.679 | 646.431 | 1292.861 | 1939.292 |
| 45.06 | 6.666 | 0.16294 | 0.13795 | 0.14603 | 1.680 | 646.495 | 1292.991 | 1939.486 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
He, X.-T.; Wang, X.; Li, F.-Y.; Sun, J.-Y. An Improved Theory for Designing and Numerically Calibrating Circular Touch Mode Capacitive Pressure Sensors. Sensors 2024, 24, 907. https://doi.org/10.3390/s24030907
He X-T, Wang X, Li F-Y, Sun J-Y. An Improved Theory for Designing and Numerically Calibrating Circular Touch Mode Capacitive Pressure Sensors. Sensors. 2024; 24(3):907. https://doi.org/10.3390/s24030907
Chicago/Turabian StyleHe, Xiao-Ting, Xin Wang, Fei-Yan Li, and Jun-Yi Sun. 2024. "An Improved Theory for Designing and Numerically Calibrating Circular Touch Mode Capacitive Pressure Sensors" Sensors 24, no. 3: 907. https://doi.org/10.3390/s24030907
APA StyleHe, X.-T., Wang, X., Li, F.-Y., & Sun, J.-Y. (2024). An Improved Theory for Designing and Numerically Calibrating Circular Touch Mode Capacitive Pressure Sensors. Sensors, 24(3), 907. https://doi.org/10.3390/s24030907






