Speed of Light in Hollow-Core Photonic Bandgap Fiber Approaching That in Vacuum
Abstract
:1. Introduction
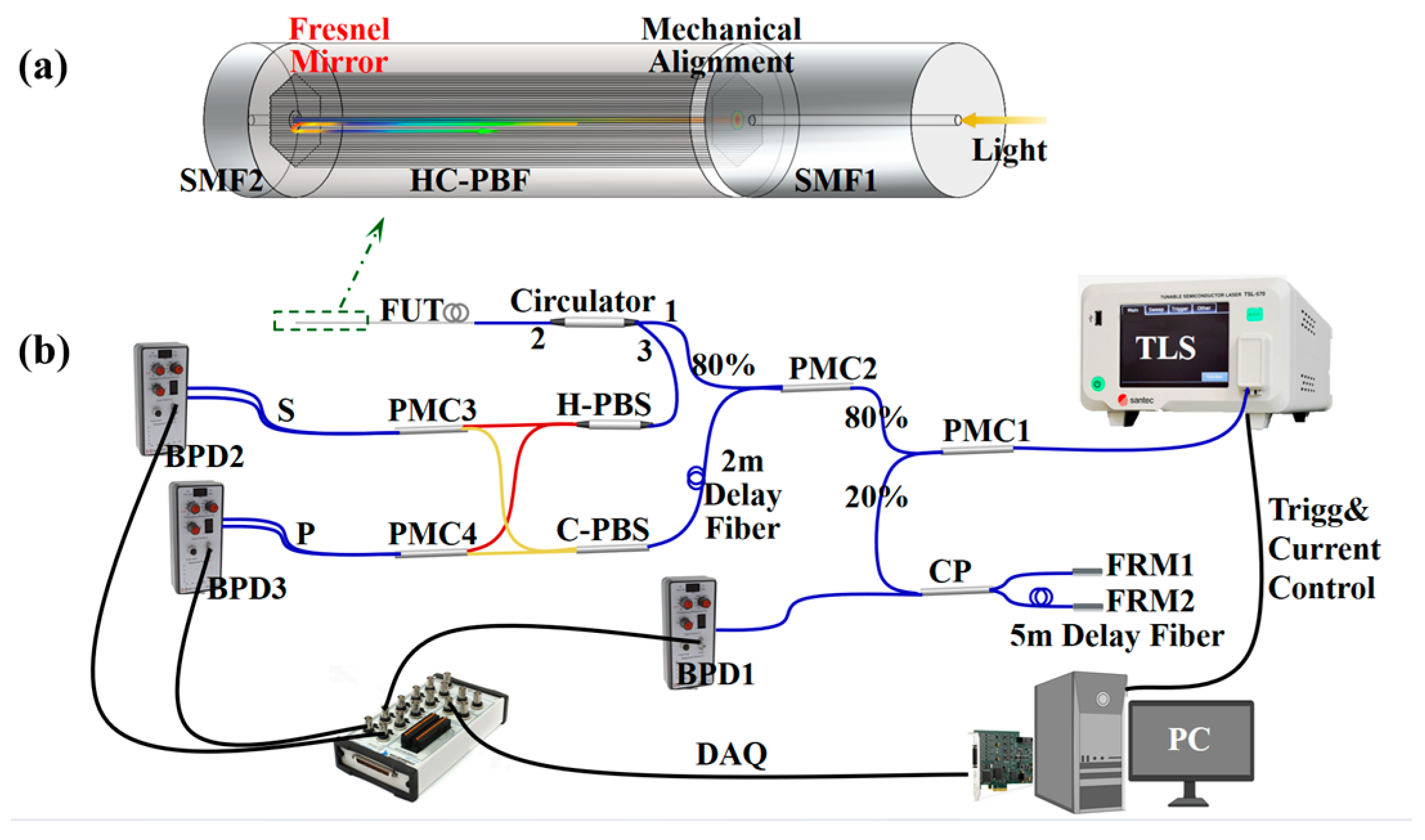
2. Principle and Experimental Setup
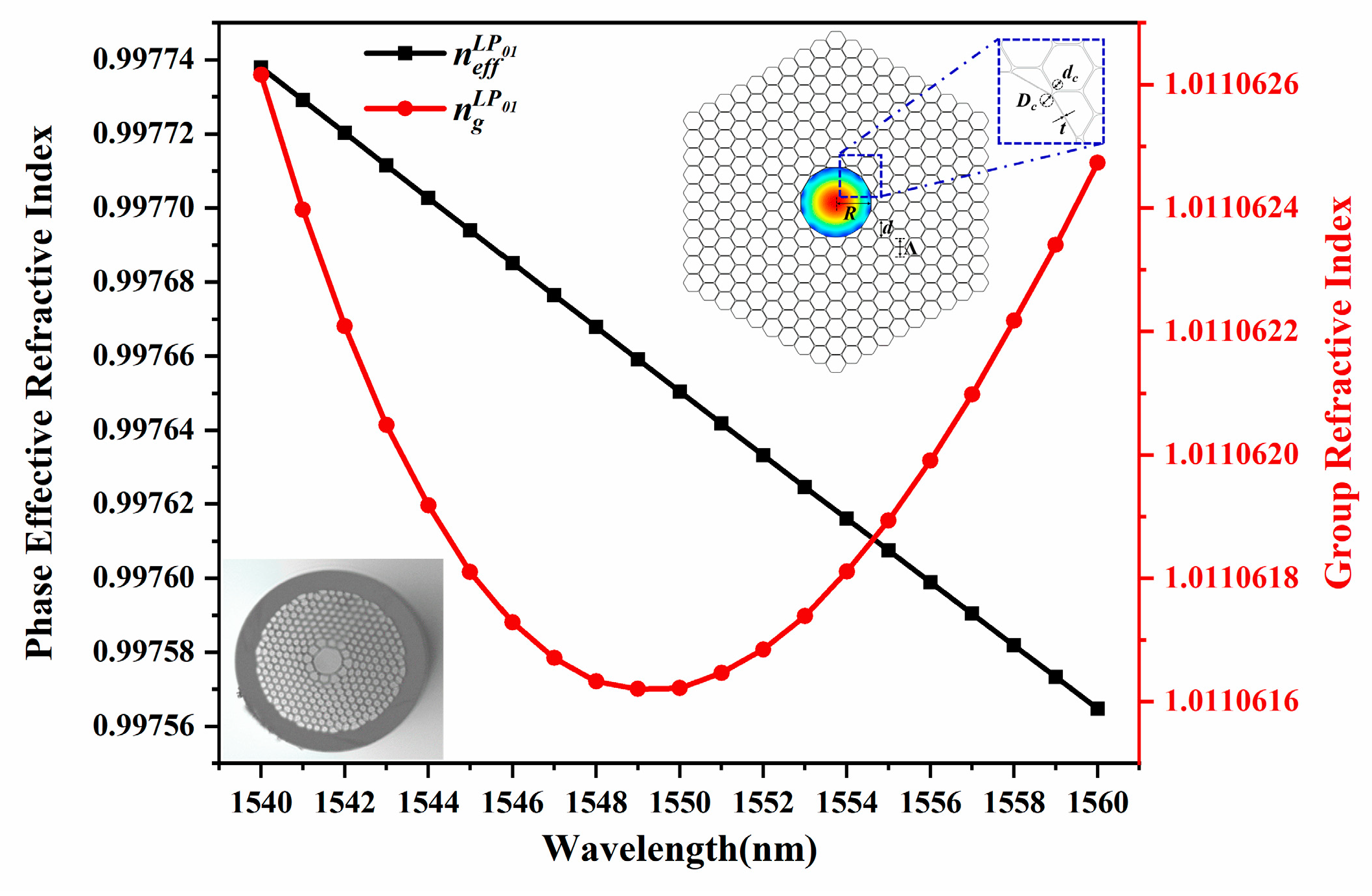
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Zhang, X.; Feng, Z.; Marpaung, D.; Fokoua, E.N.; Sakr, H.; Hayes, J.R.; Poletti, F.; Richardson, D.J.; Slavík, R. Low-loss microwave photonics links using hollow core fibres. Light. Sci. Appl. 2022, 11, 213. [Google Scholar] [CrossRef] [PubMed]
- Sakr, H.; Chen, Y.; Jasion, G.T.; Bradley, T.D.; Hayes, J.R.; Mulvad, H.C.H.; Davidson, I.A.; Fokoua, E.N.; Poletti, F. Hollow core optical fibres with comparable attenuation to silica fibres between 600 and 1100 nm. Nat. Commun. 2020, 11, 6030. [Google Scholar] [CrossRef] [PubMed]
- Gao, S.; Wang, Y.; Ding, W.; Hong, Y.; Wang, P. Conquering the Rayleigh Scattering Limit of Silica Glass Fiber at Visible Wavelengths with a Hollow-Core Fiber Approach. Laser Photonics Rev. 2019, 14, 1900241. [Google Scholar] [CrossRef]
- Cregan, R.F.; Mangan, B.J.; Knight, J.C.; Birks, T.A.; Russell, P.S.J.; Roberts, P.J.; Allan, D.C. Single-mode photonic band gap guidance of light in air. Science 1999, 285, 1537–1539. [Google Scholar] [CrossRef] [PubMed]
- Knight, J.C. Photonic crystal fibres. Nature 2003, 424, 847–851. [Google Scholar] [CrossRef]
- Poletti, F.; Wheeler, N.V.; Petrovich, M.N.; Baddela, N.; Fokoua, E.N.; Hayes, J.R.; Gray, D.R.; Li, Z.; Slavík, R.; Richardson, D.J. Towards high-capacity fibre-optic communications at the speed of light in vacuum. Nat. Photonics 2013, 7, 279–284. [Google Scholar] [CrossRef]
- Hong, Y.; Bradley, T.D.; Taengnoi, N.; Bottrill, K.R.H.; Hayes, J.R.; Jasion, G.T.; Poletti, F.; Petropoulos, P.; Richardson, D.J. Hollow-Core NANF for High-Speed Short-Reach Transmission in the S + C + L-Bands. J. Light. Technol. 2021, 39, 6167–6174. [Google Scholar] [CrossRef]
- Huang, L.; Wang, Y.; Zhang, Y.; Cheng, T.; Wang, L.; Jiang, H. High-efficiency 6-hole structure anti-resonant hollow-core fiber 2.79 μm Cr, Er:YSGG high-energy pulse laser transmission system. Opt. Laser Technol. 2024, 175, 110743. [Google Scholar] [CrossRef]
- Fokoua, E.N.; Mousavi, S.A.; Jasion, G.T.; Richardson, D.J.; Poletti, F. Loss in hollow-core optical fibers: Mechanisms, scaling rules, and limits. Adv. Opt. Photon. 2023, 15, 1–85. [Google Scholar] [CrossRef]
- Osório, J.H.; Amrani, F.; Delahaye, F.; Dhaybi, A.; Vasko, K.; Melli, F.; Giovanardi, F.; Vandembroucq, D.; Tessier, G.; Vincetti, L.; et al. Hollow-core fibers with reduced surface roughness and ultralow loss in the short-wavelength range. Nat. Commun. 2023, 14, 1146. [Google Scholar] [CrossRef]
- Jasion, G.T.; Sakr, H.; Hayes, J.R.; Sandoghchi, S.R.; Hooper, L.; Fokoua, E.N.; Saljoghei, A.; Mulvad, H.C.; Alonso, M.; Taranta, A.; et al. 0.174 dB/km hollow core double nested antiresonant nodeless fiber (DNANF). In Proceedings of the 2022 Optical Fiber Communications Conference and Exhibition (OFC), San Diego, CA, USA, 10 March 2022; pp. 1–3. [Google Scholar]
- Danielmeyer, H.G.; Weber, H.P. Direct measurement of the group velocity of light. Phys. Rev. A 1971, 3, 1708. [Google Scholar] [CrossRef]
- Danielson, B.L. Precise length measurements in multimode optical fibers. Appl. Opt. 1991, 30, 3867–3872. [Google Scholar] [CrossRef] [PubMed]
- Shen, W.; Du, J.; Sun, L.; Wang, C.; Zhu, Y.; Xu, K.; Chen, B.; He, Z. Low-Latency and High-Speed Hollow-Core Fiber Optical Interconnection at 2-Micron Waveband. J. Light. Technol. 2020, 38, 3874–3882. [Google Scholar] [CrossRef]
- Hong, Y.; Bottrill, K.R.H.; Bradley, T.D.; Sakr, H.; Jasion, G.T.; Harrington, K.; Poletti, F.; Petropoulos, P.; Richardson, D.J. Low-Latency WDM Intensity-Modulation and Direct-Detection Transmission over >100 km Distances in a Hollow Core Fiber. Laser Photonics Rev. 2021, 15, 2100102. [Google Scholar] [CrossRef]
- Kuschnerov, M.; Mangan, B.J.; Gong, K.; Sleiffer, V.A.J.M.; Herrmann, M.; Nicholson, J.W.; Fini, J.M.; Meng, L. Transmission of commercial low latency interfaces over hollow-core fiber. J. Light. Technol. 2016, 34, 314–320. [Google Scholar] [CrossRef]
- Cruz, J.L.; Barmenkov, Y.O.; Díez, A.; Andres, M.V. Measurement of phase and group refractive indices and dispersion of thermo-optic and strain-optic coefficients of optical fibers using weak fiber Bragg gratings. Appl. Opt. 2021, 60, 2824–2832. [Google Scholar] [CrossRef]
- Mori, K.; Morioka, T.; Saruwatari, M. Group velocity dispersion measurement using supercontinuum picosecond pulses generated in an optical fibre. Electron. Lett. 1993, 29, 987–989. [Google Scholar] [CrossRef]
- Arosa, Y.; Fuente, R.D.L. Refractive index spectroscopy and material dispersion in fused silica glass. Opt. Lett. 2020, 45, 4268–4271. [Google Scholar] [CrossRef]
- Zhang, X.; Gao, S.; Wang, Y.; Ding, W.; Wang, X.; Wang, P. 7-cell hollow-core photonic bandgap fiber with broad spectral bandwidth and low loss. Opt. Express 2019, 27, 11608–11616. [Google Scholar] [CrossRef]
- Michaud-Belleau, V.; Fokoua, E.N.; Bradley, T.D.; Hayes, J.R.; Chen, Y.; Poletti, F.; Richardson, D.J.; Genest, J.; Slavik, R. Backscattering in antiresonant hollow-core fibers: Over 40 dB lower than in standard optical fibers. Optica 2021, 8, 216–219. [Google Scholar] [CrossRef]
- Luo, M.; Liu, J.; Tang, C.; Wang, X.; Lan, T.; Kan, B. 0.5 mm spatial resolution distributed fiber temperature and strain sensor with position-deviation compensation based on OFDR. Opt. Express 2019, 27, 35823–35829. [Google Scholar] [CrossRef] [PubMed]
- Xu, X.; Luo, M.; Liu, J.; Gao, Q.; Li, C.; Guo, H.; Liu, Y.-G.; Ge, C. Observation and characterization of the high order modes in a six-mode fiber using an OFDR method. Opt. Express 2022, 30, 26341–26347. [Google Scholar] [CrossRef] [PubMed]
- Ahn, T.-J.; Kim, D.Y. High-resolution differential mode delay measurement for a multimode optical fiber using a modified optical frequency domain reflectometer. Opt. Express 2005, 13, 8256–8262. [Google Scholar] [CrossRef] [PubMed]
- Gao, Q.; Luo, M.; Liu, J.; Luan, N.; Xu, X.; Li, C.; He, Y. Group Birefringence Regulation and Measurement with Twin Zeros in a Selectively Infiltrated Microstructured Optical Fiber Based on OFDR. J. Light. Technol. 2022, 40, 308–312. [Google Scholar] [CrossRef]
- Meng, X.; Luo, M.; Liu, J.; Zhao, S.; Zhou, R. Birefringence characterization in a dual-hole microstructured optical fiber using an OFDR method. Appl. Opt. 2024, 63, 772–776. [Google Scholar] [CrossRef]
- Malitson, I.H. Interspecimen comparison of the refractive index of fused silica. J. Opt. Soc. Am. 1965, 55, 1205–1209. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cao, X.; Luo, M.; Liu, J.; Ma, J.; Hao, Y.; Liu, Y. Speed of Light in Hollow-Core Photonic Bandgap Fiber Approaching That in Vacuum. Sensors 2024, 24, 6954. https://doi.org/10.3390/s24216954
Cao X, Luo M, Liu J, Ma J, Hao Y, Liu Y. Speed of Light in Hollow-Core Photonic Bandgap Fiber Approaching That in Vacuum. Sensors. 2024; 24(21):6954. https://doi.org/10.3390/s24216954
Chicago/Turabian StyleCao, Xiaolu, Mingming Luo, Jianfei Liu, Jie Ma, Yundong Hao, and Yange Liu. 2024. "Speed of Light in Hollow-Core Photonic Bandgap Fiber Approaching That in Vacuum" Sensors 24, no. 21: 6954. https://doi.org/10.3390/s24216954
APA StyleCao, X., Luo, M., Liu, J., Ma, J., Hao, Y., & Liu, Y. (2024). Speed of Light in Hollow-Core Photonic Bandgap Fiber Approaching That in Vacuum. Sensors, 24(21), 6954. https://doi.org/10.3390/s24216954





