Development and Validation of a New Type of Displacement-Based Miniatured Laser Vibrometers
Abstract
1. Introduction
2. Working Principles Used in This Work
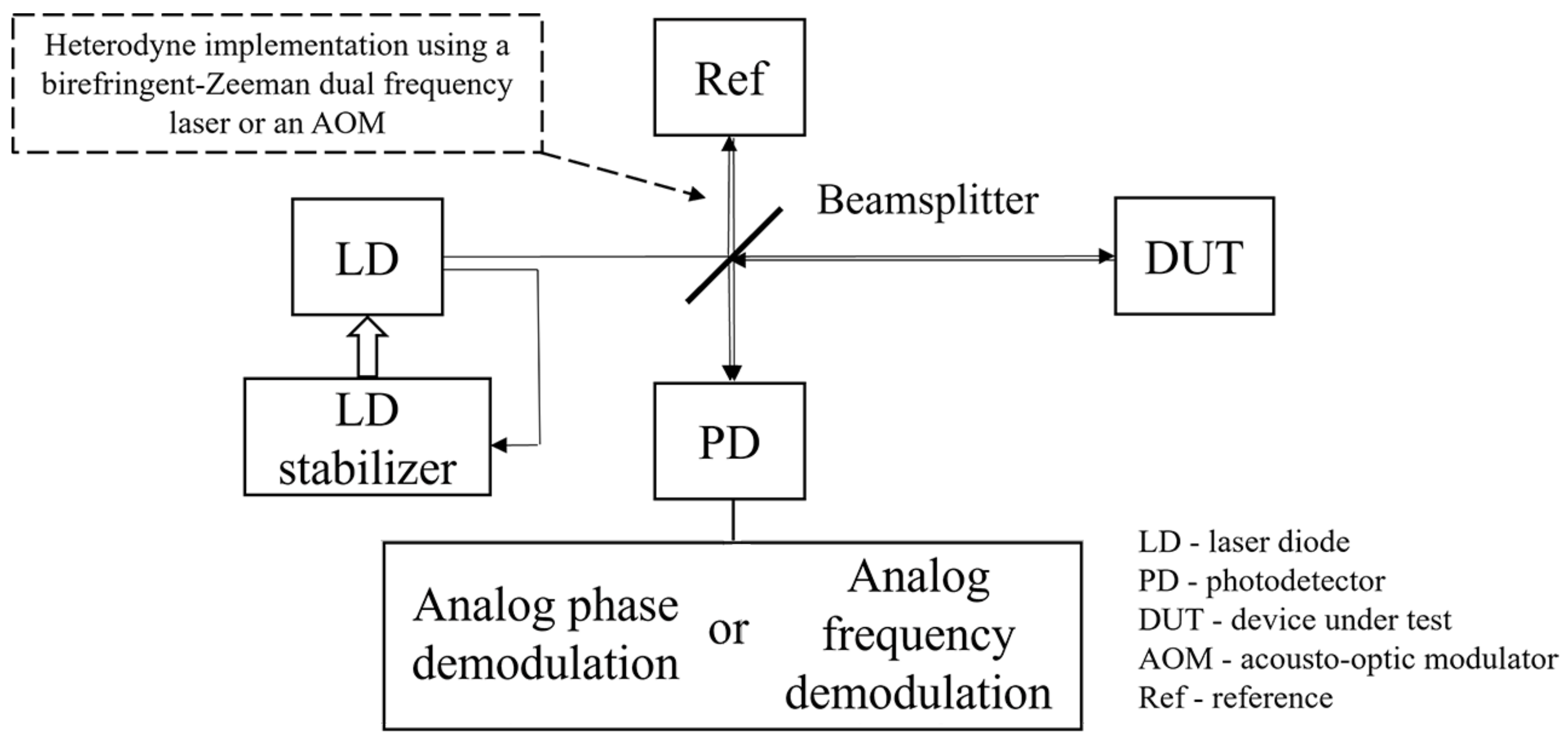
2.1. Basic Working Principles of Laser Interferometers
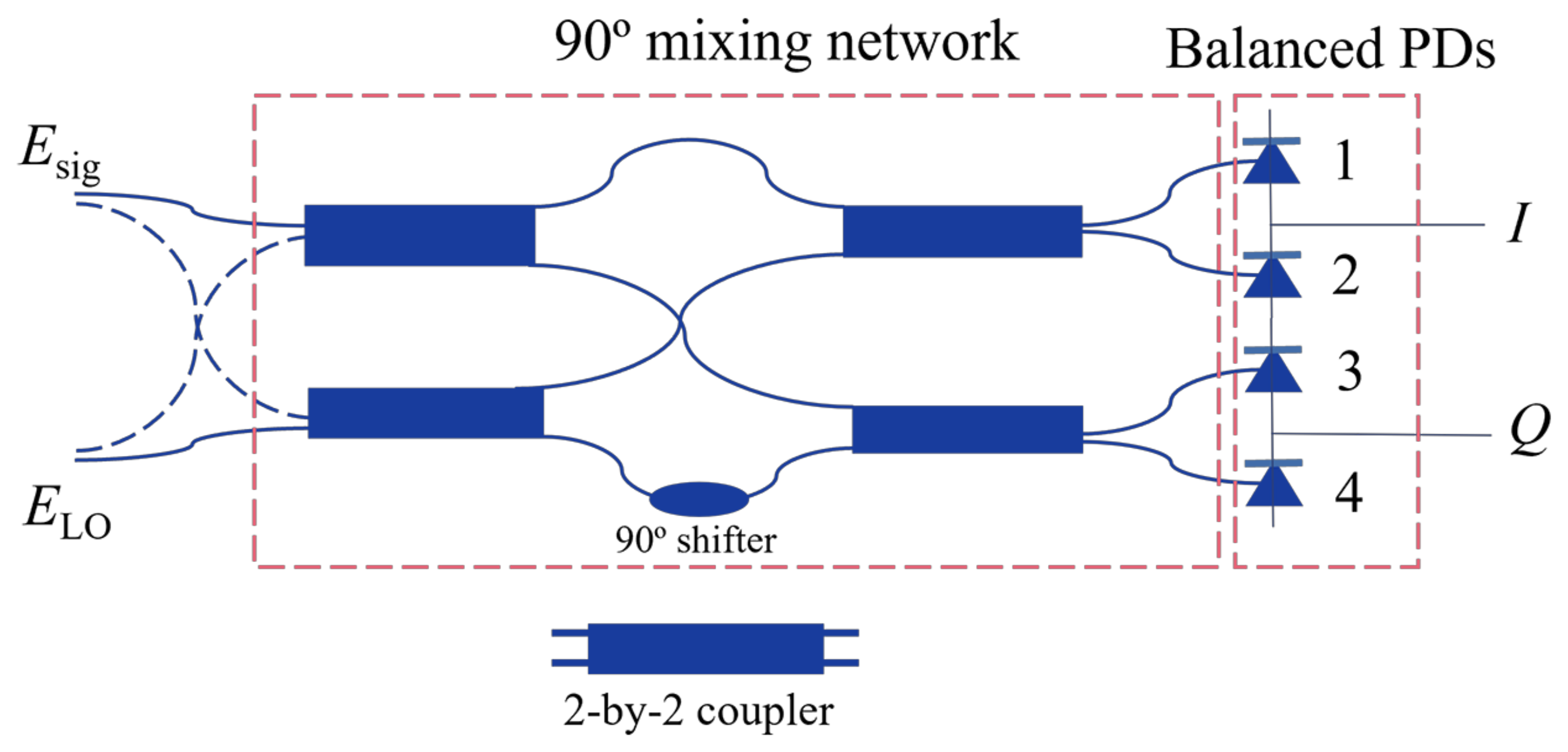
2.2. Working Principle of a Quadrature Coherent Detector
2.3. Working Principle of the Proposed Laser Vibrometer
3. Performance of Laser Vibrometers by Integrated Photonics
3.1. Advantages of Integrated Photonics-Based Laser Vibrometers
3.2. Performance of Laser Vibrometers Based on Integrated Optics
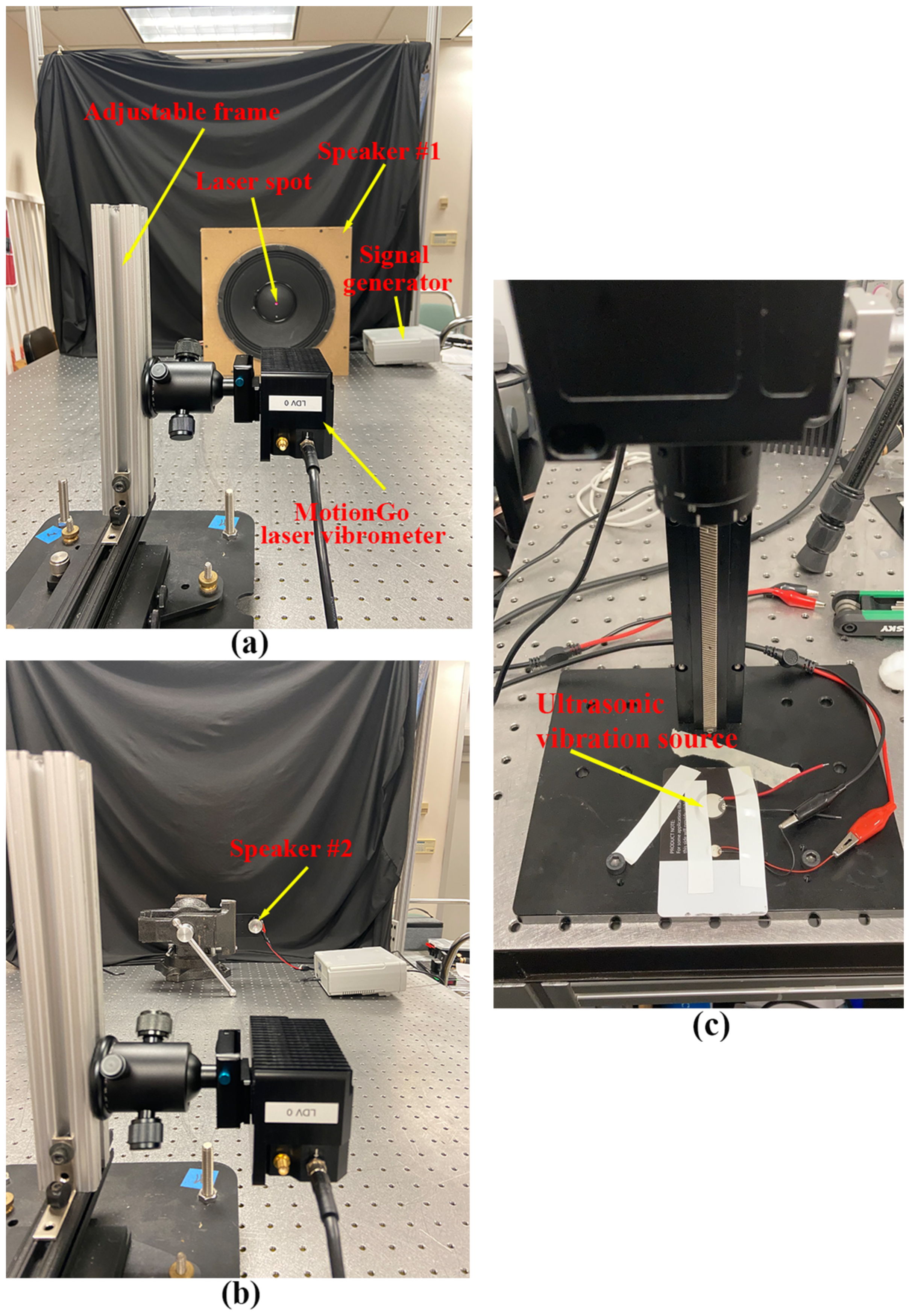
4. Experimental Validation
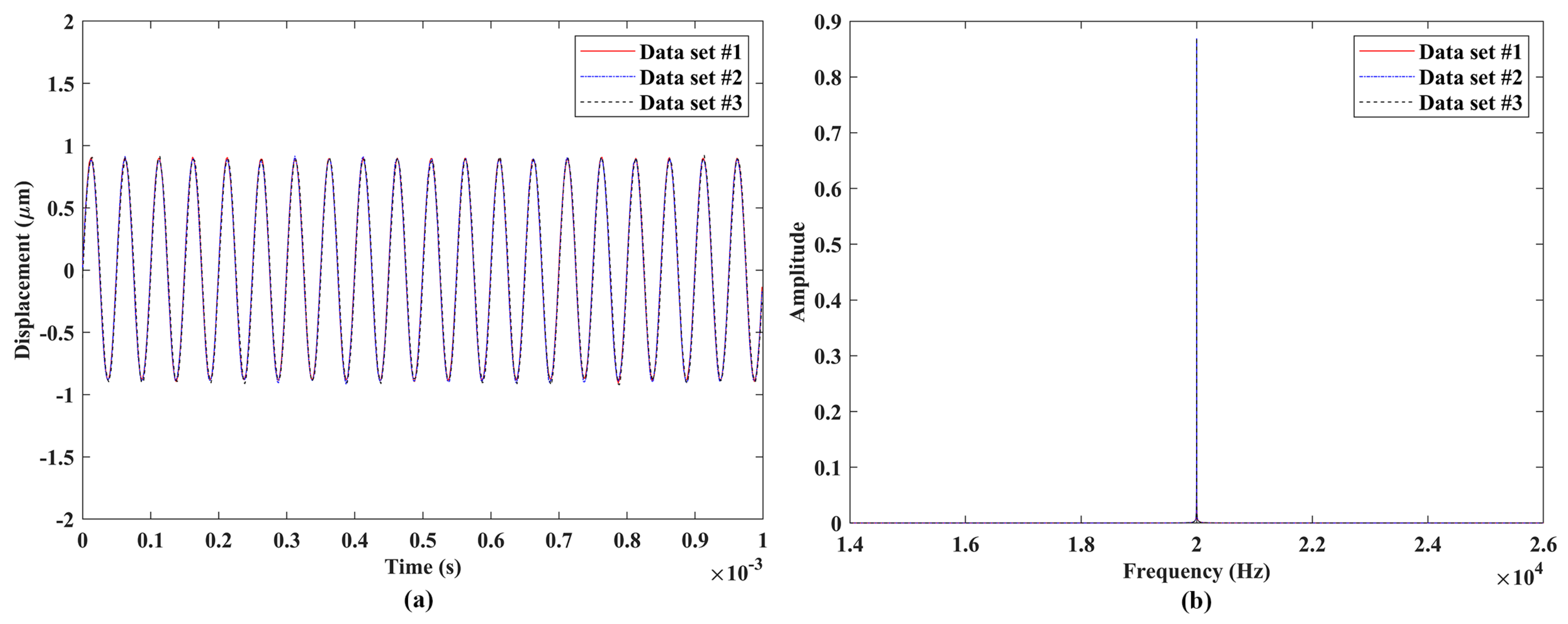
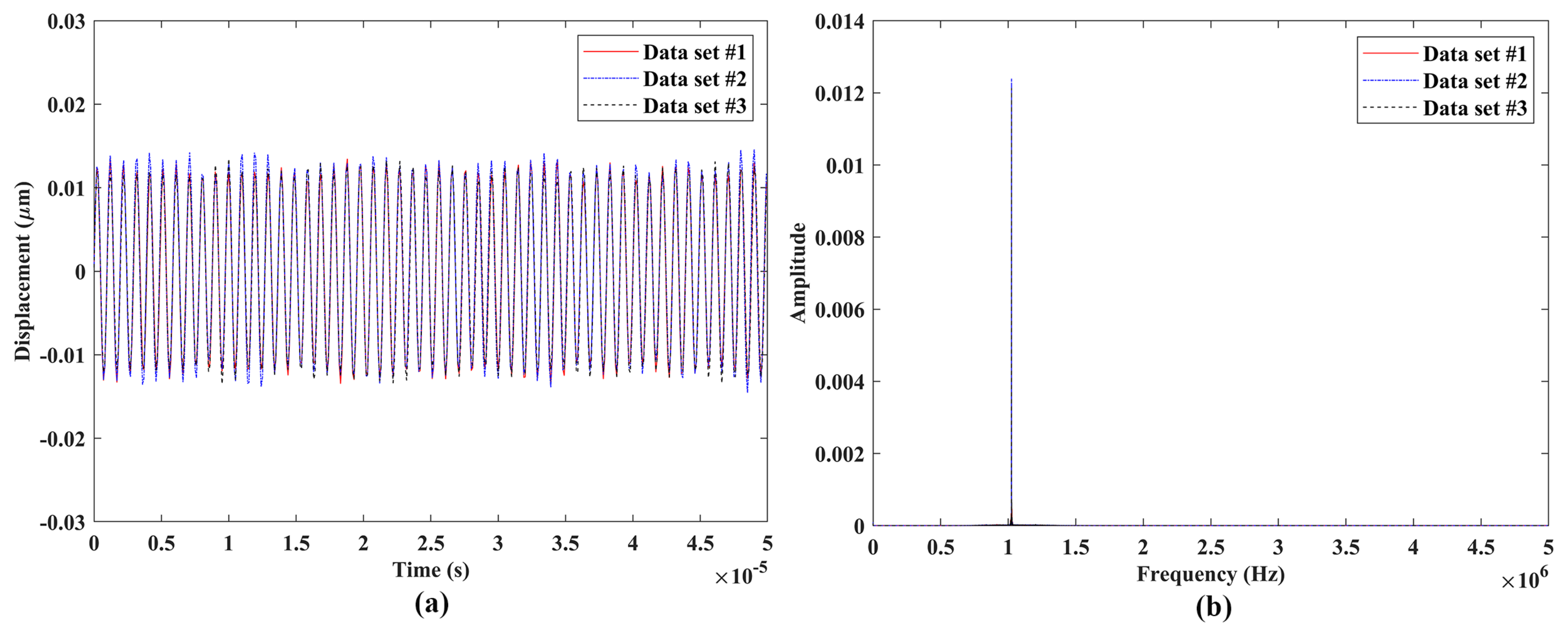
4.1. Measurement Repeatability
4.2. Measurement Accuracy
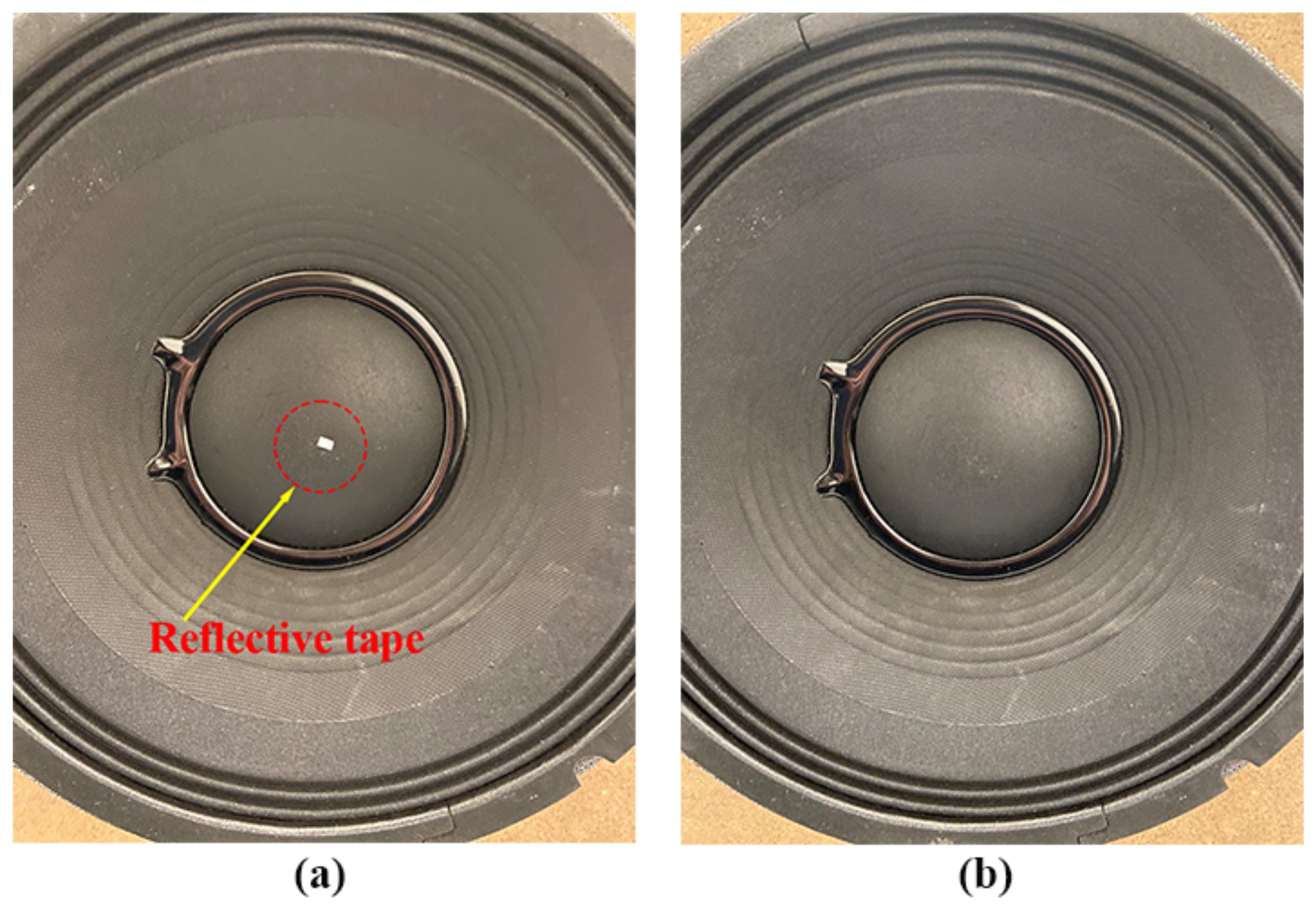
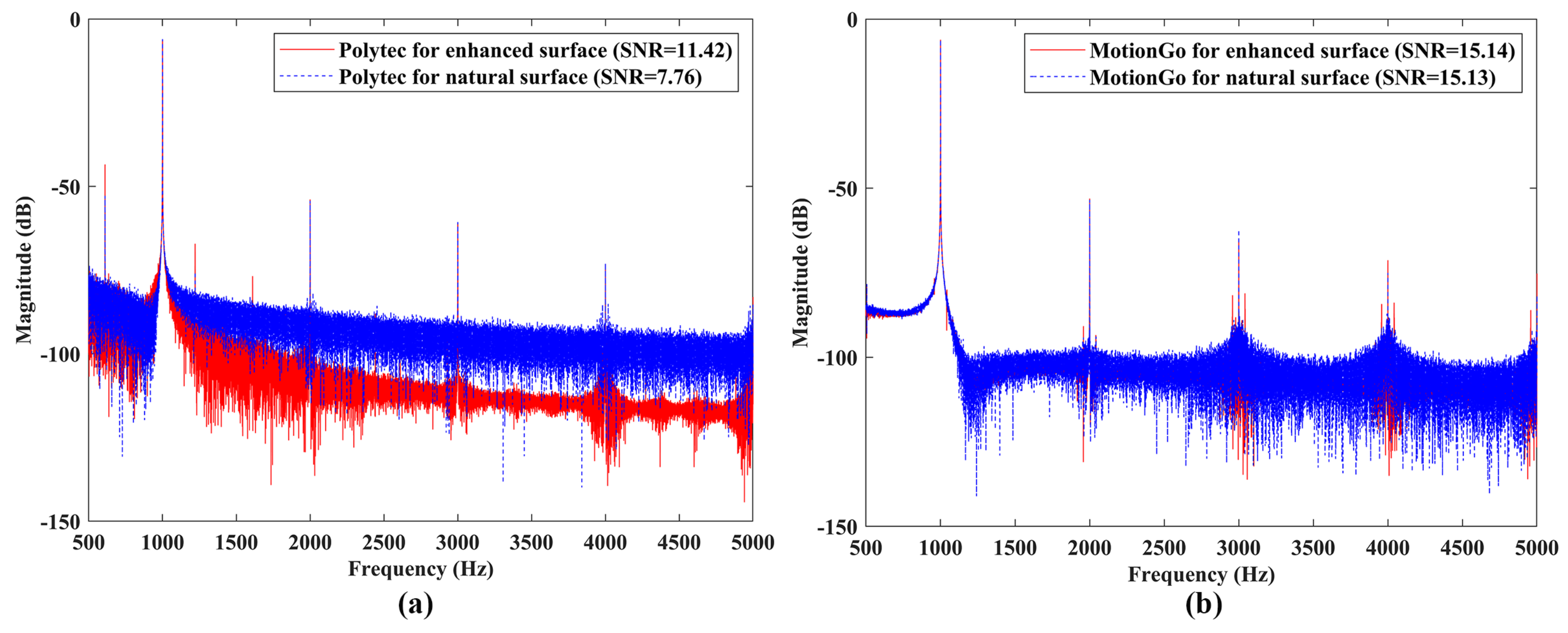
4.3. Robustness to Test Surface Conditions
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yuan, K.; Zhu, W. Identification of modal parameters of a model turbine blade with a curved surface under random excitation with a three-dimensional continuously scanning laser Doppler vibrometer system. Measurement 2023, 214, 112759. [Google Scholar] [CrossRef]
- Yuan, K.; Zhu, W. A novel mirror-assisted method for full-field vibration measurement of a hollow cylinder using a three-dimensional continuously scanning laser Doppler vibrometer system. Mech. Syst. Signal Process. 2024, 216, 111428. [Google Scholar] [CrossRef]
- Lyu, L.; Yuan, K.; Zhu, W. A novel demodulation method with a reference signal for operational modal analysis and baseline-free damage detection of a beam under random excitation. J. Sound Vib. 2024, 571, 118068. [Google Scholar] [CrossRef]
- Yuan, K.; Zhu, W. In-plane operating deflection shape measurement of an aluminum plate using a three-dimensional continuously scanning laser Doppler vibrometer system. Exp. Mech. 2022, 62, 667–676. [Google Scholar] [CrossRef]
- Rezaei, D.; Taheri, F. A novel application of a laser Doppler vibrometer in a health monitoring system. J. Mech. Mater. Struct. 2010, 5, 289–304. [Google Scholar] [CrossRef]
- Nassif, H.H.; Gindy, M.; Davis, J. Comparison of laser Doppler vibrometer with contact sensors for monitoring bridge deflection and vibration. NDT E Int. 2005, 38, 213–218. [Google Scholar] [CrossRef]
- Yu, T.; Tang, Q.; Vinayaka, S. Identifying structural properties of a steel railway bridge for structural health monitoring using laser Doppler vibrometry. Autom. Constr. 2024, 160, 105320. [Google Scholar] [CrossRef]
- Roozen, N.B.; Labelle, L.; Rychtáriková, M.; Glorieux, C. Determining radiated sound power of building structures by means of laser Doppler vibrometry. J. Sound Vib. 2015, 346, 81–99. [Google Scholar] [CrossRef]
- Huang, Z.; Xie, M.; Chen, C.; Du, Y.; Zhao, J. Engineering application of a safety-state evaluation model for hidden frame-supported glass curtain walls based on remote vibration. J. Build. Eng. 2019, 26, 100915. [Google Scholar] [CrossRef]
- Zabihi, A.; Aghdasi, F.; Ellouzi, C.; Singh, N.K.; Jha, R.; Shen, C. Non-Contact Wind Turbine Blade Crack Detection Using Laser Doppler Vibrometers. Energies 2024, 17, 2165. [Google Scholar] [CrossRef]
- Yuan, K.; Zhu, W. Modeling of welded joints in a pyramidal truss sandwich panel using beam and shell finite elements. J. Vib. Acoust. 2021, 143, 041002. [Google Scholar] [CrossRef]
- Hameury, C.; Ferrari, G.; Franchini, G.; Amabili, M. An experimental approach to multi-input multi-output nonlinear active vibration control of a clamped sandwich beam. Mech. Syst. Signal Process. 2024, 216, 111496. [Google Scholar] [CrossRef]
- Garg, P.; Moreu, F.; Ozdagli, A.; Taha, M.R.; Mascareñas, D. Noncontact dynamic displacement measurement of structures using a moving laser Doppler vibrometer. J. Bridge Eng. 2019, 24, 04019089. [Google Scholar] [CrossRef]
- Garg, P.; Nasimi, R.; Ozdagli, A.; Zhang, S.; Mascarenas, D.D.L.; Reda Taha, M.; Moreu, F. Measuring transverse displacements using unmanned aerial systems laser Doppler vibrometer (UAS-LDV): Development and field validation. Sensors 2020, 20, 6051. [Google Scholar] [CrossRef] [PubMed]
- Bertini, L.; Neri, P.; Santus, C.; Guglielmo, A. Automated experimental modal analysis of bladed wheels with an anthropomorphic robotic station. Exp. Mech. 2017, 57, 273–285. [Google Scholar] [CrossRef]
- Margerit, P.; Gobin, T.; Lebée, A.; Caron, J.F. The robotized laser doppler vibrometer: On the use of an industrial robot arm to perform 3D full-field velocity measurements. Opt. Lasers Eng. 2021, 137, 106363. [Google Scholar] [CrossRef]
- Ahmed, H.; Mohsin, A.; Hong, S.C.; Lee, J.R.; Ihn, J.B. Robotic laser sensing and laser mirror excitation for pulse-echo scanning inspection of fixed composite structures with non-planar geometries. Measurement 2021, 176, 109109. [Google Scholar] [CrossRef]
- Brethee, K.F.; Uwayed, A.N.; Alden Qwam, A.Y. A novel index for vibration-based damage detection technique in laminated composite plates under forced vibrations: Experimental study. Struct. Health Monit. 2023, 22, 3109–3125. [Google Scholar] [CrossRef]
- Li, Y.; Verstuyft, S.; Yurtsever, G.; Keyvaninia, S.; Roelkens, G.; Van Thourhout, D.; Baets, R. Heterodyne laser Doppler vibrometers integrated on silicon-on-insulator based on serrodyne thermo-optic frequency shifters. Appl. Opt. 2013, 52, 2145–2152. [Google Scholar] [CrossRef]
- Li, Y.; Baets, R. Homodyne laser Doppler vibrometer on silicon-on-insulator with integrated 90 degree optical hybrids. Opt. Express 2013, 21, 13342–13350. [Google Scholar] [CrossRef]
- Li, Y.; Dieussaert, E.; Baets, R. Miniaturization of laser doppler vibrometers—A review. Sensors 2022, 22, 4735. [Google Scholar] [CrossRef] [PubMed]
- Ramasubramanian, K.; Instruments, T. Using a complex-baseband architecture in FMCW radar systems. Tex. Instrum. 2017, 19, 1–10. [Google Scholar]
- Nguyen, T.H.; Scalart, P.; Gay, M.; Bramerie, L.; Peucheret, C.; Gomez-Agis, F.; Sentieys, O.; Simon, J.C.; Joindot, M. New metric for IQ imbalance compensation in optical QPSK coherent systems. Photonic Netw. Commun. 2018, 36, 326–337. [Google Scholar] [CrossRef]
- Magden, E.S.; Li, N.; Raval, M.; Poulton, C.V.; Ruocco, A.; Singh, N.; Vermeulen, D.; Ippen, E.P.; Kolodziejski, L.A.; Watts, M.R. Transmissive silicon photonic dichroic filters with spectrally selective waveguides. Nat. Commun. 2018, 9, 3009. [Google Scholar] [CrossRef] [PubMed]









| Parameter (Unit): | Value | Parameter (Unit): | Value |
|---|---|---|---|
| Measurement distance (m) | 0.025–100 | Measurement frequency range (MHz) | DC-2.5 |
| Noise density | <0.1 | Speed range (m/s) | >20 |
| Displacement resolution (nm) | 0.01 | Displacement repeatability (nm) (100 kHz receiver bandwidth) | <0.1 |
| Laser specification | 1310 nm measurement, 655 mm indicator | Measurement laser output power (mW) | <5 |
| Laser safety class | Class I | Indicator laser output power | Class I or adjustable |
| DUT | Excitation Frequency (Hz) | Dataset #1 | Dataset #2 | Dataset #3 | Differences |
|---|---|---|---|---|---|
| Speaker #1 | 0.1 | 1154.4 | 1149.7 | 1149.3 | 0.4% & 0.4% |
| 1 | 116.1 | 116.2 | 116.3 | 0.1% & 0.2% | |
| 10 | 75.3 | 75.2 | 75.4 | 0.1% & 0.1% | |
| 100 | 30.3 | 30.3 | 30.3 | 0 & 0 | |
| 1000 | 0 & 0 | ||||
| Speaker #2 | 5000 | 0 & 0 | |||
| 20,000 | 0 & 0 | ||||
| Ultrasonic vibration source | 1,000,000 | 0 & 0 |
| DUT | Excitation Frequency (Hz) | Polytec Measurement | MotionGo Measurement | Differences |
|---|---|---|---|---|
| Speaker #1 | 0.1 | 1157.99 | 1157.97 | <<0.01% |
| 1 | 600.95 | 595.17 | 0.96% | |
| 10 | 75.50 | 74.97 | 0.70% | |
| 100 | 44.67 | 44.61 | 0.13% | |
| 1000 | 0.40% | |||
| Speaker #2 | 5000 | 2.03 | 2.04 | 0.49% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, K.; Zhu, Z.; Chen, W.; Zhu, W. Development and Validation of a New Type of Displacement-Based Miniatured Laser Vibrometers. Sensors 2024, 24, 5230. https://doi.org/10.3390/s24165230
Yuan K, Zhu Z, Chen W, Zhu W. Development and Validation of a New Type of Displacement-Based Miniatured Laser Vibrometers. Sensors. 2024; 24(16):5230. https://doi.org/10.3390/s24165230
Chicago/Turabian StyleYuan, Ke, Zhonghua Zhu, Wei Chen, and Weidong Zhu. 2024. "Development and Validation of a New Type of Displacement-Based Miniatured Laser Vibrometers" Sensors 24, no. 16: 5230. https://doi.org/10.3390/s24165230
APA StyleYuan, K., Zhu, Z., Chen, W., & Zhu, W. (2024). Development and Validation of a New Type of Displacement-Based Miniatured Laser Vibrometers. Sensors, 24(16), 5230. https://doi.org/10.3390/s24165230






