Self-Excited Microcantilever with Higher Mode Using Band-Pass Filter
Abstract
1. Introduction
2. Method to Produce Self-Excited Oscillation with Higher Mode in Cantilever Beam
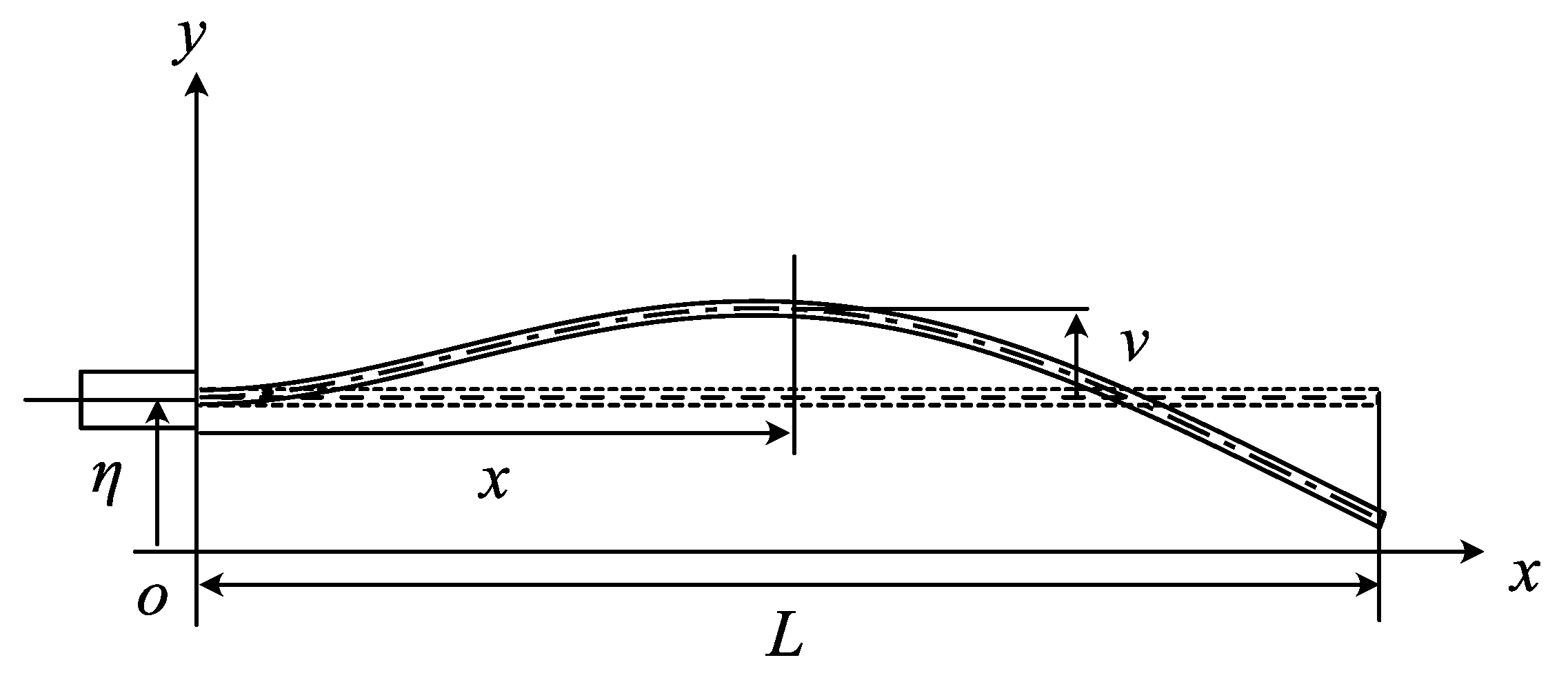
2.1. Analytical Model of the Cantilever Subject to a Base Excitation
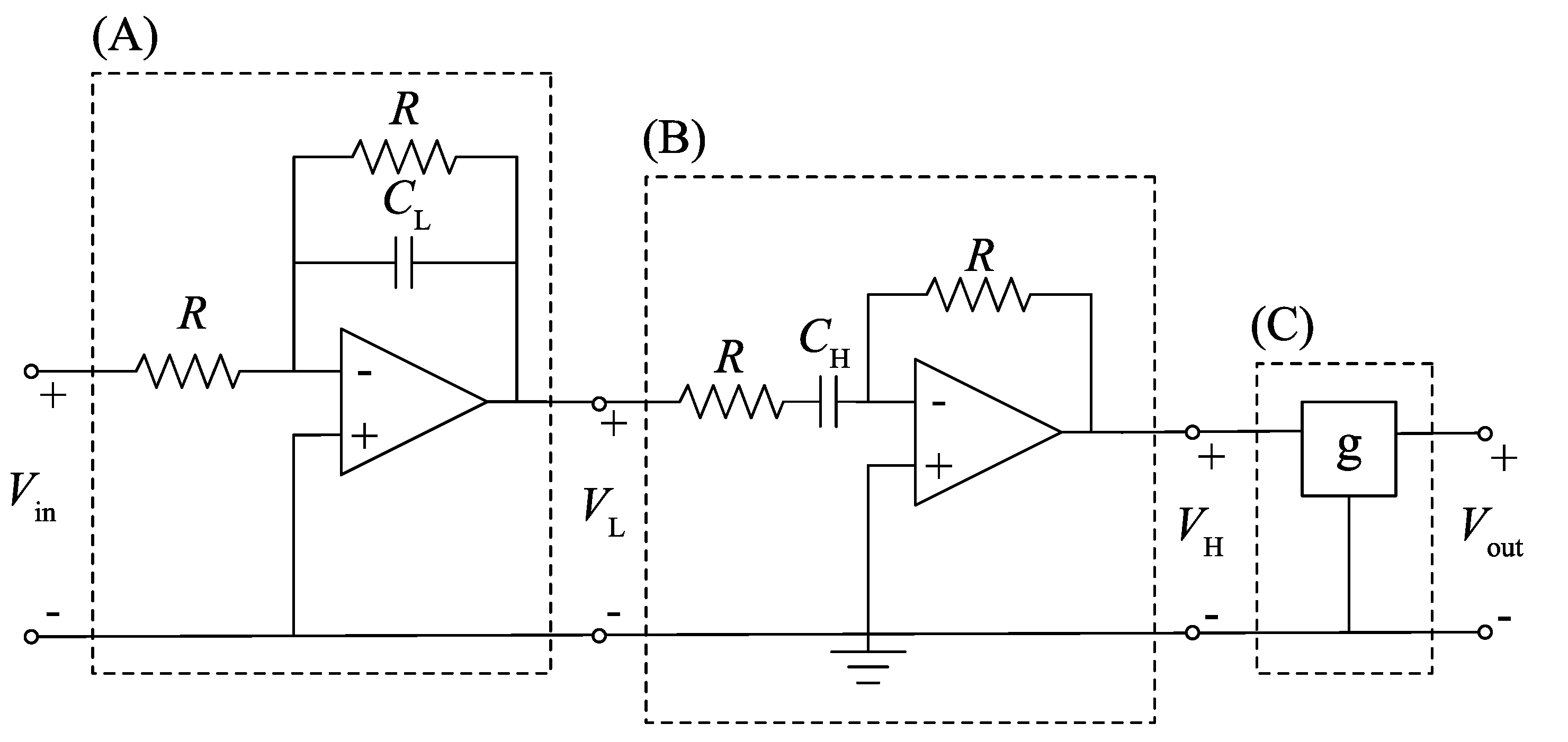
2.2. Genaration of Self-Excited Oscillation in a Higher Mode Using Band-Pass Filter
| Parameter | Value |
|---|---|
| 1.017 | |
| 0.73 | |
| 0.1 |
3. Experiments for Self-Excited Oscillation in Second Mode Using Microcantilever
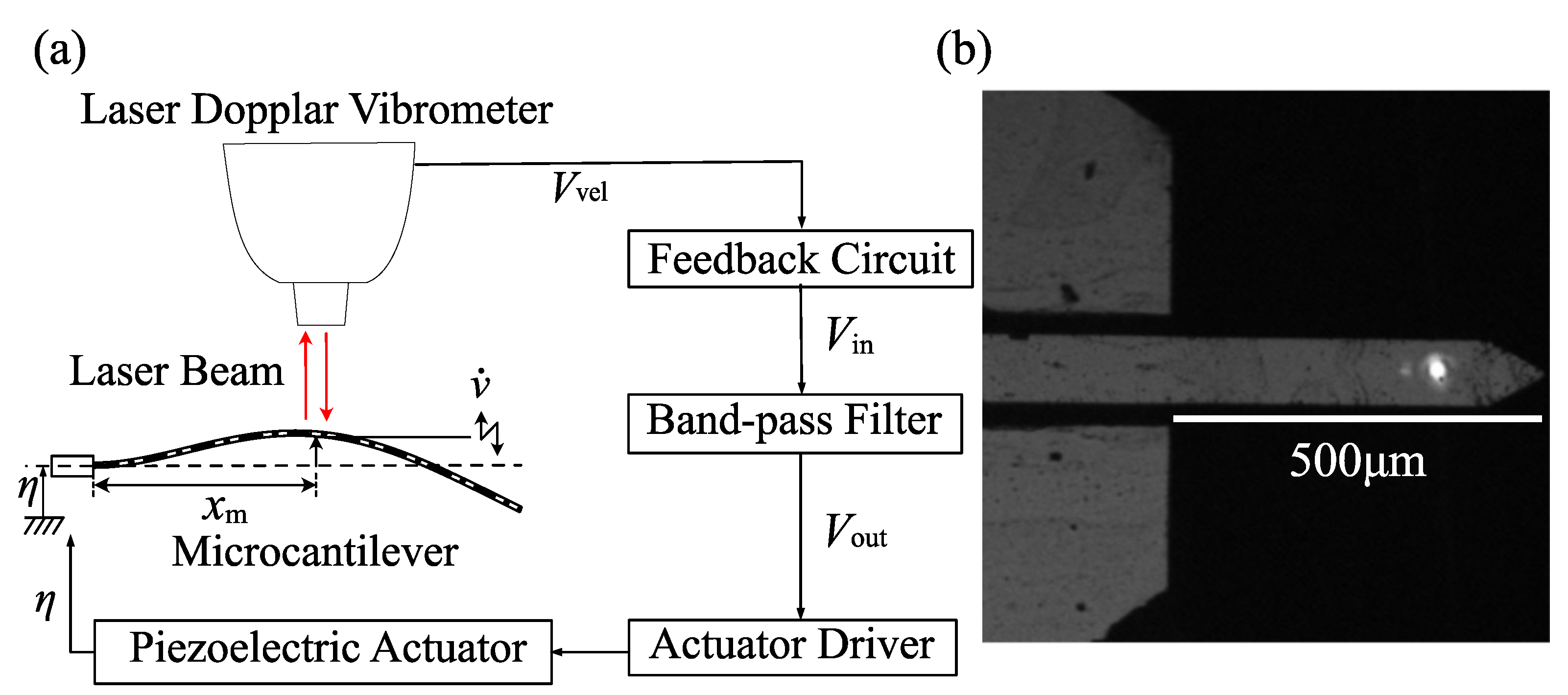
3.1. Experiment Set-Up
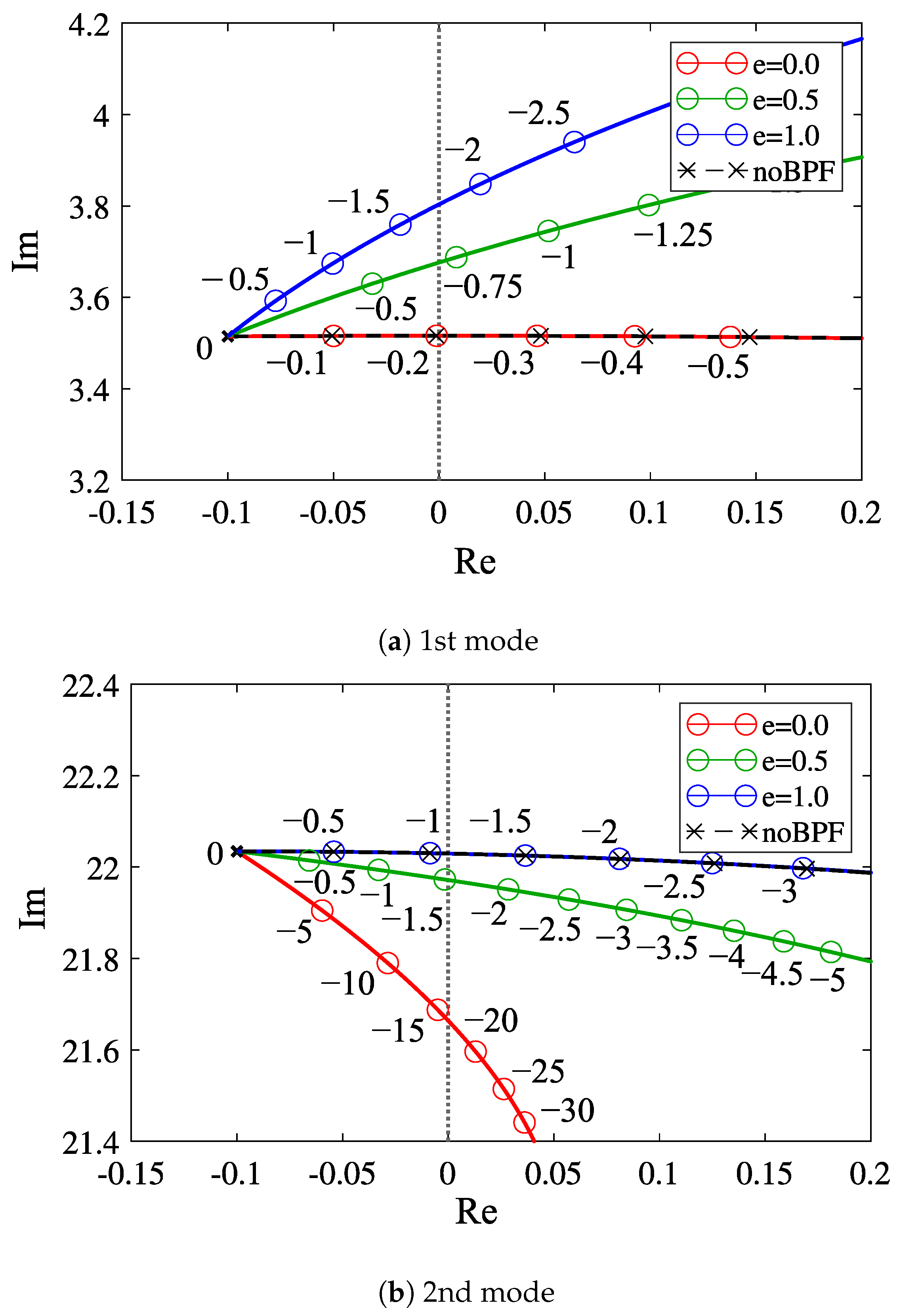
3.2. Experimental Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| FPGA | Field-Programmable Gate Array |
| FFT | Fast Fourier Translation |
Appendix A
Appendix B
References
- Abdolvand, R.; Bahreyni, B.; Lee, J.E.Y.; Nabki, F. Micromachined Resonators: A Review. Micromachines 2016, 7, 160. [Google Scholar] [CrossRef]
- Gupta, A.; Akin, D.; Bashir, R. Single virus particle mass detection using microresonators with nanoscale thickness. Appl. Phys. Lett. 2004, 84, 1976–1978. [Google Scholar] [CrossRef]
- Nyang’au, W.O.; Setiono, A.; Schmidt, A.; Bosse, H.; Peiner, E. Sampling and Mass Detection of a Countable Number of Microparticles Using on-Cantilever Imprinting. Sensors 2020, 20, 2508. [Google Scholar] [CrossRef]
- Miranda-Martínez, A.; Rivera-González, M.X.; Zeinoun, M.; Carvajal-Ahumada, L.A.; Serrano-Olmedo, J.J. Viscosity Measurement Sensor: A Prototype for a Novel Medical Diagnostic Method Based on Quartz Crystal Resonator. Sensors 2021, 21, 2743. [Google Scholar] [CrossRef]
- Ono, T.; Li, X.; Miyashita, H.; Esashi, M. Mass sensing of adsorbed molecules in sub-picogram sample with ultrathin silicon resonator. Rev. Sci. Instrum. 2003, 74, 1240–1243. [Google Scholar] [CrossRef]
- Kokubun, Y.; Yabuno, H. Stiffness Sensor for Cubic Nonlinear Elasticity Using Nonlinear Self-Excited Oscillation. J. Vib. Acoust. 2014, 136, 031011. [Google Scholar] [CrossRef]
- Matko, V.; Milanovič, M. Detection Principles of Temperature Compensated Oscillators with Reactance Influence on Piezoelectric Resonator. Sensors 2020, 20, 802. [Google Scholar] [CrossRef]
- Lochon, F.; Dufour, I.; Rebière, D. An alternative solution to improve sensitivity of resonant microcantilever chemical sensors: comparison between using high-order modes and reducing dimensions. Sens. Actuators B Chem. 2005, 108, 979–985. [Google Scholar] [CrossRef]
- Le, X.; Peng, L.; Pang, J.; Xu, Z.; Gao, C.; Xie, J. Humidity sensors based on AlN microcantilevers excited at high-order resonant modes and sensing layers of uniform graphene oxide. Sens. Actuators B Chem. 2019, 283, 198–206. [Google Scholar] [CrossRef]
- Ghatkesar, M.K.; Barwich, V.; Braun, T.; Ramseyer, J.P.; Gerber, C.; Hegner, M.; Lang, H.P.; Drechsler, U.; Despont, M. Higher modes of vibration increase mass sensitivity in nanomechanical microcantilevers. Nanotechnology 2007, 18, 445502. [Google Scholar] [CrossRef]
- Dohn, S.; Sandberg, R.; Svendsen, W.; Boisen, A. Enhanced functionality of cantilever based mass sensors using higher modes. Appl. Phys. Lett. 2005, 86, 233501. [Google Scholar] [CrossRef]
- Okajima, T.; Sekiguchi, H.; Arakawa, H.; Ikai, A. Self-oscillation technique for AFM in liquids. Appl. Surf. Sci. 2003, 210, 68–72. [Google Scholar] [CrossRef]
- Kacem, N.; Arcamone, J.; Perez-Murano, F.; Hentz, S. Dynamic range enhancement of nonlinear nanomechanical resonant cantilevers for highly sensitive NEMS gas/mass sensor applications. J. Micromech. Microeng. 2010, 20, 045023. [Google Scholar] [CrossRef]
- Sone, H.; Okano, H.; Hosaka, S. Picogram mass sensor using piezoresistive cantilever for biosensor. Jpn. J. Appl. Phys. 2004, 43, 4663. [Google Scholar] [CrossRef]
- Urasaki, S.; Yabuno, H.; Yamamoto, Y.; Matsumoto, S. Sensorless Self-Excited Vibrational Viscometer with Two Hopf Bifurcations Based on a Piezoelectric Device. Sensors 2021, 21, 1127. [Google Scholar] [CrossRef]
- Mouro, J.; Paoletti, P.; Sartore, M.; Vassalli, M.; Tiribilli, B. Photothermal Self-Excitation of a Phase-Controlled Microcantilever for Viscosity or Viscoelasticity Sensing. Sensors 2022, 22, 8421. [Google Scholar] [CrossRef]
- Zhou, K.; Urasaki, S.; Yabuno, H. Cantilever self-excited with a higher mode by a piezoelectric actuator. Nonlinear Dyn. 2021, 106, 295–307. [Google Scholar] [CrossRef]
- Nayfeh, A.H.; Mook, D.T. Nonlinear Oscillations; Wiley: Hoboken, NJ, USA, 1979. [Google Scholar]
- Bennett, J.A.; Eisley, J.G. A multiple degree-of-freedom approach to nonlinear beam vibrations. AIAA J. 1970, 8, 734–739. [Google Scholar] [CrossRef]
- Yabuno, H.; Kaneko, H.; Kuroda, M.; Kobayashi, T. Van der Pol type self-excited micro-cantilever probe of atomic force microscopy. Nonlinear Dyn. 2008, 54, 137–149. [Google Scholar] [CrossRef]
- Farokhi, H.; Ghayesh, M.H.; Hussain, S. Large-amplitude dynamical behaviour of microcantilevers. Int. J. Eng. Sci. 2016, 106, 29–41. [Google Scholar] [CrossRef]
- Urasaki, S.; Yabuno, H. Identification method for backbone curve of cantilever beam using van der Pol-type self-excited oscillation. Nonlinear Dyn. 2021, 103, 3429–3442. [Google Scholar] [CrossRef]
- Nakamura, T.; Yabuno, H.; Yano, M. Amplitude control of self-excited weakly coupled cantilevers for mass sensing using nonlinear velocity feedback control. Nonlinear Dyn. 2020, 99, 85–97. [Google Scholar] [CrossRef]
- Shen, F.; Lu, P.; O’Shea, S.; Lee, K.; Ng, T. Thermal effects on coated resonant microcantilevers. Sens. Actuators A Phys. 2001, 95, 17–23. [Google Scholar] [CrossRef]
- Mertens, J.; Finot, E.; Thundat, T.; Fabre, A.; Nadal, M.H.; Eyraud, V.; Bourillot, E. Effects of temperature and pressure on microcantilever resonance response. Ultramicroscopy 2003, 97, 119–126. [Google Scholar] [CrossRef] [PubMed]
- Alexander, C.; Sadiku, M. Fundamentals of Electric Circuits, 4th ed.; McGraw-Hill Education: New York, NY, USA, 2009. [Google Scholar]
- Hirsch, M.; Smale, S.; Devaney, R. Differential Equations, Dynamical Systems, and Linear Algebra; Pure and Applied Mathematics; Elsevier Science: Amsterdam, The Netherlands, 1974. [Google Scholar]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hyodo, Y.; Yabuno, H. Self-Excited Microcantilever with Higher Mode Using Band-Pass Filter. Sensors 2023, 23, 2849. https://doi.org/10.3390/s23052849
Hyodo Y, Yabuno H. Self-Excited Microcantilever with Higher Mode Using Band-Pass Filter. Sensors. 2023; 23(5):2849. https://doi.org/10.3390/s23052849
Chicago/Turabian StyleHyodo, Yuji, and Hiroshi Yabuno. 2023. "Self-Excited Microcantilever with Higher Mode Using Band-Pass Filter" Sensors 23, no. 5: 2849. https://doi.org/10.3390/s23052849
APA StyleHyodo, Y., & Yabuno, H. (2023). Self-Excited Microcantilever with Higher Mode Using Band-Pass Filter. Sensors, 23(5), 2849. https://doi.org/10.3390/s23052849





