Numerical Approaches for Recovering the Deformable Membrane Profile of Electrostatic Microdevices for Biomedical Applications
Abstract
1. Introduction
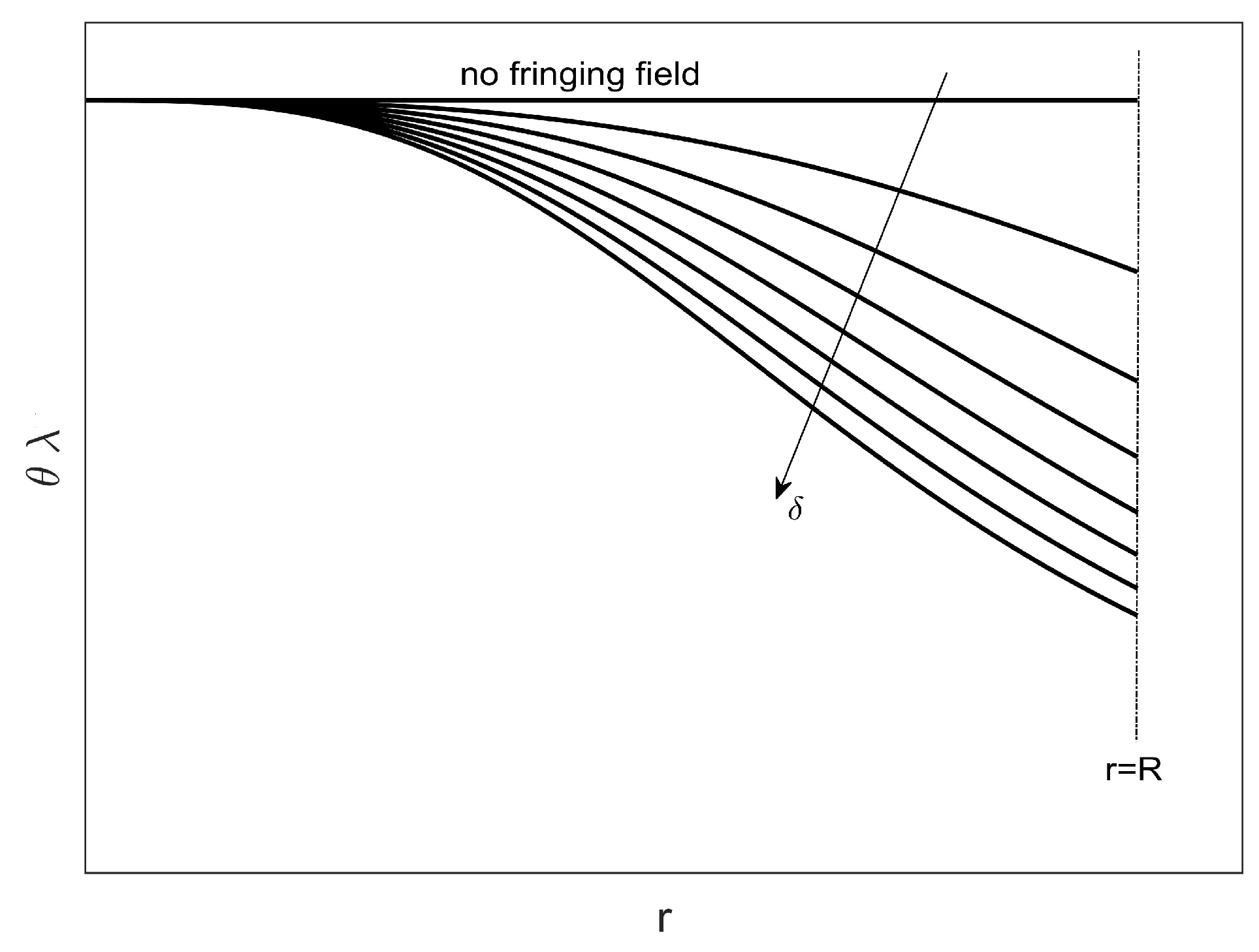
- As in [51], to take into account the effects due to the fringing field (usually dependent on the diameter/height ratio of the device that can increase the risk of electrostatic discharge being generated by possible contact of the membrane with the couter-electrode), a weighted addend has been considered in (1) according to the Pelesko–Driskoll theory [55], achieving a 2D second-order nonlinear differential model with singularity and circular symmetry.
- The proposed model, also obtained by considering that the straight line of the electric field, , on the membrane is locally proportional to the curvature of the membrane itself, models the behavior of the micropump as a function of the electromechanical and dielectric properties of the material constituting the membrane. Therefore, a positive bounded function, , , is introduced to simulate, according to the experimental results known in the literature, the dielectric properties of the membrane to accommodate the electrostatic capacitance changes that occur as the membrane deforms, while the electromechanical properties are formalized by the product that is already present in (1).
- The proposed model is formulated in order for known remarkable results to be exploited to prove the existence, uniqueness, and stability (in relation to V applied) of the solution by providing an algebraic condition depending on . If this is satisfied by the numerical solutions obtained, the profiles of the recovered membrane do not represent ghost solutions (i.e., numerical solutions that do not satisfy the conditions required for the existence, uniqueness, and stability of the analytical solution).
- Furthermore, after some calculations and considerations, an important limitation concerning the total electrostatic force (also considering the contribution due to the fringing field) in the micropump is achieved depending on both V and T, so as to connect this force with both the intended use of the device and the choice of material constituting the membrane.
- Specific numerical techniques considered the “gold standard” for these kinds of problems, characterized by different levels of convergence for showing efficiency and performance, were implemented in MatLab® R2022 running on Intel Core 2 CPU at 1.45 GHz to perform the numerical recovery of the membrane, providing results that are compatible with the existence and uniqueness conditions required for the solution. These results are all related to both V and T, obtaining a criterion that can be used for choosing a graphical approach on the Cartesian plane . Furthermore, the minimum value of V necessary to overcome the inertia of the membrane in the startup phase was quantified.
- Moreover, the pull-in voltage and the electrostatic pressure depending on both V and T were quantified so that these values are immediately attributable to the choice of membrane material and the intended use of the micropump.
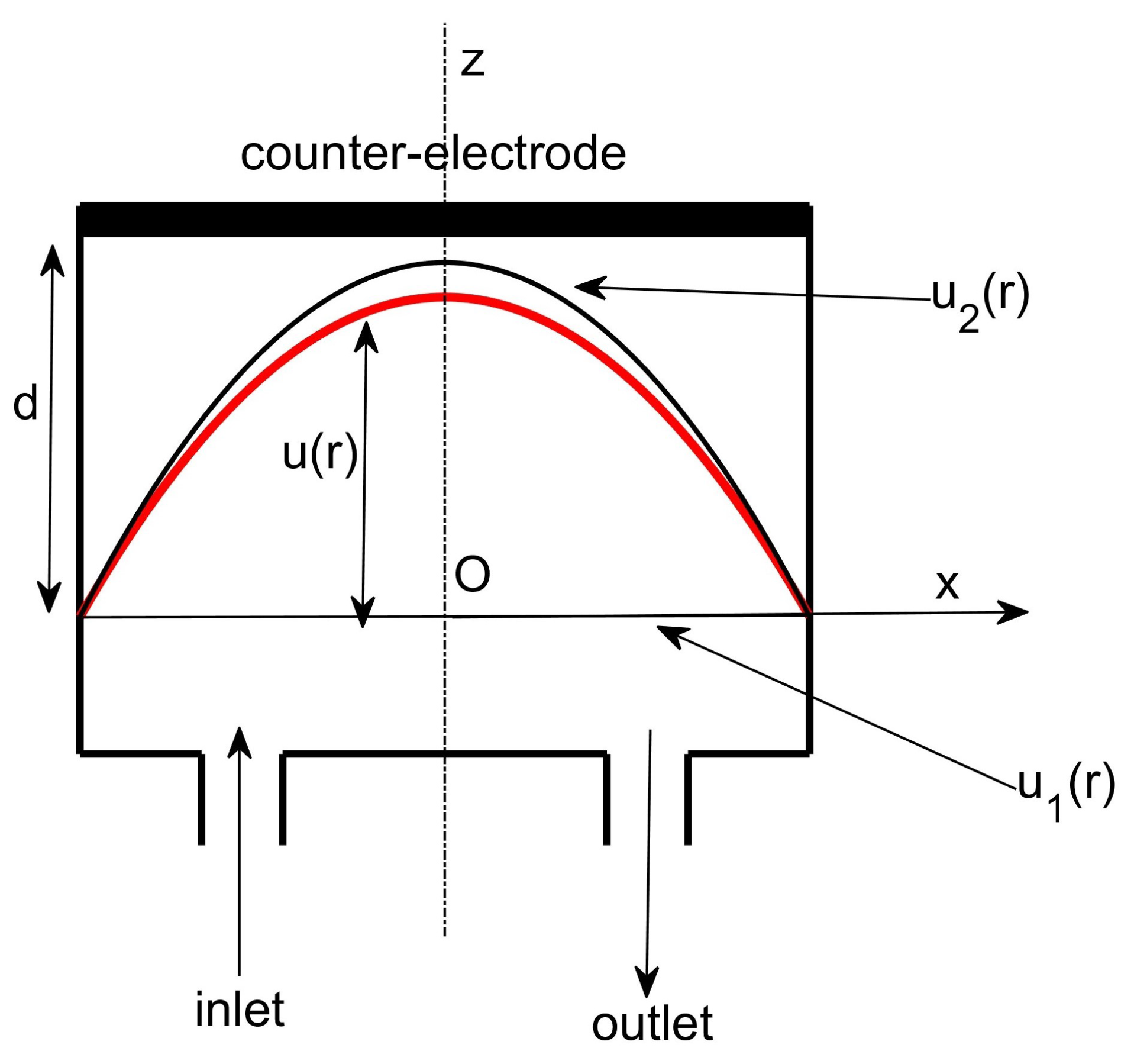
2. The Electrostatic Membrane Micropump
2.1. Electrostatic Membrane Micropump as an Actuator
2.2. Electrostatic Membrane Micropump as a Transducer
2.3. The Transducer as an Understanding Device of the Actuator
3. The Proposed Model
4. A Result Concerning the Existence of the Solution: An Approach Based on Upper and Lower Solutions
5. A Result Concerning the Uniqueness of the Solution
6. On the Existence and Uniqueness of the Solution
7. On the Stability of the Solutions
8. How to Mathematically Model the Dielectrical Properties of the Membrane
9. Numerical Recovery of the Membrane Profile
10. External Voltage Needed to Overcome the Mechanical Inertia of the Membrane
11. On the Selection of the Material Constituting the Membrane and the Intended Use of the Micropump
12. Some Considerations for Both the Pull-In Voltage and the Electrostatic Pressure
13. Conclusions and Perspectives
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
References
- Zengerle, R.; Richter, A.; Sandmaier, H. A Micro Membrane Pump with Electrostatic Actuation. In Proceedings of the IEEE Micro Electro Mechanical Systems, Travemunde, Germany, 4–7 February 1992; pp. 19–24. [Google Scholar]
- Nguyen, N.T.; Huang, X.; Chuan, T.K. MEMS-Micropumps: A Review. J. Fluid Eng. 2002, 124, 384–392. [Google Scholar] [CrossRef]
- Mizuno, H.L.; Anraku, Y.; Sakuma, I.; Akagi, Y. Effect of PEGylation on the Drug Release Performance and Hemocompatibility of Photoresponsive Drug-Loading Platform. Int. J. Mol. Sci. 2022, 23, 6686. [Google Scholar] [CrossRef] [PubMed]
- Prakash, P.; Lee, W.-H.; Loo, C.-Y.; Wong, H.S.J.; Parumasivam, T. Advances in Polyhydroxyalkanoate Nanocarriers for Effective Drug Delivery: An Overview and Challenges. Nanomaterials 2022, 12, 175. [Google Scholar] [CrossRef]
- Lee, S.H.; Bajracharya, R.; Min, J.Y.; Han, J.-W.; Park, B.J.; Han, H.-K. Strategic Approaches for Colon Targeted Drug Delivery: An Overview of Recent Advancements. Pharmaceutics 2020, 12, 68. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Zeng, L.; Song, W.; Liu, J. Influencing Factors and Drug Application of Iontophoresis in Transdermal Drug Delivery: An Overview of Recent Progress. Drug Deliv. Transl. Res. 2022, 12, 15–26. [Google Scholar] [CrossRef] [PubMed]
- Yunas, J.; Johari, J.; Hamzah, A.; Gebeshuber, I.C.; Majlis, B.Y. Design and Fabrication of MEMS Micropumps using Double Sided Etching. J. Microelectron. Electron. Packag. 2010, 7, 44–47. [Google Scholar] [CrossRef]
- Jang, J.; Lee, S.S. Theoretical and Experimental Study of MHD Magnetohydrodynamic) Micropump. Sens. Actuators A Phys. 2000, 80, 84–89. [Google Scholar] [CrossRef]
- Nisar, A.; Afzulpurkar, N.; Mahaisavariya, B.; Tuantranont, A. MEMS-Based Micropumps in Drug Delivery and Biomedical Applications. Sens. Actuators B Chem. 2008, 130, 917–942. [Google Scholar] [CrossRef]
- Ranjit, B.; Datta, S.; Chowdhury, A.R.; Datta, P. Advances in MEMS and Micro-Scale Technologies for Application in Controlled Drug-Dosing Systems: MEMS-Based Drug Delivery Systems. In Design and Development of Affordable Healthcare Technologies; IGI Global: Hershey, PA, USA, 2018; pp. 165–179. [Google Scholar]
- Barua, R.; Datta, S.; Sengupta, P.; Chowdhury, A.R. Chapter 14—Advances in MEMS micropumps and their emerging drug delivery and biomedical applications. In Advances and Challenges in Pharmaceutical Technology; Nayak, A.K., Pal, K., Banerjee, I., Maji, S., Nanda, U., Eds.; Academic Press: Cambridge, MA, USA, 2021; pp. 451–452. [Google Scholar]
- Ali, M.Y.; Kuang, C.; Khan, J.; Wang, G. A dynamic piezoelectric micropumping phenomenon. Microfluid. Nanofluid 2010, 9, 385–396. [Google Scholar]
- Lil, L.; Zhu, R.; Zhou, Z.; Ren, J. Modeling of a Micropump Membrane with Electrostatic Actuator. In Proceedings of the 2nd International Conference on Advanced Computer Control (ICACC), Shenyang, China, 27–29 March 2010. [Google Scholar]
- Chia, B.T.; Liao, H.; Yang, Y. A novel thermo-pneumatic peristaltic micropump with low temperature elevation on working fluid. Sens. Actuators A 2010, 165, 86–93. [Google Scholar] [CrossRef]
- Gong, Q.; Zhou, Z.; Yang, Y.; Wang, X. Design, optimization and simulation on microelectromagnetic pump. Sens. Actuators A 2000, 83, 200–207. [Google Scholar] [CrossRef]
- Yang, Y.; Zhaoying, Z.; Xiongying, Y.; Xiaoning, J. Bimetallic Thermally Actuated Micropump. In Proceedings of the International Mechnical Engineering Congress and Exposition (ASME 1996), Atlanta, GA, USA, 17–22 November 1996. [Google Scholar]
- Fang, T.; Tan, X. A novel diaphragm micropump actuated by conjugated polymer petals: Fabrication, modeling, and experimental results. Sens. Actuators A 2010, 158, 121–131. [Google Scholar] [CrossRef]
- Sim, W.Y.; Lee, S.W.; Yang, S.S. The Fabrication and Test of a Phase-change Type Micropump. 2008. Available online: https://asmedigitalcollection.asme.org/IMECE/proceedings-abstract/IMECE2001/35555/479/1124473 (accessed on 4 January 2023).
- Setiawan, M.A. The performance evaluation of SMA Spring as actuator for gripping manipulation. J. Teknik Elektro 2007, 7, 110–120. [Google Scholar] [CrossRef]
- Borowsky, J.; Lu, Q.; Collins, G.E. High pressure electroosmotic pump based on a packed bed planar microchip. Sens. Actuators B 2008, 131, 333–339. [Google Scholar] [CrossRef]
- Chang, J.; Choi, D.Y.; Han, S.; Pak, J.J. Driving characteristics of the electrowetting-on-dielectric device using atomic-layer-deposited aluminum oxide as the dielectric. Microfluid. Nanofluid 2010, 8, 269–273. [Google Scholar] [CrossRef]
- Kim, J.H.; Laua, K.T.; Shepherd, R.; Wu, Y.; Wallace, G.; Diamond, D. Performance characteristics of a polypyrrole modified polydimethylsiloxane (PDMS) membrane based microfluidic pump. Sens. Actuators A 2008, 148, 239–244. [Google Scholar] [CrossRef]
- Namasivayam, V.; Larson, R.G.; Burke, D.T.; Burns, M.A. Transpirationbased micropump for delivering continuous ultra low flow rates. J. Micromech. Microeng. 2003, 13, 261–271. [Google Scholar] [CrossRef]
- Chan, S.C.; Chen, C.R.; Liu, C.H. A bubble-activated micropump with high-frequency flow reversal. Sens. Actuators A 2010, 163, 501–509. [Google Scholar] [CrossRef]
- Singh, R.; Bhethanabotla, V.R. Enhancement in Ultrasonic Micro-Transport Using Focused Inter-Digital Transducers in a Surface Acoustic Wave Device: Fluid-Structure Interaction Study. In Proceedings of the IEEE Sensors, Christchurch, New Zealand, 25–28 October 2009. [Google Scholar]
- Versaci, M.; Morabito, F.C.; Angiulli, G. Adaptive Image Contrast Enhancement by Computing Distances into a 4-Dimensional Fuzzy Unit Hypercube. IEEE Access 2017, 5, 26922–26931. [Google Scholar] [CrossRef]
- Chircov, C.; Grumezescu, A.M. Microelectromechanical Systems (MEMS) for Biomedical Applications. Micromachines 2022, 13, 164. [Google Scholar] [CrossRef]
- Cicha, I.; Priefer, R.; Severino, P.; Souto, E.B.; Jain, S. Biosensor-Integrated Drug Delivery Systems as New Materials for Biomedical Applications. Biomolecules 2022, 12, 1198. [Google Scholar] [CrossRef] [PubMed]
- Dahlan, N.A.; Thiha, A.; Ibrahim, F.; Milić, L.; Muniandy, S.; Jamaluddin, N.F.; Petrović, B.; Kojić, S.; Stojanović, G.M. Role of Nanomaterials in the Fabrication of bioNEMS/MEMS for Biomedical Applications and towards Pioneering Food Waste Utilisation. Nanomaterials 2022, 12, 4025. [Google Scholar] [CrossRef] [PubMed]
- Voskerician, G.; Shive, M.S.; Shawgo, R.S.; von Recum, H.; Anderson, J.M.; Cima, M.J.; Langer, R. Biocompatibility and Biofouling of MEMS Drug Delivery Devices. Biomaterials 2022, 24, 1959–1967. [Google Scholar] [CrossRef] [PubMed]
- Vante, A.B.; Kanish, T.C. Experimental Investigation on Sigital Offset Awitching Atrategy for Precise Sosing Using Sigital Multiple Micropump Infusion Aystem. Microfluid. Nanofluidics 2022, 26, 92. [Google Scholar] [CrossRef]
- Doh, I.; Sim, D.; Kim, S.S. Microfluidic Thermal Flowmeters for Drug Injection Monitoring. Sensors 2022, 22, 3151. [Google Scholar] [CrossRef]
- Kulkarni, D.; Damiri, F.; Rojekar, S.; Zehravi, M.; Ramproshad, S.; Dhoke, D.; Musale, S.; Mulani, A.A.; Modak, P.; Paradhi, R.; et al. Recent Advancements in Microneedle Technology for Multifaceted Biomedical Applications. Pharmaceutics 2022, 14, 1097. [Google Scholar] [CrossRef]
- Wang, J.; Zeng, J.; Liu, Z.; Zhou, Q.; Wang, X.; Zhao, F.; Zhang, Y.; Wang, J.; Liu, M.; Du, R. Promising Strategies for Transdermal Delivery of Arthritis Drugs: Microneedle Systems. Pharmaceutics 2022, 14, 1736. [Google Scholar] [CrossRef]
- Tang, Y.S.; Tsai, Y.C.; Chen, T.W.; Li, S.Y. Artificial Kidney Engineering: The Development of Dialysis Membranes for Blood Purification. Membranes 2022, 12, 177. [Google Scholar] [CrossRef]
- Carvalho, A.; Kulyk, B.; Fernandes, A.J.S.; Fortunato, E.; Costa, F.M. A Review on the Applications of Graphene in Mechanical Transduction. Adv. Mater. 2021, 34, 2101326. [Google Scholar] [CrossRef]
- Fent, J.; Neuzil, J.; Manz, A.; Iliescu, C.; Neuzil, P. Microfluidic Trends in Drug Screening and Drug Delivery. TrAC Trends Anal. Chem. 2023, 158, 116821. [Google Scholar] [CrossRef]
- Dolete, G.; Chircov, C.; Motelica, L.; Ficai, D.; Oprea, O.-C.; Gheorghe, M.; Ficai, A.; Andronescu, E. Magneto-Mechanically Triggered Thick Films for Drug Delivery Micropumps. Nanomaterials 2022, 12, 3598. [Google Scholar] [CrossRef]
- Efe, I.; Dikmen, F.; Tuchkin, Y. On an electrostatic micropump with a rigorous mathematical model. Turk. J. Electr. Eng. Comput. Sci. 2021, 30, 805–817. [Google Scholar] [CrossRef]
- Liu, X.; Zhang, L.; Zhang, M. Studies on Pull-In Instability of an Electrostatic MEMS Actuator: Dynamical System Approach. J. Appl. Anal. Comput. 2022, 12, 850–861. [Google Scholar] [CrossRef]
- Lin, Z.; Li, X.; Jin, Z.; Qian, J. Fluid-Structure Interaction Analysis on Membrane Behavior of a Microfluidic Passive Valve. Membranes 2020, 10, 300. [Google Scholar] [CrossRef]
- Pal, M.; Lalengkima, C.; Maity, R.; Baishya, S.; Maity, N.P. Effects of Fringing Capacitances and Electrode’s Finiteness in Improved SiC Membrane Based Micromachined Ultrasonic Transducers. Microsyst. Technol. 2021, 27, 3679–3691. [Google Scholar] [CrossRef]
- Kottapalli, A.G.; Asadnia, M.; Miao, J.M.; Barbastathis, G.; Triantafyllou, M.S. A Flexible Liquid Crystal Polymer MEMS Pressure Sensor Array for Fish-Like Underwater Sensing. Smart Mater. Struct. 2012, 21, 281–299. [Google Scholar] [CrossRef]
- Chiou, C.-H.; Yeh, T.-Y.; Lin, J.-L. Deformation Analysis of a Pneumatically-Activated Polydimethylsiloxane (PDMS) Membrane and Potential Micro-Pump Applications. Micromachines 2015, 6, 216–229. [Google Scholar] [CrossRef]
- Zhao, L.; Zhao, Y.; Li, R.; Wu, D.; Xu, R.; Li, S.; Zhang, Y.; Ye, H.; Xin, Q. A Porphyrin-Based Optical Sensor Membrane Prepared by Electrostatic Self-Assembled Technique for Online Detection of Cadmium(II). Chemosphere 2020, 238, 124552. [Google Scholar] [CrossRef]
- Haque, M.A.; Saif, T.A. Investigation of Micro-Scale Materials Behavior with MEMS. ASME Int. Mech. Eng. Congr. Expo. 1999, 6, 283–288. [Google Scholar]
- Haque, M.A.; Saif, M.T.A. A review of MEMS-based microscale and nanoscale tensile and bending testing. Exp. Mech. 2003, 43, 248–255. [Google Scholar] [CrossRef]
- Di Barba, P.; Fattorusso, L.; Versaci, M. A 2D Membrane MEMS Device Model with Fringing Field: Curvature-Dependent Electrostatic Field and Optimal Control. Mathematics 2021, 9, 465. [Google Scholar] [CrossRef]
- Di Barba, P.; Fattorusso, L.; Versaci, M. A 2D Non-Linear Second-Order Differential Model for Electrostatic Circular Membrane MEMS Devices: A Result of Existence and Uniqueness. Mathematics 2019, 7, 1193. [Google Scholar] [CrossRef]
- Versaci, M.; Mammone, N.; Ieracitano, C.; Morabito, F.C. Micropumps for Drug Delivery Systems: A New Semi-Linear Elliptic Boundary-Value Problem. Comput. Appl. Math. 2021, 40, 60. [Google Scholar] [CrossRef]
- Versaci, M.; Jannelli, A.; Morabito, F.C.; Angiulli, G. A Semi-Linear Elliptic Model for a Circular Membrane MEMS Device Considering the Effect of the Fringing Field. Sensors 2021, 21, 5237. [Google Scholar] [CrossRef] [PubMed]
- Versaci, M.; Fattorusso, L.; Jannelli, A.; Di Barba, P. Finite Differences for Recovering the Plate Profile in Electrostatic MEMS with Fringing Field. Electronics 2022, 11, 3010. [Google Scholar] [CrossRef]
- Pelesko, J.A.; Bernstein, D.H. Modeling MEMS and NEMS; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Soleimani, M.; Friedrich, M. E-Skin Using Fringing Field Electrical Impedance Tomography with an Ionic Liquid Domain. Sensors 2022, 22, 5040. [Google Scholar] [CrossRef]
- Siddique, I.; Deaton, R.; Sabo, E.; Pelesko, J.A. An Experimental Investigation of the Theory of Electrostatic Deflections. J. Electrost. 2011, 69, 1–6. [Google Scholar] [CrossRef]
- Pelesko, J.A.; Triolo, A.A. Nonlocal Problems in MEMS Device Control. J. Eng. Math. 2001, 41, 345–366. [Google Scholar] [CrossRef]
- Chuang, W.C.; Lee, H.L.; Chang, P.Z.; Hu, Y.C. Review on the Modeling of Electrostatic MEMS. Sensors 2010, 10, 6149–6171. [Google Scholar] [CrossRef]
- Guo, Y.; Pan, Z.; Ward, M.J. Touchdown and Pull-In Voltage Behavior of a MEMS Device with Varying Dielectric Properties. SIAM J. Appl. Math. 2005, 66, 309–338. [Google Scholar] [CrossRef]
- Pinar, Z. The Symmetry Analysis of Electrostatic Micro-Electromechanical Systems (MEMS). Mod. Phys. Lett. B 2005, 34, 2050199. [Google Scholar] [CrossRef]
- Aljadiri, R.T.; Taha, L.Y.; Ivey, P. Electrostatic Energy Harvesting Systems: A Better Understanding of Their Sustainability. J. Clean Energy Technol. 2017, 5, 409–416. [Google Scholar] [CrossRef]
- Pelesko, J.A. Mathematical Modeling of Electrostatic MEMS with Tailored Dielectric Properties. SIAM J. Appl. Math. 2002, 62, 888–908. [Google Scholar] [CrossRef]
- Zhang, L.X.; Zhao, Y.P. Electromechanical model of RF MEMS switches. Microsyst. Technol. 2003, 9, 420–426. [Google Scholar] [CrossRef]
- Sadeghian, H.; Rezazadeh, G.; Sterberg, P.M. Application of the Generalized Differential Quadrature Method to the Study of Pull-In Phenomena of MEMS Switches. J. Microelectromech. Syst. 2007, 16, 1334–1340. [Google Scholar] [CrossRef]
- Pallay, M.; Miles, R.N.; Towfighian, S. A Tunable Electrostatic MEMS Pressure Switch. IEEE Trans. Ind. Electron. 2019, 67, 9833–9840. [Google Scholar] [CrossRef]

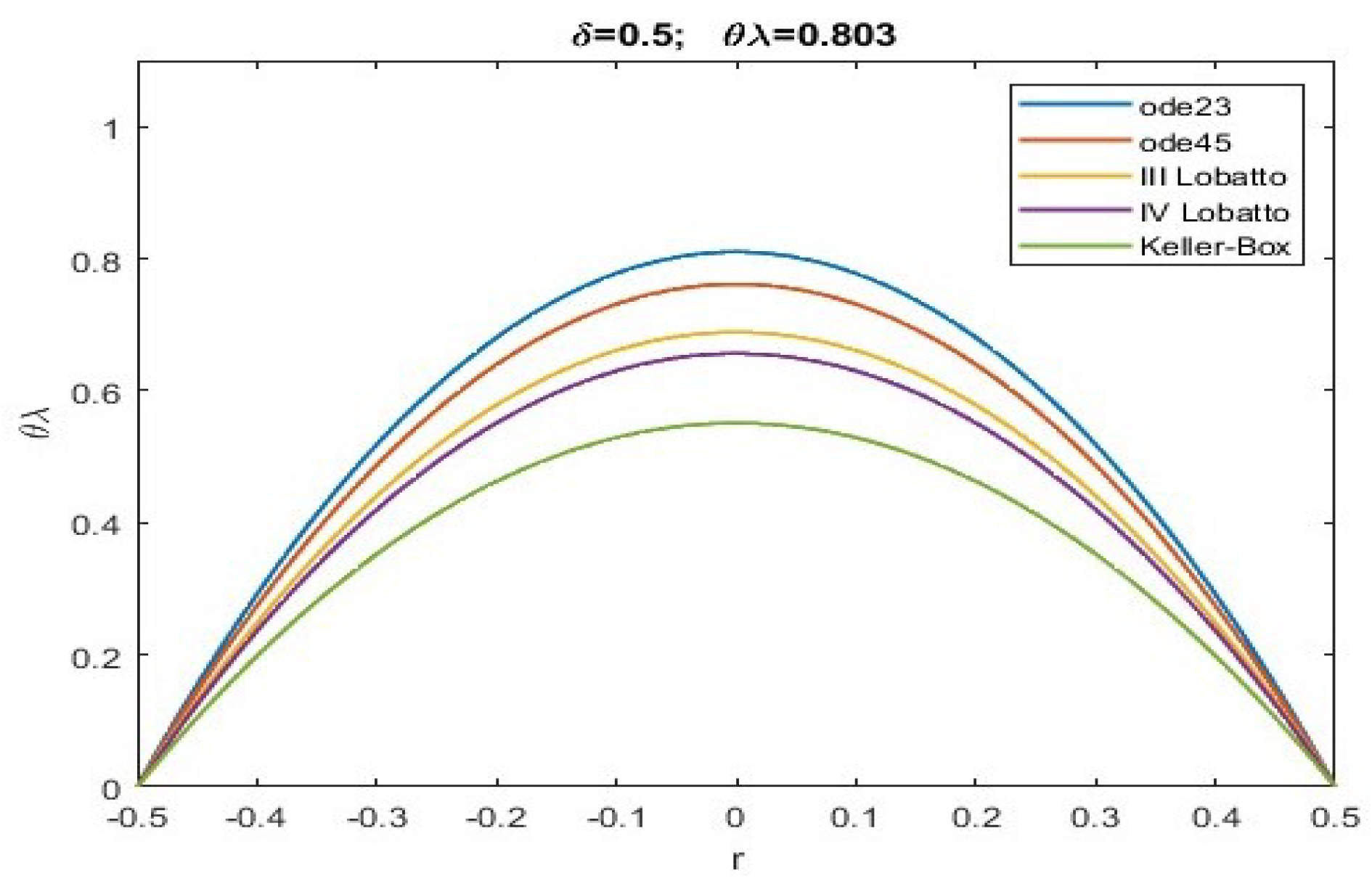
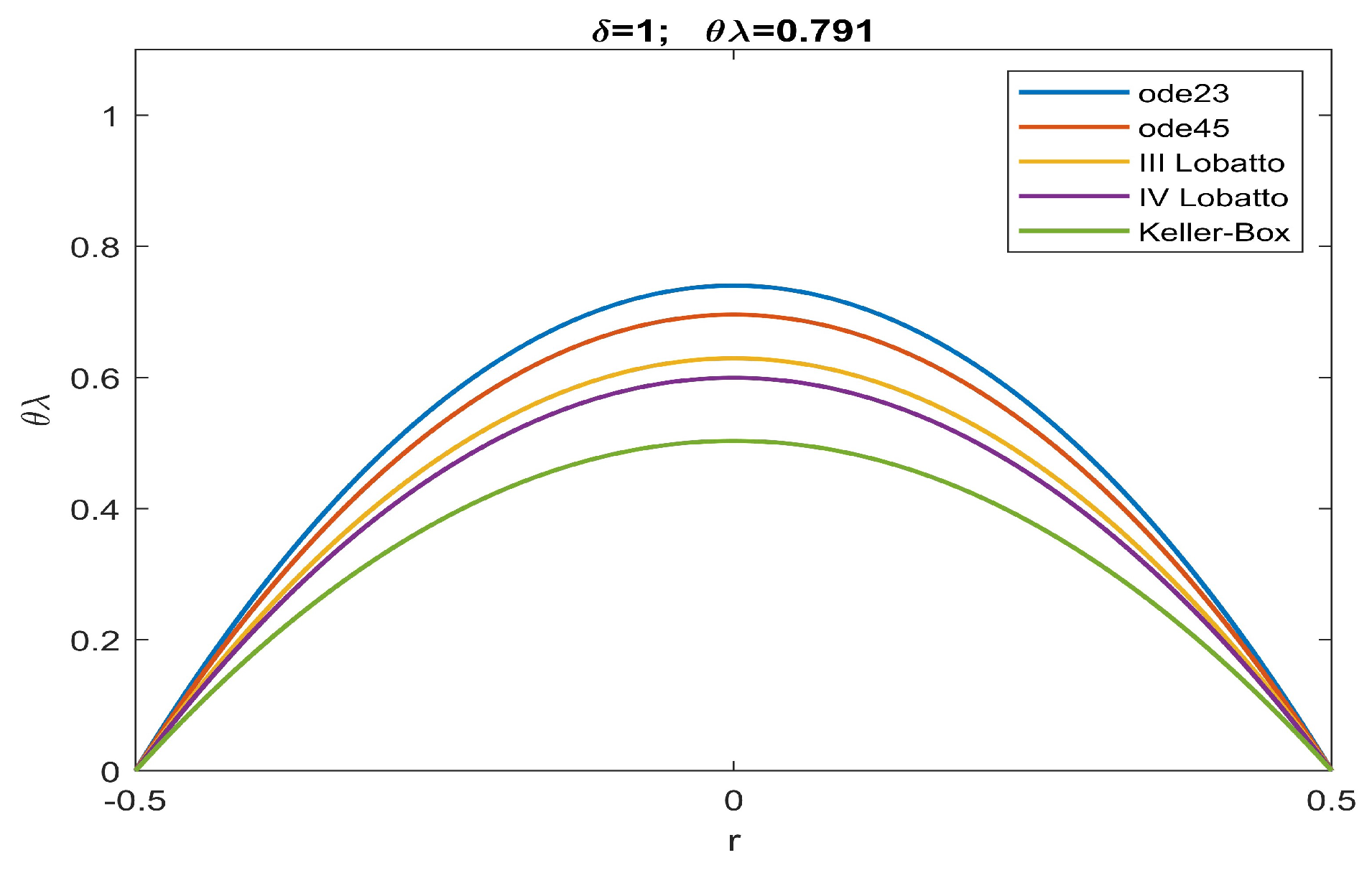

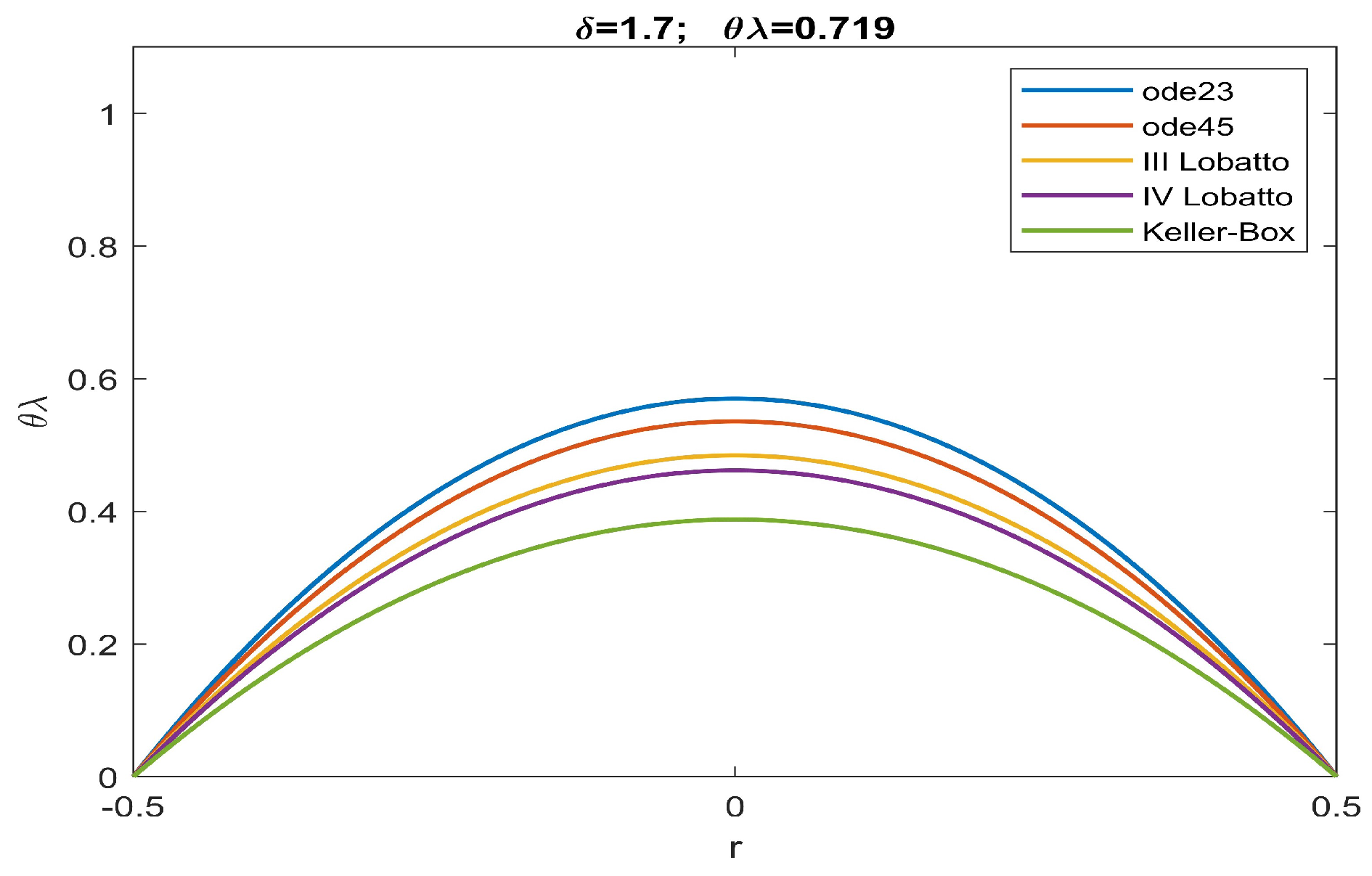
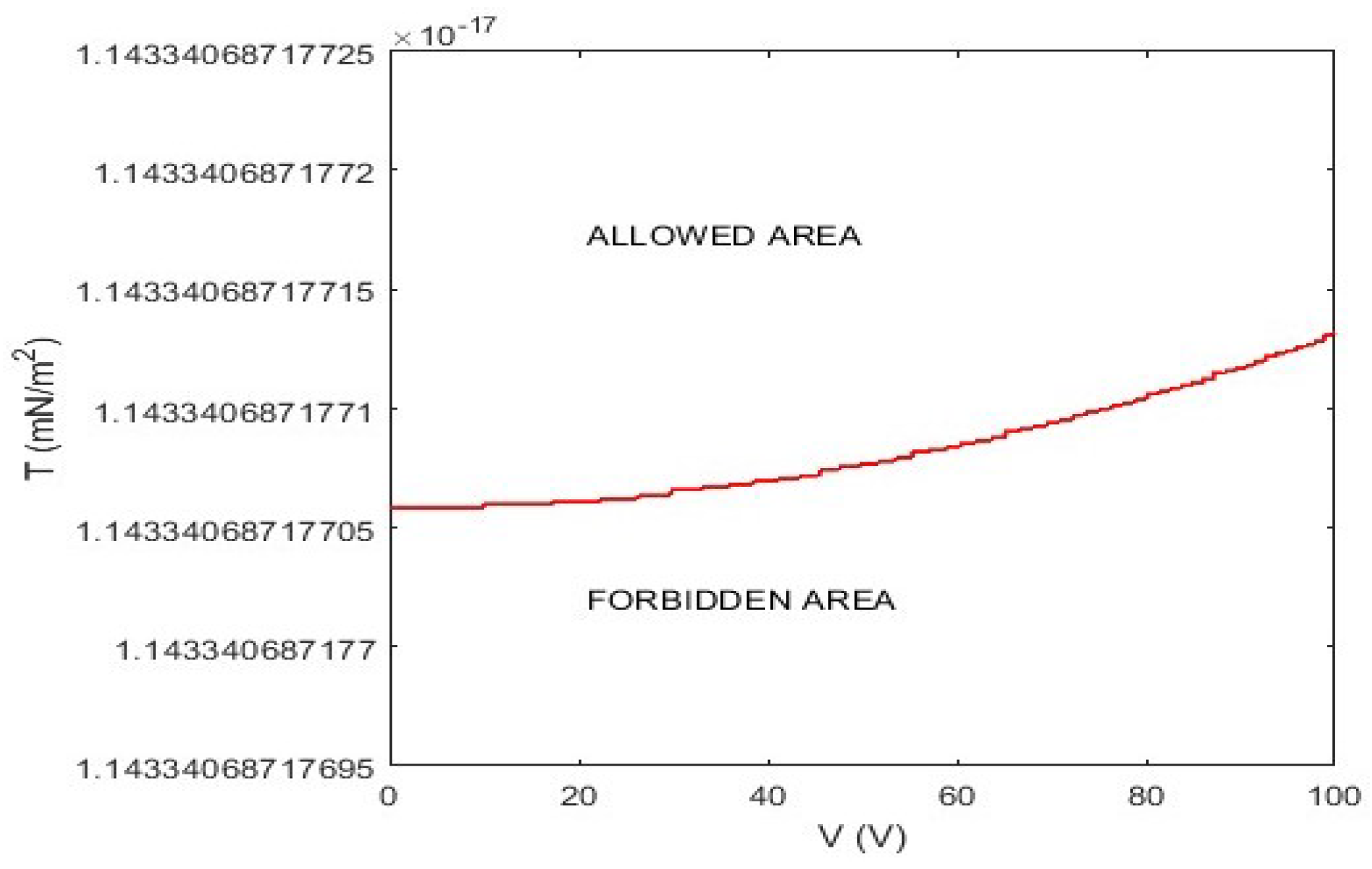

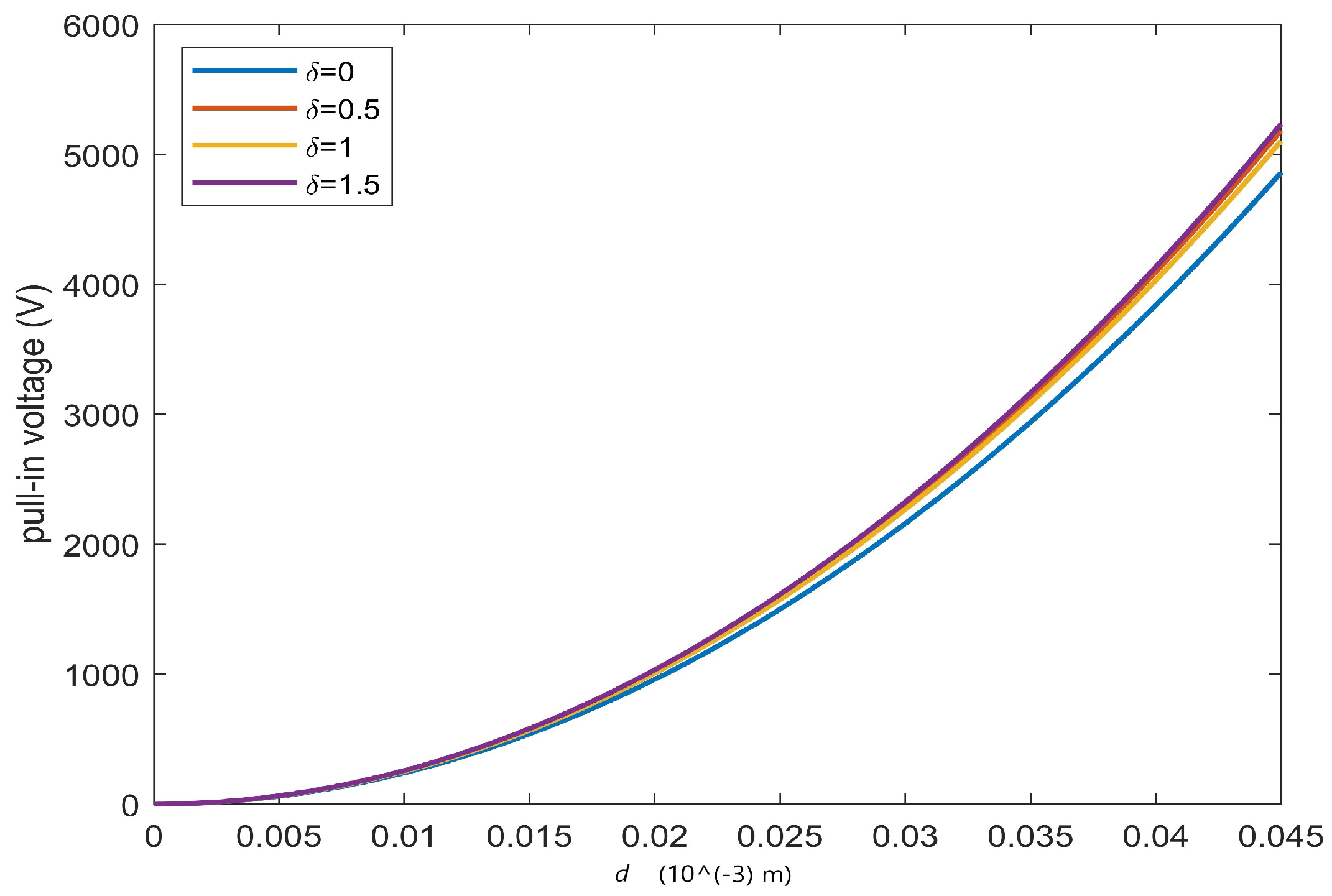

| ode23 | ode45 | Keller | bpv4c | bpv5c | (30) | ||
|---|---|---|---|---|---|---|---|
| Box | |||||||
| 0 | verified | ||||||
| 0.5 | verified | ||||||
| 1 | verified | ||||||
| 1.5 | verified | ||||||
| 1.7 | verified |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Versaci, M.; Morabito, F.C. Numerical Approaches for Recovering the Deformable Membrane Profile of Electrostatic Microdevices for Biomedical Applications. Sensors 2023, 23, 1688. https://doi.org/10.3390/s23031688
Versaci M, Morabito FC. Numerical Approaches for Recovering the Deformable Membrane Profile of Electrostatic Microdevices for Biomedical Applications. Sensors. 2023; 23(3):1688. https://doi.org/10.3390/s23031688
Chicago/Turabian StyleVersaci, Mario, and Francesco Carlo Morabito. 2023. "Numerical Approaches for Recovering the Deformable Membrane Profile of Electrostatic Microdevices for Biomedical Applications" Sensors 23, no. 3: 1688. https://doi.org/10.3390/s23031688
APA StyleVersaci, M., & Morabito, F. C. (2023). Numerical Approaches for Recovering the Deformable Membrane Profile of Electrostatic Microdevices for Biomedical Applications. Sensors, 23(3), 1688. https://doi.org/10.3390/s23031688








