Evaluation of the Relative Phase Comparison Method at Its Limits Used for Absolute Phase Determination of an AC Cage Current Shunt Group
Abstract
:1. Introduction
- Small capacitance for low current ranges;
- Small inductance for high current ranges;
- Coaxial structure;
- Good heat-conduction path from the resistive elements to the surface;
- Sufficient surface area for heat dissipation;
- An influence of the skin effect that is as small as possible;
- Small drift, i.e., good stability.
- The ratios of the nominal current;
- The resistance values of shunts used in the comparison.
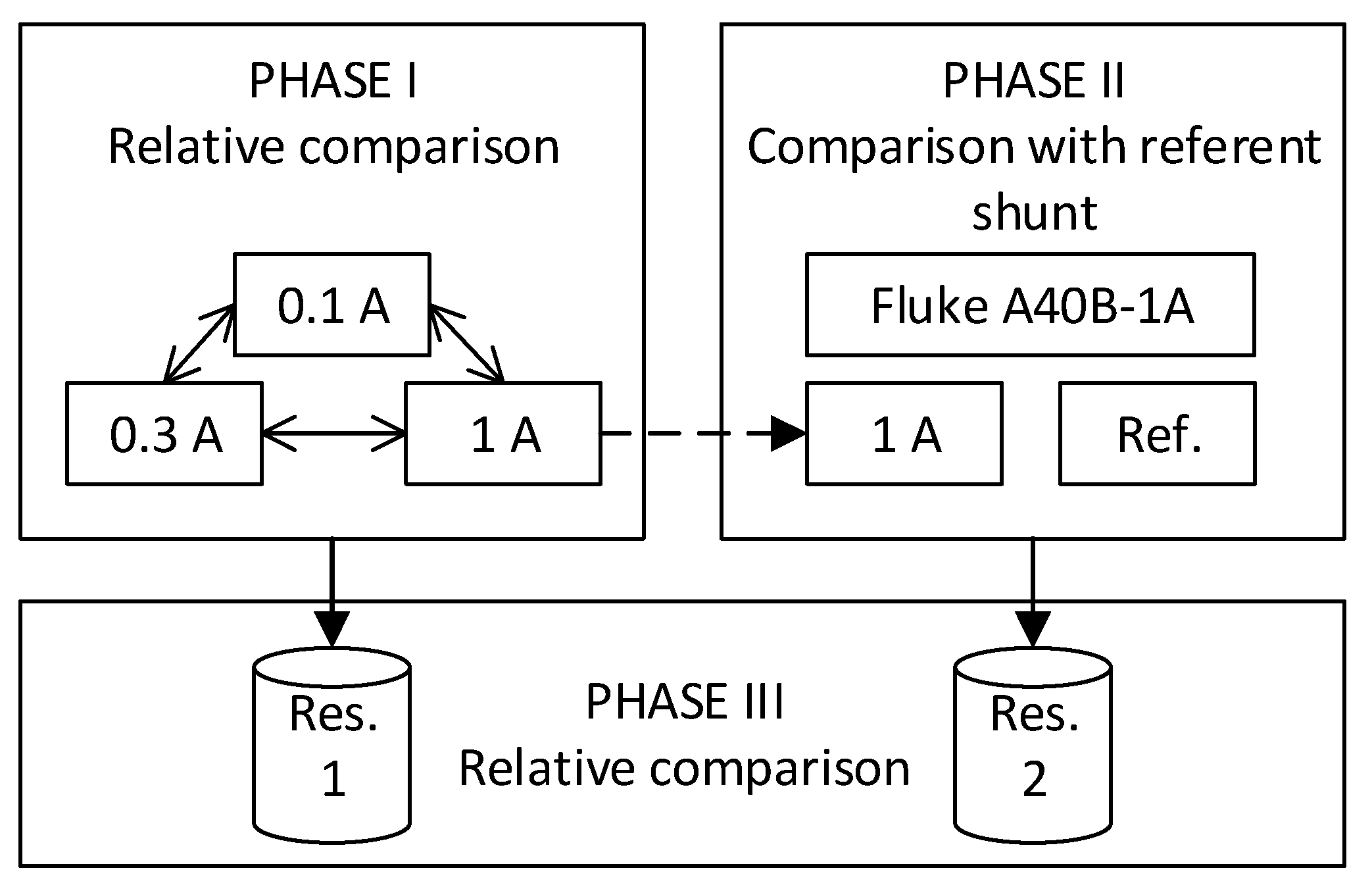
2. Procedure for Evaluation of the Relative Phase Comparison Method
3. Measurement Setup and Method
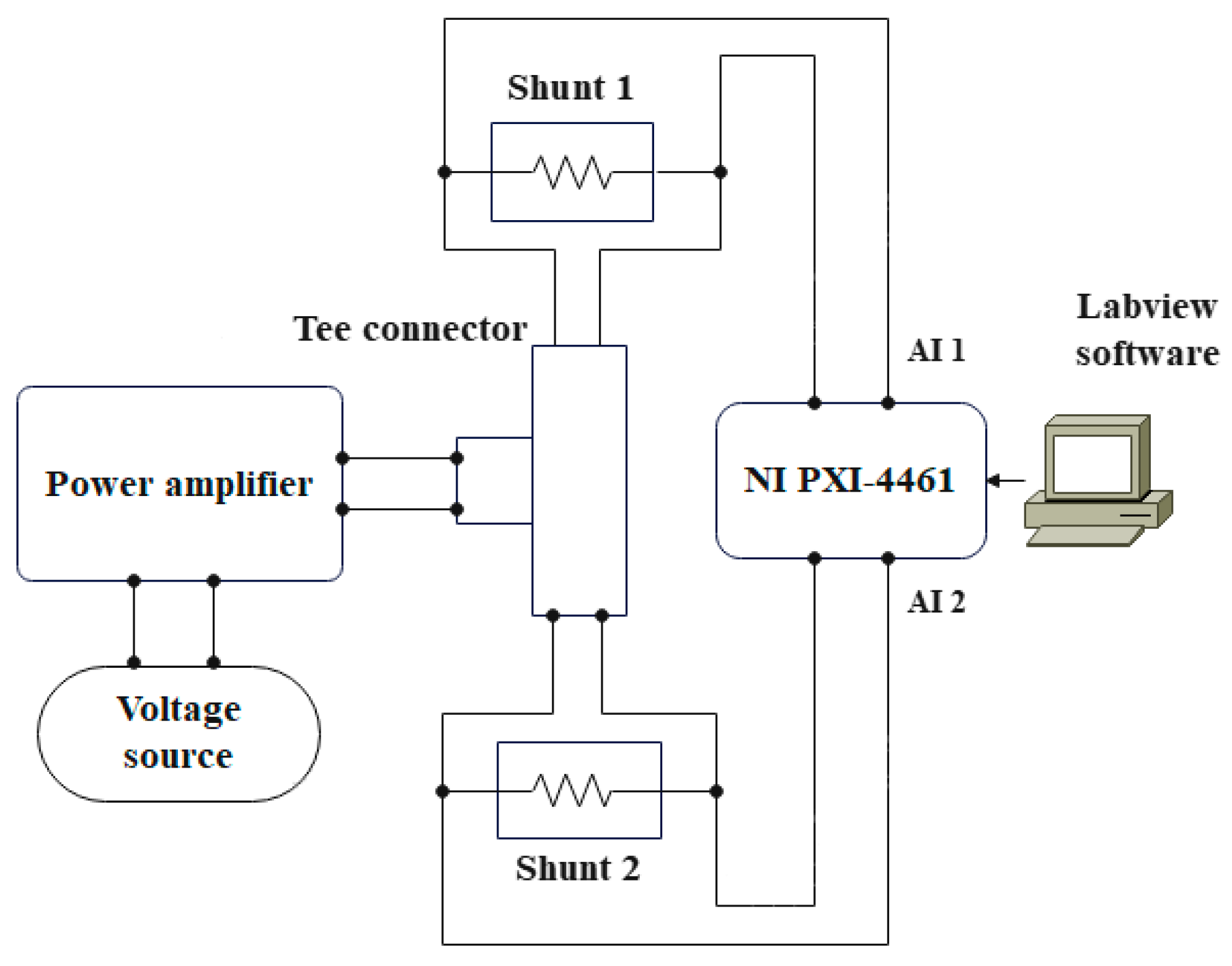
3.1. Measurement Setup
3.2. Measurement Method for Phase Difference Determination
3.2.1. Introduction to the Measurement Method
3.2.2. Sampling Rate and Frequency Range
3.2.3. Repeatability and Influence of Data Length (Number of Periods)
3.2.4. Phase Analysis from Relative Phase Comparison Method
3.3. Comparison against Reference Shunt
3.4. Phase Measurement Error of the Measurement System
4. Measurement Results and Discussion
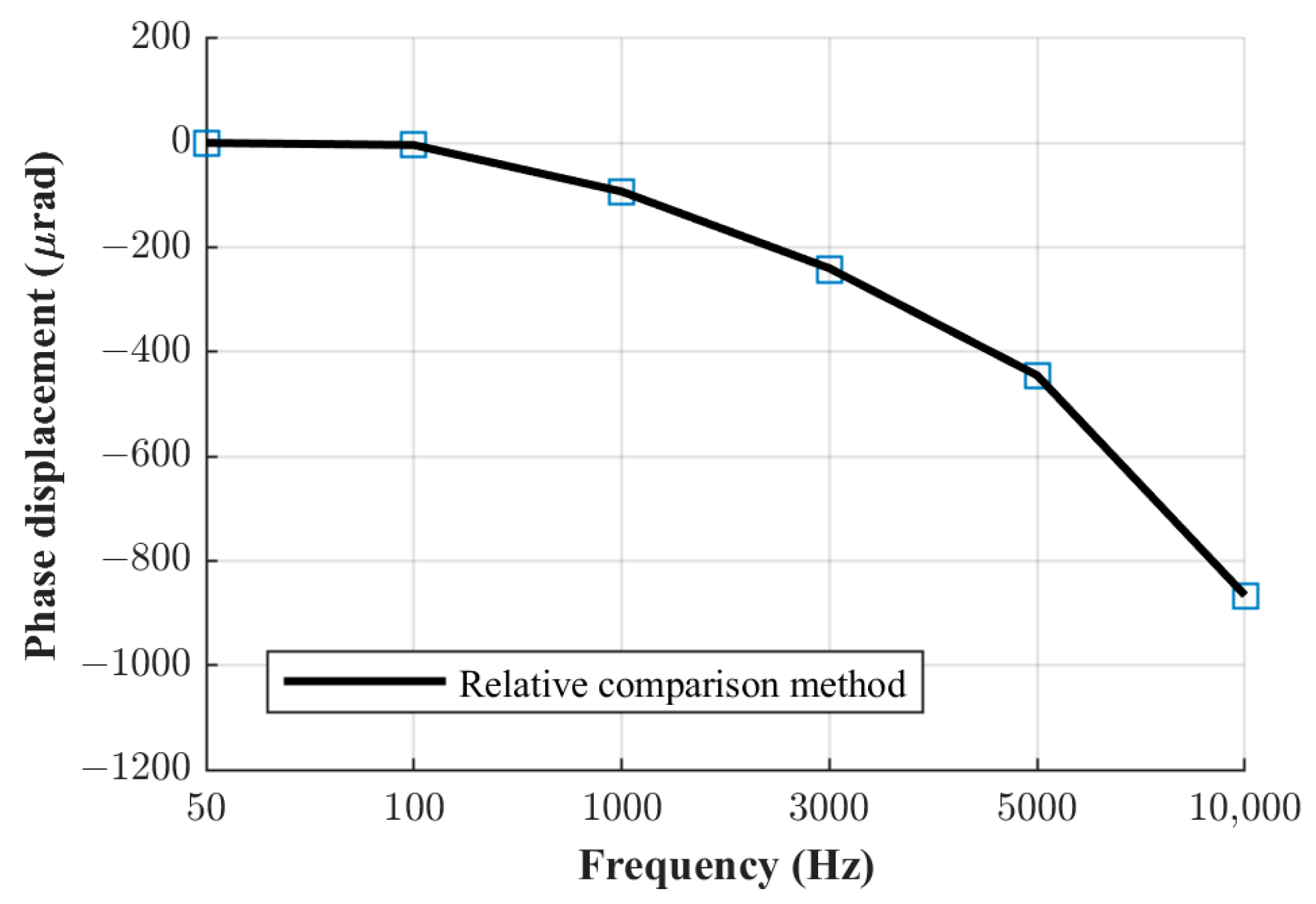
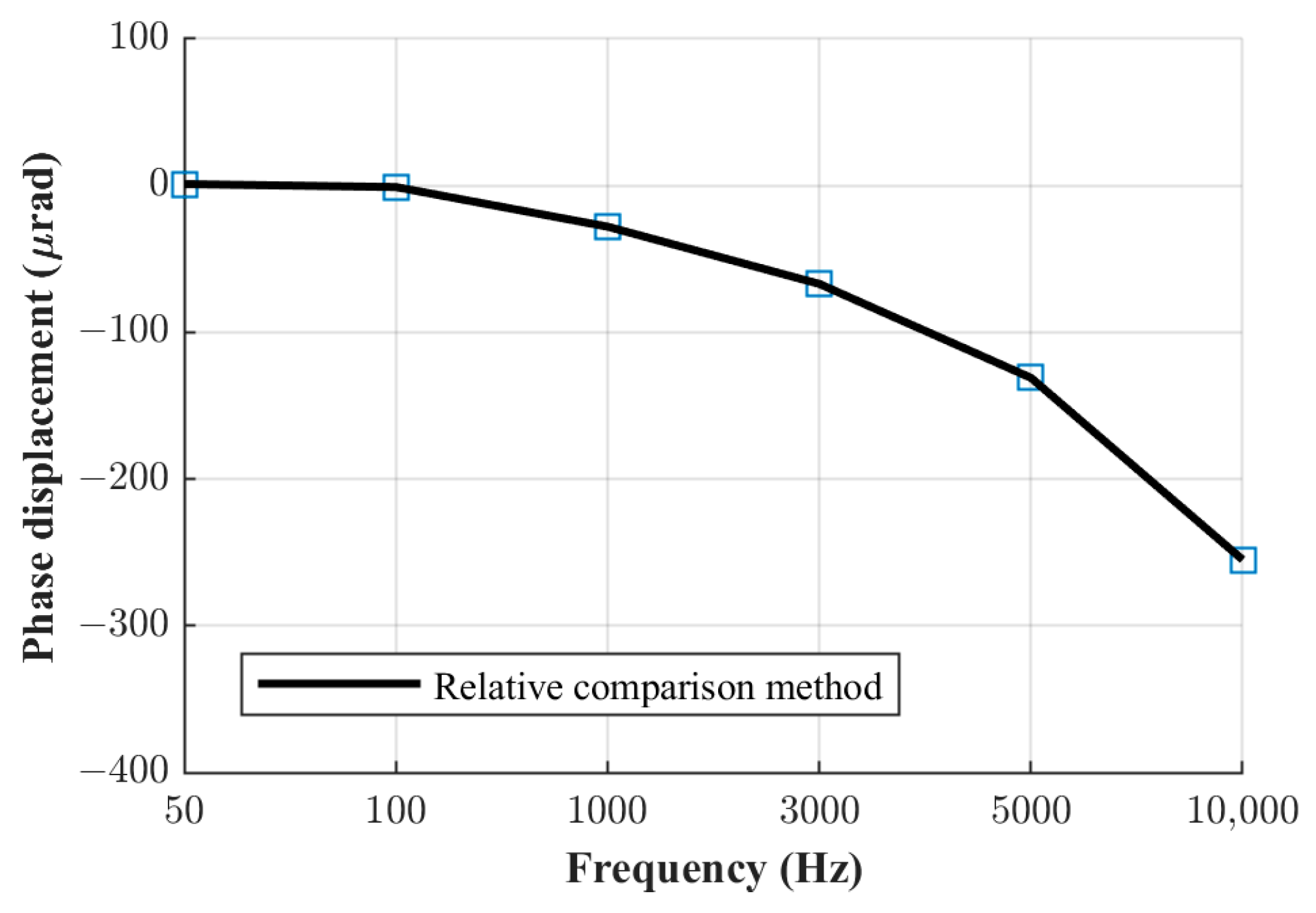
4.1. Relative Phase Comparison
4.2. Absolute Phase Verification with Reference Current Shunt
4.3. Results Comparison
5. Uncertainty Analysis
5.1. Relative Phase Comparison Method
5.2. Absolute Phase Verification Method
6. Conclusions and Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zachovalová, V.N.; Šíra, M.; Streit, J. Development of the Digital Sampling Power Measuring Standard for the Energy Monitors Calibration. In Proceedings of the 2012 Conference on Precision Electromagnetic Measurements, Washington, DC, USA, 1–6 July 2012; pp. 298–299. [Google Scholar] [CrossRef]
- Svensson, S. A Wattmeter Standard for the Audio Frequency Range. IEEE Trans. Instrum. Meas. 1999, 48, 431–433. [Google Scholar] [CrossRef]
- Konjevod, J.; Mostarac, P.; Dadić, M.; Malarić, R. Analysis and Development of Digital Sampling Wattmeter Components for Precise Electric Power Measurement. In Proceedings of the 2018 International Conference on Smart Systems and Technologies (SST), Osijek, Croatia, 10–12 October 2018; pp. 61–66. [Google Scholar] [CrossRef]
- Voljč, B.; Lindič, M.; Pinter, B.; Kokalj, M.; Svetik, Z.; Lapuh, R. Evaluation of a 100 A Current Shunt for the Direct Measurement of AC Current. IEEE Trans. Instrum. Meas. 2013, 62, 1675–1680. [Google Scholar] [CrossRef]
- Zachovalová, V.N.; Síra, M.; Bednár, P.; Maslán, S. New Generation of Cage-Type Current Shunts Developed Using Model Analysis. ACTA Imeko 2015, 4, 59. [Google Scholar] [CrossRef]
- Lind, K.; Sørsdal, T.; Slinde, H. Design, Modeling, and Verification of High-Performance AC–DC Current Shunts From Inexpensive Components. IEEE Trans. Instrum. Meas. 2008, 57, 176–181. [Google Scholar] [CrossRef]
- Kyriazis, G.A.; de Souza, R.M.; Yasuda, E.S.; Di Lillo, L. Modeling the AC–DC Transfer Difference of Wideband Cage-Type Current Shunts. IEEE Trans. Instrum. Meas. 2020, 69, 4436–4444. [Google Scholar] [CrossRef]
- Kyriazis, G.; Souza, R.; Yasuda, E.; Di Lillo, L. Modeling Wideband Low-Current Shunts with a New Design. In Proceedings of the 2020 Conference on Precision Electromagnetic Measurements (CPEM), Denver, CO, USA, 24–28 August 2020; pp. 1–2. [Google Scholar] [CrossRef]
- Malinowski, M.; Kubiczek, K.; Kampik, M. A Precision Coaxial Current Shunt for Current AC-DC Transfer. Measurement 2021, 176, 109126. [Google Scholar] [CrossRef]
- Ouameur, M.; Ziadé, F.; Le Bihan, Y. Toward a Calculable Standard Shunt for Current Measurements at 10 A and Up To 1 MHz. IEEE Trans. Instrum. Meas. 2019, 68, 2215–2222. [Google Scholar] [CrossRef]
- Kon, S.; Yamada, T. Expansion of the Impedance and Frequency Measurement Ranges of AC Shunt Resistors. IEEE Trans. Instrum. Meas. 2017, 66, 1254–1259. [Google Scholar] [CrossRef]
- Bergsten, T.; Rydler, K.-E. Realisation of Absolute Phase and Ac Resistance of Current Shunts by Ratio Measurements. In Proceedings of the 2018 Conference on Precision Electromagnetic Measurements (CPEM 2018), Paris, France, 8–13 July 2018; pp. 1–2. [Google Scholar] [CrossRef]
- Rydler, K.E.; Bergsten, T.; Tarasso, V. Determination of Phase Angle Errors of Current Shunts for Wideband Power Measurement. In Proceedings of the 2012 Conference on Precision Electromagnetic Measurements, Washington, DC, USA, 1–6 July 2012; pp. 284–285. [Google Scholar] [CrossRef]
- Budovsky, I. Measurement of Phase Angle Errors of Precision Current Shunts in the Frequency Range From 40 Hz to 200 kHz. IEEE Trans. Instrum. Meas. 2007, 56, 284–288. [Google Scholar] [CrossRef]
- Zampilis, R.R.N.; Pinto, M.V.V.; de Souza, L.A.A.; Geronymo, G.M.; Landim, R.P. Method for Validation of Absolute Phase Angle Characterization in Wideband Current Shunts. IEEE Trans. Instrum. Meas. 2022, 71, 1–7. [Google Scholar] [CrossRef]
- Yang, Z.; Wu, J.; Shao, H.; Wang, J.; Wang, L. Research on a Novel Calibration Method of Broadband Shunt. In Proceedings of the 2022 IEEE International Instrumentation and Measurement Technology Conference (I2MTC), Ottawa, ON, Canada, 16–19 May 2022; pp. 1–6. [Google Scholar] [CrossRef]
- Chen, S.F.; Hsu, J.C. Phase Error Calibration of Current Shunts at Power Frequencies Using AC-Programmable Josephson Voltage Standard. In Proceedings of the 2018 Conference on Precision Electromagnetic Measurements (CPEM 2018), Paris, France, 8–13 July 2018; pp. 1–2. [Google Scholar] [CrossRef]
- Konjevod, J.; Malarić, R.; Dadić, M.; Mostarac, P.; Hegeduš, H. Measurement of DC Properties and Relative Humidity (RH) Dependence of Wideband AC Current Shunts. Measurement 2019, 131, 1–6. [Google Scholar] [CrossRef]
- National Instruments. NI PXIe-1062Q User Manual—National Instruments. April 2012. Available online: https://www.alberding.eu/pdf/MSK-Modulator-user-guide-EN.pdf (accessed on 15 August 2023).
- TOELLNER Electronic Instrumente GmbH. Technical Specifications TOE 7621. Available online: https://www.toellner.de/datenblaetter/TOE%207621_EN_2020.pdf (accessed on 15 August 2023).
- RIGOL Technologies Inc. Data Sheet, RIGOL DG4000 Series. Available online: https://www.rigol.si/DG4000_DataSheet_EN.pdf (accessed on 15 August 2023).
- Garcocz, M. Tee Connectors for Ac-Dc Current Shunts up to 100 A. In Proceedings of the CPEM 2010, Daejeon, Republic of Korea, 13–18 June 2010; pp. 591–592. [Google Scholar] [CrossRef]
- National Instruments. NI 446x Specifications. Available online: https://www.ni.com/docs/en-US/bundle/ni-4461-4462-specs/resource/373770k.pdf (accessed on 15 August 2023).
- Konjevod, J.; Malarić, R.; Jurčević, M.; Mostarac, P.; Dadić, M. Comparison of Digitizers for High-Precision Sampling Power Meters. IEEE Trans. Instrum. Meas. 2020, 69, 3719–3728. [Google Scholar] [CrossRef]
- Konjevod, J.; Malarić, R.; Mostarac, P.; Jurčević, M. The AC Amplitude and Channel Characterization of High-Resolution Digitizers. Measurement 2022, 195, 111144. [Google Scholar] [CrossRef]
- Zachovalová, V.N. On the Current Shunts Modeling. IEEE Trans. Instrum. Meas. 2014, 63, 1620–1627. [Google Scholar] [CrossRef]
- Svensson, S.; Rydler, K.E.; Tarasso, V. Improved Model and Phase-Angle Verification of Current Shunts for AC And Power Measurements. In Proceedings of the 2004 Conference on Precision Electromagnetic Measurements, London, UK, 27 June–2 July 2004; pp. 82–83. [Google Scholar] [CrossRef]



| Shunt Group | Nominal Resistance/Current Values | ||
|---|---|---|---|
| RISE [12] | Ω | Ω | Ω |
| INMETRO [15] | Ω | Ω | Ω |
| FER [18] | Ω | Ω | Ω |
| Frequency | Sampling Rate | Number of Samples |
|---|---|---|
| 50 Hz | 100 kSa/s | 20,000 |
| 100 Hz | 100 kSa/s | 10,000 |
| 1 kHz | 200 kSa/s | 2000 |
| 3 kHz | 201 kSa/s | 670 |
| 5 kHz | 200 kSa/s | 400 |
| 10 kHz | 200 kSa/s | 200 |
| Frequency | Phase Error |
|---|---|
| 50 Hz | −1 µrad |
| 100 Hz | 0 µrad |
| 1 kHz | −8 µrad |
| 3 kHz | 6 µrad |
| 5 kHz | −20 µrad |
| 10 kHz | −31 µrad |
| Frequency | Phase Displacement | Measurement Uncertainty |
|---|---|---|
| 50 Hz | 0.3 µrad | 0.7 µrad |
| 100 Hz | 0.8 µrad | 0.7 µrad |
| 1 kHz | 7.1 µrad | 0.7 µrad |
| 3 kHz | 21 µrad | 2 µrad |
| 5 kHz | 36 µrad | 4 µrad |
| 10 kHz | 71 µrad | 7 µrad |
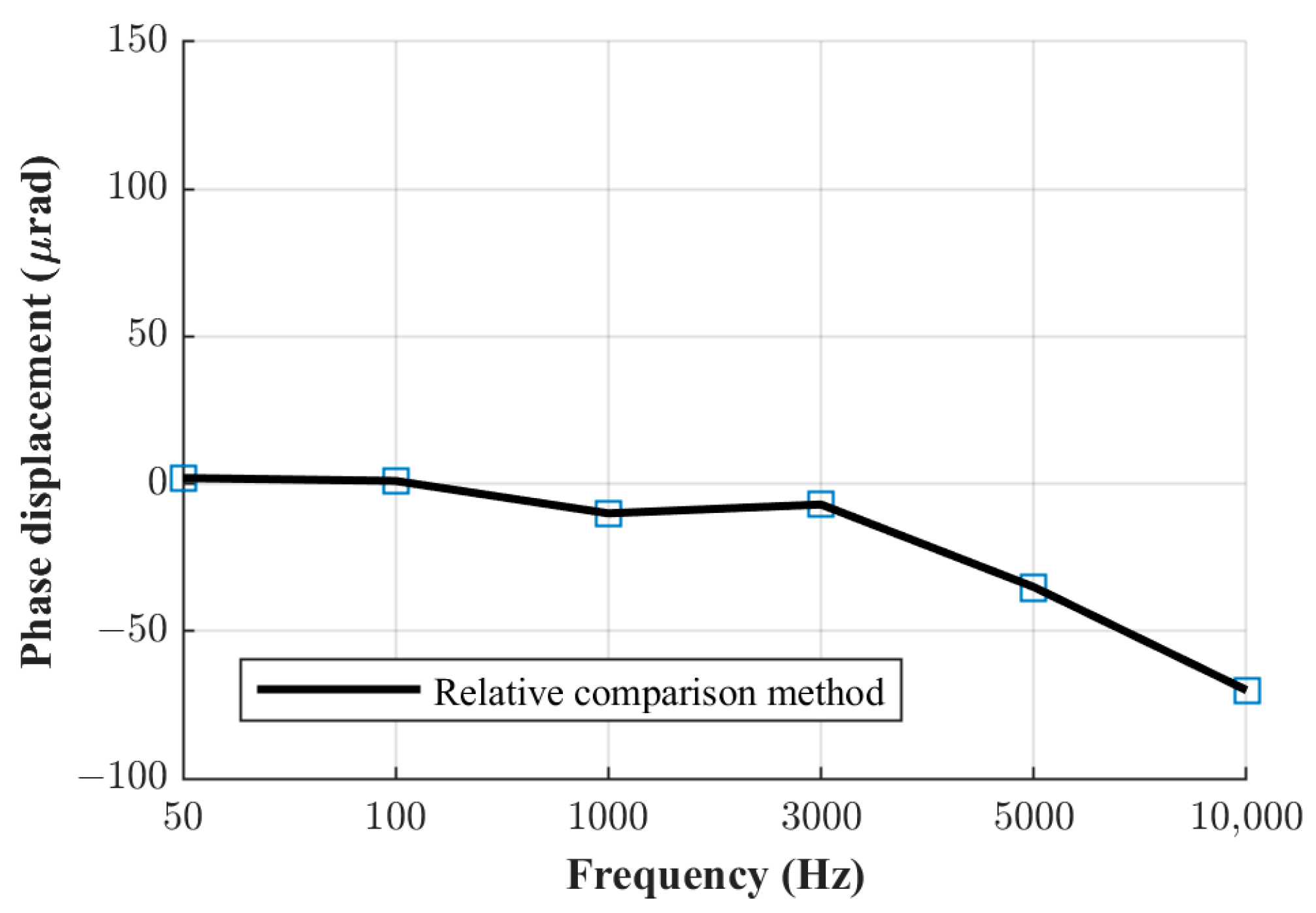
| Relative Comparison Method | |||||
|---|---|---|---|---|---|
| Frequency | |||||
| 50 Hz | 100 Hz | 1 kHz | 3 kHz | 5 kHz | 10 kHz |
| 2 µrad | 1 µrad | −10 µrad | −7 µrad | −35 µrad | −70 µrad |
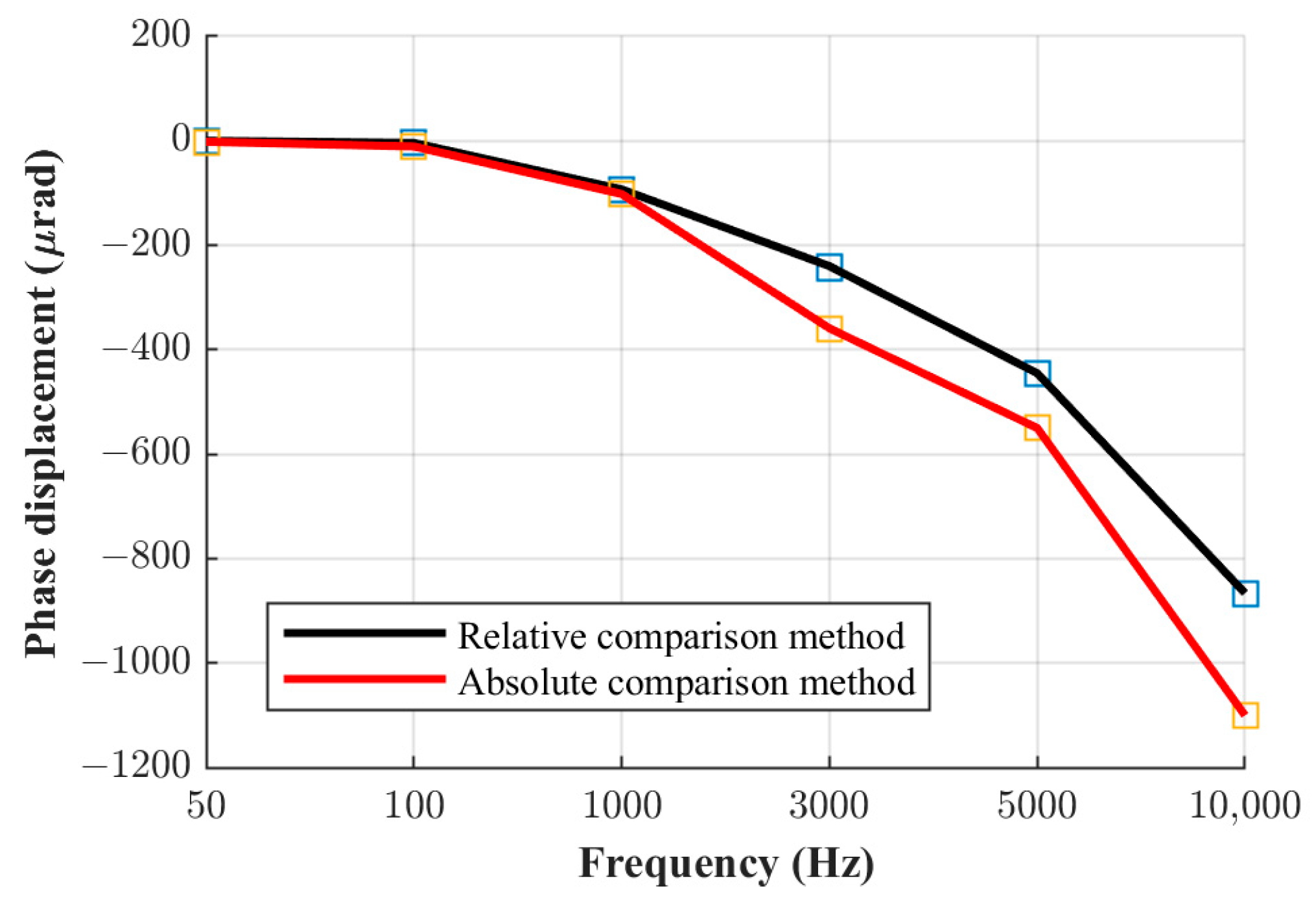
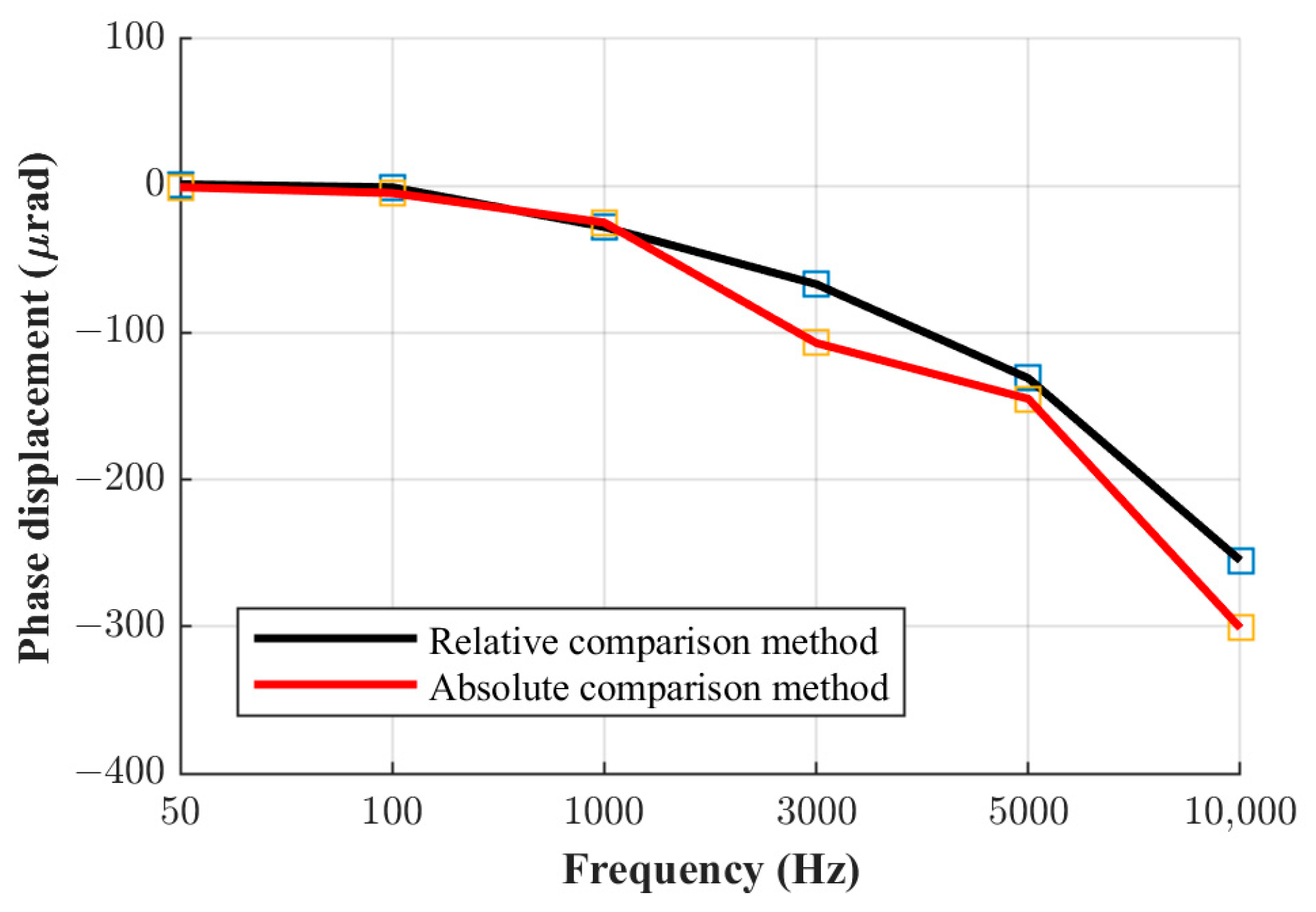
| Absolute Phase Verification with Reference Shunt | |||||
| Frequency | |||||
| 50 Hz | 100 Hz | 1 kHz | 3 kHz | 5 kHz | 10 kHz |
| 0 µrad | 0 µrad | 7 µrad | 32 µrad | 41 µrad | 72 µrad |
| 1 A Shunt | |||||
|---|---|---|---|---|---|
| Frequency | |||||
| 50 Hz | 100 Hz | 1 kHz | 3 kHz | 5 kHz | 10 kHz |
| 2 µrad | 1 µrad | 17 µrad | 39 µrad | 76 µrad | 143 µrad |
| Frequency | Measurement Uncertainty |
|---|---|
| 50 Hz | 2 µrad |
| 100 Hz | 2 µrad |
| 1 kHz | 5 µrad |
| 3 kHz | 11 µrad |
| 5 kHz | 10 µrad |
| 10 kHz | 16 µrad |
| Frequency | Measurement Uncertainty |
|---|---|
| 50 Hz | 8 µrad |
| 100 Hz | 8 µrad |
| 1 kHz | 18 µrad |
| 3 kHz | 28 µrad |
| 5 kHz | 34 µrad |
| 10 kHz | 57 µrad |
| Frequency | Measurement Uncertainty |
|---|---|
| 50 Hz | 5 µrad |
| 100 Hz | 5 µrad |
| 1 kHz | 12 µrad |
| 3 kHz | 23 µrad |
| 5 kHz | 21 µrad |
| 10 kHz | 34 µrad |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gašparini, S.; Mostarac, P.; Konjevod, J.; Malarić, R. Evaluation of the Relative Phase Comparison Method at Its Limits Used for Absolute Phase Determination of an AC Cage Current Shunt Group. Sensors 2023, 23, 8510. https://doi.org/10.3390/s23208510
Gašparini S, Mostarac P, Konjevod J, Malarić R. Evaluation of the Relative Phase Comparison Method at Its Limits Used for Absolute Phase Determination of an AC Cage Current Shunt Group. Sensors. 2023; 23(20):8510. https://doi.org/10.3390/s23208510
Chicago/Turabian StyleGašparini, Stipe, Petar Mostarac, Jure Konjevod, and Roman Malarić. 2023. "Evaluation of the Relative Phase Comparison Method at Its Limits Used for Absolute Phase Determination of an AC Cage Current Shunt Group" Sensors 23, no. 20: 8510. https://doi.org/10.3390/s23208510
APA StyleGašparini, S., Mostarac, P., Konjevod, J., & Malarić, R. (2023). Evaluation of the Relative Phase Comparison Method at Its Limits Used for Absolute Phase Determination of an AC Cage Current Shunt Group. Sensors, 23(20), 8510. https://doi.org/10.3390/s23208510






