Effect of Load on Quartz Crystal Microbalance Sensor Response Addressed Using Fractional Order Calculus
Abstract
1. Introduction
2. Materials and Methods
2.1. Fractional Order Reactive Circuit Elements
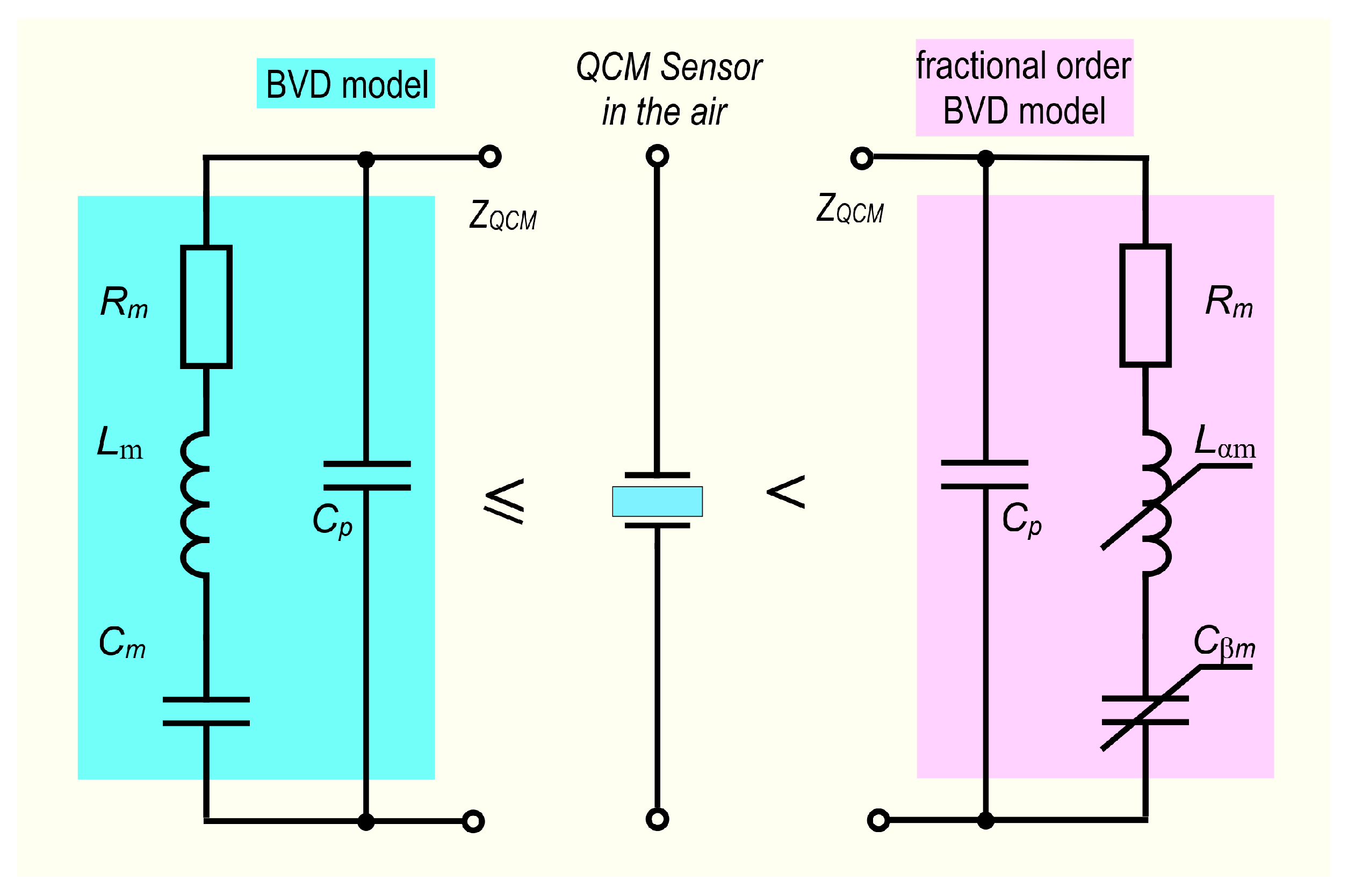
2.2. Fractional Order BVD Model
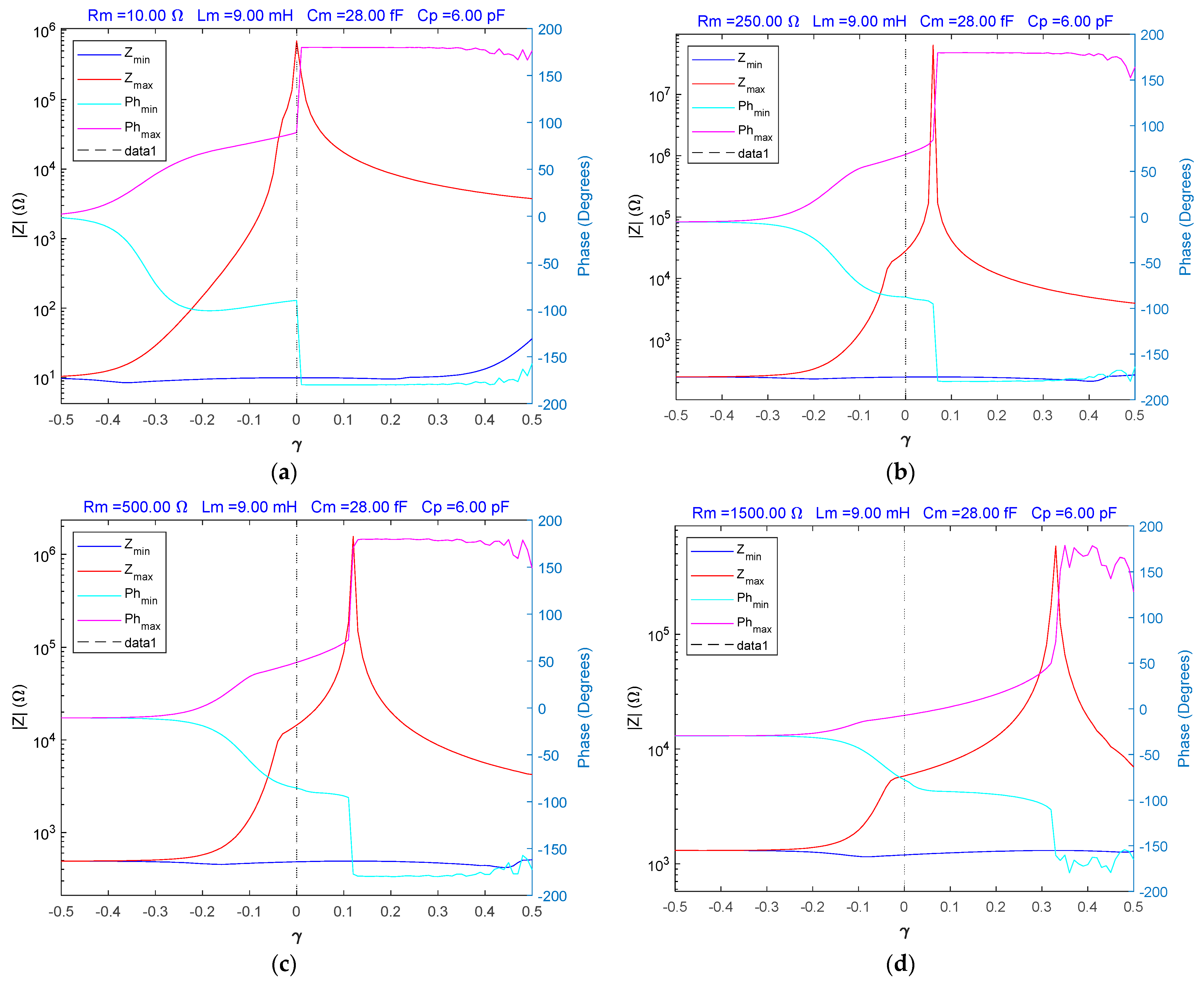
2.3. Fractional Order BVD Model Simulation
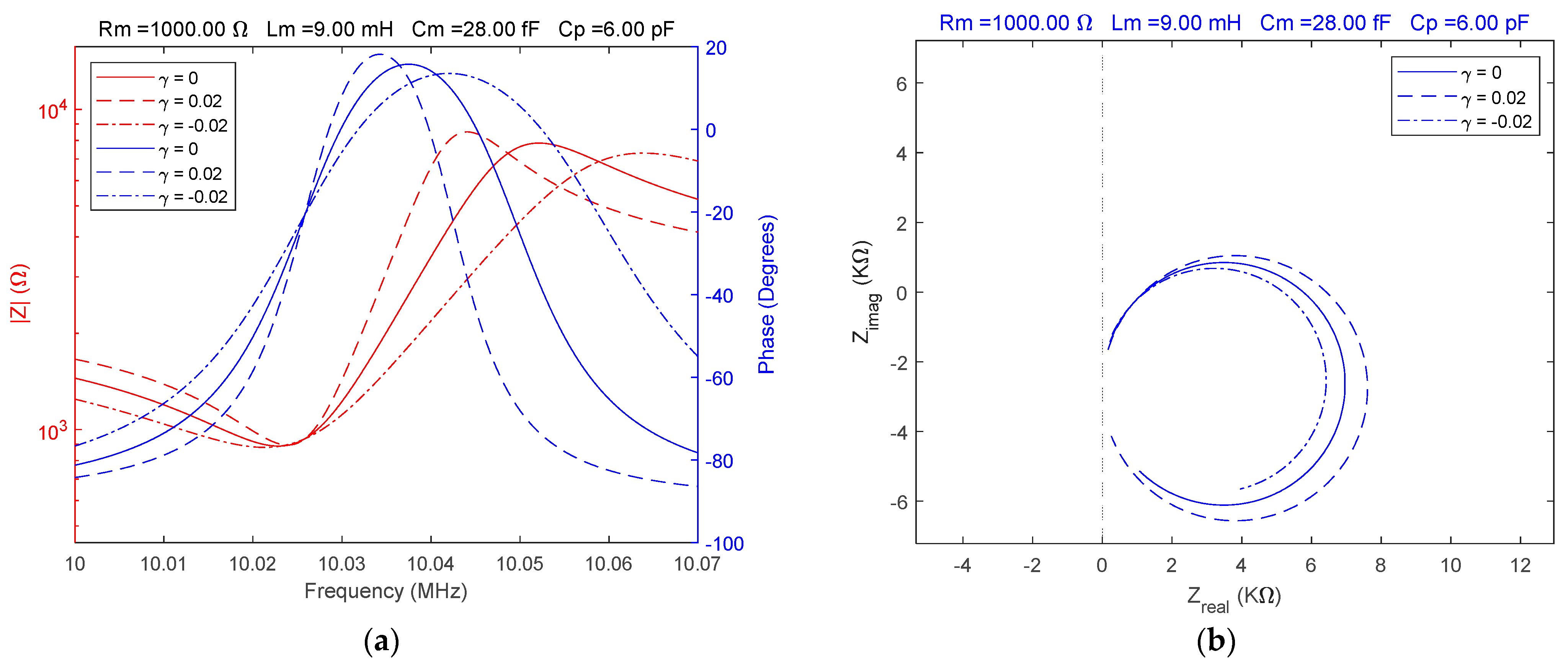
2.4. Bode and Nyquist Plot of the Fractional Order BVD Model
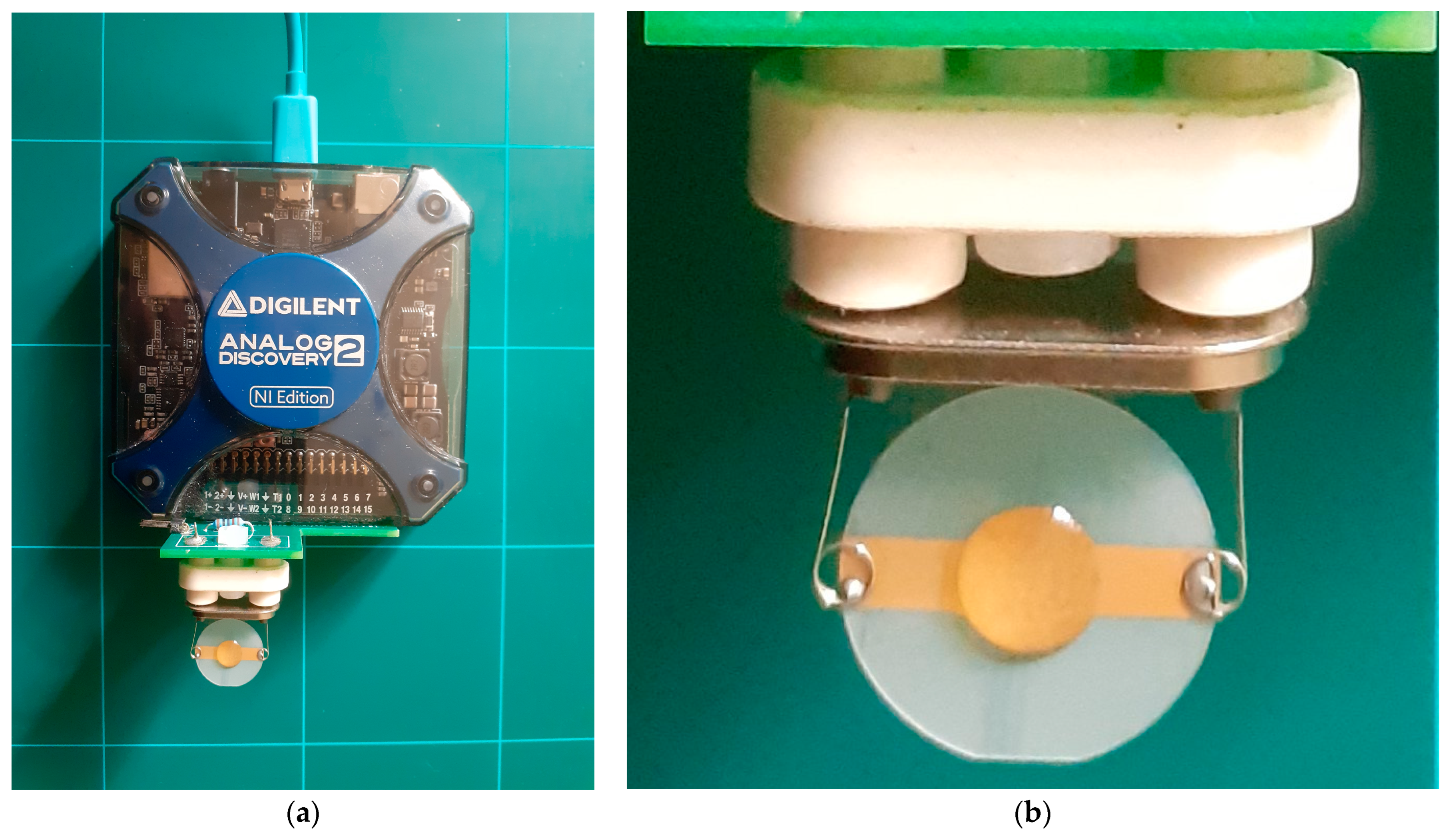
2.5. Virtual Impedance Analyzer and Experimental Setup
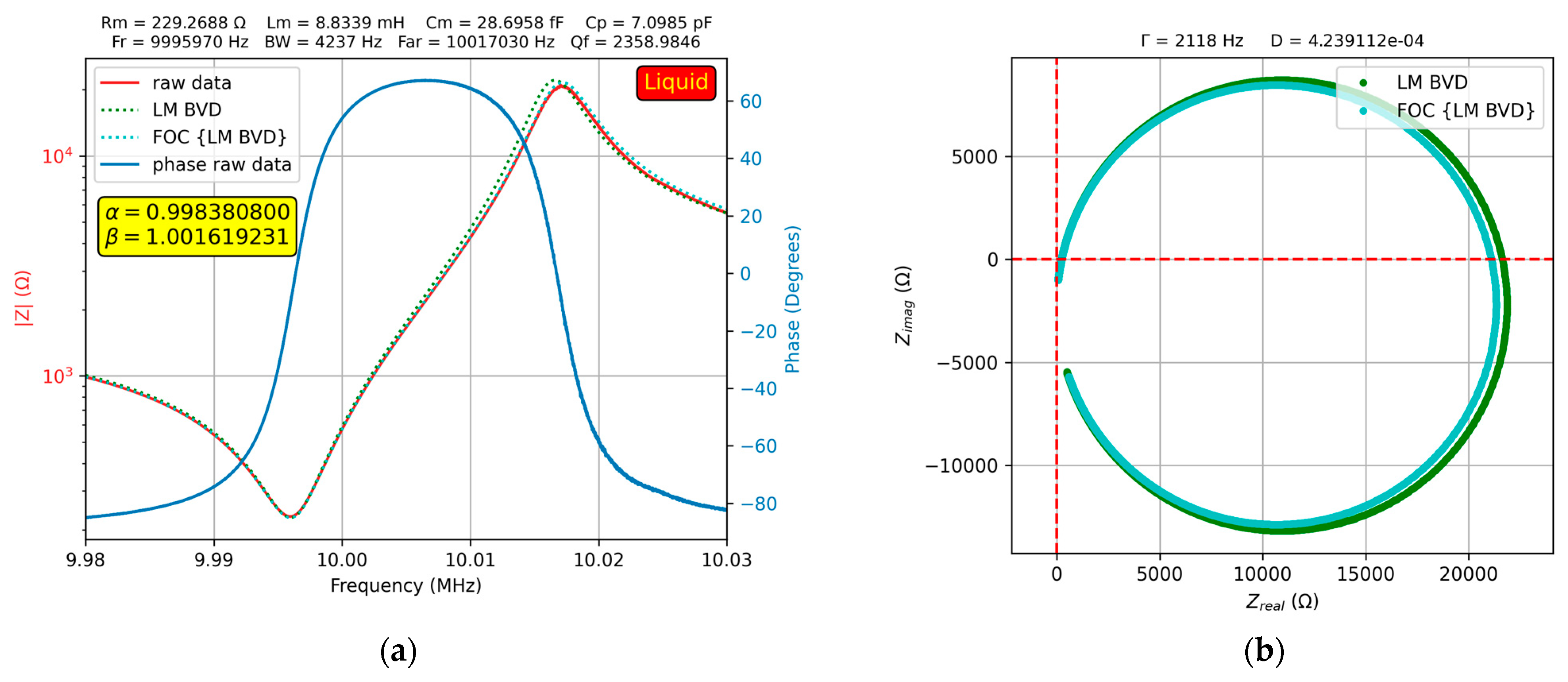
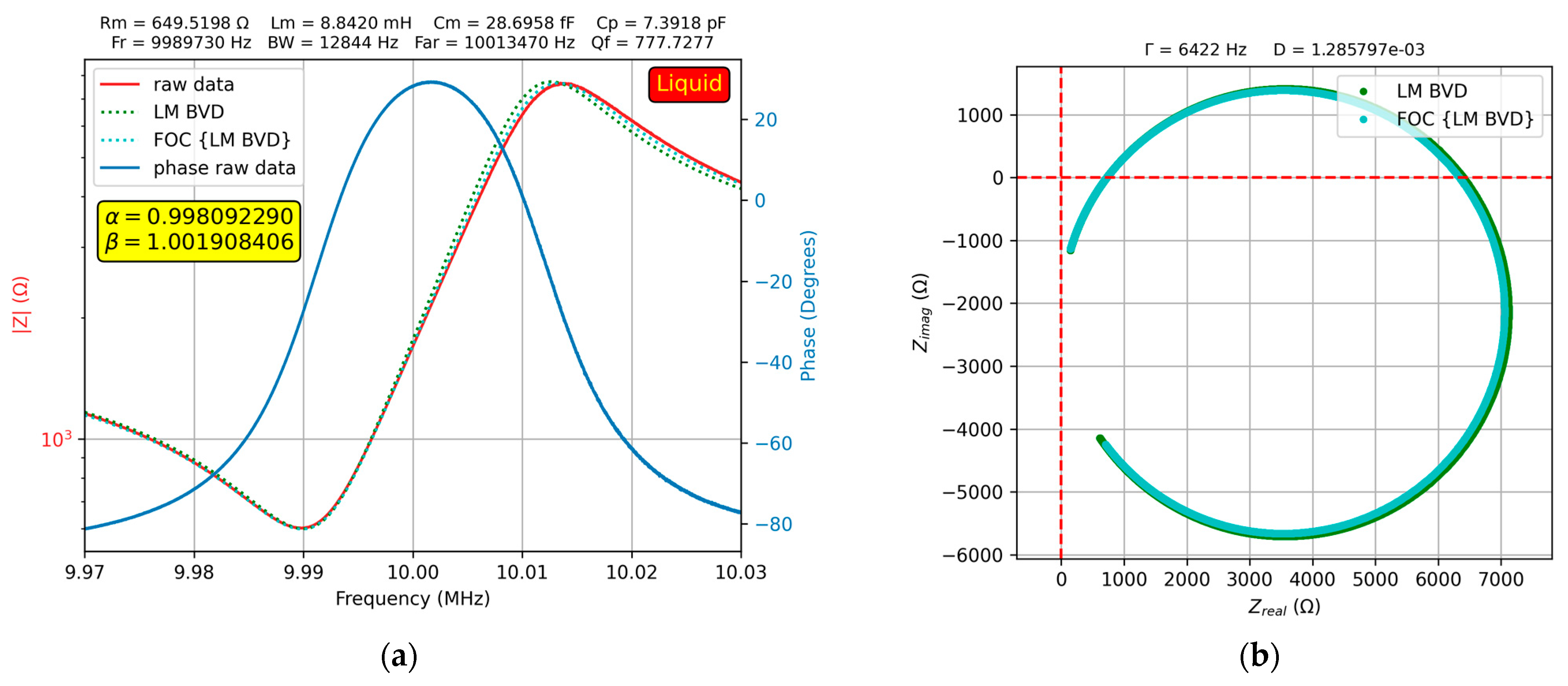
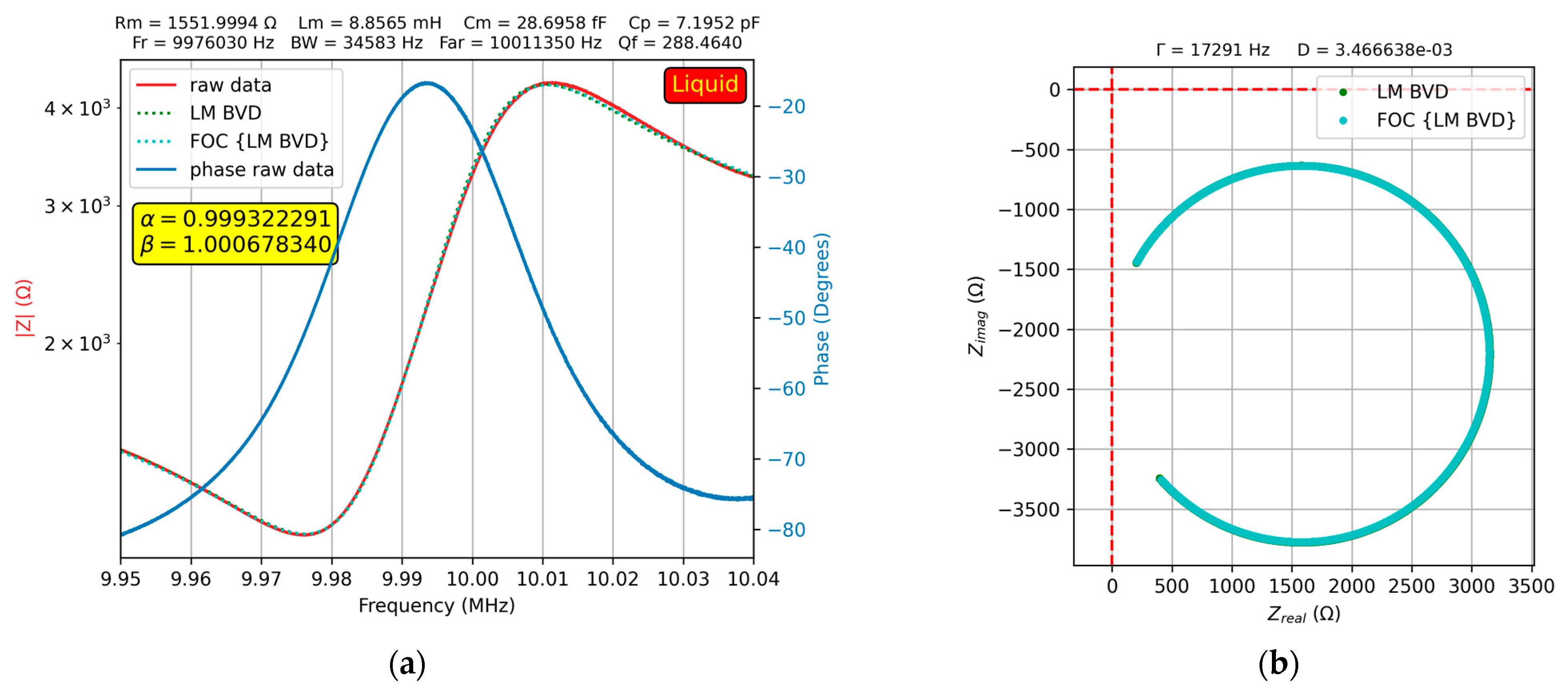
3. Results
3.1. VIA Compensation Using the Fractional Order BVD Model
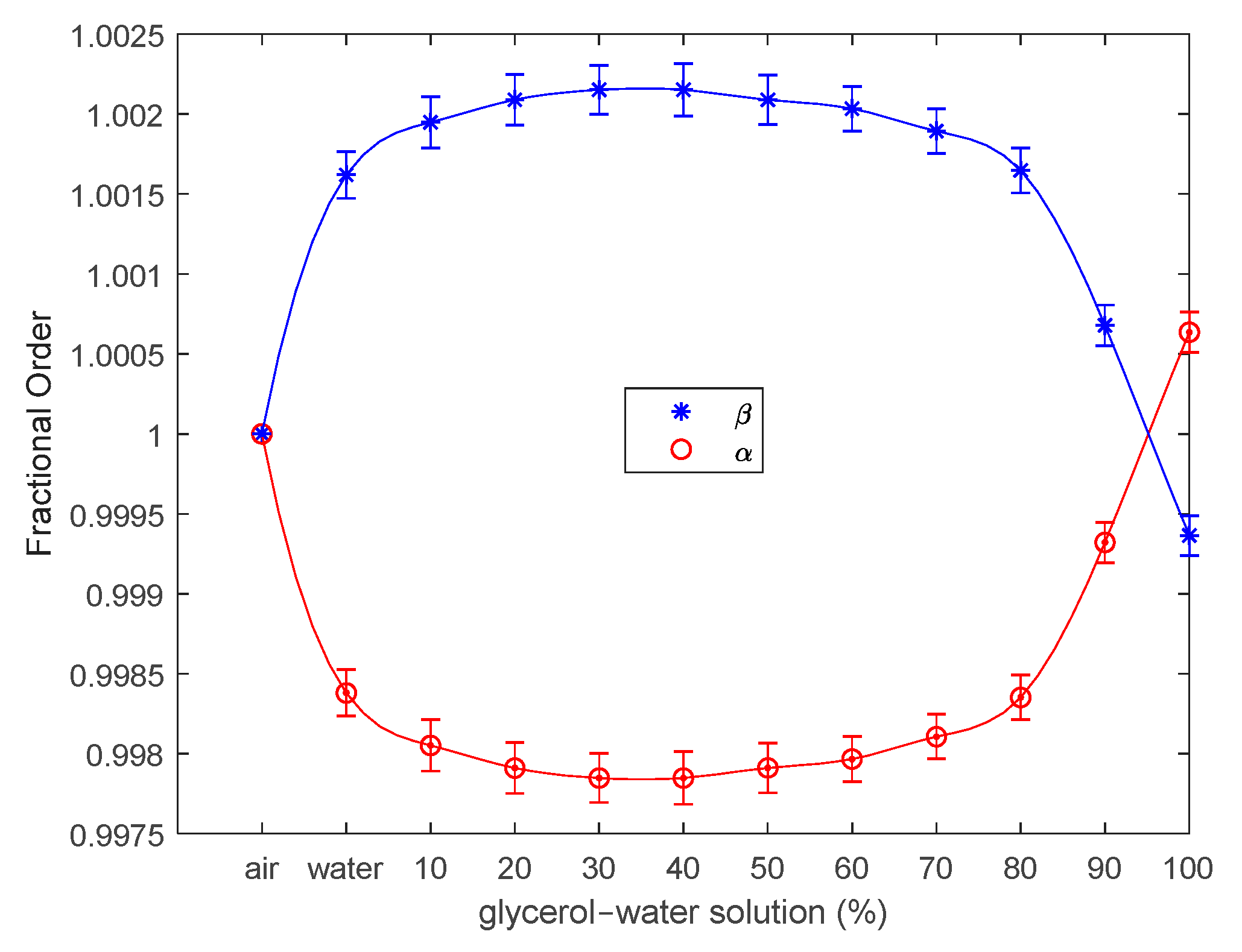
3.2. Fractional Order Effect Induced by Load on QCM Sensor Response
4. Discussion
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Oldham, K.B.; Spanier, J. The Fractional Calculus Theory and Applications of Differentiation and Integration of Arbitrary Order; Academic Press: New York, NY, USA, 1974. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; A Wiley-Interscience Publication; John Wiley & Sons, Inc.: New York, NY, USA, 1993. [Google Scholar]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives: Theory and Applications; Gordon and Breach: New York, NY, USA, 1993. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: Boston, MA, USA, 1999. [Google Scholar]
- Baleanu, D.; Diethelm, K.; Scalas, E.; Trujillo, J.J. Fractional Calculus: Models and Numerical Methods, 2nd ed.; World Scientific: New York, NY, USA, 2017. [Google Scholar]
- Elwakil, A.S. Fractional order circuits and systems: An emerging interdisciplinary research area. IEEE Circuits Syst. Mag. 2010, 10, 40–50. [Google Scholar] [CrossRef]
- Herencsar, N.; Freeborn, T.J.; Kartci, A.; Cicekoglu, O. A Comparative Study of Two Fractional order Equivalent Electrical Circuits for Modeling the Electrical Impedance of Dental Tissues. Entropy 2020, 22, 1117. [Google Scholar] [CrossRef] [PubMed]
- Caponetto, R.; Matera, F.; Murgano, E.; Privitera, E.; Xibilia, M.G. Fuel Cell Fractional order Model via Electrochemical Impedance Spectroscopy. Fractal Fract. 2021, 5, 21. [Google Scholar] [CrossRef]
- Burda, I. Quartz Crystal Microbalance with Impedance Analysis Based on Virtual Instruments: Experimental Study. Sensors 2022, 22, 1506. [Google Scholar] [CrossRef] [PubMed]
- De Beer, D.J.; Joubert, T.-H. Validation of Low-Cost Impedance Analyzer via Nitrate Detection. Sensors 2021, 21, 6695. [Google Scholar] [CrossRef] [PubMed]
- Schäfer, I.; Krüger, K. Modelling of lossy coils using fractional derivatives. J. Phys. D Appl. Phys. 2008, 41, 045001. [Google Scholar] [CrossRef]
- Valsa, J. Fractional—Order electrical components, networks and systems. In Proceedings of the 22nd International Conference Radioelektronika, Brno, Czech Republic, 17–18 April 2012. [Google Scholar]
- Westerlund, S.; Ekstam, L. Capacitor theory. IEEE Trans. Dielectr. Electr. Insul. 1994, 1, 826–839. [Google Scholar] [CrossRef]
- Fouda, M.E.; Radwan, A.G. On the fractional order memristor model. J. Fract. Calc. Appl. 2013, 4, 1–7. [Google Scholar]
- Petráš, I.; Chen, Y. Fractional order circuit elements with memory. In Proceedings of the 2012 13th International Carpathian Control Conference, High Tatras, Slovakia, 28–31 May 2012; pp. 552–558. [Google Scholar]
- Caponetto, R.; Graziani, S.; Murgano, E. Realization of a fractional order RLC circuit via constant phase element. Int. J. Dyn. Control 2021, 9, 1589–1599. [Google Scholar] [CrossRef]
- Lukács, Z.; Kristóf, T. A generalized model of the equivalent circuits in the electrochemical impedance spectroscopy. Electrochim. Acta 2020, 363, 137199. [Google Scholar] [CrossRef]
- L’vov, P.E.; Sibatov, R.T.; Yavtushenko, I.O.; Kitsyuk, E.P. Time-Fractional Phase Field Model of Electrochemical Impedance. Fractal Fract. 2021, 5, 191. [Google Scholar] [CrossRef]
- Doehring, T.C.; Freed, A.H.; Carew, E.O.; Vesely, I. Fractional order viscoelasticity of the aortic valve cusp: An alternative to quasilinear viscoelasticity. J. Biomech. Eng. 2005, 127, 700–708. [Google Scholar] [CrossRef]
- Faryad, M.; Naqvi, Q.A. Fractional rectangular waveguide. Prog. Electromagn. Res. 2007, 75, 383–396. [Google Scholar] [CrossRef]
- Li, H.; Wu, M.; Wang, X. Fractional-moment capital asset pricing model. Chaos Solitons Fractals 2009, 42, 412–421. [Google Scholar] [CrossRef]
- Martin, R.; Quintara, J.J.; Ramos, A.; Nuez, L. Modeling electrochemical double layer capacitor, from classical to fractional impedance. In Proceedings of the MELECON 2008, 14th IEEE Mediterranean Electrotechnical Conference, Ajaccio, France, 5–7 May 2008; pp. 61–66. [Google Scholar]
- Akgönüllü, S.; Özgür, E.; Denizli, A. Quartz Crystal Microbalance-Based Aptasensors for Medical Diagnosis. Micromachines 2022, 13, 1441. [Google Scholar] [CrossRef]
- Na Songkhla, S.; Nakamoto, T. Overview of Quartz Crystal Microbalance Behavior Analysis and Measurement. Chemosensors 2021, 9, 350. [Google Scholar] [CrossRef]
- Sauerbrey, G. Use of quartz crystals for weighing thin layers and for microweighing. Z. Phys. 1959, 155, 206–222. [Google Scholar] [CrossRef]
- Johannsmann, D.; Langhoff, A.; Leppin, C. Studying Soft Interfaces with Shear Waves: Principles and Applications of the Quartz Crystal Microbalance (QCM). Sensors 2021, 21, 3490. [Google Scholar] [CrossRef] [PubMed]
- Arnau, A. A review of interface electronic systems for AT-cut quartz crystal microbalance applications in liquids. Sensors 2008, 8, 370–411. [Google Scholar] [CrossRef] [PubMed]
- Burda, I. Advanced Impedance Spectroscopy for QCM Sensor in Liquid Medium. Sensors 2022, 22, 2337. [Google Scholar] [CrossRef] [PubMed]
- Matko, V. Multiple Quartz Crystals Connected in Parallel for High-Resolution Sensing of Capacitance Changes. Sensors 2022, 22, 5030. [Google Scholar] [CrossRef] [PubMed]
- Fort, A.; Panzardi, E.; Vignoli, V.; Tani, M.; Landi, E.; Mugnaini, M.; Vaccarella, P. An adaptive measurement system for the simultaneous evaluation of frequency shift and series resistance of QCM in liquid. Sensors 2021, 21, 678. [Google Scholar] [CrossRef] [PubMed]
- Rodahl, M.; Kasemo, B. A simple setup to simultaneously measure the resonant frequency and the absolute dissipation factor of a quartz crystal microbalance. Rev. Sci. Instrum. 1996, 67, 3238–3241. [Google Scholar] [CrossRef]
- Burda, I. A Study on Regenerative Quartz Crystal Microbalance. Chemosensors 2022, 10, 262. [Google Scholar] [CrossRef]
- Alassi, A.; Benammar, M.; Brett, D. Quartz Crystal Microbalance Electronic Interfacing Systems: A Review. Sensors 2017, 17, 2799. [Google Scholar] [CrossRef] [PubMed]
- Kanazawa, K.K.; Gordon, J.G., II. The oscillation frequency of a quartz resonator in contact with a liquid. Anal. Chim. Acta 1985, 175, 99–105. [Google Scholar] [CrossRef]
- Johannsmann, D.; Langhoff, A.; Leppin, C.; Reviakine, I.; Maan, A.M.C. Effect of Noise on Determining Ultrathin-Film Parameters from QCM-D Data with the Viscoelastic Model. Sensors 2023, 23, 1348. [Google Scholar] [CrossRef]
- Masruroh; Santjojo, D.J.D.H. The Impedance Analysis of a Viscoelastic Petalous Structured Stearic Acid Functional Layer Deposited on a QCM. Sensors 2022, 22, 7504. [Google Scholar] [CrossRef]
- Wudy, F.; Multerer, M.; Stock, C.; Schmeer, G.; Gores, H.J. Rapid impedance scanning QCM for electrochemical applications based on miniaturized hardware and high-performance curve fitting. Electrochim. Acta 2008, 53, 6568–6574. [Google Scholar] [CrossRef]
- Burda, I. Assessing Impedance Analyzer Data Quality by Fractional Order Calculus: A QCM Sensor Case Study. Electronics 2023, 12, 2127. [Google Scholar] [CrossRef]
- Freeborn, T.J.; Maundy, B.; Elwakil, A.S. Measurement of supercapacitor fractional order model parameters from voltage-excited step response. IEEE J. Emerg. Sel. Top. Circuits Syst. 2013, 3, 367–376. [Google Scholar] [CrossRef]
- Zhao, K. Stability of a Nonlinear Langevin System of ML-Type Fractional Derivative Affected by Time-Varying Delays and Differential Feedback Control. Fractal Fract. 2022, 6, 725. [Google Scholar] [CrossRef]
- Garrappa, R.; Kaslik, E.; Popolizio, M. Evaluation of Fractional Integrals and Derivatives of Elementary Functions: Overview and Tutorial. Mathematics 2019, 7, 407. [Google Scholar] [CrossRef]
- Caputo, M. Linear models of dissipation whose q is almost frequency independent—II. Geophys. J. R. Astron. Soc. 1967, 13, 529–539. [Google Scholar] [CrossRef]
- Beck, R.; Pittermann, U.; Weil, K.G. Impedance analysis of quartz oscillators, contacted on one side with a liquid. Ber. Bunsenges. Phys. Chem. 1988, 92, 1363–1368. [Google Scholar] [CrossRef]
- Analod Discovery 2 Reference Manual. Available online: https://digilent.com/reference/test-and-measurement/analog-discovery-2/reference-manual (accessed on 24 May 2023).






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Burda, I. Effect of Load on Quartz Crystal Microbalance Sensor Response Addressed Using Fractional Order Calculus. Sensors 2023, 23, 6768. https://doi.org/10.3390/s23156768
Burda I. Effect of Load on Quartz Crystal Microbalance Sensor Response Addressed Using Fractional Order Calculus. Sensors. 2023; 23(15):6768. https://doi.org/10.3390/s23156768
Chicago/Turabian StyleBurda, Ioan. 2023. "Effect of Load on Quartz Crystal Microbalance Sensor Response Addressed Using Fractional Order Calculus" Sensors 23, no. 15: 6768. https://doi.org/10.3390/s23156768
APA StyleBurda, I. (2023). Effect of Load on Quartz Crystal Microbalance Sensor Response Addressed Using Fractional Order Calculus. Sensors, 23(15), 6768. https://doi.org/10.3390/s23156768





