Effect of Finite-Sized Optical Components and Pixels on Light-Field Imaging through Correlated Light
Abstract
:1. Introduction
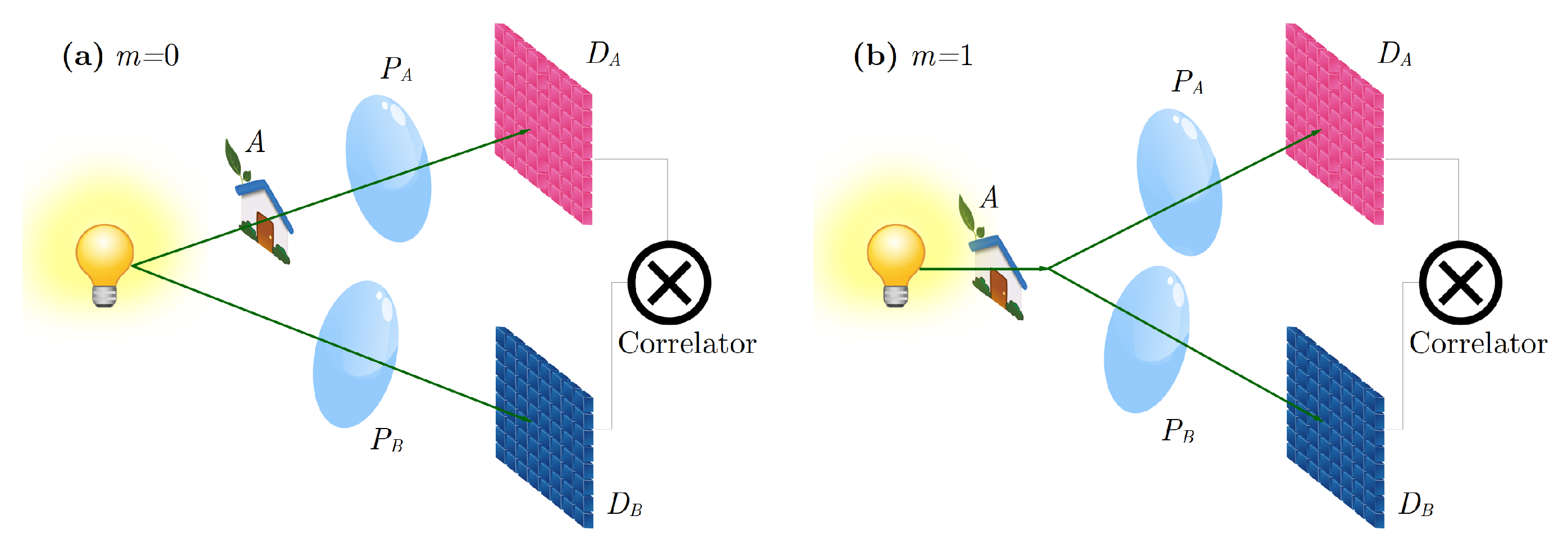
2. Materials and Methods
3. Results
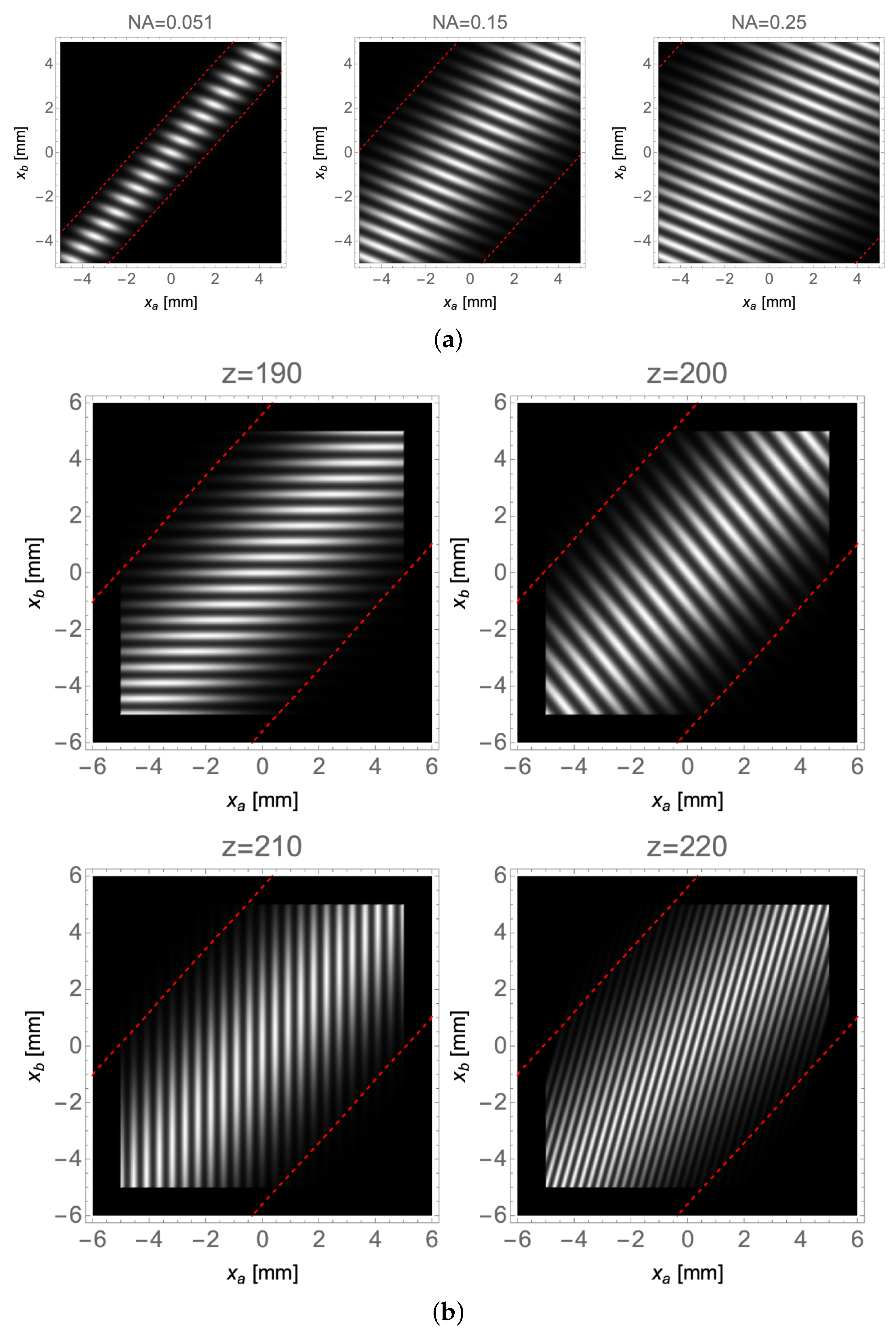
3.1. Effect of the Finite Apertures
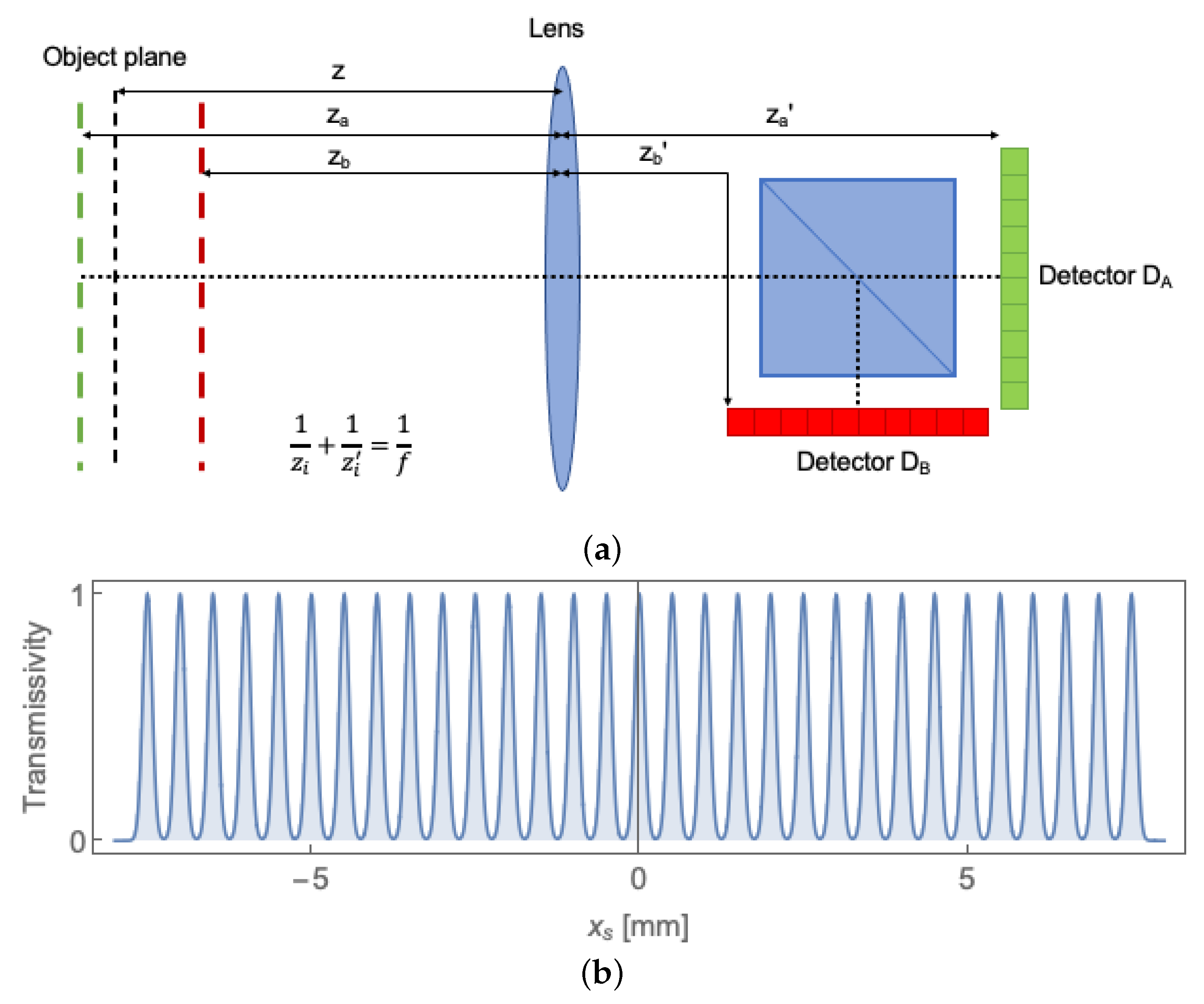
3.2. Effect of the Finite Size of the Detectors
- 1.
- Fall outside of the area defined by the detectors for slits that are outside the FOV;
- 2.
- Fall only partially outside of the detector area so that, upon reconstruction, the corresponding slits will receive a decreasing amount of contributions than that available on the lens area (red area);
- 3.
- Be fully included in the detector area, thus enabling reconstruction of the corresponding object point with all possible contributions from the lens (green area).
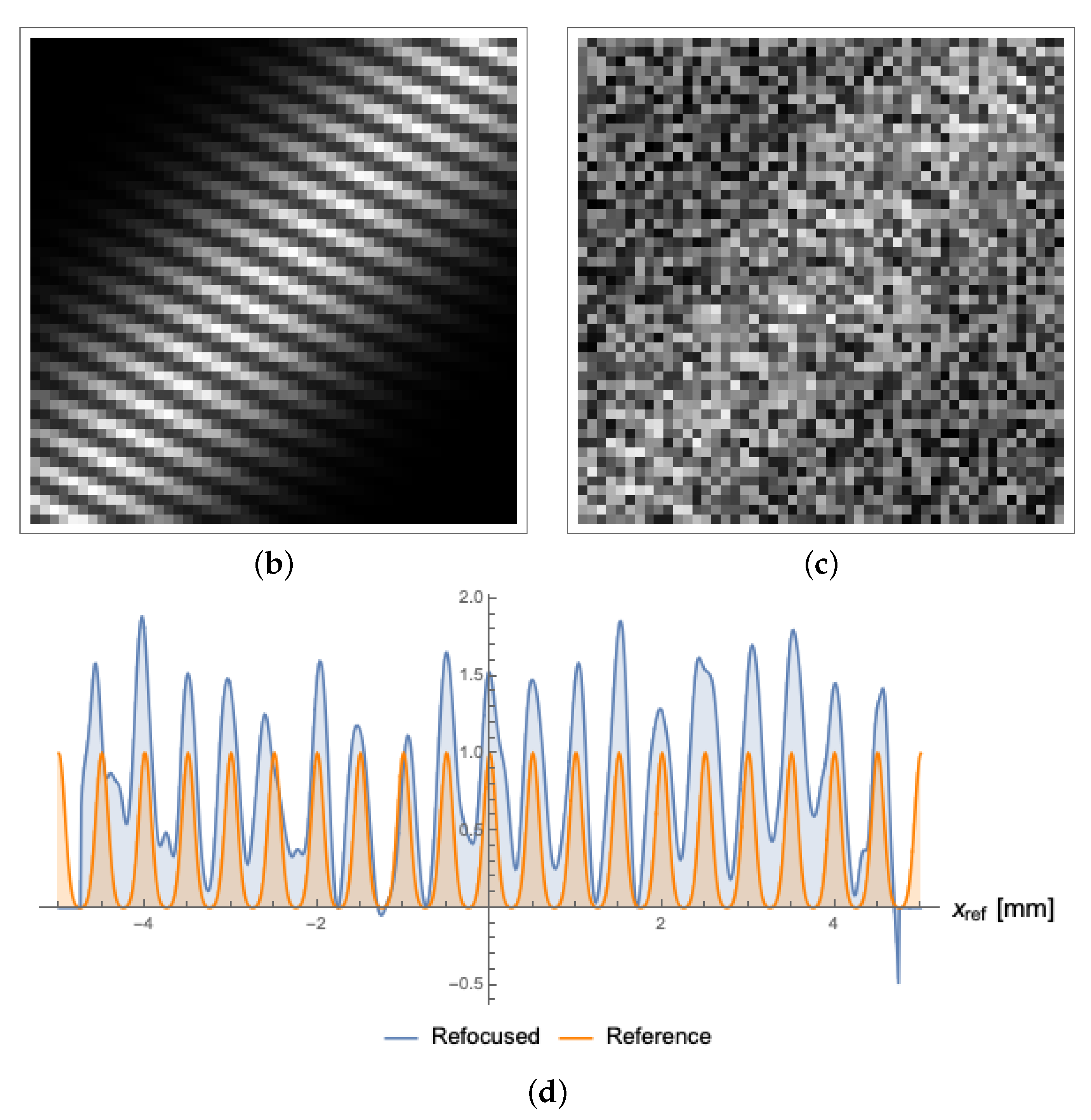
3.3. Effect of the Finite Size of the Pixels
4. Discussion
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. Derivation of the Resolution Limit Due to the Finite Pixel Size

References
- Adelson, E.H.; Wang, J.Y. Single lens stereo with a plenoptic camera. IEEE 1992, 14, 99–106. [Google Scholar] [CrossRef] [Green Version]
- Pawley, J. Handbook of Biological Confocal Microscopy; Springer: Berlin/Heidelberg, Germany, 2006; Volume 236. [Google Scholar]
- Ihrke, I.; Restrepo, J.; Mignard-Debise, L. Principles of light field imaging: Briefly revisiting 25 years of research. IEEE 2016, 33, 59–69. [Google Scholar] [CrossRef] [Green Version]
- Lien, M.B.; Liu, C.H.; Chun, I.Y.; Ravishankar, S.; Nien, H.; Zhou, M.; Fessler, J.A.; Zhong, Z.; Norris, T.B. Ranging and light field imaging with transparent photodetectors. Nat. Photonics 2020, 14, 143–148. [Google Scholar] [CrossRef]
- Ng, R.; Levoy, M.; Brédif, M.; Duval, G.; Horowitz, M.; Hanrahan, P. Light Field Photography with a Hand-Held Plenoptic Camera. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 2005. [Google Scholar]
- Ng, R. Fourier slice photography. ACM Trans. Graph. 2005, 24, 735–744. [Google Scholar] [CrossRef]
- Birklbauer, C.; Bimber, O. Panorama light-field imaging. In Computer Graphics Forum; Wiley Online Library: Hoboken, NJ, USA, 2014; Volume 33, pp. 43–52. [Google Scholar]
- Xue, Z.; Baboulaz, L.; Prandoni, P.; Vetterli, M. Light field panorama by a plenoptic camera. In Computational Imaging XII; SPIE: Bellingham, WA, USA, 2014; Volume 9020, pp. 186–196. [Google Scholar]
- Broxton, M.; Grosenick, L.; Yang, S.; Cohen, N.; Andalman, A.; Deisseroth, K.; Levoy, M. Wave optics theory and 3-D deconvolution for the light field microscope. Opt. Exp. 2013, 21, 25418–25439. [Google Scholar] [CrossRef] [PubMed]
- Prevedel, R.; Yoon, Y.G.; Hoffmann, M.; Pak, N.; Wetzstein, G.; Kato, S.; Schrödel, T.; Raskar, R.; Zimmer, M.; Boyden, E.S.; et al. Simultaneous whole-animal 3D imaging of neuronal activity using light-field microscopy. Nat. Methods 2014, 11, 727–730. [Google Scholar] [CrossRef] [PubMed]
- Ko, J.; Davis, C.C. Comparison of the plenoptic sensor and the Shack–Hartmann sensor. Appl. Opt. 2017, 56, 3689–3698. [Google Scholar] [CrossRef] [PubMed]
- Zhu, H.; Wang, Q.; Yu, J. Light field imaging: Models, calibrations, reconstructions, and applications. Front. Inf. Technol. Electron. Eng. 2017, 18, 1236–1249. [Google Scholar] [CrossRef]
- Georgiev, T.; Zheng, K.C.; Curless, B.; Salesin, D.; Nayar, S.K.; Intwala, C. Spatio-angular resolution tradeoffs in integral photography. Render. Tech. 2006, 2006, 263–272. [Google Scholar]
- Goldluecke, B.; Klehm, O.; Wanner, S.; Eisemann, E. Digital Representations of the Real World: How to Capture, Model, and Render Visual Reality. In Plenoptic Cameras; CRC Press: Boca Raton, FL, USA, 2015; pp. 65–77. [Google Scholar]
- D’Angelo, M.; Pepe, F.V.; Garuccio, A.; Scarcelli, G. Correlation plenoptic imaging. Phys. Rev. Lett. 2016, 116, 223602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pepe, F.V.; Di Lena, F.; Mazzilli, A.; Edrei, E.; Garuccio, A.; Scarcelli, G.; D’Angelo, M. Diffraction-limited plenoptic imaging with correlated light. Phys. Rev. Lett. 2017, 119, 243602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Scagliola, A.; Di Lena, F.; Garuccio, A.; D’Angelo, M.; Pepe, F.V. Correlation Plenoptic Imaging for microscopy applications. Phys. Lett. A 2020, 384, 126472. [Google Scholar] [CrossRef]
- Massaro, G.; Giannella, D.; Scagliola, A.; Lena, F.D.; Scarcelli, G.; Garuccio, A.; Pepe, F.V.; D’Angelo, M. Light-field microscopy with correlated beams for extended volumetric imaging at the diffraction limit. arXiv 2021, arXiv:2110.00807. [Google Scholar]
- Pepe, F.V.; Di Lena, F.; Garuccio, A.; Scarcelli, G.; D’Angelo, M. Correlation Plenoptic Imaging With Entangled Photons. Technologies 2016, 4, 17. [Google Scholar] [CrossRef] [Green Version]
- Rubin, M.H.; Klyshko, D.N.; Shih, Y.; Sergienko, A. Theory of two-photon entanglement in type-II optical parametric down-conversion. Phys. Rev. A 1994, 50, 5122. [Google Scholar] [CrossRef] [PubMed]
- Di Lena, F.; Pepe, F.; Garuccio, A.; D’Angelo, M. Correlation Plenoptic Imaging: An Overview. Appl. Sci. 2018, 8, 1958. [Google Scholar] [CrossRef] [Green Version]
- Mandel, L.; Wolf, E. Optical Coherence and Quantum Optics; Cambridge University Press: Cambridge, UK, 1995. [Google Scholar]
- Di Lena, F.; Massaro, G.; Lupo, A.; Garuccio, A.; Pepe, F.V.; D’Angelo, M. Correlation plenoptic imaging between arbitrary planes. Opt. Expr. 2020, 28, 35857–35868. [Google Scholar] [CrossRef] [PubMed]
- Glauber, R.J. The quantum theory of optical coherence. Phys. Rev. 1963, 130, 2529. [Google Scholar] [CrossRef] [Green Version]
- Saleh, B.E.; Teich, M.C. Fundamentals of Photonics; Wiley: Hoboken, NJ, USA, 2007. [Google Scholar]
- Bleistein, N.; Handelsman, R.A. Asymptotic Expansions of Integrals; Ardent Media: Dallas, PA, USA, 1975. [Google Scholar]
- Scala, G.; D’Angelo, M.; Garuccio, A.; Pascazio, S.; Pepe, F.V. Signal-to-noise properties of correlation plenoptic imaging with chaotic light. Phys. Rev. A 2019, 99, 053808. [Google Scholar] [CrossRef] [Green Version]
- Antolovic, I.M.; Burri, S.; Hoebe, R.A.; Maruyama, Y.; Bruschini, C.; Charbon, E. Photon-counting arrays for time-resolved imaging. Sensors 2016, 16, 1005. [Google Scholar] [CrossRef] [PubMed] [Green Version]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Massaro, G.; Di Lena, F.; D’Angelo, M.; Pepe, F.V. Effect of Finite-Sized Optical Components and Pixels on Light-Field Imaging through Correlated Light. Sensors 2022, 22, 2778. https://doi.org/10.3390/s22072778
Massaro G, Di Lena F, D’Angelo M, Pepe FV. Effect of Finite-Sized Optical Components and Pixels on Light-Field Imaging through Correlated Light. Sensors. 2022; 22(7):2778. https://doi.org/10.3390/s22072778
Chicago/Turabian StyleMassaro, Gianlorenzo, Francesco Di Lena, Milena D’Angelo, and Francesco V. Pepe. 2022. "Effect of Finite-Sized Optical Components and Pixels on Light-Field Imaging through Correlated Light" Sensors 22, no. 7: 2778. https://doi.org/10.3390/s22072778
APA StyleMassaro, G., Di Lena, F., D’Angelo, M., & Pepe, F. V. (2022). Effect of Finite-Sized Optical Components and Pixels on Light-Field Imaging through Correlated Light. Sensors, 22(7), 2778. https://doi.org/10.3390/s22072778







