High-Precision Low-Temperature Drift LDO Regulator Tailored for Time-Domain Temperature Sensors
Abstract
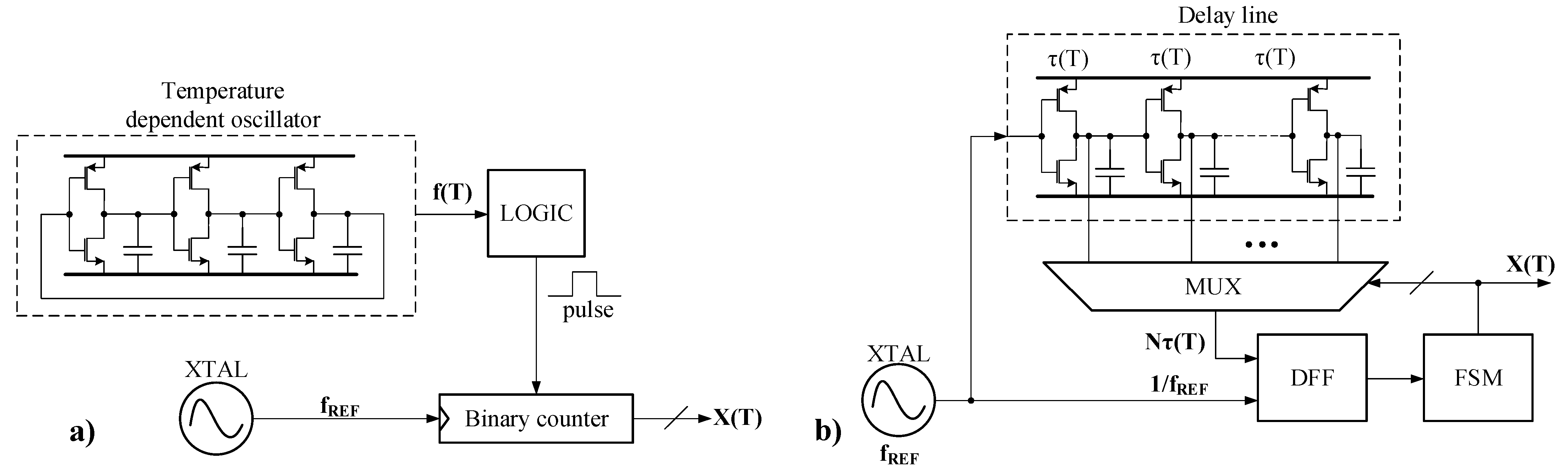
:1. Introduction
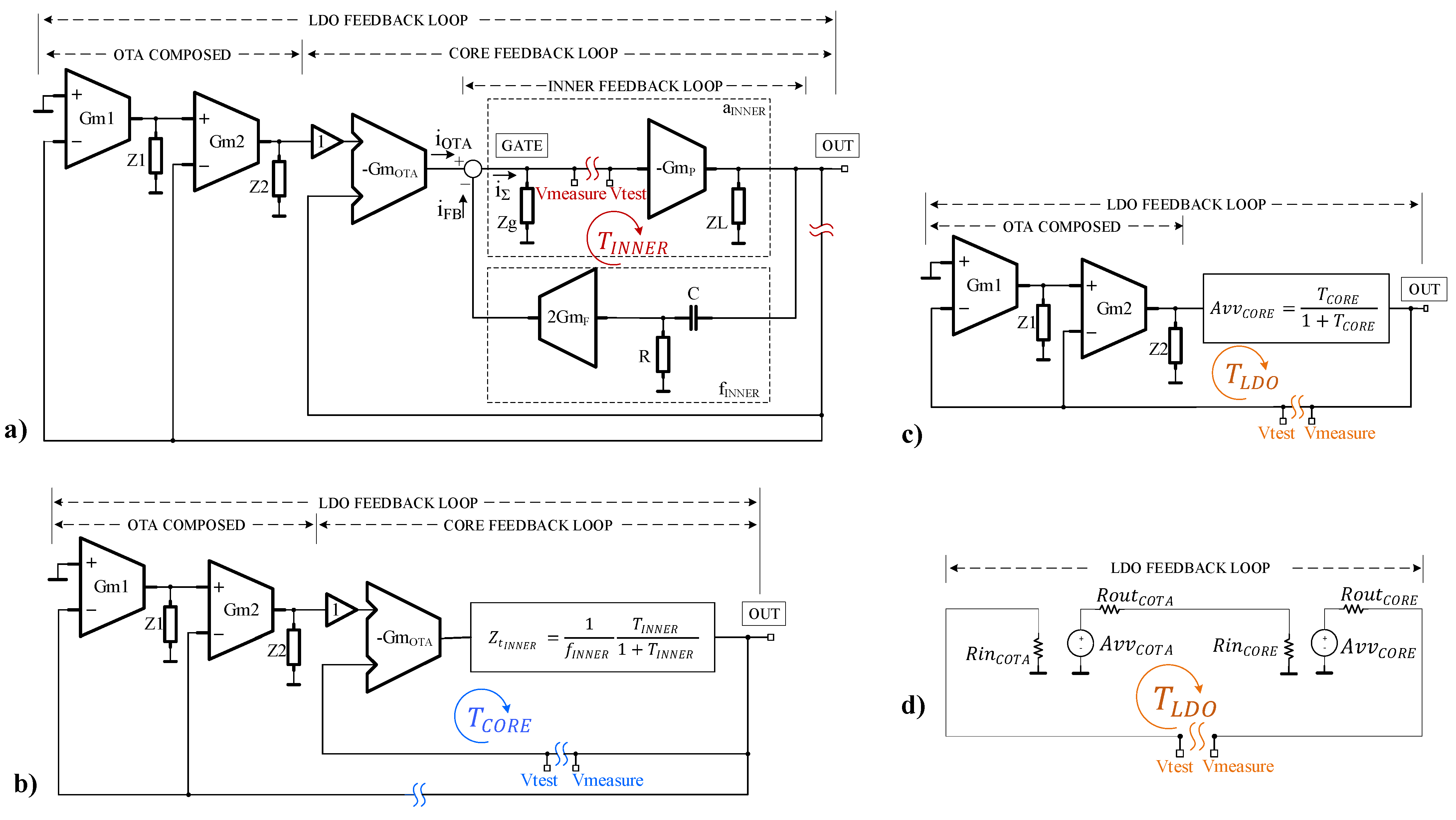
2. Proposed Fast LDO with Multiple Feedback Loops
2.1. Proposed Schematic
- (1)
- The current recycling introduced in [24], realized here by using two transistors for each input (M1A_B and M2A_B) and the current mirrors M3A-M3B and M4A-M4B.
- (2)
- The local common-mode feedback (LCMFB) introduced in [25], realized here by the resistors R0, helps to further increase both the gain and the slew rate.
- (i)
- A larger DC gain for the LDO;
- (ii)
- A feed-forward signal path is created by having the negative inputs of both Gm1 and Gm2 connected to the LDO output. This is a key feature for obtaining a suitable Phase Margin for the LDO, as it will be shown in Section 2.2.
2.2. Stability Analysis
- -
- The inner loop—whose gain is denoted in Figure 2—is closed around the Fast OTA by the frequency compensation circuit based on capacitors C1 and C2;
- -
- The total feedback loop closed around the FAST LDO CORE forms the core feedback loop—whose gain is denoted in Figure 2;
- -
- The outer loop—whose gain is denoted in Figure 2—is the main voltage control loop of the LDO; it combines the two direct connections between the LDO output and the inverting inputs of the two transconductors within the COTA, Gm1, and Gm2.
- -
- First, the multiple-loop topology can be simplified iteratively, starting from the inner loop and moving outwards. At each step, the inner-most feedback section is replaced by its closed loop equivalent yielded by using classical feedback theory, thus simplifying the analysis of the entire circuit.
- -
- -
- Note that the voltage and current transfer ratios appear “in parallel”; this suggests that, if one of these ratios is far smaller than the other one, the resulting loop gain is mainly determined by the smaller transfer ratio.
3. Design Example
3.1. LDO Requirements and Design Strategy
- (S1)
- Size the pass transistor by using a simple model for the error amplifier that includes only the DC gain and the output impedance.
- (S2)
- Design the fast LDO core focusing on getting the largest possible value for , within the current consumption budget.
- (S3)
- Use (14) to compute considering the worst-case value for obtained in the previous step. In our case this approach yielded = 111 krad/s.
- (S4)
- Derive the required DC gain from the LDO requirements, then split it between the gain stages implemented by Gm1 and Gm2.
- (S5)
- Use (13) to compute the value of .In our case, this yielded = 27 rad/s.
- (S6)
- From S4 and S5 compute the required compensation capacitor Cc. Note that this value is to be used in both Gm1 and Gm2.In our case, the required capacitor value was Cc = 6 pF.
- (S7)
- Complete the design by sizing the transistors and resistors within the circuit. Due to the modular architecture of the LDO, the composite OTA can be optimized for low offset and temperature drift, independently of the fast LDO core, without impacting the transient response. For example, transistors with large widths and lengths were used to implement the input stages of both Gm1 and Gm2 cells in Figure 3. The remaining current budget was split equally between Gm1 and Gm2.
- (S8)
- Optimize design considering Monte Carlo and PVT simulations; in particular, find a suitable value for capacitor Cm that helps improve the initial phase of the LDO response to load transients. In our case, the optimum Cm value was found to be 4 pF.
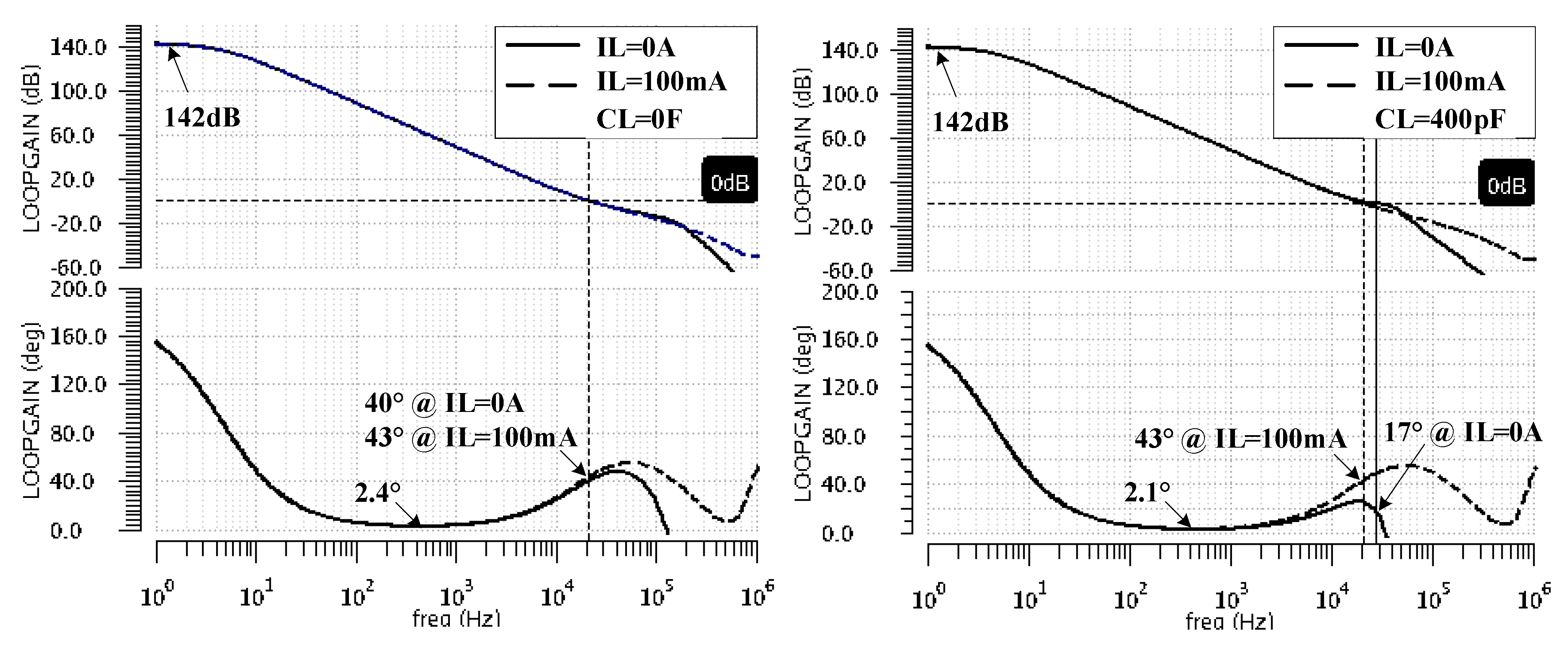
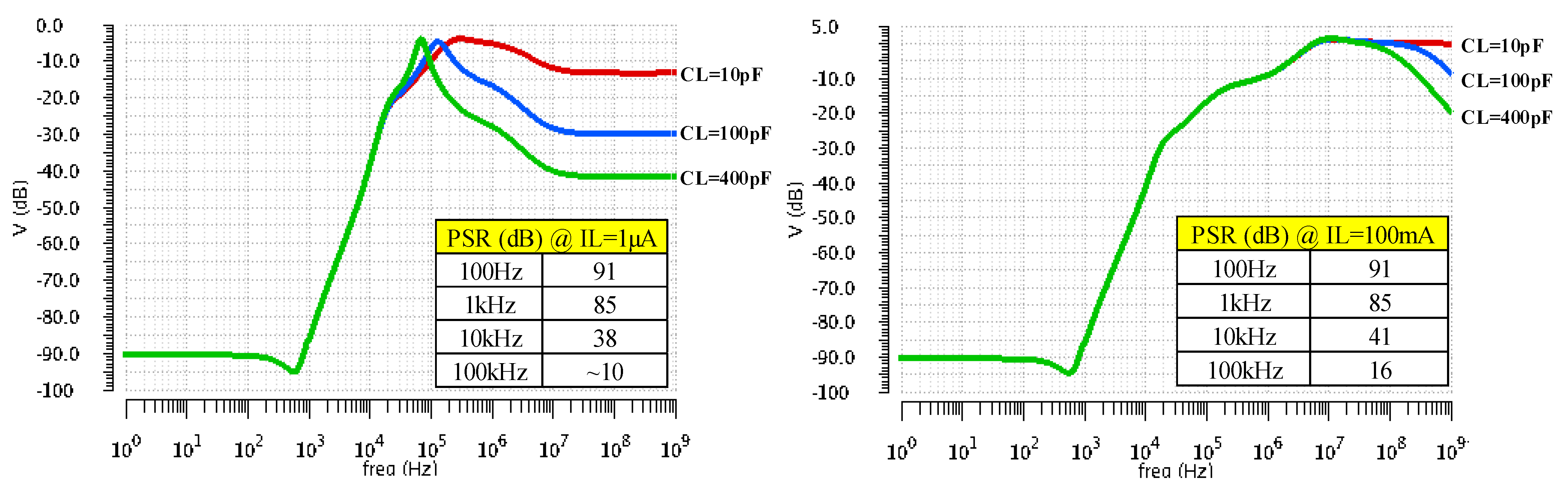
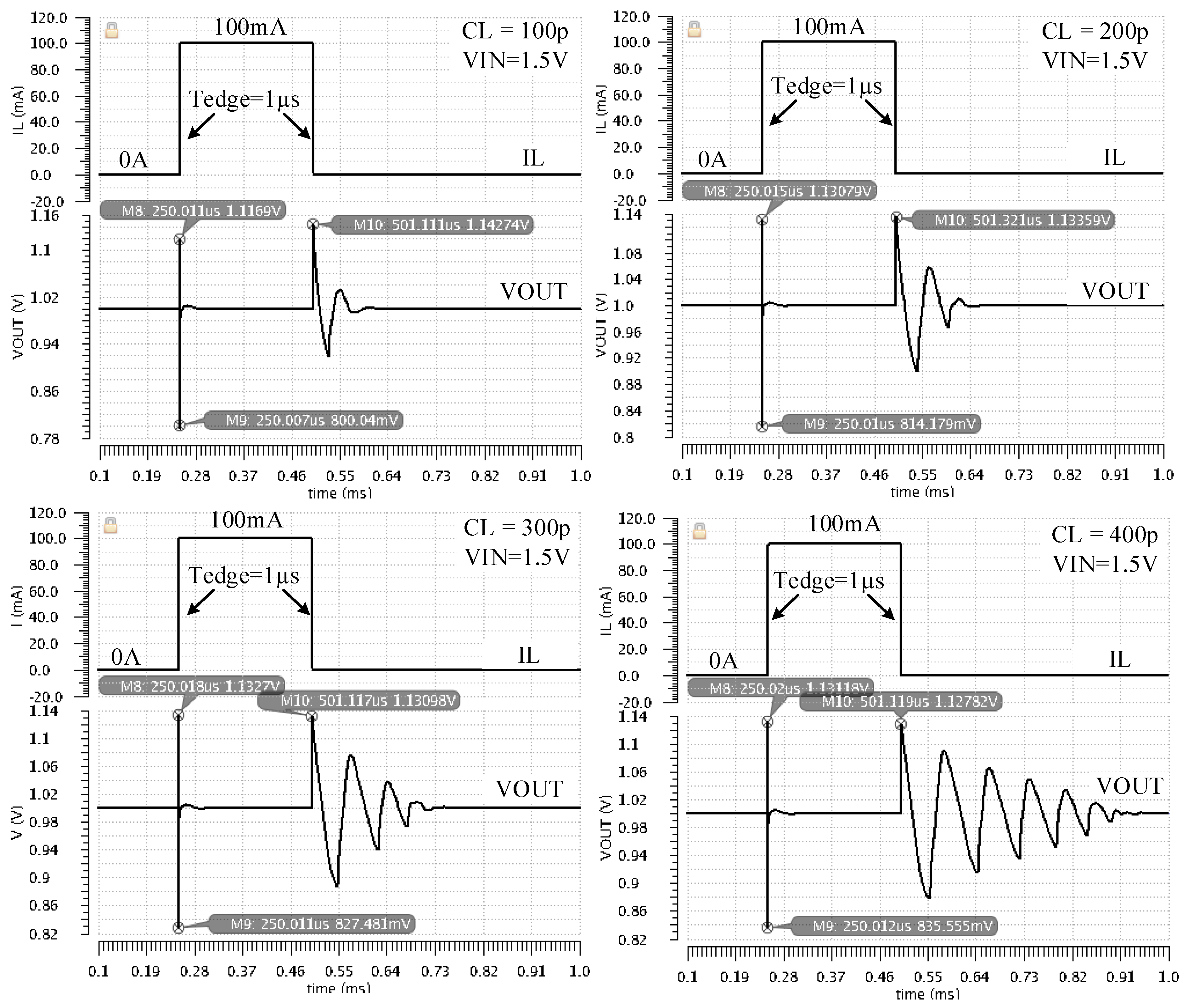
3.2. Simulation Results
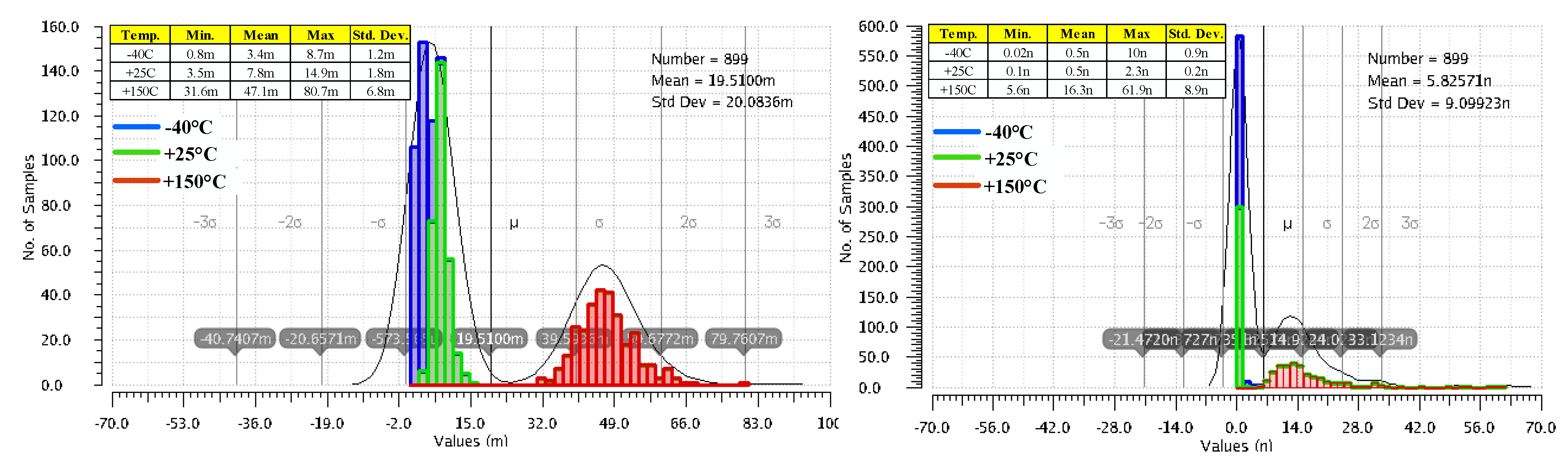
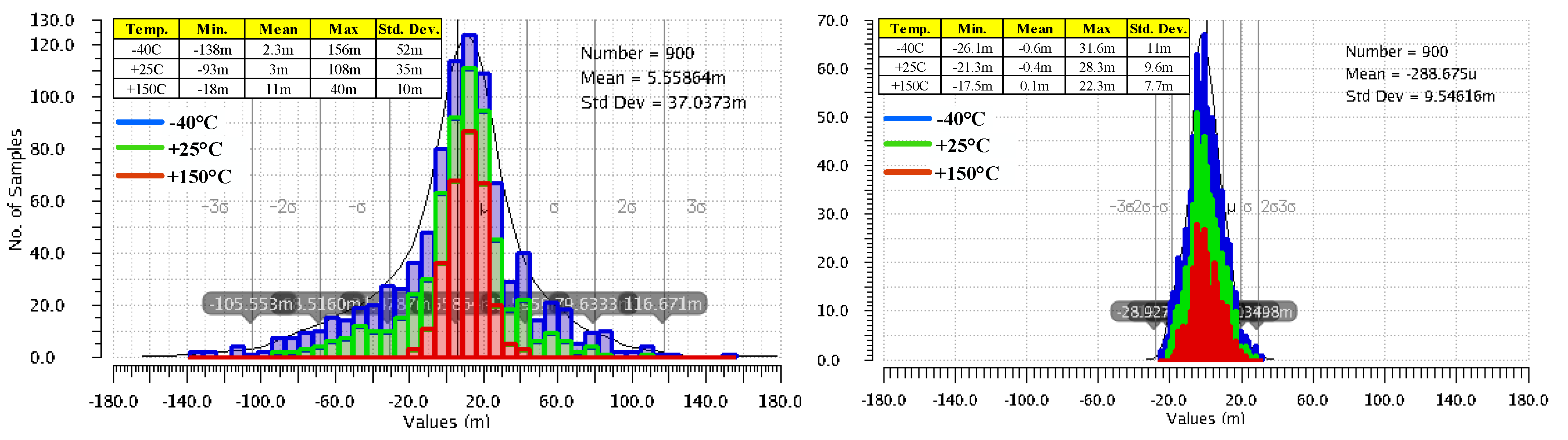
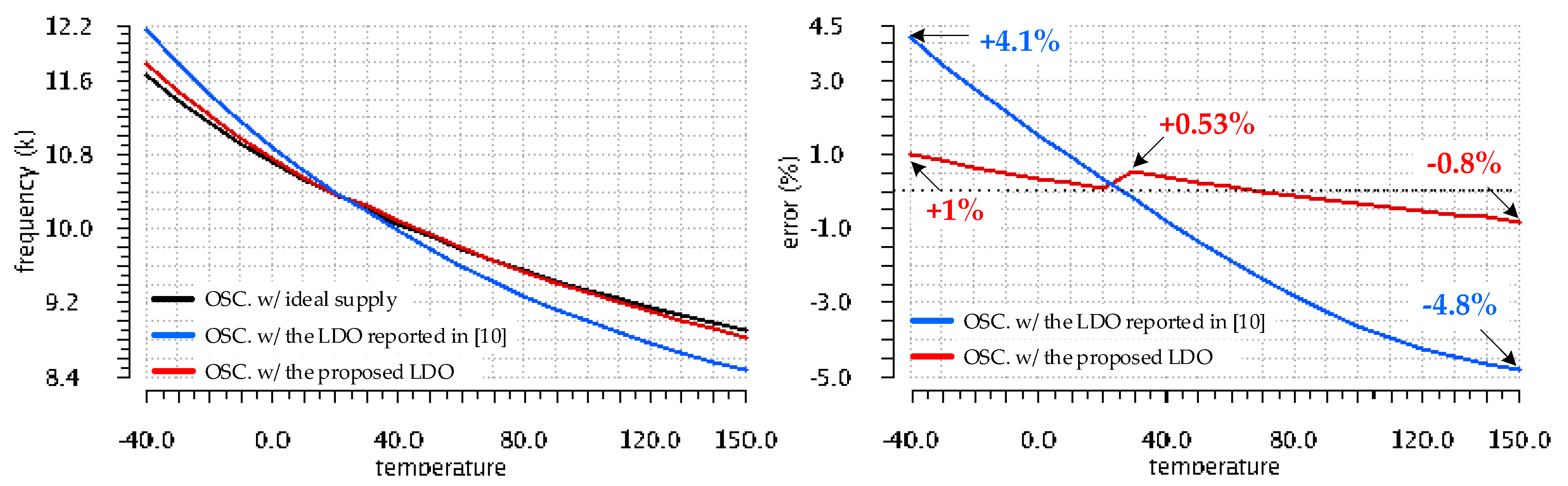
3.3. Simulation Results for the Temperature-Dependent Oscillator Supplied by the Proposed and Reference LDOs
- (1)
- The VOS variation with temperature of each LDO was monitored over 300 Monte-Carlo runs of DC temperature sweeps.
- (2)
- The worst-case runs that yielded the largest differences between the minimum and maximum VOS values over temperature, were identified.
- (3)
- The corresponding characteristics were shifted by modifying the LDO reference voltage, so that the output voltage reached its nominal value at +25 °C, that is VOS = 0.
- (4)
- The oscillators frequency and error variation with temperature was monitored for each worst-case run identified at step 2.
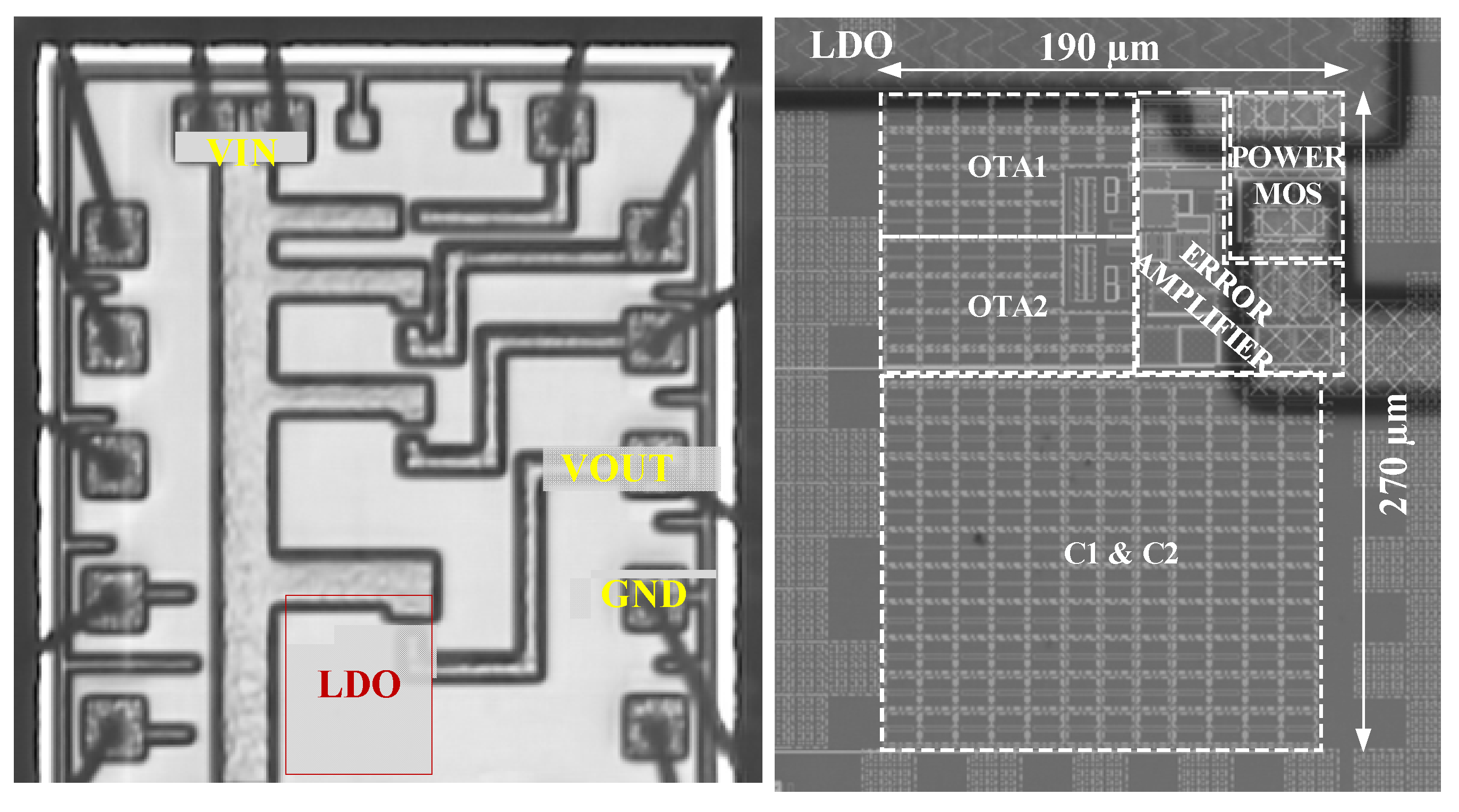
3.4. Silicon Implementation and Measurement Results
4. Comparison with State-of-the-Art and Conclusions
4.1. Comparison with State-of-the-Art
- (a)
- Has the second smallest quiescent current, 0.7 µA more than the LDO in [10].
- (b)
- Has the second-largest DC loop gain, 142 dB. This large value improves the LDO performance measured by several parameters of critical importance for high-precision LDOs:
- -
- First, it helps achieve a very good DC load regulation. The measured load regulation—larger than the simulated value due to voltage drops on the test board tracks—is 1 µ/mA, which is second best to [17].
- -
- (c)
- Is the best in respect to offset voltage: the output voltage error caused by component mismatches, VOS, has a standard deviation of σ = 9.5 mV, 3.8 times lower than the LDO reported in [10].
| Parameter | [36] † | [6] † | [19] †† | [9] † | [35] † | [10] † | This Work |
|---|---|---|---|---|---|---|---|
| Year | 2010 | 2012 | 2016 | 2019 | 2020 | 2020 | 2021 |
| CMOS [μm] | 0.35 | 0.35 | 0.5 | 0.065 | 0.065 | 0.13 | 0.13 |
| FO4Delay(ps) (c) | 90 | 90 | 130 | 17 | 17 | 35 | 35 |
| Supply Voltage [V] | 2.4–3.3 | 2.5–4 | 2.3–5.5 | 0.95–1.2 | 0.95–1.2 | 1.2–1.5 | 1.25–1.5 |
| CL [F] | 100 p | 0–100 p | 0–2.2 n | 0–100 p | 0–100 p | 0–1μ | 0–400 p |
| DC | |||||||
| Output Voltage [V] | 2.2 | 2.3 | 1.2–5.4 | 0.8 | 0.8 | 1 | 1 |
| Output current range (ILMIN–ILMAX) | 0–100 mA | 50μ–100 mA | 0–150 mA | 0–100 mA | 0–100 mA | 0–100 mA | 0–100 mA |
| Iq [μA] | 31 | 7 | 40 | 13.9 | 14 | 0.7 | 1.4 |
| Dropout Voltage [mV] | 200 | 150 | 100 | 150 | 150 | 100 | 150 |
| DC line reg. [mV/V] | 623 | 1 | 0.028 (e) | 0.48 | 12 | 16.6 | 3.3 |
| DC load reg. [μV/mA] | 2.31 | 80 | 0.5 (e) | 8.03 | 90 | 100 | 1 |
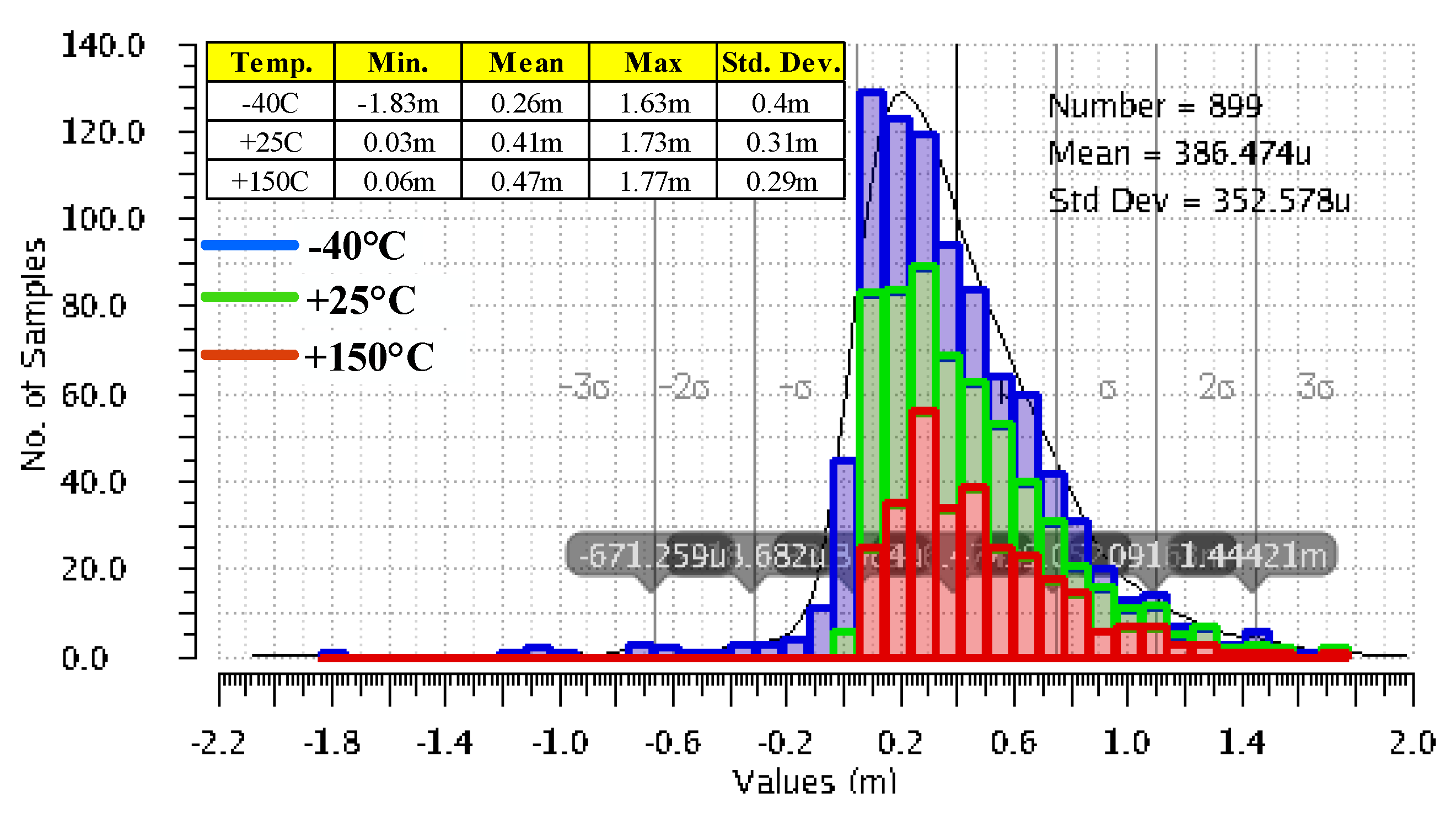
| VOS (3 σ) @ room temp | – | – | – | – | – | 105 mV * | 28.8 mV * |
| VOS thermal drift (post-trim) | – | – | – | – | – | +83.3 mV * −91.4 mV | +12 mV * −14.4 mV * |
| AC * and STB * | |||||||
| Loop gain @ DC [dB] | – | – | 159 | 62 | 71 | 80 | 142 |
| Min. phase margin @ CL = 0 and room temp | – | – | 83° | 41° | 52° (b) | 10° | 40° |
| UGF [Hz] @ IL = 0 A | – | – | 2 M | 1 M | 0.66 M (b) | 72 k | 20 k |
| UGF [Hz] @ IL = ILmax | – | – | 3 M | 10 M | 9 M (b) | 233 k | 20 k |
| PSR [dB] |
60@1 kHz * 40@10 kHz * | – |
65@1 kHz * (a) 65@10 kHz * (a) |
65@1 kHz * (a) 47@10 kHz * |
34@1 kHz * 33@10 kHz * |
50@1 kHz * 30@10 kHz * |
85@1 kHz * 41@10 kHz * |
| Response to load steps | |||||||
| Load step | |||||||
| ILMIN–ILMAX//Avg. IL trise | 0–100 mA/1000 ns | 50 μ–100 mA/500 ns | 0–150 mA/1000 ns | 0–100 mA/50 ns | 0–100 mA/132.5 ns | 0–100 mA/1000 ns | 0–100 mA/1000 ns |
| Rise time ratio (K) | 20 | 10 | 20 | 1 | 2.65 | 20 | 20 |
| Undershoot [mV] | 65.1 | 236 (b) | 106 | 404 | 230 | 76 | 100 (d) |
| Overshoot [mV] | 67 | 227 (b) | 115 | 145 | 133 | 198 | 221 (d) |
| [mV] (Undershoot + Overshoot) | 132 | 463 | 221 | 549 | 363 | 274 | 321 |
| FOM1 [fs] | 40.95 | 33.03 | 3.93 ** | 7.63 ** | 50.82 | 0.19 ** | 0.45 ** |
| FOM2 [mV] | 0.82 | 0.33 | 1.18 | 0.08 | 0.13 | 0.04 | 0.09 |
| FOM3 [V/μs] | 1.24 | 6.38 | 1.23 | 4.49 | 4.14 | 0.15 | 0.35 |
- -
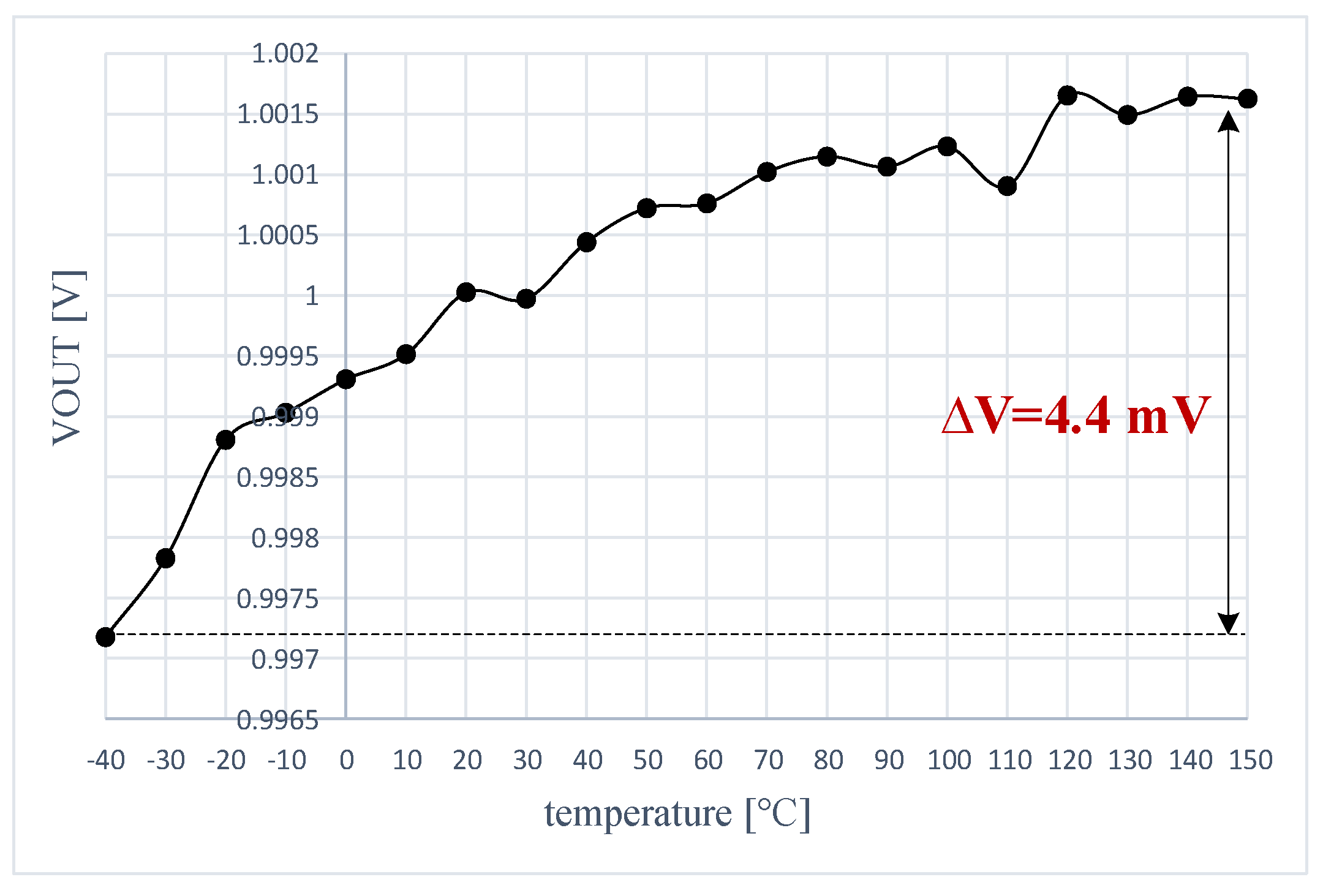
- Its output voltage error caused by component mismatches and variations of the line voltage, load current, and temperature is less than +/−35 mV. The LDO reported in [10] exhibits a DC offset of about 100 mV, with a temperature drift over 150 mV;
- -
- The thermal drift of the output voltage offset caused by component mismatches, across the temperature range of −40 °C to +150 °C, is 9.5 smaller for our LDO than the one provided by the LDO in [10], which was integrated in the same process.
4.2. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Texas Instruments. The Engineer’s Guide to Temperature Sensing; SLYY161; Texas Instruments: Dallas, TX, USA, 2019. [Google Scholar]
- Baker, B. Microchip—Temperature Sensing Technology Review; DS00679A; Appl. Note; Microchip Technology Incorporated: Chandler, AZ, USA, 1998. [Google Scholar]
- Byun, S. Categorization and Characterization of Time Domain CMOS Temperature Sensors. Sensors 2020, 20, 6700. [Google Scholar] [CrossRef]
- Man, T.Y.; Mok, P.K.T.; Chan, M. A High Slew-Rate PushPull Output Amplifier for Low-Quiescent Current Low-Dropout Regulators with Transient-Response Improvement. IEEE Trans. Circuits Syst. II Express Briefs 2007, 54, 755–759. [Google Scholar] [CrossRef]
- Ming, X.; Zhou, Z.K.; Zhang, B. A low-power ultra-fast capacitor-less LDO with advanced dynamic push-pull techniques. In Proceedings of the IFIP/IEEE International Conference on Very Large Scale Integration-System on a Chip, Patras, Greece, 3–5 October 2011; pp. 54–59. [Google Scholar]
- Ming, X.; Li, Q.; Zhou, Z.-K.; Zhang, B. An Ultrafast Adaptively Biased Capacitorless LDO with Dynamic Charging Control. IEEE Trans. Circuits Syst. II Express Briefs 2011, 59, 40–44. [Google Scholar] [CrossRef]
- Raducan, C.; Neag, M. Capacitorless LDO with fast transient response based on a high slew-rate error amplifier. In Proceedings of the 2015 International Semiconductor Conference (CAS), Sinaia, Romania, 12–14 October 2015; pp. 285–288. [Google Scholar] [CrossRef]
- Desai, C.; Mandal, D.; Bakkaloglu, B.; Kiaei, S. A 1.66 mV FOM Output Cap-Less LDO With Current-Reused Dynamic Biasing and 20 ns Settling Time. IEEE Solid-State Circuits Lett. 2018, 1, 50–53. [Google Scholar] [CrossRef]
- Qian, H.; Guo, J. A 1.26-ps-FoM Output-Capacitorless LDO with Dual-Path Active-Feeback Frequency Compensation and Current-Reused Dynamic Biasing in 65-nm CMOS Technology. In Proceedings of the IEEE 13th International Conference on ASIC (ASICON), Chongqing, China, 29 October–1 November 2019; pp. 1–4. [Google Scholar]
- Raducan, C.; Grajdeanu, A.-T.; Plesa, C.-S.; Neag, M.; Negoita, A.; Topa, M.D. LDO With Improved Common Gate Class-AB OTA Handles any Load Capacitors and Provides Fast Response to Load Transients. IEEE Trans. Circuits Syst. I Regul. Pap. 2020, 67, 3740–3752. [Google Scholar] [CrossRef]
- Saberkari, A.; Fathipour, R.; Martínez, H.; Poveda, A.; Alarcón, E. Output-Capacitorless CMOS LDO Regulator Based on High Slew-Rate Current-Mode Transconductance Amplifier. In Proceedings of the 2013 IEEE International Symposium on Circuits and Systems (ISCAS), Beijing, China, 19–23 May 2013; pp. 1484–1487. [Google Scholar]
- Chi, S.S.; Hu, W.; Fan, M.H.; Xu, Y.S.; Chen, G.L. Transient Response Enhancement with Fast Transient Controller for Capacitor-Less LDO Regulator. Appl. Mech. Mater. 2014, 543–547, 800–805. [Google Scholar] [CrossRef]
- Lim, C.-C.; Lai, N.-S.; Tan, G.-H.; Ramiah, H. A low-power fast transient output capacitor-free adaptively biased LDO based on slew rate enhancement for SoC applications. Microelectron. J. 2015, 46, 740–749. [Google Scholar] [CrossRef]
- Dong, L.; Zhao, X.; Wang, Y. Design of an Adaptively Biased Low-Dropout Regulator with a Current Reusing Cur-rent-Mode OTA Using an Intuitive Analysis Method. IEEE Trans. Power Electron. 2020, 35, 10477–10488. [Google Scholar] [CrossRef]
- Pérez-Bailón, J.; Márquez, A.; Calvo, B.; Medrano, N. A 0.18 µm CMOS LDO regulator for an on-chip sensor array impedance measurement system. Sensors 2018, 18, 1405. [Google Scholar] [CrossRef] [Green Version]
- Tang, X.; He, L. Capacitor-free, fast transient response CMOS low-dropout regulator with multiple-loop control. In Proceedings of the 2011 9th IEEE International Conference on ASIC, Xiamen, China, 25 October 2011; pp. 104–107. [Google Scholar] [CrossRef]
- Coulot, T.; Lauga-Larroze, E.; Fournier, J.-M.; Alamir, M.; Hasbani, F. Stability Analysis and Design Procedure of Multiloop Linear LDO Regulators via State Matrix Decomposition. IEEE Trans. Power Electron. 2013, 28, 5352–5363. [Google Scholar] [CrossRef]
- Han, W.; Maomao, S. A dynamic-biased dual-loop-feedback CMOS LDO regulator with fast transient response. J. Semicond. 2014, 35, 045005. [Google Scholar]
- Hong, S.W.; Cho, G.H. High-Gain Wide-Bandwidth Capacitor-Less Low-Dropout Regulator (LDO) for Mobile Applications Utilizing Frequency Response of Multiple Feedback Loops. IEEE Trans. Circuits Syst. I Regul. Pap. 2016, 63, 46–57. [Google Scholar]
- Bode, H.W. Network Analysis Feedback Amplifier Design; D. Van Nostrand Company, Inc.: Princeton, NJ, USA, 1945. [Google Scholar]
- Rosenstark, S. Loop gain measurement in feedback amplifiers. Int. J. Electron. 1984, 57, 415–421. [Google Scholar] [CrossRef]
- Neag, M.; Onet, R.; Kovacs, I.; Martari, P. Comparative Analysis of Simulation-Based Methods for Deriving the Phase- and Gain-Margins of Feedback Circuits with Op-Amps. IEEE Trans. Circuits Syst. I Regul. Pap. 2014, 62, 625–634. [Google Scholar] [CrossRef]
- Ahuja, B. An improved frequency compensation technique for CMOS operational amplifiers. IEEE J. Solid-State Circuits 1983, 18, 629–633. [Google Scholar] [CrossRef]
- Assaad, R.S.; Silva-Martinez, J. The Recycling Folded Cascode: A General Enhancement of the Folded Cascode Amplifier. IEEE J. Solid-State Circuits 2009, 44, 2535–2542. [Google Scholar] [CrossRef]
- Ramirez-Angulo, J.; Holmes, M. Simple technique using local CMFB to enhance slew rate and bandwidth of one-stage CMOS op-amps. Electron. Lett. 2002, 38, 1409–1411. [Google Scholar] [CrossRef]
- Law, M.K.; Bermak, A.; Luong, H.C. A Sub-μW Embedded CMOS Temperature Sensor for RFID Food Monitoring Application. IEEE J. Solid-State Circuits 2010, 45, 1246–1255. [Google Scholar] [CrossRef]
- Ha, D.; Woo, K.; Meninger, S.; Xanthopoulos, T.; Crain, E.; Ham, D. Time-Domain CMOS Temperature Sensors with Dual Delay-Locked Loops for Microprocessor Thermal Monitoring. IEEE Trans. Very Large Scale Integr. Syst. 2012, 20, 1590–1601. [Google Scholar] [CrossRef]
- Hwang, S.; Koo, J.; Kim, K.; Lee, H.; Kim, C. A 0.008 mm2 500 μw 469 kS/s frequency-to-digital converter based CMOS temperature sensor with process variation compensation. IEEE Trans. Circuits Syst. I Regul. Pap. 2013, 60, 2241–2248. [Google Scholar] [CrossRef]
- Kim, K.; Lee, H.; Kim, C. 366-kS/s 1.09-nJ 0.0013-m mm2 frequency-to-Digital converter based CMOS temperature sensor utilizing multiphase clock. IEEE Trans. Very Large Scale Integr. Syst. 2013, 21, 1950–1954. [Google Scholar] [CrossRef]
- An, Y.-J.; Ryu, K.; Jung, D.-H.; Woo, S.-H.; Jung, S.-O. An Energy Efficient Time-Domain Temperature Sensor for Low-Power On-Chip Thermal Management. IEEE Sensors J. 2014, 14, 104–110. [Google Scholar] [CrossRef]
- Jeong, S.; Foo, Z.; Lee, Y.; Sim, J.-Y.; Blaauw, D.; Sylvester, D. A Fully-Integrated 71 nW CMOS Temperature Sensor for Low Power Wireless Sensor Nodes. IEEE J. Solid-State Circuits 2014, 49, 1682–1693. [Google Scholar] [CrossRef]
- Deng, F.; He, Y.; Lihua, Z.; Zhang, L.; Wu, X.; Fu, Z.; Zuo, L. Design of an Embedded CMOS Temperature Sensor for Passive RFID Tag Chips. Sensors 2015, 15, 11442–11453. [Google Scholar] [CrossRef]
- Chen, P.; Chen, T.-K.; Wang, Y.-S.; Chen, C.-C. A Time-Domain Sub-Micro Watt Temperature Sensor with Digital Set-Point Programming. IEEE Sensors J. 2009, 9, 1639–1646. [Google Scholar] [CrossRef]
- Beleca, V.; Plesa, C.-S.; Onet, R.; Neag, M. A novel approach to the stability analysis of conditionally stable circuits. In Proceedings of the 2021 International Semiconductor Conference (CAS), Online, 6–8 October 2021; pp. 223–226. [Google Scholar] [CrossRef]
- Li, G.; Qian, H.; Guo, J.; Mo, B.; Lu, Y.; Chen, D. Dual Active-Feedback Frequency Compensation for Output-Capacitorless LDO with Transient and Stability Enhancement in 65-nm CMOS. IEEE Trans. Power Electron. 2020, 35, 415–429. [Google Scholar] [CrossRef]
- Ho, E.N.Y.; Mok, P.K.T. A chip-area efficient capacitor-less CMOS LDO with active feedback and damping zero compensation. In Proceedings of the 2010 53rd IEEE International Midwest Symposium on Circuits and Systems, Washington, DC, USA, 1–4 August 2010; pp. 574–577. [Google Scholar] [CrossRef]
- Hazucha, P.; Karnik, T.; Bloechel, B.; Parsons, C.; Finan, D.; Borkar, S. An area-efficient, integrated, linear regulator with ultra-fast load regulation. IEEE Symp. VLSI Circuits Dig. Tech. Pap. 2004, 40, 218–221. [Google Scholar]
- Guo, J.; Leung, K.N. A 6-μW chip-area-efficient output-capacitorless LDO in 90-nm CMOS technology. IEEE J. Solid-State Circuits 2010, 45, 1896–1905. [Google Scholar] [CrossRef]
- Furth, P.M.; Krishnapurapu, S.; Pakala, S.H.; Haque, M.A. A 5.3 μA quiescent current fully-integrated low-dropout (LDO) regulator with Transient Recovery Time Enhancement. In Proceedings of the 2013 IEEE 56th International Midwest Symposium on Circuits and Systems (MWSCAS), Columbus, OH, USA, 3–7 August 2013; pp. 9–12. [Google Scholar]











| T = 25 °C | Min | Mean | Max | Std Dev |
|---|---|---|---|---|
| f0(T) [kHz] | 8.49 | 9.99 | 11.4 | 0.5294 |
| f1(T) [kHz] | 8.42 | 10 | 11.3 | 0.5375 |
| f2(T) [kHz] | 8.03 | 9.95 | 11.7 | 0.7024 |
| f1(T) error wrt f0(T) [%] | −4.414 | 0.2 | 3.199 | 1.273 |
| f2(T) error wrt f0(T) [%] | −15.36 | −0.39 | 12.16 | 4.79 |
| T [°C] | Worst Case VOS Temp. Drift for the LDO Reported in [10] | Worst Case VOS Temp. Drift for the Proposed LDO | ||
|---|---|---|---|---|
| Error f1(T) [%] | Error f2(T) [%] | Error f1(T) [%] | Error f2(T) [%] | |
| −40 | 0.34 | 6.50 | 1.01 | 4.15 |
| −20 | 0.25 | 4.40 | 0.66 | 2.79 |
| 0 | 0.15 | 2.47 | 0.36 | 1.54 |
| 20 | 0.04 | 0.62 | 0.08 | 0.36 |
| 40 | 0.66 | −1.23 | 0.39 | −0.76 |
| 60 | 0.53 | −2.99 | 0.13 | −1.87 |
| 80 | 0.45 | −4.58 | −0.10 | −2.85 |
| 100 | 0.40 | −5.85 | −0.32 | −3.63 |
| 120 | 0.35 | −6.85 | −0.51 | −4.24 |
| 140 | 0.30 | −7.69 | −0.71 | −4.69 |
| 150 | 0.25 | −8.07 | −0.82 | −4.84 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Răducan, C.; Neag, M.; Grăjdeanu, A.; Țopa, M.; Negoiță, A. High-Precision Low-Temperature Drift LDO Regulator Tailored for Time-Domain Temperature Sensors. Sensors 2022, 22, 1518. https://doi.org/10.3390/s22041518
Răducan C, Neag M, Grăjdeanu A, Țopa M, Negoiță A. High-Precision Low-Temperature Drift LDO Regulator Tailored for Time-Domain Temperature Sensors. Sensors. 2022; 22(4):1518. https://doi.org/10.3390/s22041518
Chicago/Turabian StyleRăducan, Cristian, Marius Neag, Alina Grăjdeanu, Marina Țopa, and Andrei Negoiță. 2022. "High-Precision Low-Temperature Drift LDO Regulator Tailored for Time-Domain Temperature Sensors" Sensors 22, no. 4: 1518. https://doi.org/10.3390/s22041518
APA StyleRăducan, C., Neag, M., Grăjdeanu, A., Țopa, M., & Negoiță, A. (2022). High-Precision Low-Temperature Drift LDO Regulator Tailored for Time-Domain Temperature Sensors. Sensors, 22(4), 1518. https://doi.org/10.3390/s22041518






