Piezoresistive Conductive Microfluidic Membranes for Low-Cost On-Chip Pressure and Flow Sensing
Abstract
:1. Introduction
2. Experimental
2.1. Working Principle of Piezoresistive Sensor
2.2. Sensor Microfabrication
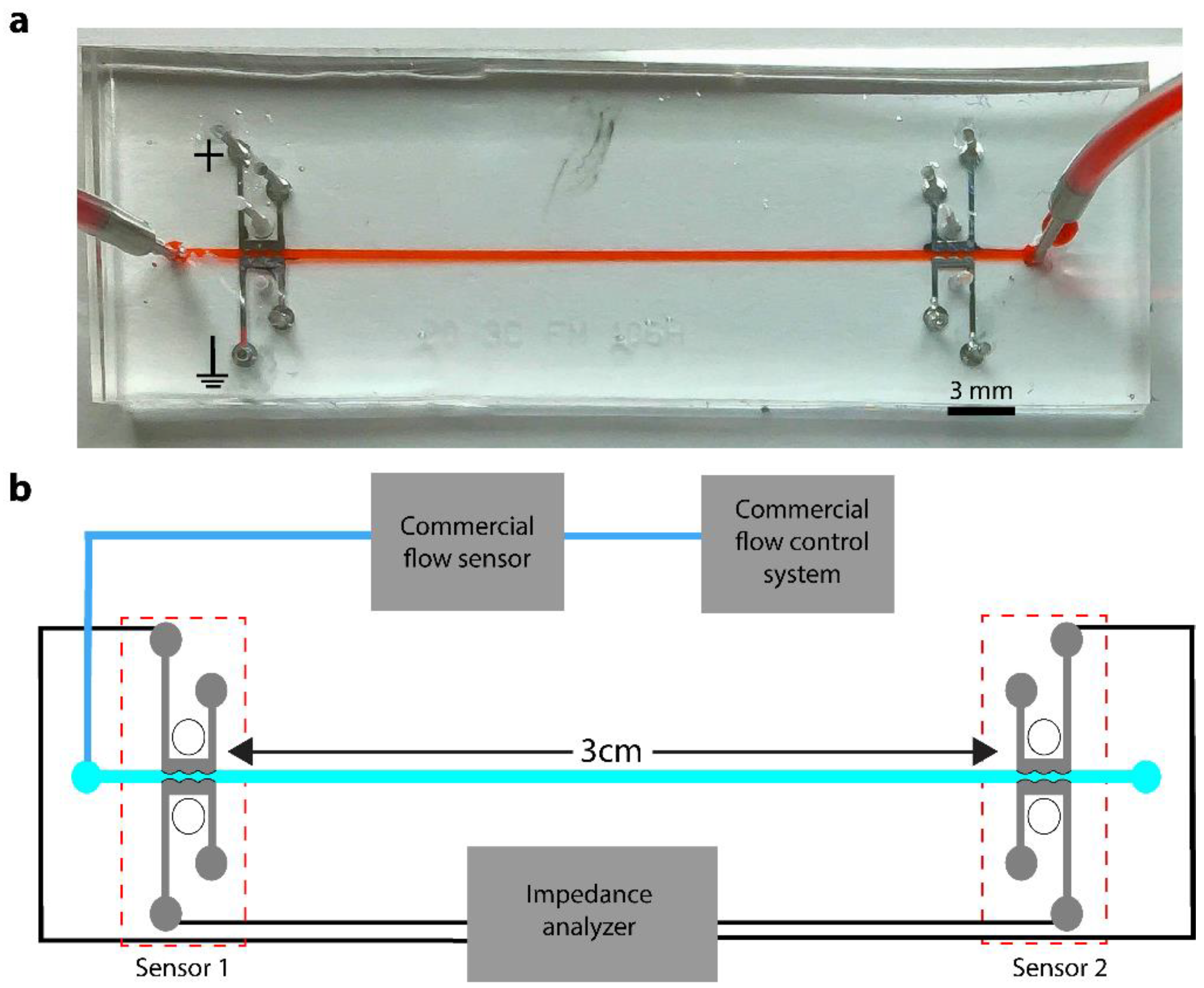
2.3. Device Experimental Setup and Operation
3. Results and Discussion
3.1. Sensor Impedance Response
3.2. Finite Element Modelling
3.3. EIS Equivalent Circuit Model
3.4. Impedance Response to Membrane Deformation
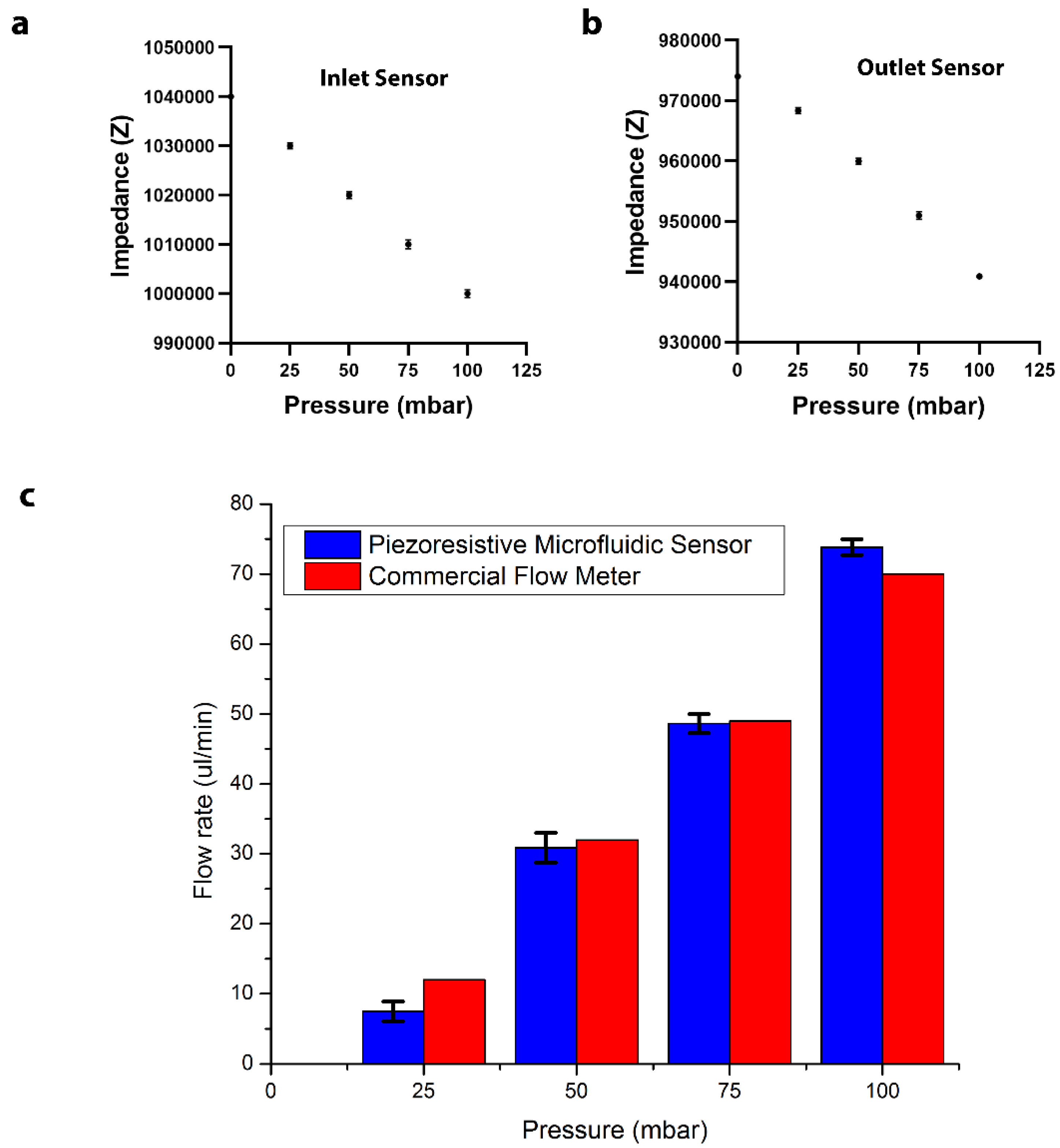
3.5. Flow Rate Measurement
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Seo, M.; Paquet, C.; Nie, Z.; Xu, S.; Kumacheva, E. Microfluidic consecutive flow-focusing droplet generators. Soft Matter 2007, 3, 986–992. [Google Scholar] [CrossRef]
- Gagnon, Z.; Mazur, J.; Chang, H.C. Integrated AC electrokinetic cell separation in a closed-loop device. Lab Chip 2010, 10, 718–726. [Google Scholar] [CrossRef] [PubMed]
- Gagnon, Z.R. Cellular dielectrophoresis: Applications to the characterization, manipulation, separation and patterning of cells. Electrophoresis 2011, 32, 2466–2487. [Google Scholar] [CrossRef]
- Fu, X.; Mavrogiannis, N.; Ibo, M.; Crivellari, F.; Gagnon, Z.R. Microfluidic free-flow zone electrophoresis and isotachophoresis using carbon black nano-composite PDMS sidewall membranes. Electrophoresis 2017, 38, 327–334. [Google Scholar] [CrossRef] [PubMed]
- Kohl, M.J.; Abdel-Khalik, S.I.; Jeter, S.M.; Sadowski, D.L. A microfluidic experimental platform with internal pressure measurements. Sens. Actuators A Phys. 2005, 118, 212–221. [Google Scholar] [CrossRef]
- Song, W.; Psaltis, D. Optofluidic membrane interferometer: An imaging method for measuring microfluidic pressure and flow rate simultaneously on a chip. Biomicrofluidics 2011, 5, 044110. [Google Scholar] [CrossRef] [Green Version]
- Hoera, C.; Kiontke, A.; Pahl, M.; Belder, D. A chip-integrated optical microfluidic pressure sensor. Sens. Actuators B Chem. 2018, 255, 2407–2415. [Google Scholar] [CrossRef]
- Li, H.; Luo, C.X.; Ji, H.; Ouyang, Q.; Chen, Y. Micro-pressure sensor made of conductive PDMS for microfluidic applications. Microelectron. Eng. 2010, 87, 1266–1269. [Google Scholar] [CrossRef]
- Wang, Z.; Tan, L.; Pan, X.; Liu, G.; He, Y.; Jin, W.; Li, M.; Hu, Y.; Gu, H. Self-Powered Viscosity and Pressure Sensing in Microfluidic Systems Based on the Piezoelectric Energy Harvesting of Flowing Droplets. ACS Appl. Mater. Interfaces 2017, 9, 28586–28595. [Google Scholar] [CrossRef]
- Wu, C.Y.; Liao, W.H.; Tung, Y.C. Integrated ionic liquid-based electrofluidic circuits for pressure sensing within polydimethylsiloxane microfluidic systems. Lab Chip 2011, 11, 1740–1746. [Google Scholar] [CrossRef]
- Jung, T.; Yang, S. Highly stable liquid metal-based pressure sensor integrated with a microfluidic channel. Sensors 2015, 15, 11823–11835. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Peng, K.; Yao, J.; Cho, S.; Cho, Y.; Kim, H.S.; Park, J. Liquid metal embedded real time microfluidic flow pressure monitoring sensor. Sens. Actuators A Phys. 2020, 305, 111909. [Google Scholar] [CrossRef]
- Fu, X.; Gagnon, Z. Contactless microfluidic pumping using microchannel-integrated carbon black composite membranes. Biomicrofluidics 2015, 9, 054122. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Dogru, S.; Aksoy, B.; Bayraktar, H.; Alaca, B.E. Poisson’s ratio of PDMS thin films. Polym. Test. 2018, 69, 375–384. [Google Scholar] [CrossRef]
- MichelleáGrandin, H. Micro-well arrays for 3D shape control and high resolution analysis of single cells. Lab Chip 2007, 7, 1074–1077. [Google Scholar]
- Wang, J.C. Realizations of Generalized Warburg Impedance with RC Ladder Networks and Transmission Lines. J. Electrochem. Soc. 1987, 134, 1915. [Google Scholar] [CrossRef]
- Bobacka, J.; Lewenstam, A.; Ivaska, A. Electrochemical impedance spectroscopy of oxidized poly(3,4-ethylenedioxythiophene) film electrodes in aqueous solutions. J. Electroanal. Chem. 2000, 489, 17–27. [Google Scholar] [CrossRef]
- Matysik, S.; Matysik, F.M.; Schulze, K.D.; Einicke, W.D. Impedance spectroscopic investigations of zeolite–polydimethylsiloxane electrodes. Electrochim. Acta 2002, 48, 297–301. [Google Scholar] [CrossRef]
- Scribner Associates, I.Z. View Impedance/Gain Phase Graphing and Analysis Software Operating Manual, Version 3.5. Available online: https://www.scribner.com/software/68-general-electrochemistr376-zview-for-windows/ (accessed on 10 February 2022).
- Jurado, L.A.; Espinosa-Marzal, R.M. Insight into the Electrical Double Layer of an Ionic Liquid on Graphene. Sci. Rep. 2017, 7, 4225. [Google Scholar] [CrossRef]
- Chaparro, C.V.; Herrera, L.V.; Meléndez, A.M.; Miranda, D.A. Considerations on electrical impedance measurements of electrolyte solutions in a four-electrode cell. J. Phys. Conf. Ser. 2016, 687, 012101. [Google Scholar] [CrossRef] [Green Version]
- Ginter, G.; Gasser, J.G.; Kleim, R. The electrical resistivity of liquid bismuth, gallium and bismuth-gallium alloys. Philos. Mag. B 2006, 54, 543–552. [Google Scholar] [CrossRef]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Islam, M.N.; Doria, S.M.; Fu, X.; Gagnon, Z.R. Piezoresistive Conductive Microfluidic Membranes for Low-Cost On-Chip Pressure and Flow Sensing. Sensors 2022, 22, 1489. https://doi.org/10.3390/s22041489
Islam MN, Doria SM, Fu X, Gagnon ZR. Piezoresistive Conductive Microfluidic Membranes for Low-Cost On-Chip Pressure and Flow Sensing. Sensors. 2022; 22(4):1489. https://doi.org/10.3390/s22041489
Chicago/Turabian StyleIslam, Md. Nazibul, Steven M. Doria, Xiaotong Fu, and Zachary R. Gagnon. 2022. "Piezoresistive Conductive Microfluidic Membranes for Low-Cost On-Chip Pressure and Flow Sensing" Sensors 22, no. 4: 1489. https://doi.org/10.3390/s22041489
APA StyleIslam, M. N., Doria, S. M., Fu, X., & Gagnon, Z. R. (2022). Piezoresistive Conductive Microfluidic Membranes for Low-Cost On-Chip Pressure and Flow Sensing. Sensors, 22(4), 1489. https://doi.org/10.3390/s22041489




