Potentiometric Chemical Sensors Based on Metal Halide Doped Chalcogenide Glasses for Sodium Detection
Abstract
:1. Introduction
2. Materials and Methods
2.1. Glass Synthesis
2.2. Glass Characterization
2.3. Na+ Ion Selective Electrodes Fabrication
2.4. Sensors Calibration and Analytical Parameters Measurements
3. Results
3.1. Glass Forming Ability, Moisture Resistance and Physico-Chemical Properties of the AgI-NaI-Ga2S3-GeS2 Glasses
3.2. Analytical Characterization of the Sodium Sensors
3.2.1. Slope, Linearity and Detection Limit
3.2.2. Selectivity Coefficient KNa+,My+ in Standard Solutions
3.2.3. PH Influence
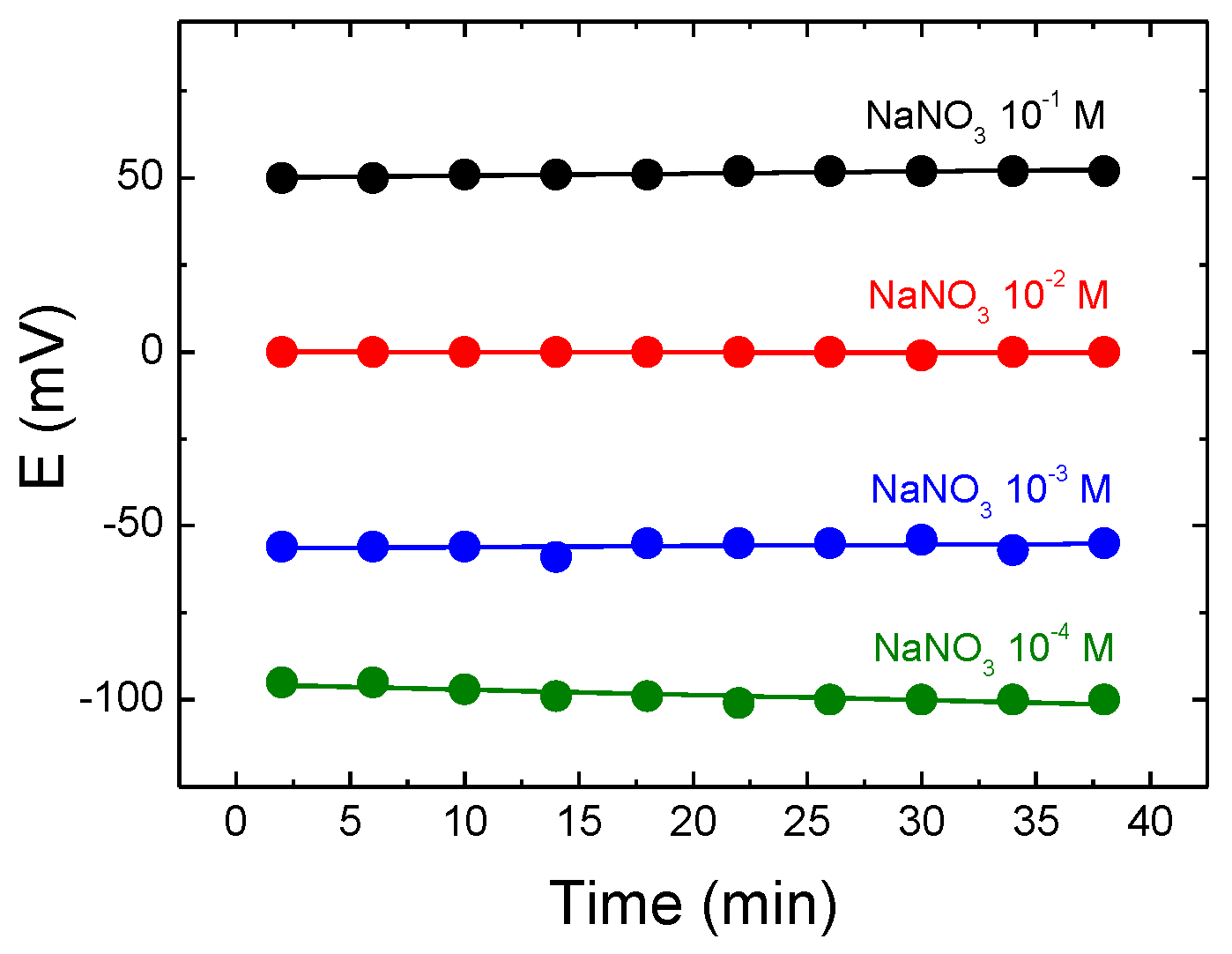
3.2.4. Reproducibility of the Electrode Potential
4. Discussion
4.1. Mixed Cation Effect Phenomenon in the (AgI)x(NaI)30−x(Ga2S3)26(GeS2)44 Glasses
4.2. The Performance of the Sodium Sensors with Different Membrane Compositions
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Vlasov, Y.G.; Bychkov, E.A. Ion-selective chalcogenide glass electrodes. Ion-Sel. Electrode Rev. 1987, 9, 5–91. [Google Scholar]
- Solsky, R.L. Ion-Selective Electrodes. Anal. Chem. 1988, 60, 106R–113R. [Google Scholar] [CrossRef] [PubMed]
- Bychkov, E.; Tveryanovich, Y.; Vlasov, Y. Chapter 3 Ion conductivity and sensors. In Semiconducting Chalcogenide Glass III, Applications of Chalcogenide Glasses, Semiconductors and Semimetals; Fairman, R., Ushkov, B., Willardson, R.K., Weber, E.R., Eds.; Elsevier Academic Press: Amsterdam, The Netherlands, 2004; Volume 80, pp. 103–168. [Google Scholar]
- Vassilev, V.S.; Boycheva, S.V. Chemical sensors with chalcogenide glassy membranes. Talanta 2005, 67, 20–27. [Google Scholar] [CrossRef]
- Pejcic, B.; De Marco, R. Impedance spectroscopy: Over 35 years of electrochemical sensors optimisation. Electrochim. Acta 2006, 51, 6217–6229. [Google Scholar] [CrossRef]
- De Marco, R.; Clarke, G.; Pejcic, B. Ion-selective electrode potentiometry in environmental analysis. Electroanalysis 2007, 19–20, 1987–2001. [Google Scholar] [CrossRef]
- Schoning, M.J.; Kloock, J.P. About 20 years of silicon-based thin-film sensors with chalcogenide glass materials for heavy metal analysis: Technological aspects of fabrication and miniaturization. Electroanalysis 2007, 19–20, 2029–2038. [Google Scholar] [CrossRef]
- Moreno, T.V.; Malacarne, L.C.; Baesso, M.L.; Qu, W.; Dy, E.; Xie, Z.; Fahlman, J.; Shen, J.; Astrath, N.G.C. Potentiometric sensors with chalcogenide glasses as sensitive membranes: A short review. J. Non-Cryst. Solids 2018, 495, 8–18. [Google Scholar] [CrossRef]
- Borisova, Z.U. Chalcogenide Semiconductors; LGU: Leningrad, Russia, 1983; pp. 1–344. [Google Scholar]
- Vlasov, Y.G.; Bychkov, E.A. Sodium ion-selective chalcogenide glass electrodes. Anal. Lett. 1989, 22, 1125–1144. [Google Scholar] [CrossRef]
- Paraskiva, A. Developpement de Membranes Pour Les Capteurs Chimiques Potentiometriques Specifiques Aux Ions Thallium et Sodium. Ph. D. Thesis, Université du Littoral Côte d’Opale, Dunkerque, France, 2017. [Google Scholar]
- Paraskiva, A.; Bokova, M.; Bychkov, E. Na+ ion conducting glasses in the NaCl-Ga2S3-GeS2 system: A critical percolation regime. Solide State Ionics 2017, 299, 2–7. [Google Scholar] [CrossRef]
- Bokova, M.; Paraskiva, A.; Kassem, M.; Bychkov, E. Alkali halide doped Ga2S3-GeS2 glasses. Phys. Status Solidi B 2020, 257, 2000115. [Google Scholar] [CrossRef]
- Bokova, M.; Paraskiva, A.; Kassem, M.; Bychkov, E. Raman spectra of MCl-Ga2S3-GeS2 (M = Na, K, Rb) glasses. Pure Appl. Chem. 2022, 94, 181–188. [Google Scholar] [CrossRef]
- Thomas, M.P.; Peterson, N.L.; Hutchinson, E. Tracer Diffusion and Electrical Conductivity in Sodium-Rubidium Silicon Sulfide Glasses. J. Am. Ceram. Soc. 1985, 68, 99–104. [Google Scholar] [CrossRef]
- Pradel, A.; Ribes, M. Ion transport in superionic conducting glasses. J. Non-Cryst. Solids 1994, 172–174, 1315–1323. [Google Scholar] [CrossRef]
- Sills, J.A.; Martin, S.W.; Torgeson, D.R. 11B NMR studies of the short range order in mixed alkali Na2S:K2S + B2S3 glasses. J. Non-Cryst. Solids 1996, 194, 260–266. [Google Scholar] [CrossRef]
- Gee, B.; Eckert, H.; Pradel, A.; Taillades, G.; Ribes, M. Alkali ion distribution in mixed-alkali chalcogenide glasses: 23Na-(7Li) spin echo double resonance NMR studies of the system [(Na2S)1-y(Li2S)y]0.5(GeS2)0.5. J. Non-Cryst. Solids 1997, 215, 32–40. [Google Scholar] [CrossRef]
- Bolotov, A.; Bychkov, E.; Gavrilov, Y.; Grushko, Y.; Pradel, A.; Ribes, M.; Tsegelnik, V.; Vlasov, Y. Degenerated mixed cation effect in CuI-AgI-As2Se3 glasses: 64Cu and 110Ag tracer diffusion studies. Solid State Ionics 1998, 113–115, 697–701. [Google Scholar] [CrossRef]
- Rau, C.; Armand, P.; Pradel, A.; Varsamis, C.P.E.; Kamitsos, E.I.; Granier, D.; Ibanez, A.; Philippot, E. Mixed cation effect in chalcogenide glasses Rb2S-Ag2S-GeS2. Phys. Rev. B 2001, 63, 184204. [Google Scholar] [CrossRef]
- Kassem, M.; Le Coq, D.; Bokova, M.; Bychkov, E. Chemical and structural origin of conductivity changes in CdSe-AgI-As2Se3 glasses. Solid State Ionics 2010, 181, 466–472. [Google Scholar] [CrossRef]
- Bokova, M.; Alekseev, I.; Bychkov, E. Mixed cation effect Ag2S-Tl2S-GeS-GeS2 glasses: Conductivity and tracer diffusion studies. Solid State Ionics 2015, 273, 55–58. [Google Scholar] [CrossRef]
- Bokova, M.; Paraskiva, A.; Kassem, M.; Bychkov, E. Mixed cation Ag2S–Tl2S–GeS2 glasses: Macroscopic properties and Raman scattering studies. J. Phys. Condens. Matter 2020, 32, 264004. [Google Scholar] [CrossRef]
- Montain, S.J.; Cheuvront, S.N.; Lukaski, H.C. Sweat mineral-element responses during 7 h of exercise-heat stress. Int. J. Sport Nutr. Exerc. Metab. 2007, 17, 574–582. [Google Scholar] [CrossRef] [PubMed]
- Alrifai, B.; Kassem, M.; Toufaily, J.; Bokova, M.; Bychkov, E. Pb2+ potentiometric chemical sensors based on lead and silver doped thioaresenate glasses. Solid State Sci. 2022, 131, 106955. [Google Scholar] [CrossRef]
- Kuznetsov, S.L.; Mikhailov, M.D.; Pecheritsyn, I.M.; Turkina, E.Y. Structural chemical processes at the synthesis of chalcogenide glasses. J. Non-Cryst. Solids 1997, 213&214, 68–71. [Google Scholar] [CrossRef]
- Dong, G.; Tao, H.; Xiao, X.; Lin, C.; Zhao, X. Formation, thermal, optical and physical properties of GeS2–Ga2S3–AgCl novel chalcohalide glasses. J. Mater. Sci. 2007, 42, 9632–9637. [Google Scholar] [CrossRef]
- Stehlik, S.; Zima, V.; Wagner, T.; Ren, J.; Frumar, M. Conductivity and permittivity study on silver and silver halide doped GeS2–Ga2S3 glassy system. Solid State Ionics 2008, 179, 1867–1875. [Google Scholar] [CrossRef]
- Ren, J.; Yan, Q.; Wagner, T.; Zima, V.; Frumar, M.; Frumarova, B.; Chen, G. Conductivity study on GeS2-Ga2S3-AgI-Ag chalcohalide glasses. J. Appl. Phys. 2013, 114, 023701. [Google Scholar] [CrossRef]
- Sato, H. Some theoretical aspects of solid electrolytes. In Solid Electrolytes; Geller, S., Ed.; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 1977; pp. 3–39. [Google Scholar]
- Eisenman, G. The origin of the glass electrode potential. In Glass Electrodes for Hydrogen and Other Cations; Principles and Practice; Eisenman, G., Ed.; Marcel Dekker: New York, NY, USA, 1967; pp. 133–173. [Google Scholar]
- Milochova, M.; Kassem, M.; Bychkov, E. Chalcogenide Glass Chemical Sensor for Cadmium Detection in Industrial Environment. ECS Trans. 2012, 50, 357–362. [Google Scholar] [CrossRef]
- Isard, J.O. The mixed alkali effect in glass. J. Non-Cryst. Solids 1969, 1, 235–261. [Google Scholar] [CrossRef]
- Day, D.E. Mixed alkali glasses—Their properties and uses. J. Non-Cryst. Solids 1976, 21, 343–372. [Google Scholar] [CrossRef]
- Bunde, A. Anomalous ionic transport in glassy electrolytes. Il Nuovo Cim. 1994, 16D, 1053–1063. [Google Scholar] [CrossRef]
- Kirchheim, R.; Paulmann, D. The relevance of site energy distribution for the mixed alkali effect. J. Non-Cryst. Solids 2001, 286, 210–223. [Google Scholar] [CrossRef]
- Usuki, T.; Saito, S.; Nakajima, K.; Uemura, O.; Kameda, Y.; Kamiyama, T.; Sakurai, M. Structural and electrical properties of AgI dispersed As-chalcogenide glasses. J. Non-Cryst. Solids 2002, 312–314, 570–574. [Google Scholar] [CrossRef]
- Onodera, Y.; Furukawa, T.; Hashimoto, S.; Usuki, T.; Amo, Y.; Kameda, Y. Vitrification and transport properties in AgBr-doped chalcogenide systems. Solid State Ion. 2006, 177, 2597–2599. [Google Scholar] [CrossRef]
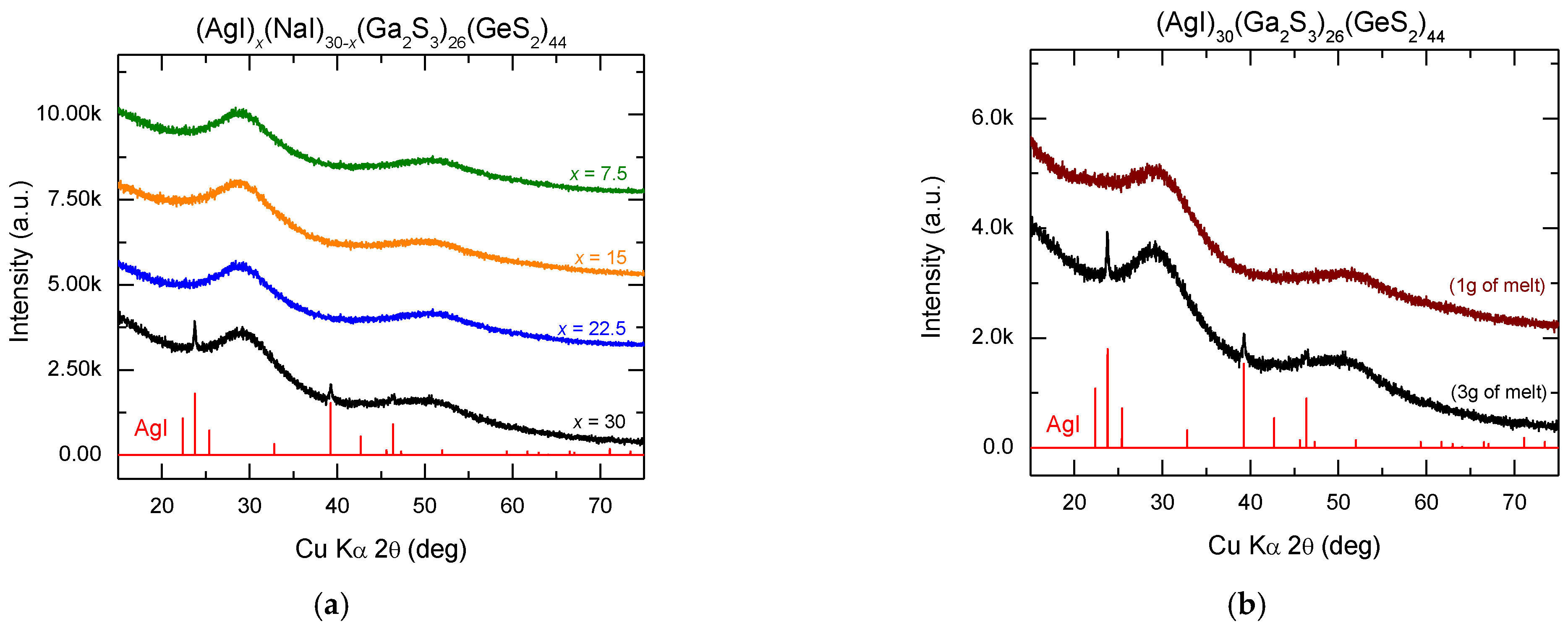

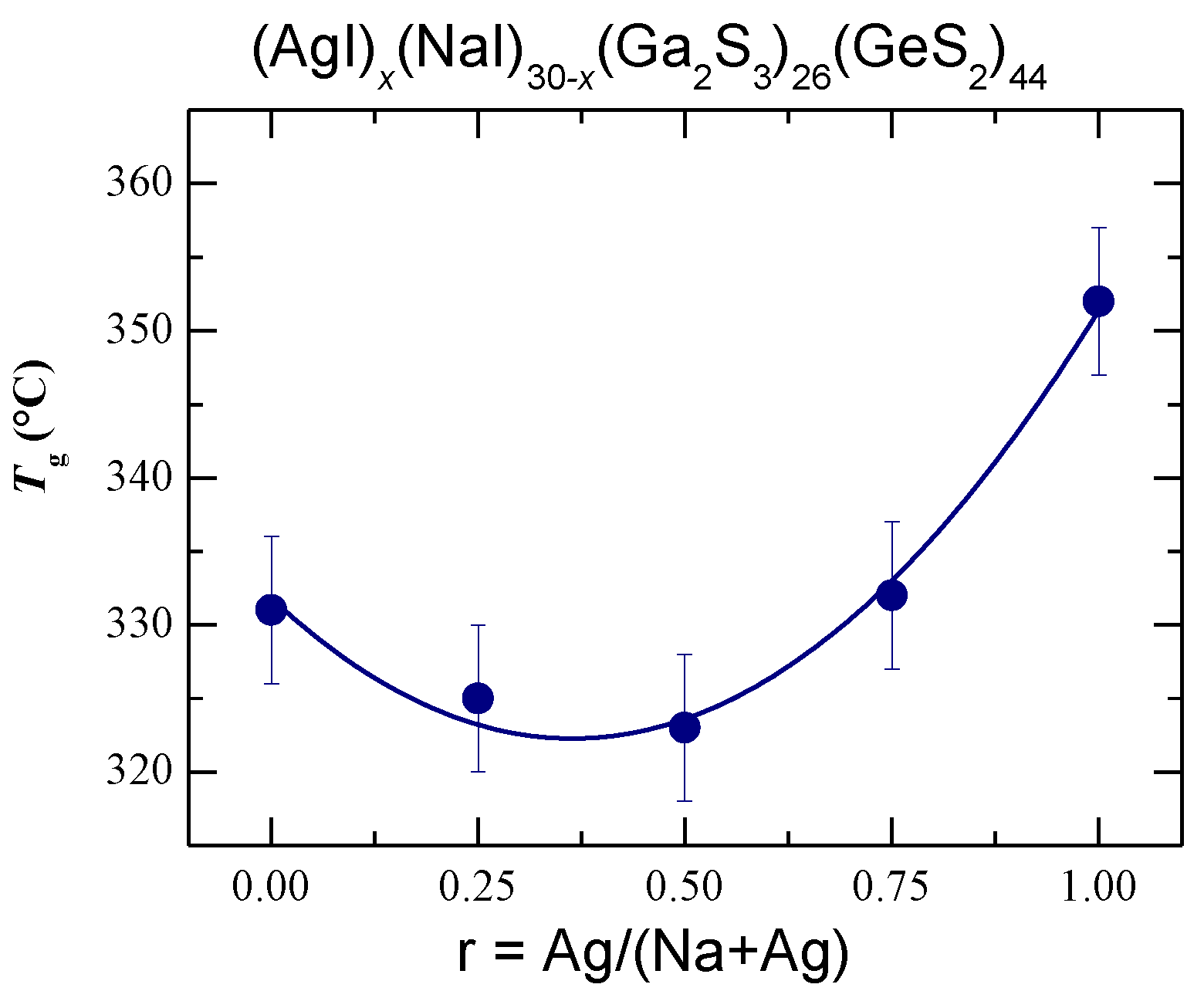

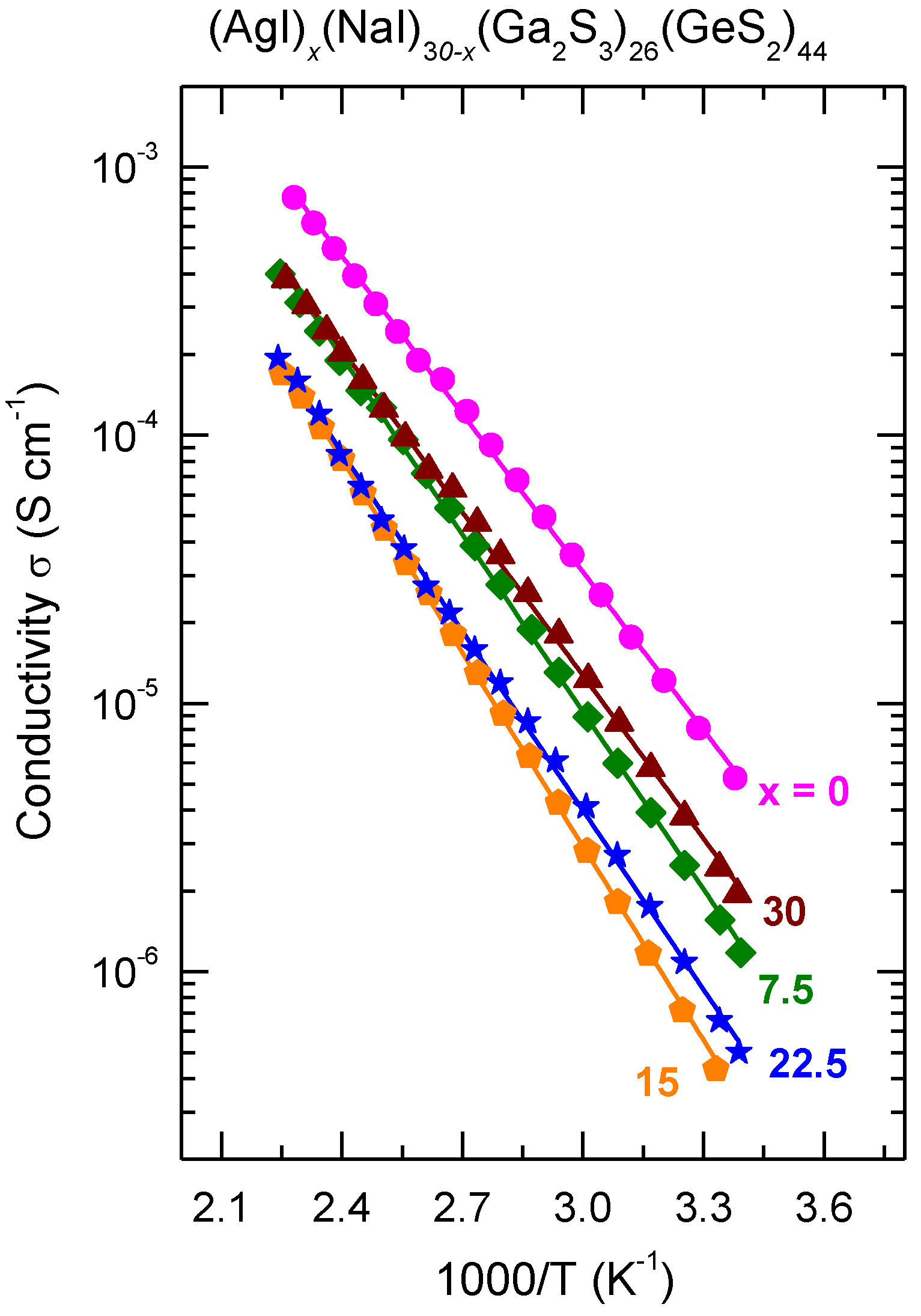
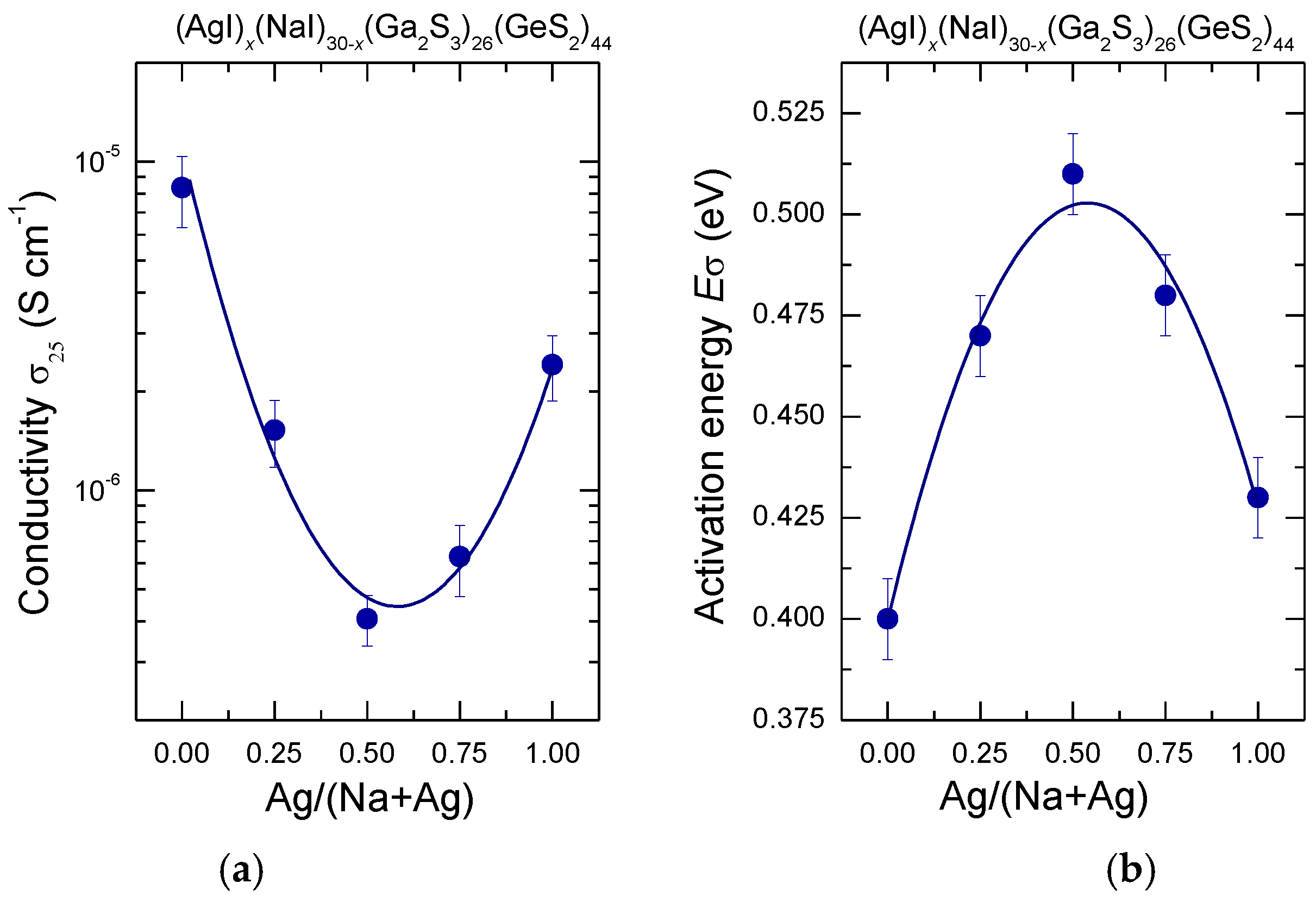


| Glass Composition | d (g cm−3) | Tg (°C) | log σ25 (S cm−1) | Eσ (eV) | log A (S cm−1 K) | |
|---|---|---|---|---|---|---|
| x, mol.% AgI | r = Ag/(Na + Ag) | |||||
| 0 | 0 | 3.13 (2) | 331 (5) | −5.08 (2) | 0.40 (1) | 4.15 (4) |
| 7.5 | 0.25 | 3.30 (2) | 325 (5) | −5.82 (1) | 0.47 (1) | 4.58 (4) |
| 15 | 0.5 | 3.43 (2) | 323 (5) | −6.39 (1) | 0.51 (1) | 4.68 (3) |
| 22.5 | 0.75 | 3.60 (2) | 332 (5) | −6.20 (3) | 0.48 (1) | 4.46 (8) |
| 30 | 1 | 3.72 (2) | 352 (5) | −5.62 (1) | 0.43 (1) | 4.17 (4) |
| Interfering Ion | Interfering Ion Concentration (mol L−1) | Selectivity Coefficient KNa+,M2+ |
|---|---|---|
| Mg2+ | 1 | 2.3 (5) × 10−4 |
| Ca2+ | 1 | 1.4 (7) × 10−4 |
| Ba2+ | 0.1 | 5.8 (4) × 10−4 |
| Zn2+ | 1 | 4.7 (4) × 10−4 |
| Na+ Primary Ion Concentration (mol L−1) | Selectivity Coefficient KNa+,K+ |
|---|---|
| 1 × 10−4 | 1.5 (2) × 10−1 |
| 1 × 10−3 | 1.9 (2) × 10−1 |
| 1 × 10−2 | 5.6 (3) × 10−2 |
| 1 × 10−1 | 1.7 (3) × 10−2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bokova, M.; Dumortier, S.; Poupin, C.; Cousin, R.; Kassem, M.; Bychkov, E. Potentiometric Chemical Sensors Based on Metal Halide Doped Chalcogenide Glasses for Sodium Detection. Sensors 2022, 22, 9986. https://doi.org/10.3390/s22249986
Bokova M, Dumortier S, Poupin C, Cousin R, Kassem M, Bychkov E. Potentiometric Chemical Sensors Based on Metal Halide Doped Chalcogenide Glasses for Sodium Detection. Sensors. 2022; 22(24):9986. https://doi.org/10.3390/s22249986
Chicago/Turabian StyleBokova, Maria, Steven Dumortier, Christophe Poupin, Renaud Cousin, Mohammad Kassem, and Eugene Bychkov. 2022. "Potentiometric Chemical Sensors Based on Metal Halide Doped Chalcogenide Glasses for Sodium Detection" Sensors 22, no. 24: 9986. https://doi.org/10.3390/s22249986
APA StyleBokova, M., Dumortier, S., Poupin, C., Cousin, R., Kassem, M., & Bychkov, E. (2022). Potentiometric Chemical Sensors Based on Metal Halide Doped Chalcogenide Glasses for Sodium Detection. Sensors, 22(24), 9986. https://doi.org/10.3390/s22249986







