Identification of Nonlinear Soil Properties from Downhole Array Data Using a Bayesian Model Updating Approach
Abstract
1. Introduction
2. Proposed Framework
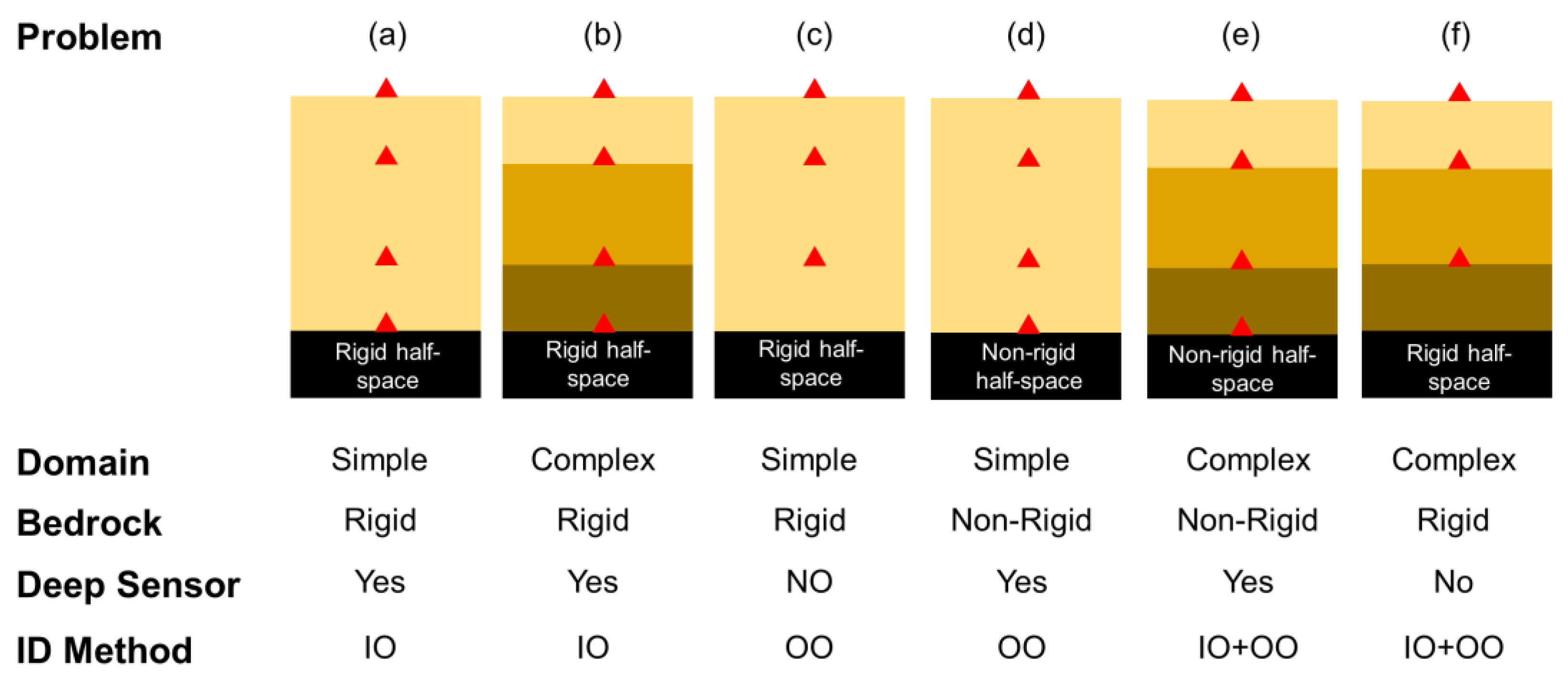
2.1. Problem Definition
2.2. Bayesian Model Updating
2.3. Material Model
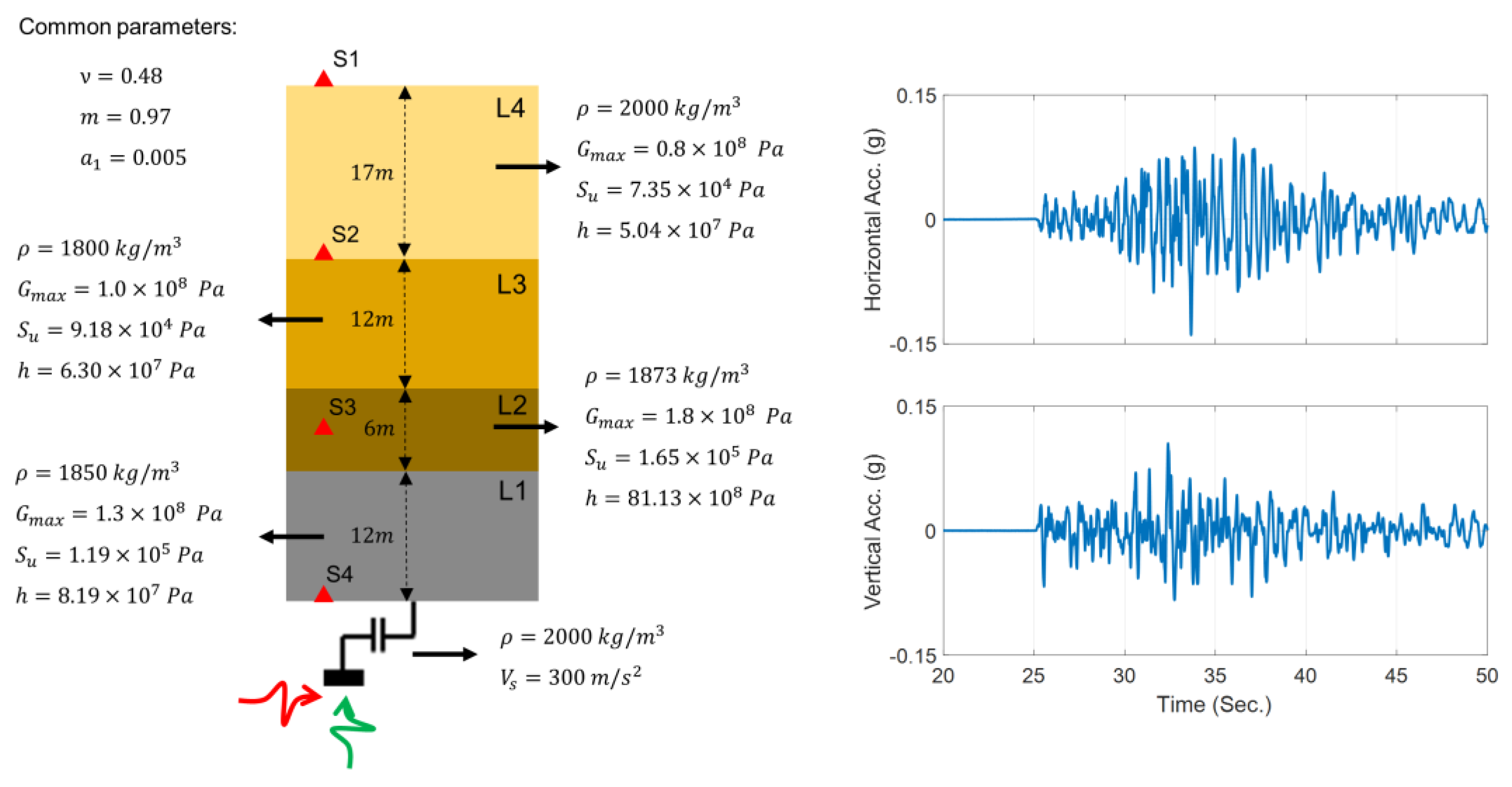
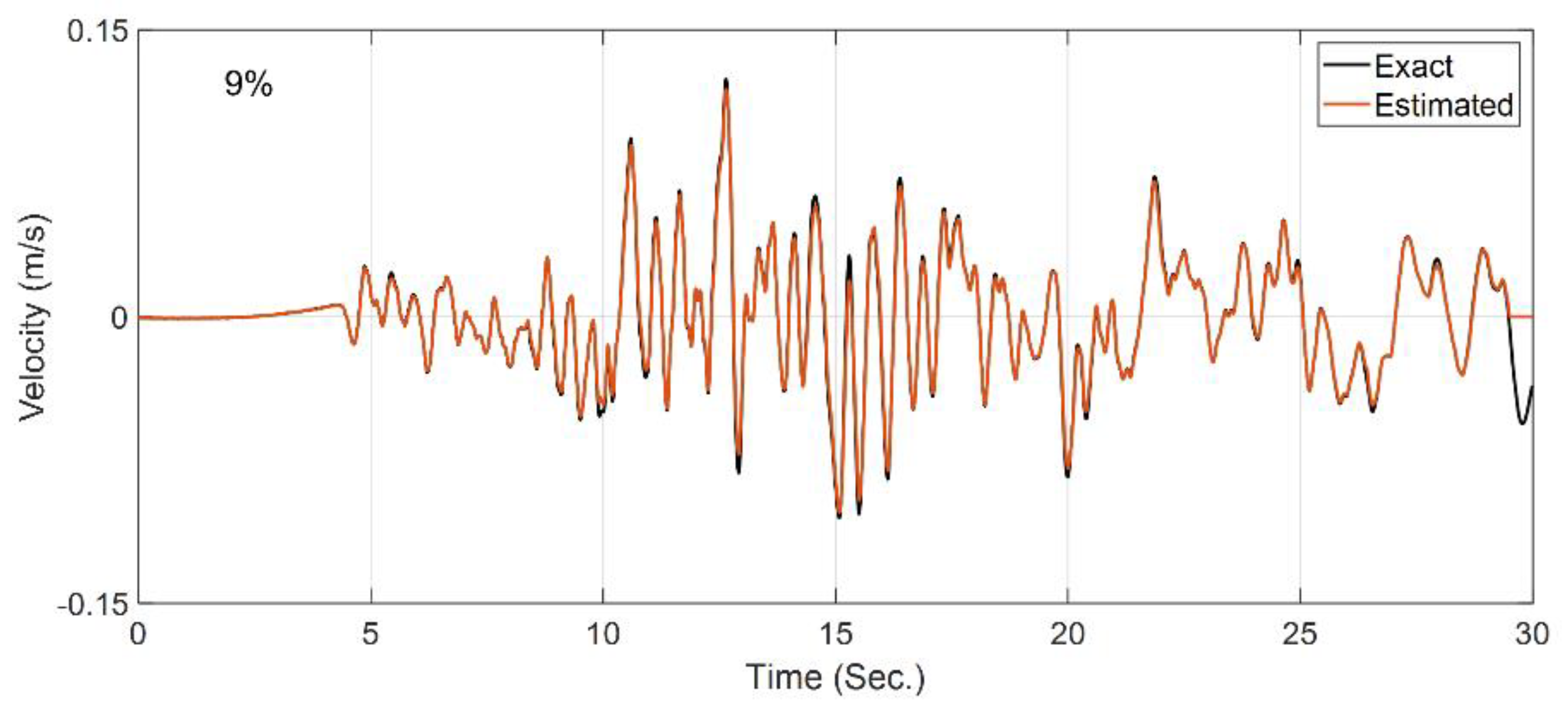
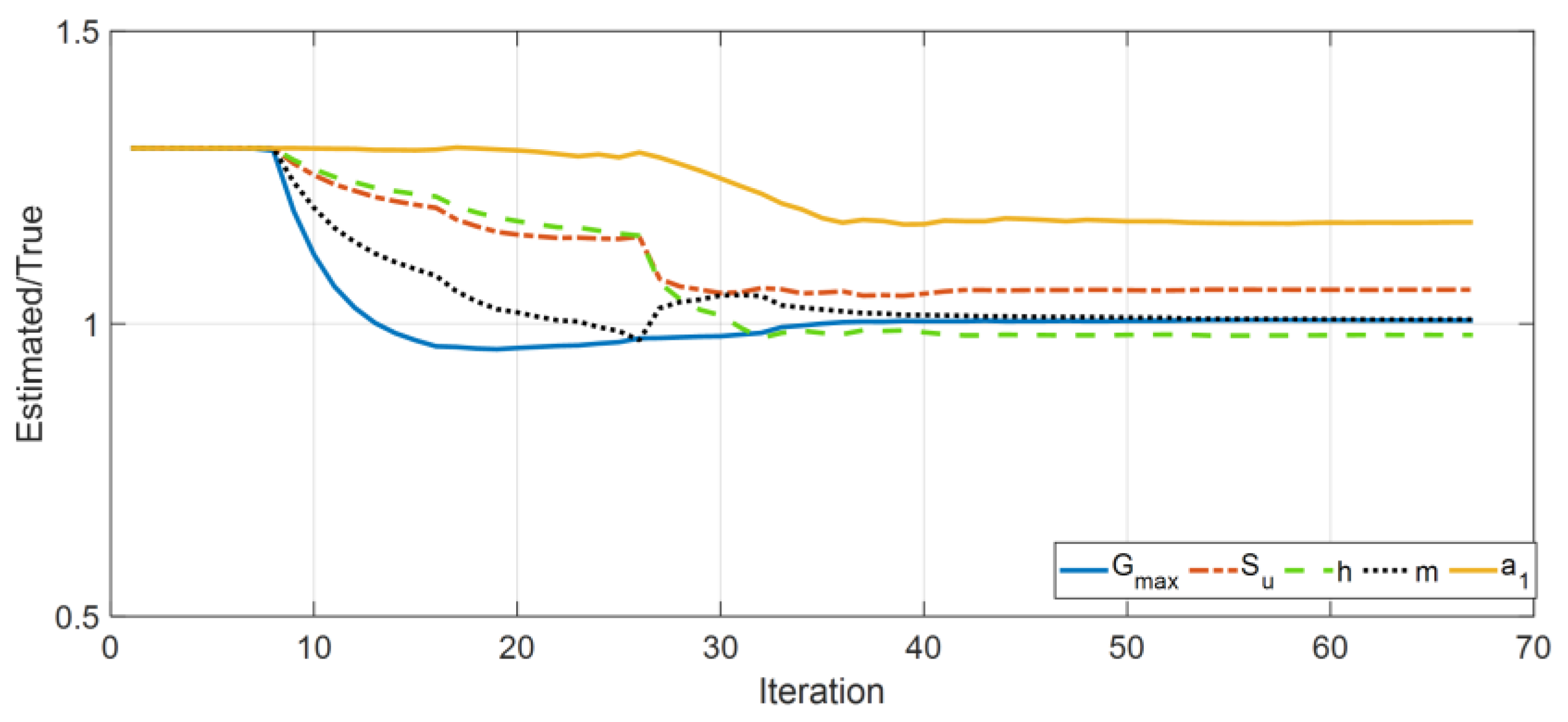
3. Verification Studies
3.1. Case 1: Unknown Complex Domain with Known Incident Motion
3.2. Case 2: Known Complex Domain with Unknown Incident Motion
3.3. Case 3: Unknown Simple Domain with Unknown Incident Motion
3.4. Case 4: Unknown Complex Domain with Unknown Incident Motion
4. Validation Studies
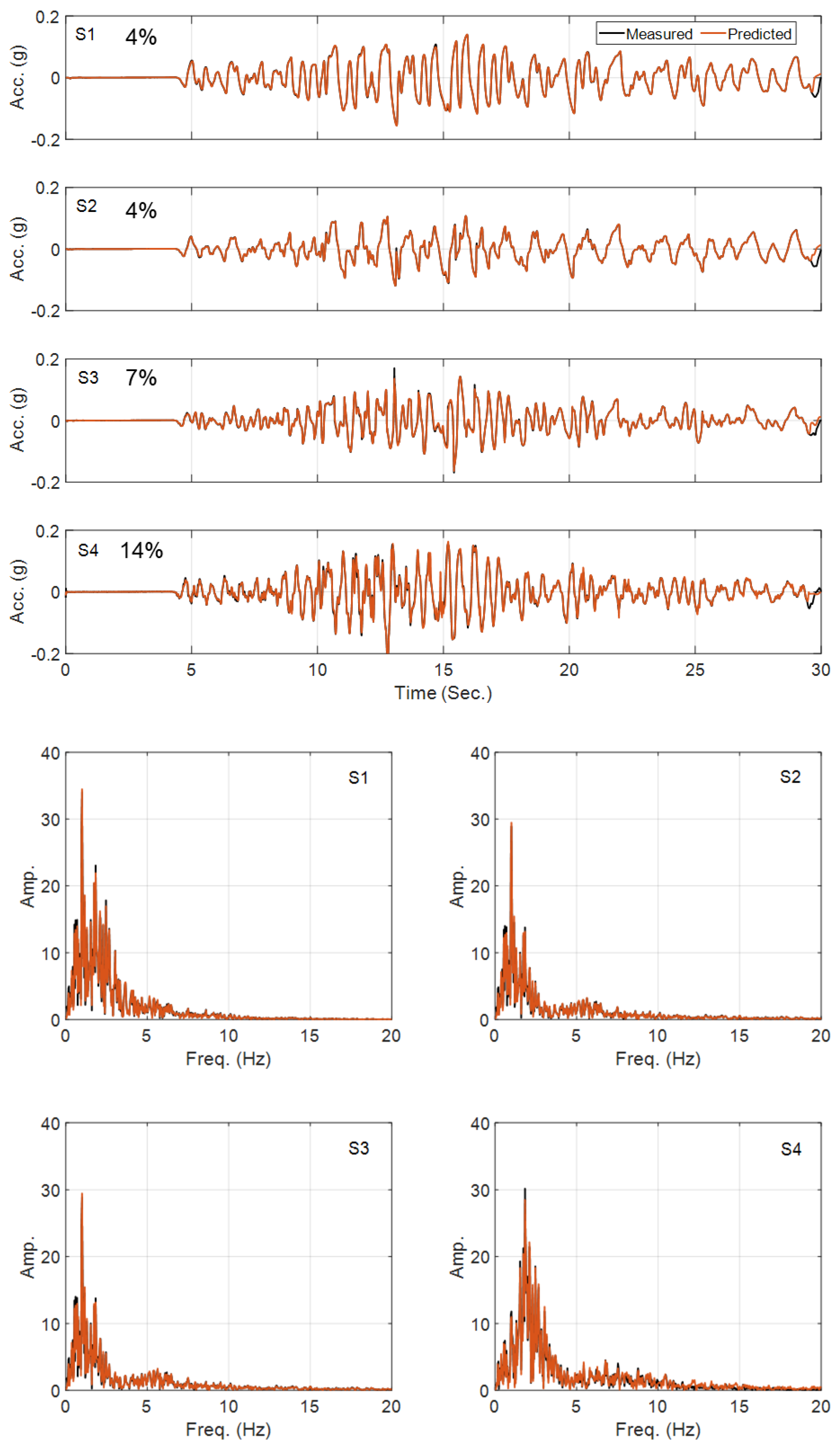
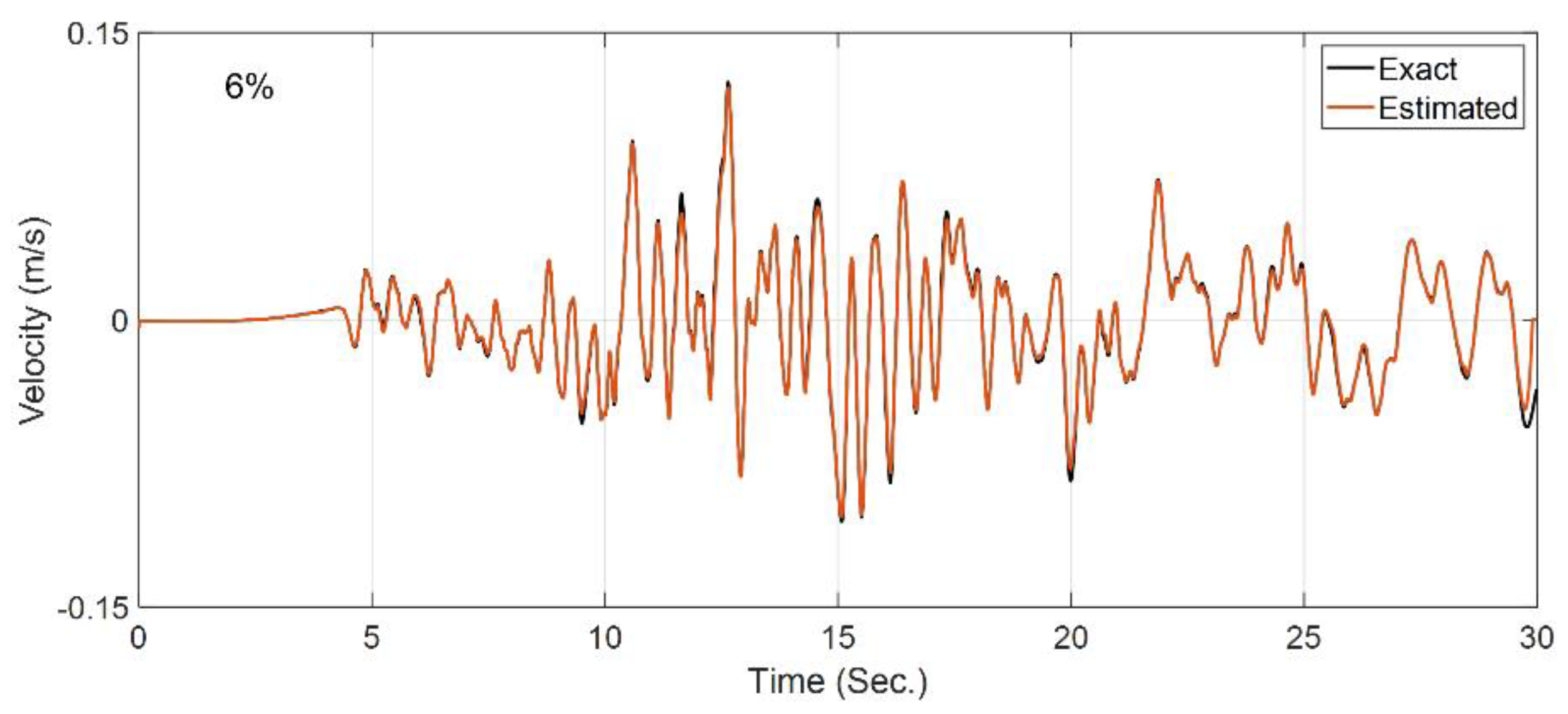
4.1. Centrifuge Test Data
4.2. Seismic Data from Lotung Site
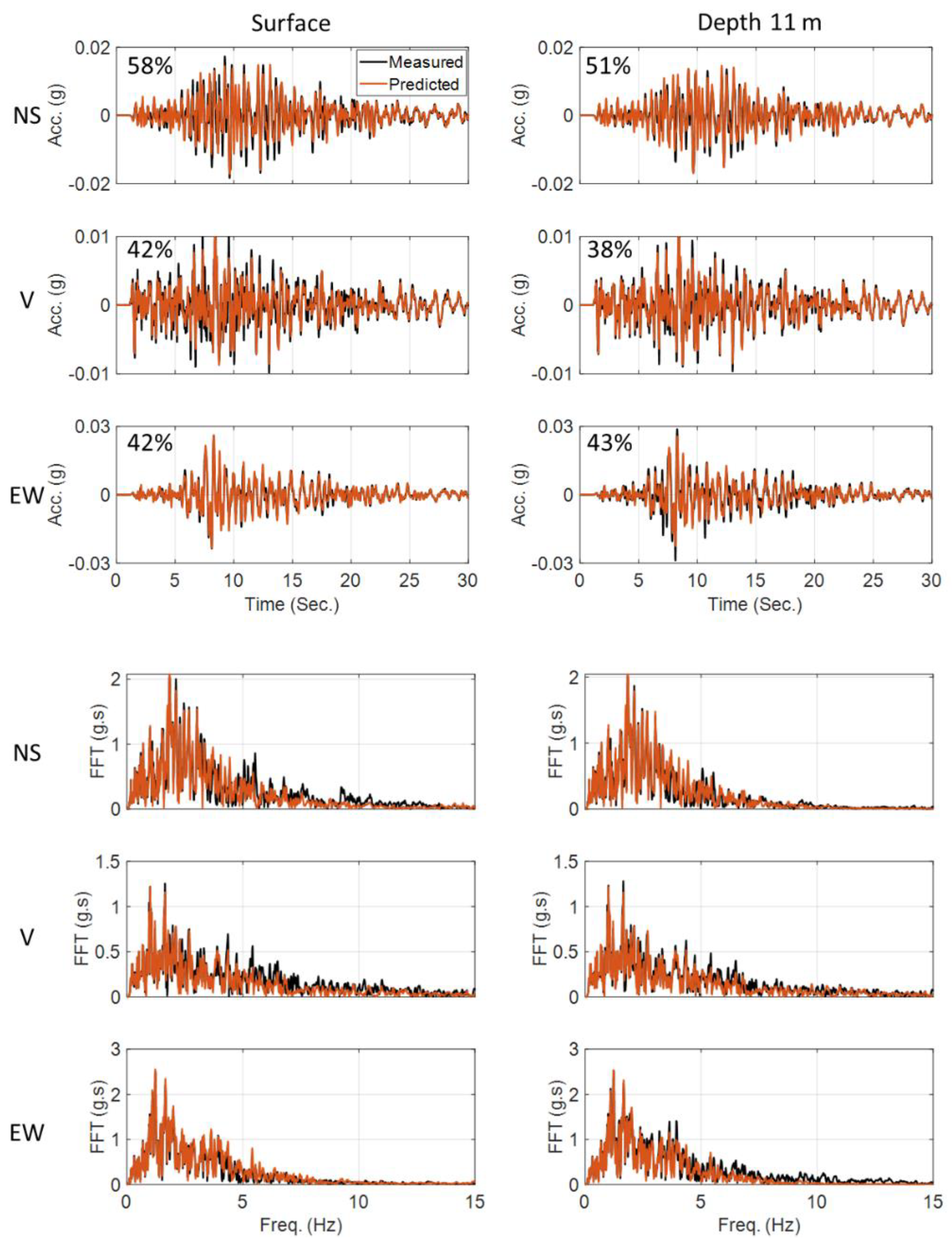
5. Application to a Blind Site
6. Discussion
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Trifunac, M.D.; Todorovska, M.I. Nonlinear Soil Response—1994 Northridge, California, Earthquake. J. Geotech. Eng. 1996, 122, 725–735. [Google Scholar] [CrossRef]
- Towhata, I. Geotechnical Earthquake Engineering; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar] [CrossRef]
- Idriss, I.M.; Seed, H.B. Seismic Response of Horizontal Soil Layers. J. Soil Mech. Found. Div. 1968, 94, 1003–1034. [Google Scholar] [CrossRef]
- Roesset, J.M. Soil Amplification of Earthquakes: Numerical Methods in Geotechnical Engineering; Desai, C.S., Christian, J.T., Eds.; McGraw-Hill: New York, NY, USA, 1977; pp. 639–682. [Google Scholar]
- Park, D.; Hashash, Y.M.A. Soil damping formulation in nonlinear time domain site response analysis. J. Earthq. Eng. 2004, 8, 249–274. [Google Scholar] [CrossRef]
- Stokoe, K.; Woods, R.D. In situ shear wave velocity by cross-hole method. J. Soil Mech. Found. Div. 1972, 98, 443–460. [Google Scholar] [CrossRef]
- Tarantola, A.; Santosa, F.; Pao, Y.H.; Symes, W.W.; Holland, C.H. The seismic reflection inverse problem. Inverse Probl. Acoust. Elastic Waves 1984, 14, 104. [Google Scholar]
- Uhlemann, S.; Hagedorn, S.; Dashwood, B.; Maurer, H.; Gunn, D.; Dijkstra, T.; Chambers, J. Landslide characterization using P- and S-wave seismic refraction tomography—The importance of elastic moduli. J. Appl. Geophys. 2016, 134, 64–76. [Google Scholar] [CrossRef]
- Kitsunezaki, C. A new method for shear-wave logging. Geophysics 1980, 45, 1489–1506. [Google Scholar] [CrossRef]
- Ganji, V.; Gucunski, N.; Nazarian, S. Automated Inversion Procedure for Spectral Analysis of Surface Waves. J. Geotech. Geoenviron. Eng. 1998, 124, 757–770. [Google Scholar] [CrossRef]
- Field, E.H.; Jacob, K.H. A comparison and test of various site-response estimation techniques, including three that are not reference-site dependent. Bull. Seismol. Soc. Am. 1995, 85, 1127–1143. [Google Scholar]
- Elgamal, A.-W.; Zeghal, M.; Tang, H.T.; Stepp, J.C. Lotung Downhole Array. I: Evaluation of Site Dynamic Properties. J. Geotech. Eng. 1995, 121, 350–362. [Google Scholar] [CrossRef]
- Ghahari, S.F.; Abazarsa, F.; Taciroglu, E. Probabilistic blind identification of site effects from ground surface signals. Bull. Earthq. Eng. 2017, 16, 1079–1104. [Google Scholar] [CrossRef]
- Ghahari, S.; Abazarsa, F.; Jeong, C.; Kurtulus, A.; Taciroglu, E. Blind identification of site effects and bedrock motion from surface response signals. Soil Dyn. Earthq. Eng. 2018, 107, 322–331. [Google Scholar] [CrossRef]
- Lin, J. Extraction of Dynamic Soil Properties Using Extended Kalman Filter. J. Geotech. Eng. 1994, 120, 2100–2117. [Google Scholar] [CrossRef]
- Zeghal, M.; Elgamal, A.-W.; Tang, H.T.; Stepp, J.C. Lotung Downhole Array. II: Evaluation of Soil Nonlinear Properties. J. Geotech. Eng. 1995, 121, 363–378. [Google Scholar] [CrossRef]
- Şafak, E. Models and Methods to Characterize Site Amplification from a Pair of Records. Earthq. Spectra 1997, 13, 97–129. [Google Scholar] [CrossRef]
- Astroza, R.; Pastén, C.; Ochoa-Cornejo, F. Site response analysis using one-dimensional equivalent-linear method and Bayesian filtering. Comput. Geotech. 2017, 89, 43–54. [Google Scholar] [CrossRef]
- Vardon, P.J.; Liu, K.; Hicks, M.A. Reduction of slope stability uncertainty based on hydraulic measurement via inverse analysis. Georisk Assess. Manag. Risk Eng. Syst. Geohazards 2016, 10, 223–240. [Google Scholar] [CrossRef]
- Yang, H.-Q.; Zhang, L.; Pan, Q.; Phoon, K.-K.; Shen, Z. Bayesian estimation of spatially varying soil parameters with spatiotemporal monitoring data. Acta Geotech. 2020, 16, 263–278. [Google Scholar] [CrossRef]
- Astroza, R.; Ebrahimian, H.; Li, Y.; Conte, J.P. Bayesian nonlinear structural FE model and seismic input identification for damage assessment of civil structures. Mech. Syst. Signal Process. 2017, 93, 661–687. [Google Scholar] [CrossRef]
- Ebrahimian, H.; Ghahari, S.F.; Asimaki, D.; Taciroglu, E. A Nonlinear Model Inversion to Estimate Dynamic Soil Stiffness of Building Structures. In Proceedings of the Geotechnical Earthquake Engineering and Soil Dynamics V, Austin, TX, USA, 10–13 June 2018. [Google Scholar] [CrossRef]
- Ebrahimian, H. Nonlinear Finite Element Model Updating for Nonlinear System and Damage Identification of Civil Structures; University of California: San Diego, CA, USA, 2015. [Google Scholar]
- JDRF CGM Study Group. JDRF Randomized Clinical Trial to Assess the Efficacy of Real-Time Continuous Glucose Monitoring in the Management of Type 1 Diabetes: Research Design and Methods. Diabetes Technol. Ther. 2008, 10, 310–321. [Google Scholar] [CrossRef]
- Joyner, W.B.; Chen, A.T.F. Calculation of nonlinear ground response in earthquakes. Bull. Seismol. Soc. Am. 1975, 65, 1315–1336. [Google Scholar]
- Ebrahimian, H.; Astroza, R.; Conte, J.P.; de Callafon, R.A. Nonlinear finite element model updating for damage identification of civil structures using batch Bayesian estimation. Mech. Syst. Signal Process. 2016, 84, 194–222. [Google Scholar] [CrossRef]
- Haykin, S. (Ed.) Kalman Filtering and Neural Networks; John Wiley & Sons, Inc.: New York, NY, USA, 2001. [Google Scholar] [CrossRef]
- Julier, S.J.; Uhlmann, J.K. New extension of the Kalman filter to nonlinear systems. In Proceedings of the Volume 3068, Signal Processing, Sensor Fusion, and Target Recognition VI, Orlando, FL, USA, 28 July 1997. [Google Scholar] [CrossRef]
- Nguyen, L.; Nestorović, T.; Fujisawa, K.; Murakami, A. Particle filter-based data assimilation for identification of soil parameters with application in tunneling. In Proceedings of the 14th International Conference of International Association for Computer Methods and Recent Advances in Geomechanics, 2014 (IACMAG 2014), Kyoto, Japan, 22 September 2014; pp. 1241–1246. [Google Scholar] [CrossRef]
- Murakami, A.; Shuku, T.; Nishimura, S.-I.; Fujisawa, K.; Nakamura, K. Data assimilation using the particle filter for identifying the elasto-plastic material properties of geomaterials. Int. J. Numer. Anal. Methods Géoméch. 2012, 37, 1642–1669. [Google Scholar] [CrossRef]
- Tao, Y.; Sun, H.; Cai, Y. Bayesian inference of spatially varying parameters in soil constitutive models by using deformation observation data. Int. J. Numer. Anal. Methods Géoméch. 2021, 45, 1647–1663. [Google Scholar] [CrossRef]
- Wang, Y.; Zhao, T.; Cao, Z. Site-specific probability distribution of geotechnical properties. Comput. Geotech. 2015, 70, 159–168. [Google Scholar] [CrossRef]
- Zhang, L.; Zuo, Z.; Ye, G.; Jeng, D.-S.; Wang, J. Probabilistic parameter estimation and predictive uncertainty based on field measurements for unsaturated soil slope. Comput. Geotech. 2013, 48, 72–81. [Google Scholar] [CrossRef]
- Ebrahimian, H.; Astroza, R.; Conte, J.P.; Papadimitriou, C. Bayesian optimal estimation for output-only nonlinear system and damage identification of civil structures. Struct. Control Health Monit. 2018, 25, e2128. [Google Scholar] [CrossRef]
- Vucetic, M.; Dobry, R. Effect of Soil Plasticity on Cyclic Response. J. Geotech. Eng. 1991, 117, 89–107. [Google Scholar] [CrossRef]
- Borja, R.I.; Amies, A.P. Multiaxial Cyclic Plasticity Model for Clays. J. Geotech. Eng. 1994, 120, 1051–1070. [Google Scholar] [CrossRef]
- Manzari, M.T.; Dafalias, Y.F. A critical state two-surface plasticity model for sands. Géotechnique 1997, 47, 255–272. [Google Scholar] [CrossRef]
- Pisanò, F.; Jeremić, B. Simulating stiffness degradation and damping in soils via a simple visco-elastic–plastic model. Soil Dyn. Earthq. Eng. 2014, 63, 98–109. [Google Scholar] [CrossRef]
- Yang, Z.; Elgamal, A.; Parra, E. Computational Model for Cyclic Mobility and Associated Shear Deformation. J. Geotech. Geoenviron. Eng. 2003, 129, 1119–1127. [Google Scholar] [CrossRef]
- Drucker, D.C.; Prager, W. Soil mechanics and plastic analysis or limit design. Q. Appl. Math. 1952, 10, 157–165. [Google Scholar] [CrossRef]
- McKenna, F. OpenSees: A Framework for Earthquake Engineering Simulation. Comput. Sci. Eng. 2011, 13, 58–66. [Google Scholar] [CrossRef]
- Borja, R.I.; Chao, H.-Y.; Montáns, F.J.; Lin, C.-H. Nonlinear Ground Response at Lotung LSST Site. J. Geotech. Geoenviron. Eng. 1999, 125, 187–197. [Google Scholar] [CrossRef][Green Version]
- Borja, R.I.; Lin, C.H.; Sama, K.M.; Masada, G.M. Modelling non-linear ground response of non-liquefiable soils. Earthq. Eng. Struct. Dyn. 2000, 29, 63–83. [Google Scholar] [CrossRef]
- Zhang, W.; Seylabi, E.E.; Taciroglu, E. Validation of a three-dimensional constitutive model for nonlinear site response and soil-structure interaction analyses using centrifuge test data. Int. J. Numer. Anal. Methods Géoméch. 2017, 41, 1828–1847. [Google Scholar] [CrossRef]
- Zhang, W.; Lim, K.; Ghahari, S.F.; Arduino, P.; Taciroglu, E. On the implementation and validation of a three-dimensional pressure-dependent bounding surface plasticity model for soil nonlinear wave propagation and soil-structure interaction analyses. Int. J. Numer. Anal. Methods Géoméch. 2021, 45, 1091–1119. [Google Scholar] [CrossRef]
- Borja, R.I.; Wu, W. Vibration of Foundations on Incompressible Soils with No Elastic Region. J. Geotech. Eng. 1994, 120, 1570–1592. [Google Scholar] [CrossRef]
- Arduino, P.; Taciroglu, E.; Bonilla, F.; Taiebat, M. Development of a Numerical Nonlinear Soil Module to Expand the Capabilities of the SCEC BroadBand Platform. 2019. Available online: https://files.scec.org/s3fs-public/reports/2018/18103_report.pdf?J_syKFm0YgnKsyfNxGf3iHRlUnTLW2NN (accessed on 8 December 2022).
- Hilber, H.M.; Hughes, T.J.R.; Taylor, R.L. Improved numerical dissipation for time integration algorithms in structural dynamics. Earthq. Eng. Struct. Dyn. 1977, 5, 283–292. [Google Scholar] [CrossRef]
- Graizer, V.; Shakal, A. Recent Data from CSMIP Instrumented Downhole Arrays. 2004. Available online: https://ui.adsabs.harvard.edu/abs/2004AGUFM.S13D1099G/abstract (accessed on 8 December 2022).
- Ghahari, F.; Malekghaini, N.; Ebrahimian, H.; Taciroglu, E. Bridge Digital Twinning Using an Output-Only Bayesian Model Updating Method and Recorded Seismic Measurements. Sensors 2022, 22, 1278. [Google Scholar] [CrossRef] [PubMed]
- Nabiyan, M.; Khoshnoudian, F.; Moaveni, B.; Ebrahimian, H. Mechanics-based model updating for identification and virtual sensing of an offshore wind turbine using sparse measurements. Struct. Control Health Monit. 2020, 28, e2647. [Google Scholar] [CrossRef]
- Seylabi, E.E.; Agapaki, E.; Pitilakis, D.; Taciroglu, E.; Brandenberg, S.; Stewart, J. DesignSafe-CI: Development of Validated Methods for Soil-Structure Interaction Analysis of Buried Structures. 2017. Available online: https://www.designsafe-ci.org/data/browser/public/designsafe.storage.published/PRJ-1299 (accessed on 8 December 2022).
- Seylabi, E.E.; Ebrahimian, H.; Zhang, W.; Asimaki, D.; Taciroglu, E. Bayesian Estimation of Nonlinear Soil Model Parameters Using Centrifuge Experimental Data. In Proceedings of the Geotechnical Earthquake Engineering and Soil Dynamics V, Austin, TX, USA, 10–13 June 2018. [Google Scholar] [CrossRef]
- Tang, H.; Tang, Y.; Stepp, J. Lotung large-scale seismic experiment and soil-structure interaction method validation. Nucl. Eng. Des. 1990, 123, 397–412. [Google Scholar] [CrossRef]
- Li, X.S.; Shen, C.K.; Wang, Z.L. Fully Coupled Inelastic Site Response Analysis for 1986 Lotung Earthquake. J. Geotech. Geoenviron. Eng. 1998, 124, 560–573. [Google Scholar] [CrossRef]
- Elia, G.; Rouainia, M.; Karofyllakis, D.; Guzel, Y. Modelling the non-linear site response at the LSST down-hole accelerometer array in Lotung. Soil Dyn. Earthq. Eng. 2017, 102, 1–14. [Google Scholar] [CrossRef]
- Glaser, S.; Baise, L. System identification estimation of soil properties at the Lotung site. Soil Dyn. Earthq. Eng. 2000, 19, 521–531. [Google Scholar] [CrossRef]
- Chang, C.-Y.; Mok, C.M.; Tang, H.-T. Inference of Dynamic Shear Modulus from Lotung Downhole Data. J. Geotech. Eng. 1996, 122, 657–665. [Google Scholar] [CrossRef]
- Borja, R.I.; Duvernay, B.G.; Lin, C.-H. Ground Response in Lotung: Total Stress Analyses and Parametric Studies. J. Geotech. Geoenviron. Eng. 2002, 128, 54–63. [Google Scholar] [CrossRef][Green Version]
- Afshari, K.; Stewart, J. Implications of California Vertical Array Data for the Analysis of site Response with 1D Geotechnical Modelling; UCLA: Los Angeles, CA, USA, 2017. [Google Scholar]














| Layer | Parameter | Initial Error (%) | Final Error (%) |
|---|---|---|---|
| 1 | +30 | 0.04 | |
| +30 | −1.86 | ||
| +30 | 1.97 | ||
| +30 | −1.16 | ||
| +30 | 1.04 | ||
| 2 | +30 | −4.72 | |
| +30 | 7.64 | ||
| +30 | 10.91 | ||
| +30 | −1.09 | ||
| +30 | 49.02 | ||
| 3 | +30 | 0.24 | |
| +30 | −0.42 | ||
| +30 | −0.19 | ||
| +30 | 0.02 | ||
| +30 | 0.32 | ||
| 4 | +30 | −1.40 | |
| +30 | −2.15 | ||
| +30 | 4.08 | ||
| +30 | 0.35 | ||
| +30 | 2.72 |
| Layer | Parameter | Initial Error (%) | Final Error (%) |
|---|---|---|---|
| 1 | +30 | 0.56 | |
| +30 | 5.80 | ||
| +30 | −1.92 | ||
| +30 | 0.71 | ||
| +30 | 17.33 |
| Layer | Parameter | Initial Error (%) | Final Error (%) |
|---|---|---|---|
| 1 | +30 | −1.41 | |
| +30 | −1.17 | ||
| +30 | −0.52 | ||
| +30 | −3.63 | ||
| +30 | −7.73 | ||
| 2 | +30 | 3.48 | |
| +30 | 19.69 | ||
| +30 | 22.09 | ||
| +30 | 12.4 | ||
| +30 | 24.59 | ||
| 3 | +30 | −0.21 | |
| +30 | −1.06 | ||
| +30 | −1.32 | ||
| +30 | −1.53 | ||
| +30 | −0.35 | ||
| 4 | +30 | −1.86 | |
| +30 | −0.51 | ||
| +30 | 3.54 | ||
| +30 | 0.55 | ||
| +30 | −12.09 |
| Parameter | Baseline Values | Initial Error (%) | Final Error (%) |
|---|---|---|---|
| 0.0032 | +50 | −1.4% | |
| 0.33 | +50 | +91% | |
| 193 (m/s) | +50 | +50% | |
| 1.58 | +50 | +7% | |
| 0.47 | +50 | +800% | |
| 4.56 | +50 | −33% | |
| 0.0015 | +50 | −13% |
| Parameter | Baseline Value | Initial Error (%) | Final Error (%) |
|---|---|---|---|
| +30 | −9 | ||
| +30 | 111 | ||
| +30 | 43 | ||
| +30 | −18 | ||
| * | +30 | −29 | |
| +30 | −36 | ||
| +30 | −39 | ||
| +30 | −41 | ||
| +30 | 36 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghahari, F.; Abazarsa, F.; Ebrahimian, H.; Zhang, W.; Arduino, P.; Taciroglu, E. Identification of Nonlinear Soil Properties from Downhole Array Data Using a Bayesian Model Updating Approach. Sensors 2022, 22, 9848. https://doi.org/10.3390/s22249848
Ghahari F, Abazarsa F, Ebrahimian H, Zhang W, Arduino P, Taciroglu E. Identification of Nonlinear Soil Properties from Downhole Array Data Using a Bayesian Model Updating Approach. Sensors. 2022; 22(24):9848. https://doi.org/10.3390/s22249848
Chicago/Turabian StyleGhahari, Farid, Fariba Abazarsa, Hamed Ebrahimian, Wenyang Zhang, Pedro Arduino, and Ertugrul Taciroglu. 2022. "Identification of Nonlinear Soil Properties from Downhole Array Data Using a Bayesian Model Updating Approach" Sensors 22, no. 24: 9848. https://doi.org/10.3390/s22249848
APA StyleGhahari, F., Abazarsa, F., Ebrahimian, H., Zhang, W., Arduino, P., & Taciroglu, E. (2022). Identification of Nonlinear Soil Properties from Downhole Array Data Using a Bayesian Model Updating Approach. Sensors, 22(24), 9848. https://doi.org/10.3390/s22249848







