Review on Carbon Nanomaterials-Based Nano-Mass and Nano-Force Sensors by Theoretical Analysis of Vibration Behavior
Abstract
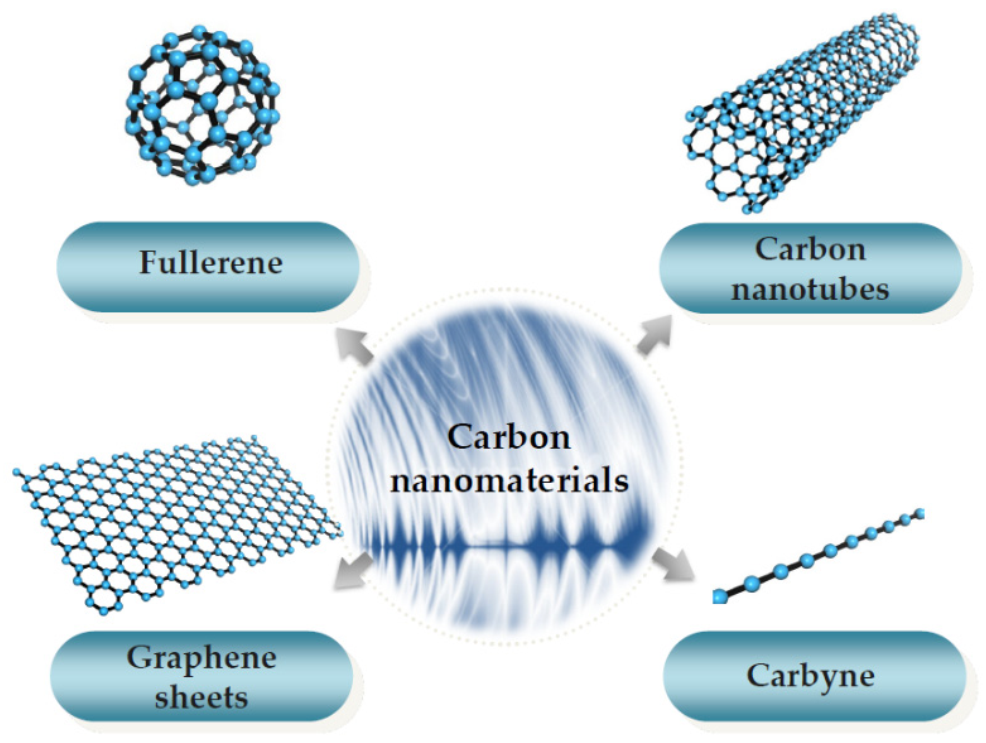
1. Introduction
2. Mechanism of Frequency-Based Nano-Mass and Nano-Force Sensors
2.1. Nano-Mass Sensor
2.2. Nano-Force Sensor
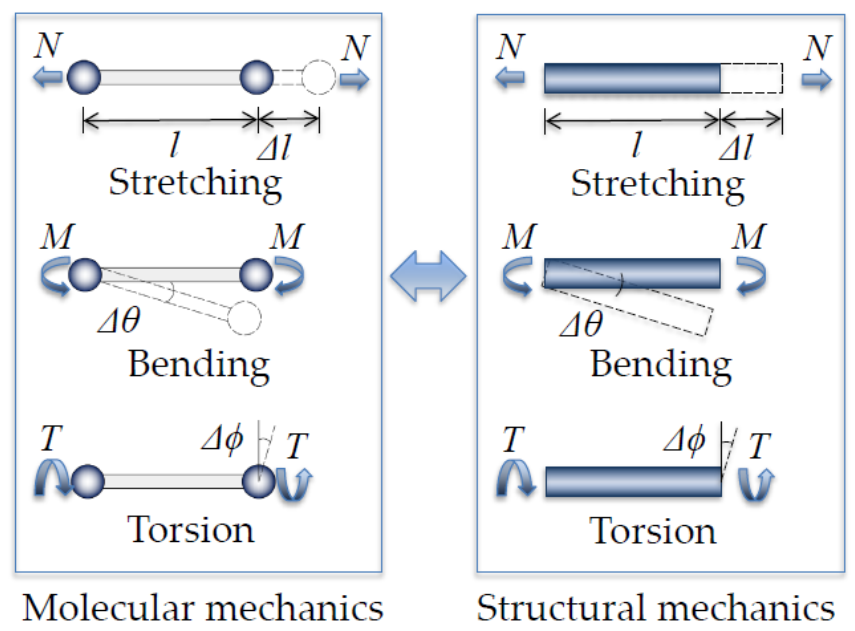
3. Continuum Models of Carbon Nanomaterials
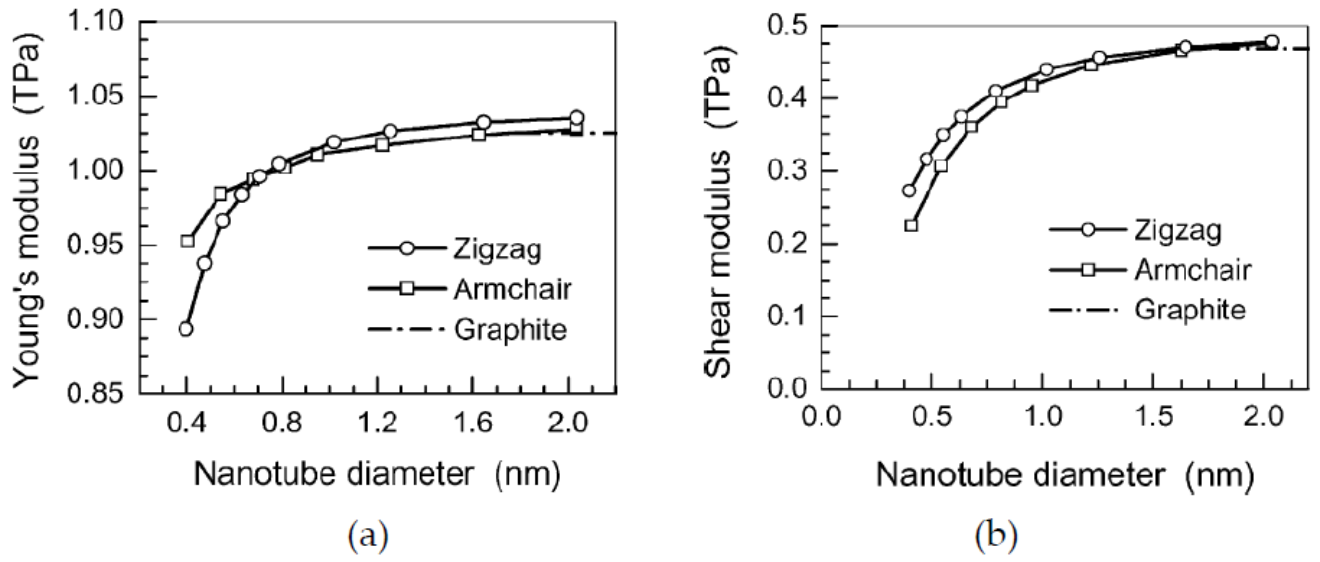
3.1. Carbon Nanotubes and Graphene Sheets
3.2. Carbyne
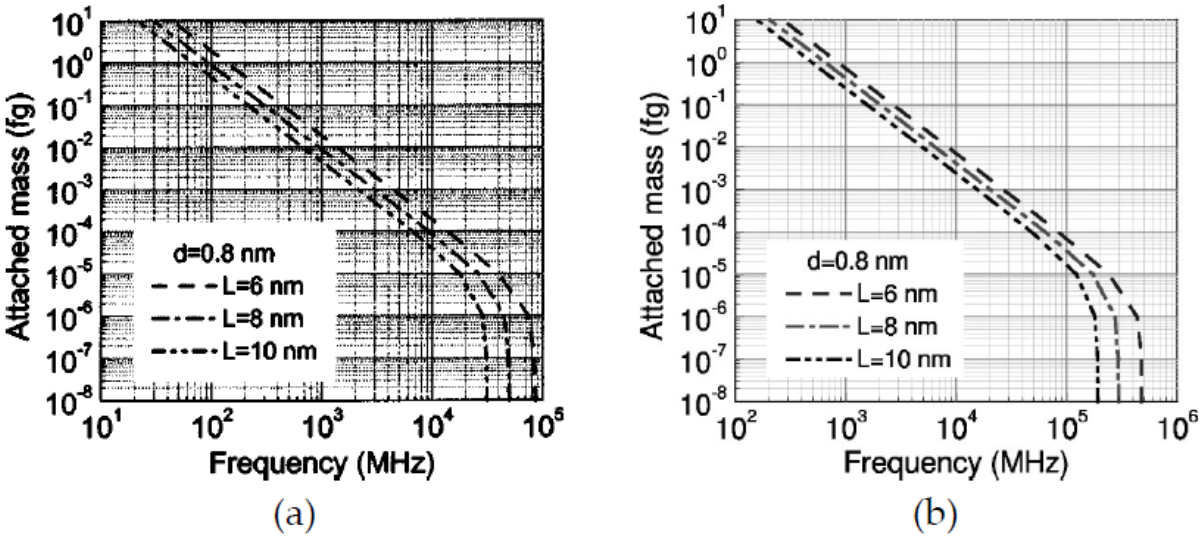
4. Nano-Mass Sensor
4.1. Carbon Nanotubes-Based Nano-Mass Sensor
4.2. Graphene Sheets-Based Nano-Mass Sensor
4.3. Carbyne-Based Nano-Mass Sensor
5. Nano-Force Sensor
6. Conclusions
- The mechanism of nano-mass and nano-force sensors based on vibration analysis were introduced theoretically.
- The methods of modeling CNTs, GSs, and carbyne as continuum structures were reviewed in detail. Especially, we have proposed that, in the vibration analysis of CNTs and GSs, besides Young’s modulus, shear modulus, and thickness, their densities should be determined simultaneously, which will be an essential work for studying CNTs/GSs-based nano sensors in the future.
- By summarizing the recent studies of carbon nanomaterials-based nano-mass sensors, CNTs, GS, and carbyne-based nano-mass sensors owned the minimum sensitivity of 10−23 g, 10−24~10−22 g, and 10−26~10−23 g, respectively. Hence, nano-mass sensors using carbyne resonators can provide the highest sensitivity among the three kinds of carbon nanomaterial resonators.
- Carbon nanomaterials-based nano-force sensors are seldom investigated. However, because of their extremely excellent material properties, CNTs/GSs/carbyne-based nano-force sensors should be studied further by vibration analysis. Moreover, discussion of detecting external forces acting in different directions would also be a deserved work toward the real application of nano-force sensors in the future.
- At present, the nanobalance technique for measuring the frequency shift of CNTs was demonstrated that could be applied to measure the mass of a tiny particle of light as 22 × 10−15 g [38]. Fifty-one gold atoms loaded on CNTs resonators could be experimentally measured using the relationship between the resonance frequency and atom numbers [40]. However, the real-time application of the nano-testing techniques would be a big challenge due to the small size and weight of carbon nanomaterials. New methods and approaches should be well established to reduce the measurement uncertainly and increase testing accuracy.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Kroto, H.W.; Heath, J.R.; O Brien, S.C.; Curl, R.F.; E Smalley, R. C60: Buckminsterfullerene. Nat. Cell Biol. 1985, 318, 162–163. [Google Scholar] [CrossRef]
- Oberlin, A.; Endo, M.; Koyama, T. Filamentous growth of carbon through benzene decomposition. J. Cryst. Growth 1976, 32, 335–349. [Google Scholar] [CrossRef]
- Iijima, S. Helical microtubules of graphitic carbon. Nat. Cell Biol. 1991, 354, 56–58. [Google Scholar] [CrossRef]
- Iijima, S.; Ichihashi, T. Single-shell carbon nanotubes of 1-nm diameter. Nat. Cell Biol. 1993, 363, 603–605. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Zhang, Y.; Dubonos, S.V.; Grigorieva, I.V.; Firsov, A.A. Electric field effect in atomically thin carbon films. Science 2004, 306, 666–669. [Google Scholar] [CrossRef] [PubMed]
- Heimann, R.B.; Kleiman, J.; Salansky, N.M. A unified structural approach to linear carbon polytypes. Nat. Cell Biol. 1983, 306, 164–167. [Google Scholar] [CrossRef]
- Shokrieh, M.M.; Rafiee, R. A review of the mechanical properties of isolated carbon nanotubes and carbon nanotube composites. Mech. Compos. Mater. 2010, 46, 155–172. [Google Scholar] [CrossRef]
- Kumar, A.; Sharma, K.; Dixit, A.R. A review of the mechanical and thermal properties of graphene and its hybrid polymer nanocomposites for structural applications. J. Mater. Sci. 2019, 54, 5992–6026. [Google Scholar] [CrossRef]
- Sun, D.-M.; Liu, C.; Ren, W.-C.; Cheng, H.-M. A review of carbon nanotube- and graphene-based flexible thin-film transistors. Small 2013, 9, 1188–1205. [Google Scholar] [CrossRef]
- Che, Y.; Chen, H.; Gui, H.; Liu, J.; Liu, B.; Zhou, C. Review of carbon nanotube nanoelectronics and macroelectronics. Semicond. Sci. Technol. 2014, 29, 1–17. [Google Scholar] [CrossRef]
- Yang, Z.; Tian, J.; Yin, Z.; Cui, C.; Qian, W.; Wei, F. Carbon nanotube- and graphene-based nanomaterials and applications in high-voltage supercapacitor: A review. Carbon 2019, 141, 467–480. [Google Scholar] [CrossRef]
- Kumanek, B.; Janas, D. Thermal conductivity of carbon nanotube networks: A review. J. Mater. Sci. 2019, 54, 7397–7427. [Google Scholar] [CrossRef]
- Yamashita, S. A tutorial on nonlinear photonic applications of carbon nanotube and graphene. J. Light. Technol. 2011, 30, 427–447. [Google Scholar] [CrossRef]
- Wang, J. Carbon-nanotube based electrochemical biosensors: A review. Electroanalysis 2005, 17, 7–14. [Google Scholar] [CrossRef]
- Fam, D.; Palaniappan, A.; Tok, A.; Liedberg, B.; Moochhala, S. A review on technological aspects influencing commercialization of carbon nanotube sensors. Sens. Actuators B Chem. 2011, 157, 1–7. [Google Scholar] [CrossRef]
- Castillo-Henríquez, L.; Brenes-Acuña, M.; Castro-Rojas, A.; Cordero-Salmerón, R.; Lopretti-Correa, M.; Vega-Baudrit, J.R. Biosensors for the detection of bacterial and viral clinical pathogens. Sensors 2020, 20, 6926. [Google Scholar] [CrossRef] [PubMed]
- Mahar, B.; Laslau, C.; Yip, R.; Sun, Y. Development of carbon nanotube-based sensors—a review. IEEE Sens. J. 2007, 7, 266–284. [Google Scholar] [CrossRef]
- Li, C.; Thostenson, E.T.; Chou, T.-W. Sensors and actuators based on carbon nanotubes and their composites: A review. Compos. Sci. Technol. 2008, 68, 1227–1249. [Google Scholar] [CrossRef]
- Her, S.-C.; Hsu, W.-C. Strain and temperature sensitivities along with mechanical properties of CNT buckypaper sensors. Sensors 2020, 20, 3067. [Google Scholar] [CrossRef] [PubMed]
- Zhang, X.; Cui, H.; Gui, Y.; Tang, J. Mechanism and application of carbon nanotube sensors in sf6 decomposed production detection: A review. Nanoscale Res. Lett. 2017, 12, 1–12. [Google Scholar] [CrossRef] [PubMed]
- Pandhi, T.; Chandnani, A.; Subbaraman, H.; Estrada, D. A review of inkjet printed graphene and carbon nanotubes based gas sensors. Sensors 2020, 20, 5642. [Google Scholar] [CrossRef]
- Nikolic, M.V.; Milovanovic, V.; Vasiljevic, Z.Z.; Stamenkovic, Z. Semiconductor gas sensors: Materials, technology, design, and application. Sensors 2020, 20, 6694. [Google Scholar] [CrossRef] [PubMed]
- Sauerbrey, G. Verwendung von Schwingquarzen zur Wägung Dünner Schichten und zur Mikrowägung. Eur. Phys. J. A 1959, 155, 206–222. [Google Scholar] [CrossRef]
- Abadal, G.; Davis, Z.J.; Barniol, N.; Helbo, B.; Borrisé, X.; Ruiz, R.; Boisen, A.; Campabadal, F.; Esteve, J.; Figueras, E.; et al. Electromechanical model of a resonating nano-cantilever-based sensor for high-resolution and high-sensitivity mass detection. Nanotechnology 2001, 12, 100–104. [Google Scholar] [CrossRef]
- Davis, Z.; Abadal, G.; Helbo, B.; Hansen, O.; Campabadal, F.; Perez-Murano, F.; Esteve, J.; Figueras, E.; Verd, J.; Barniol, N.; et al. Monolithic integration of mass sensing nano-cantilevers with CMOS circuitry. Sens. Actuators A Phys. 2003, 105, 311–319. [Google Scholar] [CrossRef]
- Ono, T.; Li, X.; Miyashita, H.; Esashi, M. Mass sensing of adsorbed molecules in sub-picogram sample with ultrathin silicon resonator. Rev. Sci. Instrum. 2003, 74, 1240–1243. [Google Scholar] [CrossRef]
- Forsén, E.; Abadal, G.; Ghatnekar-Nilsson, S.; Teva, J.; Verd, J.; Sandberg, R.; Svendsen, W.E.; Perez-Murano, F.; Esteve, J.; Figueras, E.; et al. Ultrasensitive mass sensor fully integrated with complementary metal-oxide-semiconductor circuitry. Appl. Phys. Lett. 2005, 87, 043507. [Google Scholar] [CrossRef]
- Zhang, W.; Turner, K.L. Application of parametric resonance amplification in a single-crystal silicon micro-oscillator based mass sensor. Sens. Actuators A Phys. 2005, 122, 23–30. [Google Scholar] [CrossRef]
- Naik, A.K.; Hanay, M.S.; Hiebert, W.K.; Feng, X.L.; Roukes, M.L. Towards single-molecule nanomechanical mass spectrometry. Nat. Nanotechnol. 2009, 4, 445–450. [Google Scholar] [CrossRef] [PubMed]
- Barton, R.A.; Ilic, B.; Verbridge, S.S.; Cipriany, B.R.; Parpia, J.M.; Craighead, H.G. Fabrication of a nanomechanical mass sensor containing a nanofluidic channel. Nano Lett. 2010, 10, 2058–2063. [Google Scholar] [CrossRef]
- Gil-Santos, E.; Ramos, D.; Martínez, J.; Fernández-Regúlez, M.; García, R.; San Paulo, Á.; Calleja, M.; Tamayo, J. Nanomechanical mass sensing and stiffness spectrometry based on two-dimensional vibrations of resonant nanowires. Nat. Nanotechnol. 2010, 5, 641–645. [Google Scholar] [CrossRef]
- Hanay, M.S.; Kelber, S.; Naik, A.K.; Chi, D.; Hentz, S.; Bullard, E.C.; Colinet, E.; Duraffourg, L.; Roukes, M.L. Single-protein nanomechanical mass spectrometry in real time. Nat. Nanotechnol. 2012, 7, 602–608. [Google Scholar] [CrossRef]
- Wasisto, H.S.; Merzsch, S.; Waag, A.; Uhde, E.; Salthammer, T.; Peiner, E. Airborne engineered nanoparticle mass sensor based on a silicon resonant cantilever. Sens. Actuators B Chem. 2013, 180, 77–89. [Google Scholar] [CrossRef]
- Olcum, S.; Cermak, N.; Wasserman, S.C.; Manalis, S.R. High-speed multiple-mode mass-sensing resolves dynamic nanoscale mass distributions. Nat. Commun. 2015, 6, 7070. [Google Scholar] [CrossRef] [PubMed]
- Hanay, M.S.; Kelber, S.I.; O’Connell, C.D.; Mulvaney, P.; Sader, J.E.; Roukes, M.L. Inertial imaging with nanomechanical systems. Nat. Nanotechnol. 2015, 10, 339–344. [Google Scholar] [CrossRef] [PubMed]
- Cermak, N.; Olcum, S.; Delgado, F.F.; Wasserman, S.C.; Payer, K.R.; Murakami, A.M.; Knudsen, S.M.; Kimmerling, R.J.; Stevens, M.M.; Kikuchi, Y.; et al. High-throughput measurement of single-cell growth rates using serial microfluidic mass sensor arrays. Nat. Biotechnol. 2016, 34, 1052–1059. [Google Scholar] [CrossRef]
- Sader, J.E.; Hanay, M.S.; Neumann, A.P.; Roukes, M.L. Mass spectrometry using nanomechanical systems: Beyond the point-mass approximation. Nano Lett. 2018, 18, 1608–1614. [Google Scholar] [CrossRef]
- Poncharal, P.; Wang, Z.L.; Ugarte, D.; De Heer, W.A. Electrostatic deflections and electromechanical resonances of carbon nanotubes. Science 1999, 283, 1513–1516. [Google Scholar] [CrossRef]
- Bunch, J.S.; Van Der Zande, A.M.; Verbridge, S.S.; Frank, I.W.; Tanenbaum, D.M.; Parpia, J.M.; Craighead, H.G.; McEuen, P.L. Electromechanical resonators from graphene sheets. Science 2007, 315, 490–493. [Google Scholar] [CrossRef]
- Jensen, K.H.; Kim, K.; Zettl, A. An atomic-resolution nanomechanical mass sensor. Nat. Nanotechnol. 2008, 3, 533–537. [Google Scholar] [CrossRef] [PubMed]
- Eom, K.; Park, H.S.; Yoon, D.S.; Kwon, T. Nanomechanical resonators and their applications in biological/chemical detection: Nanomechanics principles. Phys. Rep. 2011, 503, 115–163. [Google Scholar] [CrossRef]
- Chaste, J.; Eichler, A.; E Moser, J.; Ceballos, G.; Rurali, R.; Bachtold, A. A nanomechanical mass sensor with yoctogram resolution. Nat. Nanotechnol. 2012, 7, 301–304. [Google Scholar] [CrossRef]
- Chen, H.-J.; Zhu, K.-D. Graphene-based nanoresonator with applications in optical transistor and mass sensing. Sensors 2014, 14, 16740–16753. [Google Scholar] [CrossRef]
- Wang, Q.; Arash, B. A review on applications of carbon nanotubes and graphenes as nano-resonator sensors. Comput. Mater. Sci. 2014, 82, 350–360. [Google Scholar] [CrossRef]
- Asadi, E.; Askari, H.; Khamesee, M.B.; Khajepour, A. High frequency nano electromagnetic self-powered sensor: Concept, modelling and analysis. Measurements 2017, 107, 31–40. [Google Scholar] [CrossRef]
- Duan, K.; Li, L.; Hu, Y.; Wang, X. Pillared graphene as an ultra-high sensitivity mass sensor. Sci. Rep. 2017, 7, 14012. [Google Scholar] [CrossRef] [PubMed]
- Kwon, S.H.; Kim, H.J.; Choi, W.S.; Kang, H. Development and performance analysis of carbon nanowall-based mass sensor. J. Nanosci. Nanotechnol. 2018, 18, 6552–6554. [Google Scholar] [CrossRef]
- Ricci, F.; Cuairan, M.T.; Conangla, G.P.; Schell, A.W.; Quidant, R. Accurate mass measurement of a levitated nanomechanical resonator for precision force-sensing. Nano Lett. 2019, 19, 6711–6715. [Google Scholar] [CrossRef]
- Willemsen, O.H.; Snel, M.M.; Cambi, A.; Greve, J.; De Grooth, B.G.; Figdor, C.G. Biomolecular interactions measured by atomic force microscopy. Biophys. J. 2000, 79, 3267–3281. [Google Scholar] [CrossRef]
- Stampfer, C.; Helbling, T.; Obergfell, D.; Schöberle, B.; Tripp, M.K.; Jungen, A.; Roth, S.; Bright, A.V.M.; Hierold, C. Fabrication of single-walled carbon-nanotube-based pressure sensors. Nano Lett. 2006, 6, 233–237. [Google Scholar] [CrossRef] [PubMed]
- Gopal, A.; Luo, Z.; Kumar, K.; Lee, J.Y.; Hoshino, K.; Li, B.; Schmidt, C.; Ho, P.S.; Zhang, X. Nano-grating force sensor for measurement of neuron membrane characteristics under growth and cellular differentiation. In Proceedings of the TRANSDUCERS 2007-2007 International Solid-State Sensors, Actuators and Microsystems Conference, Lion, France, 10–14 June 2007; pp. 1239–1242. [Google Scholar]
- Abadie, J.; Piat, E.; Oster, S.; Boukallel, M. Modeling and experimentation of a passive low frequency nanoforce sensor based on diamagnetic levitation. Sens. Actuators A Phys. 2012, 173, 227–237. [Google Scholar] [CrossRef]
- Piat, E.; Abadie, J.; Oster, S. Nanoforce estimation based on Kalman filtering and applied to a force sensor using diamagnetic levitation. Sens. Actuators A Phys. 2012, 179, 223–236. [Google Scholar] [CrossRef]
- Li, T.; Li, L.; Song, W.; Zhang, G.; Li, Y. Design and modeling of a novel two dimensional nano-scaled force sensor based on silicon photonic crystal. ECS Trans. 2014, 58, 65–73. [Google Scholar] [CrossRef]
- Yu, N.; Nakajima, M.; Shi, Q.; Yang, Z.; Wang, H.; Sun, L.; Huang, Q.; Fukuda, T. Characterization of the resistance and force of a carbon nanotube/Metal side contact by nanomanipulation. Scanning 2017, 2017, 1–11. [Google Scholar] [CrossRef]
- Mata-Hernandez, D.; Fernández, D.; Banerji, S.; Madrenas, J. Resonant mems pressure sensor in 180 NM cmos technology obtained by BEOL isotropic etching. Sensors 2020, 20, 6037. [Google Scholar] [CrossRef]
- Wang, X.; Zhou, J.; Song, J.; Liu, J.; Xu, A.N.; Wang, Z.L. Piezoelectric field effect transistor and nanoforce sensor based on a single zno nanowire. Nano Lett. 2006, 6, 2768–2772. [Google Scholar] [CrossRef] [PubMed]
- Kim, M.-S.; Choi, J.-H.; Park, Y.-K.; Kim, J.-H. Atomic force microscope cantilever calibration device for quantified force metrology at micro- or nano-scale regime: The nano force calibrator (NFC). Metrologia 2006, 43, 389–395. [Google Scholar] [CrossRef]
- Duc, T.C.; Creemer, J.F.; Sarro, P.M. Lateral nano-Newton force-sensing piezoresistive cantilever for microparticle handling. J. Micromech. Microeng. 2006, 16, S102–S106. [Google Scholar] [CrossRef]
- Mandal, D.; Yoon, S.; Kim, K.J. Origin of piezoelectricity in an electrospun poly(Vinylidene fluoride-trifluoroethylene) Nanofiber web-based nanogenerator and nano-pressure sensor. Macromol. Rapid Commun. 2011, 32, 831–837. [Google Scholar] [CrossRef] [PubMed]
- Zhou, Y.S.; Hinchet, R.; Mouis, M.; Wang, Z.L.; Yang, Y.; Ardila, G.; Songmuang, R.; Zhang, F.; Zhang, Y.; Han, W.; et al. Nano-newton transverse force sensor using a vertical gan nanowire based on the piezotronic effect. Adv. Mater. 2012, 25, 883–888. [Google Scholar] [CrossRef]
- Kou, J.; Zhang, Y.; Liu, Y.; Zhang, K.; Liu, W.; Zhai, J. Nano-force sensor based on a single tellurium microwire. Semicond. Sci. Technol. 2017, 32, 074001. [Google Scholar] [CrossRef]
- Paul, S.J.; Sharma, I.; Elizabeth, I.; Gahtori, B.; Titus, S.S.; Chandra, P.; Gupta, B.K. A comparative study of compressible and conductive vertically aligned carbon nanotube forest in different polymer matrixes for high-performance piezoresistive force sensors. ACS Appl. Mater. Interfaces 2020, 12, 16946–16958. [Google Scholar] [CrossRef]
- Mahata, C.; Algadi, H.; Lee, J.; Kim, S.; Lee, T. Biomimetic-inspired micro-nano hierarchical structures for capacitive pressure sensor applications. Measurement 2020, 151, 107095. [Google Scholar] [CrossRef]
- Bahadoran, M.; Noorden, A.F.A.; Chaudhary, K.; Aziz, M.S.; Ali, J.; Yupapin, P. Nano force sensing using symmetric double stage micro resonator. Measurement 2014, 58, 215–220. [Google Scholar] [CrossRef]
- Hong, T.; Wang, T.; Xu, Y.-Q. Direct measurement of π coupling at the single-molecule level using a carbon nanotube force sensor. Nano Lett. 2018, 18, 7883–7888. [Google Scholar] [CrossRef]
- Lu, H.; Zheng, Y.; Jiang, J.; Yin, W.; Meng, Y.; Tian, Y. A shadow-based nano scale precision force sensor. IEEE Sens. J. 2018, 19, 2072–2078. [Google Scholar] [CrossRef]
- Yoo, J.-Y.; Seo, M.-H.; Lee, J.-S.; Choi, K.-W.; Jo, M.-S.; Yoon, J.-B. Industrial grade, bending-insensitive, transparent nanoforce touch sensor via enhanced percolation effect in a hierarchical nanocomposite film. Adv. Funct. Mater. 2018, 28. [Google Scholar] [CrossRef]
- Hierold, C.; Jungen, A.; Stampfer, C.; Helbling, T. Nano electromechanical sensors based on carbon nanotubes. Sens. Actuators A Phys. 2007, 136, 51–61. [Google Scholar] [CrossRef]
- Zang, H.; Zhang, X.; Zhu, B.; Fatikow, S. Recent advances in non-contact force sensors used for micro/nano manipulation. Sens. Actuators A Phys. 2019, 296, 155–177. [Google Scholar] [CrossRef]
- Li, C.-Y.; Chou, T.-W. Strain and pressure sensing using single-walled carbon nanotubes. Nanotechnology 2004, 15, 1493–1496. [Google Scholar] [CrossRef]
- Kürti, J.; Kresse, G.; Kuzmany, H. First-principles calculations of the radial breathing mode of single-wall carbon nanotubes. Phys. Rev. B 1998, 58, R8869–R8872. [Google Scholar] [CrossRef]
- Liu, H.J.; Chan, C.T. Properties of4Åcarbon nanotubes from first-principles calculations. Phys. Rev. B 2002, 66, 115416. [Google Scholar] [CrossRef]
- Gillen, R.; Mohr, M.; Thomsen, C.; Maultzsch, J. Vibrational properties of graphene nanoribbons by first-principles calculations. Phys. Rev. B 2009, 80, 155418. [Google Scholar] [CrossRef]
- Muller, S.E.; Nair, A.K. Carbyne as a fiber in metal-matrix nanocomposites: A first principle study. Comput. Mater. Sci. 2019, 159, 187–193. [Google Scholar] [CrossRef]
- Yakobson, B.I.; Brabec, C.J.; Bernholc, J. Nanomechanics of carbon tubes: Instabilities beyond linear response. Phys. Rev. Lett. 1996, 76, 2511–2514. [Google Scholar] [CrossRef] [PubMed]
- Prylutskyy, Y.; Durov, S.; Ogloblya, O.; Buzaneva, E.; Scharff, P. Molecular dynamics simulation of mechanical, vibrational and electronic properties of carbon nanotubes. Comput. Mater. Sci. 2000, 17, 352–355. [Google Scholar] [CrossRef]
- Ansari, R.; Ajori, S.; Arash, B. Vibrations of single- and double-walled carbon nanotubes with layerwise boundary conditions: A molecular dynamics study. Curr. Appl. Phys. 2012, 12, 707–711. [Google Scholar] [CrossRef]
- Jing, N.; Xue, Q.; Ling, C.; Shan, M.; Zhang, T.; Zhou, X.; Jiao, Z. Effect of defects on Young’s modulus of graphene sheets: A molecular dynamics simulation. RSC Adv. 2012, 2, 9124–9129. [Google Scholar] [CrossRef]
- Arash, B.; Wang, Q. A review on the application of nonlocal elastic models in modeling of carbon nanotubes and graphenes. Comput. Mater. Sci. 2012, 51, 303–313. [Google Scholar] [CrossRef]
- Arash, B.; Jiang, J.-W.; Rabczuk, T. A review on nanomechanical resonators and their applications in sensors and molecular transportation. Appl. Phys. Rev. 2015, 2, 021301. [Google Scholar] [CrossRef]
- Eltaher, M.; Khater, M.; Emam, S.A. A review on nonlocal elastic models for bending, buckling, vibrations, and wave propagation of nanoscale beams. Appl. Math. Model. 2016, 40, 4109–4128. [Google Scholar] [CrossRef]
- Askari, H.; Younesian, D.; Esmailzadeh, E.; Cveticanin, L. Nonlocal effect in carbon nanotube resonators: A comprehensive review. Adv. Mech. Eng. 2017, 9, 1–24. [Google Scholar] [CrossRef]
- Lei, X.-W.; Natsuki, T.; Shi, J.-X.; Ni, Q.-Q. Radial breathing vibration of double-walled carbon nanotubes subjected to pressure. Phys. Lett. A 2011, 375, 2416–2421. [Google Scholar] [CrossRef]
- Lei, X.-W.; Ni, Q.-Q.; Shi, J.-X.; Natsuki, T. Radial breathing mode of carbon nanotubes subjected to axial pressure. Nanoscale Res. Lett. 2011, 6, 492. [Google Scholar] [CrossRef]
- Fazelzadeh, S.; Ghavanloo, E. Nonlocal anisotropic elastic shell model for vibrations of single-walled carbon nanotubes with arbitrary chirality. Compos. Struct. 2012, 94, 1016–1022. [Google Scholar] [CrossRef]
- Ansari, R.; Rouhi, H.; Sahmani, S. Free vibration analysis of single- and double-walled carbon nanotubes based on nonlocal elastic shell models. J. Vib. Control. 2012, 20, 670–678. [Google Scholar] [CrossRef]
- Avramov, K. Nonlinear vibrations characteristics of single-walled carbon nanotubes by nonlocal elastic shell model. Int. J. Non-Linear Mech. 2018, 107, 149–160. [Google Scholar] [CrossRef]
- Lei, X.-W.; Natsuki, T.; Shi, J.-X.; Ni, Q.-Q. Surface effects on the vibrational frequency of double-walled carbon nanotubes using the nonlocal Timoshenko beam model. Compos. Part B Eng. 2012, 43, 64–69. [Google Scholar] [CrossRef]
- Shi, J.-X.; Natsuki, T.; Lei, X.-W.; Ni, Q.-Q. Buckling instability of carbon nanotube atomic force microscope probe clamped in an elastic medium. J. Nanotechnol. Eng. Med. 2012, 3, 020903. [Google Scholar] [CrossRef]
- Natsuki, T.; Ni, Q.-Q.; Elishakoff, I. Influence of the axial compression on the natural frequency of AFM probes using double-walled carbon nanotubes with different wall lengths. Appl. Phys. A 2012, 110, 1–7. [Google Scholar] [CrossRef]
- Natsuki, T.; Urakami, K. Analysis of vibration frequency of carbon nanotubes used as nano-force sensors considering clamped boundary condition. Electronics 2019, 8, 1082. [Google Scholar] [CrossRef]
- Shi, J.-X.; Ni, Q.-Q.; Lei, X.-W.; Natsuki, T. Nonlocal elasticity theory for the buckling of double-layer graphene nanoribbons based on a continuum model. Comput. Mater. Sci. 2011, 50, 3085–3090. [Google Scholar] [CrossRef]
- Shi, J.-X.; Ni, Q.-Q.; Lei, X.-W.; Natsuki, T. Wave propagation in embedded double-layer graphene nanoribbons as electromechanical oscillators. J. Appl. Phys. 2011, 110, 84321. [Google Scholar] [CrossRef]
- Shi, J.-X.; Ni, Q.-Q.; Lei, X.-W.; Natsuki, T. Nonlocal vibration of embedded double-layer graphene nanoribbons in in-phase and anti-phase modes. Phys. E Low-Dimens. Syst. Nanostruct. 2012, 44, 1136–1141. [Google Scholar] [CrossRef]
- Nazemnezhad, R.; Kamali, K.; Hosseini-Hashemi, S. Study on tensile-compressive and shear effects of van der Waals interactions on free vibration of bilayer graphene nanoribbons. Meccanica 2016, 52, 263–282. [Google Scholar] [CrossRef]
- Shenderova, O.A.; Zhirnov, V.V.; Brenner, D.W. Carbon nanostructures. Crit. Rev. Solid State Mater. Sci. 2002, 27, 227–356. [Google Scholar] [CrossRef]
- DiBiasio, C.M.; Cullinan, M.A.; Culpepper, M.L. Difference between bending and stretching moduli of single-walled carbon nanotubes that are modeled as an elastic tube. Appl. Phys. Lett. 2007, 90, 203116. [Google Scholar] [CrossRef]
- Pine, P.; Yaish, Y.E.; Adler, J. Vibrational analysis of thermal oscillations of single-walled carbon nanotubes under axial strain. Phys. Rev. B 2014, 89, 115405. [Google Scholar] [CrossRef]
- Li, C.; Chou, T.-W. A structural mechanics approach for the analysis of carbon nanotubes. Int. J. Solids Struct. 2003, 40, 2487–2499. [Google Scholar] [CrossRef]
- Cornell, W.D.; Cieplak, P.; Bayly, C.I.; Gould, I.R.; Merz, K.M.; Ferguson, D.M.; Spellmeyer, D.C.; Fox, T.; Caldwell, J.W.; Kollman, P.A. A second generation force field for the simulation of proteins, nucleic acids, and organic molecules. J. Am. Chem. Soc. 1995, 117, 5179–5197. [Google Scholar] [CrossRef]
- Natsuki, T.; Tantrakarn, K.; Endo, M. Prediction of elastic properties for single-walled carbon nanotubes. Carbon 2004, 42, 39–45. [Google Scholar] [CrossRef]
- Li, C.; Chou, T.-W. Single-walled carbon nanotubes as ultrahigh frequency nanomechanical resonators. Phys. Rev. B 2003, 68, 073405. [Google Scholar] [CrossRef]
- Xiao, J.; Gama, B.; Gillespie, J. An analytical molecular structural mechanics model for the mechanical properties of carbon nanotubes. Int. J. Solids Struct. 2005, 42, 3075–3092. [Google Scholar] [CrossRef]
- Meo, M.; Rossi, M. Prediction of Young’s modulus of single wall carbon nanotubes by molecular-mechanics based finite element modelling. Compos. Sci. Technol. 2006, 66, 1597–1605. [Google Scholar] [CrossRef]
- Shokrieh, M.M.; Rafiee, R. Prediction of Young’s modulus of graphene sheets and carbon nanotubes using nanoscale continuum mechanics approach. Mater. Des. 2010, 31, 790–795. [Google Scholar] [CrossRef]
- Rafiee, R.; Moghadam, R.M. On the modeling of carbon nanotubes: A critical review. Compos. Part B Eng. 2014, 56, 435–449. [Google Scholar] [CrossRef]
- Eltaher, M.A.; Agwa, M.; Kabeel, A. Vibration analysis of material size-dependent CNTs using energy equivalent model. J. Appl. Comput. Mech. 2018, 4, 75–86. [Google Scholar] [CrossRef]
- Mohamed, N.; Mohamed, S.A.; Eltaher, M.A. Buckling and post-buckling behaviors of higher order carbon nanotubes using energy-equivalent model. Eng. Comput. 2020. [Google Scholar] [CrossRef]
- Reddy, C.D.; Rajendran, S.; Liew, K.M. Equilibrium configuration and continuum elastic properties of finite sized graphene. Nanotechnology 2006, 17, 864–870. [Google Scholar] [CrossRef]
- Duan, W.H.; Wang, C.M. Nonlinear bending and stretching of a circular graphene sheet under a central point load. Nanotechnology 2009, 20, 075702. [Google Scholar] [CrossRef]
- Shi, J.-X.; Natsuki, T.; Lei, X.-W.; Ni, Q.-Q. Equivalent Young’s modulus and thickness of graphene sheets for the continuum mechanical models. Appl. Phys. Lett. 2014, 104, 223101. [Google Scholar] [CrossRef]
- Yeh, Y.-K.; Hwu, C. A modified molecular-continuum model for estimating the strength and fracture toughness of graphene and carbon nanotube. Eng. Fract. Mech. 2017, 176, 326–342. [Google Scholar] [CrossRef]
- Korobeynikov, S.; Alyokhin, V.; Babichev, A. On the molecular mechanics of single layer graphene sheets. Int. J. Eng. Sci. 2018, 133, 109–131. [Google Scholar] [CrossRef]
- Yeh, Y.-K.; Hwu, C. Molecular-continuum model for the prediction of stiffness, strength and toughness of nanomaterials. Acta Mech. 2017, 230, 1451–1467. [Google Scholar] [CrossRef]
- Mokhalingam, A.; Ghaffari, R.; Sauer, R.A.; Gupta, S.S. Comparing quantum, molecular and continuum models for graphene at large deformations. Carbon 2020, 159, 478–494. [Google Scholar] [CrossRef]
- Dresselhaus, M.; Dresselhaus, G.; Saito, R. Physics of carbon nanotubes. Carbon 1995, 33, 883–891. [Google Scholar] [CrossRef]
- Lu, J.P. Elastic properties of single and multilayered nanotubes. J. Phys. Chem. Solids 1997, 58, 1649–1652. [Google Scholar] [CrossRef]
- Huang, Y.; Wu, J.; Hwang, K.C. Thickness of graphene and single-wall carbon nanotubes. Phys. Rev. B 2006, 74, 245413. [Google Scholar] [CrossRef]
- Lee, C.; Wei, X.; Kysar, J.W.; Hone, J. Measurement of the elastic properties and intrinsic strength of monolayer graphene. Science 2008, 321, 385–388. [Google Scholar] [CrossRef] [PubMed]
- Liu, M.; Artyukhov, V.I.; Lee, H.; Xu, F.; Yakobson, B.I. Carbyne from first principles: Chain of C atoms, a nanorod or a nanorope. ACS Nano 2013, 7, 10075–10082. [Google Scholar] [CrossRef]
- Liu, M.; Artyukhov, V.I.; Lee, H.; Xu, F.; Yakobson, B.I. Correction to carbyne from first-principles: Chain of C atoms, a nanorod or a nanorope. ACS Nano 2017, 11, 5186. [Google Scholar] [CrossRef]
- Shi, J.-X.; Liu, Y.; Shimoda, M. Vibration analysis of a carbyne-based resonator in nano-mechanical mass sensors. J. Phys. D Appl. Phys. 2015, 48, 115303. [Google Scholar] [CrossRef]
- Agwa, M.A.; Eltaher, M.A. Vibration of a carbyne nanomechanical mass sensor with surface effect. Appl. Phys. A 2016, 122, 335. [Google Scholar] [CrossRef]
- Zhao, Y.; Luo, Q.; Wu, J.; Sui, C.; Tong, L.; He, X.; Wang, C. Mechanical properties of helically twisted carbyne fibers. Int. J. Mech. Sci. 2020, 186, 105823. [Google Scholar] [CrossRef]
- Li, C.; Chou, T.-W. Mass detection using carbon nanotube-based nanomechanical resonators. Appl. Phys. Lett. 2004, 84, 5246–5248. [Google Scholar] [CrossRef]
- Wu, D.H.; Chien, W.T.; Chen, C.S.; Chen, H.H. Resonant frequency analysis of fixed-free single-walled carbon nanotube-based mass sensor. Sens. Actuators A Phys. 2006, 126, 117–121. [Google Scholar] [CrossRef]
- Li, Y.; Qiu, X.; Yang, F.; Wang, X.-S.; Yin, Y. Ultra-high sensitivity of super carbon-nanotube-based mass and strain sensors. Nanotechnology 2008, 19, 165502. [Google Scholar] [CrossRef] [PubMed]
- Chowdhury, R.; Adhikari, S.; Mitchell, J. Vibrating carbon nanotube based bio-sensors. Phys. E Low-Dimens. Syst. Nanostruct. 2009, 42, 104–109. [Google Scholar] [CrossRef]
- Georgantzinos, S.; Anifantis, N. Carbon nanotube-based resonant nanomechanical sensors: A computational investigation of their behavior. Phys. E Low-Dimens. Syst. Nanostruct. 2010, 42, 1795–1801. [Google Scholar] [CrossRef]
- Joshi, A.Y.; Harsha, S.; Sharma, S.C. Vibration signature analysis of single walled carbon nanotube based nanomechanical sensors. Phys. E: Low-Dimens. Syst. Nanostruct. 2010, 42, 2115–2123. [Google Scholar] [CrossRef]
- Cho, S.-H.; Choi, M.-S.; Kang, D.-K.; Lee, J.H.; Kim, C.-W. Analysis on mass sensing characteristics of SWCNT-based nano-mechanical resonators using continuum mechanics based finite element analysis. J. Mech. Sci. Technol. 2015, 29, 4801–4806. [Google Scholar] [CrossRef]
- Lee, H.-L.; Hsu, J.-C.; Chang, W.-J. Frequency shift of carbon-nanotube-based mass sensor using nonlocal elasticity theory. Nanoscale Res. Lett. 2010, 5, 1774–1778. [Google Scholar] [CrossRef]
- Aydogdu, M.; Filiz, S. Modeling carbon nanotube-based mass sensors using axial vibration and nonlocal elasticity. Phys. E Low-Dimens. Syst. Nanostruct. 2011, 43, 1229–1234. [Google Scholar] [CrossRef]
- Mehdipour, I.; Barari, A.; Domairry, G. Application of a cantilevered SWCNT with mass at the tip as a nanomechanical sensor. Comput. Mater. Sci. 2011, 50, 1830–1833. [Google Scholar] [CrossRef]
- Shen, Z.-B.; Tang, G.-J.; Zhang, L.; Li, X.-F. Vibration of double-walled carbon nanotube based nanomechanical sensor with initial axial stress. Comput. Mater. Sci. 2012, 58, 51–58. [Google Scholar] [CrossRef]
- Shen, Z.-B.; Li, D.-K.; Li, N.; Tang, G.-J. Frequency shift of a nanomechanical sensor carrying a nanoparticle using nonlocal Timoshenko beam theory. J. Mech. Sci. Technol. 2012, 26, 1577–1583. [Google Scholar] [CrossRef]
- Natsuki, T.; Matsuyama, N.; Shi, J.-X.; Ni, Q.-Q. Vibration analysis of nanomechanical mass sensor using carbon nanotubes under axial tensile loads. Appl. Phys. A 2014, 116, 1001–1007. [Google Scholar] [CrossRef]
- Natsuki, T.; Matsuyama, N.; Ni, Q.-Q. Vibration analysis of carbon nanotube-based resonator using nonlocal elasticity theory. Appl. Phys. A 2015, 120, 1309–1313. [Google Scholar] [CrossRef]
- Bouchaala, A.; Nayfeh, A.H.; Younis, M.I. Frequency shifts of micro and nano cantilever beam resonators due to added masses. J. Dyn. Syst. Meas. Control. 2016, 138, 091002. [Google Scholar] [CrossRef]
- Eltaher, M.; Agwa, M. Analysis of size-dependent mechanical properties of CNTs mass sensor using energy equivalent model. Sens. Actuators A Phys. 2016, 246, 9–17. [Google Scholar] [CrossRef]
- Eltaher, M.A.; Agwa, M.A.; Mahmoud, F.F. Nanobeam sensor for measuring a zeptogram mass. Int. J. Mech. Mater. Des. 2015, 12, 211–221. [Google Scholar] [CrossRef]
- Ghaffari, S.S.; Ceballes, S.; Abdelkefi, A. Effects of thermal loads representations on the dynamics and characteristics of carbon nanotubes-based mass sensors. Smart Mater. Struct. 2019, 28, 074003. [Google Scholar] [CrossRef]
- Ghaffari, S.S.; Ceballes, S.; Abdelkefi, A. Nonlinear dynamical responses of forced carbon nanotube-based mass sensors under the influence of thermal loadings. Nonlinear Dyn. 2020, 100, 1013–1035. [Google Scholar] [CrossRef]
- Natsuki, T. Carbon nanotube-based nanomechanical sensor: Theoretical analysis of mechanical and vibrational properties. Electronics 2017, 6, 56. [Google Scholar] [CrossRef]
- Tsiamaki, A.; Georgantzinos, S.; Anifantis, N. Monolayer graphene resonators for mass detection: A structural mechanics feasibility study. Sens. Actuators A Phys. 2014, 217, 29–38. [Google Scholar] [CrossRef]
- Xu, C.; Rong, D.; Tong, Z.; Zhou, Z.; Hu, J.; Deng, Z. Analytical modeling of a magnetically affected cantilever nanoplate-based mass detector. Superlattices Microstruct. 2020, 137, 106338. [Google Scholar] [CrossRef]
- Xu, C.; Qu, J.; Rong, D.; Zhou, Z.; Leung, A. Theory and modeling of a novel class of nanoplate-based mass sensors with corner point supports. Thin-Walled Struct. 2021, 159, 107306. [Google Scholar] [CrossRef]
- Natsuki, T.; Shi, J.-X.; Ni, Q.-Q. Vibration analysis of nanomechanical mass sensor using double-layered graphene sheets resonators. J. Appl. Phys. 2013, 114, 094307. [Google Scholar] [CrossRef]
- Lei, X.-W.; Natsuki, T.; Shi, J.-X.; Ni, Q.-Q. An atomic-resolution nanomechanical mass sensor based on circular monolayer graphene sheet: Theoretical analysis of vibrational properties. J. Appl. Phys. 2013, 113, 154313. [Google Scholar] [CrossRef]
- Shen, Z.-B.; Tang, H.-L.; Li, D.-K.; Tang, G.-J. Vibration of single-layered graphene sheet-based nanomechanical sensor via nonlocal Kirchhoff plate theory. Comput. Mater. Sci. 2012, 61, 200–205. [Google Scholar] [CrossRef]
- Lee, H.-L.; Yang, Y.-C.; Chang, W.-J. Mass detection using a graphene-based nanomechanical resonator. Jpn. J. Appl. Phys. 2013, 52, 25101. [Google Scholar] [CrossRef]
- Jalali, S.K.; Naei, M.H.; Pugno, N.M. A mixed approach for studying size effects and connecting interactions of planar nano structures as resonant mass sensors. Microsyst. Technol. 2014, 21, 2375–2386. [Google Scholar] [CrossRef]
- Zhou, S.-M.; Sheng, L.-P.; Shen, Z.-B. Transverse vibration of circular graphene sheet-based mass sensor via nonlocal Kirchhoff plate theory. Comput. Mater. Sci. 2014, 86, 73–78. [Google Scholar] [CrossRef]
- Natsuki, T. Theoretical analysis of vibration frequency of graphene sheets used as nanomechanical mass sensor. Electronics 2015, 4, 723–738. [Google Scholar] [CrossRef]
- Natsuki, T.; Yiwada, A.; Natsuki, J. Influence of temperature on vibrational frequency of graphene sheet used as nano-scale sensing. C J. Carbon Res. 2017, 3, 4. [Google Scholar] [CrossRef]
- Shen, Z.-B.; Jiang, R.-W.; Zhang, L.; Tang, G.-J. Nonlocal galerkin strip transfer function method for vibration of double-layered graphene mass sensor. Acta Mech. Solida Sin. 2018, 31, 94–107. [Google Scholar] [CrossRef]
- Rajabi, K.; Hosseini-Hashemi, S. Effect of the interparticle interactions on adsorption-induced frequency shift of nano-beam-based nanoscale mass-sensors: A Theoretical study. J. Solid Mech. 2018, 10, 864–873. [Google Scholar]
- Li, H.; Wang, X.; Wang, H.; Chen, J. The nonlocal frequency behavior of nanomechanical mass sensors based on the multi-directional vibrations of a buckled nanoribbon. Appl. Math. Model. 2020, 77, 1780–1796. [Google Scholar] [CrossRef]
- Whittaker, A.G. Carbon: A new view of its high-temperature behavior. Science 1978, 200, 763–764. [Google Scholar] [CrossRef]
- Whittaker, A. Carbon: Occurrence of carbyne forms of carbon in natural graphite. Carbon 1979, 17, 21–24. [Google Scholar] [CrossRef]
- Smith, P.P.K.; Buseck, P.R.; Pettengill, G.H.; Ford, P.G.; Nozette, S. Carbyne forms of carbon: Do they exist? Science 1982, 216, 984–986. [Google Scholar] [CrossRef] [PubMed]
- Menacer, F.; Kadr, A.; Dibi, Z. Modeling of a smart nano force sensor using finite elements and neural networks. Int. J. Autom. Comput. 2018, 17, 279–291. [Google Scholar] [CrossRef]

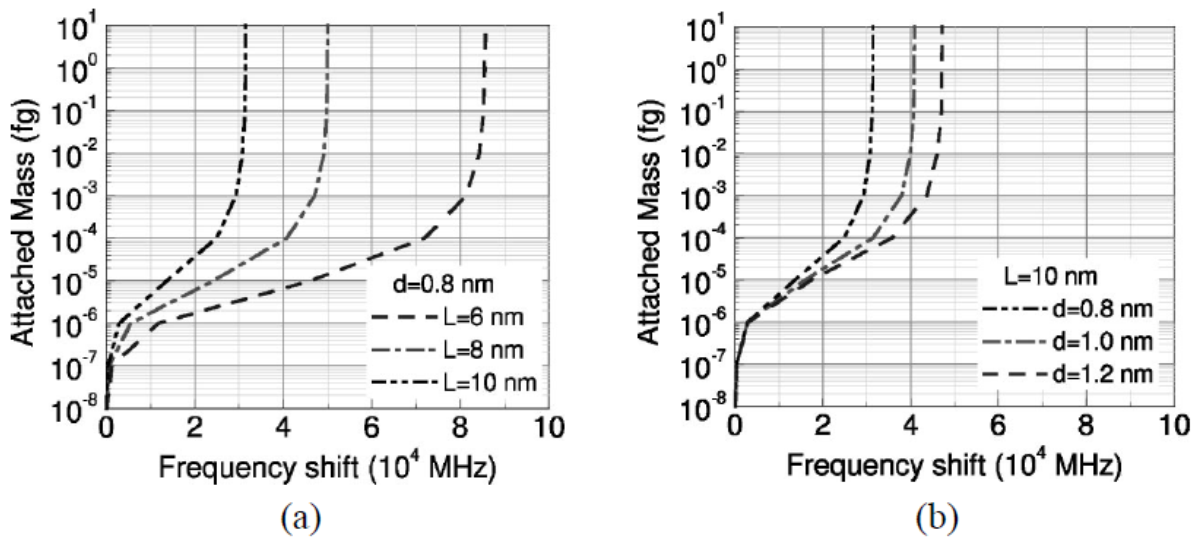

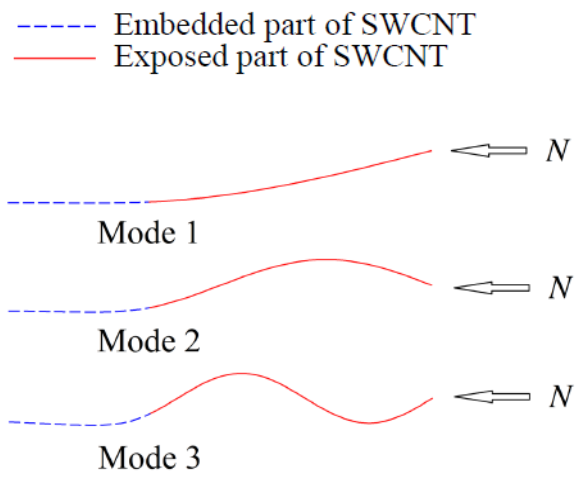
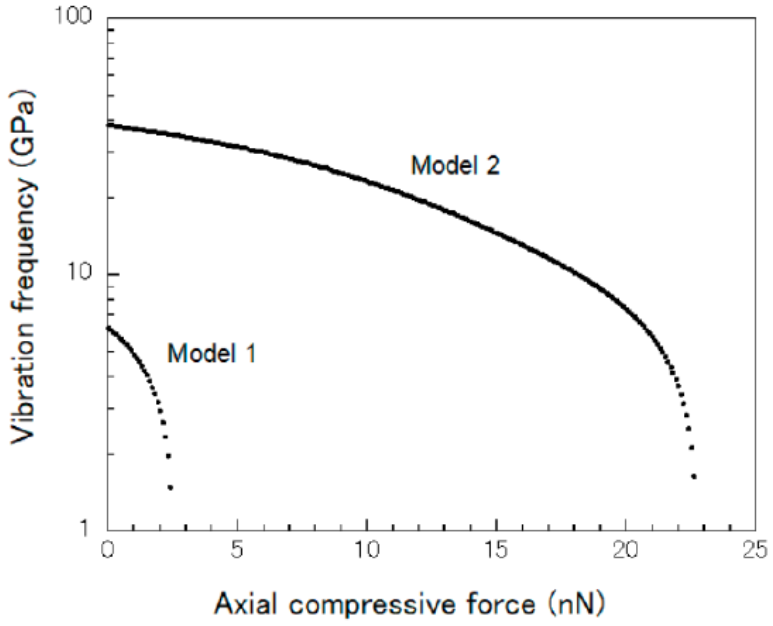
| Author (s) | Information of CNTs | Boundary Condition (s) | Methods | Sensitivity |
|---|---|---|---|---|
| Li and Chou [123] | SWCNTs with a diameter of 0.8 nm, lengths of 6, 8 and 10 nm | Cantilevered, bridged | MSM, FEM | >10−21 g |
| Wu et al. [127] | SWCNTs with diameters of 24.9, 37.55 and 44.9 nm, lengths of 5.55, 4.65 and 5.75 µm | Cantilevered | EBT, FEM | >10−21 g |
| Li et al. [128] | [6,0]@(6,0) Super CNTs with diameters of 10.81, 7.99 and 5.17 nm, lengths of 29.273, 21.6 and 13.926 nm | Cantilevered, bridged | MSM, FEM | Super CNTs is 6.2~8.87 times of SWCNTs |
| Chowdhury et al. [129] | SWCNTs with diameter of 1.1m, lengths of 4.1, 5.6 and 8.0 nm | Cantilevered, bridged | EBT, FEM | >10−21 g |
| Georgantzinos and Anifantis [130] | SWCNTs with diameters of 0.54, 0.8 and 1.09 nm, lengths of 6, 8 and 10 nm DWCNTs with inner diameter of 0.41, 1.09 and 1.76 nm, outer diameter of 2.44 nm, length of 17 nm | Cantilevered, bridged | MSM, FEM | SWCNTs is 2 times of DWCNTs |
| Joshi et al. [131] | SWCNTs with diameter of 0.8 nm, lengths of 6, 8 and 10 nm | Cantilevered, bridged | EBT, FEM | >10−21 g |
| Cho et al. [132] | SWCNTs with diameter of 2.7 nm, length of 55 nm | Cantilevered, bridged | FEM | >2 × 10−18 g |
| Lee et al. [133] | SWCNTs with diameter of 1.1 nm, lengths of 4.1, 5.6 and 8.0 nm | Cantilevered | Nonlocal EBT | >10−21 g |
| Aydogdu and Filiz [134] | SWCNTs with diameter of 1 nm, length of 10 nm | Cantilevered, bridged | Nonlocal EBT | >10−21 g |
| Mehdipour et al. [135] | SWCNTs with diameter of 25.3 nm, length of 5.5 µm | Cantilevered | EBT | >2 × 10−14 g |
| Shen et al. [136] | SWCNT with diameter of 1.05 nm, lengths of 14, 28 and 42 nm DWCNTs with inner diameter of 0.7 nm, outer diameter of 1.4 nm, lengths of 14, 28 and 42 nm | Bridged | Nonlocal EBT | >10−21 g |
| Shen et al. [137] | SWCNTs with diameter of 1.1 nm, lengths of 11, 22, and 33 | Bridged | Nonlocal TBT | >10−21 g |
| Natsuki et al. [138] | SWCNTs with diameter of 1 nm, lengths of 10, 20 and 50 nm | Bridged under axial tensile load | EBT | >10−22 g |
| Natsuki et al. [139] | SWCNTs with diameter of 1 nm, length of 20 nm | Bridged under axial tensile load | Nonlocal EBT | >10−22 g |
| Bouchaala et al. [140] | CNTs with diameter of 5 nm, length of 1000 nm | Cantilevered under direct current load | EBT | >7.735 × 10−21 g |
| Eltaher and Agwa [141] | armchair (5,5), (7,7), (10,10), (15,15) and zigzag (5,0), (7,0), (10,0), (15,0) SWCNTs with length of 1.6 nm | Bridged under axial tensile load | MSM, TBT | >10−22 g |
| Eltaher et al. [142] | CNTs with diameter of 5 nm, lengths of 50, 100 and 250 nm | Bridged | Nonlocal EBT | >5 × 10−21 g |
| Ghaffari et al. [143] | CNTs with diameter of 0.8 nm~ 8 nm, lengths of 25, 50, 75 and 100 nm | Bridged under thermal load | Nonlocal EBT | >6.65 × 10−24 g |
| Ghaffari et al. [144] | CNTs with dimensionless parameters | Bridged under thermal load | Nonlocal EBT | >0.218 × 10−24 g |
| Author (s) | Information of GSs | Boundary Condition (s) | Method (s) | Sensitivity |
|---|---|---|---|---|
| Tsiamaki et al. [146] | Circular SLGSs with diameter of 1 nm~10 nm | Clamped | MSM, FEM | >10−22 g |
| Xu et al. [147] | Rectangular SLGSs of 10 × 5~20 nm | Cantilevered | EPT, FEM | >10−22 g |
| Xu et al. [148] | Rectangular SLGSs of 10 × 5~20 nm | Three cases | EPT, FEM | >10−22 g |
| Natsuki et al. [149] | Rectangular SLGSs of 13.6 × 13.6 nm Rectangular DLGSs of 13.6 × 6.8~27.2 nm | Simply supported | EPT | >10−22 g DLGSs is higher than SLGSs |
| Lei et al. [150] | Circular SLGSs with diameter of 3.4~17 nm | Clamped | EPT | >10−24 g |
| Shen et al. [151] | Rectangular SLGSs of 10~30 nm × 10~30 nm | Simply supported | Nonlocal EPT | >10−21 g |
| Lee et al. [152] | Rectangular SLGSs of 10 × 10 nm | Simply supported | Nonlocal EPT | >10−27 g/Hz |
| Jalali et al. [153] | Rectangular SLGSs with dimensionless parameters | Clamped, simply supported | Nonlocal EBT | Not mentioned |
| Zhou et al. [154] | Circular SLGSs with diameter of 10 nm, 15 nm, and 20 nm | Clamped, simply supported | Nonlocal EPT | >10− 21 g |
| Natsuki [155] | Rectangular SLGSs of 5.08 × 5.08 nm Rectangular DLGSs of 5.08 × 2.54~10.16 nm | Simply supported | Nonlocal EPT | >10−22 g DLGSs is higher than SLGSs |
| Natsuki et al. [156] | Rectangular SLGSs of 5.08 × 5.08 nm Rectangular DLGSs of 5.08 × 5.08 nm | Simply supported under thermal load | Nonlocal EPT | >10−22 g DLGSs is higher than SLGSs |
| Shen et al. [157] | Rectangular DLGSs of 10 × 10 nm | Clamped, simply supported | Nonlocal EPT | >10−24 g |
| Rajabi and Hosseini-Hashemi [158] | SLGNR of 16 × 2 nm | Cantilevered | EBT | >10−15 g |
| Li et al. [159] | Buckled GNR of 50 × 5 nm | Clamped | Nonlocal EBT, FEM | Not mentioned |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, J.-X.; Lei, X.-W.; Natsuki, T. Review on Carbon Nanomaterials-Based Nano-Mass and Nano-Force Sensors by Theoretical Analysis of Vibration Behavior. Sensors 2021, 21, 1907. https://doi.org/10.3390/s21051907
Shi J-X, Lei X-W, Natsuki T. Review on Carbon Nanomaterials-Based Nano-Mass and Nano-Force Sensors by Theoretical Analysis of Vibration Behavior. Sensors. 2021; 21(5):1907. https://doi.org/10.3390/s21051907
Chicago/Turabian StyleShi, Jin-Xing, Xiao-Wen Lei, and Toshiaki Natsuki. 2021. "Review on Carbon Nanomaterials-Based Nano-Mass and Nano-Force Sensors by Theoretical Analysis of Vibration Behavior" Sensors 21, no. 5: 1907. https://doi.org/10.3390/s21051907
APA StyleShi, J.-X., Lei, X.-W., & Natsuki, T. (2021). Review on Carbon Nanomaterials-Based Nano-Mass and Nano-Force Sensors by Theoretical Analysis of Vibration Behavior. Sensors, 21(5), 1907. https://doi.org/10.3390/s21051907







