Soft and Hard Iron Compensation for the Compasses of an Operational Towed Hydrophone Array without Sensor Motion by a Helmholtz Coil
Abstract
:1. Introduction
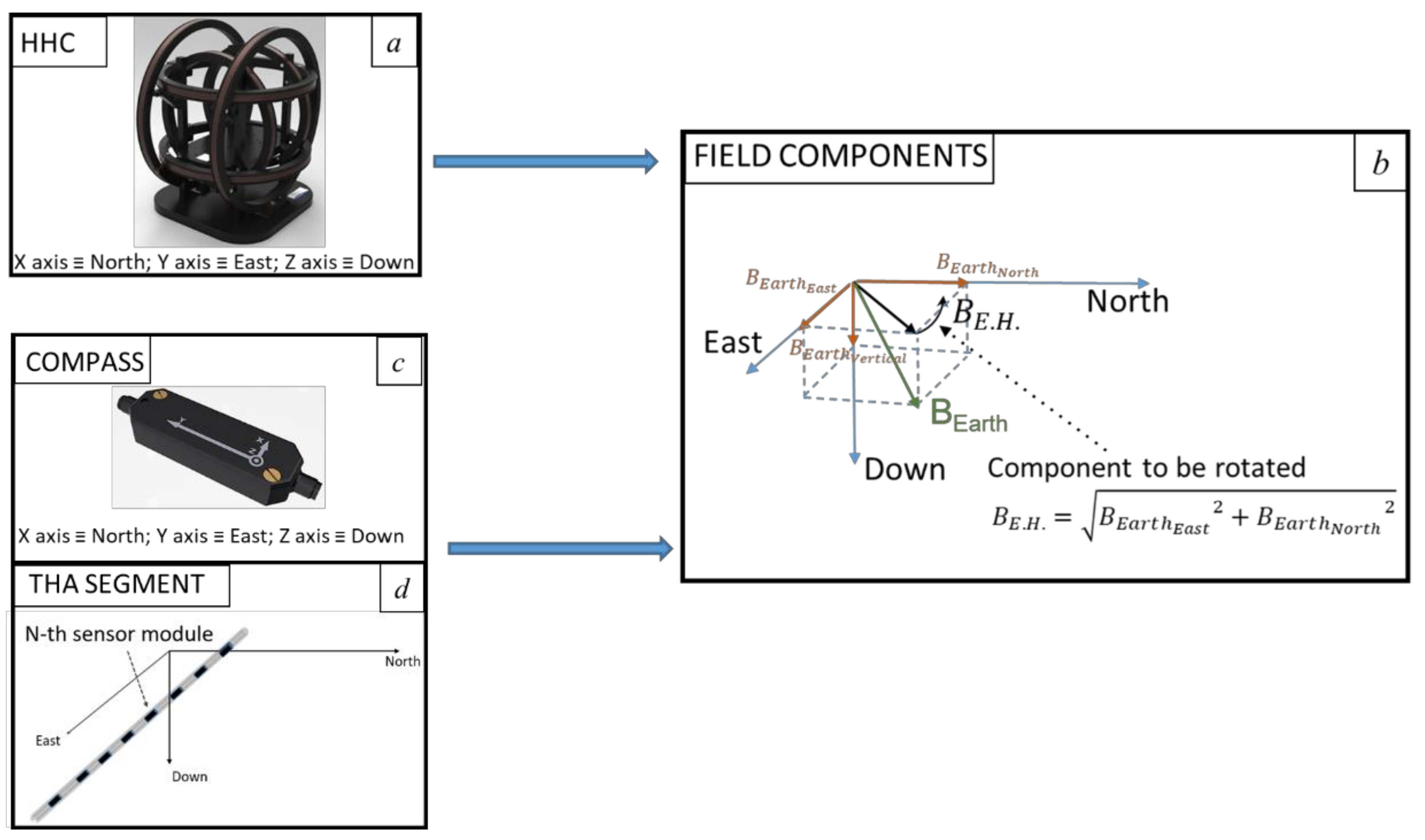
2. Soft and Hard Iron Compensation Simulating Towed Hydrophone Array Motion
- (1)
- To generate a magnetic field sufficiently homogeneous inside a region. This region shall be large to contain the segment of the hydrophone array;
- (2)
- To be able to produce a uniform magnetic field in any direction;
- (3)
- To be reprogrammable through a PC since the field produced by the laboratory set-up depends upon the location;
- (4)
- To generate a magnetic induction comparable with the Earth’s magnetic flux density (i.e., about 50 μT or 500 mG).
3. Experimental Procedure
4. Discussion on the Artificially Generated Magnetic Field
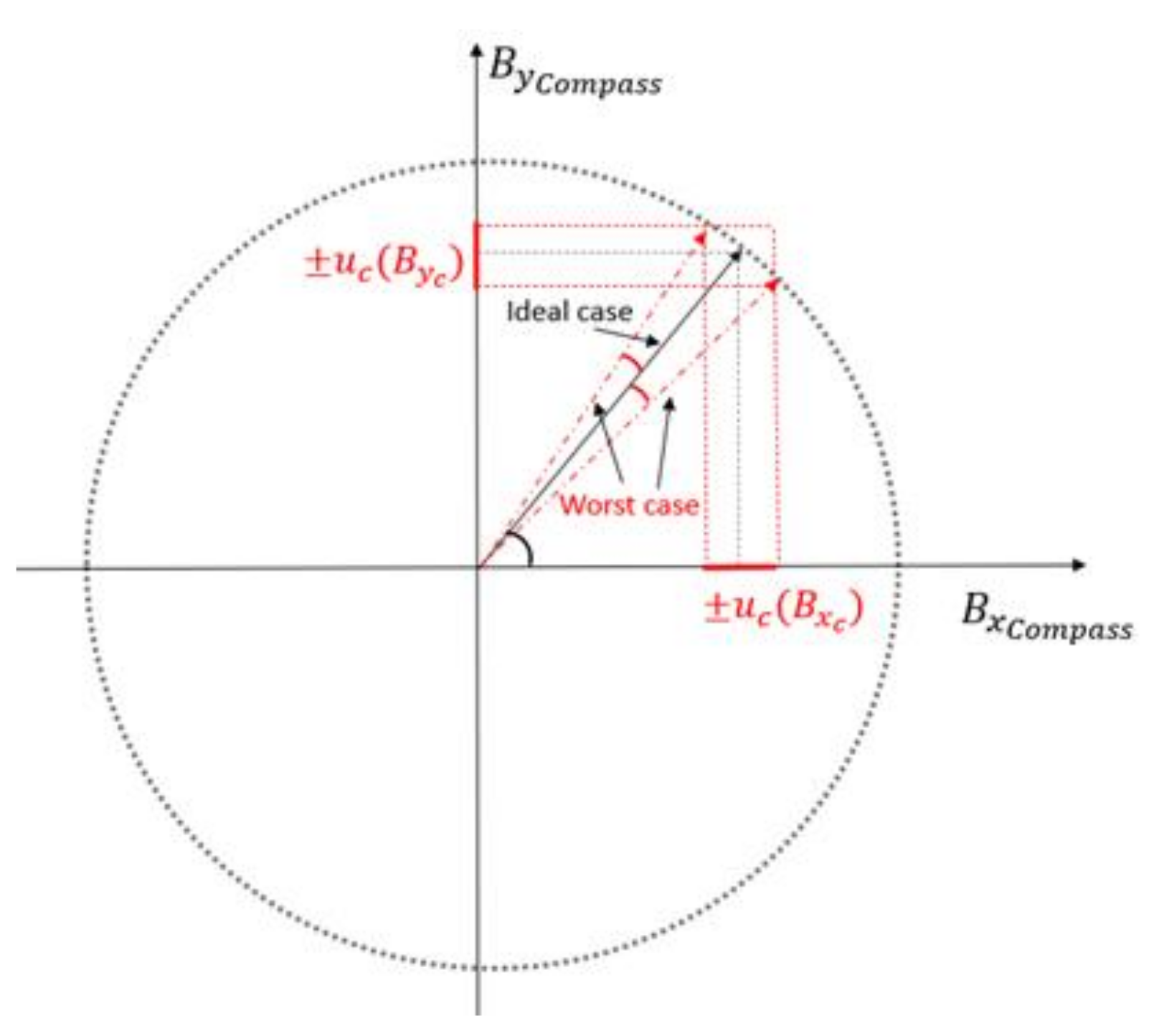
5. Uncertainty Analysis
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Barbagelata, A.; Guerrini, P.; Troiano, L. Thirty Years of Towed Arrays at NURC. Oceanography 2008, 21, 24–33. [Google Scholar] [CrossRef]
- Felisberto, P.; Jesus, S.M. Towed array beamforming during ship’s maneuvering. IEE Radar Sonar Navig. 1996, 143, 210–215. [Google Scholar] [CrossRef]
- Calibration of Compasses Using Helmholtz Coil Systems. Application Note. Available online: https://www.bartington.com/wp-content/uploads/pdfs/application_notes/AN0051.pdf (accessed on 18 November 2019).
- Zikmund, A.; Janosek, M.; Ulvr, M.; Kupec, J. Precise Calibration Method for Triaxial Magnetometers Not Requiring Earth’s Field Compensation. IEEE Trans. Instrum. Meas. 2015, 64, 1242–1247. [Google Scholar] [CrossRef]
- Available online: https://patents.google.com/patent/WO2018006020A1/en (accessed on 15 September 2021).
- Liu, Z.; Zhang, Q.; Pan, M.; Shan, Q.; Geng, Y.; Guan, F.; Chen, D.; Tian, W. Distortion Magnetic Field Compensation of Geomagnetic Vector Measurement System Using a 3-D Helmholtz Coil. IEEE Geosci. Remote Sens. Lett. 2017, 14, 48–51. [Google Scholar] [CrossRef]
- Inoue, A.; Kong, F. Soft Magnetic Materials. Encycl. Smart Mater. 2022, 5, 10–23. [Google Scholar] [CrossRef]
- Xsens. Magnetic Calibration Manual. Document MT0202P. Available online: https://www.xsens.com/hubfs/Downloads/Manuals/MT_Magnetic_Calibration_Manual.pdf (accessed on 1 March 2020).
- Garton, M.; Wutka, A.; Leuzinger, A. Local Magnetic Distortion Effects on 3-Axis Compassing. PNI Sens. Corp. Tech. Rep. 2009. Available online: https://www.pnicorp.com/download/magnetic-distortion-explanation-testing/ (accessed on 18 November 2019).
- Calibrating an eCompass in the Presence of Hard-and Soft-Iron Interference. Application Note Document Number: AN4246 Rev. 4.0, 11/2015. Available online: https://www.nxp.com/docs/en/application-note/AN4246.pdf (accessed on 18 November 2019).
- Fan, B.; Li, Q.; Tao, L. How Magnetic Disturbance Influences the Attitude and Heading in Magnetic and Inertial Sensor-Based Orientation Estimation. Sensors 2018, 18, 76. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Papafotis, K.; Nikitas, D.; Sotiriadis, P.P. Magnetic Field Sensors’ Calibration: Algorithms’ Overview and Comparison. Sensors 2021, 21, 5288. [Google Scholar] [CrossRef] [PubMed]
- Fang, J.; Sun, H.; Cao, J.; Zhang, X.; Tao, Y. A novel calibration method of magnetic compass based on ellipsoid fitting. IEEE Trans. Instrum. Meas. 2011, 60, 2053–2061. [Google Scholar] [CrossRef]
- Liu, Y.X.; Li, X.S.; Zhang, X.J.; Feng, Y.B. Novel Calibration Algorithm for a Three-Axis Strapdown Magnetometer. Sensors 2014, 14, 8485–8504. [Google Scholar] [CrossRef] [PubMed]
- MicroMagnetics. SpinCoil 7-9-11-XYZ: Three-Axis Helmholtz Coils User Manual Rev.3. Available online: http://www.micromagnetics.com/products_hhccontrol.html (accessed on 18 November 2019).
- Maguer, A.; Dymond, R.; Mazzi, M.; Biagini, S. SLITA: A new slim towed array for AUV applications. J. Acoust. Soc. Am. 2008, 123, 3005. [Google Scholar] [CrossRef] [Green Version]
- Caruso, M.J. Applications of magnetic sensors for low cost compass systems. In Proceedings of the IEEE 2000 Position Location and Navigation Symposium, San Diego, CA, USA, 13–16 March 2000. [Google Scholar]
- Joint Committee for Guides in Metrology. Evaluation of measurement data—Guide to the expression of uncertainty in measurement. JCGM 2008, 100, 1–116. [Google Scholar]



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lapucci, T.; Troiano, L.; Carobbi, C.; Capineri, L. Soft and Hard Iron Compensation for the Compasses of an Operational Towed Hydrophone Array without Sensor Motion by a Helmholtz Coil. Sensors 2021, 21, 8104. https://doi.org/10.3390/s21238104
Lapucci T, Troiano L, Carobbi C, Capineri L. Soft and Hard Iron Compensation for the Compasses of an Operational Towed Hydrophone Array without Sensor Motion by a Helmholtz Coil. Sensors. 2021; 21(23):8104. https://doi.org/10.3390/s21238104
Chicago/Turabian StyleLapucci, Tommaso, Luigi Troiano, Carlo Carobbi, and Lorenzo Capineri. 2021. "Soft and Hard Iron Compensation for the Compasses of an Operational Towed Hydrophone Array without Sensor Motion by a Helmholtz Coil" Sensors 21, no. 23: 8104. https://doi.org/10.3390/s21238104
APA StyleLapucci, T., Troiano, L., Carobbi, C., & Capineri, L. (2021). Soft and Hard Iron Compensation for the Compasses of an Operational Towed Hydrophone Array without Sensor Motion by a Helmholtz Coil. Sensors, 21(23), 8104. https://doi.org/10.3390/s21238104







