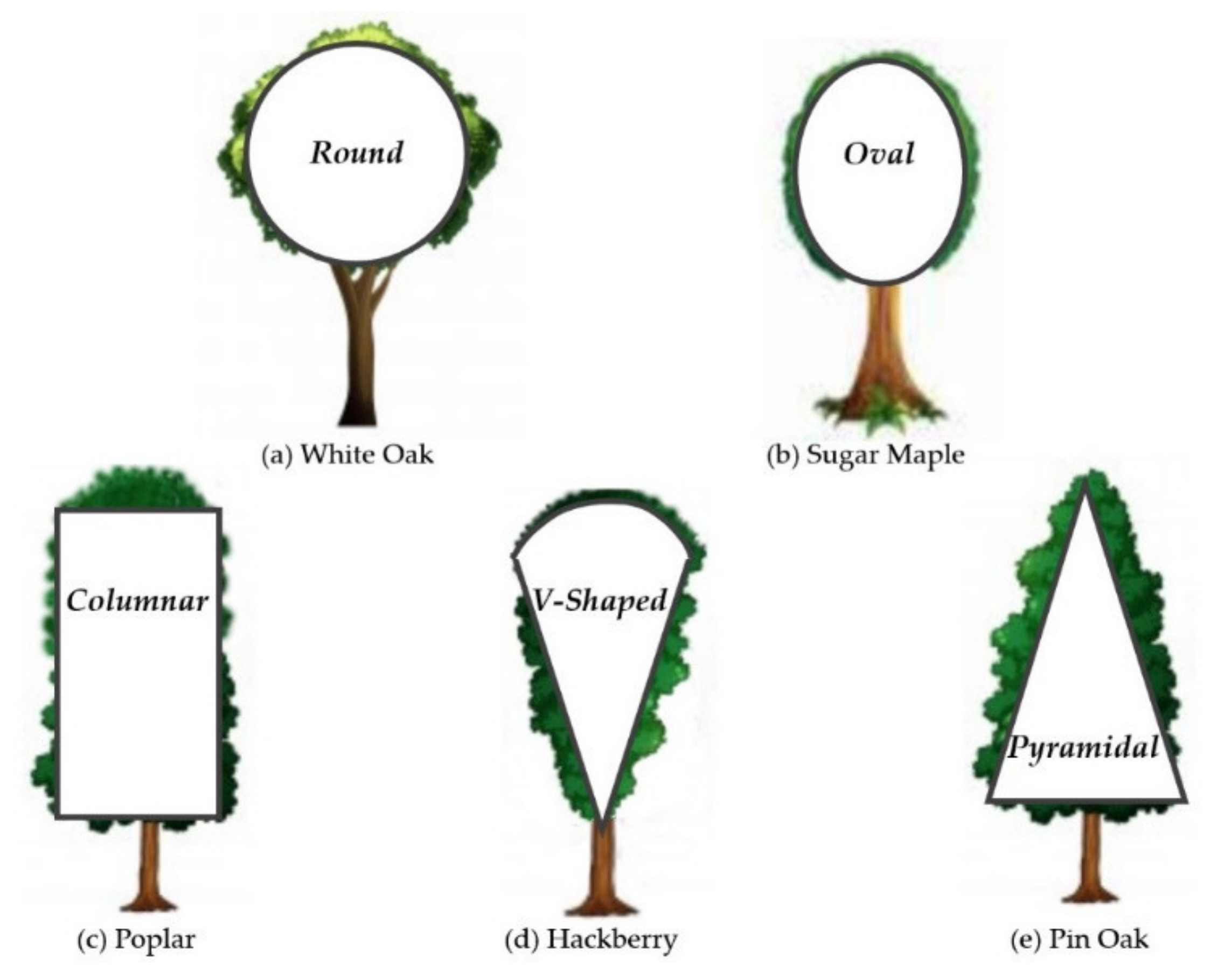
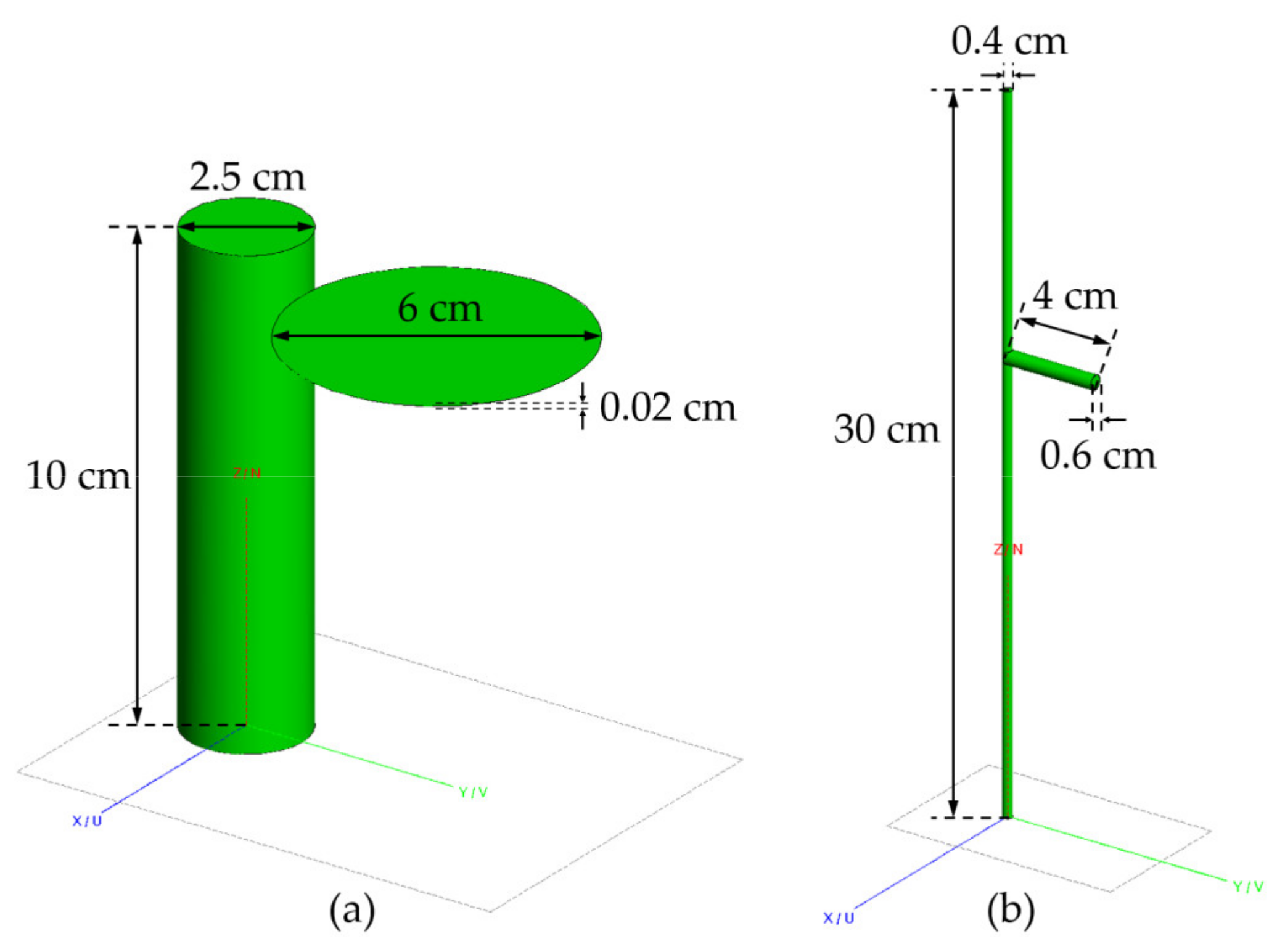
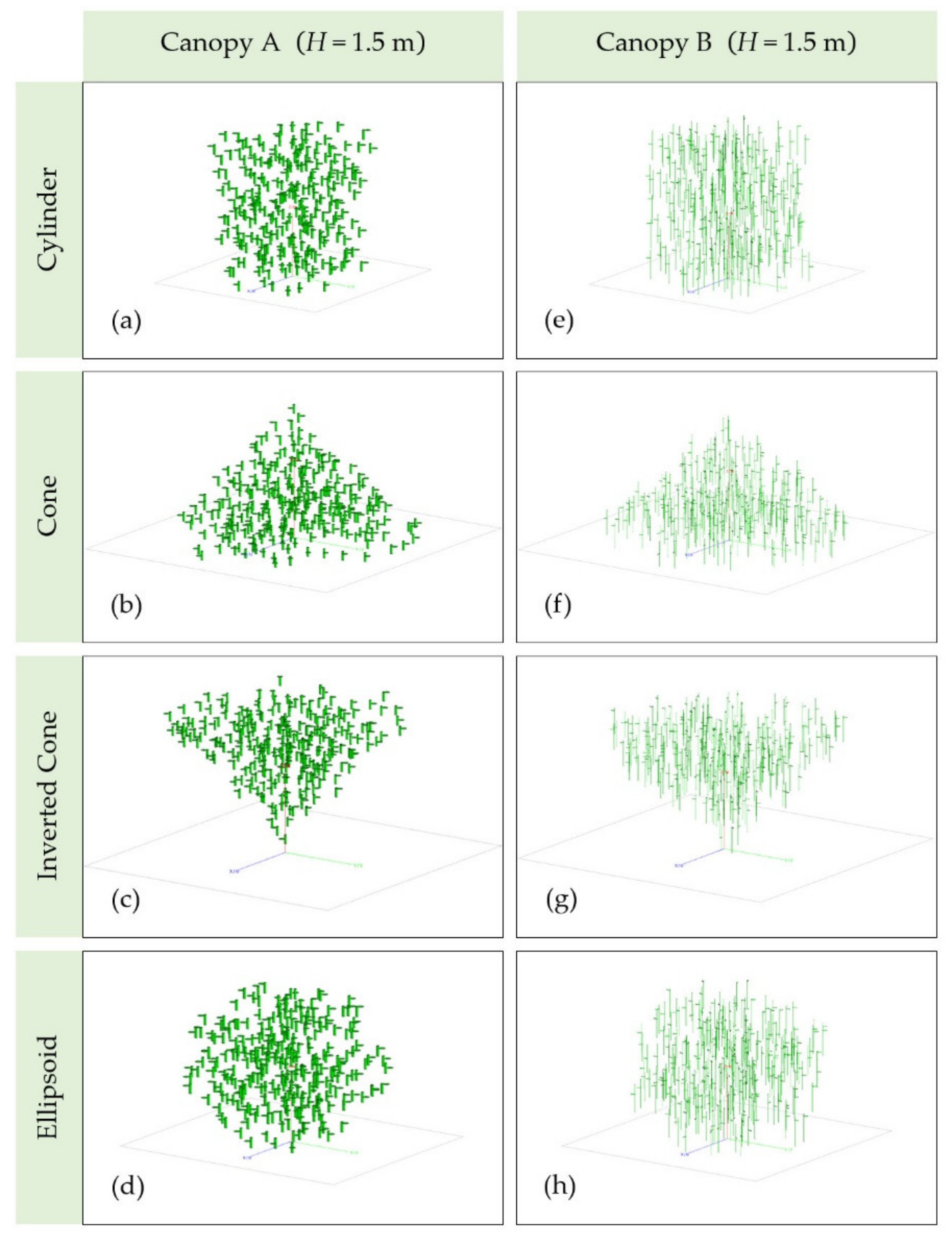
3.1. MTVM Simulation Results
Using the Modified Tor Vergata Model, the simulation results for the four vegetation canopies with different crown shapes are shown in
Figure 7,
Figure 8,
Figure 9,
Figure 10,
Figure 11 and
Figure 12.
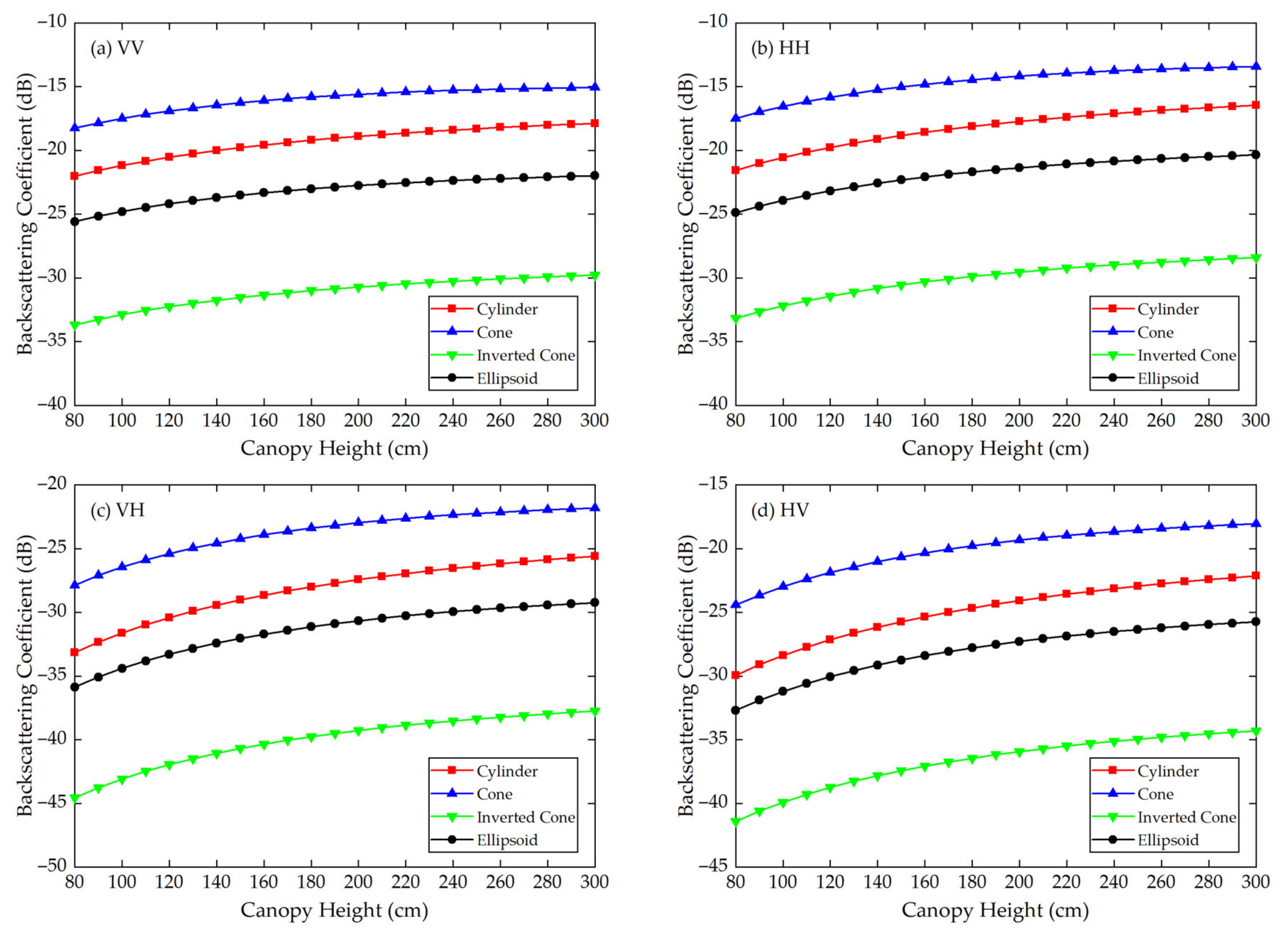
Figure 7,
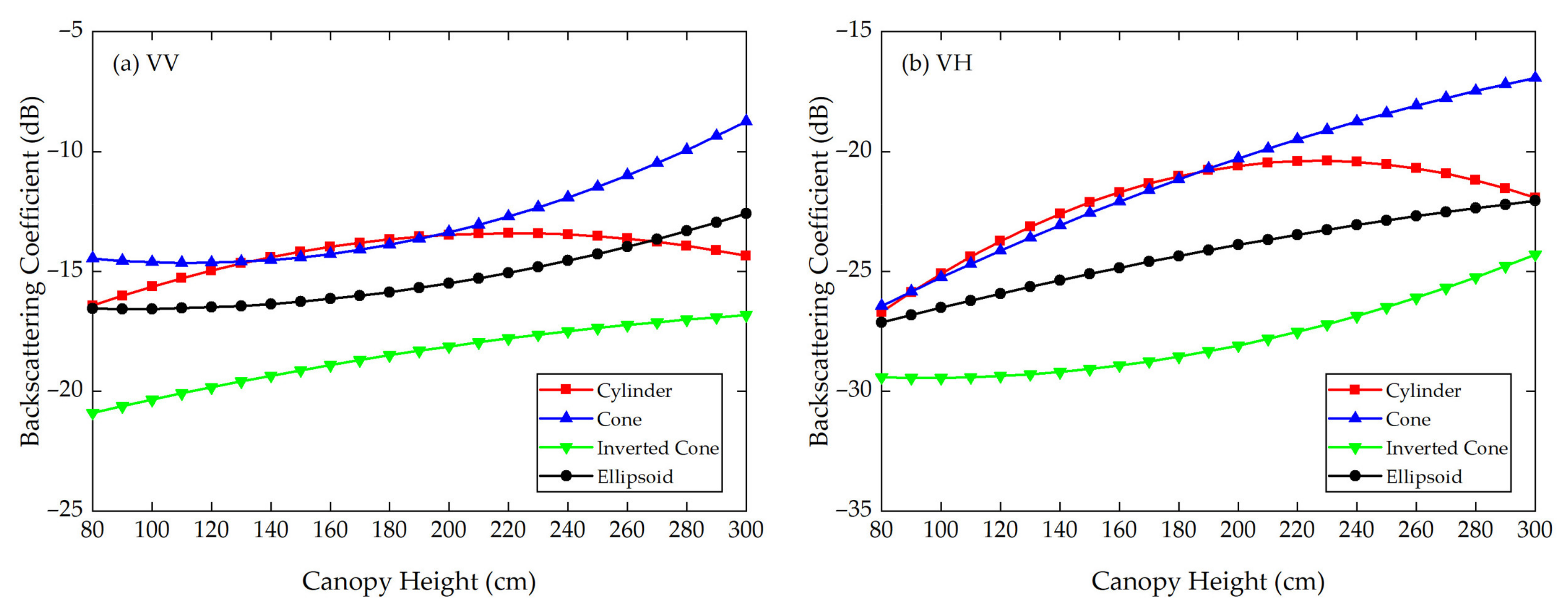
Figure 8 and
Figure 9 illustrate the results at VV, HH, VH and HV polarizations in L (1.2 GHz), C (5.3 GHz) and X (9.6 GHz) bands with the parameters of canopy A as inputs;
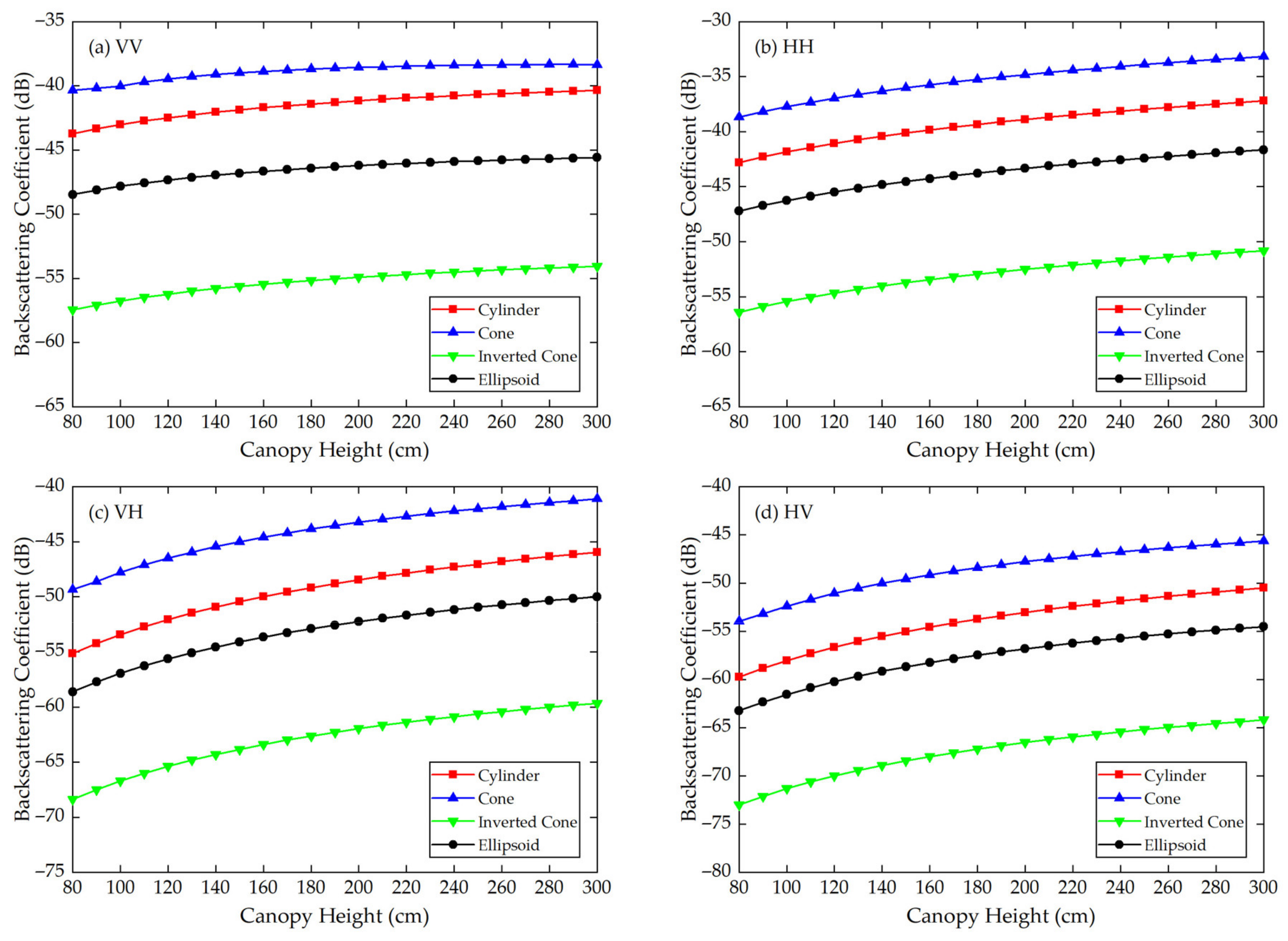
Figure 10,
Figure 11 and
Figure 12 show analogous results but with the parameters of canopy B as inputs. The four vegetation canopies have vegetation components with the same volume density (
8 × 10
−5 cm
−3), and the crown heights of the four canopies remain the same (
H = 80–300 cm).
For canopies A and B, at VV, HH, HV and VH polarizations in L, C and X bands,
Figure 7,
Figure 8,
Figure 9,
Figure 10,
Figure 11 and
Figure 12 all show that the backscattering coefficients of vegetation canopies with different crown shapes increase gradually as the canopy height increases from 80 cm to 300 cm, except for the cone canopy at VV, VH and HV polarizations in the L band and at VV polarization in the X band, for which the MTVM simulation results slightly decrease with the increase in crown height.
The mean absolute difference (MAD)
and the mean relative difference (MRD)
were used to evaluate the differences between the backscattering coefficients for the three crown shapes studied and the reference cylinder crown shape. The results are presented in
Table 4 and
Table 5. For two series of numbers
and
, expressed in dB,
and
are defined as
The units of
and
are dB and percent, which we do not repeatedly mention in the following content. In
Table 4 and
Table 5, the upper row is
and the lower row is
for every polarization.
For canopy A, the largest values between the three crown shapes studied and the reference cylinder crown shape are 14.55 dB, 11.82 dB and 11.76 dB, and they occur at VH (L band), HV (C band) and VV (X band) polarizations, respectively. The smallest values occur at VV (L band), HV (C band) and VH/HV (X band) polarizations, and the corresponding values are 1.32 dB, 3.17 dB and 2.97 dB. Moreover, the largest occurs between the inverted cone and reference cylinder canopies, and the smallest occurs between the cone and reference cylinder canopies for the L band and between the ellipsoid and cylinder canopies for C and X bands.
The largest values for canopy A are 126.68%, 64.87% and 50.26% and occur between the inverted cone and cylinder canopies at VV (L band) and HH (C and X bands) polarizations. The smallest values occur between cone and cylinder canopies at HV (L band) polarization with a value of 11.17% and between the ellipsoid and cylinder canopies at VH (C and X bands) polarization with values of 11.39% and 9.99%.
Analogously, for canopy B, the largest values between the three crown shapes studied and the reference cylinder crown shape are 11.60 dB, 13.74 dB and 11.70 dB, which occur between the inverted cone and cylinder canopies at VV (L, C and X bands) polarization. The smallest values occur between the ellipsoid and cylinder canopies at HV (L band) polarization with a value of 2.37 dB and between the cone and cylinder canopies at VV (C and X bands) polarization with values of 2.63 dB and 2.59 dB.
The largest values for canopy B are 29.41%, 34.61% and 32.90% and occur between the inverted cone and cylinder canopies at VV (L band) and HH (C and X bands) polarizations. The smallest values occur between the ellipsoid and cylinder canopies at HV (L and X bands) polarization with values of 2.88% and 5.94% and between the cone and cylinder canopies at VV (C band) polarization with a value of 6.31%.
For the given parameters, the maximum mean absolute difference in backscattering coefficients between different crown shapes is 14.55 dB (for canopy A at VH polarization in L band), and the maximum mean relative deviation between the studied canopy and the reference cylinder canopy is 126.68% (for canopy A at VV polarization in L band) (
Table 4). Taking
Figure 9a as an example, the mean absolute differences between the cone, inverted cone and ellipsoid canopies and the reference cylinder canopy are 4.40 dB, 11.76 dB and 3.19 dB, and the absolute differences between values for the cone and inverted cone are as high as about 18 dB. Therefore, it can be concluded that the crown shape has a non-negligible influence on microwave backscattering coefficients of the vegetation canopy.
The ranking of backscattering coefficients is , which is approximately the same ranking order as that of the volume fraction of the lower half of the vegetation canopy, for which the fractions are cone (75%) > cylinder (50%) = ellipsoid (50%) > inverted cone (25%). This correspondence can possibly be attributed to the attenuation effects of the upper canopy components; in other words, a lower volume fraction produces less attenuation, increasing the backscattering coefficients of the lower part of the canopy. However, a quantitative explanation of this result requires further analysis.
Moreover, we can see in
Figure 7,
Figure 8,
Figure 9,
Figure 10,
Figure 11 and
Figure 12 that, for a given crown shape, the backscattering coefficients and their relative differences for A are both greater than those for B in the same band and polarization. This indicates that the crown shape effect acts synergistically with the effects of vegetation component parameters, which mainly include the geometrical and physical parameters of the vegetation components.
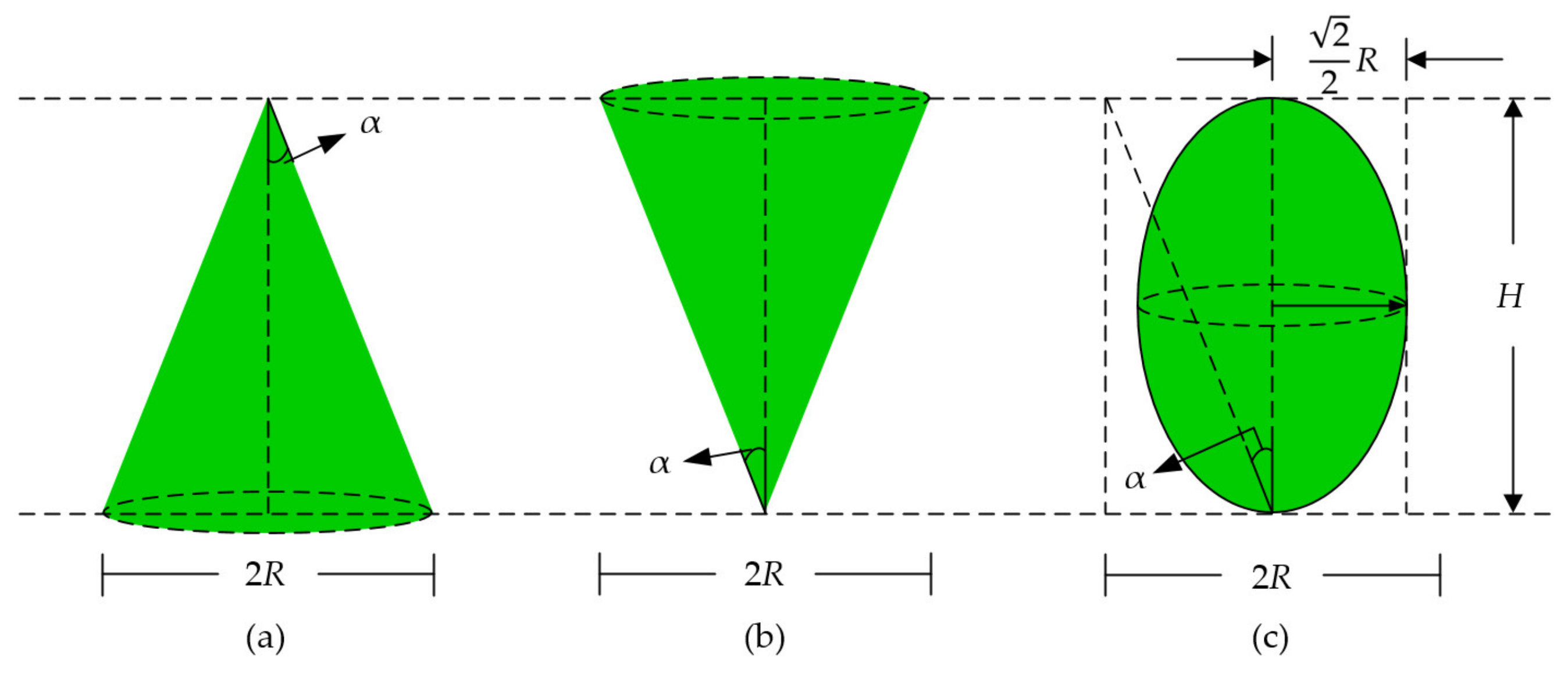
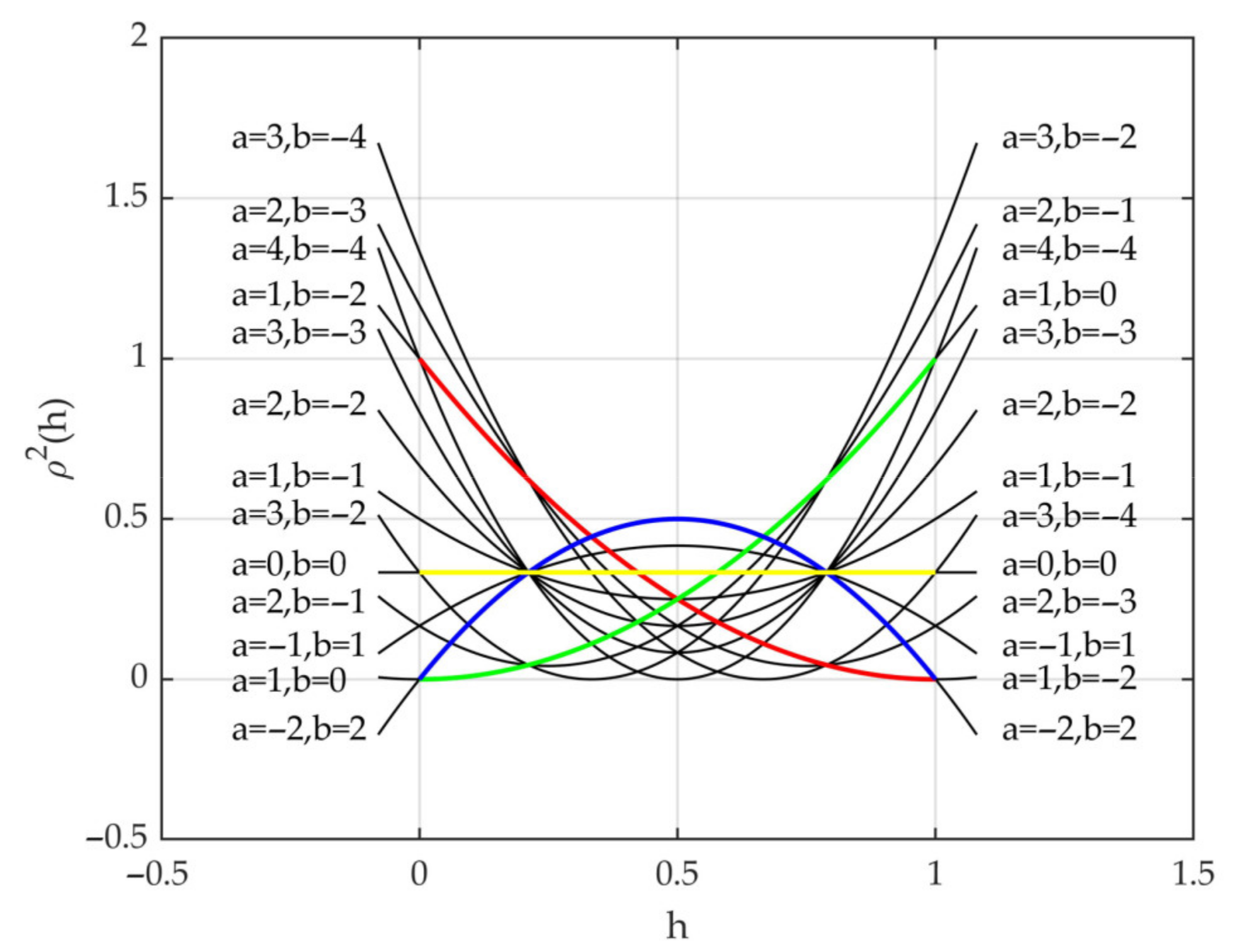
In addition, we also note that a great difference exists between the cone and inverted cone canopies. A set of curves is used to illustrate the gradualness of the continuous change from the cone canopy to the inverted cone canopy and its influence on the backscattering coefficients of the vegetation canopy. For the four crown shapes, the horizontal profiles at a certain height are all circles whose areas can be expressed with a quadratic curve as
, so the parabolic curve is the best choice to represent the continuous change in crown shapes. The parabolic vegetation canopy can be defined as
Likewise, to ensure that the crown shape is the unique variable of the vegetation canopy, assuming that all other canopy parameters are the same, the volume of the three vegetation canopies in
Figure 3 ought to remain the same as that of the reference cylinder canopy. It is defined as
By integrating Equation (27), we obtain
As a result, the parabolic vegetation canopies should meet the requirements in Equation (28), which constrains the geometries of the crown shapes. The constraint conditions are as follows
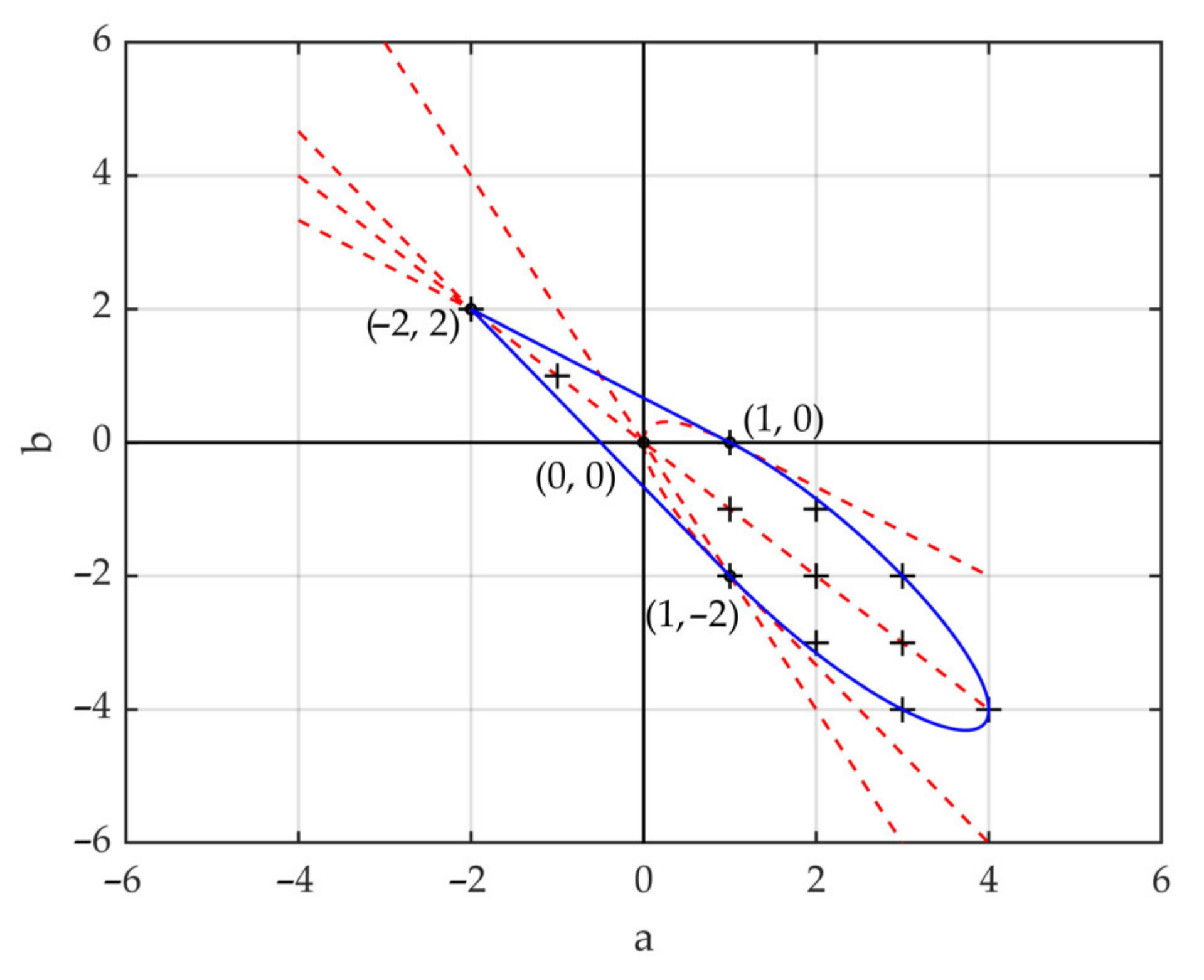
In particular, when
H = 1 m and
, the feasible region of parabola factors a and b is confined by the blue line in
Figure 13. In the figure, the blue line represents the feasible region boundary of a and b, the cross symbols represent the integer feasible solutions, and the four bold dots with coordinates correspond to the four crown shapes, respectively. The crown shapes and the corresponding values of factors a, b and c are as follows.
Cylinder: a = 0, b = 0, c = 1/3;
Cone: a = 1, b = −2, c = 1;
Inverted Cone: a = 1, b = 0, c = 0;
Ellipsoid: a = −2, b = 2, c = 0.
Figure 14 shows the squares of the parabola equations with different integer feasible solutions, which correspond to the cross symbols in
Figure 13.
Furthermore, in the feasible region, we chose parabola factors a and b with 0.1 as the interval and calculated factor c with Equation (28). Then, the backscattering coefficients of the corresponding vegetation canopies were simulated at VV polarization in C band, with the parameters of canopy A as the Modified Tor Vergata Model input, and the results are presented in
Figure 15. In
Figure 15, the bold dots represent the four crown shapes and correspond to the points in
Figure 13, and different colors correspond to different backscattering coefficients. From
Figure 15, we can conclude that the variation in the backscattering coefficients maintains good continuity over different crown shapes.
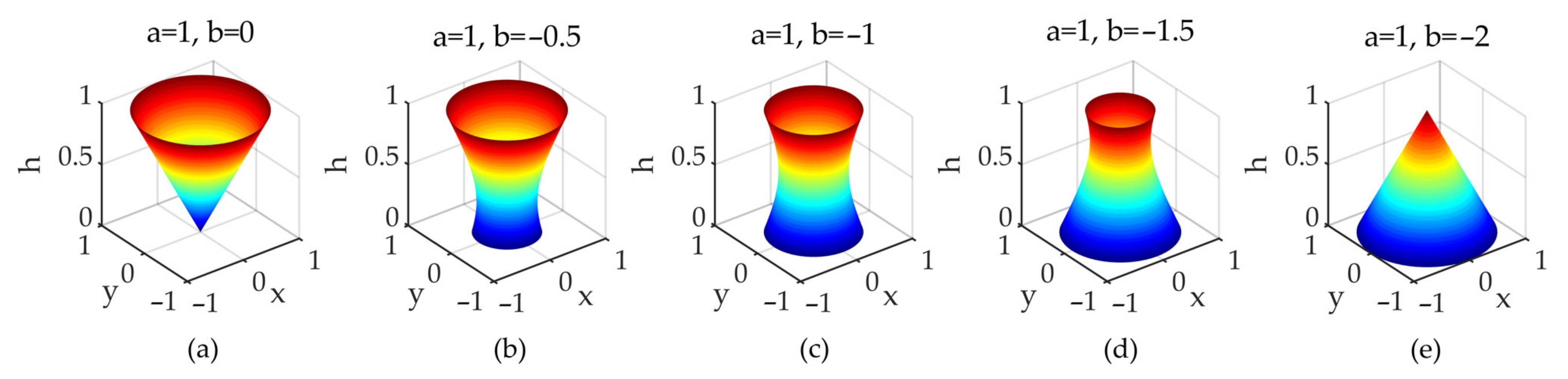
Specifically, three transition points were chosen between the cone and inverted cone to form a point set, written as {(a, b): (1, 0), (1, −0.5), (1, −1), (1, −1.5), (1, −2)}.
Figure 16 shows successive transformations of the crown shapes corresponding to the point set from (a) to (e); the corresponding backscattering coefficients (as shown in
Figure 15) of the vegetation canopies decrease gradually, which is a good indication that the continuous transformation of the crown shapes gives rise to the variation in backscattering coefficients of the corresponding vegetation canopies.
3.2. FEKO Simulation Results
Considering the long computation time and high memory requirements of FEKO, we only simulated the variation in the canopy backscattering coefficients at different canopy heights for the VV and VH polarizations in the C band (5.3 GHz). The simulation results are shown in
Figure 17,
Figure 18 and
Figure 19.
In
Figure 17, the red lines represent the averaged simulation results of eight azimuthal angles in FEKO when using canopy A’s parameters as inputs, and the blue lines denote the quadratic polynomial fitting results of the simulation results, which are in good agreement with the averaged simulation results; (a), (b), (c) and (d) correspond to the simulation results of the cylinder, cone, inverted cone and ellipsoid canopies at VV polarization of the C band. The processes of fitting at VH polarization for canopy A and at VV and VH polarizations for canopy B are the same as in
Figure 17, so they are not repeated here. For different canopy heights,
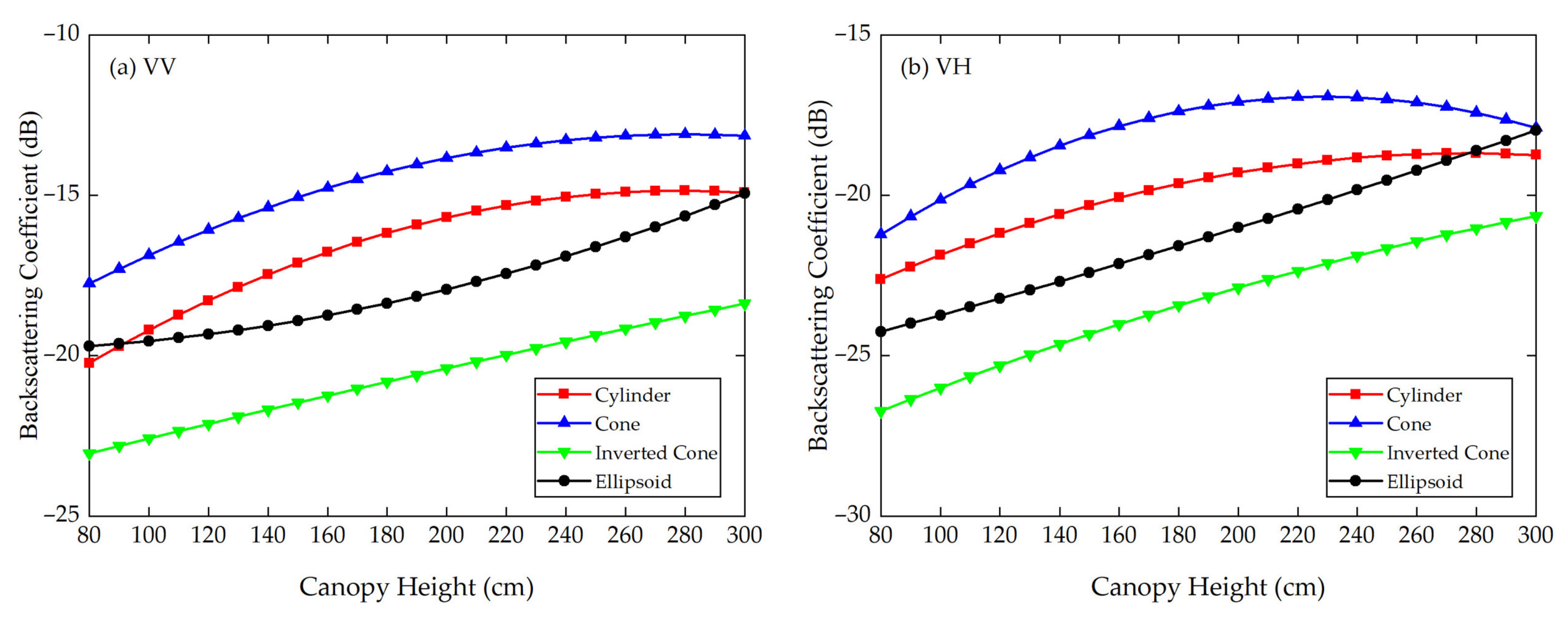
Figure 18 and
Figure 19 show the fitted FEKO results for canopies A and B with different crown shapes at VV and VH polarizations of the C band. On the whole, the fitted backscattering coefficients increase gradually as the canopy height increases from 80 cm to 300 cm.
In the same way, the mean absolute difference
and the mean relative difference
were used to evaluate the differences between the simulated backscattering coefficients for the three crown shapes studied and the reference cylinder crown shape. The results are presented in
Table 6, in which the upper row is
and the lower row is
for a certain polarization.
For canopies A and B in
Table 6, the largest MAD and MRD both occur between the inverted cone and cylinder canopies. Specifically, for canopy A, the largest MAD is 5.76 dB at VH polarization, and the largest MRD is as high as 31.65% at VV polarization. The smallest MAD is 1.84 dB between the ellipsoid and cylinder canopies at VV polarization, and the smallest MRD is 10.48% between the cone and cylinder canopies at VH polarization. Accordingly, for canopy B, the maximum MAD and MRD are 4.17 dB and 26.56%, both occurring between the inverted cone and cylinder canopies, and the minimum MAD and MRD are 2.08 dB and 10.77%, both occurring between the ellipsoid and cylinder canopies.
Taking canopy A as an example, for VV polarization at the C band, we can see from
Table 6 that different crown shapes produce a significant MAD (4.31 dB) in the backscattering coefficients, with the MRD reaching as high as 31.65% (VV polarization). At VH polarization, the MAD and MRD reach 5.76 dB and 27.22%. Furthermore, the absolute difference between the values for the cone and inverted cone at VH polarization is as high as 12 dB when the crown height is 220 cm. Hence, it can also be concluded that the crown shape has a non-negligible influence on microwave backscattering coefficients of the vegetation canopy at the given frequency and polarizations.
Overall, for FEKO simulation results at VV and VH polarizations of the C band, the ranking of the backscattering coefficients of the four vegetation canopies is
, which is approximately the same as the ranking of the volume fraction of the lower half of the vegetation canopy, for which the fractions are cone (75%) > cylinder (50%) = ellipsoid (50%) > inverted cone (25%). To be specific, there are some differences between the results of MTVM and FEKO. As shown in
Figure 18a for canopy A, the backscattering coefficients of the cylinder canopy are a little higher than those of the cone canopy when the canopy height is between 140 cm and 190 cm, and they are smaller than those of the ellipsoid canopy after 270 cm. In
Figure 18b, the backscattering coefficients of the cylinder canopy are slightly higher than those of the ellipsoid canopy at canopy heights between 100 cm and 180 cm.
Furthermore, it is clear that the backscattering coefficients and their relative differences for canopy A are greater than those for canopy B at VV polarization of the C band, but they are lower than those for canopy B at VH polarization of the C band. This is probably because of the difference in the geometrical and physical parameters of the vegetation components.
3.3. Comparative Analysis
In this section, the mean absolute difference
and the mean relative difference
are used to evaluate differences in the MTVM and FEKO simulation results between canopies A and B. The MADs and MRDs between canopies A and B for the same crown shapes are shown in
Table 7 and
Table 8, which show the MTVM and FEKO simulation results, respectively. In
Table 7 and
Table 8,
denotes the mean absolute difference between canopies A and B for the same crown shape, and
and
denote the mean relative differences for canopies A and B. The units remain the same as previously described.
For the MTVM simulation results at VV polarization of the C band, the maximum MAD between canopies A and B is 24.12 dB for the inverted cone canopy, and the minimum MAD is 22.18 dB for the cylinder canopy. The maximum MRD for canopy A is as high as 143.28% for the cone canopy. The MRDs of canopy A are greater than those of canopy B, but the magnitudes remain the same. The results at VH polarization follow similar rules.
For the FEKO simulation results at VV polarization of the C band, the maximum MAD between canopies A and B is 3.09 dB for the ellipsoid canopy, and the minimum MAD is 2.47 dB for the inverted cone canopy, which does not considerably differ from the others. The MRDs for canopy A are larger than those for canopy B, and the maximum MRD is 22.83% for the cone canopy at VV polarization. The FEKO results at VH polarization are bound by similar rules, but the MRDs for canopy B are larger than those for canopy A at VH polarization.
We can also notice that the MADs and MRDs greatly differ between the MTVM and FEKO simulation results. By contrasting
Figure 8 and
Figure 18 and
Figure 11 and
Figure 19, the results show that the FEKO simulation results are generally higher than the MTVM simulation results at VV and VH polarizations of the C band (5.3 GHz), which may be due to different unreasonable assumptions or the parameter settings of the two simulations. The actual reasons need to be further explored by analytical simulation and experimental measurement, which will be the focus of our future work.