The Torsional Response of Civil Engineering Structures during Earthquake from an Observational Point of View
Abstract
1. Introduction
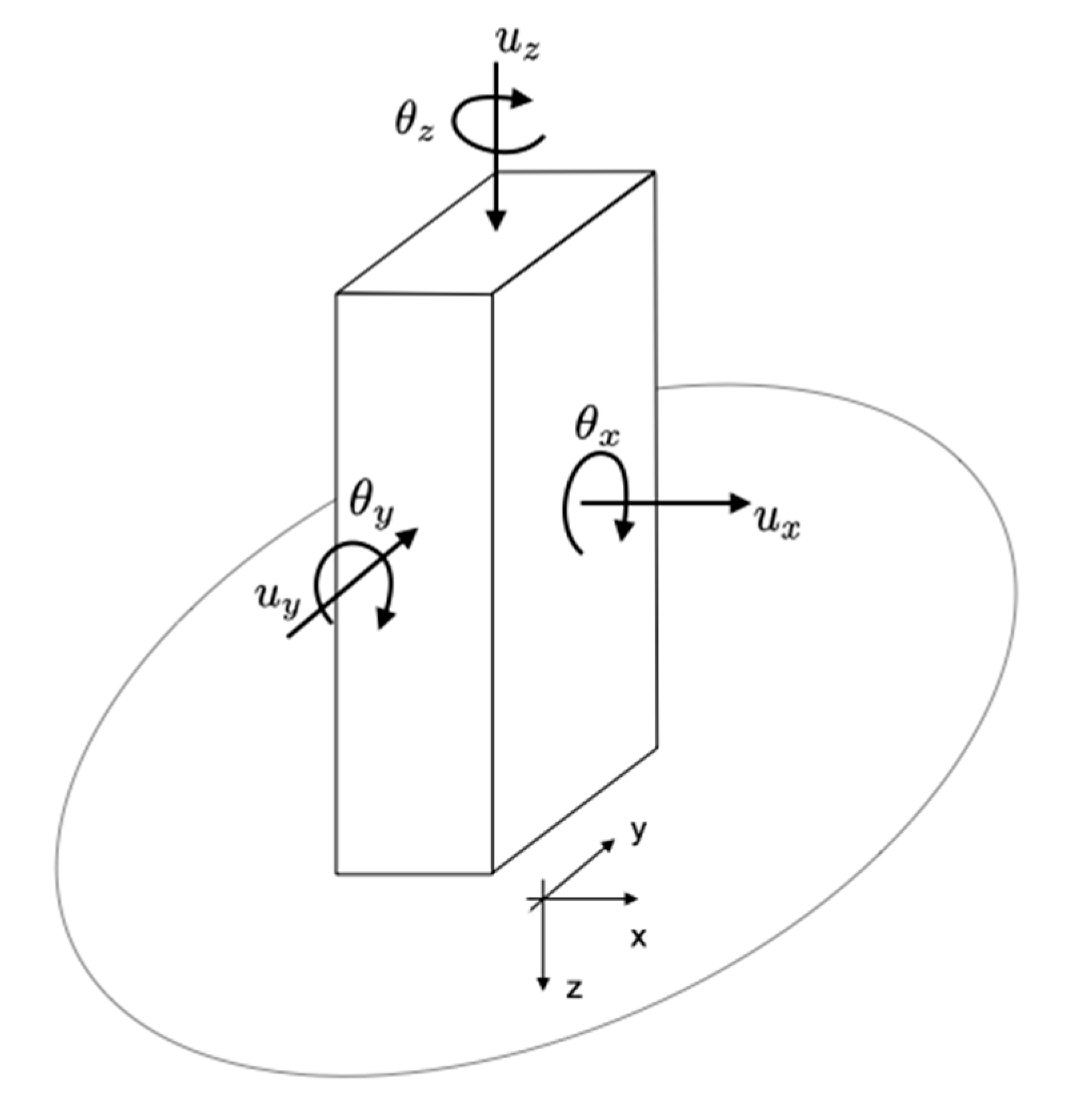
- rotations around the vertical axis (or torsion) due to the symmetric or asymmetric design of the building;
- rotations around the horizontal axis (or rocking) due to the inertial translational response of structures under earthquake loading, coupled with soil–structure interaction.
2. The Primary Origin of Torsional Motion in Buildings Linked to Static Eccentricity
3. Accidental Eccentricity
3.1. Discrepancies between the Calculated and the Actual Values of the Stiffness and Mass of Structural Elements
3.2. The Synchronised Orthogonal Components of Horizontal Ground Motion
3.3. Rotation of Ground Motion at the Base
4. Experimental and Numerical Observation of Torsion
4.1. Experimental Observation
4.2. Numerical Observation
4.3. Array-Derived Rotation
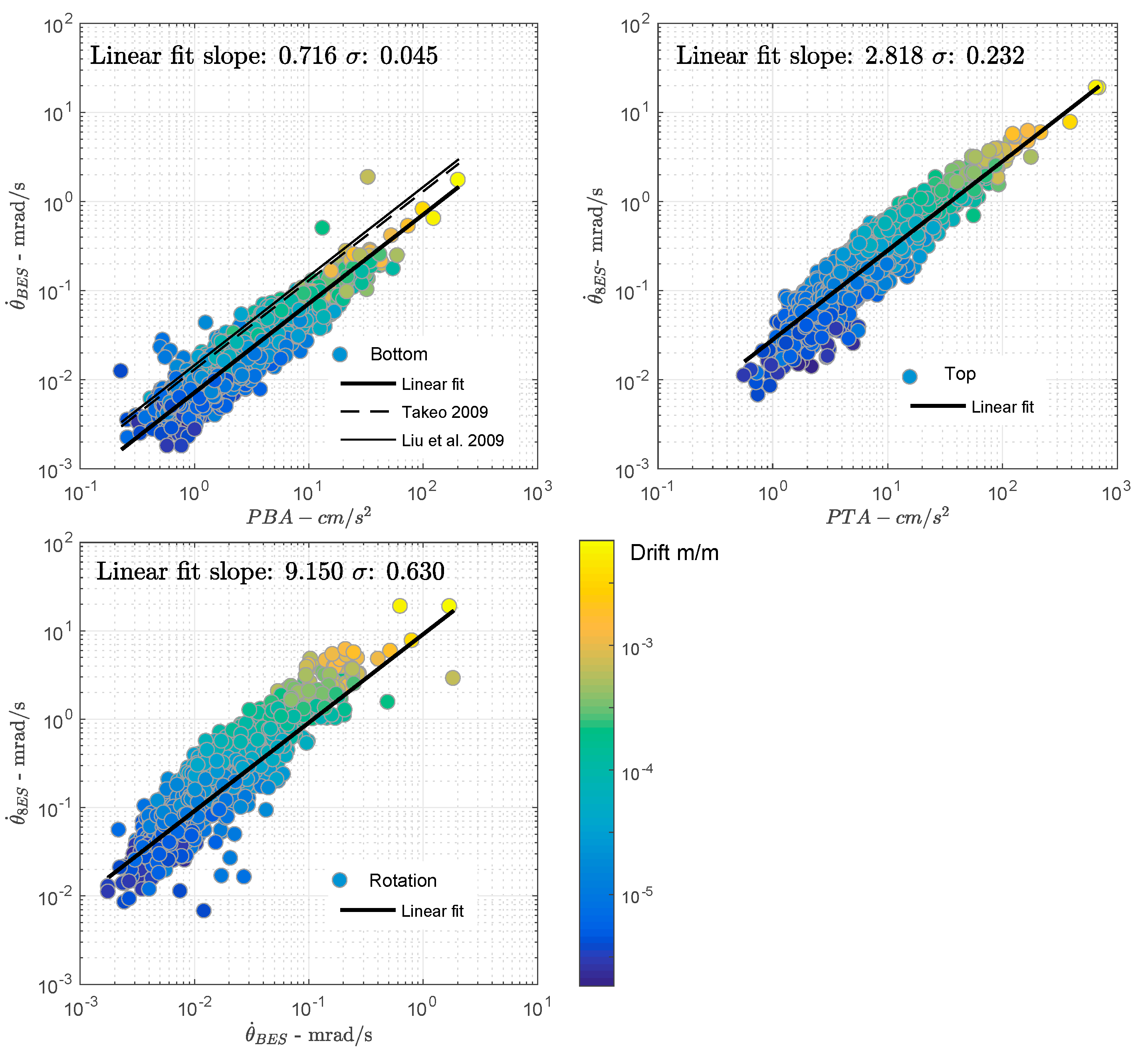
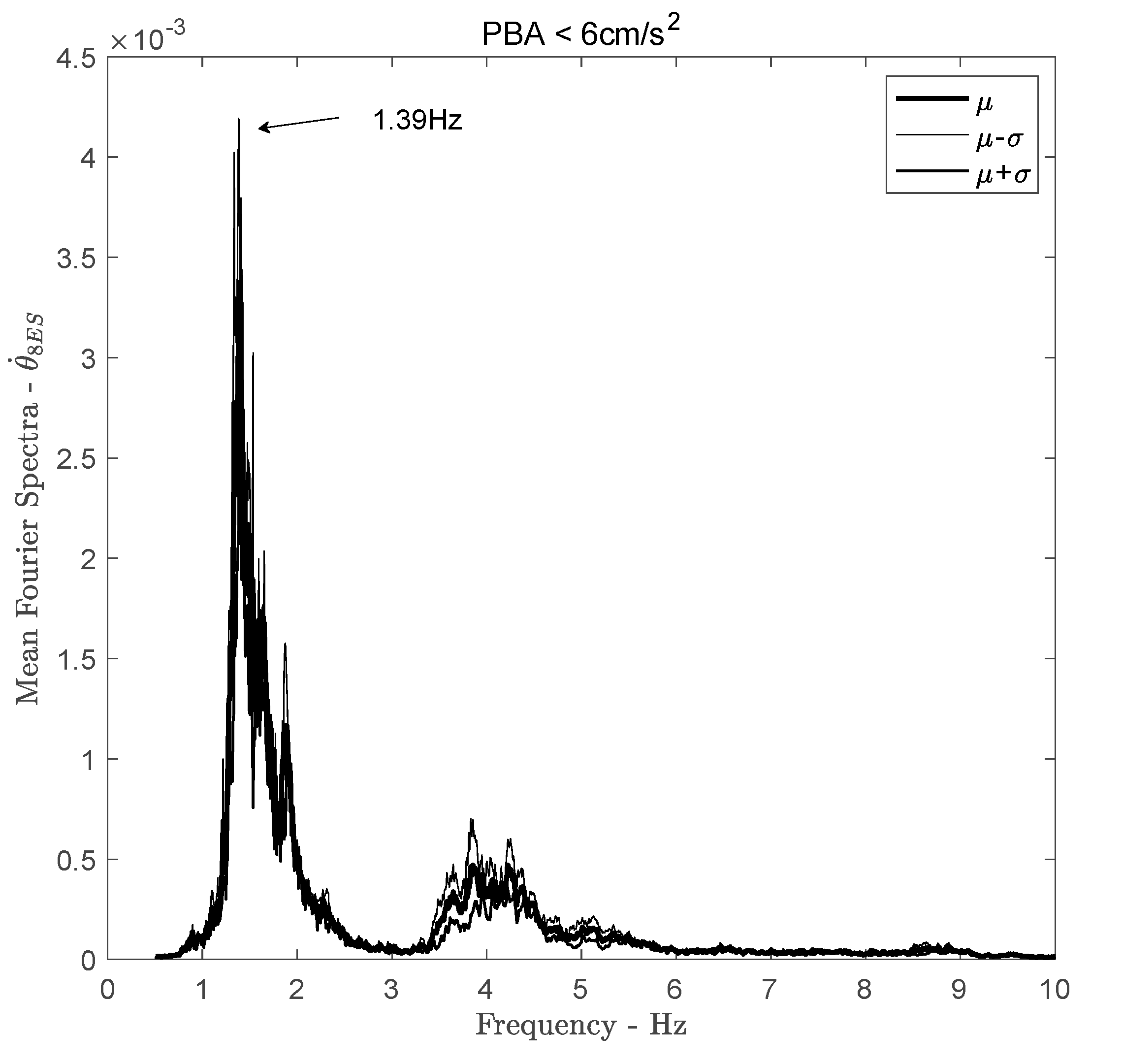
5. Rotational Structural Response for a Wide Range of Earthquakes
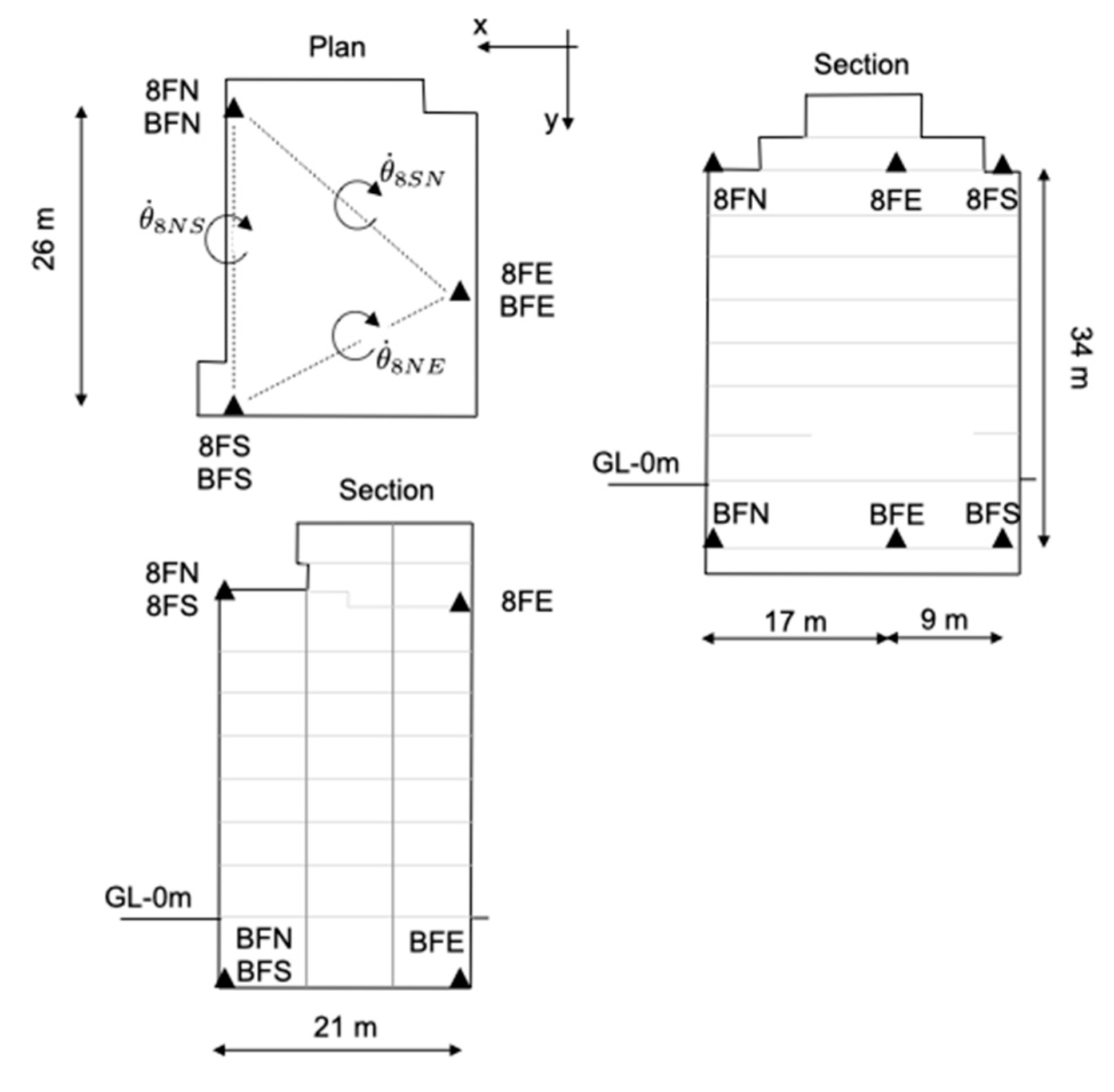
5.1. Data
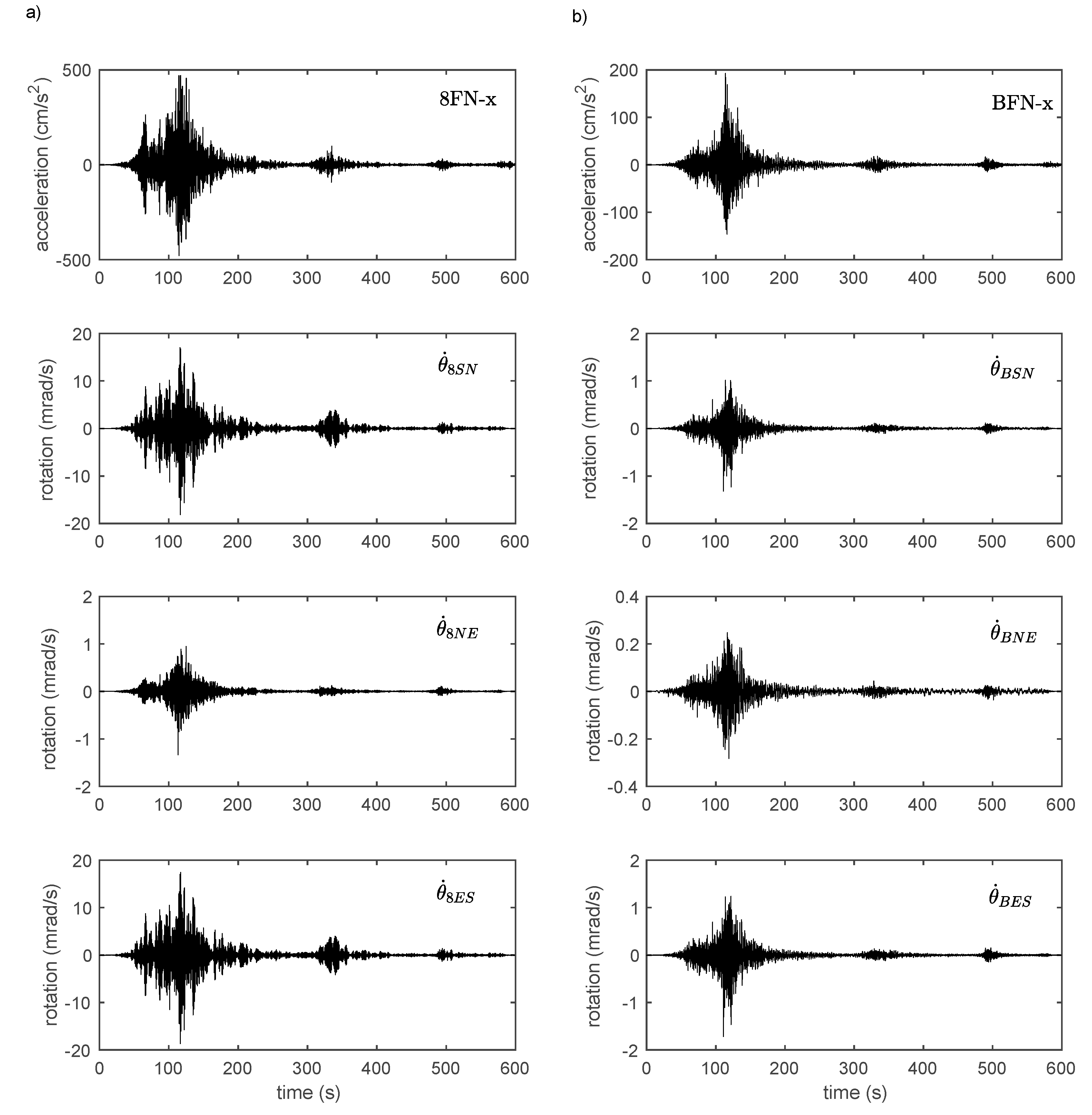
5.2. Rotation Rate
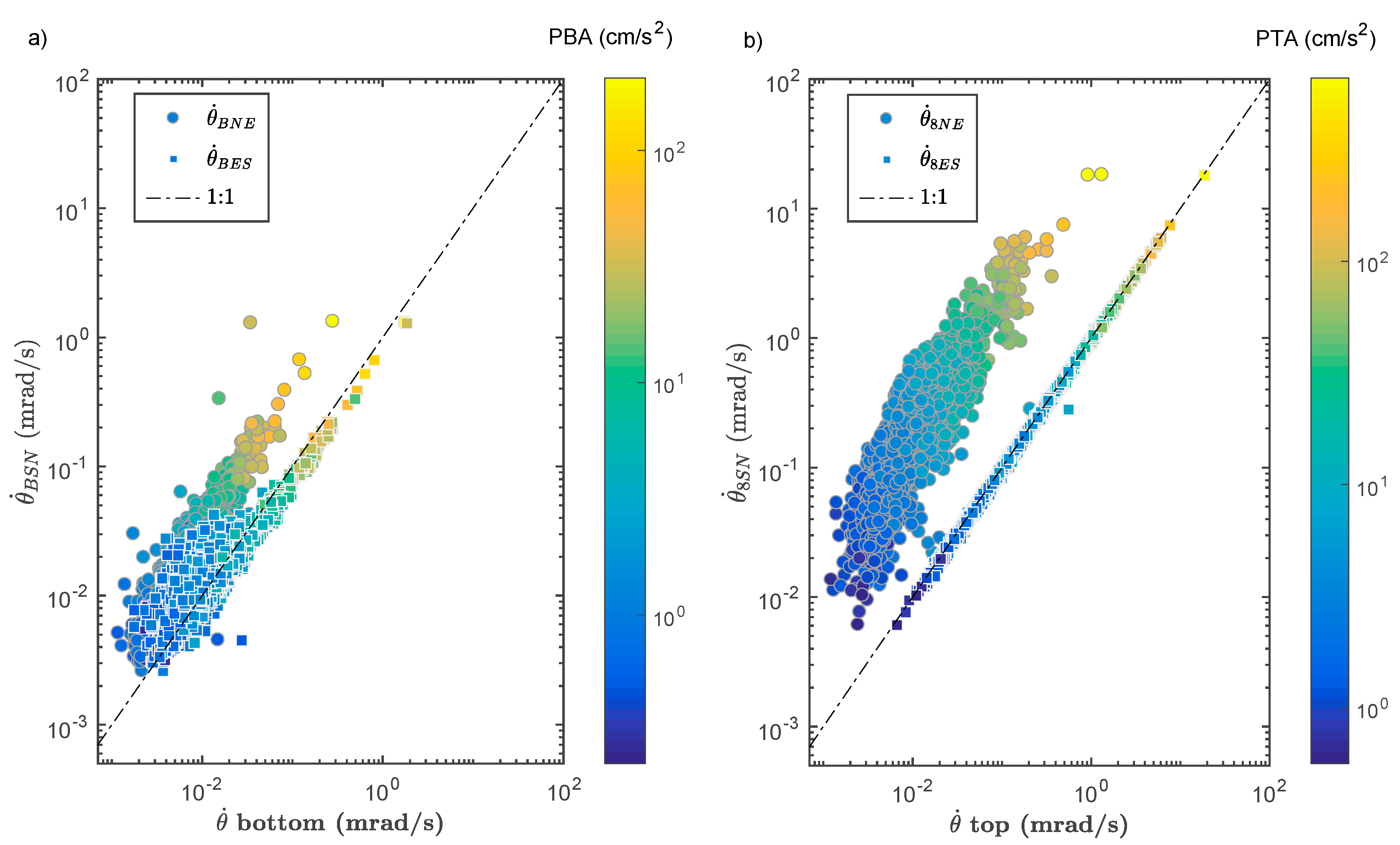
5.3. Comparison of Rotations and Translations
6. Conclusions and Perspectives
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Verderame, G.M.; Ricci, P.; Manfredi, G.; Cosenza, E. Ultimate chord rotation of RC columns with smooth bars: Some consid-erations about EC8 prescriptions. Bull. Earthq. Eng. 2010, 8, 1351–1373. [Google Scholar] [CrossRef]
- Beneke, D.; Kwok, K. Aerodynamic effect of wind induced torsion on tall buildings. J. Wind. Eng. Ind. Aerodyn. 1993, 50, 271–280. [Google Scholar] [CrossRef]
- Xu, Y.L.; Kwok, K.C.S.; Samali, B. Torsion response and vibration suppression of wind-excited buildings. J. Wind Eng. Ind. Aerod. 1992, 43, 1997–2008. [Google Scholar] [CrossRef]
- Rutenberg, A. Nonlinear response asymmetric building structures and seismic codes: A state of the art review. In Nonlinear Seismic Analysis and Design of Reinforced ConcreteB; Fajfar, P., Krawinkler, H., Eds.; Elsevier: Amsterdam, The Netherlands, 1992; pp. 328–356. [Google Scholar]
- Anagnostopoulos, S.A.; Kyrkos, M.; Stathopoulos, K. Earthquake induced torsion in buildings: Critical review and state of the art. Earthq. Struct. 2015, 8, 305–377. [Google Scholar] [CrossRef]
- Kausel, E. Early history of soil–structure interaction. Soil Dyn. Earthq. Eng. 2010, 30, 822–832. [Google Scholar] [CrossRef]
- EC8.6. EN 1998-6 Eurocode 8: Design of Structures for Earthquake Resistance; Part 6: Towers, Masts, and Chimneys 2005, European Committee for Standardization. Available online: http://eurocodes.jrc.ec.europa.eu/ (accessed on 22 August 2020).
- NZS 1170.5:2004. Structural Design Actions. In Parts 5 Earthquake Actions–New Zealand; NZS1170.5:2004, Standards New Zealand; 2002. Available online: https://shop.standards.govt.nz/ (accessed on 22 August 2020).
- Zembaty, Z. Rotational Seismic Load Definition in Eurocode 8, Part 6, for Slender Tower-Shaped Structures. Bull. Seism. Soc. Am. 2009, 99, 1483–1485. [Google Scholar] [CrossRef]
- Falamarz-Sheikhabadi, M.R.; Ghafory-Ashtiany, M. Approximate formulas for rotational effects in earthquake engineering. J. Seism. 2012, 16, 815–827. [Google Scholar] [CrossRef]
- Mazor, E.; Korjenkov, A.M. Applied archaeoseismology: Decoding earthquake parameters recorded in archaeological Ruins. In The Makhteshim Country: A Laboratory of Nature-geological and Ecological Studies in the Desert Region of Israel; Krasnov, B., Mazor, E., Eds.; Pensoft Publishers: Sofia-Moscow, Bulgaria, 2001; Part 1; pp. 123–153. [Google Scholar]
- Trifunac, M.; Todorovska, M.; Manić, M.I.; Bulajić, B. Variability of the fixed-base and soil-structure system frequencies of a building-The case of Borik-2 building. Struct. Control. Healt Monit. 2010, 17, 120–151. [Google Scholar] [CrossRef]
- Astorga, A.; Guéguen, P.; Kashima, T. Nonlinear Elasticity Observed in Buildings during a Long Sequence of Earthquakes. Bull. Seism. Soc. Am. 2018, 108, 1185–1198. [Google Scholar] [CrossRef]
- Astorga, A.; Guéguen, P.; Riviere, J.; Kashima, T.; Johnson, P.A. Recovery of the resonance frequency of buildings following strong seismic deformation as a proxy for structural health. Struct. Health Monit. 2019, 18, 1966–1981. [Google Scholar] [CrossRef]
- Astorga, A.; Guéguen, P. Influence of seismic strain rates on the co- and post-seismic response of civil engineering buildings. Earthq. Eng. Struct. Dyn. 2020, 49, 1758–1764. [Google Scholar] [CrossRef]
- Lee, V.W.; Trifunac, M. Empirical Scaling of Rotational Spectra of Strong Earthquake Ground Motion. Bull. Seism. Soc. Am. 2009, 99, 1378–1390. [Google Scholar] [CrossRef]
- Igel, H.; Bernauer, M.; Wassermann, J.; Schreiber, K.U. Seismology, Rotational, Complexity. Encycl. Complex. Syst. Sci. 2015, 1–26. [Google Scholar] [CrossRef]
- Castellani, A.; Boffi, G. Rotational components of the surface ground motion during an earthquake. Earthq. Eng. Struct. Dyn. 1986, 14, 751–767. [Google Scholar] [CrossRef]
- Spudich, P.; Steck, L.K.; Hellweg, M.; Fletcher, J.B.; Baker, L.M. Transient stresses at Parkfield, California, produced by theM7.4 Landers earthquake of 28 June 1992: Observations from the UPSAR dense seismograph array. J. Geophys. Res. Space Phys. 1995, 100, 675–690. [Google Scholar] [CrossRef]
- Ghayamghamian, M.R.; Nouri, G.R. On the characteristics of ground motion rotational components using Chiba dense array data. Earthq. Eng. Struct. Dyn. 2007, 36, 1407–1429. [Google Scholar] [CrossRef]
- Yin, J.; Nigbor, R.L.; Chen, Q.; Steidl, J. Engineering analysis of measured rotational ground motions at GVDA. Soil Dyn. Earthq. Eng. 2016, 87, 125–137. [Google Scholar] [CrossRef]
- Nigbor, R.L. Six-degree-of-freedom ground-motion measurement. B Seismol. Soc. Am. 1994, 84, 1665–1669. [Google Scholar]
- Igel, H.; Cochard, A.; Wassermann, J.; Flaws, A.; Schreiber, U.; Velikoseltsev, A.; Dinh, N.P. Broad-band observations of earthquake-induced rotational ground motions. Geophys. J. Int. 2007, 168, 182–196. [Google Scholar] [CrossRef]
- Lee, W.H.; Huang, B.S.; Langston, C.A.; Lin, C.J.; Liu, C.C.; Shin, T.C.; Wu, C.F. Progress in rotational ground-motion ob-servations from explosions and local earthquakes in Taiwan. B Seismol. Soc. Am. 2009, 99, 958–967. [Google Scholar] [CrossRef]
- Liu, C.C.; Huang, B.S.; Lee, W.H.K.; Lin, C.J. Observing Rotational and Translational Ground Motions at the HGSD Station in Taiwan from 2007 to 2008. Bull. Seism. Soc. Am. 2009, 99, 1228–1236. [Google Scholar] [CrossRef]
- Schreiber, K.U.; Hautmann, J.N.; Velikoseltsev, A.; Wassermann, J.; Igel, H.; Otero, J.; Wells, J.P. Ring laser measurements of ground rotations for seismology. B Seismol. Soc. Am. 2009, 99, 1190–1198. [Google Scholar] [CrossRef]
- Bernauer, F.; Wassermann, J.; Igel, H. Rotational sensors—a comparison of different sensor types. J. Seism. 2012, 16, 595–602. [Google Scholar] [CrossRef]
- Bouchon, M.; Aki, K. Strain, tilt, and rotation associated with strong ground motion in the vicinity of earthquake faults. B Seismol. Soc. Am. 1982, 72, 1717–1738. [Google Scholar]
- Lee, W.K.; Celebi, M.; Todorovska, M.I.; Igel, H. Introduction to the special issue on rotational seismology and engineering ap-plications. B Seismol. Soc. Am. 2009, 99, 945–957. [Google Scholar] [CrossRef]
- Teisseyre, R.; Nagahama, H.; Majewski, E. Physics of Asymmetric Continuum: Extreme and Fracture Processes; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Teisseyre, R.; Minoru, T.; Majewski, E. Earthquake Source Asymmetry, Structural Media and Rotation Effects; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Evans, J.R.; Cochard, A.; Graizer, V.; Huang, B.S.; Hudnut, K.; Hutt, C.R.; Nigbor, R. Rotational seismology workshop of February 2006. USA Geol. Surv. Open-File Rept 2007, 1145, 20. [Google Scholar]
- IWGoRS. International Working Group on Rotational Seismology (IWGoRS). Available online: http://www.rotational-seismology.org/ (accessed on 20 November 2020).
- Lee, W.H.K.; Igel, H.; Trifunac, M. Recent Advances in Rotational Seismology. Seism. Res. Lett. 2009, 80, 479–490. [Google Scholar] [CrossRef]
- Igel, H.; Brokesova, J.; Evans, J.; Zembaty, Z. Preface to the special issue on advances in rotational seismology: Instrumentation, theory, observations and engineering. J. Seismol. 2012, 16, 571–572. [Google Scholar] [CrossRef]
- Limongelli, M.P.; Dolce, M.; Spina, D.; Guéguen, P.; Langlais, M.; Wolinieck, D.; Maufroy, E.; Karakostas, C.Z.; Vassilios, A.; Lekidis, K.M.; et al. S2HM in Some European Countries. In Seismic Structural Health Monitoring. Springer Tracts in Civil Engineering Limongelli; Çelebi, M.M., Ed.; Springer: Berlin/Heidelberg, Germany, 2019. [Google Scholar] [CrossRef]
- Sung, S.H.; Park, J.W.; Nagayama, T.; Jung, H.J. A multi-scale sensing and diagnosis system combining accelerometers and gyroscopes for bridge health monitoring. Smart Mater. Struct. 2013, 23, 015005. [Google Scholar] [CrossRef]
- Mallet, R. On the dynamics of earthquakes; being an attempt to reduce their observed phenomena to the known laws of wave motion in solids and fluids. Trans. R. Irish Acad. 1846, 21, 51–105. [Google Scholar]
- Chandler, A.M. Building damage in Mexico City earthquake. Nat. Cell Biol. 1986, 320, 497–501. [Google Scholar] [CrossRef]
- Ayre, R.S. Interconnection of translational and torsional vibrations in buildings. B Seismol. Soc. Am. 1938, 28, 89–130. [Google Scholar]
- Ayre, R.S. Experimental response of an asymmetric, one-story building model to an idealized, transient ground motion. B Seismol. Soc. Am. 1943, 33, 91–119. [Google Scholar]
- Humar, J.L. Design for seismic torsional forces. Can. J. Civ. Eng. 1984, 11, 150–163. [Google Scholar] [CrossRef]
- Kan, C.L.; Chopra, A.K. Elastic earthquake analysis of torsionally coupled multistorey buildings. Earthq. Eng. Struct. Dyn. 1977, 5, 395–412. [Google Scholar] [CrossRef]
- Paulay, T. Displacement-based design approach to earthquake-induced torsion in ductile buildings. Eng. Struct. 1997, 19, 699–707. [Google Scholar] [CrossRef]
- Paulay, T. Some design principles relevant to torsional phenomena in ductile buildings. J. Earthq. Eng. 2001, 5, 273–308. [Google Scholar] [CrossRef]
- Housner, G.W.; Outinen, H. The effect of torsional oscillations on earthquake stresses. B Seismol. Soc. Am. 1958, 48, 221–229. [Google Scholar]
- Bustamante, J.I.; Rosenblueth, E. Building code provisions on torsional oscillation. In Proceedings of the 2nd World Conference on Earthquake Engineering, Tokyo-Kyoto, Japan, 11–18 July 1960. [Google Scholar]
- Kan, C.L.; Chopra, A.K. Closure to “Torsional Coupling and Earthquake Response of Simple Elastic and Inelastic Systems ”by Christopher, L. Kan and Anil, K. Chopra (August, 1981). J. Struct. Eng. 1983, 109, 285. [Google Scholar] [CrossRef]
- Dempsey, K.M.; Irvine, H.M. Envelopes of maximum seismic response for a partially symmetric single storey building model. Earthq. Eng. Struct. Dyn. 1979, 7, 161–180. [Google Scholar] [CrossRef]
- Reinhorn, A.; Rutenberg, A.; Gluck, J. Dynamic torsional coupling in asymmetric building structures. Build. Environ. 1977, 12, 251–261. [Google Scholar] [CrossRef]
- Chandler, A.; Hutchinson, G. Torsional coupling effects in the earthquake response of asymmetric buildings. Eng. Struct. 1986, 8, 222–236. [Google Scholar] [CrossRef]
- Tsicnias, T.; Hutchinson, G. Evaluation of code requirements for the earthquake resistant design of torsionally coupled buildings. Proc. Inst. Civ. Eng. 1981, 71, 821–843. [Google Scholar] [CrossRef]
- Hoerner, J.B. Modal Coupling and Earthquake Response of Tall Buildings. Ph.D. Thesis, California Institute of Technology, Pasadena, CA, USA, 24 May 1971. [Google Scholar]
- Lin, J.L.; Tsai, K.C. Seismic analysis of two-way asymmetric building systems under bi-directional seismic ground motions. Earthq. Eng. Struct. Dyn. 2007, 37, 305–328. [Google Scholar] [CrossRef]
- Tso, W.K. Induced torsional oscillations in symmetrical structures. Earthq. Eng. Struct. Dyn. 1974, 3, 337–346. [Google Scholar] [CrossRef]
- Antonelli, R.G.; Meyer, K.J.; Oppenheim, I.J. Torsional instability in structures. Earthq. Eng. Struct. Dyn. 1981, 9, 221–237. [Google Scholar] [CrossRef]
- Ivanović, S.S.; Trifunac, M.D.; Novikova, E.I.; Gladkov, A.A.; Todorovska, M.I. Ambient vibration tests of a seven-story rein-forced concrete building in Van Nuys, California, damaged by the 1994 Northridge earthquake. Soil Dyn. Earthq. Eng. 2000, 19, 391–411. [Google Scholar] [CrossRef]
- Clinton, J.F.; Bradford, S.C.; Heaton, T.H.; Favela, J. The observed wander of the natural frequencies in a structure. B Seismol. Soc. Am. 2006, 96, 237–257. [Google Scholar] [CrossRef]
- Chandler, A.M.; Duan, X.N. Inelastic torsional behaviour of asymmetric buildings under severe earthquake shaking. Struct. Eng. Rev. 1990, 2, 141–159. [Google Scholar]
- De la Llera, J.C.; Chopra, A.K. Accidental torsion in buildings due to base rotational excitation. Earthq. Eng. Struct. Dyn. 1994, 23, 1003–1021. [Google Scholar] [CrossRef]
- De la Llera, J.C.; Chopra, A.K. Accidental torsion in buildings due to stiffness uncertainty. Earthq. Eng. Struct. Dyn. 1994, 23, 117–136. [Google Scholar] [CrossRef]
- Douglas, B.M.; Trabert, T.E. Coupled torsional dynamic analysis of a multistory building. B Seismol. Soc. Am. 1973, 63, 1025–1039. [Google Scholar]
- Chopra, A.K.; Goel, R.K. A modal pushover analysis procedure to estimate seismic demands for unsymmetric-plan buildings. Earthq. Eng. Struct. Dyn. 2004, 33, 903–927. [Google Scholar] [CrossRef]
- Ghobarah, A.A.; Tso, W.K. Seismic analysis of skewed highway bridges with intermediate supports. Earthq. Eng. Struct. Dyn. 1973, 2, 235–248. [Google Scholar] [CrossRef]
- Bycroft, G.N. Soil—foundation interaction and differential ground motions. Earthq. Eng. Struct. Dyn. 1980, 8, 397–404. [Google Scholar] [CrossRef]
- Todorovska, M.I.; Trifunac, M.D. A note on excitation of long structures by ground waves. J. Eng. Mech. Div. ASCE 1990, 116, 952–996. [Google Scholar] [CrossRef]
- Trifunac, M.D.; Todorovska, M.I. Response spectra and differential motion of columns. Earthq. Eng. Struct. Dyn. 1997, 26, 251–268. [Google Scholar] [CrossRef]
- Trifunac, M.; Gičev, V. Response spectra for differential motion of columns paper II: Out-of-plane response. Soil Dyn. Earthq. Eng. 2006, 26, 1149–1160. [Google Scholar] [CrossRef]
- Jalali, R.S.; Trifunac, M. Response Spectra for Near-Source, Differential, and Rotational Strong Ground Motion. Bull. Seism. Soc. Am. 2009, 99, 1404–1415. [Google Scholar] [CrossRef]
- Cornou, C.; Bard, P.Y.; Dietrich, M. Contribution of dense array analysis to the identification and quantification of basin-edge-induced waves, Part II: Application to Grenoble basin (French Alps). B Seismol. Soc. Am. 2003, 93, 2624–2648. [Google Scholar] [CrossRef]
- Smerzini, C.; Paolucci, R.; Stupazzini, M. Experimental and numerical results on earthquake-induced rotational ground motions. J. Earthq. Eng. 2009, 13, 66–82. [Google Scholar] [CrossRef]
- Stupazzini, M.; De La Puente, J.; Smerzini, C.; Kaser, M.; Igel, H.; Castellani, A. Study of Rotational Ground Motion in the Near-Field Region. Bull. Seism. Soc. Am. 2009, 99, 1271–1286. [Google Scholar] [CrossRef]
- Guéguen, P.; Bard, P.Y.; Chávez-García, F.J. Site-city seismic interaction in Mexico city–like environments: An analytical study. B Seismol. Soc. Am. 2002, 92, 794–811. [Google Scholar] [CrossRef]
- Guéguen, P.; Bard, P.Y. Soil-structure and soil-structure-soil interaction: Experimental evidence at the Volvi test site. J. Earthq. Eng. 2005, 9, 657–693. [Google Scholar] [CrossRef]
- Guéguen, P.; Colombi, A. Experimental and Numerical Evidence of the Clustering Effect of Structures on Their Response during an Earthquake: A Case Study of Three Identical Towers in the City of Grenoble, France. Bull. Seism. Soc. Am. 2016, 106, 2855–2864. [Google Scholar] [CrossRef]
- Lee, V.W.; Trifunac, M.D. Torsional accelerograms. Int. J. Soil Dyn. Earthq. Eng. 1985, 4, 132–139. [Google Scholar] [CrossRef]
- Saito, M. Synthesis of Rotational and Dilatational Seismograms. J. Phys. Earth 2011, 16, 53–61. [Google Scholar] [CrossRef]
- Bolt, B.A.; Tsai, Y.B.; Yeh, K.; Hsu, M.K. Earthquake strong motions recorded by a large near-source array of digital seismo-graphs. Earthq. Eng. Struct. Dyn. 1982, 10, 561–573. [Google Scholar] [CrossRef]
- Reissner, E.; Sagoci, H.F. Forced Torsional Oscillations of an Elastic Half-Space. I. J. Appl. Phys. 1944, 15, 652. [Google Scholar] [CrossRef]
- Bycroft, G.N. Forced vibrations of a rigid circular plate on a semi-infinite elastic space and on an elastic stratum. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Sci. 2006, 248, 327–368. [Google Scholar] [CrossRef]
- Apsel, R.J.; Luco, J.E. Torsional response of rigid embedded foundation. J. Eng. Mech. Div. 1976, 102, 957–970. [Google Scholar]
- Luco, J.E. Torsional response of structures to obliquely incident seismic sh waves. Earthq. Eng. Struct. Dyn. 1976, 4, 207–219. [Google Scholar] [CrossRef]
- Housner, G.W. Interaction of building and ground during an earthquake. B Seismol. Soc. Am. 1957, 47, 179–186. [Google Scholar]
- Newmark, N.M. Torsion in symmetrical buildings. In Proceedings of the 4th World Conference Earthquake Engineering, Santiago, Chile, 13–18 January 1969; pp. 19–32. [Google Scholar]
- Nathan, N.D.; MacKenzie, J.R. Rotational Components of Earthquake Motion. Can. J. Civ. Eng. 1975, 2, 430–436. [Google Scholar] [CrossRef]
- Tso, W.K.; Hsu, T.I. Torsional spectrum for earthquake motions. Earthq. Eng. Struct. Dyn. 1978, 6, 375–382. [Google Scholar] [CrossRef]
- Castellani, A.; Stupazzini, M.; Guidotti, R. Free-field rotations during earthquakes: Relevance on buildings. Earthq. Eng. Struct. Dyn. 2011, 41, 875–891. [Google Scholar] [CrossRef]
- Stanley, W.F. The Earthquake. Nat. Cell Biol. 2008, 30, 125. [Google Scholar] [CrossRef]
- Hodgson, E.A. The rotation rffects of the St. Lawrence earthquake of February 28 1925. J. R. Astrono. Soc. Can. 1925, 19, 169. [Google Scholar]
- Ulrich, F.P. Helena earthquakes. Bull. Seismol. Soc. Am. 1936, 26, 323–339. [Google Scholar]
- Rouse, J.T.; Priddy, R.R. Recent Earthquakes in Western Ohio. 1938. Available online: https://kb.osu.edu/bitstream/handle/1811/2921/V38N01_025.pdf (accessed on 22 August 2020).
- Thornton, D.L. Earthquakes and structures. J. R. Soc. Arts 1940, 88, 799–815. [Google Scholar]
- Dewell, H.D. Report of Committee on Building for Safety against Earthquakes: Preliminary report of Subcommittee on Framed Structures: Wood, steel, and ferro-concrete. Bull. Seismol. Soc. Am. 1925, 15, 175–195. [Google Scholar]
- Sargeant, S.L.; Musson, R.M.W. Rotational Earthquake Effects in the United Kingdom. Bull. Seism. Soc. Am. 2009, 99, 1475–1479. [Google Scholar] [CrossRef]
- Hinzen, K.G. Rotation of vertically oriented objects during earthquakes. J. Seism. 2011, 16, 797–814. [Google Scholar] [CrossRef]
- Hart, G.C.; Lew, M.; DiJulio, R.M. Torsional response of high-rise buildings. J. Struct. Division ASCE 1975, 101, 397–416. [Google Scholar]
- Şafak, E. Response of a 42-storey steel-frame building to the MS=7.1 Loma Prieta earthquake. Eng. Struct. 1993, 15, 403–421. [Google Scholar] [CrossRef]
- Li, Y.; Mau, S.T. Learning from Recorded Earthquake Motion of Buildings. J. Struct. Eng. 1997, 123, 62–69. [Google Scholar] [CrossRef]
- Todorovska, M.; Trifunac, M.D. Earthquake damage detection in the Imperial County Services Building III: Analysis of wave travel times via impulse response functions. Soil Dyn. Earthq. Eng. 2008, 28, 387–404. [Google Scholar] [CrossRef]
- Lemnitzer, A.; Massone, L.M.; Skolnik, D.A.; De la Llera Martin, J.C.; Wallace, J.W. Aftershock response of RC buildings in Santiago, Chile, succeeding the magnitude 8.8 Maule earthquake. Eng. Struct. 2014, 76, 324–338. [Google Scholar] [CrossRef]
- Çelebi, M.; Liu, H.P. Before and after retrofit–response of a building during ambient and strong motions. J. Wind. Eng. Ind. Aerodyn. 1998, 77, 259–268. [Google Scholar] [CrossRef]
- Çelebi, M. Recorded Earthquake Responses from the Integrated Seismic Monitoring Network of the Atwood Building, Anchorage, Alaska. Earthq. Spectra 2006, 22, 847–864. [Google Scholar] [CrossRef]
- Michel, C.; Guéguen, P.; El Arem, S.; Mazars, J.; Kotronis, P. Full-scale dynamic response of an RC building under weak seismic motions using earthquake recordings, ambient vibrations and modelling. Earthq. Eng. Struct. Dyn. 2009, 39, 419–441. [Google Scholar] [CrossRef]
- Michel, C. Vulnérabilité Sismique de L’échelle du Bâtiment à Celle de la Ville-Apport des Techniques Expérimentales in Situ-Application à Grenoble. Ph.D. Thesis, Université Joseph-Fourier-Grenoble I, Grenoble, France, 2007. (In French). Available online: https://tel.archives-ouvertes.fr/tel-00191024 (accessed on 22 August 2020).
- Ghayamghamian, M.R.; Nouri, G.; Igel, H.; Tobita, T. The Effect of Torsional Ground Motion on Structural Response: Code Recommendation for Accidental Eccentricity. Bull. Seism. Soc. Am. 2009, 99, 1261–1270. [Google Scholar] [CrossRef]
- Tso, W.K.; Dempsey, K.M. Seismic torsional provisions for dynamic eccentricity. Earthq. Eng. Struct. Dyn. 1980, 8, 275–289. [Google Scholar] [CrossRef]
- Shakib, H.; Tohidi, R.Z. Evaluation of accidental eccentricity in buildings due to rotational component of earthquake. J. Earthq. Eng. 2002, 6, 431–445. [Google Scholar] [CrossRef]
- Newmark, N.M.; Rosenblueth, E. Fundamentals in Earthquake Engineering; Prentice Hall: Englewood Cliffs, NJ, USA, 1971. [Google Scholar]
- Luco, J.E.; Sotiropoulos, D.A. Local characterization of free-field ground motion and effects of wave passage. B Seismol. Soc. Am. 1980, 70, 2229–2244. [Google Scholar]
- Dahmardeh, S.R.; Motamedi, M.; Aziminejad, A. A study on the effects of torsional component of ground motions on seismic response of low- and mid-rise buildings. Struct. Des. Tall Speéc. Build. 2019, 29, 1699. [Google Scholar] [CrossRef]
- Castellani, A.; Zembaty, Z. Comparison between earthquake rotation spectra obtained by different experimental sources. Eng. Struct. 1996, 18, 597–603. [Google Scholar] [CrossRef]
- Zembaty, Z. Tutorial on Surface Rotations from Wave Passage Effects: Stochastic Spectral Approach. Bull. Seism. Soc. Am. 2009, 99, 1040–1049. [Google Scholar] [CrossRef]
- Trifunac, M. A note on rotational components of earthquake motions on ground surface for incident body waves. Int. J. Soil Dyn. Earthq. Eng. 1982, 1, 11–19. [Google Scholar] [CrossRef]
- Basu, D.; Whittaker, A.S.; Constantinou, M.C. Estimating Rotational Components of Ground Motion Using Data Recorded at a Single Station. J. Eng. Mech. 2012, 138, 1141–1156. [Google Scholar] [CrossRef]
- Oliveira, C.; Bolt, B. Rotational components of the surface strong ground motion. Earthq. Eng. Struct. Dyn. 1989, 18, 517–526. [Google Scholar] [CrossRef]
- Huang, B.S. Ground rotational motions of the 1999 Chi-Chi, Taiwan earthquake as inferred from dense array observations. Geophys. Res. Lett. 2003, 30. [Google Scholar] [CrossRef]
- Castellani, A. Array-derived rotational seismic motions: Revisited. B Earthq. Eng. 2017, 15, 813–825. [Google Scholar] [CrossRef]
- Cochard, A.; Igel, H.; Schuberth, B.; Suryanto, W.; Velikoseltsev, A.; Schreiber, U.; Wassermann, J.; Scherbaum, F.; Vollmer, D. Rotational Motions in Seismology: Theory, Observation, Simulation. In Earthquake Source Asymmetry, Structural Media and Rotation Effects; Springer: Berlin/Heidelberg, Germany, 2006; pp. 391–411. [Google Scholar]
- Trifunac, M.D. Differential earthquake motion of building foundations. J. Struct. Eng. ASCE 1997, 4, 414–422. [Google Scholar] [CrossRef]
- Çelebi, M.; Okawa, I.; Kashima, T.; Koyama, S.; Iiba, M. Response of a tall building far from the epicenter of the 11 March 2011 M 9.0 Great East Japan earthquake and aftershocks. Struct. Des. Tall Speéc. Build. 2014, 23, 427–441. [Google Scholar] [CrossRef]
- Rahmani, M.; Todorovska, M. 1D System identification of a 54-story steel frame building by seismic interferometry. Earthq. Eng. Struct. Dyn. 2013, 43, 627–640. [Google Scholar] [CrossRef]
- Lin, C.J.; Huang, W.G.; Huang, H.P.; Huang, B.; Ku, C.S.; Liu, C.C. Investigation of array-derived rotation in TAIPEI 101. J. Seism. 2012, 16, 721–731. [Google Scholar] [CrossRef]
- Cao, Y.; Mavroeidis, G.P.; Ashoory, M. Comparison of observed and synthetic near-fault dynamic ground strains and rotations from the 2004 M w 6.0 Parkfield, California, earthquake. B Seismol. Soc. Am. 2018, 108, 1240–1256. [Google Scholar] [CrossRef]
- Spudich, P.; Fletcher, J.B. Observation and Prediction of Dynamic Ground Strains, Tilts, and Torsions Caused by the Mw 6.0 2004 Parkfield, California, Earthquake and Aftershocks, Derived from UPSAR Array Observations. Bull. Seism. Soc. Am. 2008, 98, 1898–1914. [Google Scholar] [CrossRef]
- Gičev, V.; Trifunac, M.D.; Orbović, N. Translation, torsion, and wave excitation of a building during soil–structure interaction excited by an earthquake SH pulse. Soil Dyn. Earthq. Eng. 2015, 77, 391–401. [Google Scholar] [CrossRef]
- Kohler, M.D.; Davis, P.M.; Safak, E. Earthquake and Ambient Vibration Monitoring of the Steel-Frame UCLA Factor Building. Earthq. Spectra 2005, 21, 715–736. [Google Scholar] [CrossRef]
- Basu, D.; Whittaker, A.S.; Constantinou, M.C. Extracting rotational components of earthquake ground motion using data recorded at multiple stations. Earthq. Eng. Struct. Dyn. 2012, 42, 451–468. [Google Scholar] [CrossRef]
- Basu, D.; Whittaker, A.S.; Constantinou, M.C. Characterizing rotational components of earthquake ground motion using a surface distribution method and response of sample structures. Eng. Struct. 2015, 99, 685–707. [Google Scholar] [CrossRef]
- Kashima, T. Dynamic behavior of an 8th-storey SRC building examined from strong motion records. In Proceedings of the 13th World Conference on Earthquake Engineering (13WCEE), Vancouver, BC, Canada, 1–6 August 2004. Paper Number 196. [Google Scholar]
- Kashima, T. Dynamic behavior of SRC buildings damaged by the 2011 great east Japan earthquake based on strong motion records. In Proceedings of the Second European Conference on Earthquake Engineering and Seismology, Istanbul, Turkey, 25–29 August 2014. [Google Scholar]
- Astorga, A.; Guéguen, P.; Ghimire, S.; Kashima, T. NDE1.0: A new database of earthquake data recordings from buildings for engineering applications. Bull. Earthq. Eng. 2020, 18, 1321–1344. [Google Scholar] [CrossRef]
- Takeo, M. Rotational Motions Observed during an Earthquake Swarm in April 1998 Offshore Ito, Japan. Bull. Seism. Soc. Am. 2009, 99, 1457–1467. [Google Scholar] [CrossRef]
- Pandey, B.H.; Finn, W.L.; Ventura, C.E. Modification of free-field motions by soil-foundation-structure interaction for shallow foundations. In Proceedings of the 15th World Conference on Earthquake Engineering, Lisbon, Portugal, 24–28 September 2012. [Google Scholar]
- Bońkowski, P.A.; Bobra, P.; Zembaty, Z.; Jędraszak, B. Application of Rotation Rate Sensors in Modal and Vibration Analyses of Reinforced Concrete Beams. Sensors 2020, 20, 4711. [Google Scholar] [CrossRef]


| Description | Building Features | Qualitative Effect | Refs. | |
|---|---|---|---|---|
| Static es −CM/CR Shift | asym | Max for Ty | [40,41] | |
| Dynamic ed − ed =α es with α = 1.5 in EC8 | asym Ω = 1 | Amplification of es | [46,53] | |
| Accidental ea − ea =βL with β = 0.05 to 0.10 in EC8 | CM/CR uncertainties | asym/sym | smaller than β value | [60,61] |
| 2D trans. ground motion | asym/sym | [61,62] | ||
| Ground motion rotation | ||||
| Differential trans. motion | asym/sym | strong when site effects | [64,65] | |
| Rotational component | ||||
| Bldg features consideration | ||||
| MR-to-HR bldg | Ty > 0.5s | small | [60] | |
| HR building | Ty > 1.5 s | strong (up to 20%) | [87] | |
| sym/asym torsionally stiff bldg | Ω>1 | small | [107] | |
| tall and torsionally stiff bldg | Ω > 1 all Ty | increase | [11] | |
| tall asym. bldg | Ty < 1 | decrease | [11] | |
| LR-to-MR, short period and torsionally flexible bldg | Ty < 1 s – Ω < 1 | more prevalent for sym. | [107] | |
| LR-to-MR, short period and torsionally flexible bldg | Ty < 0.5 to 1s – Ω < 2/3 to 1 | strong | [60,105] | |
| β consideration | ||||
| LR-to-MR sym. bldg | Ty>0.2 | β = 0.05 significant | [60,108,109] | |
| LR-to-MR, short period and torsionally flexible bldg | Ty < 0.3 – Ω < 2/3 | β > 0.05 for sym. bldg | [105,110] | |
| torsionally stiff bldg | Ω > 1 all Ty | β < 0.05 | [107] | |
| torsionally flexible bldg | Ω < 1 all Ty | β > 0.05 | [107] | |
| asym. bldg | β = 0.05 conservative | [11] | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guéguen, P.; Astorga, A. The Torsional Response of Civil Engineering Structures during Earthquake from an Observational Point of View. Sensors 2021, 21, 342. https://doi.org/10.3390/s21020342
Guéguen P, Astorga A. The Torsional Response of Civil Engineering Structures during Earthquake from an Observational Point of View. Sensors. 2021; 21(2):342. https://doi.org/10.3390/s21020342
Chicago/Turabian StyleGuéguen, Philippe, and Ariana Astorga. 2021. "The Torsional Response of Civil Engineering Structures during Earthquake from an Observational Point of View" Sensors 21, no. 2: 342. https://doi.org/10.3390/s21020342
APA StyleGuéguen, P., & Astorga, A. (2021). The Torsional Response of Civil Engineering Structures during Earthquake from an Observational Point of View. Sensors, 21(2), 342. https://doi.org/10.3390/s21020342






