Optomechanical Design and Application of Solar-Skylight Spectroradiometer
Abstract
1. Introduction
2. Instrument Characterization
2.1. Overview Design
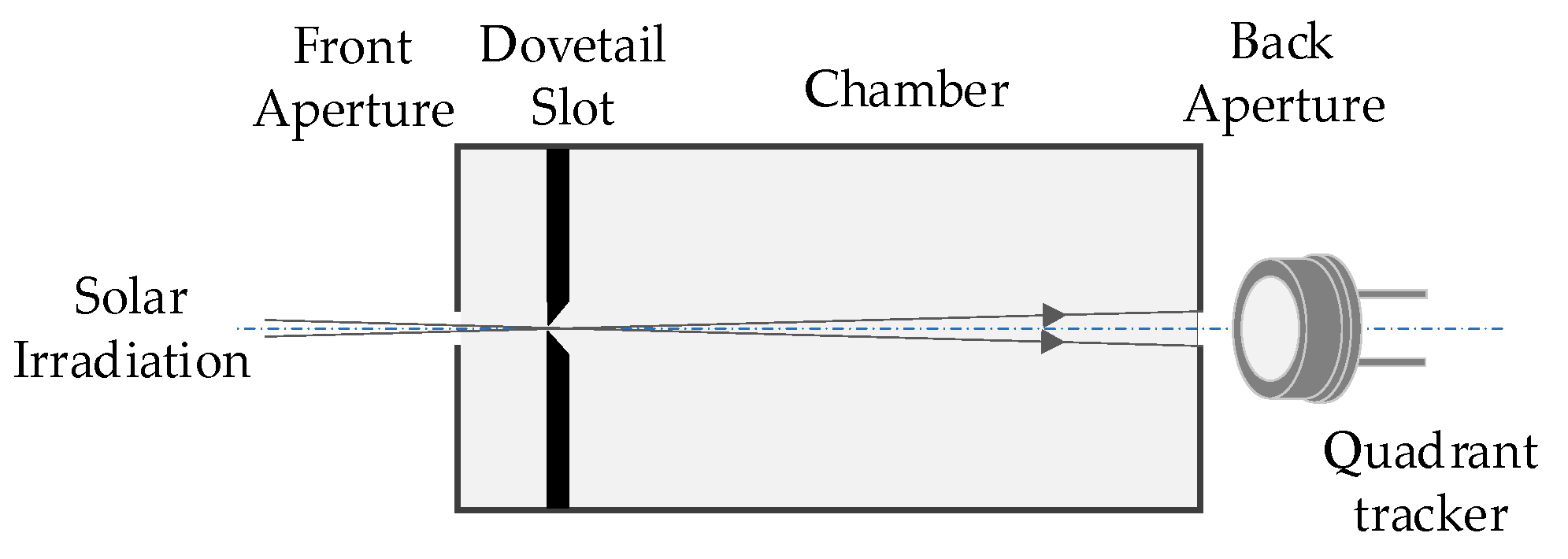
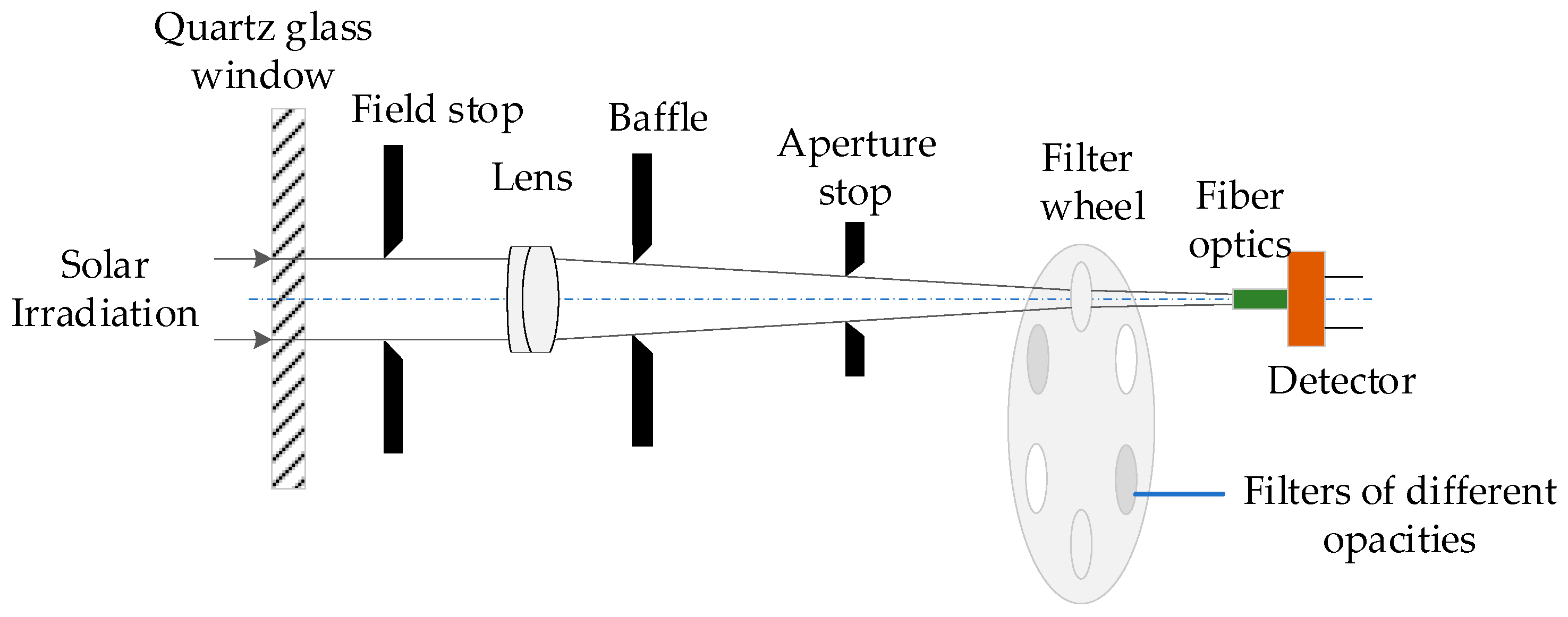
2.2. Light-Path Design
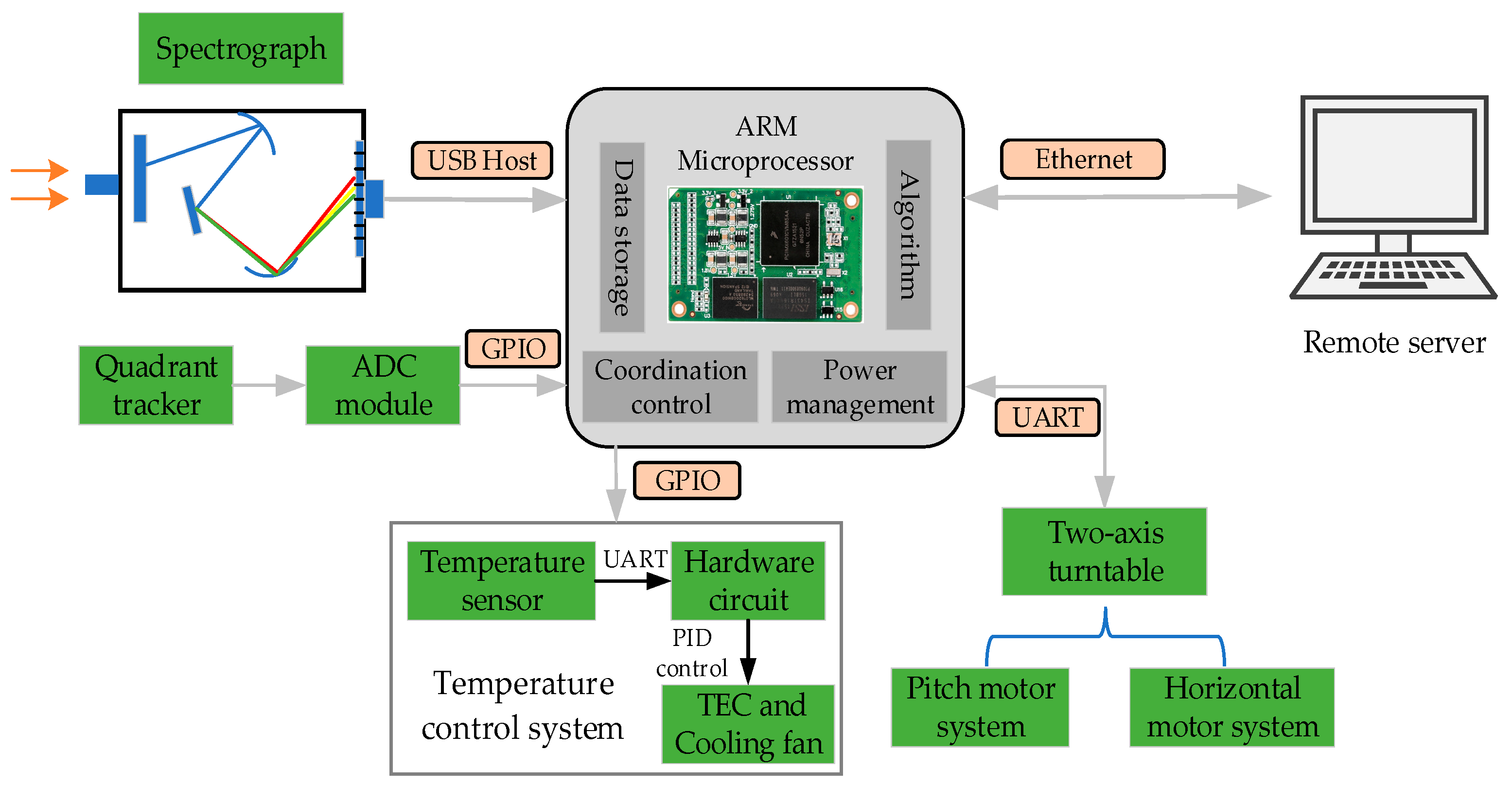
2.3. Radiometer System with Embedded Linux
2.3.1. Two-Axis Turntable
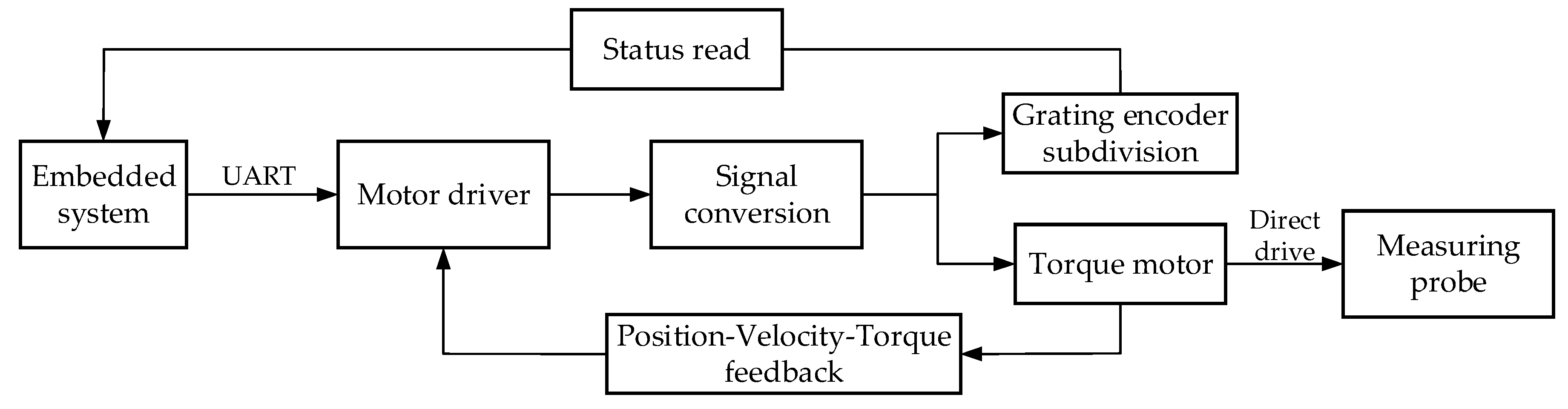
2.3.2. Measuring Probe
2.3.3. Temperature Control System
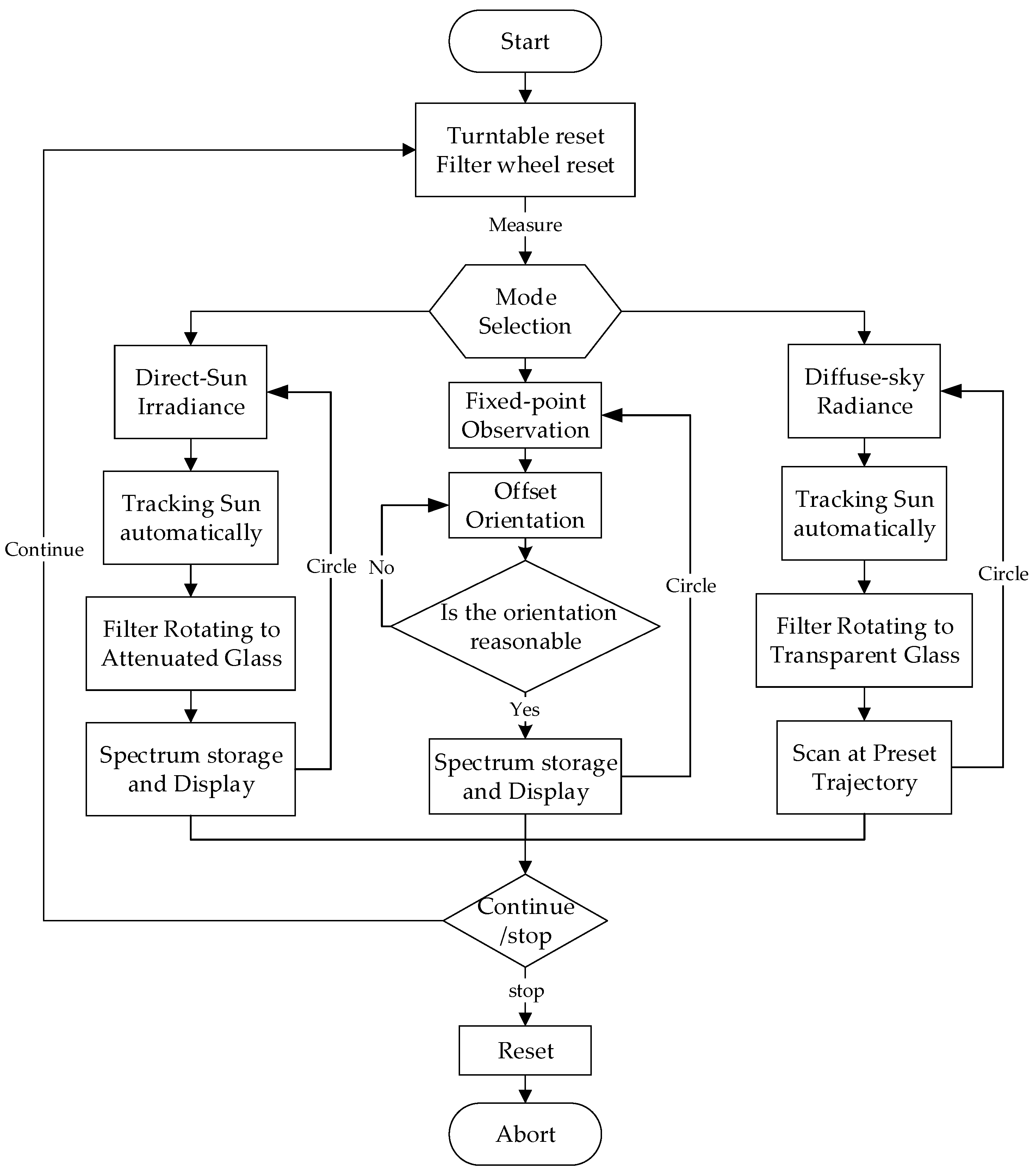
2.4. Workflow
- During the process of direct-sun measurement, solar tracking and radiation collection programs circulate in the microprocessor, so that the direct solar irradiance of the whole day sequence from sunrise to sunset can be obtained. Using the relative flux of directly transmitted radiance varying with the zenith angle, atmospheric parameters such as atmospheric transmittance and water vapor column can be calculated by the inversion model.
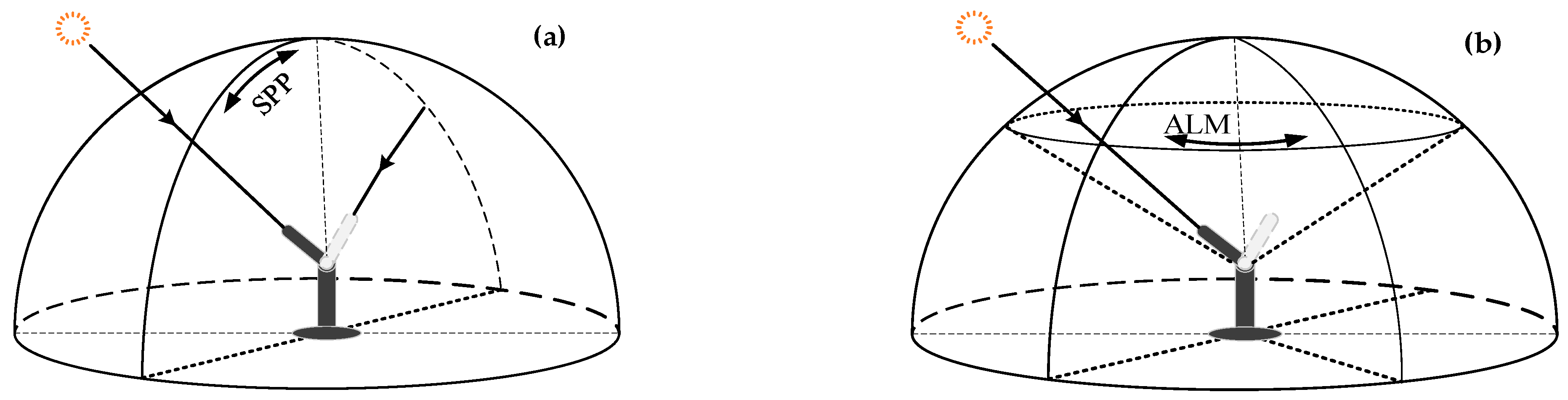
- In diffuse-sky measurement, dual-mode tracking technology is executed to accurately track solar position, after which the spectroradiometer scans the solar principal plane (SPP) and almucantar (ALM) shown in Figure 7 at non-equal intervals to obtain the whole sky radiance.
- As for fixed-point observation, the measuring probe aims at a specific direction to obtain continuous fixed-point radiance. The measurement cycle and integration time of the spectrometer can be dynamically adjusted, but the increase in integration time will lead to the elevation of dark noise and compression of the signal-to-noise ratio. Therefore, the adjustment of integration time adopts the principle of 1~320 ms classification.
3. Calibration
3.1. Calibration of Diffuse-Sky Radiance Measurement
3.2. Calibration of Direct-Sun Irradiance Measurement
- , is the output voltage produced by the spectroradiometer at wavelength when points to the sun at the ground and at the atmospheric top, respectively. is the relative Earth–Sun correction factor.
- is the optical airmass, which describes the increase in the direct optical pathlength from the sun to the detector.
- is the total atmospheric optical depth at wavelength , equal to the sum of aerosol (), ozone () and Rayleigh () optical depth.
4. Result Analysis
4.1. Verification of Transmittance and Water Vapor
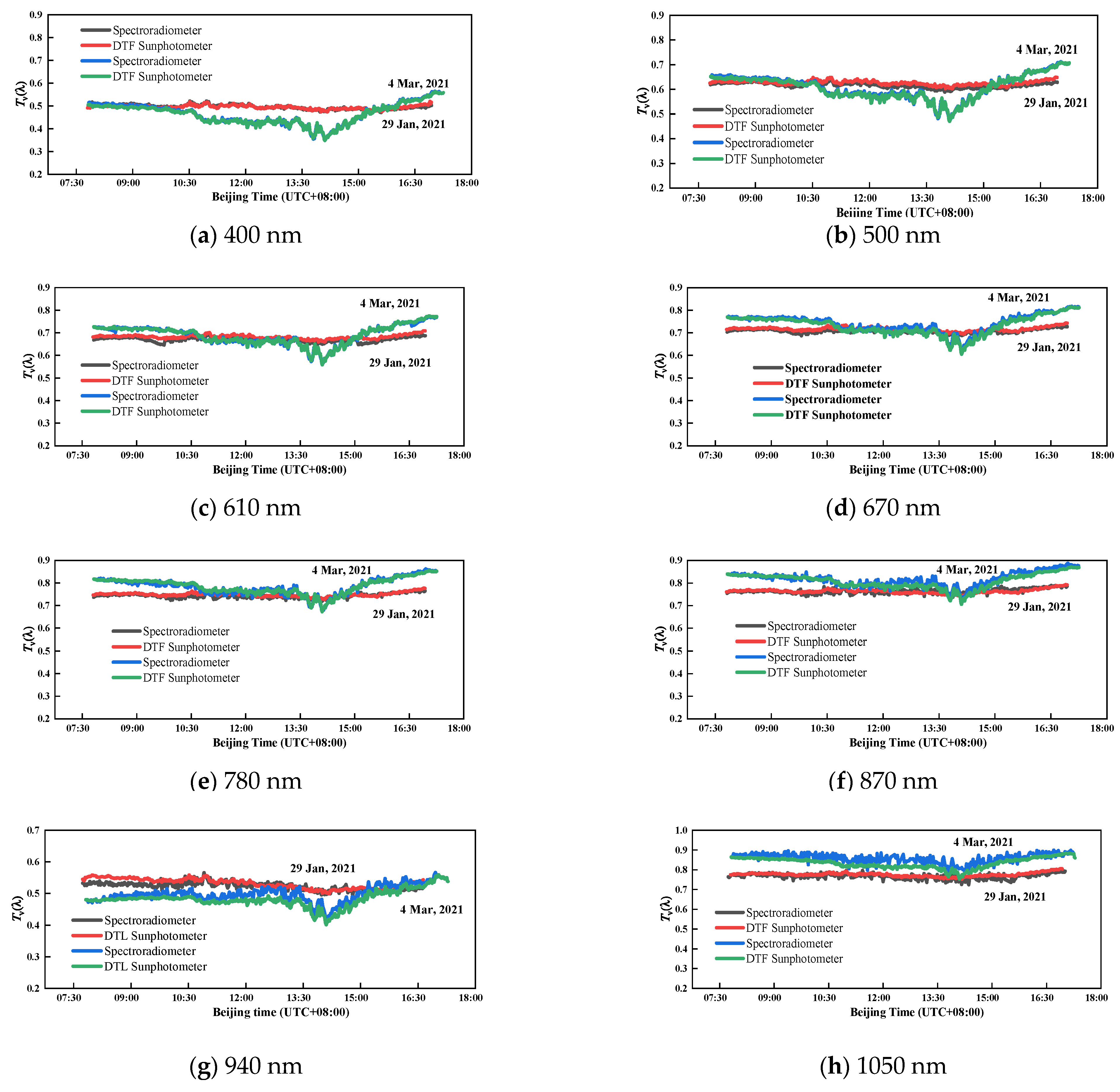
4.1.1. Whole Atmospheric Transmittance
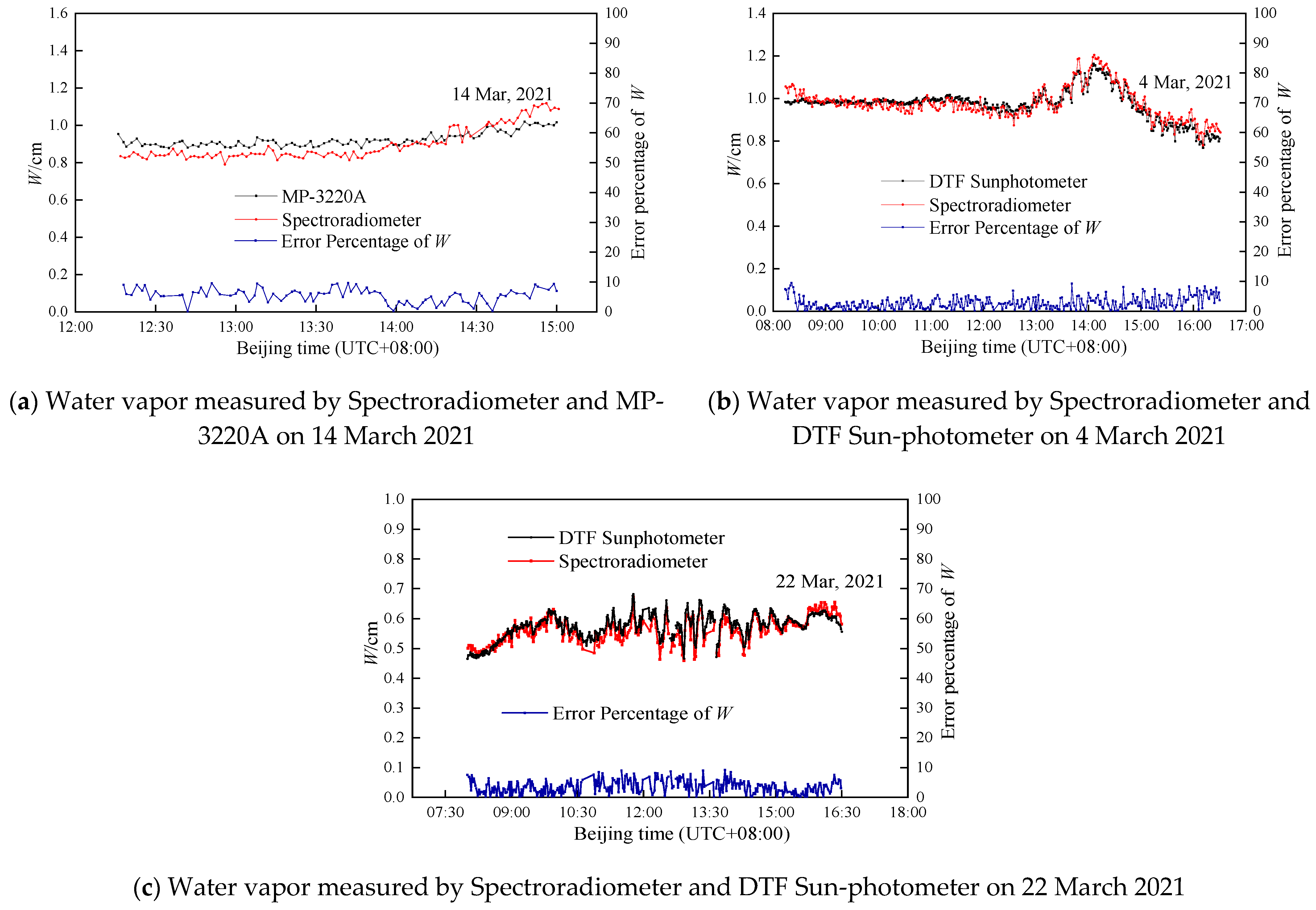
4.1.2. Total Water Vapor
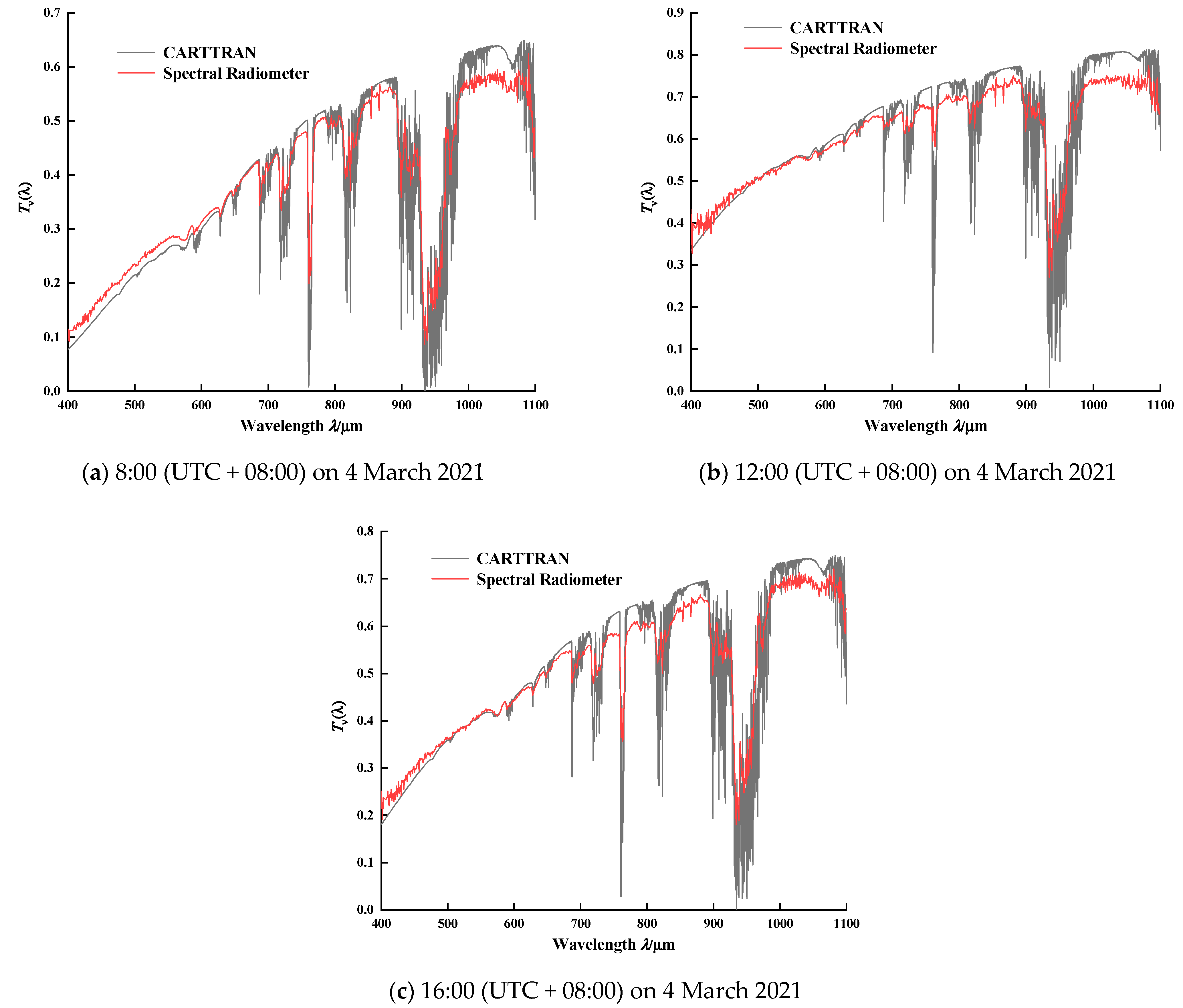
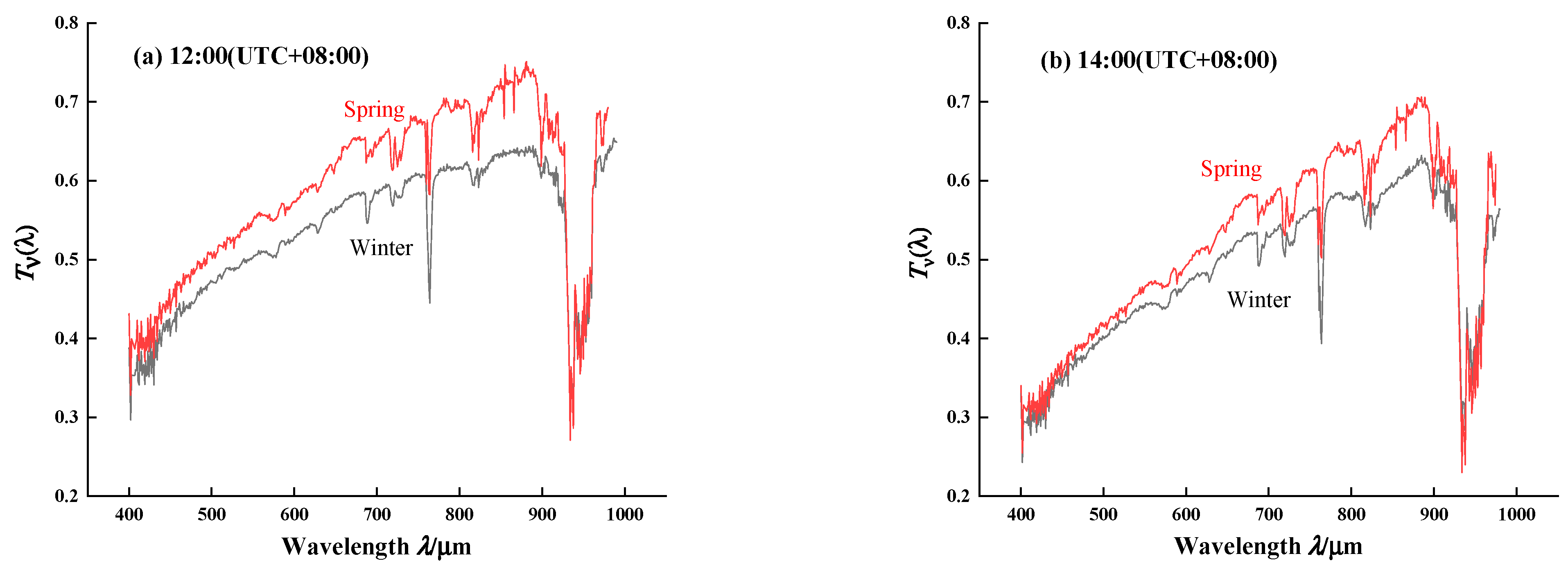
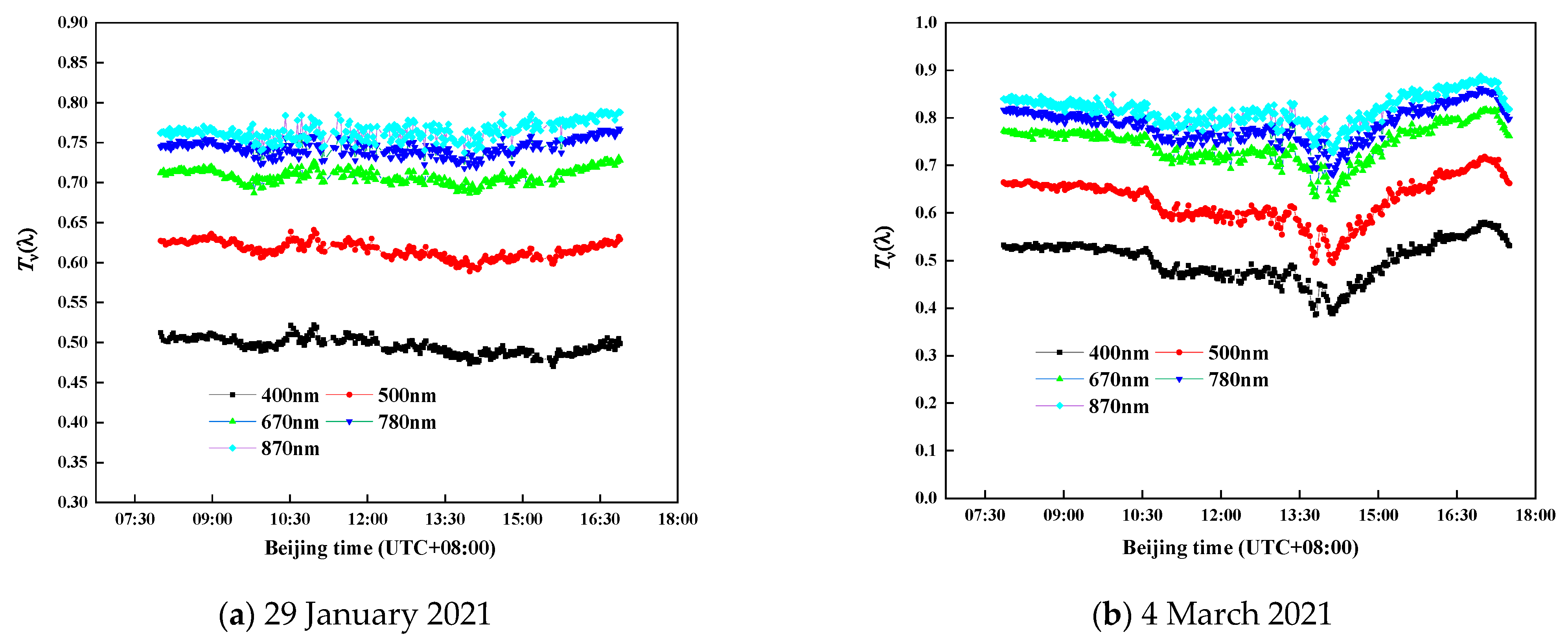
4.2. Continuous Spectrum Transmittance
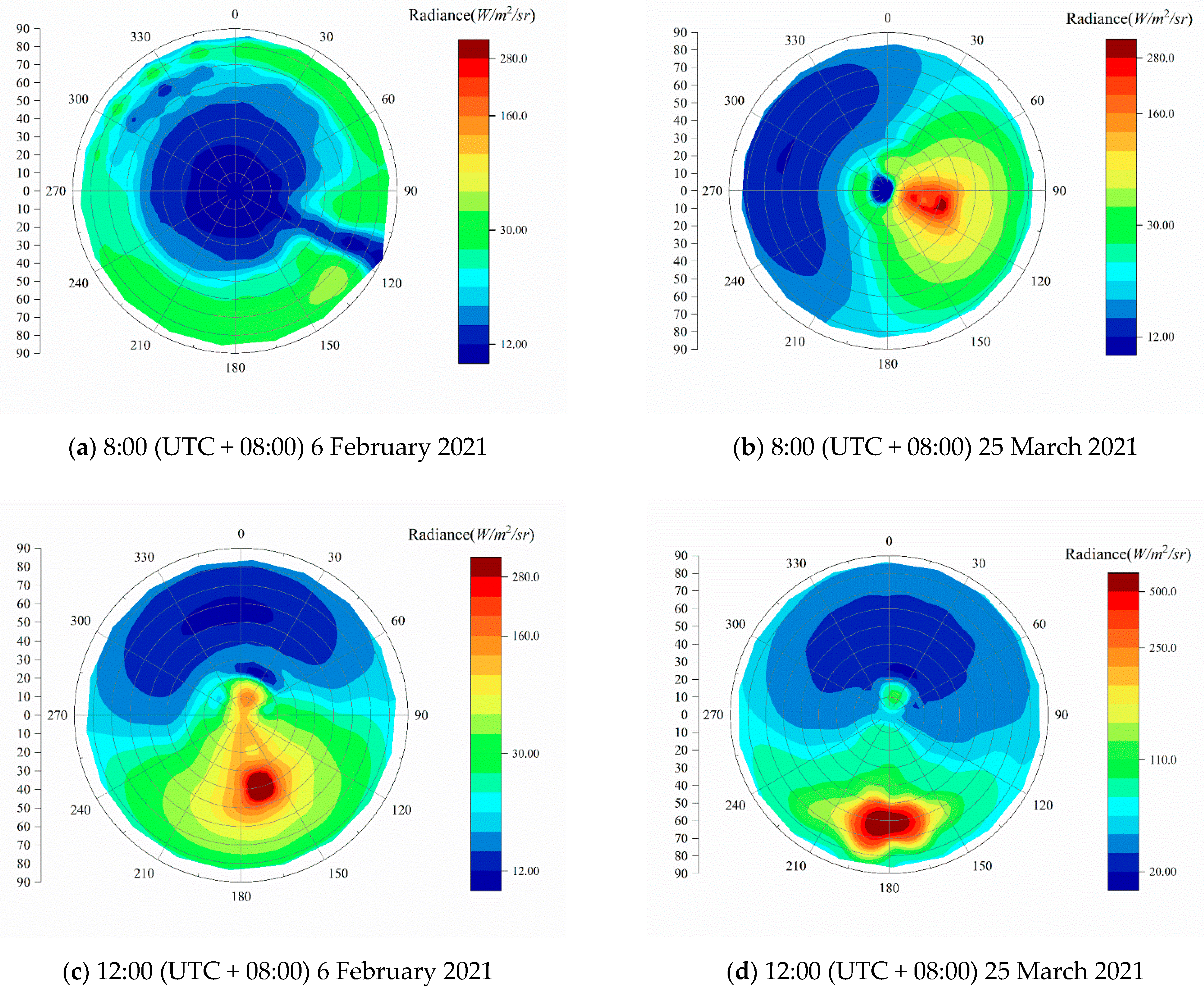
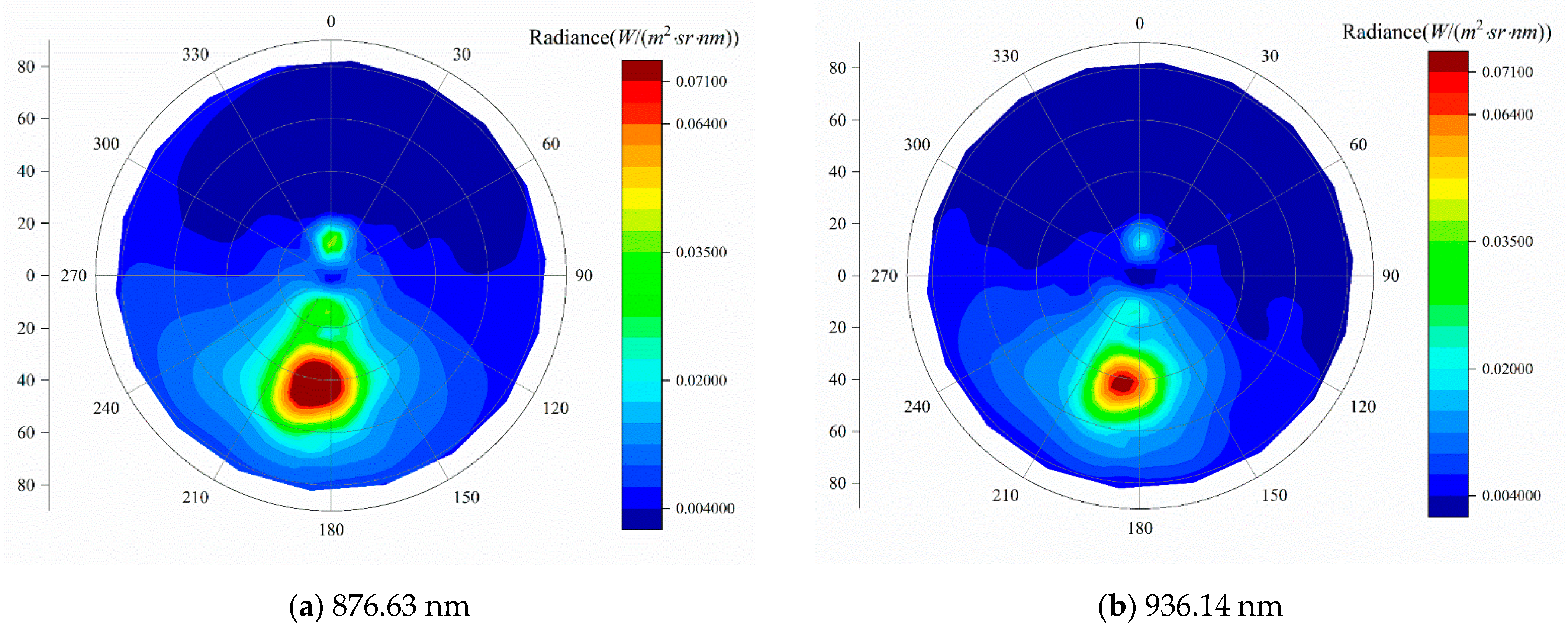
4.3. Diffuse-Sky Radiance
- The radiance distribution under cloud conditions (Figure 15a) is affected by the clouds and the solar zenith angle, which presents an ambiguous result and no obvious regularity.
- On a sunny day, the radiation in the whole sky shows a symmetrical distribution concerning the line connecting the Sun and the zenith. The solar zenith angle is the main factor determining the value of sky radiance. With the increase in solar zenith angle, the sky radiance decreases accordingly.
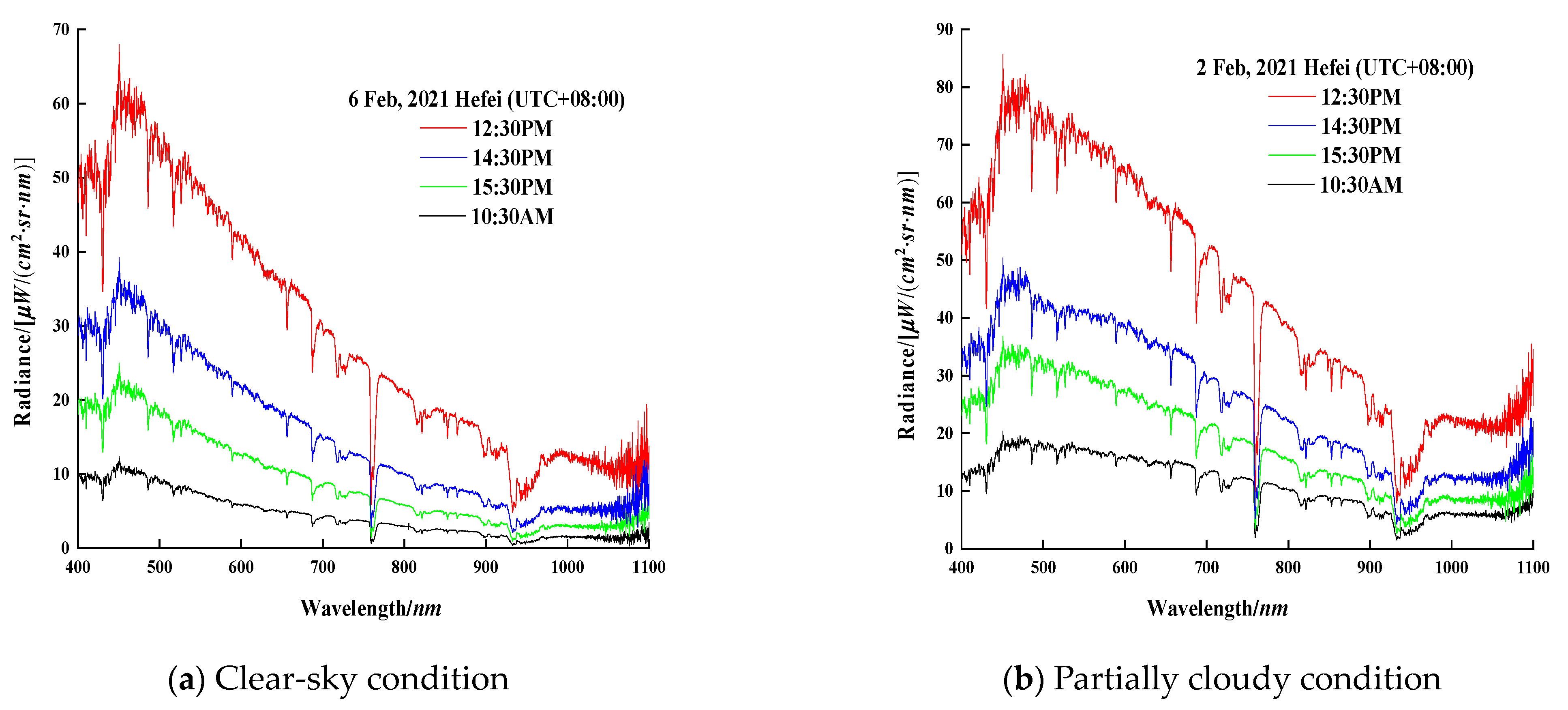
4.4. Fixed-Point Radiance
- Under clear-sky conditions, the radiation curve is relatively smooth with slight fluctuation. With the solar zenith angle becoming lower, the proportion of shortwave radiation decreases and the long-wave radiation increases correspondingly.
- The existence of clouds will affect the distributions of sky radiation. In a partially cloudy condition, the radiation distributions in the entire waveband are relatively uniform, and the curve descent tends to be gentle. The proportion of long-wave radiation is significantly higher when compared to that in the clear-sky condition.
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Aldabash, M.; Balcik, F.B.; Glantz, P. Validation of MODIS C6.1 and MERRA-2 AOD using AERONET observations: A comparative study over Turkey. Atmosphere 2020, 11, 905. [Google Scholar] [CrossRef]
- Liu, L.; Zheng, F.; Zhang, G.; Xu, Y.; Yang, L.; Lü, W.; Bian, Z.; Chong, W.; Li, Y. Meteorological radiation observation based on precision solar spectroradiometer. Spectrosc. Spectr. Anal. 2018, 38, 3663–3672. [Google Scholar]
- Bai, J. A calibration method of solar radiometers. Atmos. Pollut. Res. 2019, 10, 1365–1373. [Google Scholar] [CrossRef]
- Bolsee, D.; Pereira, N.; Decuyper, W.; Gillotay, D.; Yu, H.; Sperfeld, P.; Pape, S.; Cuevas, E.; Redondas, A.; Hernandez, Y.; et al. Accurate determination of the TOA solar spectral NIR irradiance using a primary standard source and the Bouguer-Langley technique. Sol. Phys. 2014, 289, 2433–2457. [Google Scholar] [CrossRef]
- Shindell, D.T.; Schmidt, G.A.; Mann, M.E.; Rind, D.; Waple, A. Solar forcing of regional climate change during the maunder minimum. Science 2001, 294, 2149–2152. [Google Scholar] [CrossRef] [PubMed]
- International Organization for Standardization. Solar Energy—Reference Solar Spectral Irradiance at the Ground at Different Receiving Conditions—Part 1: Direct Normal and Hemispherical Solar Irradiance for Air Mass 1.5; ISO 9845-1; ISO: Geneva, Switzerland, 1992.
- Giles, D.M.; Sinyuk, A.; Sorokin, M.G.; Schafer, J.S.; Smirnov, A.; Slutsker, I.; Eck, T.F.; Holben, B.N.; Lewis, J.R.; Campbell, J.R.; et al. Advancements in the Aerosol Robotic Network (AERONET) Version 3 database—Automated near-real-time quality control algorithm with improved cloud screening for Sun photometer aerosol optical depth (AOD) measurements. Atmos. Meas. Tech. 2019, 12, 169–209. [Google Scholar] [CrossRef]
- Holben, B.N.; Eck, T.F.; Slutsker, I.; Tanre, D.; Buis, J.P.; Setzer, A.; Vermote, E.; Reagan, J.A.; Kaufman, Y.J.; Nakajima, T.; et al. AERONET—A federated instrument network and data archive for aerosol characterization. Remote Sens. Environ. 1998, 66, 1–16. [Google Scholar] [CrossRef]
- Wei, Y.; Li, Z.; Zhang, Y.; Chen, C.; Dubovik, O.; Zhang, Y.; Xu, H.; Li, K.; Chen, J.; Wang, H.; et al. Validation of POLDER GRASP aerosol optical retrieval over China using SONET observations. J. Quant. Spectrosc. Radiat. Transf. 2020, 246, 106931. [Google Scholar] [CrossRef]
- Li, Z.; Li, K.; Li, L.; Xu, H.; Xie, Y.; Ma, Y.; Li, D.; Goloub, P.; Yuan, Y.; Zheng, X. Calibration of the degree of linear polarization measurements of the polarized Sun-sky radiometer based on the POLBOX system. Appl. Opt. 2018, 57, 1011–1018. [Google Scholar] [CrossRef]
- Grob, H.; Emde, C.; Wiegner, M.; Seefeldner, M.; Forster, L.; Mayer, B. The polarized Sun and sky radiometer SSARA: Design, calibration, and application for ground-based aerosol remote sensing. Atmos. Meas. Tech. 2020, 13, 239–258. [Google Scholar] [CrossRef]
- Qi, C.; Zhou, F.; Wu, C.; Hu, X.; Gu, M. Spectral calibration of Fengyun-3 satellite high-spectral resolution infrared sounder. Opt. Precis. Eng. 2019, 27, 747–755. [Google Scholar]
- Li, L.; Chen, H.; Guan, L. Retrieval of snow depth on arctic sea ice from the FY3B/MWRI. Remote Sens. 2021, 13, 1457. [Google Scholar] [CrossRef]
- Liu, L.; Liao, Z.; Chen, C.; Chen, J.; Niu, J.; Jia, Y.; Guo, X.; Chen, Z.; Deng, L.; Xu, H.; et al. A seabed real-time sensing system for in-situ long-term multi-parameter observation applications. Sensors 2019, 9, 1255. [Google Scholar] [CrossRef] [PubMed]
- Mera-Romo, D.E.; Rodriguez-Solis, R.A. Low power and miniaturized back-end processing system for an L-Band radiometer based on ARM embedded microprocessor. In Proceedings of the 2019 IEEE Radio and Wireless Symposium, Orlando, FL, USA, 20–23 January 2019; pp. 220–223. [Google Scholar]
- Munoz-Martin, J.F.; Fernandez, C.L.; Ruiz-de-Azua, J.A.; Camps, A. The flexible microwave payload-2: A SDR-based GNSS-reflectometer and L-Band radiometer for CubeSats. IEEE J. Sel. Top. Appl. Earth Obs. Remote Sens. 2020, 13, 1298–1311. [Google Scholar] [CrossRef]
- Li, J.; Liu, Q.; Xu, W.; Huang, H.; Zhu, W. Solar radiometer for measurement of multi-waveband laser atmospheric transmittance. Opt. Precis. Eng. 2020, 28, 261–270. [Google Scholar]
- Luo, C.; Ma, B.; Chen, F.; Guan, Q.; Yu, H.; Ji, F. Multicore-processor based software-defined communication/network platform for underwater Internet of things. Sensors 2019, 19, 5168. [Google Scholar] [CrossRef]
- Fernandez, J.P.; Vargas, M.A.; Garcia, J.M.V.; Carrillo, J.A.C.; Aguilar, J.J.C. Low-Cost FPGA-Based Electronic Control Unit for Vehicle Control Systems. Sensors 2019, 19, 1834. [Google Scholar] [CrossRef]
- Wahrburg, A.; Bös, J.; Listmann, K.D.; Dai, F.; Matthias, B.; Ding, H. Motor-current-based estimation of cartesian contact forces and torques for robotic manipulators and its application to force control. IEEE Trans. Autom. Sci. Eng. 2018, 15, 879–886. [Google Scholar] [CrossRef]
- Yen, S.; Tang, P.; Lin, Y.; Lin, C. Development of a virtual force sensor for a low-cost collaborative robot and applications to safety control. Sensors 2019, 19, 2603. [Google Scholar] [CrossRef]
- Libusb. Available online: https://github.com/libusb/libusb/releases (accessed on 2 May 2021).
- Zhang, Q.; Li, X.; Zhang, Y.; Huang, D.; Zheng, X. Opto-mechanical design and thermodynamic finite element analysis of automatic multi-band solar radiometer. Acta Photonica Sin. 2019, 48, 177–187. [Google Scholar]
- Xu, Q.; Zheng, X.; Li, Z.; Zhang, W.; Wang, X.; Li, J.; Li, X. Absolute spectral radiance responsivity calibration of sun photometers. Rev. Sci. Instrum. 2010, 81, 033103. [Google Scholar] [CrossRef] [PubMed]
- Thome, K.J.; Herman, B.M.; Reagan, J.A. Determination of precipitable water from solar transmission. J. Appl. Meteorol. 1992, 31, 157–165. [Google Scholar] [CrossRef]
- Li, Z.; Blarel, L.; Podvin, T.; Goloub, P.; Buis, J.P.; Morel, J.P. Transferring the calibration of direct solar irradiance to diffuse-sky radiance measurements for CIMEL Sun-sky radiometers. Appl. Opt. 2008, 47, 1368–1377. [Google Scholar] [CrossRef] [PubMed]
- Shaw, G.E.; Reagan, J.A.; Herman, B.M. Investigations of atmospheric extinction using direct solar radiation measurements made with a multiple wavelength radiometer. J. Appl. Meteorol. 1973, 12, 374–380. [Google Scholar] [CrossRef]
- Uchiyama, A.; Yamazaki, A.; Kudo, R. Column water vapor retrievals from sky radiometer (POM-02) 940 nm Data. J. Meteorol. Soc. Jpn. 2014, 92A, 195–203. [Google Scholar] [CrossRef]
- Zhou, N.; Liu, M. Total column water vapor retrieval methods and results comparison by using sun photometer. J. Remote Sens. 2011, 15, 568–577. [Google Scholar]
- Bedoya-Velasquez, A.E.; Herreras-Giralda, M.; Roman, R.; Wiegner, M.; Lefebvre, S.; Toledano, C.; Huet, T.; Ceolato, R. Ceilometer inversion method using water-vapor correction from co-located microwave radiometer for aerosol retrievals. Atmos. Res. 2021, 250, 105379. [Google Scholar] [CrossRef]
- Wei, H.; Dai, C.; Wu, P.; Tang, C.; Zhao, F.; Wu, X.; Rao, R.; Wang, Y. An upgraded combined atmospheric radiative transfer CART2(Invited). Infrared Laser Eng. 2020, 49, 61–68. [Google Scholar]
- Nakajima, T.; King, M.D. Determination of the optical-thickness and effective particle radius of clouds from reflected solar-radiation measurements. Part Ι: Theory. J. Atmos. Sci. 1990, 47, 1878–1893. [Google Scholar] [CrossRef]
- Yang, D.; Li, J.; Xu, W.; Xu, Q.; Wei, H. Fast measurement and cloud detection of solar transmission ratio at different fields of view. Acta Opt. Sin. 2018, 38, 186–193. [Google Scholar]




| Performance Characteristics | Specifications |
|---|---|
| Measurement waveband | 380~1100 nm |
| Spectrum resolution | <1 nm |
| Measuring mode | direct-sun irradiance, diffuse-sky radiance |
| Field of view | 0.8° |
| Tracking angle resolution | <5 arcsec |
| Positioning accuracy | <40 arcsec |
| Control architecture | ARM-Linux architecture |
| Working temperature | −30~55 °C |
| Date | Parameter | Selected Eight Wavebands | |||||||
|---|---|---|---|---|---|---|---|---|---|
| 400 nm | 500 nm | 610 nm | 670 nm | 780 nm | 870 nm | 940 nm | 1050 nm | ||
| 29 January 2021 | InG0 | 11.0146 | 13.0431 | 13.5085 | 13.2467 | 12.3778 | 11.52 | 12.5569 | 11.236 |
| R | −0.9990 | −0.9994 | −0.9986 | −0.9988 | −0.9985 | −0.9984 | −0.9957 | −0.9969 | |
| SD | 0.00237 | 0.00132 | 0.00163 | 0.00132 | 0.00126 | 0.0012 | 0.00261 | 0.00163 | |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Qi, Z.; Li, J.; Xu, W.; Zhu, W.; Sun, F.; Huang, Y.; Xu, G.; Dai, C. Optomechanical Design and Application of Solar-Skylight Spectroradiometer. Sensors 2021, 21, 3751. https://doi.org/10.3390/s21113751
Qi Z, Li J, Xu W, Zhu W, Sun F, Huang Y, Xu G, Dai C. Optomechanical Design and Application of Solar-Skylight Spectroradiometer. Sensors. 2021; 21(11):3751. https://doi.org/10.3390/s21113751
Chicago/Turabian StyleQi, Zhaoyang, Jianyu Li, Wenqing Xu, Wenyue Zhu, Fengying Sun, Yao Huang, Gang Xu, and Congming Dai. 2021. "Optomechanical Design and Application of Solar-Skylight Spectroradiometer" Sensors 21, no. 11: 3751. https://doi.org/10.3390/s21113751
APA StyleQi, Z., Li, J., Xu, W., Zhu, W., Sun, F., Huang, Y., Xu, G., & Dai, C. (2021). Optomechanical Design and Application of Solar-Skylight Spectroradiometer. Sensors, 21(11), 3751. https://doi.org/10.3390/s21113751






