Determination of the Dielectric Properties of Storage Materials for Exhaust Gas Aftertreatment Using the Microwave Cavity Perturbation Method
Abstract
1. Introduction
2. Laboratory Setup, Simulation Model and MCP-Fundamentals
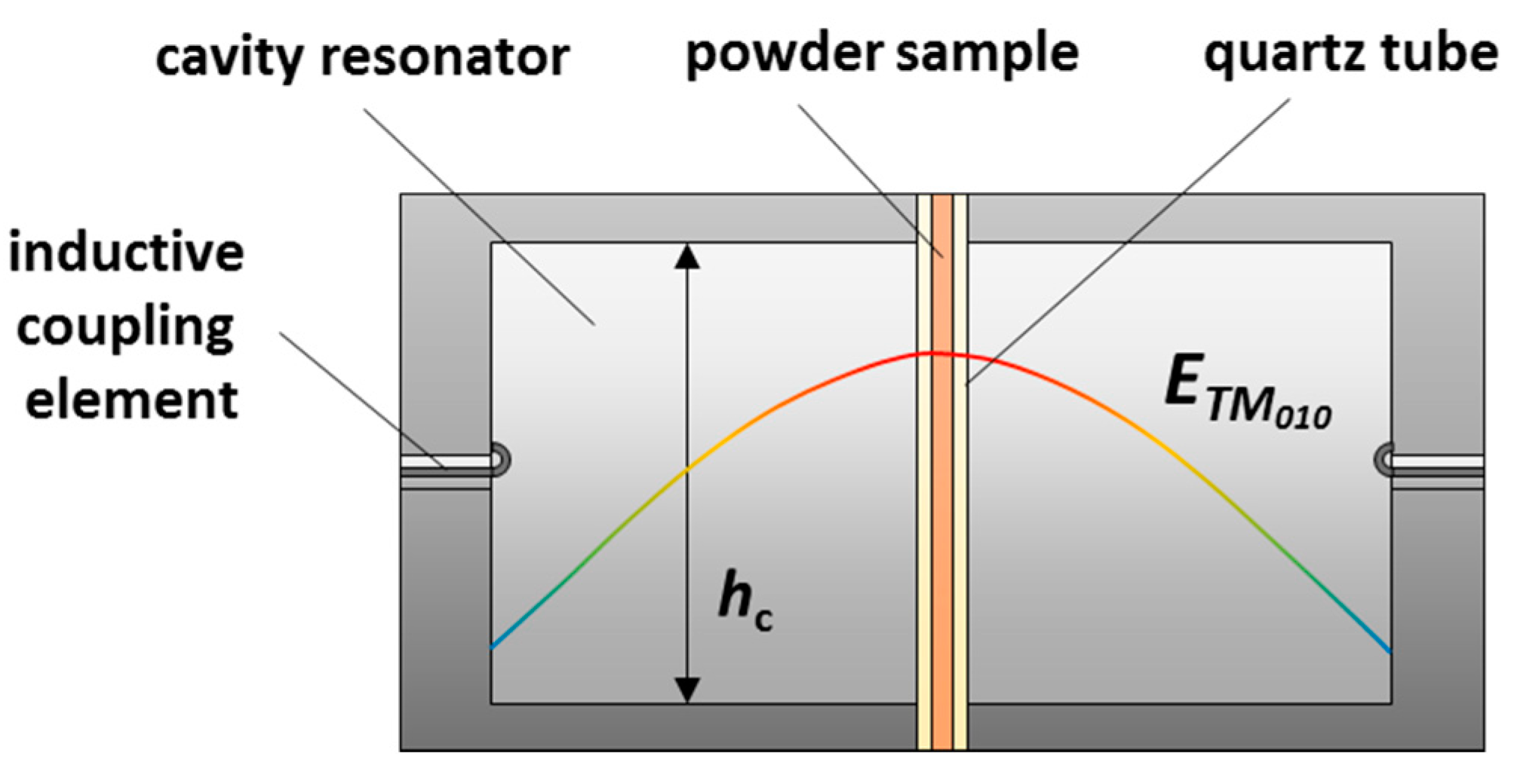
2.1. Resonator Setup
2.2. Microwave Cavity Perturbation Theory
- The sample volume is small compared to the resonator volume.
- The sample height and the resonator height are identical (hs = hc) or the sample has the shape of a thin rod at least.
- The sample material is homogeneous and isotropic,
2.3. Evaluation for Investigations on Powders for Exhaust Gas Aftertreatment
3. Extension of the Microwave Cavity Perturbation Theory
3.1. Electric Field Distribution Inside the Cavity Resonator
3.2. Depolarization Field of the Sample
3.3. Bulk Properties of the Powder Samples
4. Validation of the Measurement Method, Transferability and Alternative Approaches
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Moos, R.; Wedemann, M.; Spörl, M.; Reiß, S.; Fischerauer, G. Direct Catalyst Monitoring by Electrical Means: An Overview on Promising Novel Principles. Top. Catal. 2009, 52, 2035–2040. [Google Scholar] [CrossRef]
- Birkhofer, T.; Hofmann, P.; Knezevic, A.; Moos, R.; Plog, C.; Schneider, R. Verfahren zur Erkennung des Zustands Eines Katalysators Mittels Mikrowellen. German Patent Specification DE10358495, 13 December 2003. [Google Scholar]
- Reiß, S.; Spörl, M.; Hagen, G.; Fischerauer, G.; Moos, R. Combination of Wirebound and Microwave Measurements for In-Situ Characterization of Automotive Three-Way Catalysts. IEEE Sens. J. 2011, 11, 434–438. [Google Scholar] [CrossRef]
- Moos, R.; Spörl, M.; Hagen, G.; Gollwitzer, A.; Wedemann, M.; Fischerauer, G. TWC: Lambda Control and OBD without Lambda Probe—An Initial Approach. SAE Tech. Pap. 2008. [Google Scholar] [CrossRef]
- Beulertz, G.; Votsmeier, M.; Moos, R. In operando Detection of Three-Way Catalyst Aging by a Microwave-Based Method: Initial Studies. Appl. Sci. 2015, 5, 174–186. [Google Scholar] [CrossRef]
- Reiß, S.; Wedemann, M.; Spörl, M.; Fischerauer, G.; Moos, R. Effects of H2O, CO2, CO, and Flow Rates on the RF-Based Monitoring of Three-Way Catalysts. Sens. Lett. 2011, 9, 316–320. [Google Scholar] [CrossRef]
- Beulertz, G.; Votsmeier, M.; Moos, R. Effect of Propene, Propane, and Methane on Conversion and Oxidation State of Three-Way Catalysts: A Microwave Cavity Perturbation Study. Appl. Catal. B 2015, 165, 369–377. [Google Scholar] [CrossRef]
- Steiner, C.; Malashchuk, V.; Kubinski, D.; Hagen, G.; Moos, R. Catalyst State Diagnosis of Three-Way Catalytic Converters Using Different Resonant Parameters—A Microwave Cavity Perturbation Study. Sensors 2019, 19, 3559. [Google Scholar] [CrossRef] [PubMed]
- Dietrich, M.; Jahn, C.; Lanzerath, P.; Moos, R. Microwave-Based Oxidation State and Soot Loading Determination on Gasoline Particulate Filters with Three-Way Catalyst Coating for Homogenously Operated Gasoline Engines. Sensors 2015, 15, 21971–21988. [Google Scholar] [CrossRef] [PubMed]
- Walter, S.; Schwanzer, P.; Hagen, G.; Haft, G.; Dietrich, M.; Rabl, H.-P.; Moos, R. Hochfrequenzsensorik zur direkten Beladungserkennung von Benzinpartikelfiltern. In Automobil-Sensorik 3; Tille, T., Ed.; Springer: Berlin, Germany, 2020; pp. 185–208. [Google Scholar]
- Reiß, S.; Schönauer, D.; Hagen, G.; Fischerauer, G.; Moos, R. Monitoring the Ammonia Loading of Zeolite-Based Ammonia SCR Catalysts by A Microwave Method. Chem. Eng. Technol. 2011, 34, 791–796. [Google Scholar] [CrossRef]
- Rauch, D.; Kubinski, D.; Simon, U.; Moos, R. Detection of the Ammonia Loading of a Cu Chabazite SCR Catalyst by a Radio Frequency-Based Method. Sens. Actuators B 2014, 205, 88–93. [Google Scholar] [CrossRef]
- Rauch, D.; Kubinski, D.; Cavataio, G.; Upadhyay, D.; Moos, R. Ammonia Loading Detection of Zeolite SCR Catalysts Using a Radio Frequency Based Method. SAE Int. J. Engines 2015, 8, 1126–1135. [Google Scholar] [CrossRef]
- Fremerey, P.; Reiß, S.; Geupel, A.; Fischerauer, G.; Moos, R. Determination of the NOx Loading of an Automotive Lean NOx Trap by Directly Monitoring the Electrical Properties of the Catalyst Material Itself. Sensors 2011, 11, 8261–8280. [Google Scholar] [CrossRef] [PubMed]
- Walter, S.; Ruwisch, L.; Göbel, U.; Hagen, G.; Moos, R. Radio Frequency-Based Determination of the Oxygen and the NOx Storage Level of NOx Storage Catalysts. Top. Catal. 2019, 62, 157–163. [Google Scholar] [CrossRef]
- Feulner, M.; Hagen, G.; Moos, R.; Piontkowski, A.; Müller, A.; Fischerauer, G.; Brüggemann, D. In-Operation Monitoring of the Soot Load of Diesel Particulate Filters: Initial Tests. Top. Catal. 2013, 56, 483–488. [Google Scholar] [CrossRef]
- Sappok, A.; Parks, J.; Prikhodko, V. Loading and Regeneration Analysis of a Diesel Particulate Filter with a Radio Frequency-Based Sensor. SAE Tech. Pap. 2010. [Google Scholar] [CrossRef]
- Dietrich, M.; Rauch, D.; Porch, A.; Moos, R. A laboratory Test Setup for in Situ Measurements of the Dielectric Properties of Catalyst Powder Samples under Reaction Conditions by Microwave Cavity Perturbation: Set Up and Initial Tests. Sensors 2014, 14, 16856–16868. [Google Scholar] [CrossRef]
- Rauch, D.; Dietrich, M.; Simons, T.; Simon, U.; Porch, A.; Moos, R. Microwave Cavity Perturbation Studies on H-form and Cu Ion-Exchanged SCR Catalyst Materials: Correlation of Ammonia Storage and Dielectric Properties. Top. Catal. 2017, 60, 243–249. [Google Scholar] [CrossRef]
- Dietrich, M.; Rauch, D.; Simon, U.; Porch, A.; Moos, R. Ammonia Storage Studies on H-ZSM-5 Zeolites by Microwave Cavity Perturbation: Correlation of dielectric Properties with Ammonia Storage. J. Sens. Sens. Syst. 2015, 4, 263–269. [Google Scholar] [CrossRef]
- Steiner, C.; Gänzler, A.M.; Zehentbauer, M.; Hagen, G.; Casapu, M.; Müller, S.; Grunwaldt, J.-D.; Moos, R. Oxidation State and Dielectric Properties of Ceria-Based Catalysts by Complementary Microwave Cavity Perturbation and X-Ray Absorption Spectroscopy Measurements. Top. Catal. 2019, 62, 227–236. [Google Scholar] [CrossRef]
- Chen, L.F.; Ong, C.K.; Neo, C.P.; Varadan, V.V.; Varadan, V.K. Microwave Electronics—Measurement and Materials; John Wiley & Sons: Hoboken, NJ, USA, 2004. [Google Scholar] [CrossRef]
- Pozar, D.M. Microwave Engineering, 4th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2012. [Google Scholar]
- Kim, C.-K.; Minz, L.; Park, S.-O. Improved Measurement Method of Material Properties Using Continuous Cavity Perturbation Without Relocation. IEEE Trans. Instrum. Meas. 2020, 69, 5702–5716. [Google Scholar] [CrossRef]
- Parkash, A.; Vaid, J.K.; Mansingh, A. Measurement of Dielectric Parameters at Microwave Frequencies by Cavity Perturbation Technique. IEEE Trans. Microw. Theory Tech. 1979, 27, 791–795. [Google Scholar] [CrossRef]
- Waldron, R.A. Perturbation Theory of Resonant Cavities. Proc. IEEC Monogr. 1970, 107, 272–274. [Google Scholar] [CrossRef]
- Lin, M.; Afsar, M. A New Cavity Perturbation Technique for Accurate Measurement of Dielectric Parameters. In Proceedings of the 2006 IEEE MTT-S International Microwave Symposium Digest, San Francisco, CA, USA, 11–16 June 2006; pp. 1630–1633. [Google Scholar] [CrossRef]
- Lin, M.; Wang, Y.; Afsar, M.N. Precision Measurement of Complex Permittivity and Permeability by Microwave Cavity Perturbation Technique. In Proceedings of the 2005 Joint 30th International Conference on Infrared and Millimeter Waves and 13th International Conference on Terahertz Electronics, Williamsburg, VA, USA, 19–23 September 2005; pp. 62–63. [Google Scholar] [CrossRef]
- Yang, R.B.; Tsay, C.J.; Hung, D.S.; Liang, W.F.; Yao, Y.D.; Lin, C.K. Complex Permittivity and Permeability of Iron-Based Composite Absorbers Measured by Cavity Perturbation Method in X-Bandfrequency Range. J. Appl. Phys. 2009, 105. [Google Scholar] [CrossRef]
- Peng, Z.; Hwang, J.-Y.; Andriese, M. Maximum Sample Volume for Permittivity Measurements by Cavity Perturbation Technique. IEEE Trans. Instrum. Meas. 2014, 63, 450–455. [Google Scholar] [CrossRef]
- Verma, A.; Dube, D. Measurement of Dielectric Parameters of Small Samples at X-Band Frequencies by Cavity Perturbation Technique. IEEE Trans. Instrum. Meas. 2005, 54, 2120–2123. [Google Scholar] [CrossRef]
- Cuenca, J.A.; Klein, S.; Ruger, R.; Porch, A. Microwave Complex Permeability of Magnetite Using Non-Demagnetising and Demagnetising Cavity Modes. Proc. Eur. Microw. Conf. 2014, 44, 128–131. [Google Scholar] [CrossRef]
- Chen, L.; Ong, C.; Tan, B. Amendment of Cavity Perturbation Method for Permittivity Measurement of Extremely Low-Loss Dielectrics. IEEE Trans. Instrum. Meas. 1999, 48, 1031–1037. [Google Scholar] [CrossRef]
- Meng, B.; Booske, J.; Cooper, R. Extended Cavity Perturbation Technique to Determine the Complex Permittivity of Dielectric Materials. IEEE Trans. Microw. Theory Tech. 1995, 43, 2633–2636. [Google Scholar] [CrossRef]
- Orloff, N.D.; Obrzut, J.; Long, C.J.; Lam, T.; Kabos, P.; Novotny, D.R.; Booth, J.C.; Liddle, J.A. Dielectric Characterization by Microwave Cavity Perturbation Corrected for Nonuniform Fields. IEEE Trans. Microw. Theory Tech. 2014, 62, 2149–2159. [Google Scholar] [CrossRef]
- Tuller, H.L.; Nowick, A.S. Defect Structure and Electrical Properties of Nonstoichiometric CeO2 Single Crystals. J. Electrochem. Soc. 1979, 126, 209–217. [Google Scholar] [CrossRef]
- Xiong, Y.-P.; Kishimoto, H.; Yamaji, K.; Yoshinaga, M.; Horita, T.; Brito, M.E.; Yokokawa, H. Electronic Conductivity of Pure Ceria. Solid State Ion. 2011, 192, 476–479. [Google Scholar] [CrossRef]
- Rowe, D.J.; al-Malki, S.; Abduljabar, A.A.; Porch, A.; Barrow, D.A.; Allender, C.J. Improved Split-Ring Resonator for Microfluidic Sensing. IEEE Trans. Microw. Theory Tech. 2014, 62, 689–699. [Google Scholar] [CrossRef]
- Kobayashi, H.; Ogawa, S. Dielectric Constant and Conductivity Measurement of Powder Samples by the Cavity Peturbation Method. Jpn. J. Appl. Phys. 1971, 10, 345–350. [Google Scholar] [CrossRef]
- Subramanian, V.; Sivasubramanian, V.; Murthy, V.R.K.; Sobhanadri, J. Measurement of Complex Dielectric Permittivity of Partially Inserted Samples in a Cavity Perturbation Technique. Rev. Sci. Instrum. 1996, 67, 279–282. [Google Scholar] [CrossRef]
- Dube, D.C. Study of Landau-Lifshitz-Looyenga’s Formula for Dielectric Correlation between Powder and Bulk. J. Phys. D Appl. Phys. 1970, 3, 1648–1652. [Google Scholar] [CrossRef]
- Tuhkala, M.; Juuti, J.; Jantunen, H. An Indirectly Coupled Open-Ended Resonator Applied to Characterize Dielectric Properties of MgTiO3–CaTiO3 Powders. J. Appl. Phys. 2014, 115, 184101. [Google Scholar] [CrossRef]
- Cheng, E.M.; Malek, F.; Ahmed, M.; You, K.Y.; Lee, K.Y.; Nornikman, H. The Use of Dielectric Mixture Equations to Analyse the Dielectric Properties of a Mixture of Rubber Tire Dust and Rice Husk in a Microwave Absorber. Prog. Electromagn. Res. 2012, 129, 559–578. [Google Scholar] [CrossRef][Green Version]
- Santha, N.I.; Sebastian, M.T.; Mohanan, P.; Alford, N.M.; Sarma, K.; Pullar, R.C.; Kamba, S.; Pashkin, A.; Samukhina, P.; Petzelt, J. Effect of Doping on the Dielectric Properties of Cerium Oxide on the Microwave and Far-Infrared Frequency Range. J. Am. Ceram. Soc. 2004, 87, 1233–1237. [Google Scholar] [CrossRef]
- Chiu, F.-C.; Lai, C.-M. Optical and Electrical Characterizations of Cerium Oxide Thin Films. J. Phys. D Appl. Phys. 2010, 43, 075104. [Google Scholar] [CrossRef]
- Yamamoto, T.; Momida, H.; Hamada, T.; Uda, T.; Ohno, T. First-Principles Study of Dielectric Properties of Cerium Oxide. Thin Solid Film. 2005, 486, 136–140. [Google Scholar] [CrossRef]
- Bozorth, R.M.; Chapin, D.M. Demagnetizing Factors of Rods. J. Appl. Phys. 1942, 13, 320–326. [Google Scholar] [CrossRef]
- Ogawa, T. Measurement of the Electrical Conductivity and Dielectric Constant without Contacting Electrodes. J. Appl. Phys. 1961, 32, 583–592. [Google Scholar] [CrossRef]
- Venermo, J.; Sihvola, S. Dielectric Polarizability of Circular Cylinder. J. Electrost. 2005, 63, 101–117. [Google Scholar] [CrossRef]
- Birchak, J.R.; Gardner, L.G.; Hipp, J.W.; Victor, J.M. High Dielectric Constant Microwave Probes for Sensing Soil Moisture. Proc. IEEE 1974, 62, 93–98. [Google Scholar] [CrossRef]
- Jusoh, M.A.; Abbas, Z.; Hassan, J.; Azmi, B.Z.; Ahmad, A.F. A Simple Procedure to Determine Complex Permittivity of Moist Materials Using Standard Commercial Coaxial Sensor. Meas. Sci. Rev. 2011, 11, 19–22. [Google Scholar] [CrossRef]
- Goncharenko, A. Generalizations of the Bruggeman Equation and a Concept of Shape-Distributed Particle Composites. Phys. Rev. 2003, 68, 041108. [Google Scholar] [CrossRef]
- Looyenga, H. Dielectric Constants of Heterogeneous Mixtures. Physica 1965, 31, 401–406. [Google Scholar] [CrossRef]
- Marquardt, P.; Nimtz, G. Size-Governed Electromagnetic Absorption by Metal Particles. Phys. Rev. B 1989, 40, 7996. [Google Scholar] [CrossRef]
- Marquardt, P. Quantum-size affected conductivity of mesoscopic metal particles. Phys. Lett. A 1987, 123, 365–368. [Google Scholar] [CrossRef]
- Marquardt, P.; Nimtz, G. Critical Dielectric Behavior of Micellar Water below the Cloud point of a Water-Oil Microemulsion. Phys. Rev. Lett. 1986, 57, 1036. [Google Scholar] [CrossRef]
- Lichtenecker, K.; Rother, K. Die Herleitung des Logarithmischen Mischungsgesetzes aus Allgemeinen Prinzipien der Stationären Strömung. Phys. Z. 1931, 32, 255–260. [Google Scholar]
- Sihvola, A. Mixing Rules with Complex Dielectric Coefficients. Subsurf. Sens. Technol. Appl. 2000, 1, 393–415. [Google Scholar] [CrossRef]
- Maxwell Garnett, J.C. Colors in Metal Glasses and Metal Films and in Metallic Solutions. Trans. R. Soc. 1905, 53, 385–420. [Google Scholar] [CrossRef]
- Wiener, O. Zur Theorie der Refraktionskonstanten. Math.-Phys. Kl. 1910, 62, 256–277. [Google Scholar]
- Farra, R.; Garcia-Melchor, M.; Eichelbaum, M.; Hashagen, M.; Frandsen, W.; Allan, J.; Girgsdies, F.; Szentmiklosi, L.; Lopez, N.; Teschner, D. Promoted Ceria: A Structural, Catalytic, and Computational Study. ACS Catal. 2013, 3, 2256–2268. [Google Scholar] [CrossRef]

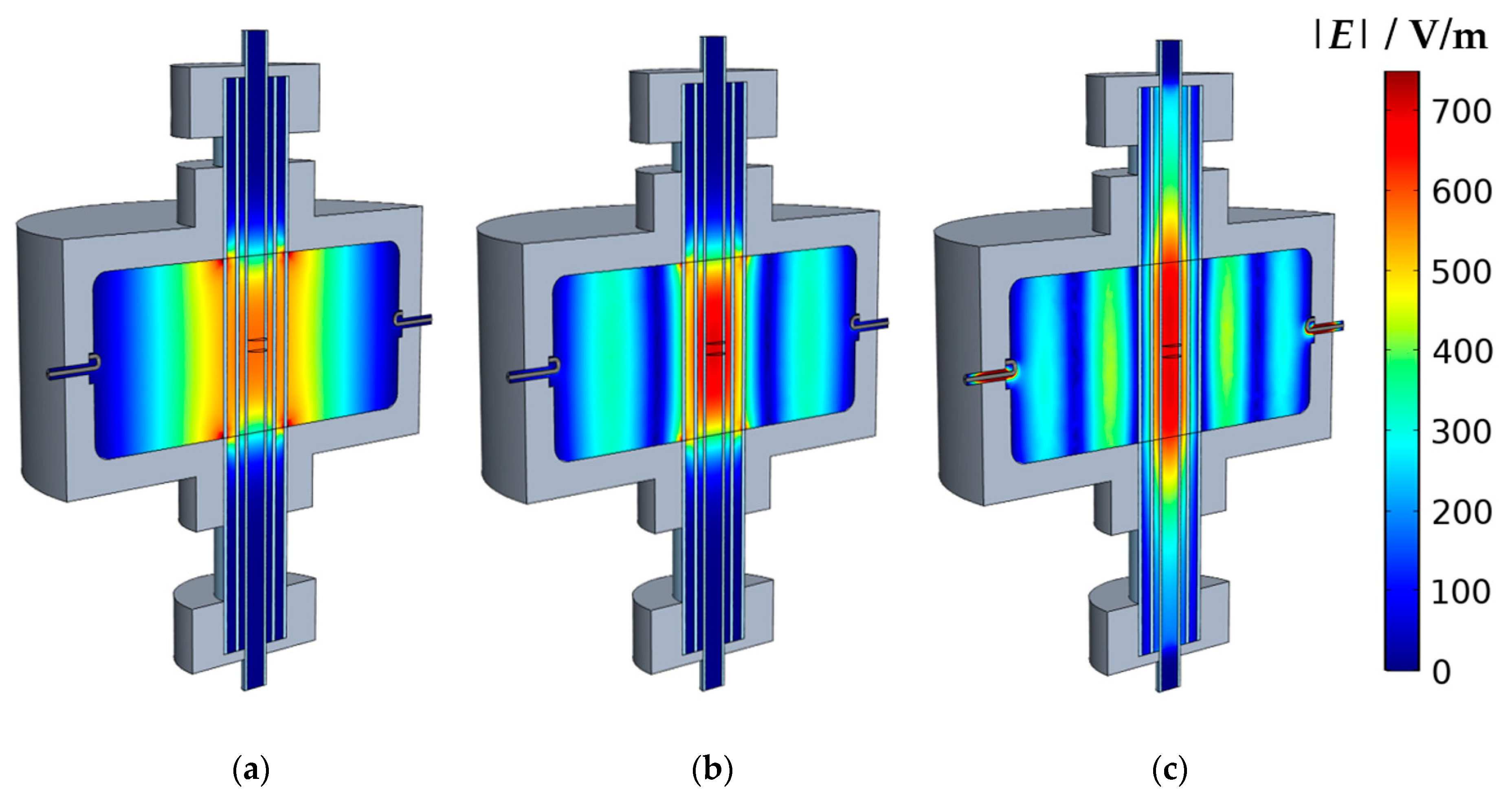
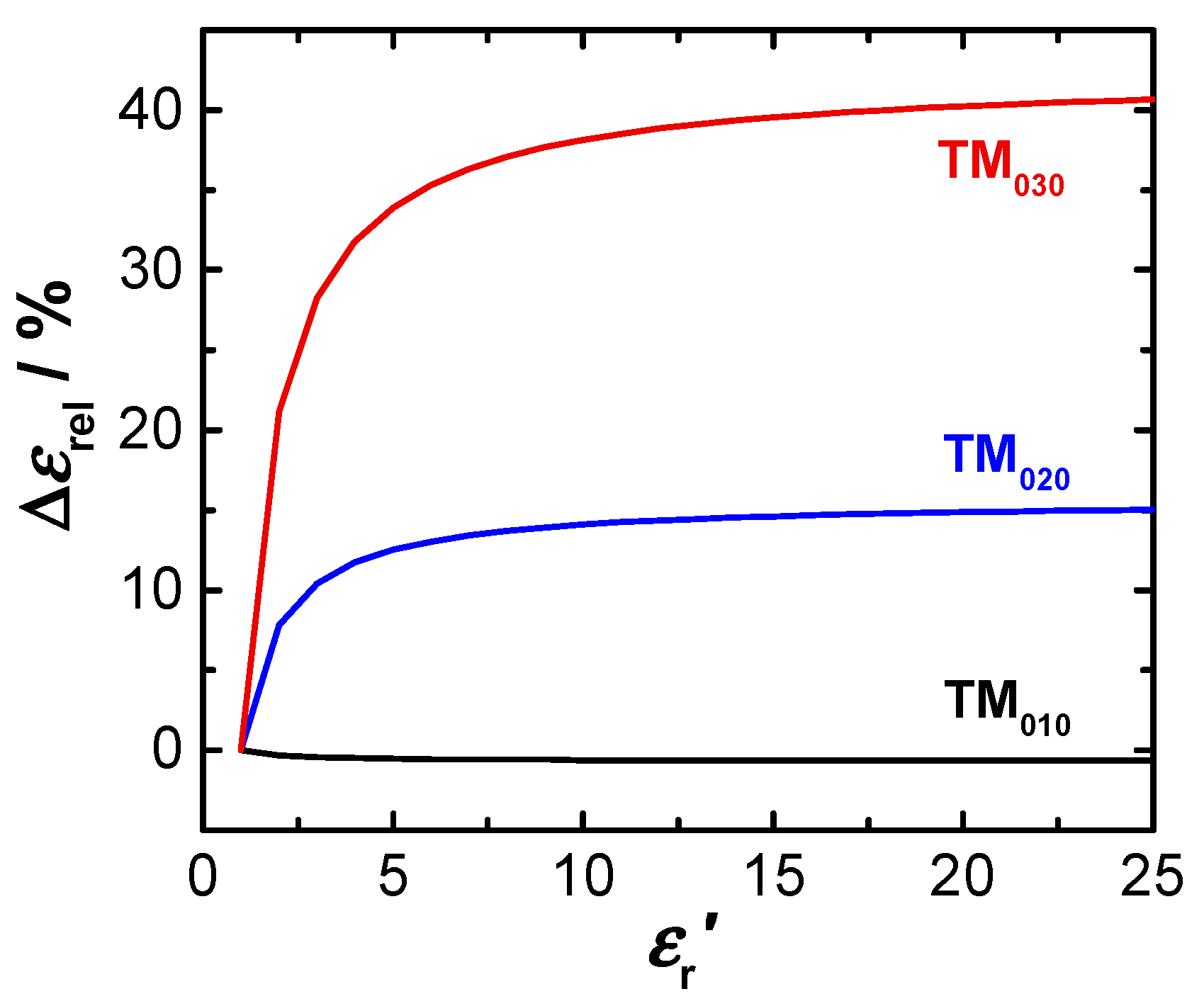
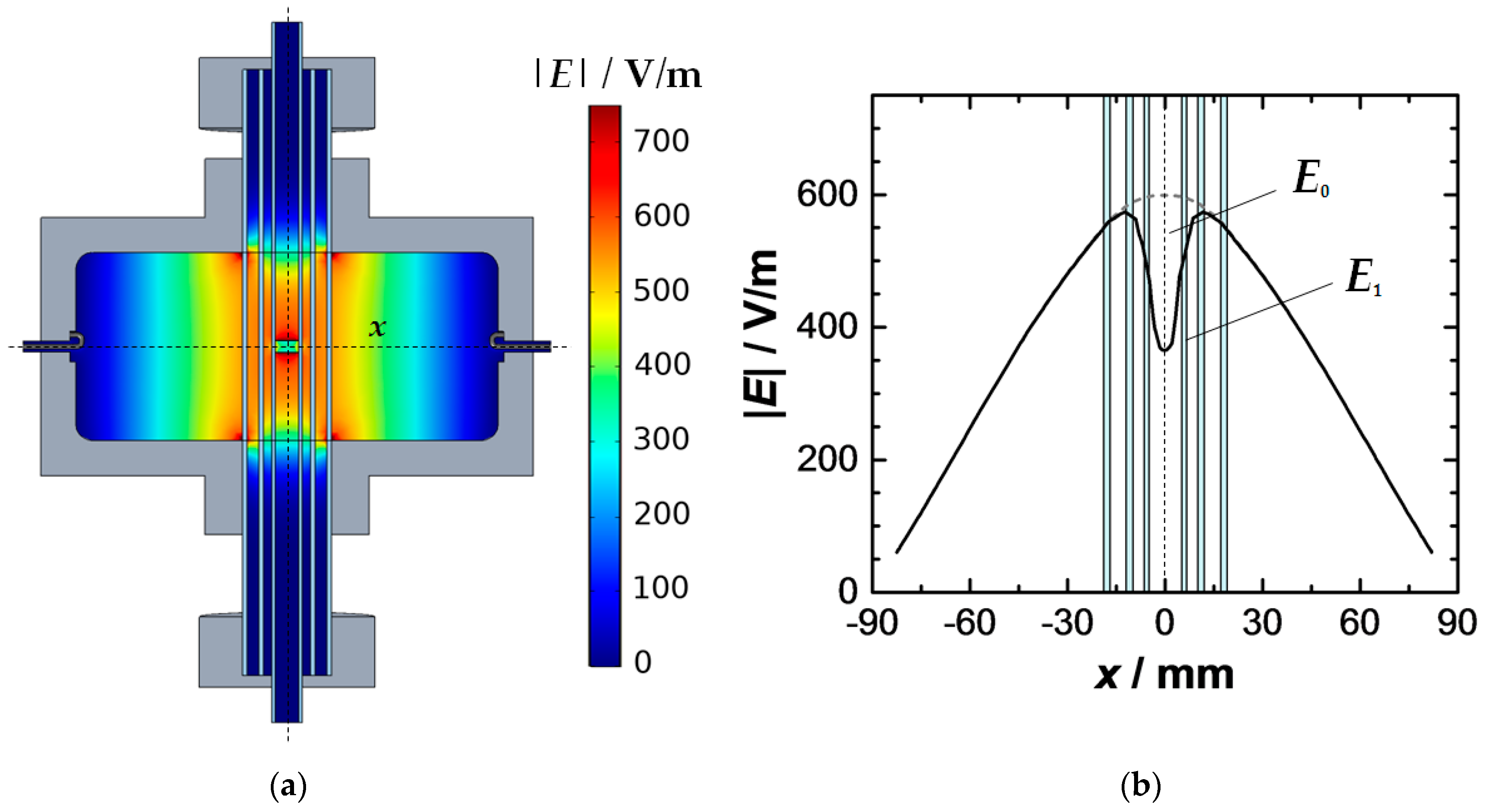
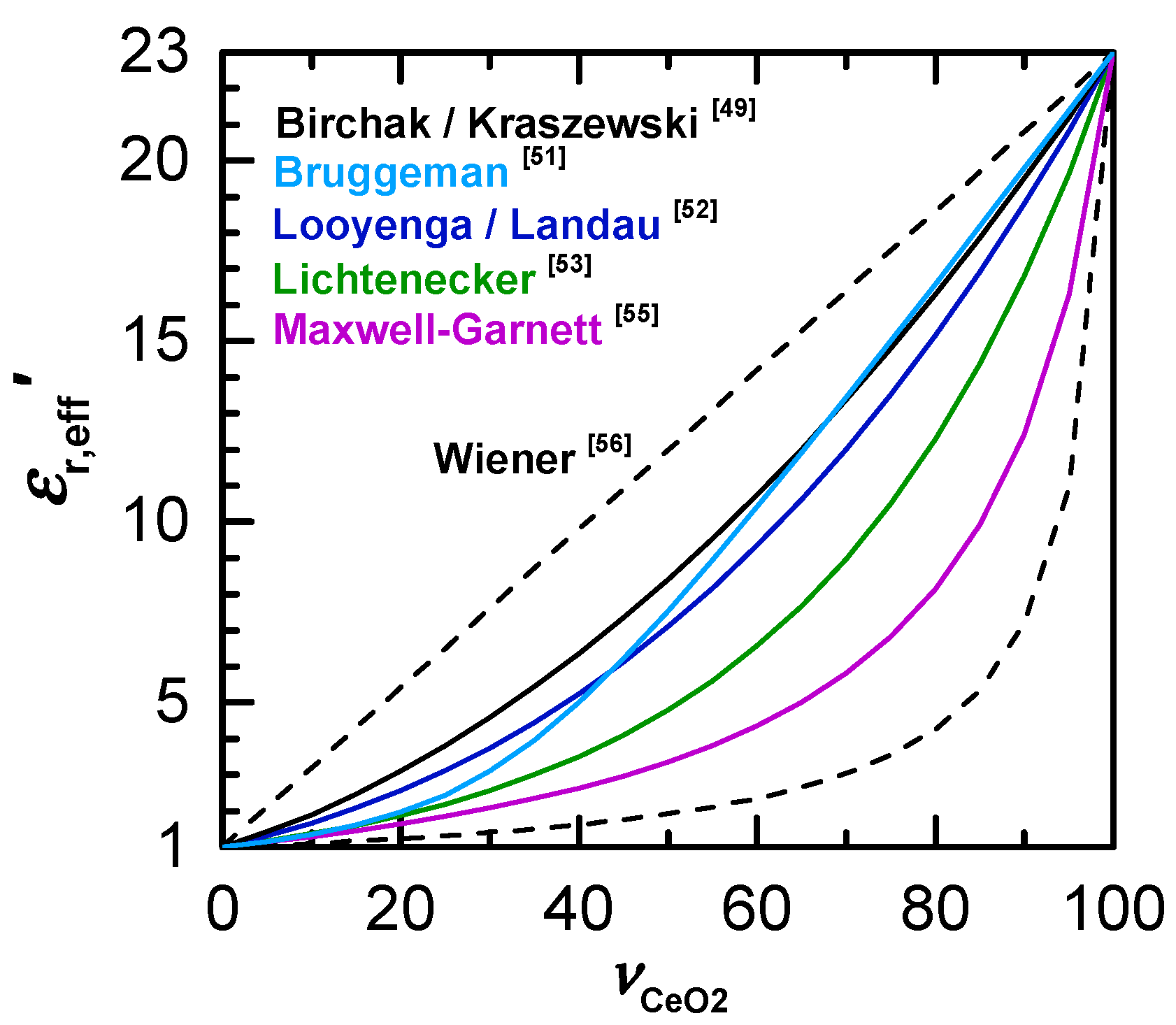
| Mode | TM010 | TM020 | TM030 |
|---|---|---|---|
| Veff/VC (simulation) | 26.78% | 13.73% | 12.84% |
| Veff, th/VC (simplified approach) | 26.95% | 11.58% | 7.37% |
| Mode | fs/GHz | f0/GHz | QS | Q0 | Ne | (Equation (14)) | (Equation (13)) |
|---|---|---|---|---|---|---|---|
| TM010 | 1.179563 | 1.179973 | 19,787 | 19,781 | 0.435 | 2.66 | 1.97 |
| TM020 | 2.624186 | 2.625898 | 30,183 | 30,818 | 0.435 | 2.56 | 1.93 |
| TM030 | 4.237119 | 4.240028 | 25,821 | 26,628 | 0.435 | 2.52 | 1.91 |
| fs/GHz | f0/GHz | (Looyenga) | (Birchak) | (Lichtenecker) | |
| 2.470882 | 2.478675 | 2.59 | 22.4 | 15.7 | 101 |
| (a) Conditions: 25 °C, pO2 = 0.21 bar | Literature:= 23 [44,45,46] | |||||||
| Mode | fs/GHz | f0/GHz | QS | Q0 | Ne | Veff/VC | σ/(S/cm) | |
| TM010 | 1.180624 | 1.180870 | 13,890 | 13,930 | 0.402 | 26.78% | 22.6 | -- |
| TM020 | 2.629937 | 2.631025 | 13015 | 12,756 | 0.402 | 13.73% | 23.6 | -- |
| TM030 | 4.200389 | 4.203252 | 893.34 | 898.65 | 0.402 | 12.84% | 23.8 | -- |
| For comparison: Calculation with simplified electric field calibration (Veff): | ||||||||
| TM010 | 1.180624 | 1.180870 | 13,890 | 13,930 | 0.402 | 26.95% | 23.0 | -- |
| TM020 | 2.629937 | 2.631025 | 13015 | 12,756 | 0.402 | 11.58% | 16.0 | -- |
| TM030 | 4.200389 | 4.203252 | 893.34 | 898.65 | 0.402 | 7.37% | 7.91 | -- |
| For comparison: Calculation without considering depolarization (Ne = 0): | ||||||||
| TM010 | 1.180624 | 1.180870 | 13,890 | 13930 | 0 | 26.78% | 11.2 | -- |
| TM020 | 2.629937 | 2.631025 | 13,015 | 12756 | 0 | 13.73% | 11.5 | -- |
| TM030 | 4.200389 | 4.203252 | 893.34 | 898.65 | 0 | 12.84% | 11.5 | -- |
| (b) Conditions: 600 °C, pO2 = 0.21 bar | Literature:= 23 [8,43,44,45,46], σ = 3.9 10−5 S/cm [36] | |||||||
| Mode | fs/GHz | f0/GHz | QS | Q0 | Ne | Veff/VC | σ/(S/cm) | |
| TM010 | 1.179082 | 1.179328 | 12,327 | 12826 | 0.402 | 26.78% | 22.7 | 1.79·10−5 |
| TM020 | 2.625354 | 2.626425 | 9572.3 | 10341 | 0.402 | 13.73% | 22.8 | 1.58·10−5 |
| TM030 | 4.192808 | 4.194524 | 943.23 | 1210.1 | 0.402 | 12.84% | <17.6> | -- |
| (c) Conditions: 600 °C, pO2 = 10−20 bar | Literature: σ = 2.0 10−2 S/cm [36] | |||||||
| Mode | fs/GHz | f0/GHz | QS | Q0 | Ne | Veff/VC | σ/(S/cm) | |
| TM010 | 1.178967 | 1.179341 | 3441.6 | 12,878 | 0.402 | 26.78% | 42.8 | 4.22 10−2 |
| TM020 | 2.625034 | 2.626473 | 2488.3 | 10,335 | 0.402 | 13.73% | 39.0 | 5.50 10−2 |
| TM030 | 4.192956 | 4.194998 | 806.22 | 1210.8 | 0.402 | 12.84% | 23.5 | 5.48 10−2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Steiner, C.; Walter, S.; Malashchuk, V.; Hagen, G.; Kogut, I.; Fritze, H.; Moos, R. Determination of the Dielectric Properties of Storage Materials for Exhaust Gas Aftertreatment Using the Microwave Cavity Perturbation Method. Sensors 2020, 20, 6024. https://doi.org/10.3390/s20216024
Steiner C, Walter S, Malashchuk V, Hagen G, Kogut I, Fritze H, Moos R. Determination of the Dielectric Properties of Storage Materials for Exhaust Gas Aftertreatment Using the Microwave Cavity Perturbation Method. Sensors. 2020; 20(21):6024. https://doi.org/10.3390/s20216024
Chicago/Turabian StyleSteiner, Carsten, Stefanie Walter, Vladimir Malashchuk, Gunter Hagen, Iurii Kogut, Holger Fritze, and Ralf Moos. 2020. "Determination of the Dielectric Properties of Storage Materials for Exhaust Gas Aftertreatment Using the Microwave Cavity Perturbation Method" Sensors 20, no. 21: 6024. https://doi.org/10.3390/s20216024
APA StyleSteiner, C., Walter, S., Malashchuk, V., Hagen, G., Kogut, I., Fritze, H., & Moos, R. (2020). Determination of the Dielectric Properties of Storage Materials for Exhaust Gas Aftertreatment Using the Microwave Cavity Perturbation Method. Sensors, 20(21), 6024. https://doi.org/10.3390/s20216024






