Optimization of Weighting Window Functions for SAR Imaging via QCQP Approach
Abstract
:1. Introduction
2. Optimization Model for Window Function
3. Optimized Window Function Design
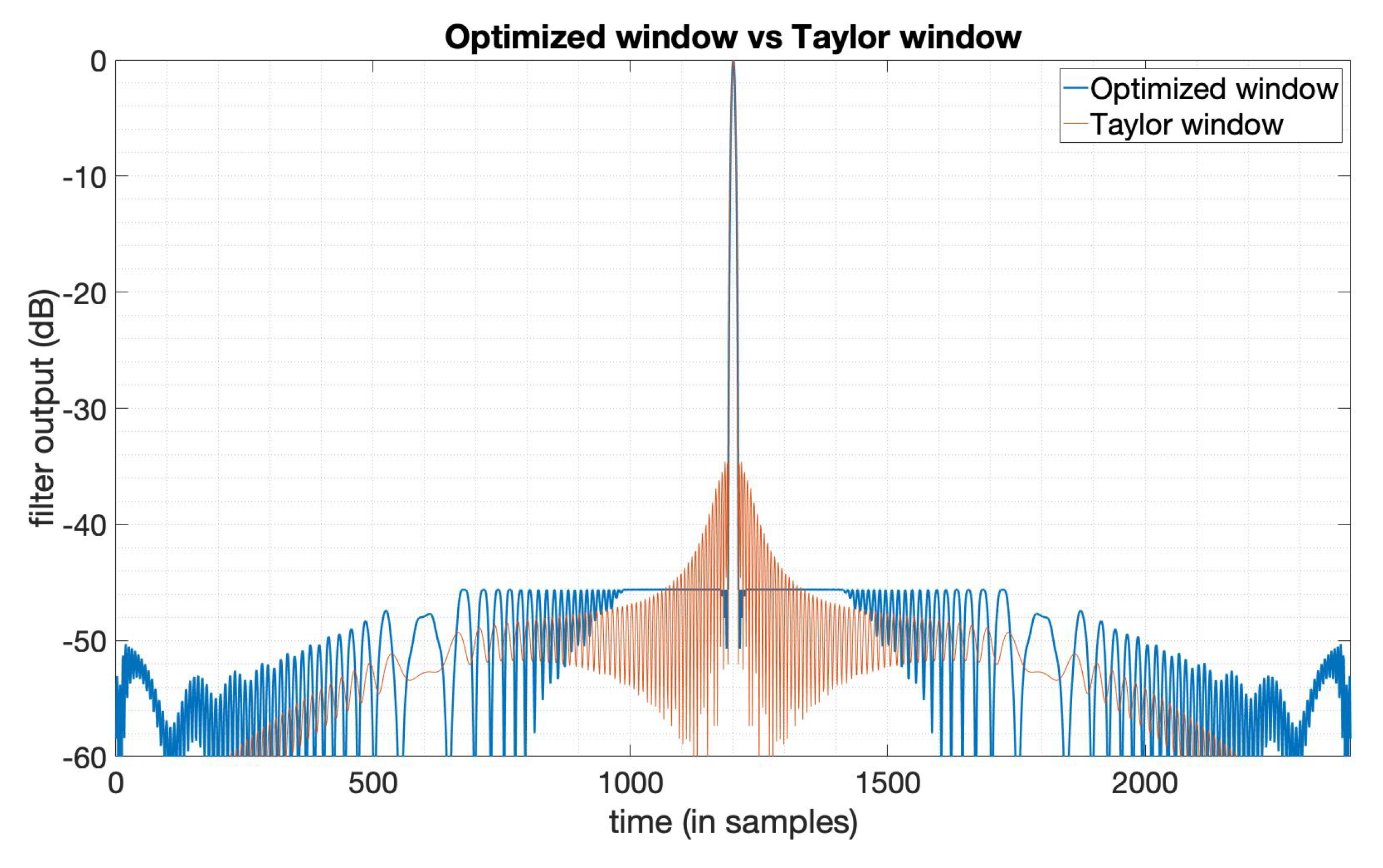
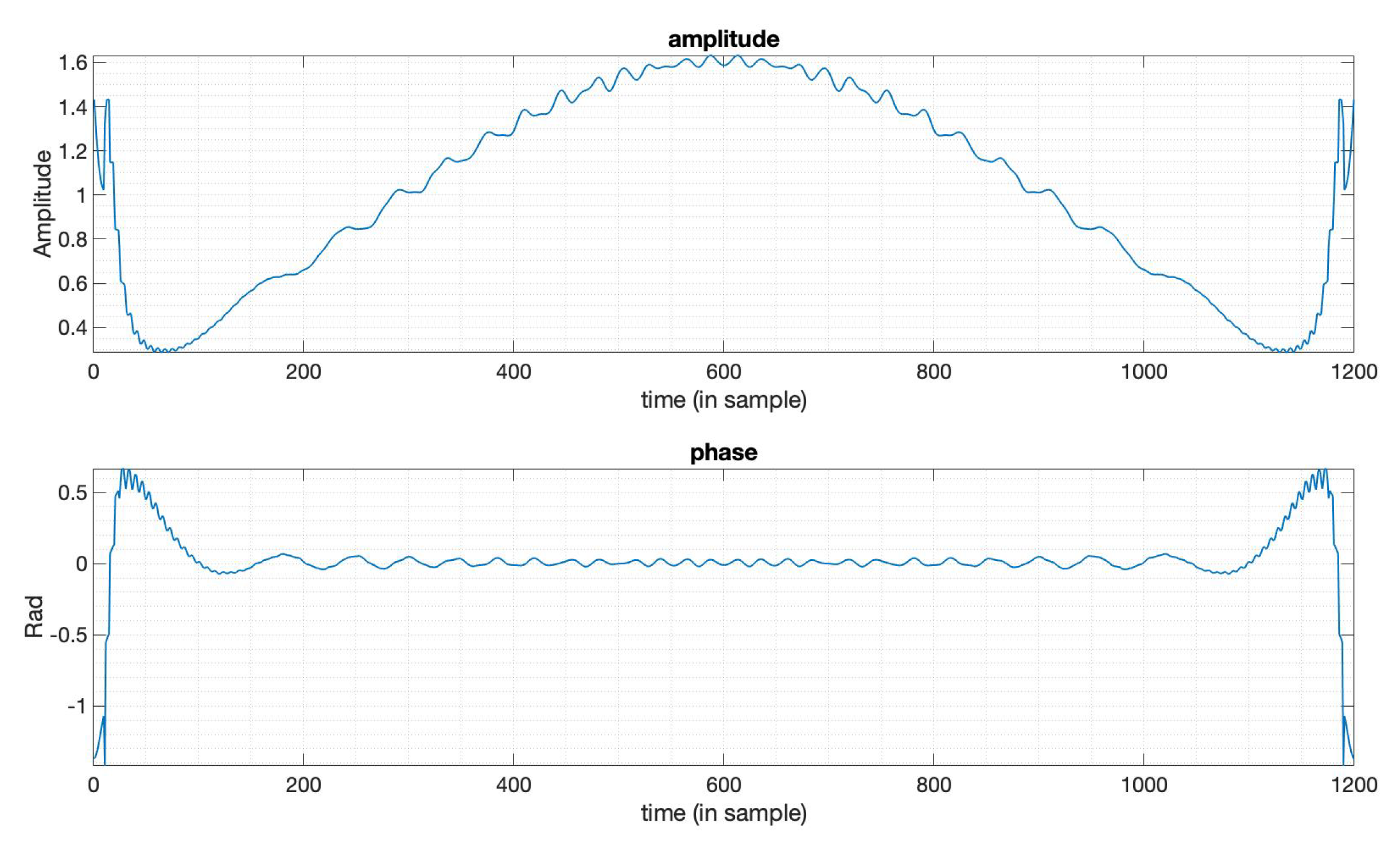
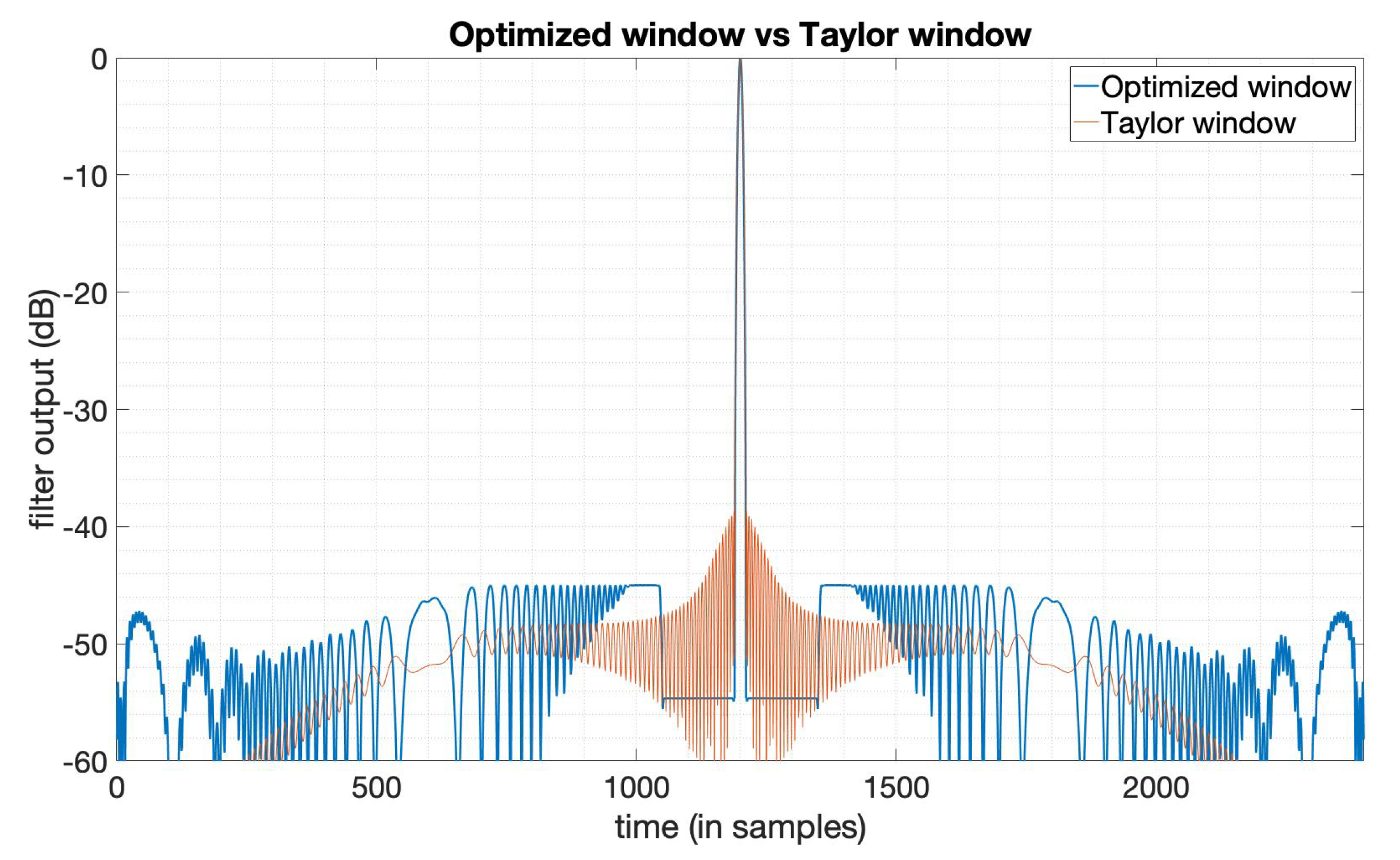
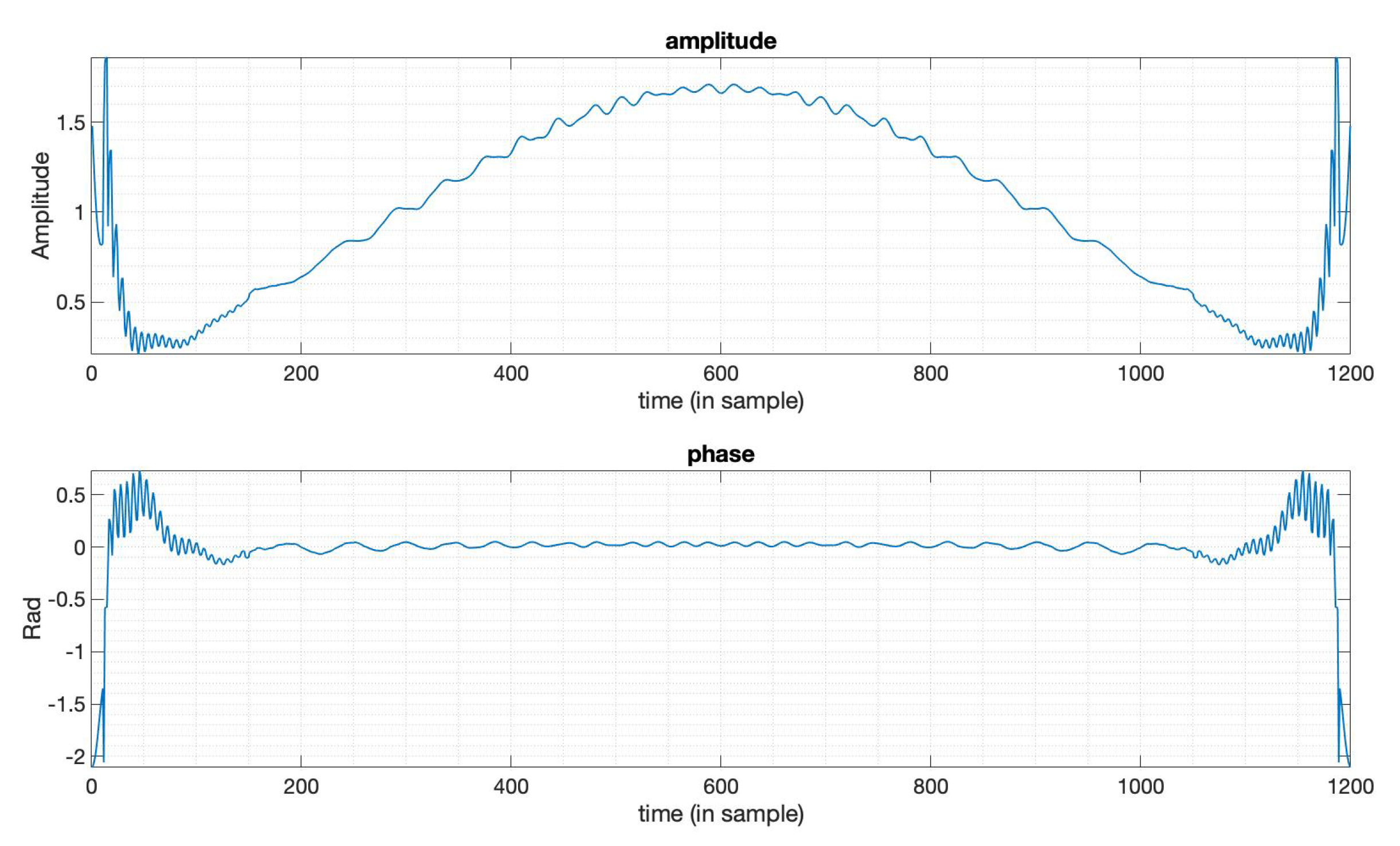
3.1. Window Function Design with Optimized PSLR
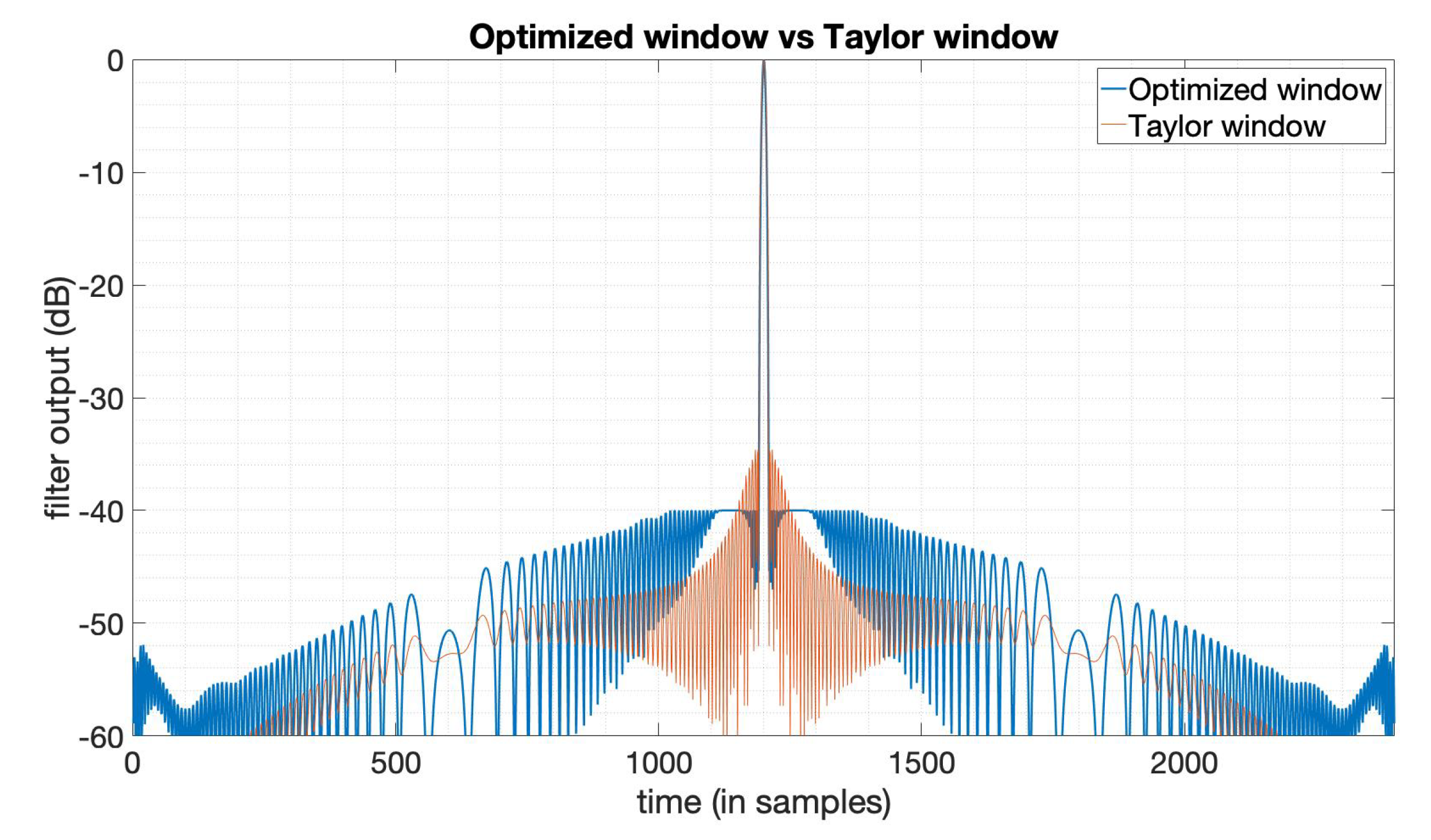
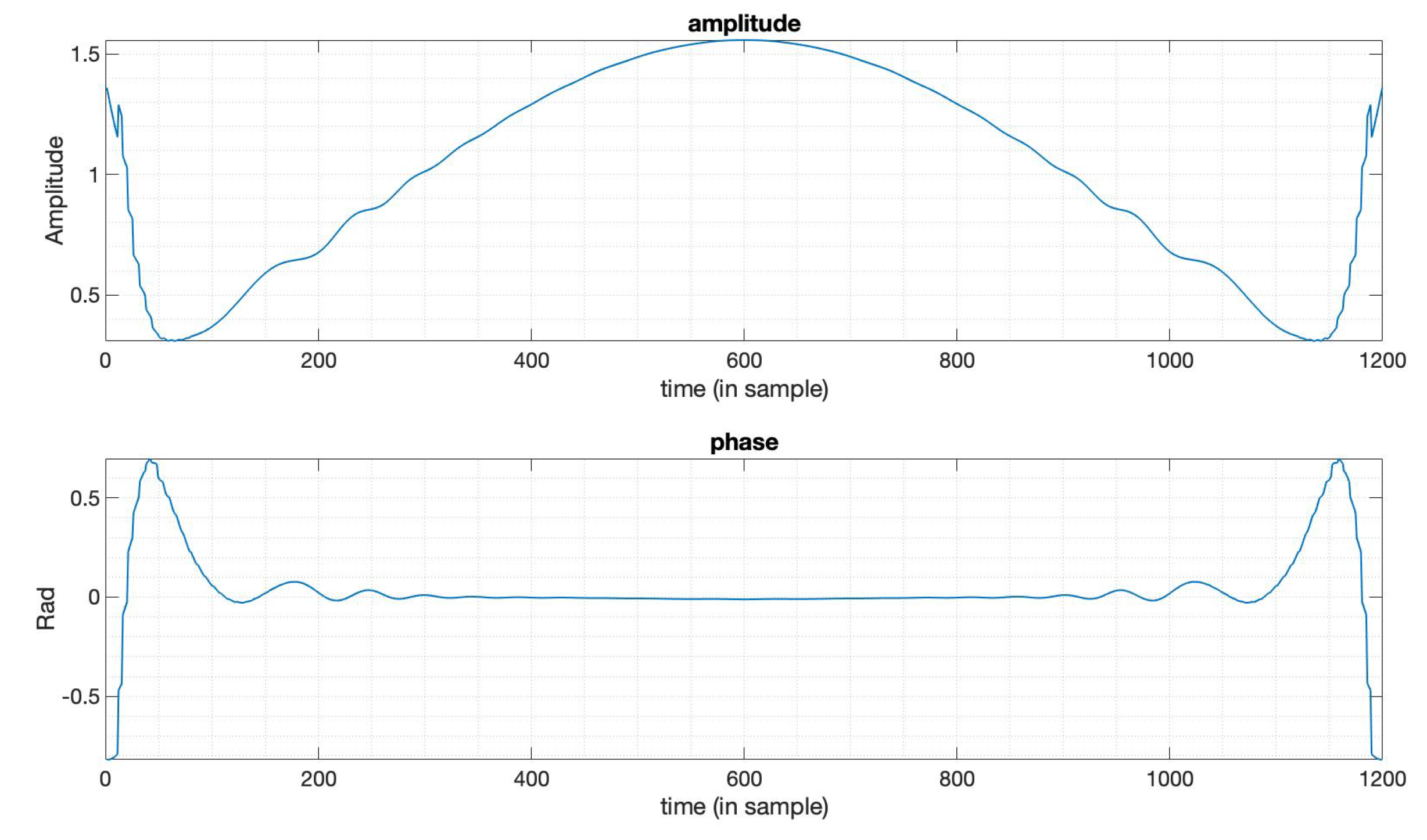
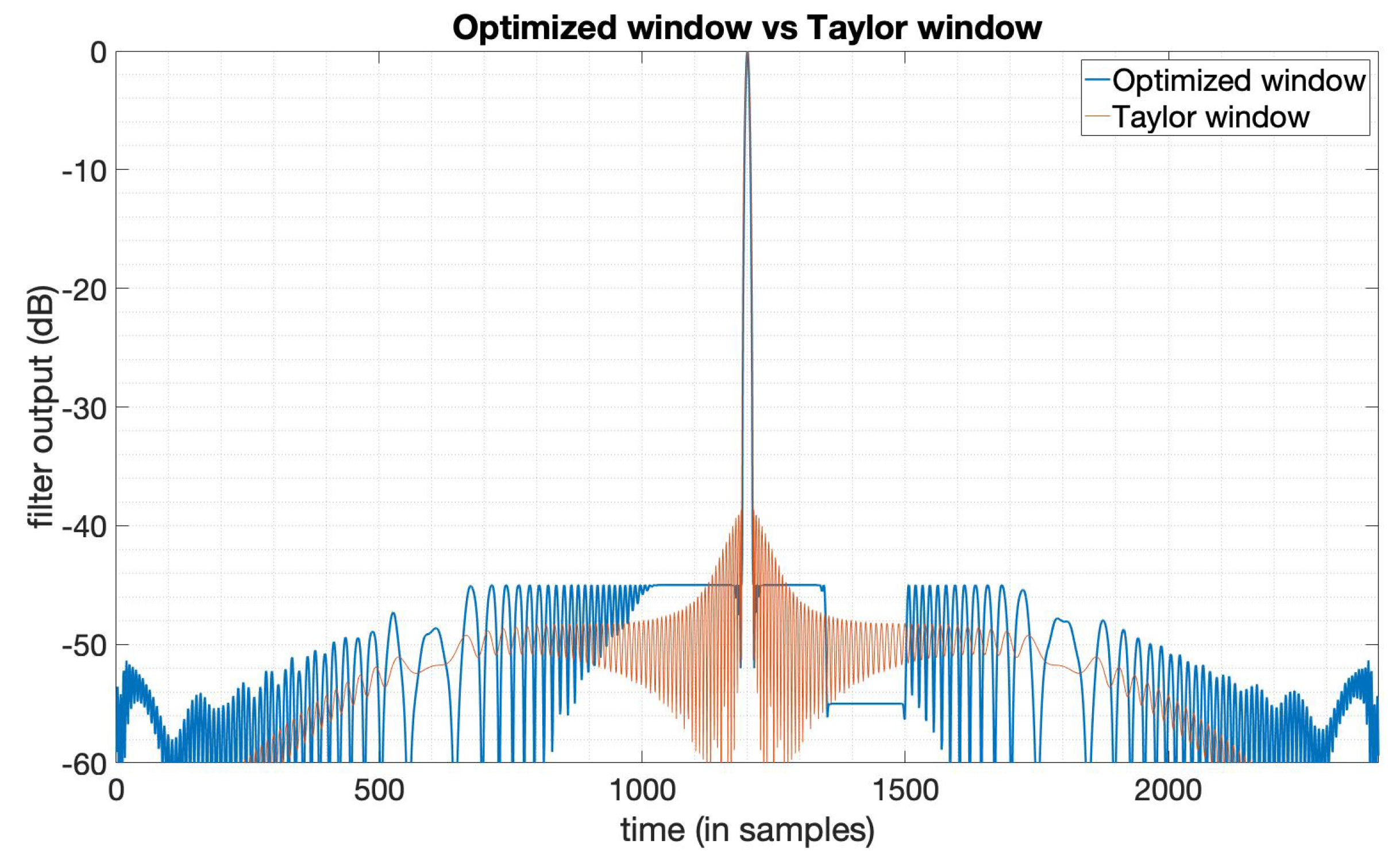
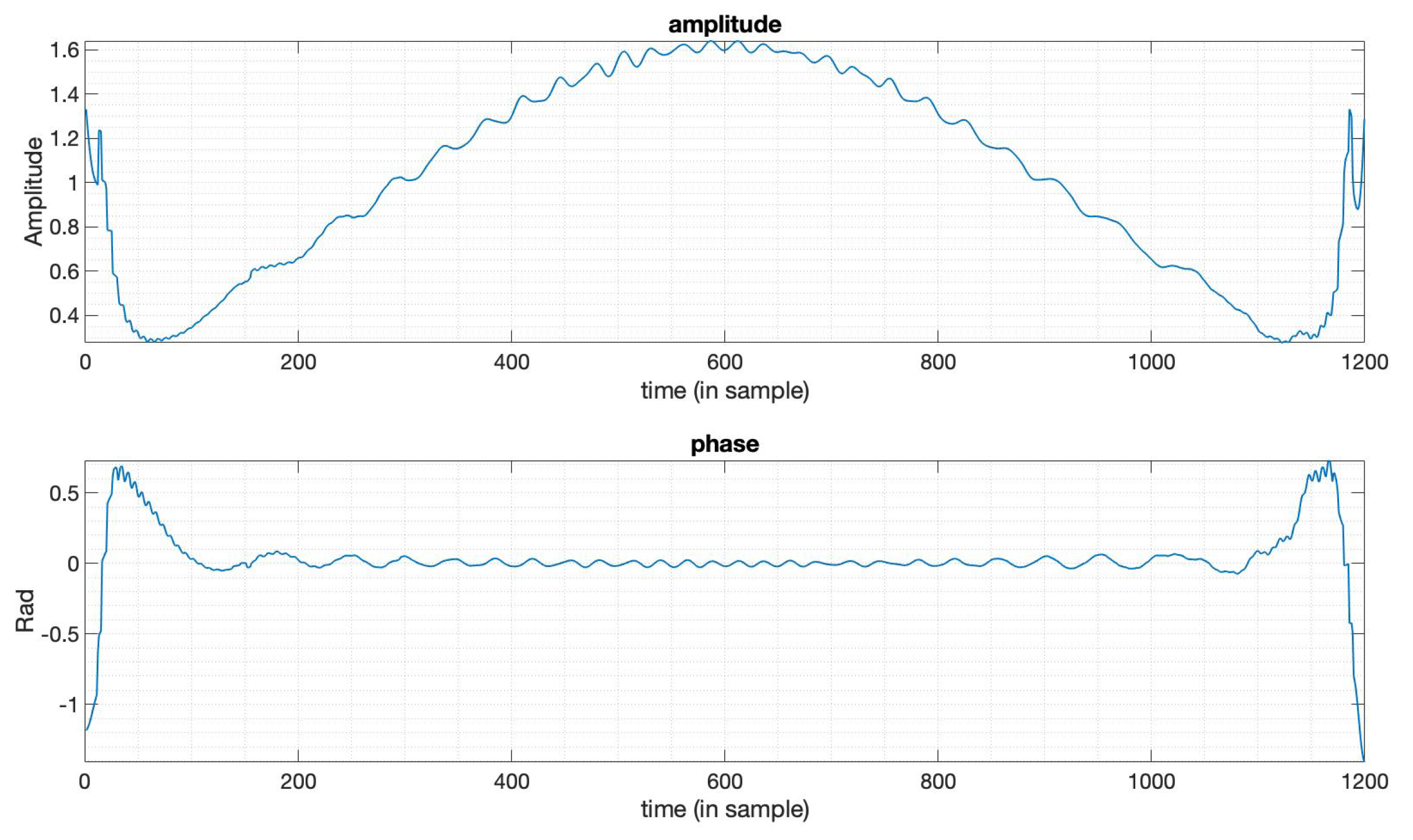
3.2. Window Function Design with Optimized SNR Loss
3.3. Optimized Window with Special Sidelobe Shape
4. SAR Point Target Imaging Simulation
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Henke, D.; Mccormick, P.; Blunt, S.D.; Higgins, T. Practical aspects of optimal mismatch filtering and adaptive pulse compression for FM waveforms. In Proceedings of the IEEE National Radar Conference, Arlington, VA, USA, 10–15 May 2015; pp. 1149–1155. [Google Scholar]
- Kay, S.M. Fundamentals of Statistical Signal Processing; Prentice Hall PTR: Upper Saddle River, NJ, USA, 1993; pp. 94–140. [Google Scholar]
- Harris, F.J. On the use of windows for harmonic analysis with the discrete Fourier transform. Proc. IEEE 1978, 66, 51–83. [Google Scholar] [CrossRef]
- Levanon, N.; Mozeson, E. Radar Signals; John Wiley & Sons: Hoboken, NJ, USA, 2004; pp. 100–167. [Google Scholar]
- Doerry, A.W. Catalog of Window Taper Functions for Sidelobe Control; Sandia National Laboratories: Albuquerque, NM, USA, 2017. [Google Scholar]
- Kajenski, P.J. Mismatch filter design via convex optimization. IEEE Trans. Aerosp. Electron. Syst. 2016, 52, 1587–1591. [Google Scholar] [CrossRef]
- Davis, M.; Lanterman, A. Minimum integrated sidelobe ratio filters for mimo radar. IEEE Trans. Aerosp. Electron. Syst. 2015, 51, 405–416. [Google Scholar] [CrossRef]
- Censor, Y. Pareto optimality in multiobjective problems. Appl. Math. Optim. 1977, 4, 41–59. [Google Scholar] [CrossRef]
- Knapp, T. An application of nonlinear programming to mismatched filters. IEEE Trans. Circuit Theory 1965, 12, 185–193. [Google Scholar] [CrossRef]
- Ackroyd, M.H.; Ghani, F. Optimum mismatched filters for sidelobe suppression. IEEE Trans. Aerosp. Electron. Syst. 1973, AES-9, 214–218. [Google Scholar] [CrossRef]
- Zoraster, S. Minimum peak range sidelobe filters for binary phase-coded waveforms. IEEE Trans. Aerosp. Electron. Syst. 1980, AES-16, 112–115. [Google Scholar] [CrossRef]
- Cohen, M.N.; Fox, M.R.; Baden, J.M. Minimum peak sidelobe pulse compression codes. In Proceedings of the IEEE International Conference on Radar, Arlington, VA, USA, 7–10 May 1990; pp. 633–638. [Google Scholar]
- Griep, K.R.; Ritcey, J.A.; Burlingame, J.J. Poly-phase codes and optimal filters for multiple user ranging. IEEE Trans. Aerosp. Electron. Syst. 1995, 31, 752–767. [Google Scholar] [CrossRef]
- Blunt, S.D.; Gerlach, K. Adaptive pulse compression via mmse estimation. IEEE Trans. Aerosp. Electron. Syst. 2006, 42, 572–584. [Google Scholar] [CrossRef]
- Cilliers, J.E.; Smit, J.C. Pulse Compression Sidelobe Reduction by Minimization of Lp-Norms. IEEE Trans. Aerosp. Electron. Syst. 2007, 43, 1238–1247. [Google Scholar] [CrossRef]
- Sarkar, I.; Adly, T. Multiplicative mismatched filters for sidelobe suppression in barker codes. IEEE Trans. Aerosp. Electron. Syst. 2008, 44, 349–359. [Google Scholar] [CrossRef]
- Levanon, N.; Scharf, A. Range sidelobes blanking using contrasting mismatched filters. In Proceedings of the International Conference on Digital Signal Processing, Santorini-Hellas, Greece, 5–7 July 2009. [Google Scholar]
- Hu, L.; Liu, H.; Feng, D.Z.; Jiu, B.; Wang, X.; Wu, S. Optimal mismatched filter bank design for MIMO radar via convex optimization. In Proceedings of the Waveform Diversity and Design Conference, Niagara Falls, ON, Canada, 8–13 August 2010. [Google Scholar]
- De Maio, A.; Huang, Y.; Piezzo, M.; Zhang, S.; Farina, A. Design of radar receive filters optimized according to Lp-norm based criteria. IEEE Trans. Signal Process. 2011, 59, 4023–4029. [Google Scholar] [CrossRef]
- Rabaste, O.; Savy, L. Mismatched filter optimization via quadratic convex programming for radar applications. In Proceedings of the 2014 International Radar Conference, Lille, France, 13–17 October 2014; pp. 1–6. [Google Scholar]
- Rabaste, O.; Savy, L. Mismatched filter optimization for radar applications using quadratically constrained quadratic programs. IEEE Trans. Aerosp. Electron. Syst. 2015, 51, 3107–3122. [Google Scholar] [CrossRef]
- Aittomaki, T.; Koivunen, V. Mismatched filter design for radar waveforms by semidefinite relaxation. In Proceedings of the 2015 IEEE International Conference on Acoustics, Speech and Signal Processing, Brisbane, QLD, Australia, 19–24 April 2015. [Google Scholar]
- Aittomaki, T.; Koivunen, V. Mismatched filter design and interference mitigation for mimo radars. IEEE Trans. Signal Process. 2017, 65, 454–466. [Google Scholar] [CrossRef]
- Xu, L.; Zhou, S.; Liu, H. Simultaneous optimization of radar waveform and mismatched filter with range and delay-Doppler sidelobes suppression. Digit. Signal Process. 2018, 83, 346–358. [Google Scholar] [CrossRef]
- Xie, R.; Qiao, S.; Qiu, W.; Wang, B.; Rui, Y.; Li, P.; Guo, S. Design of ultra-low sidelobe pulse compression filter for LFM signal. In International Conference on Signal Processing Systems; International Society for Optics and Photonics: Bellingham, WA, USA, 2019; Volume 11071, p. 1107105. [Google Scholar]
- Sun, Y.; Liu, Q.; Cai, J.; Long, T. A Novel Weighted Mismatched Filter for Reducing Range Sidelobes. IEEE Trans. Aerosp. Electron. Syst. 2019, 55, 1450–1460. [Google Scholar] [CrossRef]
- Blunt, S.D.; Mokole, E.L. Overview of radar waveform diversity. IEEE Aerosp. Electron. Syst. Mag. 2016, 31, 2–42. [Google Scholar] [CrossRef]
- Boyd, S.; Vandenberghe, L. Convex Optimization; Cambridge University Press: Cambridge, UK, 2004; pp. 561–630. [Google Scholar]
- Grant, M.C.; Boyd, S.P. Graph implementations for nonsmooth convex programs. In Recent Advances in Learning and Control (a Tribute to M. Vidyasagar); Blondel, V., Boyd, S., Kimura, H., Eds.; Lecture Notes in Control and Information Sciences; Springer: London, UK, 2008; pp. 95–110. Available online: https://web.stanford.edu/~boyd/papers/pdf/graph_dcp.pdf (accessed on 11 January 2020).
- Cumming, I.G.; Wong, F.H. Digital Processing Synthetic Aperture Radar Data; Artech House: Norwood, MA, USA, 2005; pp. 69–79. [Google Scholar]
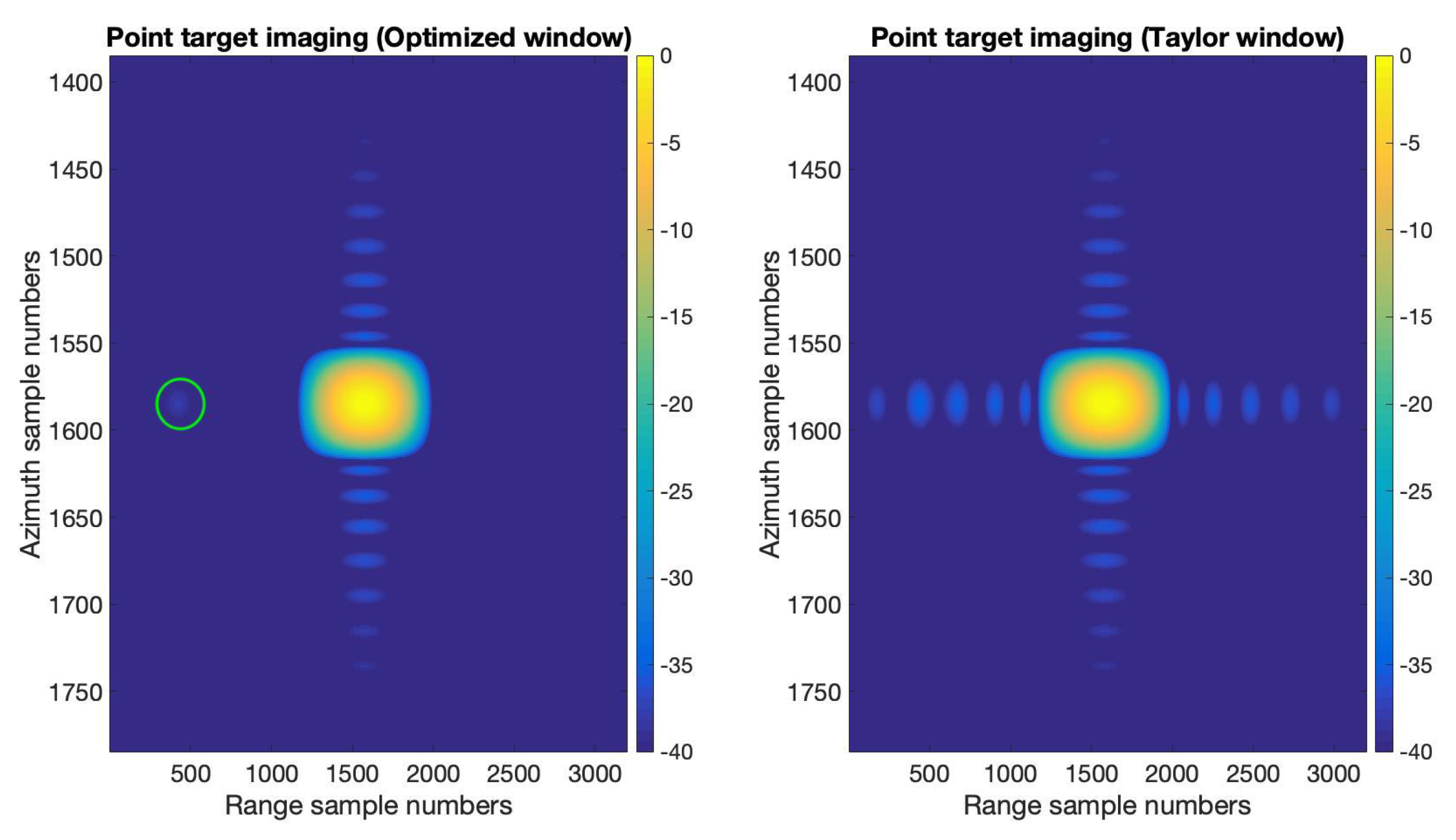
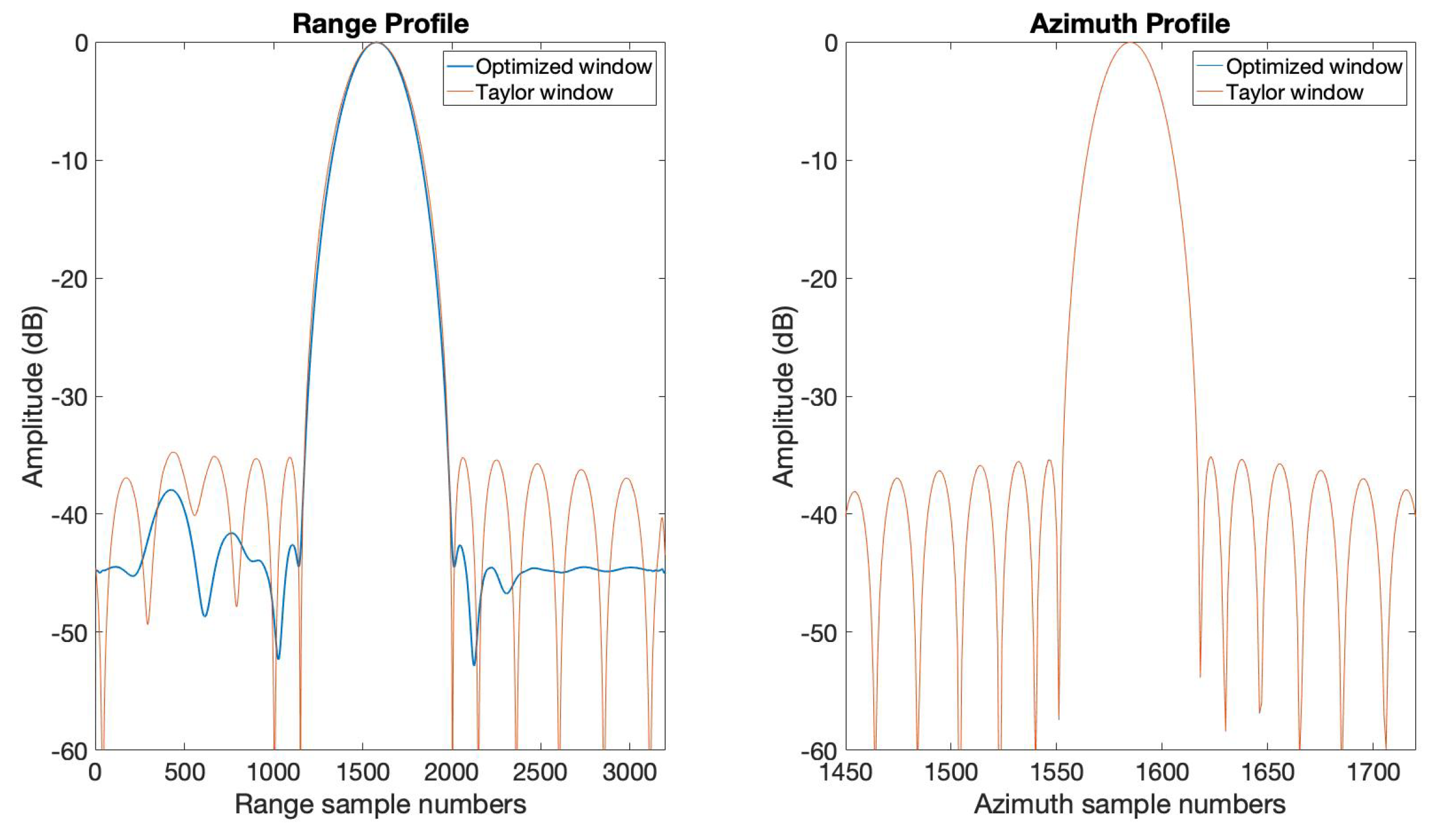
| Taylor Window | Optimized Window | |
|---|---|---|
| PSLR | −34.60 dB | −45.62 dB |
| SNR Loss | −0.92 dB | −0.92 dB |
| Mainlobe width | 1 | 95.18% |
| Taylor Window | Optimized Window | |
|---|---|---|
| SNR Loss | −0.92 dB | −0.78 dB |
| PSLR | −34.60 dB | −40.00 dB |
| Mainlobe width | 1 | 92.77% |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, J.; Wang, W.; Song, H. Optimization of Weighting Window Functions for SAR Imaging via QCQP Approach. Sensors 2020, 20, 419. https://doi.org/10.3390/s20020419
Liu J, Wang W, Song H. Optimization of Weighting Window Functions for SAR Imaging via QCQP Approach. Sensors. 2020; 20(2):419. https://doi.org/10.3390/s20020419
Chicago/Turabian StyleLiu, Jin, Wei Wang, and Hongjun Song. 2020. "Optimization of Weighting Window Functions for SAR Imaging via QCQP Approach" Sensors 20, no. 2: 419. https://doi.org/10.3390/s20020419
APA StyleLiu, J., Wang, W., & Song, H. (2020). Optimization of Weighting Window Functions for SAR Imaging via QCQP Approach. Sensors, 20(2), 419. https://doi.org/10.3390/s20020419





