Study of the Influence of Phase Noise on the MEMS Disk Resonator Gyroscope Interface Circuit
Abstract
1. Introduction
2. Working Principle of the MEMS DRG
2.1. Two-Dimensional (2-D) Coriolis Vibratory Gyroscope Model
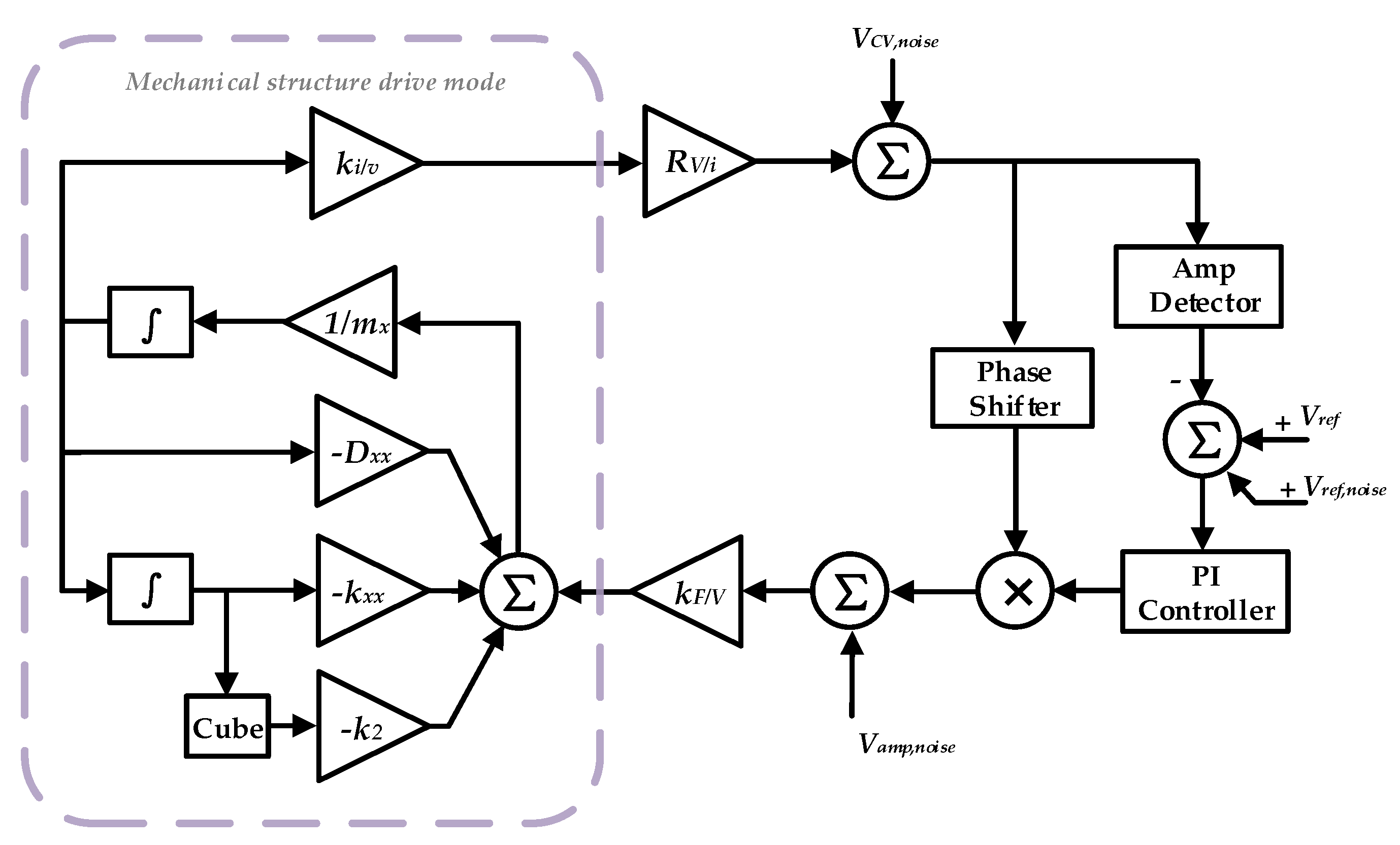
2.2. The Working Principle of Drive Loop
3. Phase Noise in Gyroscope System
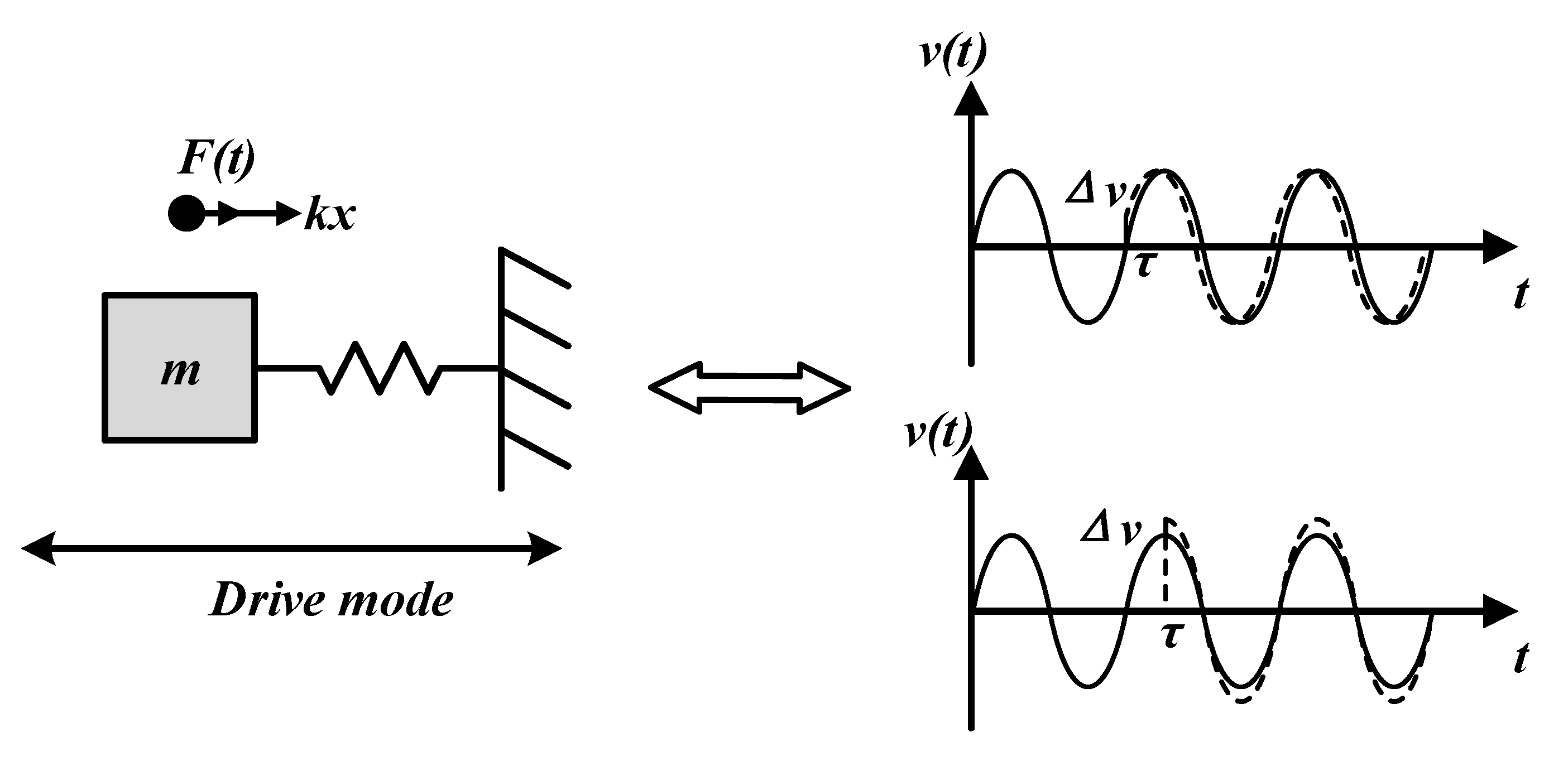
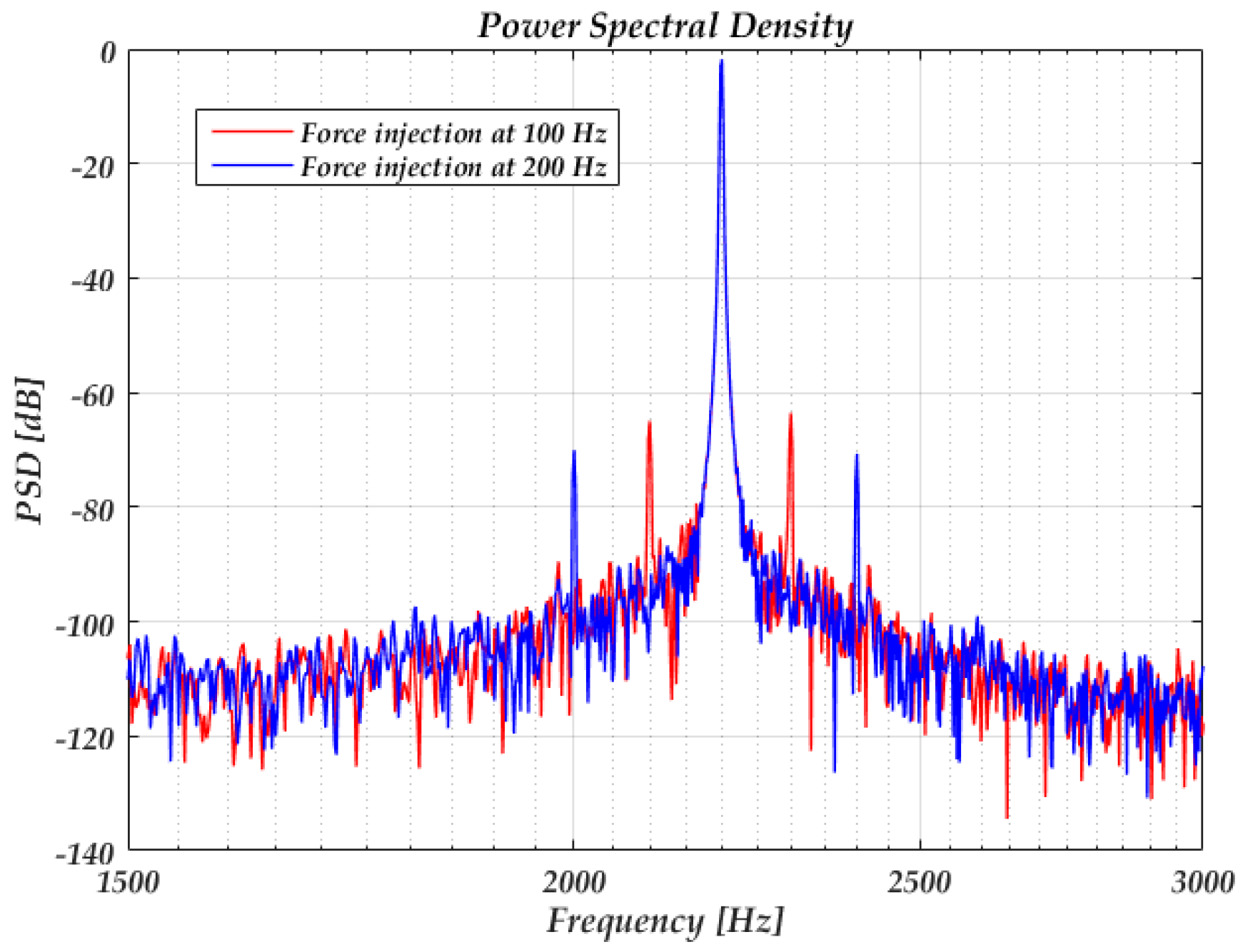
3.1. Force Noise Act on the Mass
3.2. The Established Phase Noise Model in Gyroscope System
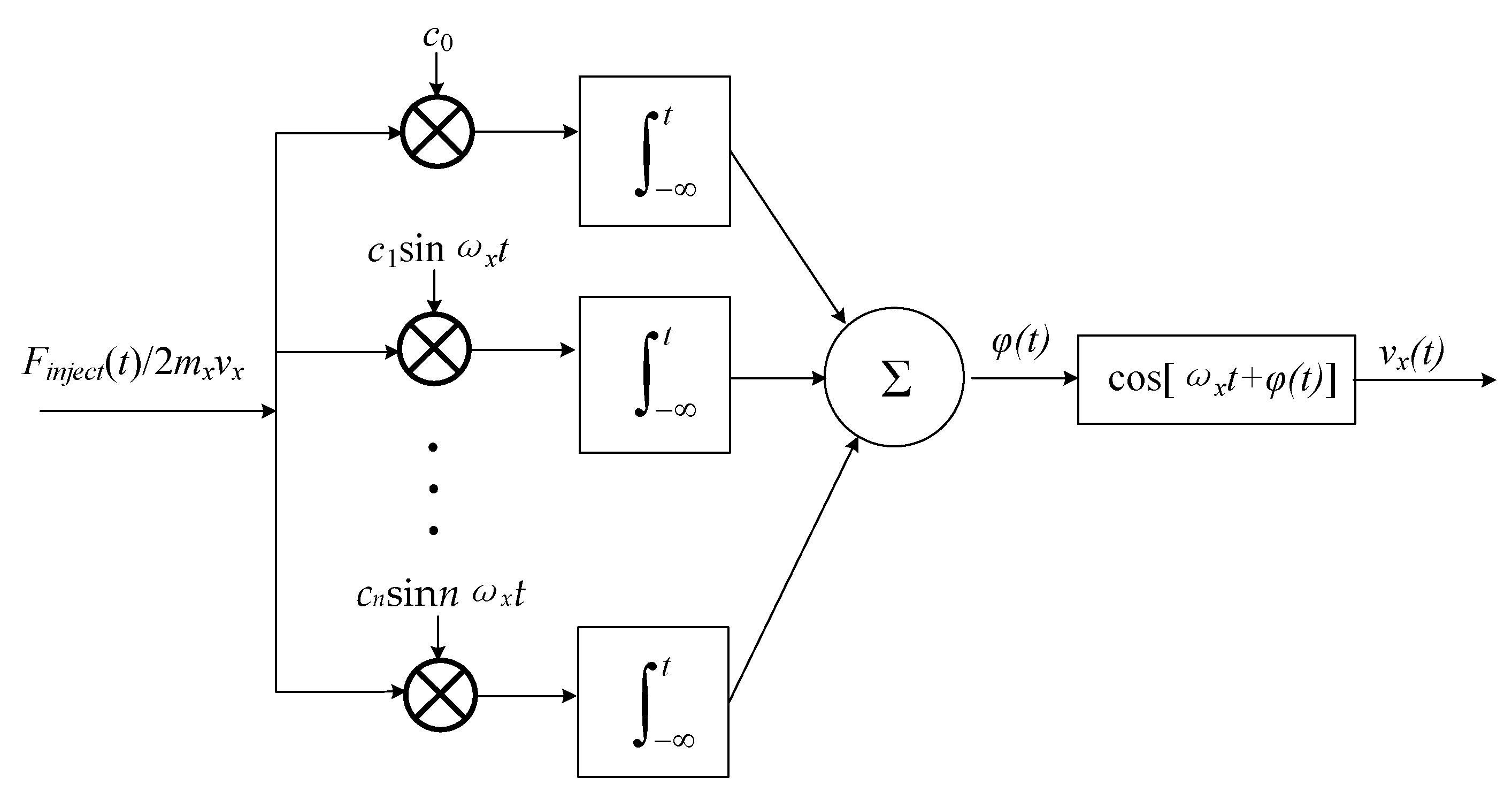
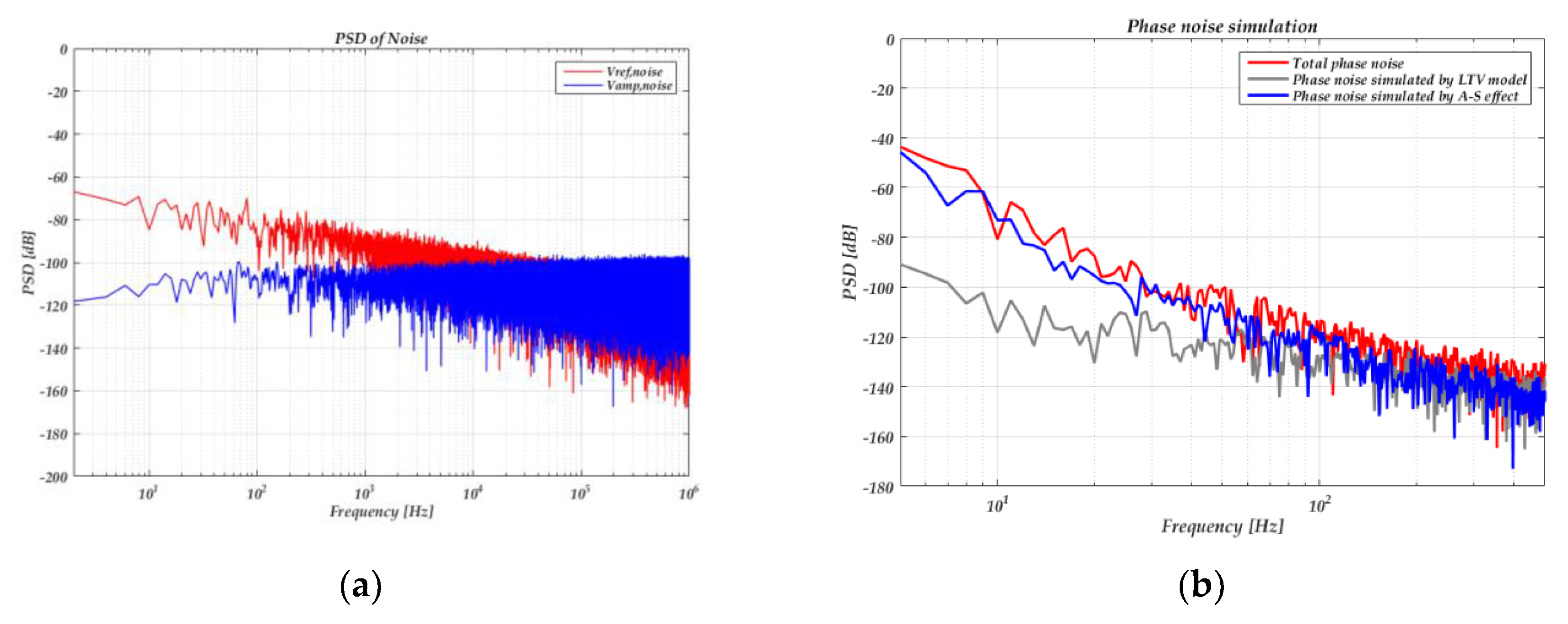
3.2.1. Phase Noise Caused by Injected Force Noise
3.2.2. Phase Noise Caused by A-S Effect
3.2.3. Total Phase Noise
4. The Influence of Time-Varying Phase Noise on the MEMS DRG System
4.1. y-Directional Displacement
4.2. Synchronous Demodulation
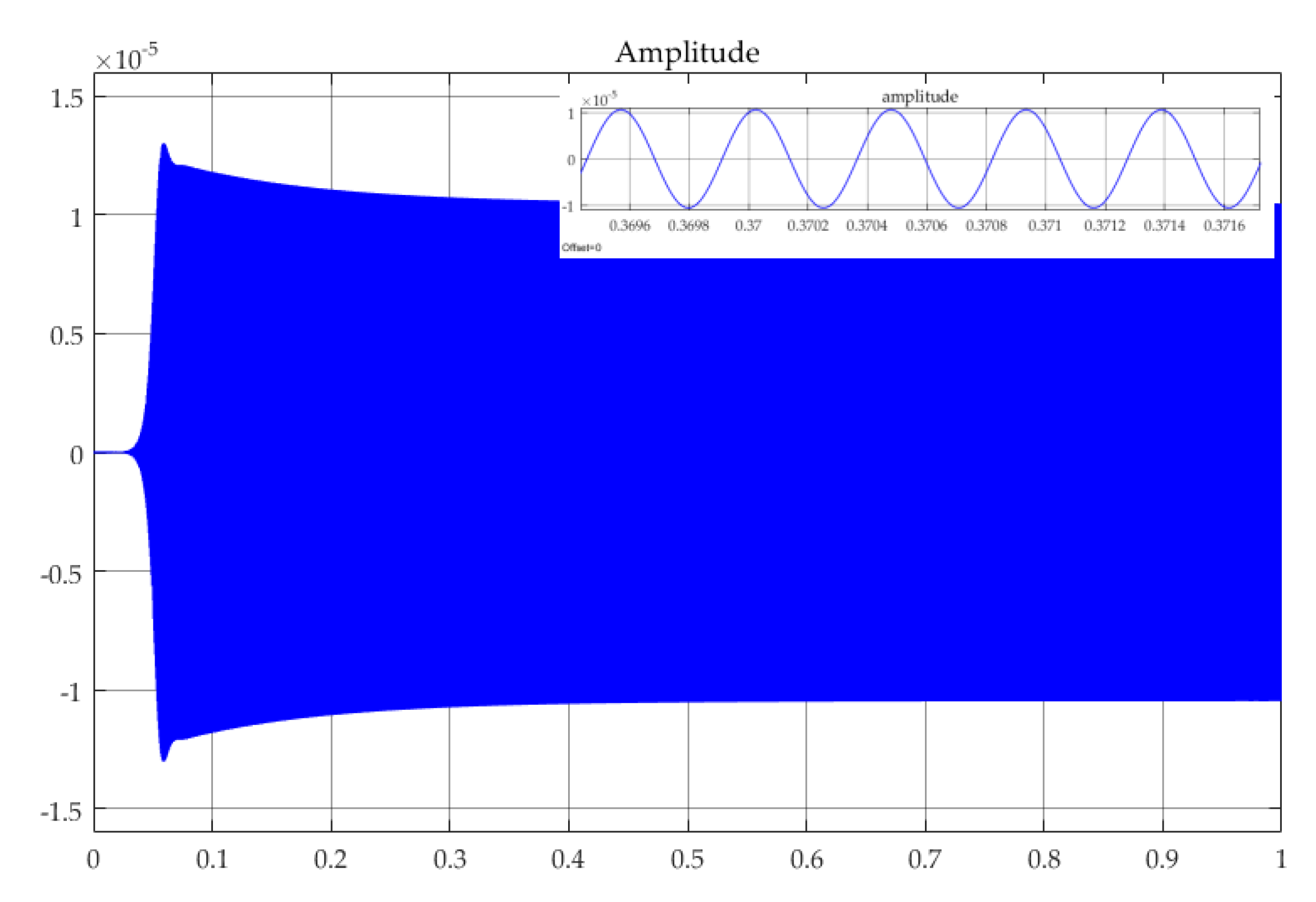
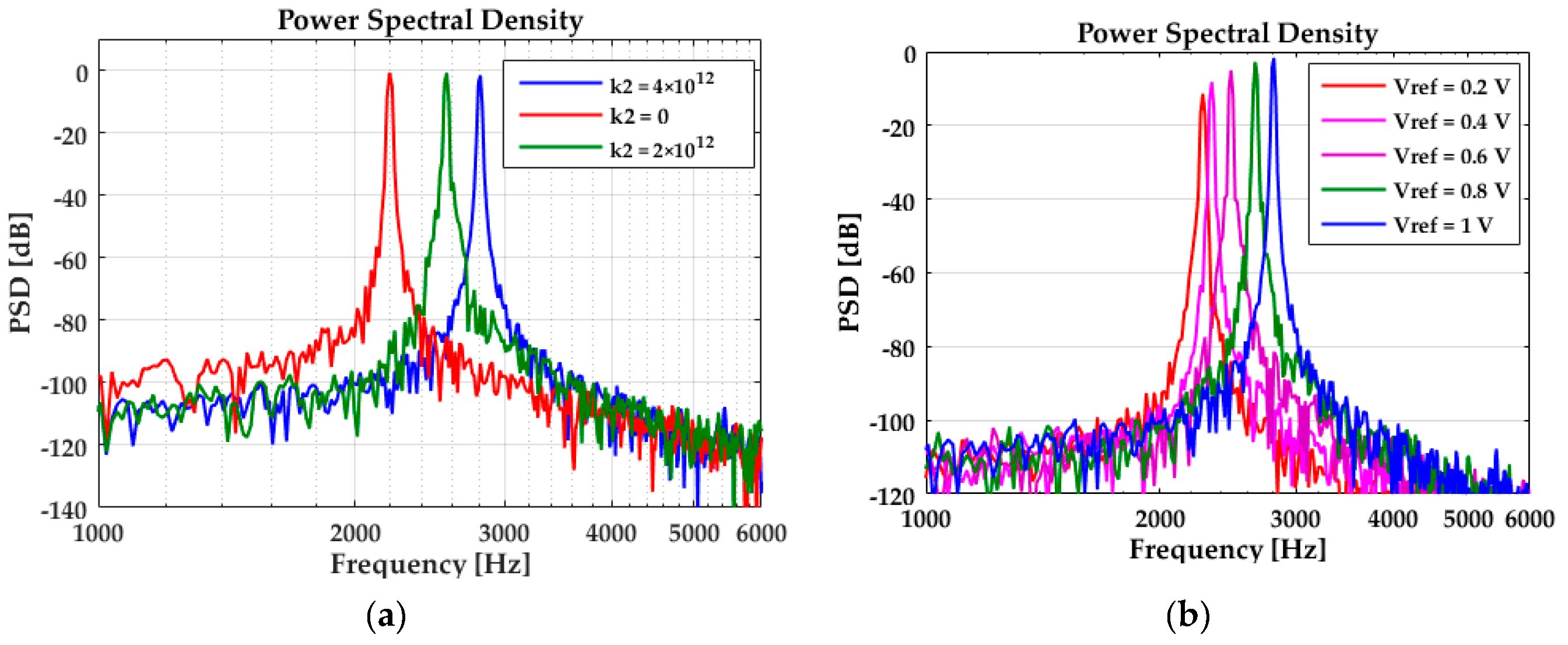
5. Numerical Simulations
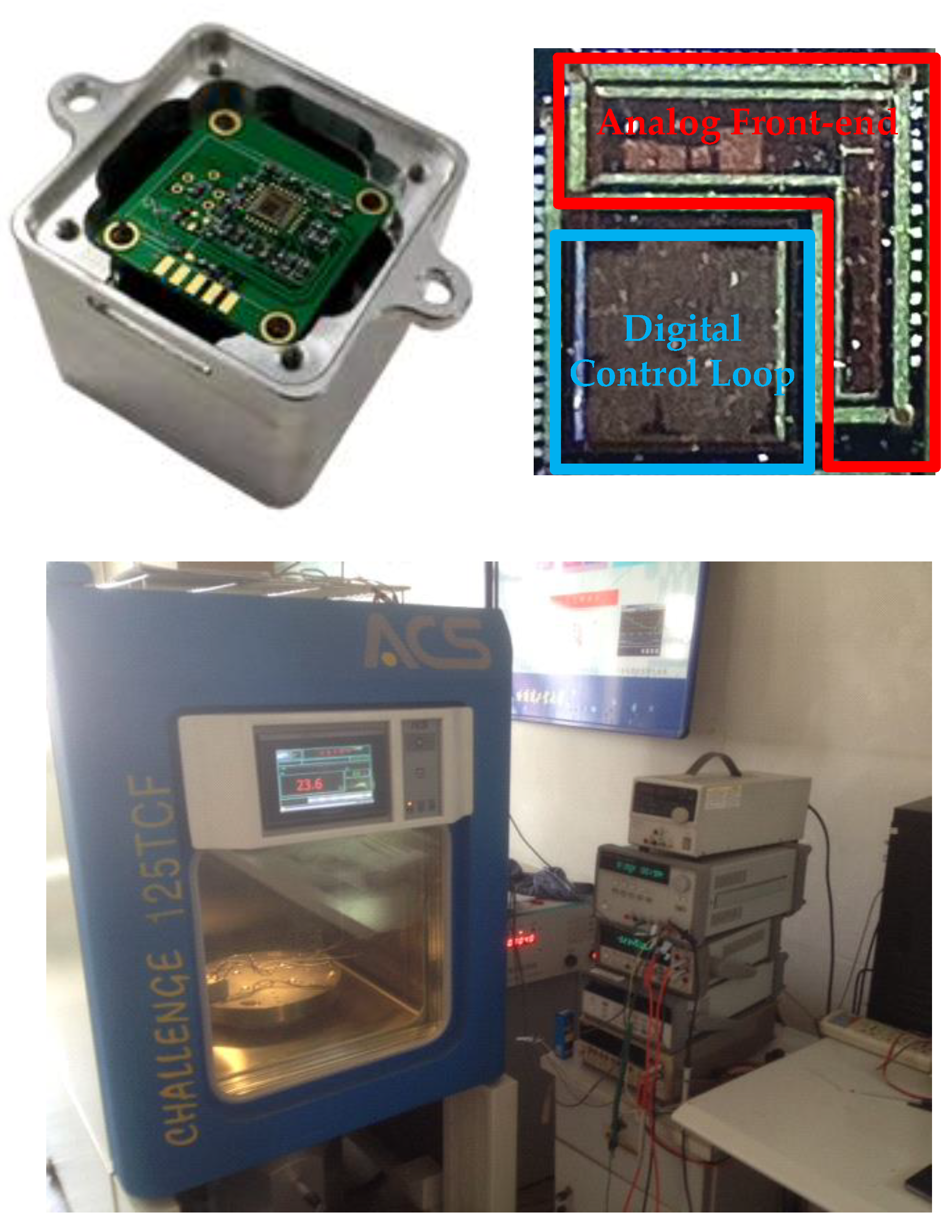
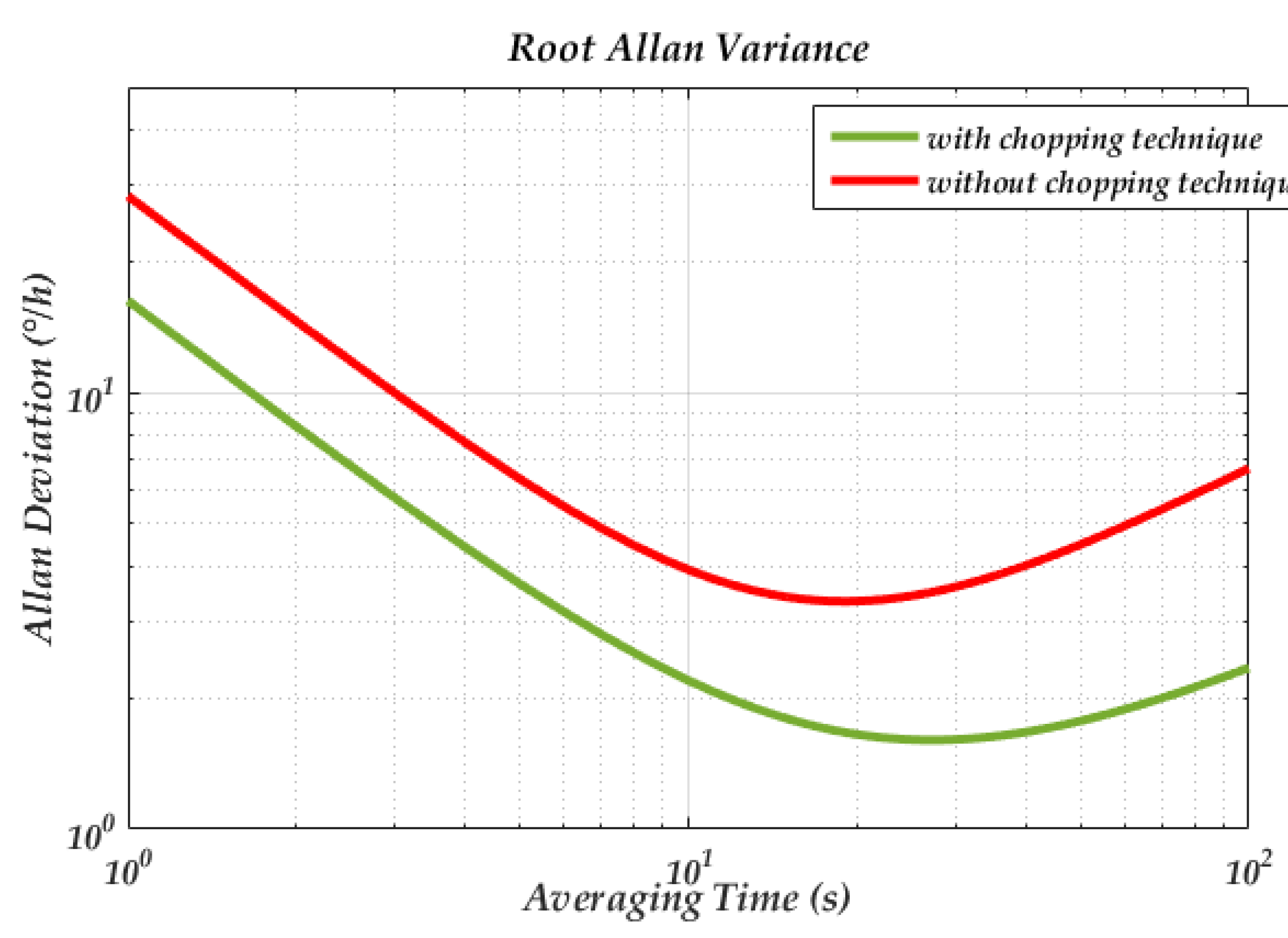
6. Experimental Results
7. Discussion
8. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Challoner, A.D.; Ge, H.H.; Liu, J.Y. Boeing Disc Resonator Gyroscope. In Proceedings of the 2014 IEEE/ION Position, Location and Navigation Symposium-PLANS 2014, Monterey, CA, USA, 5–8 May 2014; pp. 504–514. [Google Scholar]
- Ge, H.H.; Liu, J.Y.; Buchanan, B. Bias Self-calibration Techniques using Silicon Disc Resonator Gyroscope. In Proceedings of the 2015 IEEE International Symposium on Inertial Sensors and Systems (ISISS) Proceedings, Hapuna Beach, HI, USA, 23–26 March 2015; pp. 66–69. [Google Scholar]
- Uppalapati, B.; Ahamed, M.J.; Chodavarapu, V.P. Design and Analysis of Wafer-level Vacuum-Encapsulated Disk Resonator Gyroscope using a Commercial MEMS Process. In Proceedings of the 2017 IEEE National Aerospace and Electronics Conference (NAECON), Dayton, OH, USA, 27–30 June 2017; pp. 108–112. [Google Scholar]
- Hamelin, B.; Yang, J.; Daruwalla, A.; Wen, H.; Ayazi, F. Monocrystalline Silicon Carbide Disk Resonators on Phononic Crystals with Ultra-Low Dissipation Bulk Acoustic Wave Modes. Sci. Rep. 2019, 9, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Li, Q.S.; Xiao, D.B.; Zhou, X.; Xu, Y.; Zhuo, M.; Hou, Z.G.; He, K.X.; Zhang, Y.M.; Wu, X.Z. 0.04 degree-per-hour MEMS disk resonator gyroscope with high-quality factor (510 k) and long decaying time constant (74.9 s). Microsyst. Nanoeng. 2018, 4, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Rozelle, D.M. The Hemispherical Resonator Gyro: From Wineglass To the Planets. Adv. Astronaut. Sci 2009, 134, 1157–1178. [Google Scholar]
- Ahn, C.H.; Nitzan, S.; Ng, E.J.; Hong, V.A.; Yang, Y.; Kimbrell, T.; Horsley, D.A.; Kenny, T.W. Encapsulated high frequency (235 kHz), high-Q (100 k) disk resonator gyroscope with electrostatic parametric pump. Appl. Phys. Lett. 2014, 105, 243504. [Google Scholar] [CrossRef]
- Gerrard, D.D.; Ahn, C.H.; Flader, I.B.; Chen, Y.H.; Ng, E.J.; Yang, Y.S.; Kenny, T.W. Q-Factor Optimization in Disk Resonator Gyroscopes Via Geometric Parameterization. In Proceedings of the 2016 IEEE 29th International Conference on Micro Electro Mechanical Systems (MEMS), Shanghai, China, 24–28 January 2016; pp. 994–997. [Google Scholar]
- Mirjalili, R.; Wen, H.; Serrano, D.E.; Ayazi, F. Substrate-Decoupled Silicon Disk Resonators Having Degenerate Gyroscopic Modes with Q in Excess of 1-Million. In Proceedings of the 2015 Transducers-2015 18th International Conference on Solid-State Sensors, Actuators and Microsystems (TRANSDUCERS), Anchorage, AK, USA, 21–25 June 2015; pp. 15–18. [Google Scholar]
- Behbahani, A.H.; M’Closkey, R.T. Frequency analysis of a uniform ring perturbed by point masses and springs. J. Sound Vib. 2017, 397, 204–221. [Google Scholar] [CrossRef]
- Behbahani, A.H.; Kim, D.; Stupar, P.; DeNatale, J.; M’Closkey, R.T. Tailored Etch Profiles for Wafer-Level Frequency Tuning of Axisymmetric Resonators. J. Microelectromech. Syst. 2017, 26, 333–343. [Google Scholar] [CrossRef]
- Xiao, D.; Yu, D.; Zhou, X.; Hou, Z.; He, H.; Wu, X. Frequency Tuning of a Disk Resonator Gyroscope via Stiffness Perturbation. IEEE Sens. J. 2017, 17, 4725–4734. [Google Scholar] [CrossRef]
- Schwartz, D.M.; Kim, D.; Stupar, P.; DeNatale, J.; M’Closkey, R.T. Modal Parameter Tuning of an Axisymmetric Resonator via Mass Perturbation. J. Microelectromech. Syst. 2015, 24, 545–555. [Google Scholar] [CrossRef]
- Prikhodko, I.P.; Gregory, J.A.; Clark, W.A.; Geen, J.A.; Judy, M.W.; Ahn, C.H.; Kenny, T.W. Mode-Matched MEMS Coriolis Vibratory Gyroscopes: Myth or Reality? In Proceedings of the 2016 IEEE/ION Position, Location and Navigation Symposium (PLANS), Savannah, GA, USA, 11–14 April 2016; pp. 1–4. [Google Scholar]
- Kim, D.; M’Closkey, R. A MEM Vibratory Gyro with Mode-Matching Achieved by Resonator Mass Loading. In Proceedings of the 2014 IEEE/ION Position, Location and Navigation Symposium-PLANS, Monterey, CA, USA, 5–8 May 2014; pp. 499–503. [Google Scholar]
- Hegazi, E.; Abidi, A.A. Varactor characteristics, oscillator tuning curves, and AM-FM conversion. IEEE J. Solid-St Circ. 2003, 38, 1033–1039. [Google Scholar] [CrossRef]
- Rael, J.J.; Abidi, A.A. Physical processes of phase noise in differential LC oscillators. In Proceedings of the IEEE 2000 Custom Integrated Circuits Conference, Orlando, FL, USA, USA, 21–24 May 2000; pp. 569–572. [Google Scholar]
- Levantino, S.; Samori, C.; Bonfanti, A.; Gierkink, S.L.J.; Lacaita, A.L.; Boccuzzi, V. Frequency dependence on bias current in 5-GHz CMOS VCOs: Impact on tuning range and flicker noise upconversion. IEEE J. Solid State Circuits 2002, 37, 1003–1011. [Google Scholar] [CrossRef]
- Nguyen, C.T.C. MEMS technology for timing and frequency control. IEEE Trans. Ultrason. Ferroelectr. Freq. Control 2007, 54, 251–270. [Google Scholar] [CrossRef] [PubMed]
- Coram, G.J. A simple 2-D oscillator to determine the correct decomposition of perturbations into amplitude and phase noise. IEEE Trans. Circuits Syst. I 2001, 48, 896–898. [Google Scholar] [CrossRef]
- Leeson, D.B. A simple model of feedback oscillator noise spectrum. Proc. IEEE 1966, 54, 329–330. [Google Scholar] [CrossRef]
- Hajimiri, A.; Lee, T.H. A general theory of phase noise in electrical oscillators. IEEE J. Solid State Circuits 1998, 33, 179–194. [Google Scholar] [CrossRef]
- Kaajakari, V.; Koskinen, J.K.; Mattila, T. Phase noise in capacitively coupled micromechanical oscillators. IEEE Trans. Ultrason. Ferroelectr. Freq. 2005, 52, 2322–2331. [Google Scholar] [CrossRef] [PubMed]
- Pardo, M.; Sorenson, L.; Ayazi, F. A Phase-Noise Model for Nonlinear Piezoelectrically-Actuated MEMS Oscillators. In Proceedings of the 2011 IEEE International Symposium of Circuits and Systems (ISCAS), Rio de Janeiro, Brazil, 15–18 May 2011; pp. 221–224. [Google Scholar]
- Kaertner, F.X. Analysis of White and F-Alpha Noise in Oscillators. Int. J. Circuit. Theory Appl. 1990, 18, 485–519. [Google Scholar] [CrossRef]
- Razavi, B. A study of phase noise in CMOS oscillators. IEEE J. Solid State Circuits 1996, 31, 331–343. [Google Scholar] [CrossRef]
- Demir, A.; Mehrotra, A.; Roychowdhury, J. Phase noise in oscillators: A unifying theory and numerical methods for characterization. IEEE Trans. Circuits Syst. I Fundam. Theory Appl. 2000, 47, 655–674. [Google Scholar] [CrossRef]
- Ward, P.; Duwel, A. Oscillator Phase Noise: Systematic Construction of an Analytical Model Encompassing Nonlinearity. IEEE Trans. Ultrason. Ferroelectr. Freq. 2011, 58, 195–205. [Google Scholar] [CrossRef]
- Imani, A.; Hashemi, H. Analysis and Design of Low Phase-Noise Oscillators With Nonlinear Resonators. IEEE Trans. Microw Theory 2012, 60, 3749–3760. [Google Scholar] [CrossRef]
- Pardo, M.; Sorenson, L.; Pan, W.; Ayazi, F. Phase Noise Shaping Via Forced Nonlinearity in Piezoelectrically Actuated Silicon Micromechanical Oscillators. In Proceedings of the 2011 IEEE 24th International Conference on Micro Electro. Mechanical Systems (MEMS), Cancun, Mexico, 23–27 January 2011; pp. 780–783. [Google Scholar]
- He, L.; Xu, Y.P.; Palaniapan, M. A State-Space Phase-Noise Model for Nonlinear MEMS Oscillators Employing Automatic Amplitude Control. IEEE Trans. Circuits Syst. I 2010, 57, 189–199. [Google Scholar] [CrossRef]
- Zhao, J.; Zhao, Y.; Wang, X.; Xia, G.M.; Qiu, A.P.; Su, Y.; Xu, Y.P. A System Decomposition Model for Phase Noise in Silicon Oscillating Accelerometers. IEEE Sens. J. 2016, 16, 5259–5269. [Google Scholar] [CrossRef]
- Agrawal, D.K.; Seshia, A.A. An Analytical Formulation for Phase Noise in MEMS Oscillators. IEEE Trans. Ultrason. Ferroelectr. Freq. 2014, 61, 1938–1952. [Google Scholar] [CrossRef] [PubMed]
- Nayfeh, A.H.; Younis, M.I.; Abdel-Rahman, E.M. Reduced-order models for MEMS applications. Nonlinear Dyn. 2005, 41, 211–236. [Google Scholar] [CrossRef]
- Younis, M.I.; Nayfeh, A.H. A study of the nonlinear response of a resonant microbeam to an electric actuation. Nonlinear Dyn. 2003, 31, 91–117. [Google Scholar] [CrossRef]
- Chorsi, M.T.; Chorsi, H.T. Modeling and analysis of MEMS disk resonators. Microsyst. Technol. 2018, 24, 2517–2528. [Google Scholar] [CrossRef]
- Chorsi, M.T.; Chorsi, H.T.; Gedney, S.D. Radial-contour mode microring resonators: Nonlinear dynamics. Int. J. Mech. Sci. 2017, 130, 258–266. [Google Scholar] [CrossRef]
- Li, Q.; Xiao, D.; Xu, Y.; Zhuo, M.; Zhou, X.; Zhang, Y.; Yu, L.; Wu, X. Nonlinearity Reduction in Disk Resonator Gyroscopes Based on the Vibration Amplification Effect. IEEE Trans. Ind. Electron. 2020, 67, 6946–6954. [Google Scholar] [CrossRef]
- Agrawal, D.K.; Woodhouse, J.; Seshia, A.A. Modeling Nonlinearities in MEMS Oscillators. IEEE Trans. Ultrason. Ferroelectr. Freq. 2013, 60, 1646–1659. [Google Scholar] [CrossRef]
- Wang, G.S.; Liu, X.W. The Analysis of the Phase Noise of the Closed-Loop Driver Circuit in Micromechanical Gyroscope Based on the Phase-Locked Principle. Adv. Mat. Res. 2014, 981, 526–529. [Google Scholar] [CrossRef]
- Phani, A.S.; Seshia, A.A.; Palaniapan, M.; Howe, R.T.; Yasaitis, J.A. Modal coupling in micromechanical vibratory rate gyroscopes. IEEE Sens. J. 2006, 6, 1144–1152. [Google Scholar] [CrossRef]
- IEEE Standard Specification Format Guide and Test Procedure for Coriolis Vibratory Gyros; IEEE: Piscataway, NJ, USA, 2004.
- Zhou, X.; Xiao, D.B.; Li, Q.S.; Hou, Z.Q.; He, K.X.; Chen, Z.H.; Wu, Y.L.; Wu, X.Z. Decaying Time Constant Enhanced MEMS Disk Resonator for High Precision Gyroscopic Application. IEEE-ASME Trans. Mech. 2018, 23, 452–458. [Google Scholar] [CrossRef]
- Chen, F.; Li, X.; Kraft, M. Electromechanical Sigma–Delta Modulators (ΣΔM) Force Feedback Interfaces for Capacitive MEMS Inertial Sensors: A Review. IEEE Sens. J. 2016, 16, 6476–6495. [Google Scholar] [CrossRef]
- Newland, D.E. Mechanical Vibration Analysis and Computation. J. Acoust Soc. Am. 1990, 88, 2506. [Google Scholar] [CrossRef]
- Saukoski, M.; Aaltonen, L.; Halonen, K.A.I. Zero-rate output and quadrature compensation in vibratory MEMS gyroscopes. IEEE Sens. J. 2007, 7, 1639–1652. [Google Scholar] [CrossRef]
- Ismail, A.; Ashraf, K.; Metawea, A.; Mostfa, I.; Saeed, A.; Helal, E.; Essawy, M.; Abdelazim, M.; Ibrahim, M.; Raafat, R.; et al. A High-Performance Self-clocked Digital-Output Quartz Gyroscope. In Proceedings of the 2015 IEEE Sensors, Busan, Korea, 1–4 November 2015; pp. 1146–1149. [Google Scholar]
- Omar, A.; Elshennawy, A.; AbdelAzim, M.; Ismail, A.H. Analyzing the Impact of Phase Errors in Quadrature Cancellation Techniques for MEMS Capacitive Gyroscopes. In Proceedings of the 2018 IEEE Sensor, New Delhi, India, 28–31 October 2018; pp. 1264–1267. [Google Scholar]
- Wang, Y.H.; Fu, Q.; Zhang, Y.F.; Zhang, W.B.; Chen, D.L.; Yin, L.; Liu, X.W. A Digital Closed-Loop Sense MEMS Disk Resonator Gyroscope Circuit Design Based on Integrated Analog Front-end. Sensors 2020, 20, 687. [Google Scholar] [CrossRef]
- Enz, C.C.; Temes, G.C. Circuit techniques for reducing the effects of op-amp imperfections: Autozeroing, correlated double sampling, and chopper stabilization. Proc. IEEE 1996, 84, 1584–1614. [Google Scholar] [CrossRef]




| Mechanical Domain Model | Electrical Model |
|---|---|
| Mass mx | Inductance L |
| Damping coefficient Dxx | Resistance R |
| Elasticity coefficient kxx | Capacitance C |
| Drive force Fx | Drive voltage ud |
| Elastic force Fk | Voltage on Capacitance VC |
| Frictional force FD | Voltage on Resistance VR |
| Resultant force Fm | Voltage on Inductance VL |
| Velocity vx | Current i |
| Injected impulse ΔJ = FinjΔt | Injected flux linkage Δψ = uinjΔt |
| Parameter | Value | Unit |
|---|---|---|
| mx | 2.54 × 10−6 | kg |
| Dxx | 2.2 × 10−8 | N/m/s |
| kxx | 485 | N/m |
| k2 | 4 × 1012 | N/m3 |
| kF/V | 5 × 10−5 | N/V |
| ki/v | 3 × 10−6 | A/m/s |
| RV/i | 3.62 × 106 | Ω |
| Vref | 1 | V |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, W.; Chen, W.; Yin, L.; Di, X.; Chen, D.; Fu, Q.; Zhang, Y.; Liu, X. Study of the Influence of Phase Noise on the MEMS Disk Resonator Gyroscope Interface Circuit. Sensors 2020, 20, 5470. https://doi.org/10.3390/s20195470
Zhang W, Chen W, Yin L, Di X, Chen D, Fu Q, Zhang Y, Liu X. Study of the Influence of Phase Noise on the MEMS Disk Resonator Gyroscope Interface Circuit. Sensors. 2020; 20(19):5470. https://doi.org/10.3390/s20195470
Chicago/Turabian StyleZhang, Wenbo, Weiping Chen, Liang Yin, Xinpeng Di, Dongliang Chen, Qiang Fu, Yufeng Zhang, and Xiaowei Liu. 2020. "Study of the Influence of Phase Noise on the MEMS Disk Resonator Gyroscope Interface Circuit" Sensors 20, no. 19: 5470. https://doi.org/10.3390/s20195470
APA StyleZhang, W., Chen, W., Yin, L., Di, X., Chen, D., Fu, Q., Zhang, Y., & Liu, X. (2020). Study of the Influence of Phase Noise on the MEMS Disk Resonator Gyroscope Interface Circuit. Sensors, 20(19), 5470. https://doi.org/10.3390/s20195470




