A Spatially Distributed Fiber-Optic Temperature Sensor for Applications in the Steel Industry
Abstract
1. Introduction
2. Sensing Principle and Interrogation System
2.1. Rayleigh-Scattering-Based Temperature Measurements
2.2. Optical Frequency Domain Reflectometry (OFDR)
3. Distributed Temperature Sensing Experiments
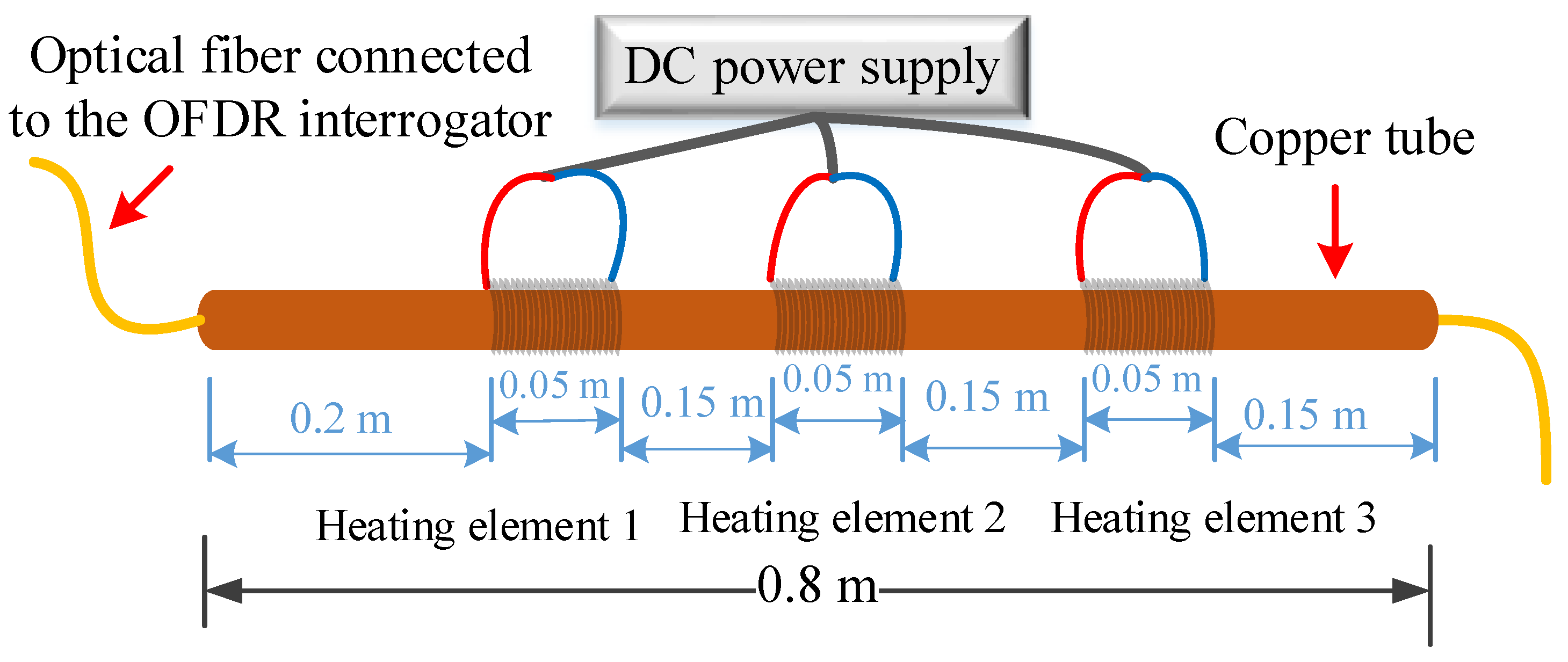
3.1. Optical Fiber Encased in a Copper Tube Equipped with Heating Coils
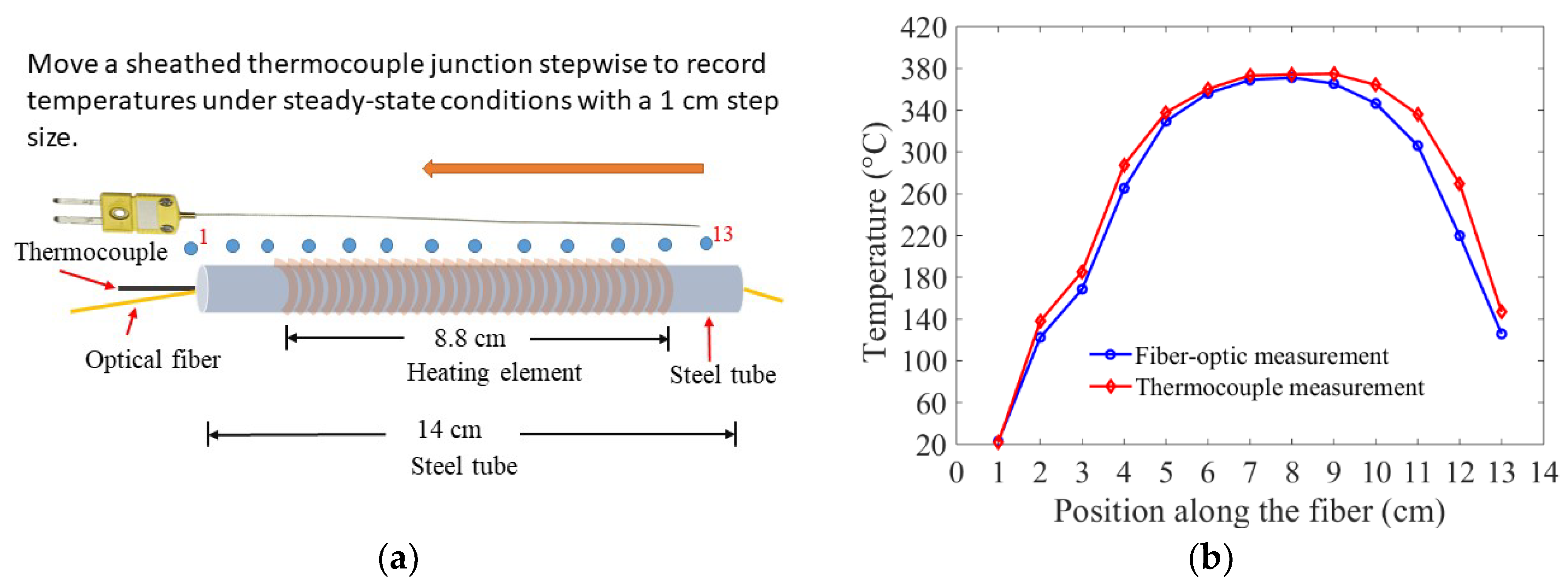
3.2. Comparison of Fiber-Optic Temperature Measurement System with Thermocouples
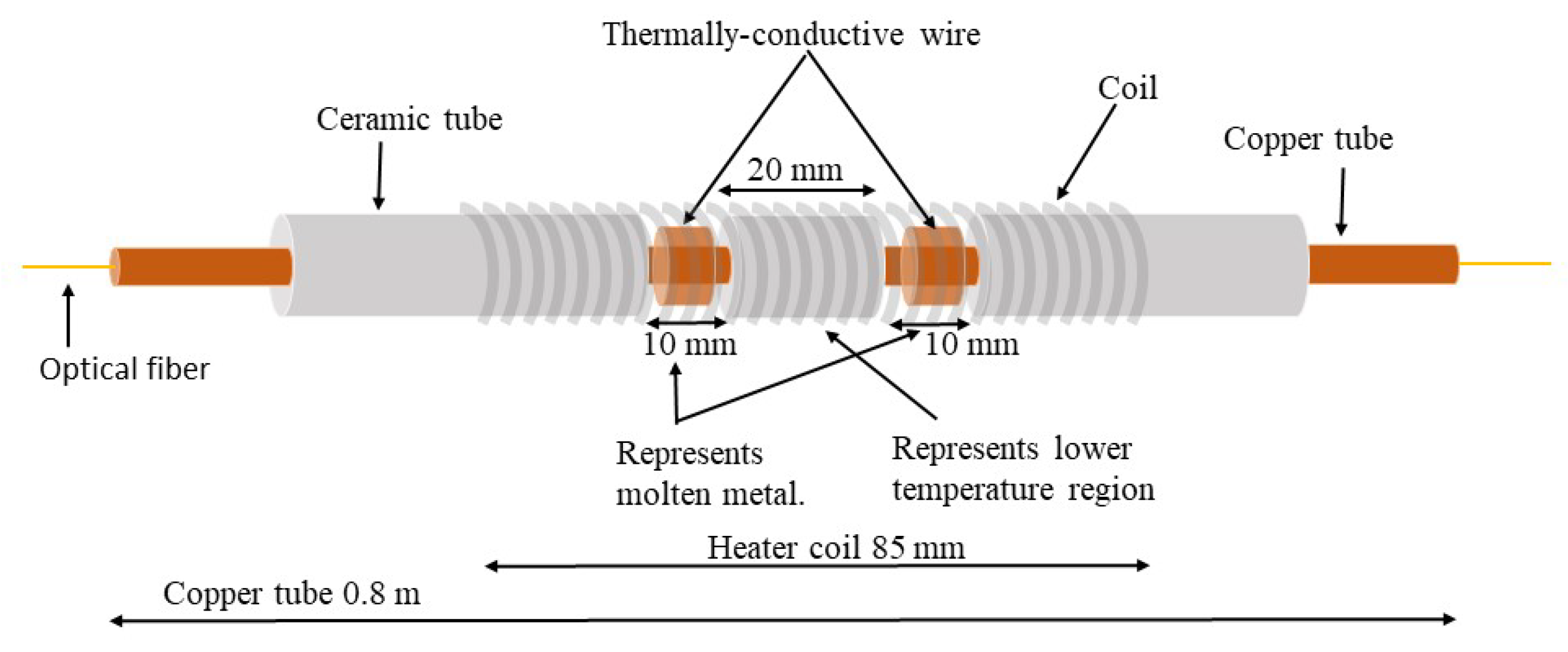
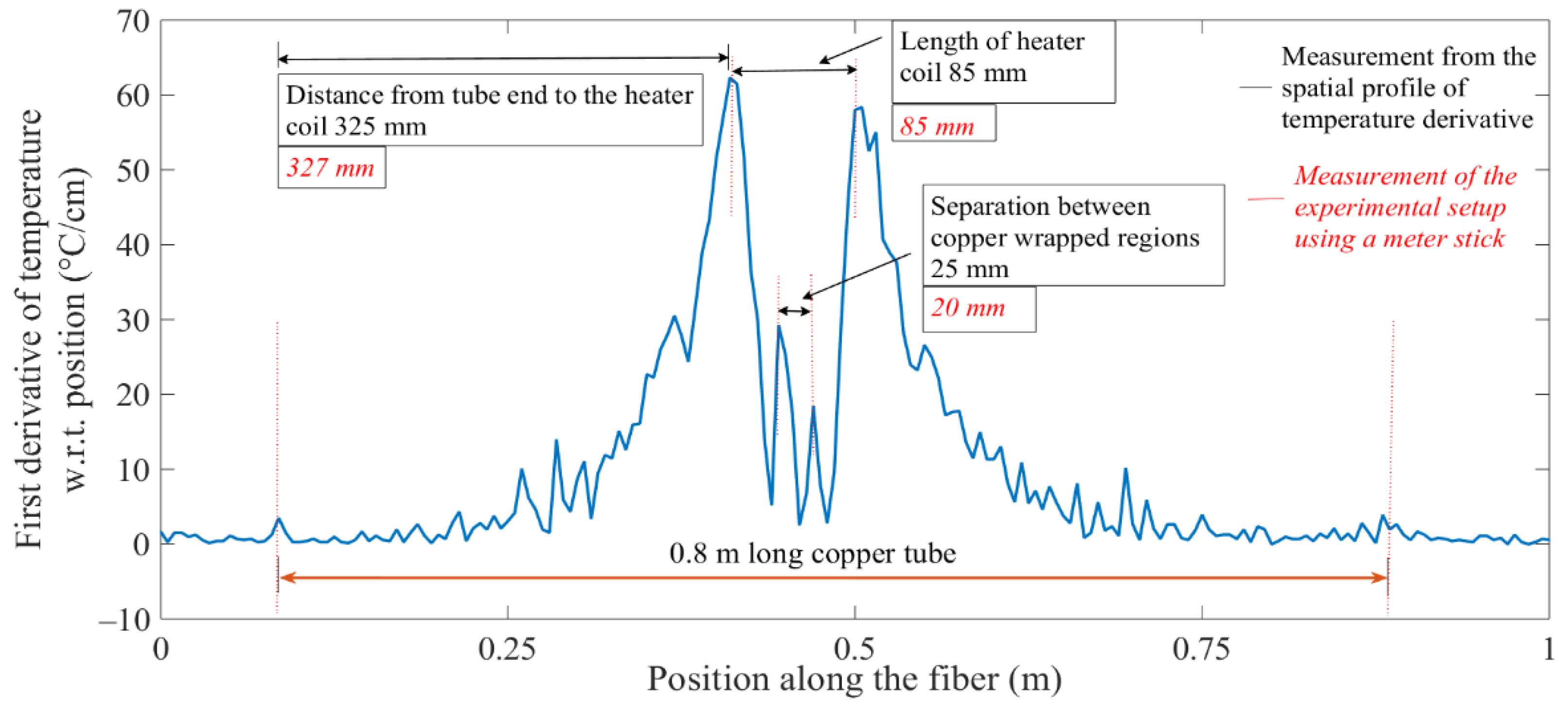
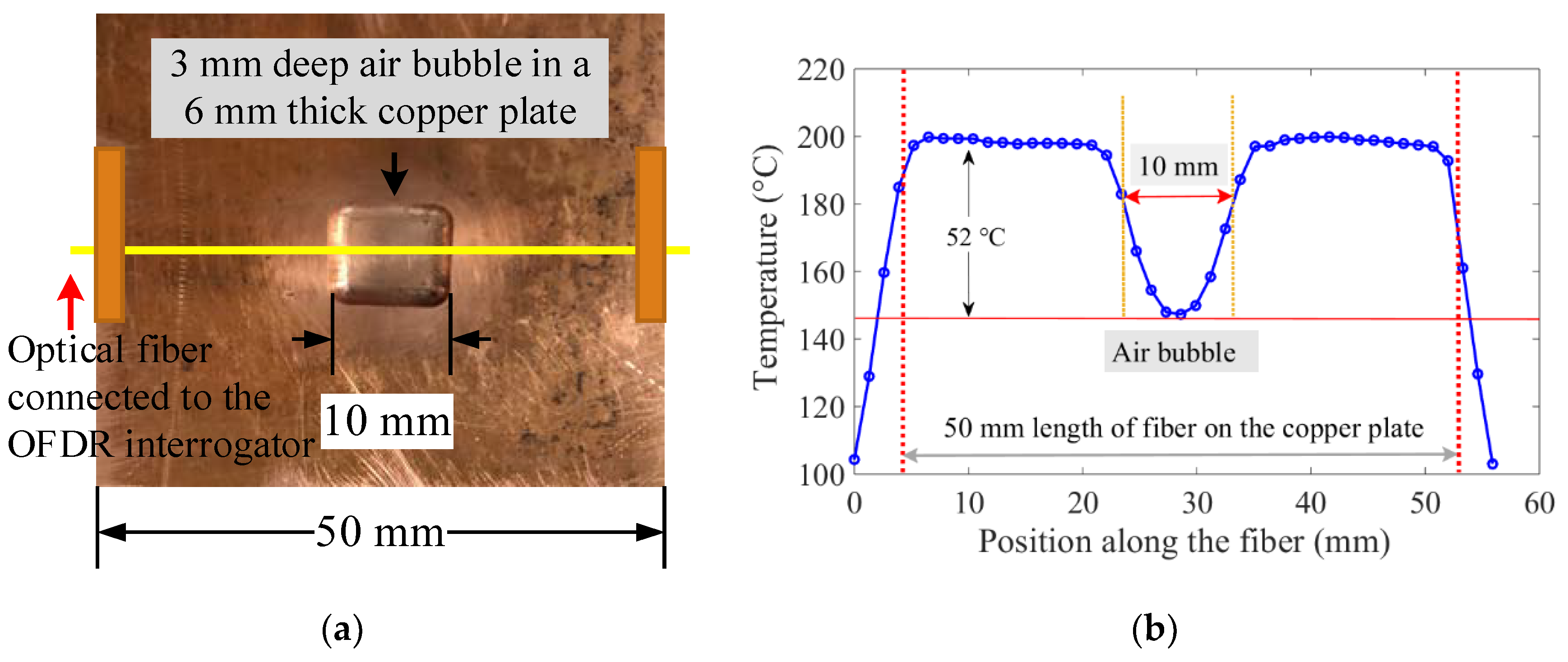
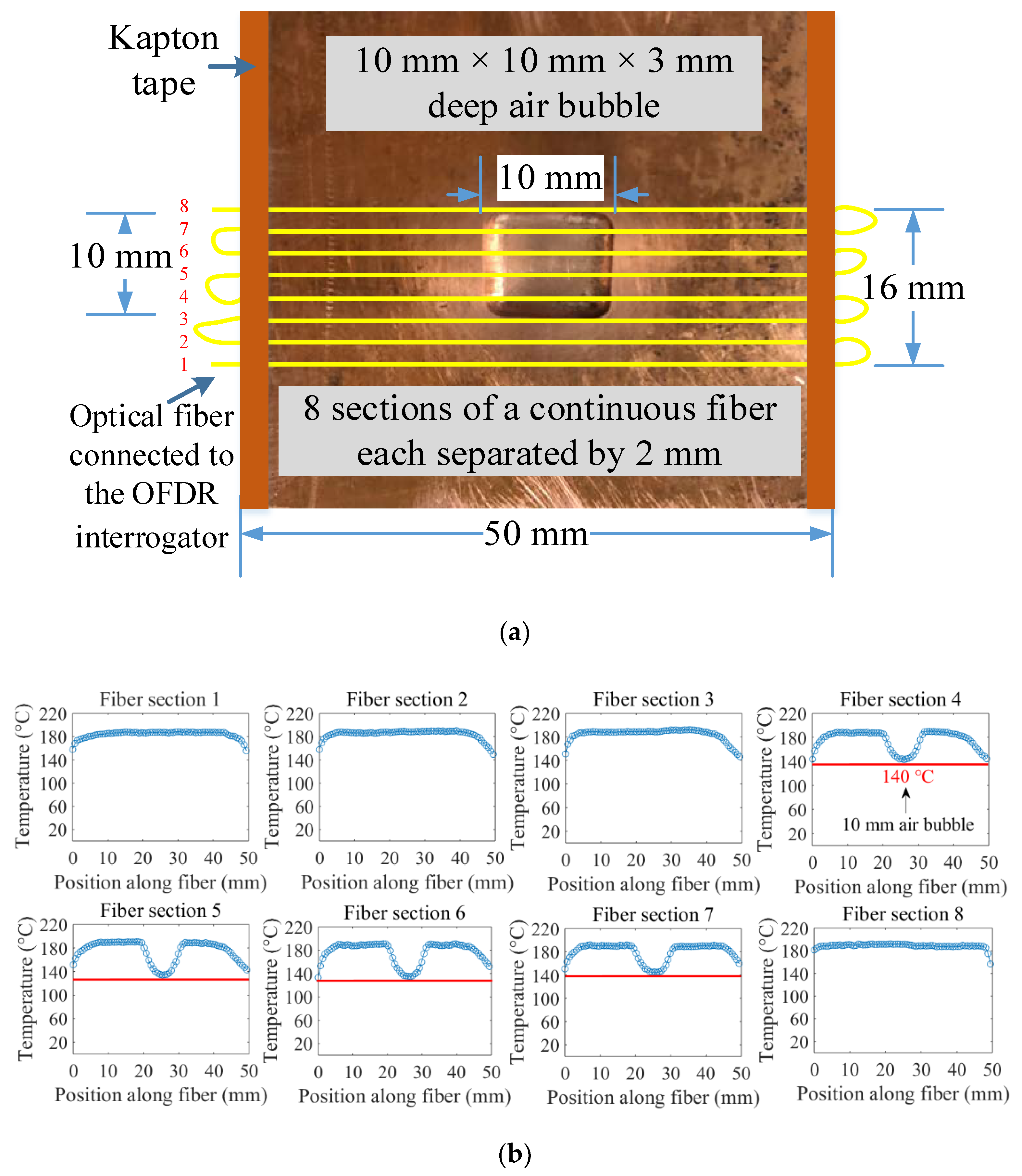
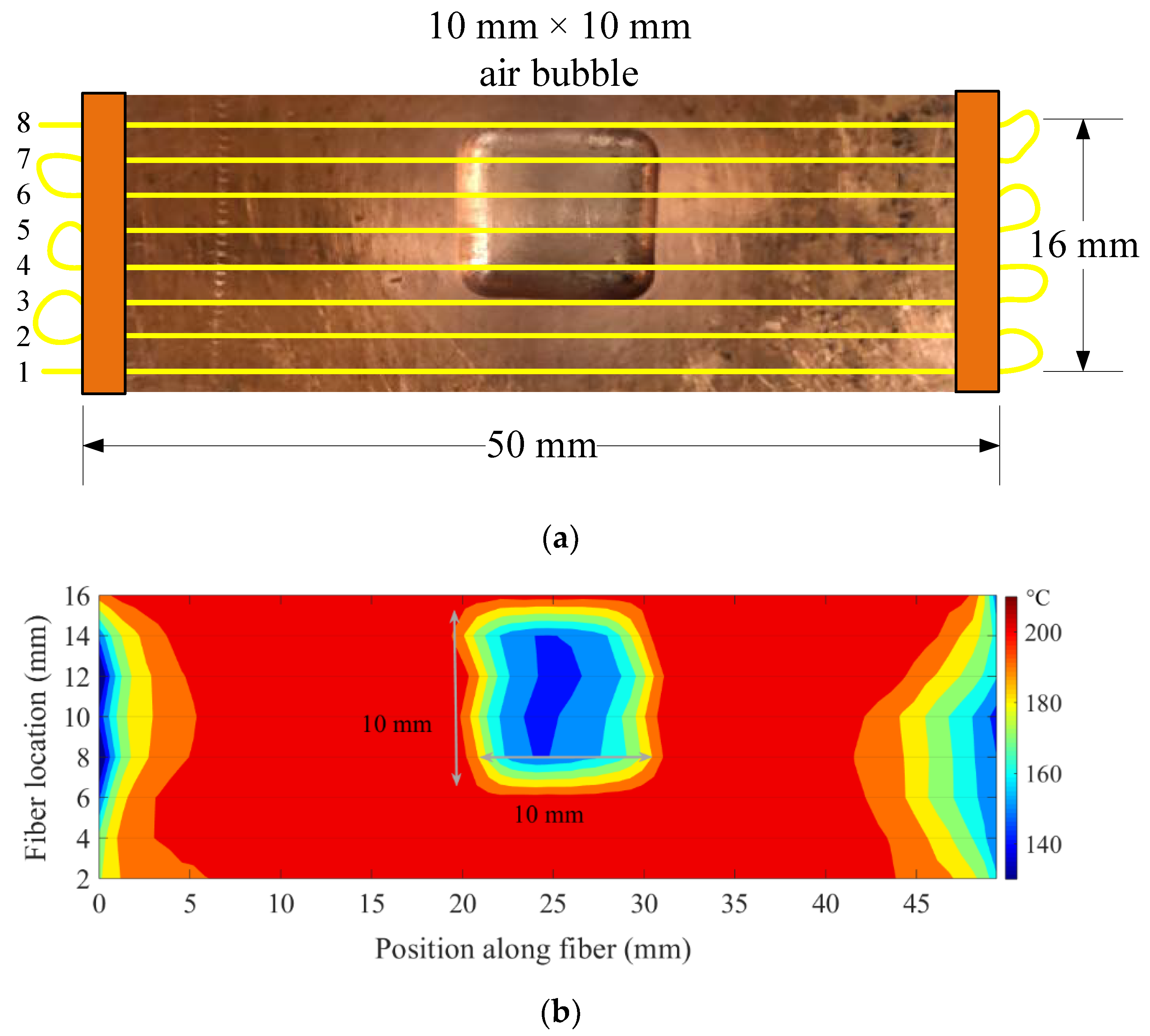
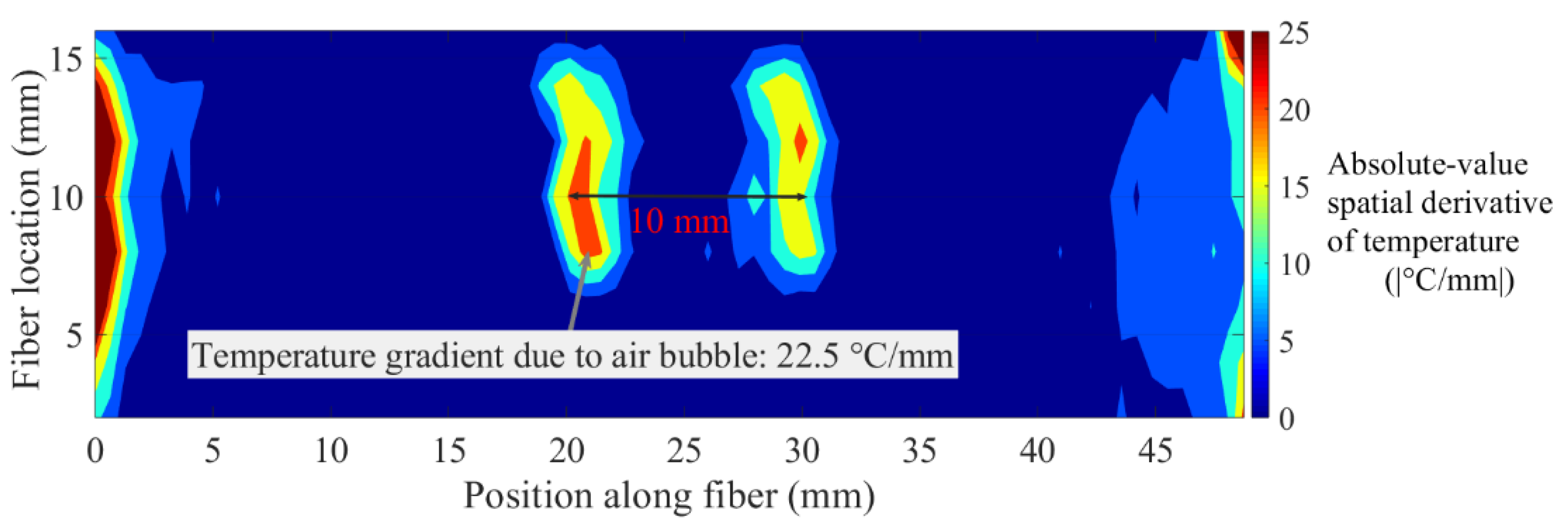
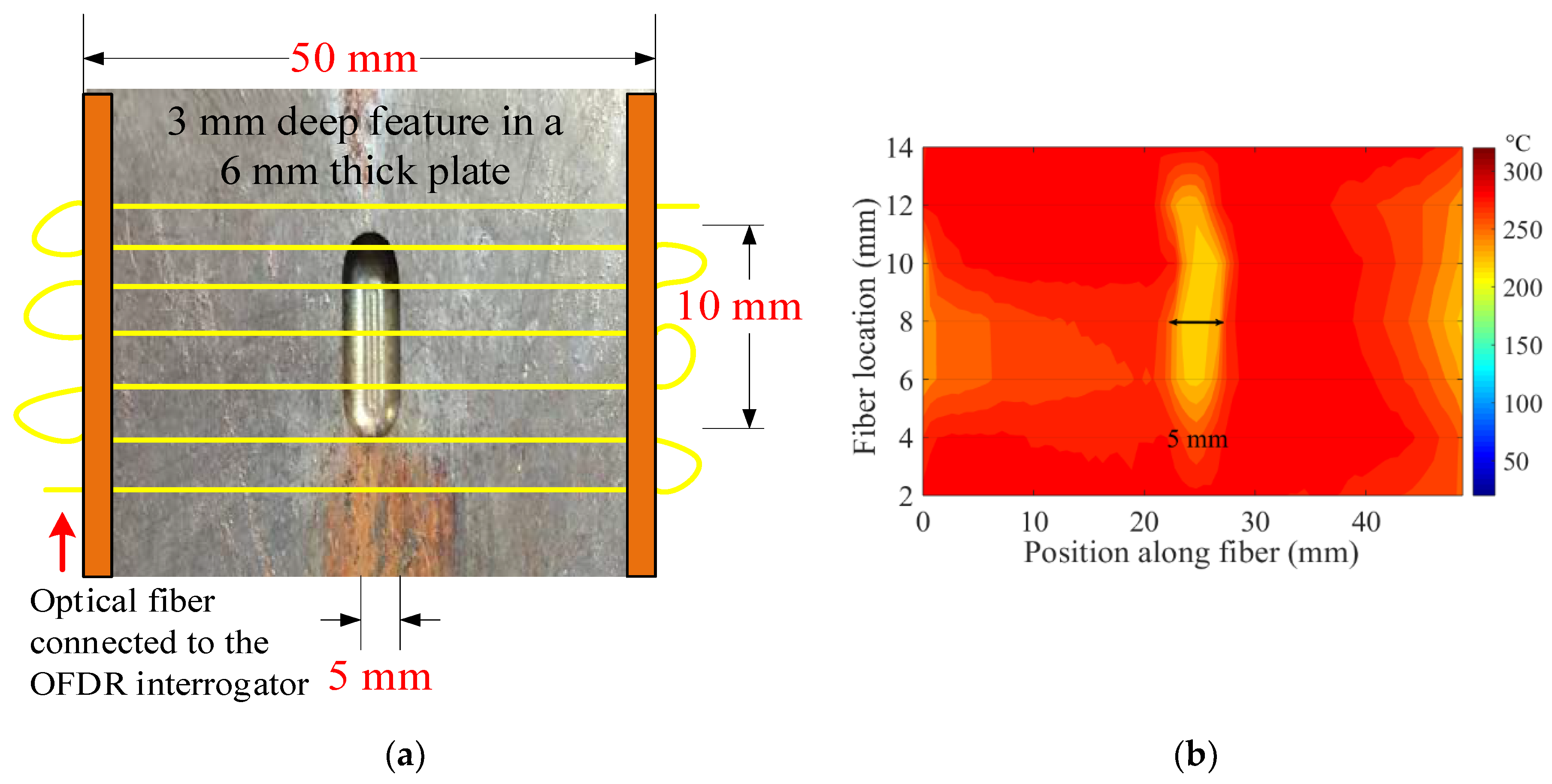
3.3. Thermal Mapping of Test Objects with Localized Temperature Variations
3.3.1. One-Dimensional (1D) Thermal Mapping
3.3.2. Two-Dimensional (2D) Thermal Mapping
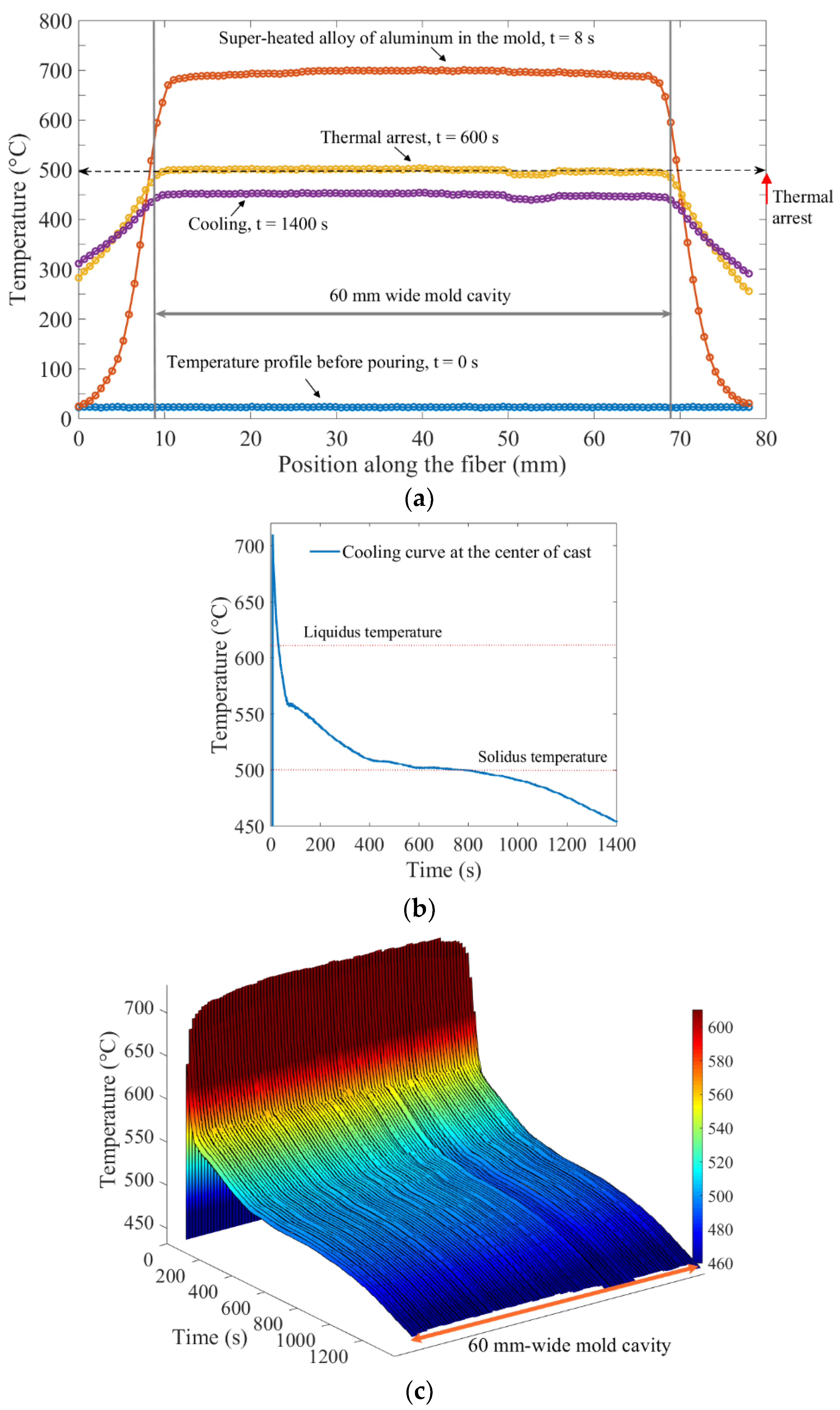
3.4. Distributed Temperature Measurements across a Mold Cavity during Aluminum Casting
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Suzuki, M.; Cho, J.W.; Sato, H.; Shibata, H.; Emi, T. Analysis of Heat Transfer and Solidifying Shell Deformation in Mold in High Speed Continuous Casting of Peritectic Medium Carbon Steels. In Proceedings of the Steelmaking Conference, Toronto, ON, Canada, 22–24 March 1998. [Google Scholar]
- Xia, G.; Narzt, H.P.; Fürst, C.; Mörwald, K.; Moertl, J.; Reisinger, P.; Lindenberger, L. Investigation of mould thermal behaviour by means of mould instrumentation. Ironmak. Steelmak. 2004, 31, 364–370. [Google Scholar] [CrossRef]
- Thomas, B.G.; Wells, M.; Li, D. Monitoring of Meniscus Thermal Phenomena with Thermocouples in Continuous Casting of Steel. In Sensors, Sampling, and Simulation for Process Control; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2011. [Google Scholar]
- Natarajan, T.T.; Story, S.R.; Piccone, T.J.; van Ness, K.D. Peritectic Range Study Using Mold Thermocouple Data. In Proceedings of the 2017 AISTech Conference, Nashville, TN, USA, 8–11 May 2017. [Google Scholar]
- Lee, B. Review of the present status of optical fiber sensors. Opt. Fiber Technol. 2003, 9, 57–79. [Google Scholar] [CrossRef]
- Zhu, C.; Zhuang, Y.; Zhang, B.; Muhammad, R.; Wang, P.P.; Huang, J. A Miniaturized Optical Fiber Tip High-Temperature Sensor Based on Concave-Shaped Fabry-Perot Cavity. IEEE Photonics Technol. Lett. 2019, 31, 35–38. [Google Scholar] [CrossRef]
- Lecoeuche, V.; Hathaway, M.; Webb, D.; Pannell, C.; Jackson, D. 20-km distributed temperature sensor based on spontaneous Brillouin scattering. IEEE Photonics Technol. Lett. 2000, 12, 1367–1369. [Google Scholar] [CrossRef]
- Bao, X.; Dhliwayo, J.; Heron, N.; Webb, D.; Jackson, D.A. Experimental and theoretical studies on a distributed temperature sensor based on Brillouin scattering. J. Lightwave Technol. 1995, 13, 1340–1348. [Google Scholar] [CrossRef]
- Shimizu, K.; Horiguchi, T.; Koyamada, Y. Measurement of distributed strain and temperature in a branched optical fiber network by use of Brillouin optical time-domain reflectometry. Opt. Lett. 1995, 20, 507–509. [Google Scholar] [CrossRef] [PubMed]
- Weng, Y.; Ip, E.; Pan, Z.; Wang, T. Single-end simultaneous temperature and strain sensing techniques based on Brillouin optical time domain reflectometry in few-mode fibers. Opt. Express 2015, 23, 9024–9039. [Google Scholar] [CrossRef]
- Guan, B.-O.; Tam, H.Y.; Tao, X.-M.; Dong, X.-Y. Simultaneous strain and temperature measurement using a superstructure fiber Bragg grating. IEEE Photonics Technol. Lett. 2000, 12, 675–677. [Google Scholar] [CrossRef]
- Patrick, H.; Williams, G.; Kersey, A.; Pedrazzani, J.; Vengsarkar, A. Hybrid fiber Bragg grating/long period fiber grating sensor for strain/temperature discrimination. IEEE Photonics Technol. Lett. 1996, 8, 1223–1225. [Google Scholar] [CrossRef]
- Jung, J.; Nam, H.; Lee, B. Fiber Bragg grating temperature sensor with controllable high sensitivity. In Proceedings of the 11th Annual Meeting—IEEE Lasers and Electro-Optics Society 1998 Annual Meeting, Orlando, FL, USA, 1–4 December 1998. [Google Scholar]
- Zhang, B.; Kahrizi, M. High-Temperature Resistance Fiber Bragg Grating Temperature Sensor Fabrication. IEEE Sens. J. 2007, 7, 586–591. [Google Scholar] [CrossRef]
- Thomas, B.G.; Okelman, M. Implementation of Temperature and Strain Micro-Sensors into a Casting Mold Surface. In Sensors, Sampling, and Simulation for Process Control; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2011. [Google Scholar]
- Lieftucht, D.; Reifferscheid, M.; Schramm, T.; Krasilnikov, A.; Kirsch, D. HD Mold—A New Fiber-Optical-Based Mold Monitoring System. Iron Steel Technol. 2013, 10, 87–95. [Google Scholar]
- Spierings, T.; Kamperman, A.; Hengeveld, H.; Kromhout, J.; Dekker, E. Development and Application of Fiber Bragg Gratings for Slab Casting. In Proceedings of the 2017 AISTech Conference, Nashville, TN, USA, 8–11 May 2017. [Google Scholar]
- Du, Y.; Jothibasu, S.; Zhuang, Y.; Zhu, C.; Huang, J. Rayleigh backscattering based macrobending single mode fiber for distributed refractive index sensing. Sens. Actuators B Chem. 2017, 248, 346–350. [Google Scholar] [CrossRef]
- Du, Y.; Yang, Q.; Huang, J. Soft Prosthetic Forefinger Tactile Sensing via a String of Intact Single Mode Optical Fiber. IEEE Sens. J. 2017, 17, 7455–7459. [Google Scholar] [CrossRef]
- Jothibasu, S.; Du, Y.; Anandan, S.; Dhaliwal, G.S.; Gerald, R.E.; Watkins, S.E.; Chandrashekhara, K.; Huang, J. Spatially continuous strain monitoring using distributed fiber optic sensors embedded in carbon fiber composites. Opt. Eng. 2019, 58, 072004. [Google Scholar] [CrossRef]
- Du, Y.; Jothibasu, S.; Zhuang, Y.; Zhu, C.; Huang, J. Unclonable Optical Fiber Identification Based on Rayleigh Backscattering Signatures. J. Lightwave Technol. 2017, 35, 4634–4640. [Google Scholar] [CrossRef]
- Zhu, C.; Zhuang, Y.; Chen, Y. Distributed fiber-optic pressure sensor based on Bourdon tubes metered by optical frequency-domain reflectometry. Opt. Eng. 2019, 58, 072010. [Google Scholar] [CrossRef]
- Du, Y.; Liu, T.; Ding, Z.; Han, Q.; Liu, K.; Jiang, J.; Chen, Q.; Feng, B. Cryogenic Temperature Measurement Using Rayleigh Backscattering Spectra Shift by OFDR. IEEE Photonics Technol. Lett. 2014, 26, 1150–1153. [Google Scholar]
- Yan, A.; Huang, S.; Li, S.; Chen, R.; Ohodnicki, P.; Buric, M.; Lee, S.; Li, M.-J.; Chen, K.P. Distributed Optical Fiber Sensors with Ultrafast Laser Enhanced Rayleigh Backscattering Profiles for Real-Time Monitoring of Solid Oxide Fuel Cell Operations. Sci. Rep. 2017, 7, 1–9. [Google Scholar] [CrossRef]
- Boyd, C.D.; Dickerson, B.D.; Fitzpatrick, B.K. Monitoring distributed temperatures along superconducting degaussing cables via Rayleigh backscattering in optical fibers. In Proceedings of the Intelligent Ships Symposium IX, Philadelphia, PA, USA, 25–26 May 2011. [Google Scholar]
- Kwon, Y.-S.; Naeem, K.; Jeon, M.-Y.; Kwon, I.-B. Enhanced sensitivity of distributed-temperature sensor with Al-coated fiber based on OFDR. Opt. Fiber Technol. 2019, 48, 229–234. [Google Scholar] [CrossRef]
- Wood, T.W.; Blake, B.; Blue, T.E.; Petrie, C.M.; Hawn, D. Evaluation of the Performance of Distributed Temperature Measurements With Single-Mode Fiber Using Rayleigh Backscatter up to 1000^{\circ}{\rm C}. IEEE Sens. J. 2013, 14, 124–128. [Google Scholar] [CrossRef]
- McCary, K.; Wilson, B.; Birri, A.; Blue, T.E. Response of Distributed Fiber Optic Temperature Sensors to High-Temperature Step Transients. IEEE Sens. J. 2018, 18, 8755–8761. [Google Scholar] [CrossRef]
- Sang, A.K.; Froggatt, M.E.; Gifford, D.K.; Dickerson, B.D. One centimeter spatial resolution temperature measurements from 25 to 850 C using Rayleigh scatter in gold coated fiber. In Proceedings of the Conference on Lasers and Electro-Optics/Quantum Electronics and Laser Science Conference and Photonic Applications Systems Technologies, Baltimore, MD, USA, 6–11 May 2007. [Google Scholar]
- Suzuki, M.; Yu, C.H.; Sato, H.; Tsui, Y.; Shibata, H.; Emi, T. Origin of Heat Transfer Anomaly and Solidifying Shell Deformation of Peritectic Steels in Continuous Casting. ISIJ Int. 1996, 36, S171–S174. [Google Scholar] [CrossRef]
- Abraham, S.; Bodnar, R.; Lonnqvist, J.; Shahbazian, F.; Lagerstedt, A.; Andersson, M. Investigation of Peritectic Behavior of Steel Using a Thermal Analysis Technique. Met. Mater. Trans. A 2019, 50, 2259–2271. [Google Scholar] [CrossRef]







© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Roman, M.; Balogun, D.; Zhuang, Y.; Gerald, R.E., II; Bartlett, L.; O’Malley, R.J.; Huang, J. A Spatially Distributed Fiber-Optic Temperature Sensor for Applications in the Steel Industry. Sensors 2020, 20, 3900. https://doi.org/10.3390/s20143900
Roman M, Balogun D, Zhuang Y, Gerald RE II, Bartlett L, O’Malley RJ, Huang J. A Spatially Distributed Fiber-Optic Temperature Sensor for Applications in the Steel Industry. Sensors. 2020; 20(14):3900. https://doi.org/10.3390/s20143900
Chicago/Turabian StyleRoman, Muhammad, Damilola Balogun, Yiyang Zhuang, Rex E. Gerald, II, Laura Bartlett, Ronald J. O’Malley, and Jie Huang. 2020. "A Spatially Distributed Fiber-Optic Temperature Sensor for Applications in the Steel Industry" Sensors 20, no. 14: 3900. https://doi.org/10.3390/s20143900
APA StyleRoman, M., Balogun, D., Zhuang, Y., Gerald, R. E., II, Bartlett, L., O’Malley, R. J., & Huang, J. (2020). A Spatially Distributed Fiber-Optic Temperature Sensor for Applications in the Steel Industry. Sensors, 20(14), 3900. https://doi.org/10.3390/s20143900





