On-Channel Integrated Optofluidic Pressure Sensor with Optically Boosted Sensitivity
Abstract
1. Introduction
2. Materials and Methods
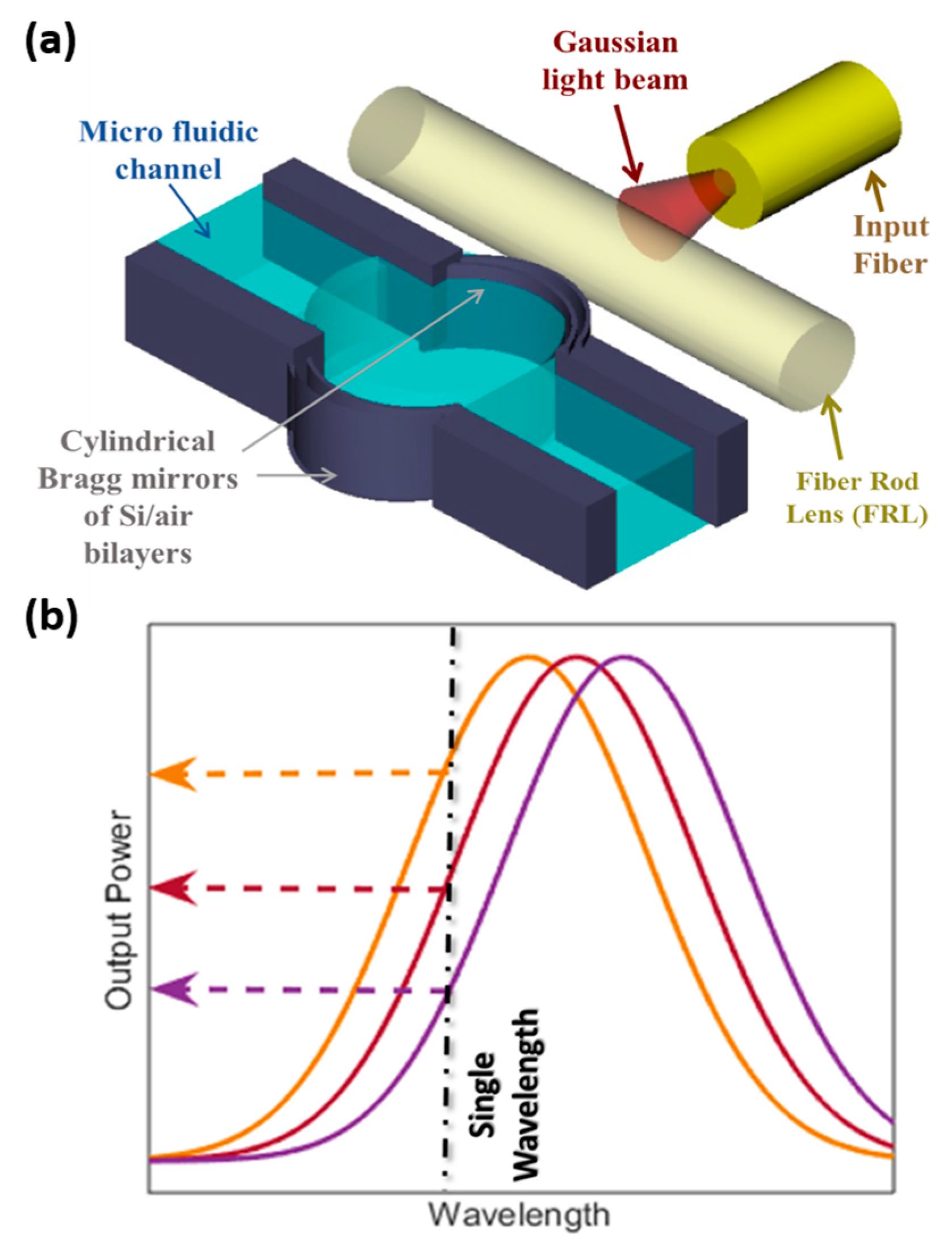
2.1. Proposed Structure
2.2. Numerical Simulation
3. Experimental
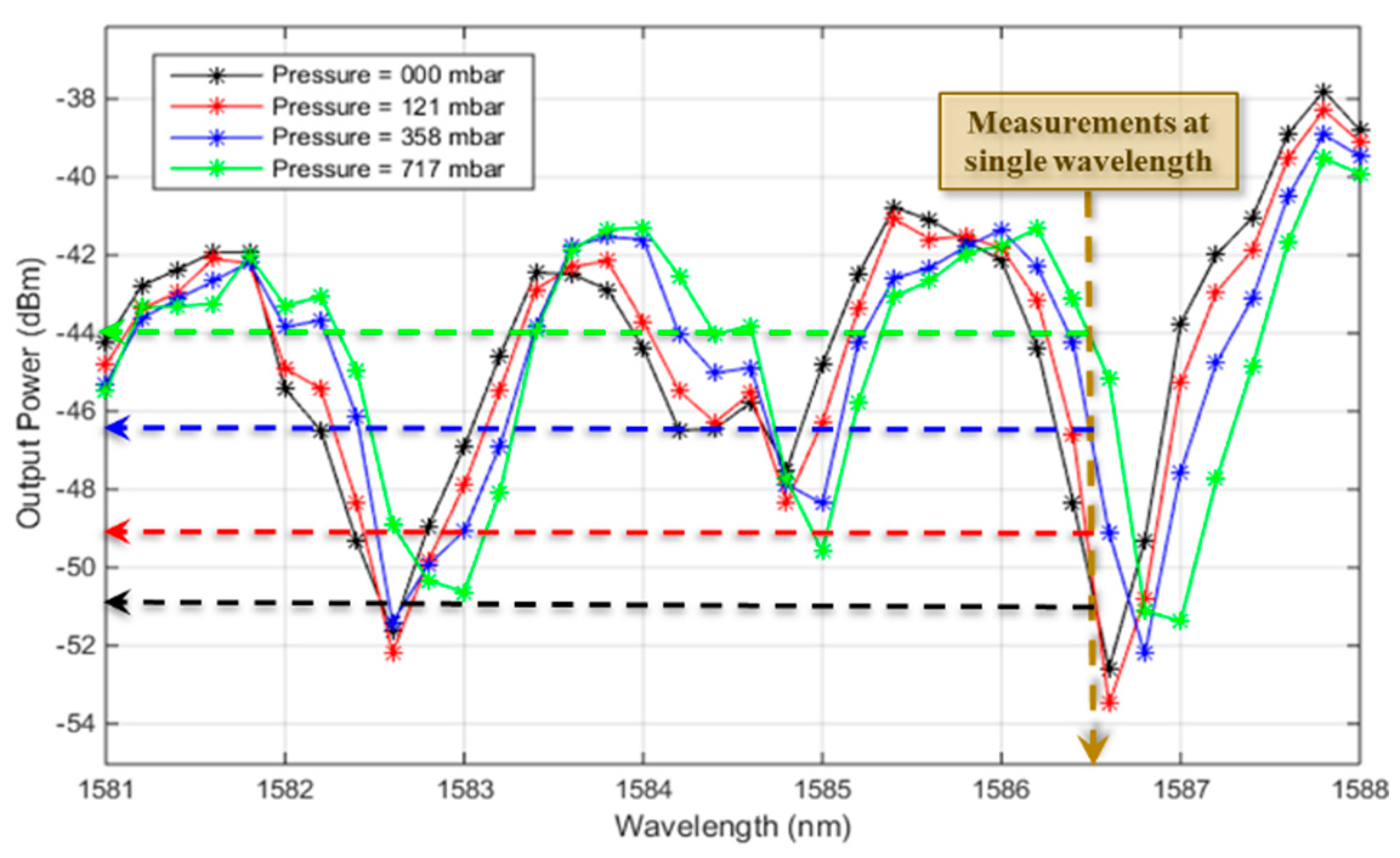
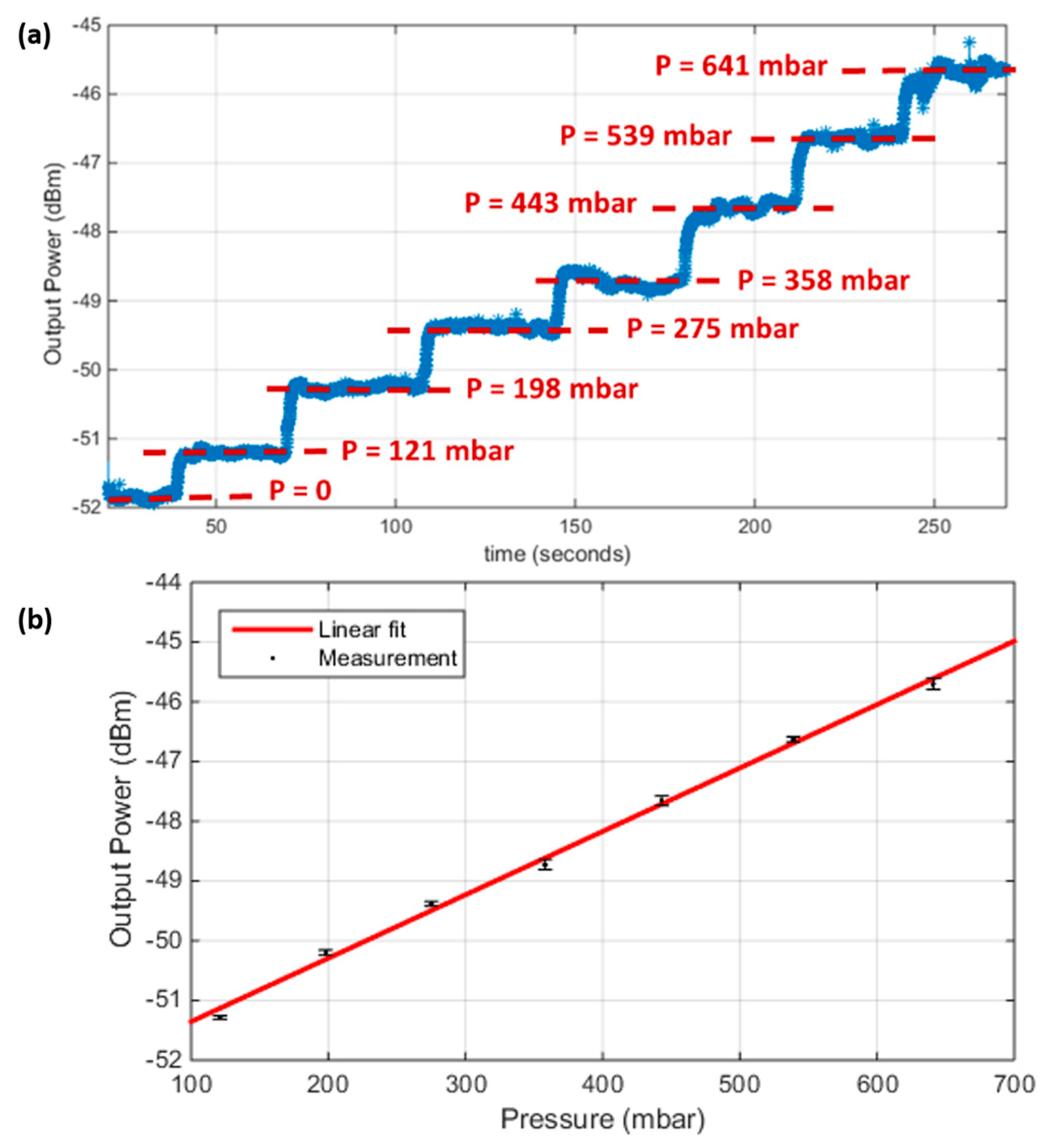
4. Results and Discussion
4.1. First Cavity
4.2. Second Cavity
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Eddy, D.S.; Sparks, D.R. Application of MEMS Technology in Automotive Sensors and Actuators. Proc. IEEE 1998, 86, 1747–1755. [Google Scholar] [CrossRef]
- Pramanik, C.; Saha, H.; Gangopadhyay, U. Design optimization of a high performance silicon MEMS piezoresistive pressure sensor for biomedical applications. J. Micromech. Microeng 2006, 16, 2060–2066. [Google Scholar] [CrossRef]
- Lin, L.; Yun, W. MEMS Pressure Sensors for Aerospace Applications. In Proceedings of the 1998 IEEE Aerospace Conference Proceedings (Cat. No.98TH8339), Snowmass at Aspen, CO, USA, 28 March 1998; pp. 429–436. [Google Scholar]
- Faivre, M.; Abkarian, M.; Bickraj, K.; Stone, H.A. Geometrical focusing of cells in a microfluidic device: an approach to separate blood plasma. Biorheology 2006, 43, 147–159. [Google Scholar] [PubMed]
- Abkarian, M.; Faivre, M.; Stone, H.A. High-speed microfluidic differential manometer for cellular-scale hydrodynamics. Proc. Natl. Acad. Sci. USA 2006, 103, 538–542. [Google Scholar] [CrossRef] [PubMed]
- Gervinskas, G.; Day, D.; Juodkazis, S. Optofluidic Fabry-Pérot sensor for water solutions at high flow rates. Opt. Mater. Express 2012, 2, 451–455. [Google Scholar] [CrossRef]
- Song, W.; Psaltis, D. Optofluidic membrane interferometer: An imaging method for measuring microfluidic pressure and flow rate simultaneously on a chip. Biomicrofluidics 2011, 5, 1–11. [Google Scholar] [CrossRef] [PubMed]
- Bae, H.; Zhang, X.M.; Liu, H.; Yu, M. Miniature surface-mountable Fabry-Perot pressure sensor constructed with a 45 degrees angled fiber. Opt. Lett. 2010, 35, 1701–1703. [Google Scholar] [CrossRef] [PubMed]
- Jung, T.; Yang, S. Highly stable liquid metal-based pressure sensor integrated with a microfluidic channel. Sensors 2015, 15, 11823–11835. [Google Scholar] [CrossRef] [PubMed]
- Cooksey, G.A.; Ahmed, Z. Optofluidic Temperature and Pressure Measurements with Fiber Bragg Gratings Embedded in Microfluidic Devices. In Proceedings of the TechConnect World Innovation Conference & Expo, Washington, DC, USA, 22–25 May 2016; pp. 8–10. [Google Scholar]
- Ganjalizadeh, V.; Veladi, H.; Yadipour, R. A novel pressure sensor based on optofluidic micro-ring resonator. In Proceedings of the 2014 International Conference on Optical MEMS and Nanophotonics, Glasgow, UK, 17–21 August 2014; pp. 133–134. [Google Scholar]
- Chin, L.K.; Liu, A.Q.; Lim, C.S.; Zhang, X.M.; Ng, J.H.; Hao, J.Z.; Takahashi, S. Differential single living cell refractometry using grating resonant cavity with optical trap. Appl. Phys. Lett. 2007, 91, 243901. [Google Scholar] [CrossRef]
- Gaber, N.; Malak, M.; Marty, F.; Angelescu, D.E.; Richalot, E.; Bourouina, T. Particles Optical Trapping and Binding in Optofluidic Stable Fabry–Pérot Resonator with Single-Sided Injection. Lab Chip 2014, 14, 2259–2265. [Google Scholar] [CrossRef] [PubMed]
- Gaber, N.; Sabry, Y.M.; Erfan, M.; Marty, F.; Bourouina, T. High-Q fabry-pérot micro-cavities for high-sensitivity volume refractometry. Micromachines 2018, 9, 54–63. [Google Scholar] [CrossRef] [PubMed]
- Dai, D.; He, S. Highly sensitive sensor based on an ultra-high-Q Mach–Zehnder interferometer-coupled microring. J. Opt. Soc. Am. B 2009, 26, 511–516. [Google Scholar] [CrossRef]
- Song, W.Z.; Zhang, X.M.; Liu, A.Q.; Lim, C.S. Refractive index measurement of single living cells using on-chip Fabry-Pérot cavity. Appl. Phys. Lett. 2006, 89, 203901. [Google Scholar] [CrossRef]
- Pang, C.; Bae, H.; Gupta, A.; Bryden, K.; Yu, M. MEMS Fabry-Perot sensor interrogated by optical system-on-a-chip for simultaneous pressure and temperature sensing. Opt. Express 2013, 21, 21829–21839. [Google Scholar] [CrossRef] [PubMed]
- Kim, H.-T.; Yu, M. High-speed optical sensor interrogator with a silicon-ring-resonator-based thermally tunable filter. Opt. Lett. 2017, 42, 1305–1308. [Google Scholar] [CrossRef] [PubMed]
- Song, W.; Psaltis, D. Optofluidic pressure sensor based on interferometric imaging. Opt. Lett. 2010, 35, 3604. [Google Scholar] [CrossRef] [PubMed]
- Wagner, D.; Frankenberger, J.; Deimel, P.P. Optical pressure sensor using two Mach-Zehnder interferometers for the TE and TM polarization. J. Micromech. Microeng. 1994, 4, 35. [Google Scholar] [CrossRef]
- Grattan, K.T.V.; Sun, T. Fiber optic sensor technology: An overview. Sens. Actuators A Phys. 2000, 82, 40–61. [Google Scholar] [CrossRef]
- Abeysinghe, D.C.; Dasgupta, S.; Boyd, J.T.; Jackson, H.E. A novel MEMS pressure sensor fabricated on an optical fiber. IEEE Photonics Technol. Lett. 2001, 13, 993–995. [Google Scholar] [CrossRef]
- Nesson, S.; Yu, M.; Zhang, X.; Hsieh, A.H. Miniature fiber optic pressure sensor with composite polymer- metal diaphragm for intradiscal pressure measurements. J. Biomed. Opt. 2008, 13, 044040. [Google Scholar] [CrossRef] [PubMed]
- Cheri, M.S.; Shahraki, H.; Sadeghi, J.; Moghaddam, M.S.; Latifi, H. Measurement and control of pressure driven flows in microfluidic devices using an optofluidic flow sensor. Biomicrofluidics 2014, 8, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Kim, Y.; Neikirk, D.P. Micromachined Fabry-Perot Cavity Pressure Transducer. IEEE Photonics Technol. Lett. 1995, 7, 1471–1473. [Google Scholar] [CrossRef]
- St-Gelais, R.; Masson, J.; Peter, Y.-A. All-silicon integrated Fabry–Pérot cavity for volume refractive index measurement in microfluidic systems. Appl. Phys. Lett. 2009, 94, 243905. [Google Scholar] [CrossRef]
- Li, B.; Lee, C. NEMS diaphragm sensors integrated with triple-nano-ring resonator. Sens. Actuators A Phys. 2011, 172, 61–68. [Google Scholar] [CrossRef]
- Cheng, X.; Hong, J.; Spring, A.M.; Yokoyama, S. Fabrication of a high-Q factor ring resonator using LSCVD deposited Si3N4 film. Opt. Mater. Express 2017, 7, 2182–2187. [Google Scholar] [CrossRef]
- Hallynck, E.; Bienstman, P. Integrated Optical Pressure Sensors in Silicon-on-Insulator. IEEE Photonics J. 2012, 4, 443–450. [Google Scholar] [CrossRef]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gaber, N.; Altayyeb, A.; Soliman, S.A.; Sabry, Y.M.; Marty, F.; Bourouina, T. On-Channel Integrated Optofluidic Pressure Sensor with Optically Boosted Sensitivity. Sensors 2019, 19, 944. https://doi.org/10.3390/s19040944
Gaber N, Altayyeb A, Soliman SA, Sabry YM, Marty F, Bourouina T. On-Channel Integrated Optofluidic Pressure Sensor with Optically Boosted Sensitivity. Sensors. 2019; 19(4):944. https://doi.org/10.3390/s19040944
Chicago/Turabian StyleGaber, Noha, Ahmad Altayyeb, Sherif A. Soliman, Yasser M. Sabry, Frédéric Marty, and Tarik Bourouina. 2019. "On-Channel Integrated Optofluidic Pressure Sensor with Optically Boosted Sensitivity" Sensors 19, no. 4: 944. https://doi.org/10.3390/s19040944
APA StyleGaber, N., Altayyeb, A., Soliman, S. A., Sabry, Y. M., Marty, F., & Bourouina, T. (2019). On-Channel Integrated Optofluidic Pressure Sensor with Optically Boosted Sensitivity. Sensors, 19(4), 944. https://doi.org/10.3390/s19040944





