Adaptive Controller Based on Spatial Disturbance Observer in a Microgravity Environment
Abstract
1. Introduction
- Static friction models, such as the Column–Viscous model and the Stribeck model.
1.1. Related Work
1.1.1. Friction Compensation
1.1.2. Sliding Mode Control
1.1.3. Disturbance Observer
1.2. Significance of This Paper
2. Materials and Methods
2.1. Dynamic Model
2.1.1. Ground Debugging Model
2.1.2. Space Experiment Model
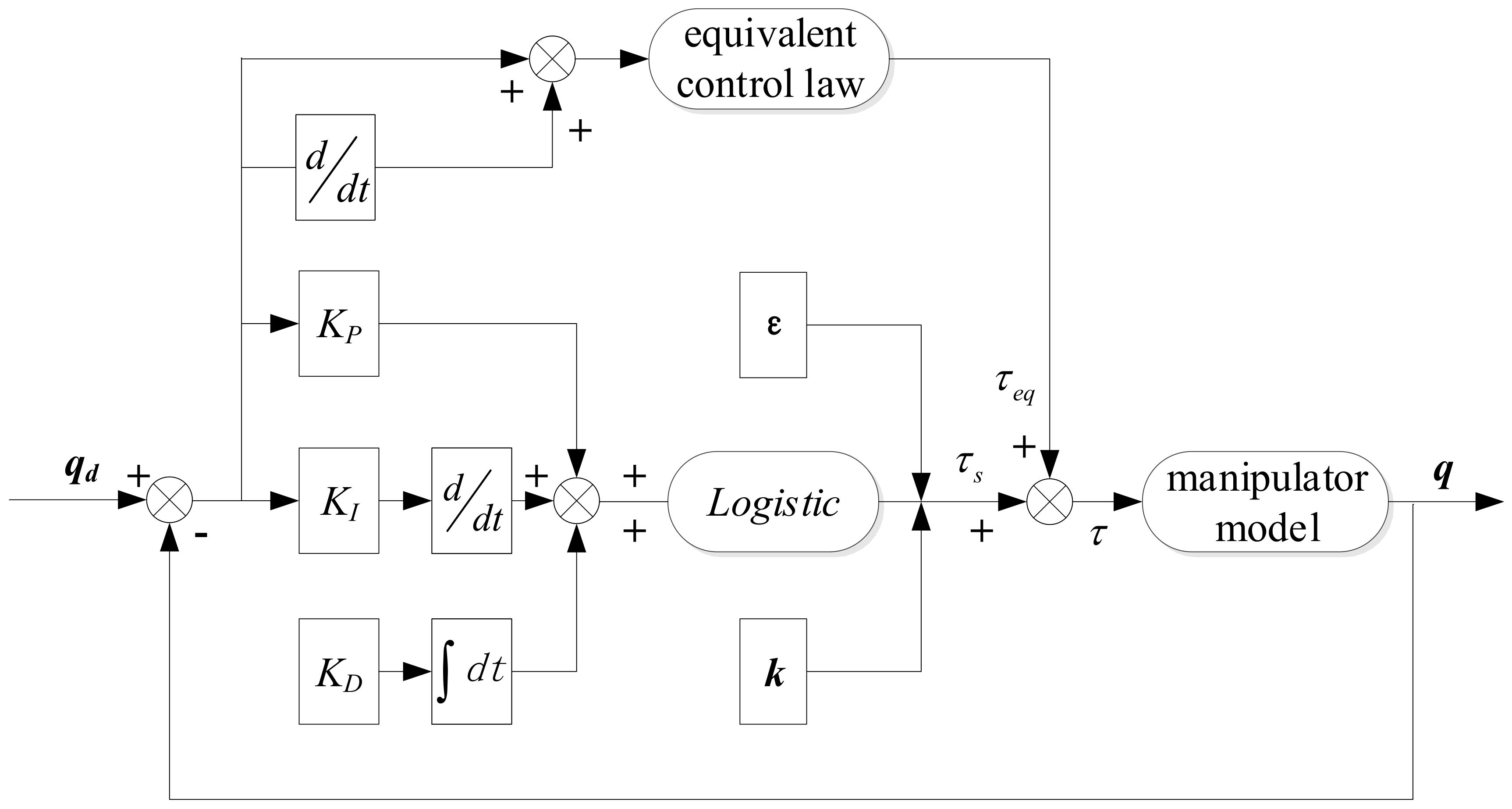
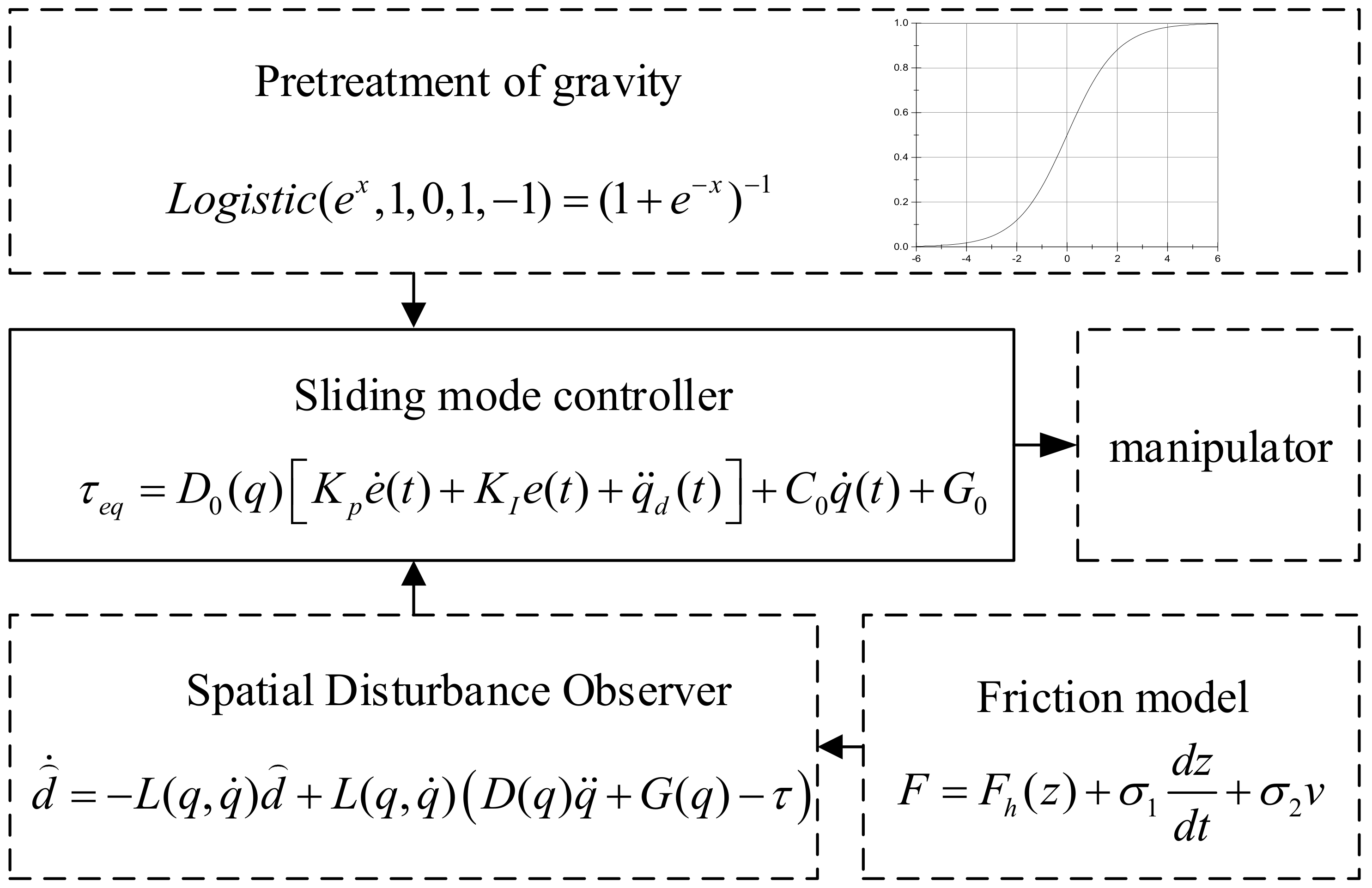
2.2. Design of Controller
2.2.1. Pretreatment of Gravity Term
2.2.2. Friction Compensation
2.3. Stability Analysis
Stability
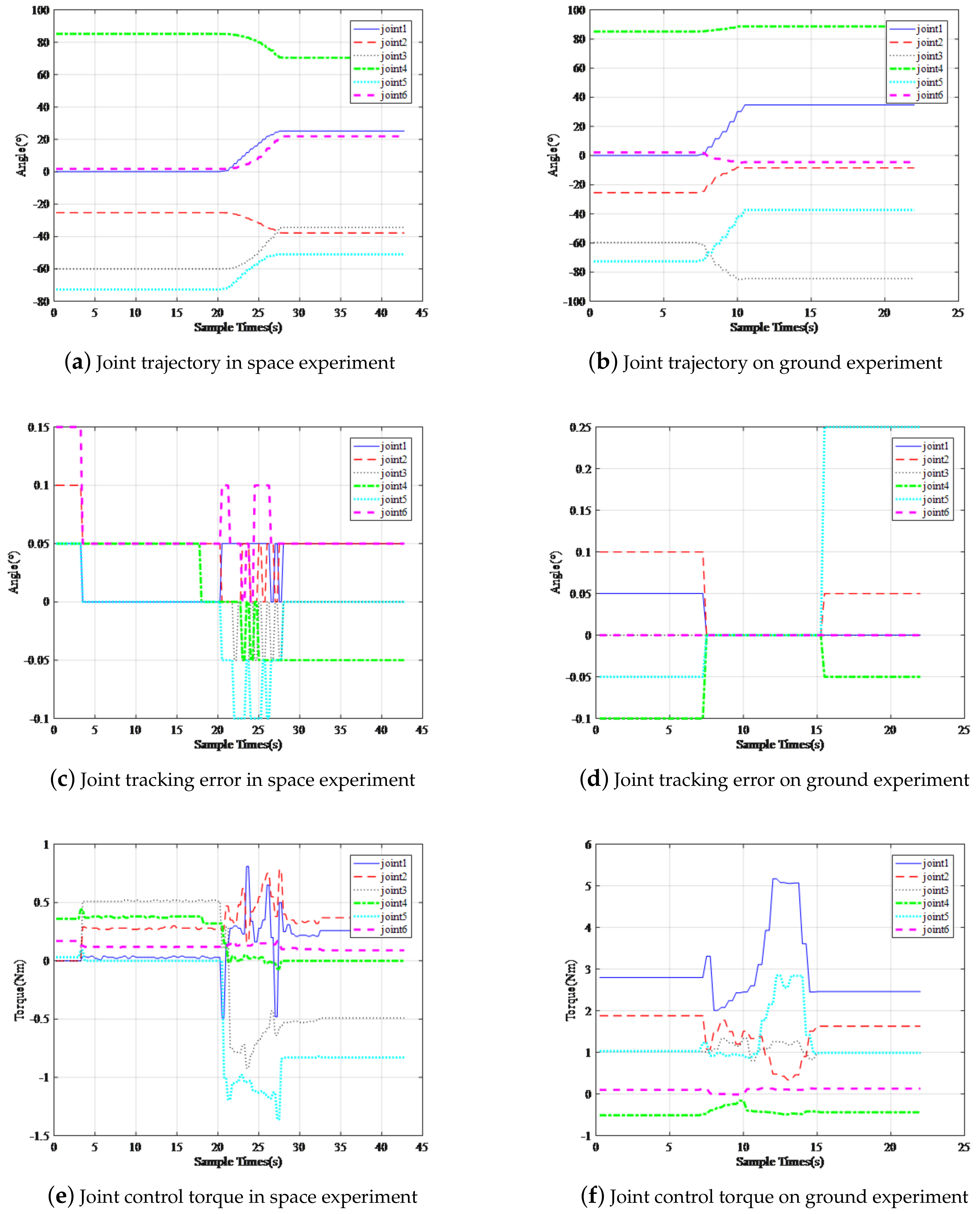
3. Experiment and Results
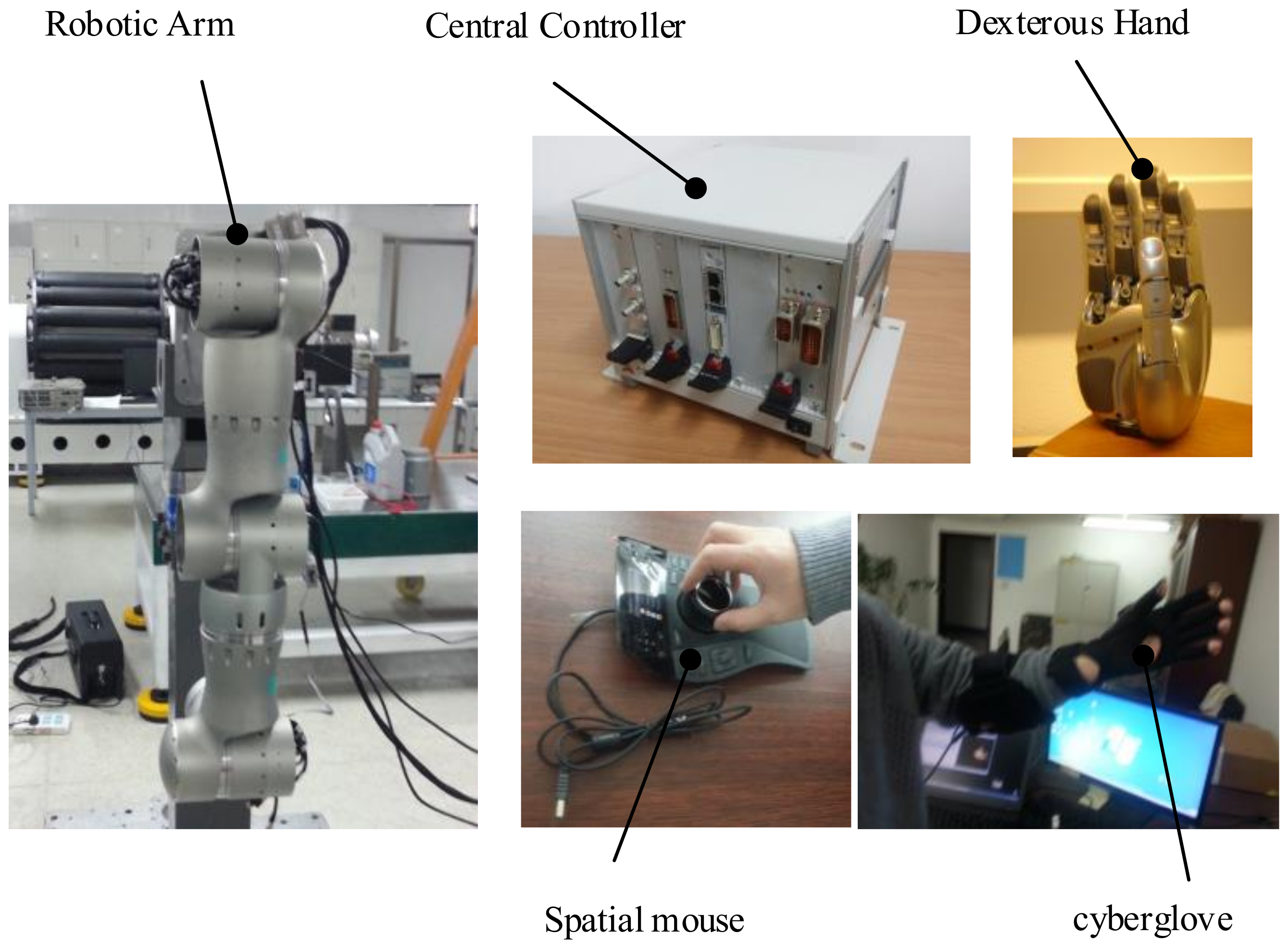
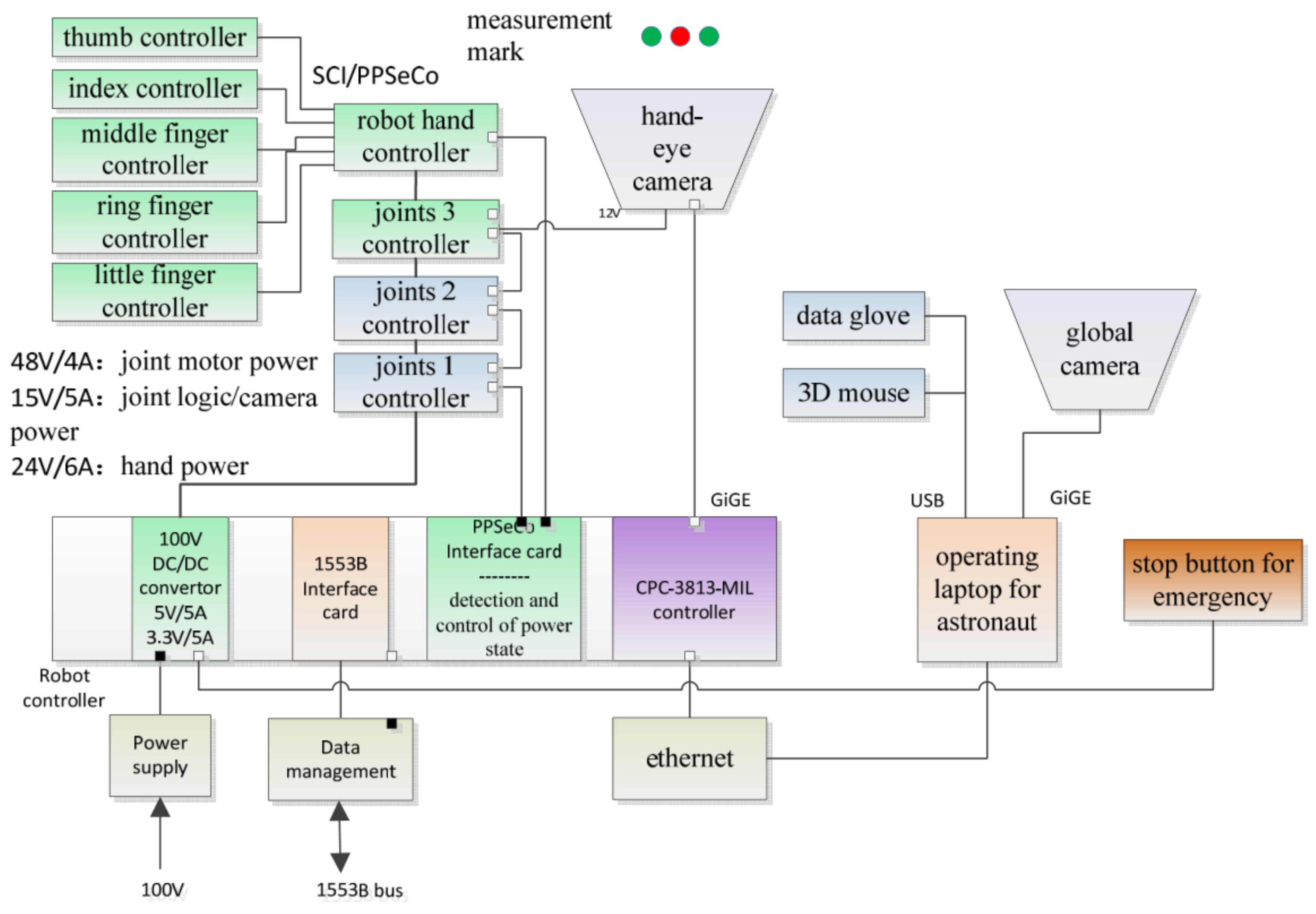
3.1. Experimental Platform
3.1.1. Overview of Space Robotic Arm-Hand System
3.1.2. Robotic Arm
3.1.3. Dexterous Hand
3.1.4. Teleoperation System
3.1.5. Central Controller
3.1.6. Visual System
3.2. Design of Experimental System
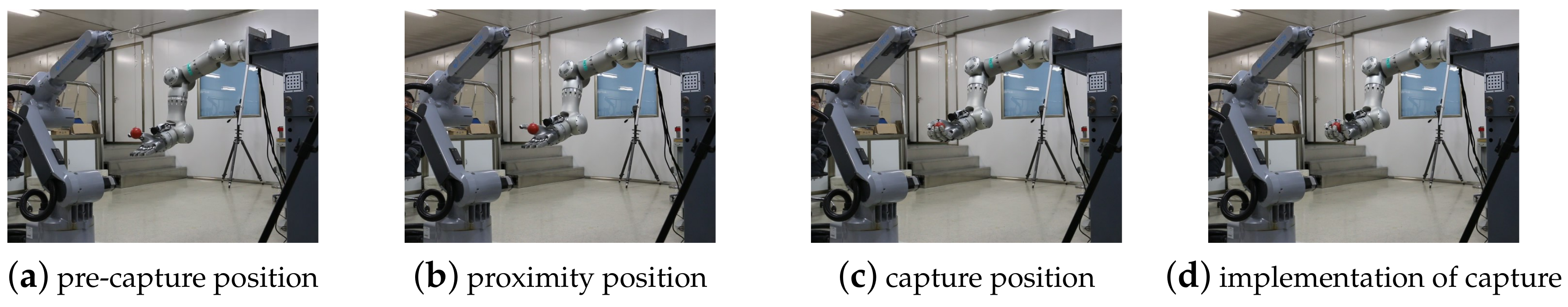
3.2.1. Capture Task
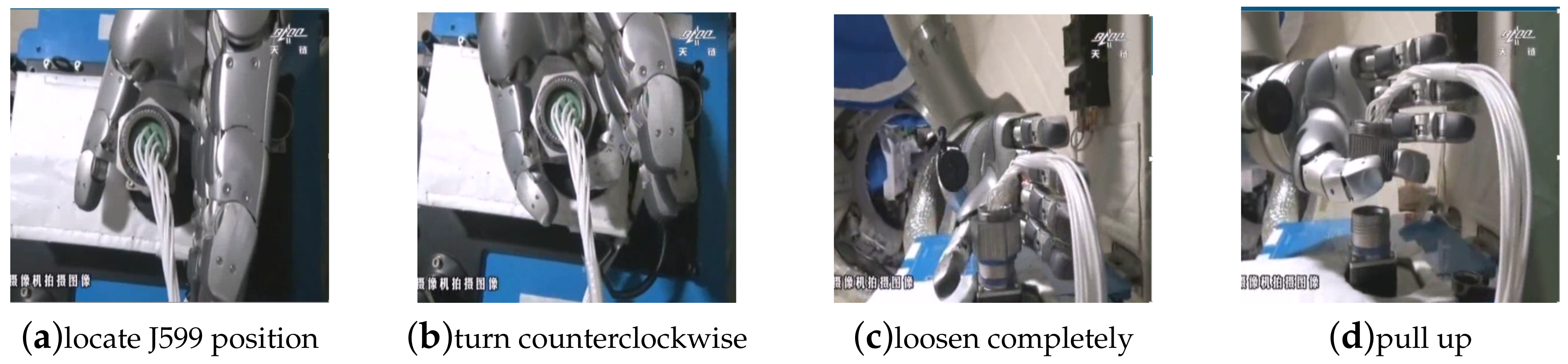
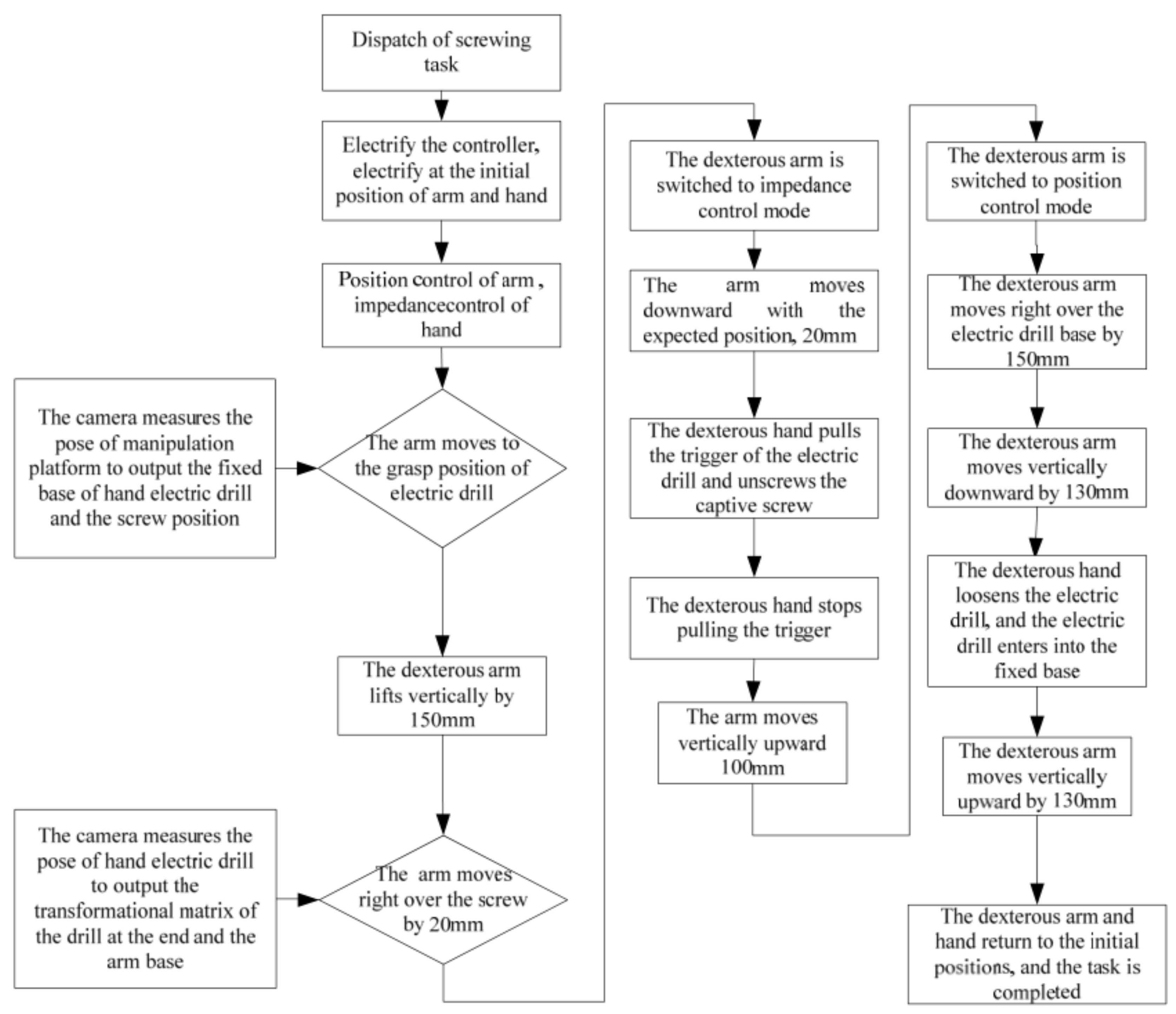
3.2.2. Maintenance Task
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
Abbreviations
| PPSeCo | Point-to-Point High Speed Serial Communication |
| FPGA | Very-High-Speed Integrated Circuit Hardware Description Language |
| SDO | Space Disturbance Observer |
| DSP | Digital Signal Processor |
| SMC | Sliding-mode Control |
References
- Rehnmark, F.; Bluethmann, W.; Mehling, J.; Ambrose, R.O.; Diftler, M.; Chu, M.; Necessary, R. Robonaut: The ‘short list’ of technology hurdles. Computer 2005, 38, 28–37. [Google Scholar] [CrossRef]
- Diftler, M.A.; Mehling, J.; Abdallah, M.E.; Radford, N.A.; Bridgwater, L.B.; Sanders, A.M.; Askew, R.S.; Linn, D.M.; Yamokoski, J.D.; Permenter, F. Robonaut 2—The first humanoid robot in space. In Proceedings of the IEEE International Conference on Robotics and Automation, Anchorage, Alaska, 3–8 May 2010. [Google Scholar]
- Brooks, R.A.; Breazeal, C.; Scassellati, B.; Williamson, M.M. The cog project: Building a humanoid robot. In Computation for Metaphors, Analogy, and Agents; Springer-Verlag: Berlin/Heidelberg, Germany, 1999. [Google Scholar]
- Han, J.D.; Zeng, S.Q.; Tham, K.Y.; Badgero, M.; Weng, J.Y. Dav: A humanoid robot platform for autonomous mental development. In Proceedings of the International Conference on Development and Learning, Cambridge, MA, USA, 12–15 June 2002. [Google Scholar]
- Edsinger-Gonzales, A.; Weber, J. Domo: A force sensing humanoid robot for manipulation research. In Proceedings of the IEEE/RAS International Conference on Humanoid Robots, Santa Monica, CA, USA, 10–12 November 2004. [Google Scholar]
- Borst, C.; Ott, C.; Wimbock, T.; Brunner, B. A humanoid upper body system for two-handed manipulation. In Proceedings of the IEEE International Conference on Robotics and Automation, Rome, Italy, 10–14 April 2007. [Google Scholar]
- Wimbock, T.; Nenchev, D.; Albu-Schaffer, A.; Hirzinger, G. Experimental study on dynamic reactionless motions with DLR’s humanoid robot Justin. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems, St. Louis, MO, USA, 11–15 October 2009. [Google Scholar]
- Jorgensen, G.; Bains, E. SRMS History, Evolution and Lessons Learned. In Proceedings of the Aiaa Space Conference and Exposition, Long Beach, CA, USA, 27–29 September 2013. [Google Scholar]
- Abramovici, A. The Special Purpose Dexterous Manipulator (SPDM) Systems Engineering Effort—A successful exercise in cheaper, faster and (hopefully) better systems engineering. J. Reduc. Space Mission Cost 1998, 1, 177–199. [Google Scholar] [CrossRef]
- Whelan, D.A.; Adler, E.A.; Wilson, S.B.; Roesler, G.M. DARPA Orbital Express program: Effecting a revolution in space-based systems. In Proceedings of the SPIE 4136, Small Payloads in Space, San Diego, CA, USA, 7 November 2000. [Google Scholar]
- Hirzinger, G.; Brunner, B.; Dietrich, J.; Heindl, J. ROTEX-the first remotely controlled robot in space. In Proceedings of the IEEE International Conference on Robotics and Automation, Washington, DC, USA, 10–17 May 2002. [Google Scholar]
- Susanto, W.; Babuška, R.; Liefhebber, F.; Weiden, T.V.D. Adaptive Friction Compensation: Application to a Robotic Manipulator. IFAC Proc. Vol. 2008, 41, 2020–2024. [Google Scholar] [CrossRef]
- Dahl, P.R. Measurement of Solid Friction Parameters of Ball Bearings. In Proceedings of the Annual Symposium on Incremental Motion Control Systems and Devices, Urbana, IL, USA, 24–27 May 1977. [Google Scholar]
- Canudas-De-Wit, C. Comments on “A new model for control of systems with friction”. IEEE Trans. Autom. Control 2002, 43, 1189–1190. [Google Scholar] [CrossRef]
- Chen, W.H.; Ballance, D.J.; Gawthrop, P.J.; O’Reilly, J. A Nonlinear Disturbance Observer for Robotic Manipulators. Ind. Electron. IEEE Trans. 2000, 47, 932–938. [Google Scholar] [CrossRef]
- Swevers, J.; Al-Bender, F.; Ganseman, C.G.; Projogo, T. An integrated friction model structure with improved presliding behavior for accurate friction compensation. IEEE Trans. Autom. Control 2000, 45, 675–686. [Google Scholar] [CrossRef]
- Wit, C.C.D.; Lischinsky, P. Adaptive Friction Compensation with Partially Known Dynamics Friction Model. Int. J. Adapt. Control Signal Process. 2015, 11, 65–80. [Google Scholar] [CrossRef]
- Canudas de Wit, C.; Noël, P.; Aubin, A.; Brogliato, B. Adaptive friction compensation in robot manipulators: Low-velocities. In Proceedings of the IEEE International Conference on Robotics and Automation, Sacramento, CA, USA, 9–11 April 1991. [Google Scholar]
- Yoo, B.K.; Ham, W.C. Adaptive control of robot manipulator using fuzzy compensator. Fuzzy Syst. IEEE Trans. 2000, 8, 186–199. [Google Scholar]
- Makkar, C.; Hu, G.; Sawyer, W.G.; Dixon, W.E. Lyapunov-Based Tracking Control in the Presence of Uncertain Nonlinear Parameterizable Friction. IEEE Trans. Autom. Control 2007, 52, 1988–1994. [Google Scholar] [CrossRef]
- Xie, W.F. Sliding-Mode-Observer-Based Adaptive Control for Servo Actuator With Friction. IEEE Trans. Ind. Electron. 2007, 54, 1517–1527. [Google Scholar] [CrossRef]
- Lu, L.; Yao, B.; Wang, Q.; Zheng, C. Adaptive Robust Control of Linear Motor Systems with Dynamic Friction Compensation Using Modified LuGre Model. In Proceedings of the IEEE/ASME International Conference on Advanced Intelligent Mechatronics, Xi’an, China, 2–5 July 2008. [Google Scholar]
- Jin, M.; Sang, H.K.; Chang, P.H. A Robust Compliant Motion Control of Robot with Certain Hard Nonlinearities Using Time Delay Estimation. In Proceedings of the IEEE International Symposium on Industrial Electronics, Vigo, Spain, 4–7 June 2007. [Google Scholar]
- Selmic, R.R.; Lewis, F.L. Neural-network approximation of piecewise continuous functions: Application to friction compensation. IEEE Trans. Neural Netw. 2002, 13, 745. [Google Scholar] [CrossRef] [PubMed]
- Armstrong, B.; Neevel, D.; Kusik, T. New results in NPID control: Tracking, integral control, friction compensation and experimental results. IEEE Trans. Control Syst. Technol. 1999, 9, 399–406. [Google Scholar] [CrossRef]
- Yao, J.; Deng, W.; Jiao, Z. Adaptive Control of Hydraulic Actuators With LuGre Model-Based Friction Compensation. IEEE Trans. Ind. Electron. 2015, 62, 6469–6477. [Google Scholar] [CrossRef]
- Han, S.I.; Lee, K.S. Robust friction state observer and recurrent fuzzy neural network design for dynamic friction compensation with backstepping control. Mechatronics 2010, 20, 384–401. [Google Scholar] [CrossRef]
- Han, M.K.; Park, S.H.; Han, S.I. Precise friction control for the nonlinear friction system using the friction state observer and sliding mode control with recurrent fuzzy neural networks. Mechatronics 2009, 19, 805–815. [Google Scholar]
- Makkar, C.; Dixon, W.E.; Sawyer, W.G.; Hu, G. A new continuously differentiable friction model for control systems design. In Proceedings of the IEEE/ASME International Conference on Advanced Intelligent Mechatronics, Monterey, CA, USA, 24–28 July 2005. [Google Scholar]
- Lee, W.; Lee, C.Y.; Jeong, Y.H.; Min, B.K. Friction compensation controller for load varying machine tool feed drive. Int. J. Mach. Tools Manuf. 2015, 96, 47–54. [Google Scholar] [CrossRef]
- Wu, S.; Wang, R.; Radice, G.; Wu, Z. Robust attitude maneuver control of spacecraft with reaction wheel low-speed friction compensation. Aerosp. Sci. Technol. 2015, 43, 213–218. [Google Scholar] [CrossRef]
- Draženović, B. The invariance conditions in variable structure systems. Automatica 1969, 5, 287–295. [Google Scholar] [CrossRef]
- Li, Q.; Liu, F.; Liang, L.; Gao, J. Fuzzy adaptive robust control for space robot considering the effect of the gravity. Chin. J. Aeronaut. 2014, 27, 1562–1570. [Google Scholar]
- Yu, S.; Yu, X.; Shirinzadeh, B.; Man, Z. Continuous finite-time control for robotic manipulators with terminal sliding mode. Automatica 2005, 41, 1957–1964. [Google Scholar] [CrossRef]
- Guo, Y.; Woo, P.Y. An adaptive fuzzy sliding mode controller for robotic manipulators. Syst. Man Cybern. Part A Syst. Hum. IEEE Trans. 2003, 33, 149–159. [Google Scholar]
- Hashimoto, H.; Maruyama, K.; Harashima, F. A Microprocessor-Based Robot Manipulator Control with Sliding Mode. IEEE Trans Ind. Electron. 1987, 34, 11–18. [Google Scholar] [CrossRef]
- Islam, S.; Liu, X.P. Robust Sliding Mode Control for Robot Manipulators. IEEE Trans. Ind. Electron. 2011, 58, 2444–2453. [Google Scholar] [CrossRef]
- Jafarov, E.M.; Parlakci, M.N.A.; Istefanopulos, Y. A new variable structure PID-controller design for robot manipulators. IEEE Trans. Control Syst. Technol. 2004, 13, 122–130. [Google Scholar] [CrossRef]
- Nazari, I.; Hosainpour, A.; Emamzadeh, S.; Mirzaie, M.; Piltan, F. Design Sliding Mode Controller with Parallel Fuzzy Inference System Compensator to Control of Robot Manipulator. Int. J. Intell. Syst. Appl. 2014, 6, 63–75. [Google Scholar] [CrossRef][Green Version]
- Wai, R.J.; Muthusamy, R. Fuzzy-neural-network inherited sliding-mode control for robot manipulator including actuator dynamics. IEEE Trans. Neural Netw. Learn. Syst. 2013, 24, 274–287. [Google Scholar]
- Chen, Z.; Zhang, J.; Wang, Z.; Zeng, J. Sliding Mode Control of Robot Manipulators Based on Neural Network Reaching Law. In Proceedings of the IEEE International Conference on Control and Automation, Guangzhou, China, 30 May–1 June 2007. [Google Scholar]
- Capisani, L.M.; Ferrara, A. Trajectory Planning and Second-Order Sliding Mode Motion/Interaction Control for Robot Manipulators in Unknown Environments. IEEE Trans. Ind. Electron. 2012, 59, 3189–3198. [Google Scholar] [CrossRef]
- Li, F.; Wu, L.; Shi, P.; Lim, C.C. State estimation and sliding mode control for semi-Markovian jump systems with mismatched uncertainties. Automatica 2015, 51, 385–393. [Google Scholar] [CrossRef]
- Li, H.; Shi, P.; Yao, D.; Wu, L. Observer-based adaptive sliding mode control for nonlinear Markovian jump systems. Automatica 2016, 64, 133–142. [Google Scholar] [CrossRef]
- Rabiee, H.; Ataei, M.; Ekramian, M. Continuous nonsingular terminal sliding mode control based on adaptive sliding mode disturbance observer for uncertain nonlinear systems. Automatica 2019, 109. [Google Scholar] [CrossRef]
- Lai, J.; Yin, X.; Jiang, L.; Yin, X.; Wang, Z.; Ullah, Z. Disturbance-Observer-Based PBC for Static Synchronous Compensator Under System Disturbances. IEEE Trans. Power Electron. 2019, 34, 11467–11481. [Google Scholar] [CrossRef]
- Mi, Y.; Song, Y.; Fu, Y.; Su, X.; Wang, C.; Wang, J. Frequency and Voltage Coordinated Control for Isolated Wind–Diesel Power System Based on Adaptive Sliding Mode and Disturbance Observer. IEEE Trans. Sustain. Energy 2019, 10, 2075–2083. [Google Scholar] [CrossRef]
- Zhang, M.; Zhou, M.; Liu, H.; Zhang, B.; Zhang, Y.; Chu, H. Friction compensation and observer-based adaptive sliding mode control of electromechanical actuator. Adv. Mech. Eng. 2018, 10. [Google Scholar] [CrossRef]
- Liu, X.; Li, K. A novel sliding mode single-loop speed control method based on disturbance observer for permanent magnet synchronous motor drives. Adv. Mech. Eng. 2018, 10. [Google Scholar] [CrossRef]
- Dev, A.; Léchappé, V.; Sarkar, M.K. Prediction-based super twisting sliding mode load frequency control for multi-area interconnected power systems with state and input time delays using disturbance observer. Int. J. Control 2019, 1–14. [Google Scholar] [CrossRef]
- Armstrong-Hélouvry, B. Control of Machines with Friction; Springer: New York, NY, USA, 1991. [Google Scholar]
- Mayergoyz, I.D. Mathematical Models of Hysteresis; Springer: New York, NY, USA, 1991. [Google Scholar]
- Futami, S.; Furutani, A.; Yoshida, S. Nanometer positioning and its micro-dynamics. Nanotechnology 1990, 1, 31. [Google Scholar] [CrossRef]
- Li, C.B.; Pavelescu, D. The friction-speed relation and its influence on the critical velocity of stick-slip motion. Wear 1982, 82, 277–289. [Google Scholar]
- Liu, H.; Yu, H.; Systems, C. Finite-Time Control of Continuous-Time Networked Dynamical Systems. IEEE Trans. Syst. Man Cybern. Syst. 2018, 1–10. [Google Scholar] [CrossRef]
- Liu, H.B.; Wang, D.Q. Stability and stabilisation of a class of networked dynamic systems. Int. J. Syst. Sci. 2018, 49, 964–973. [Google Scholar] [CrossRef]





| No. | Sensor Type | Amount of Joints | Measurement Principle |
|---|---|---|---|
| 1 | Joint torque | 1 | Strain |
| 2 | Joint position | 1 | Magnetic encoder |
| 3 | Motor end position | 1 | Rotary transformer |
| 4 | Motor position | 3 | Digital hall |
| 5 | Current sensor | 2 | Resistance drop |
| 6 | Temperature sensor | 1 | Thermometer |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, C.; Xie, Z.; Liu, Y.; Li, C.; Liu, H. Adaptive Controller Based on Spatial Disturbance Observer in a Microgravity Environment. Sensors 2019, 19, 4759. https://doi.org/10.3390/s19214759
Fan C, Xie Z, Liu Y, Li C, Liu H. Adaptive Controller Based on Spatial Disturbance Observer in a Microgravity Environment. Sensors. 2019; 19(21):4759. https://doi.org/10.3390/s19214759
Chicago/Turabian StyleFan, Chunguang, Zongwu Xie, Yiwei Liu, Chongyang Li, and Hong Liu. 2019. "Adaptive Controller Based on Spatial Disturbance Observer in a Microgravity Environment" Sensors 19, no. 21: 4759. https://doi.org/10.3390/s19214759
APA StyleFan, C., Xie, Z., Liu, Y., Li, C., & Liu, H. (2019). Adaptive Controller Based on Spatial Disturbance Observer in a Microgravity Environment. Sensors, 19(21), 4759. https://doi.org/10.3390/s19214759





