Power Pattern Sensitivity to Calibration Errors and Mutual Coupling in Linear Arrays through Circular Interval Arithmetics
Abstract
:1. Introduction
2. Mathematical Formulation
3. Numerical Results
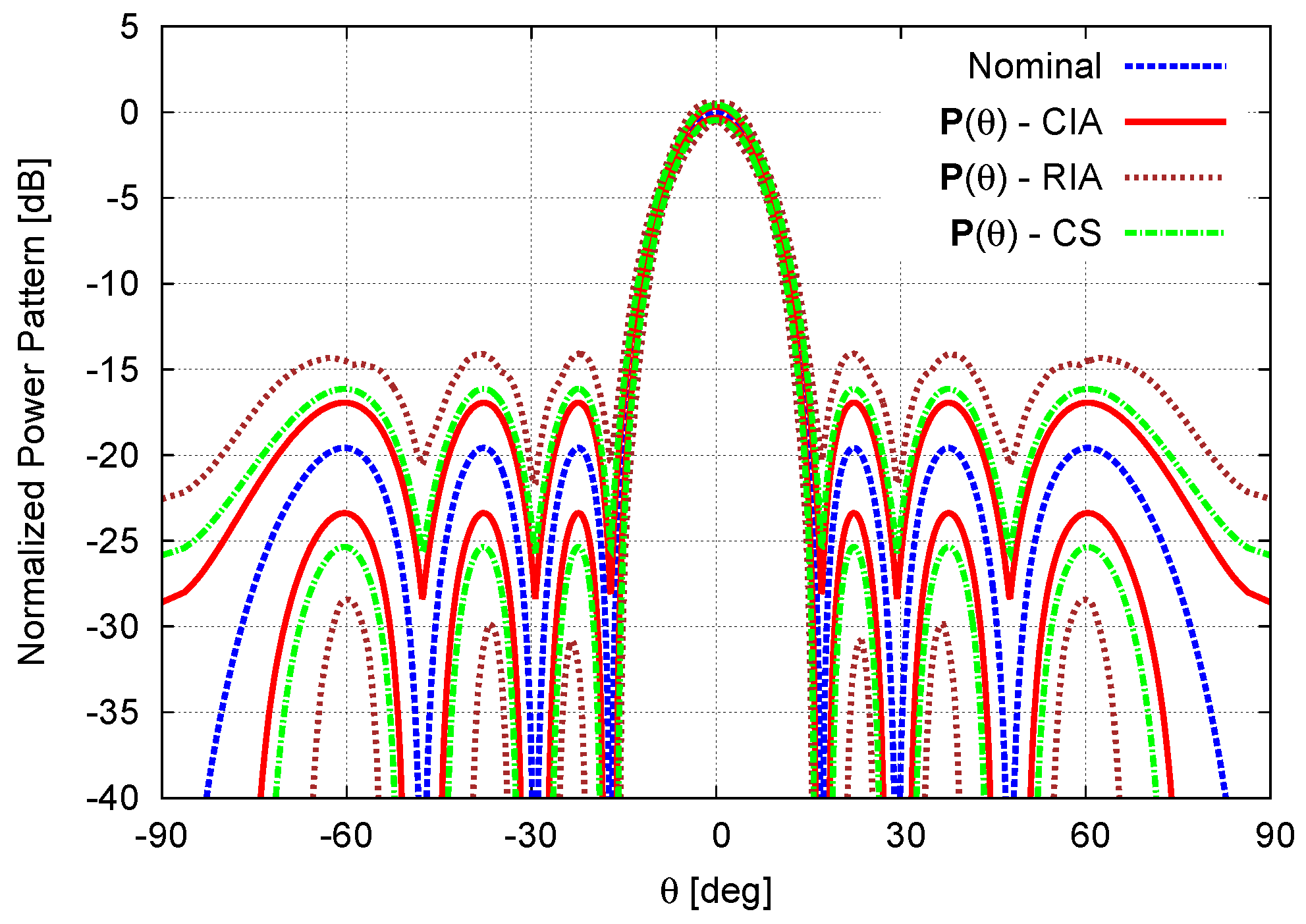
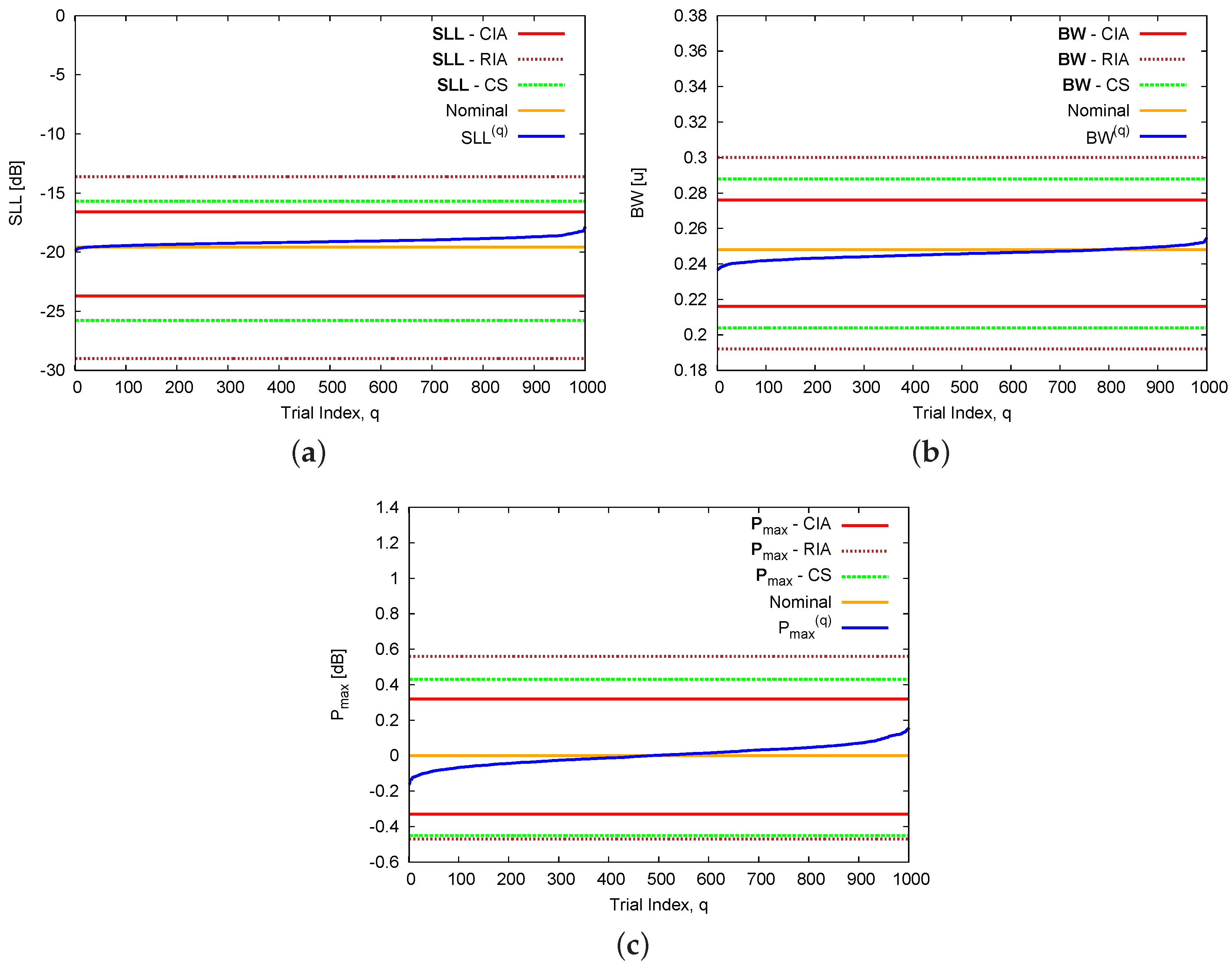
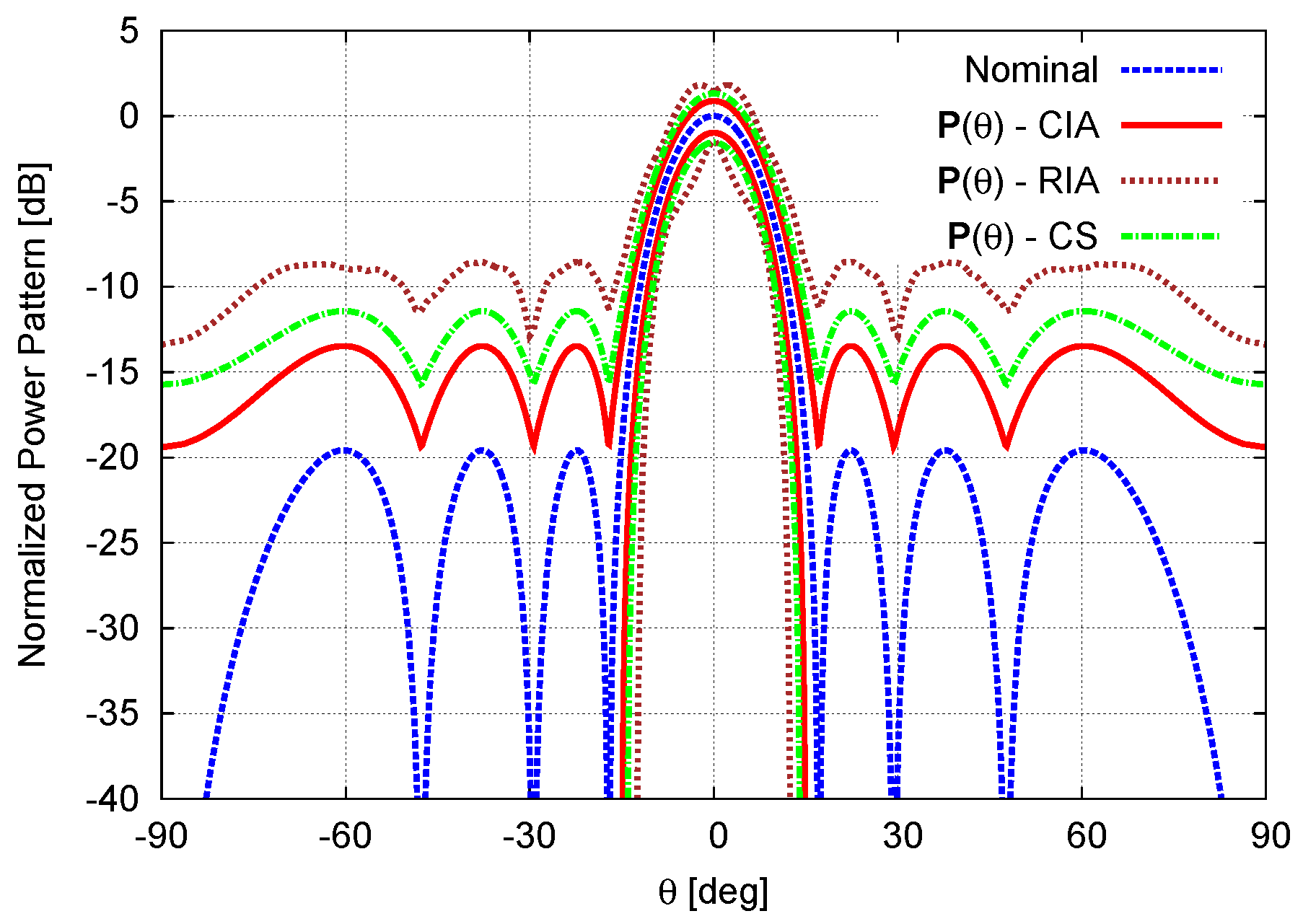
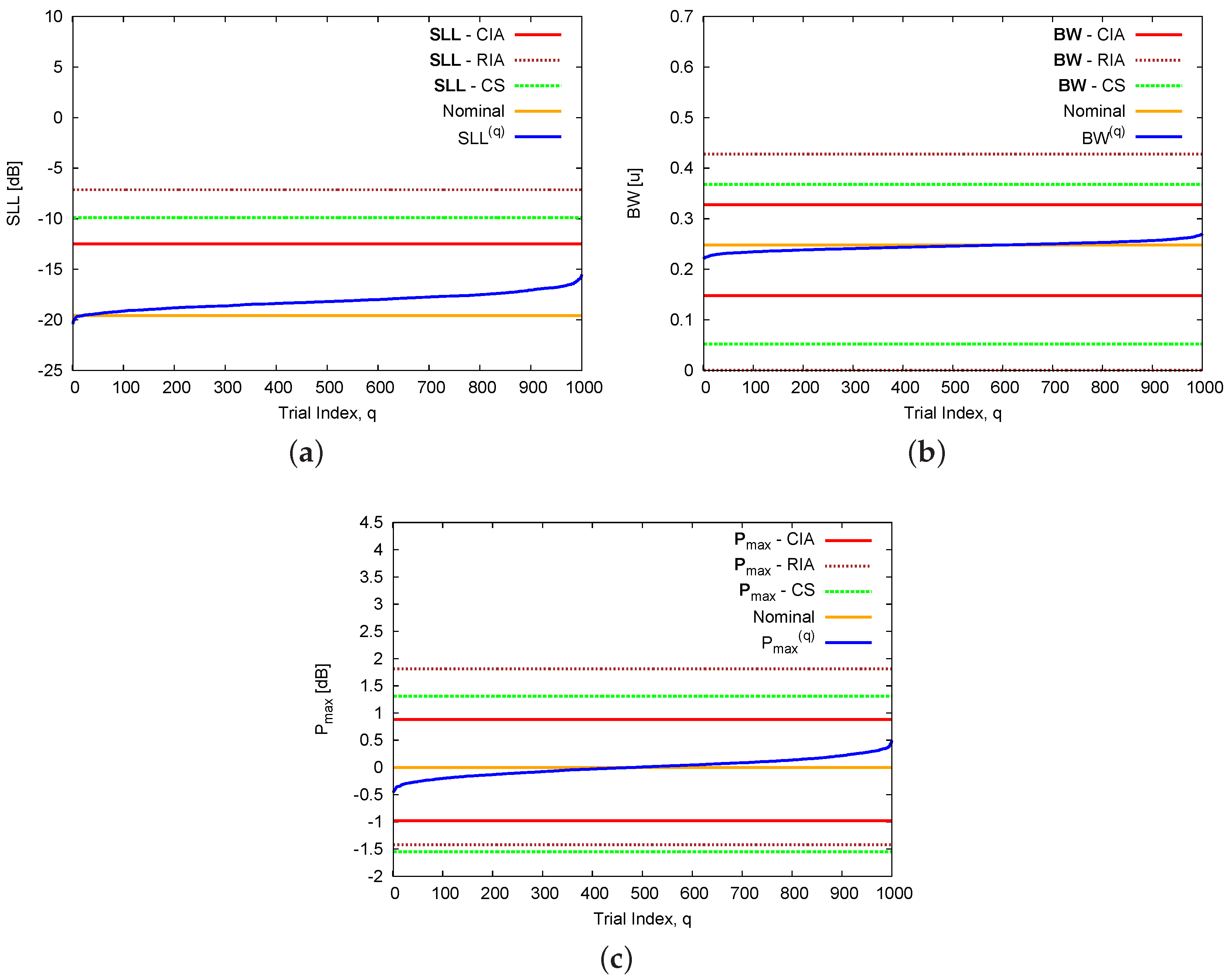
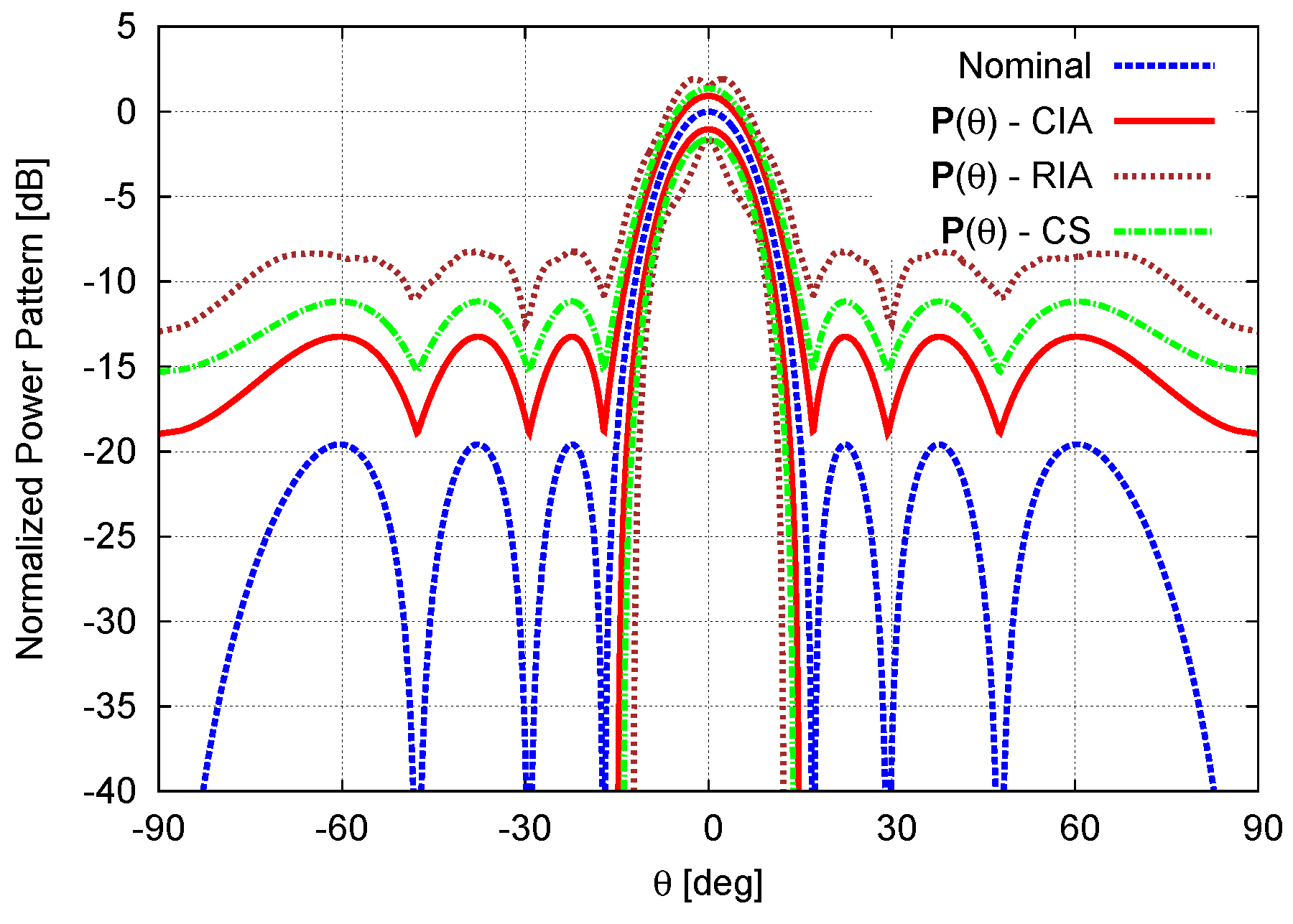
3.1. Validation and Comparative Assessment
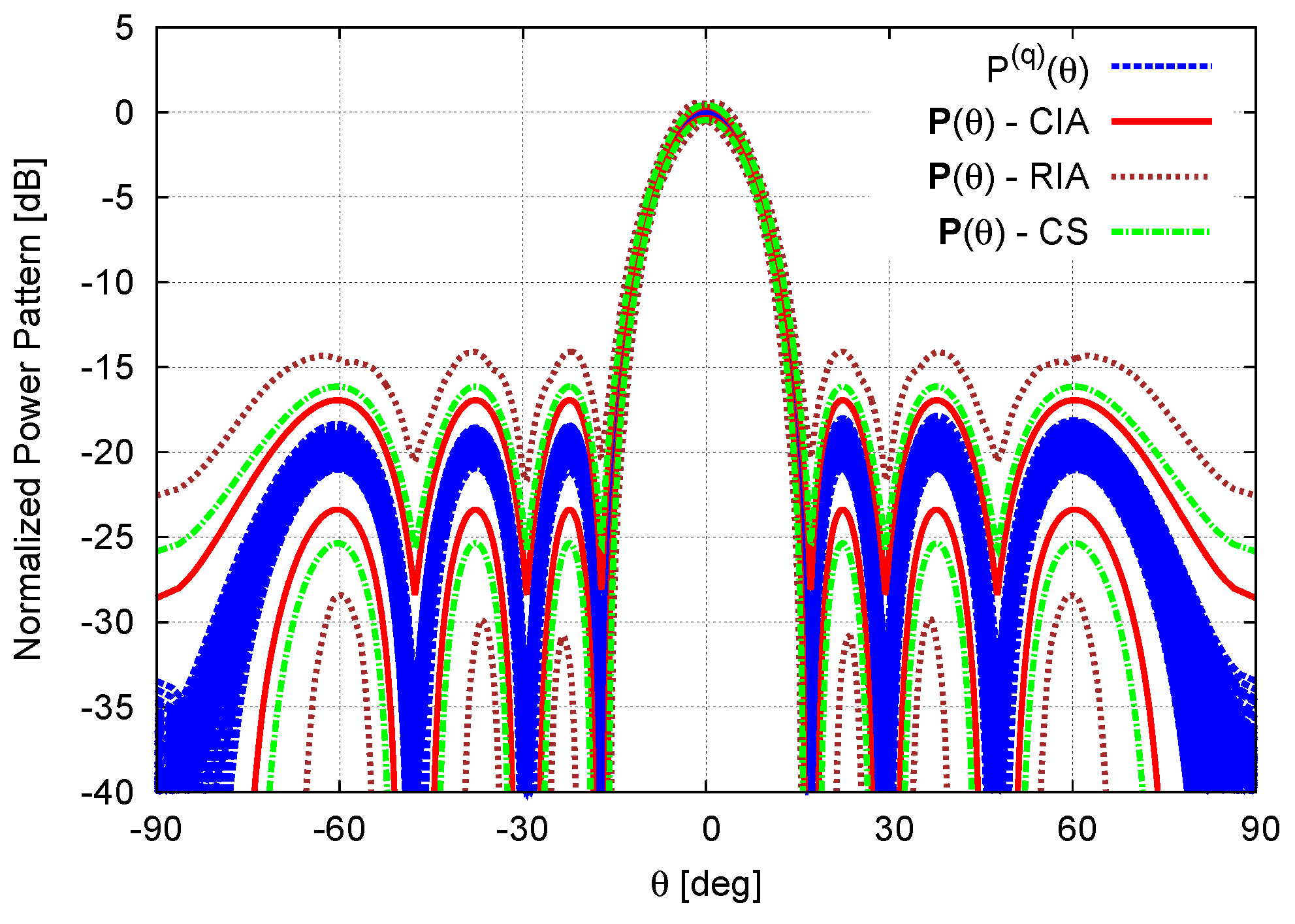
3.2. Prediction Accuracy Evaluation
4. Conclusions
- to the best of the authors’ knowledge, the first time exploitation of the CIA for the sensitivity analysis in antenna arrays when uncertainties and mutual coupling arise in complex (i.e., amplitude and phase) excitations coefficients;
- a compact and efficient definition of complex intervals in terms of their barycenters (i.e., nominal/uncertainty-free values) and radii (i.e., maximum amplitude deviations);
- the definition of analytic power pattern bounds requiring neither the knowledge nor an estimation of the phase deviations or uncertainties, but only based on the value of the nominal array factor and the maximum amplitude error.
- the CIA bounds are accurate and reliable as well as inclusive;
- the CIA-based tool provides a more accurate worst-case prediction of the power pattern tolerances since the CIA bounds turn out to be narrower than those from [16] and the RIA;
- the CIA approach allows one a faithful a priori estimation of the behavior of actual power pattern in the high-energy angular regions.
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| CIA | Circular Interval Analysis |
| CS | Cauchy–Schwartz |
| IA | Interval Analysis |
| MC | Mutual Coupling |
| RIA | Rectangular Interval Analysis |
Appendix A. Circular Interval Definition
Appendix B. Circular Interval Arithmetics
Appendix B.1. Product between Circular Intervals and Complex Numbers
Appendix B.2. Sum of Circular Intervals
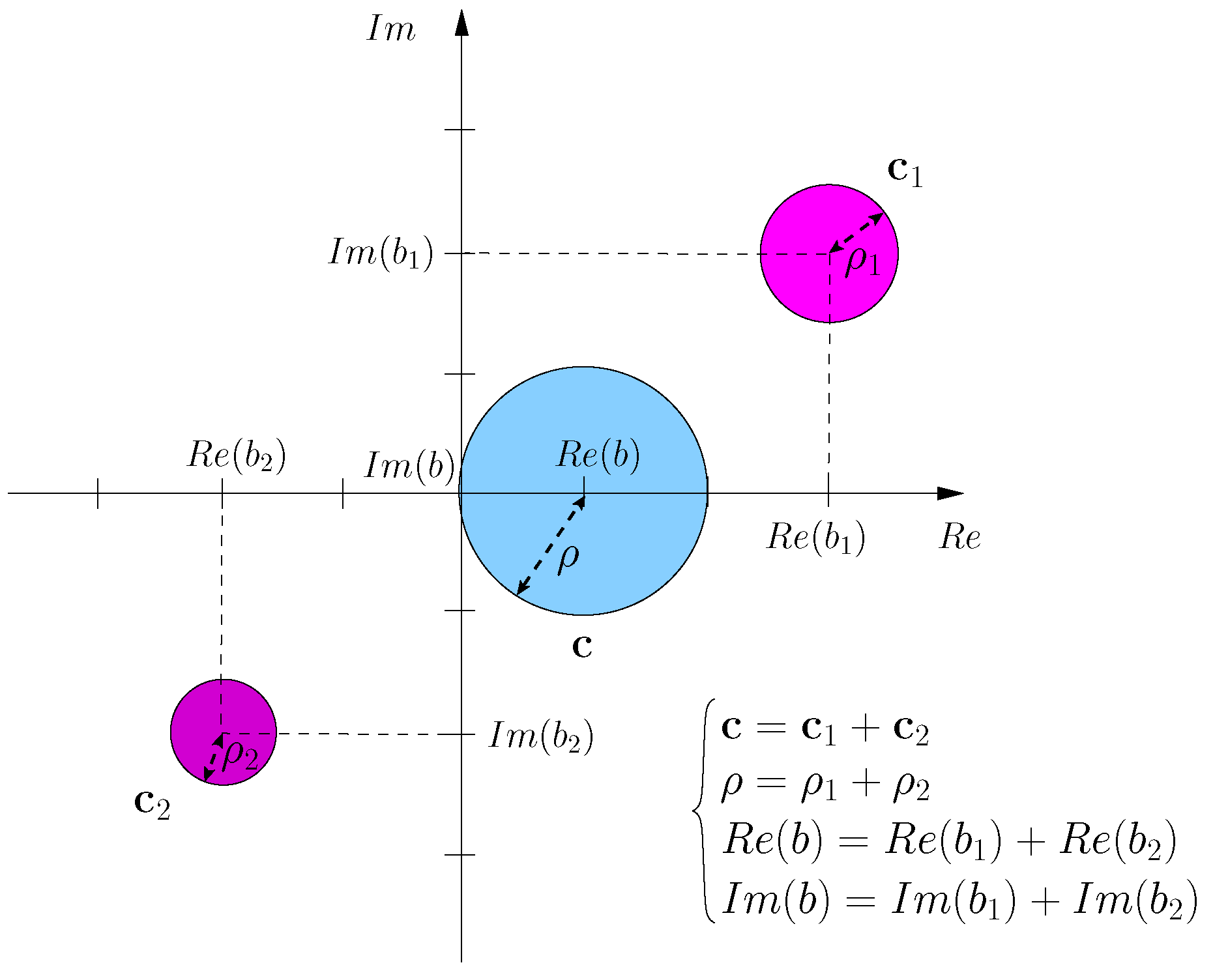
Appendix B.3. Module of a Circular Interval
References
- Mailloux, R.J. Phased Array Antenna Handbook, 2nd ed.; Artech House: Norwood, MA, USA, 2005. [Google Scholar]
- Van Trees, H.L. Optimum Array Processing (Part IV); Wiley & Sons: New York, NY, USA, 2002. [Google Scholar]
- Elliott, R.S. Antenna Theory and Design, 2nd ed.; Wiley & Sons: Hoboken, NJ, USA, 2003. [Google Scholar]
- Ruze, J. The effect of aperture errors on the antenna radiation pattern. Nuovo Cimento 1952, 9, 364–380. [Google Scholar] [CrossRef]
- Elliott, R.E. Mechanical and electrical tolerances for two-dimensional scanning antenna arrays. IRE Trans. 1958, 6, 114–120. [Google Scholar] [CrossRef]
- Lee, J.; Lee, Y.; Kim, H. Decision of error tolerance in array element by the Monte Carlo method. IEEE Trans. Antennas Propag. 2005, 53, 1325–1331. [Google Scholar]
- De Menezes, L.R.A.X.; Soares, A.J.M.; Silva, F.C.; Terada, M.A.B.; Correia, D. A new procedure for assessing the sensitivity of antennas using the unscented transform. IEEE Trans. Antennas Propag. 2010, 58, 988–993. [Google Scholar] [CrossRef]
- Gupta, I.J.; Ksienski, A.A. Effect of mutual coupling on the performance of adaptive arrays. IEEE Trans. Antennas Propag. 1983, 31, 785–791. [Google Scholar] [CrossRef]
- Liao, B.; Chan, S.-C. Adaptive beamforming for uniform linear arrays with unknown mutual coupling. IEEE Antennas Wirel. Propag. Lett. 2012, 11, 464–467. [Google Scholar] [CrossRef]
- Liao, B.; Chan, S.-C. A cumulant-based method for direction finding in uniform linear array with mutual coupling. IEEE Antennas Wirel. Propag. Lett. 2014, 13, 1717–1720. [Google Scholar] [CrossRef]
- Su, T.; Ling, H. On modeling mutual coupling in antenna arrays using the coupling matrix. Microw. Opt. Technpl. Lett. 2001, 28, 231–237. [Google Scholar] [CrossRef]
- Pozar, D.M. Input impedance and mutual coupling of rectangular microstrip antennas. IEEE Trans. Antennas Propag. 1982, 30, 1191–1196. [Google Scholar] [CrossRef]
- Adve, R.S.; Sarkar, T.K. Compensation for the effects of mutual coupling on direct data domain adaptive algorithms. IEEE Trans. Antennas Propag. 2000, 48, 86–94. [Google Scholar] [CrossRef]
- Steyskal, H.; Herd, J.S. Mutual coupling compensation in small array antennas. IEEE Trans. Antennas Propag. 1990, 38, 1971–1975. [Google Scholar] [CrossRef]
- Balanis, C.A. Antenna Theory: Analysis and Design; Wiley & Sons: Hoboken, NJ, USA, 2005. [Google Scholar]
- Schmid, C.M.; Schuster, S.; Feger, R.; Stelzer, A. On the effects of calibration errors and mutual coupling on the beam pattern of an antenna array. IEEE Trans. Antennas Propag. 2013, 61, 4063–4072. [Google Scholar] [CrossRef]
- Moore, R.E. Interval Analysis; Prentice-Hall: Englewood Cliffs, NJ, USA, 1966. [Google Scholar]
- Anselmi, N.; Manica, L.; Rocca, P.; Massa, A. Tolerance analysis of antenna arrays through interval arithmetic. IEEE Trans. Antennas Propag. 2013, 61, 5496–5507. [Google Scholar] [CrossRef]
- Rocca, P.; Manica, L.; Anselmi, N.; Massa, A. Analysis of the pattern tolerance in linear arrays with arbitrary amplitude errors. IEEE Antennas Wirel. Propag. Lett. 2013, 12, 639–642. [Google Scholar] [CrossRef]
- Manica, L.; Anselmi, N.; Rocca, P.; Massa, A. Robust mask-constrained linear array synthesis through an interval-based Particle Swarm Optimization. IET Microw. Antennas Propag. 2013, 7, 976–984. [Google Scholar] [CrossRef]
- Poli, L.; Rocca, P.; Anselmi, N.; Massa, A. Dealing with uncertainties on phase weighting of linear antenna arrays by means of interval-based tolerance analysis. IEEE Trans. Antennas Propag. 2015, 63, 3229–3234. [Google Scholar] [CrossRef]
- Anselmi, N.; Rocca, P.; Salucci, M.; Massa, A. Optimization of excitation tolerances for robust beamforming in linear arrays. IET Microw. Antennas Propag. 2016, 10, 208–214. [Google Scholar] [CrossRef]
- Rocca, P.; Anselmi, A.; Massa, A. Interval arithmetic for pattern tolerance analysis of parabolic reflectors. IEEE Trans. Antennas Propag. 2014, 62, 4952–4960. [Google Scholar] [CrossRef]
- Rocca, P.; Manica, L.; Massa, A. Interval-based analysis of pattern distortions in reflector antennas with bump-like surface deformations. IET Microw. Antennas Propag. 2014, 8, 1277–1285. [Google Scholar] [CrossRef]
- Hansen, E.; Walster, G.W. Global Optimization Using Interval Analysis; CRC Press: New York, NY, USA, 2004. [Google Scholar]
- Gargantini, I.; Henrici, P. Circular arithmetic and the determination of polynomial zeros. Numer. Math. 1972, 18, 305–320. [Google Scholar] [CrossRef]
- FEKO. Available online: https://www.feko.info/ (accessed on 27 May 2016).

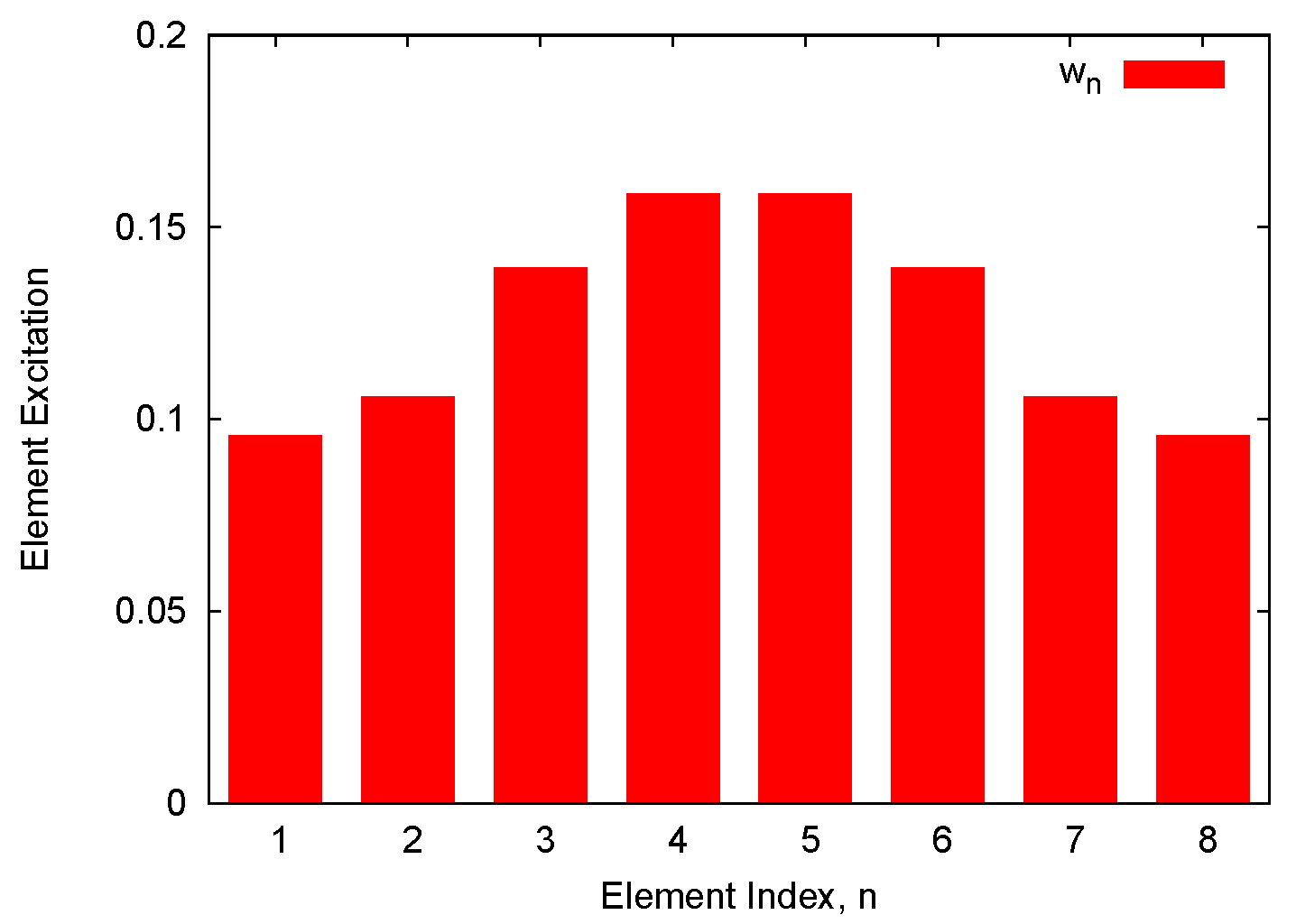
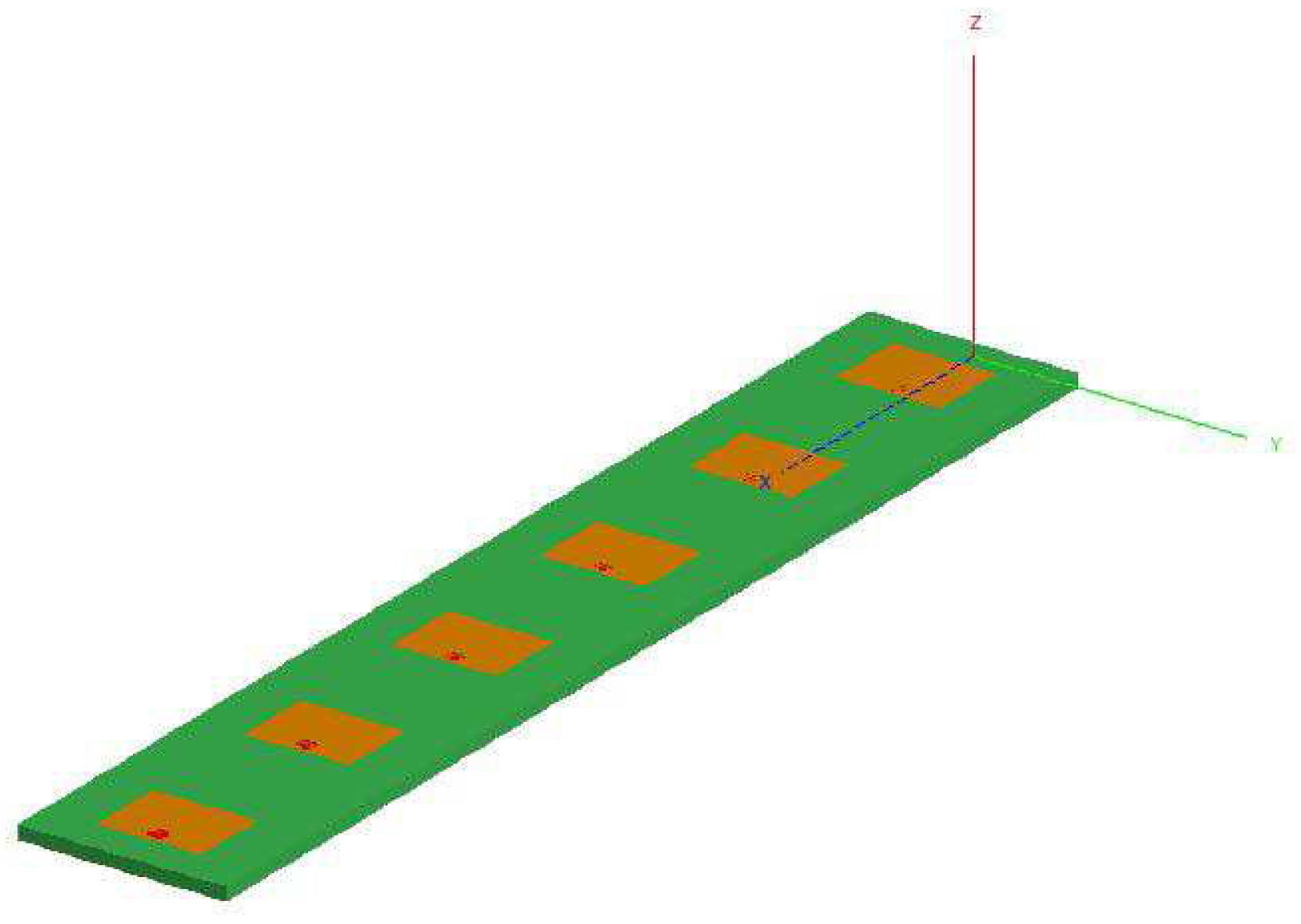
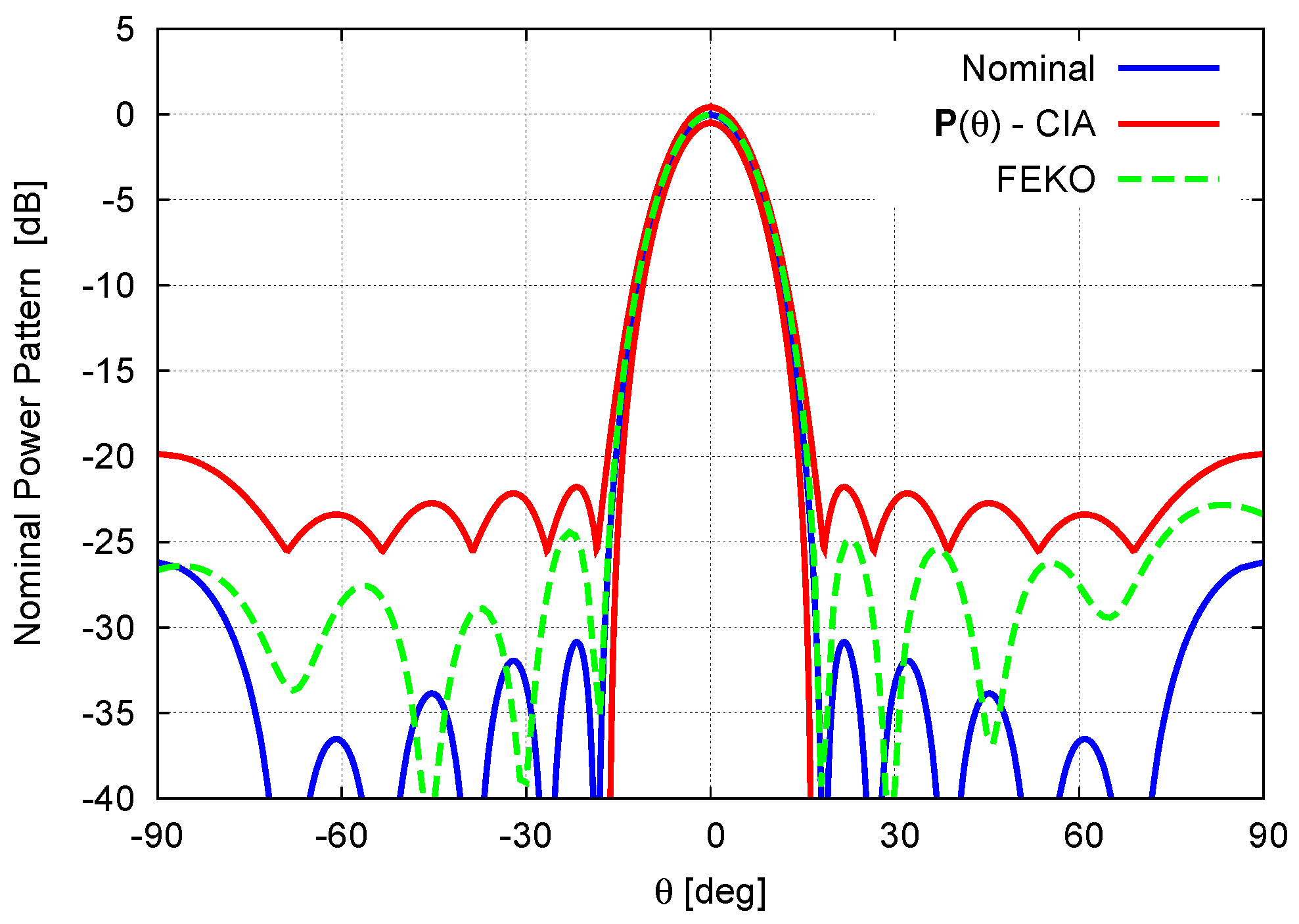
| Nominal Excitations | ||||||||
|---|---|---|---|---|---|---|---|---|
| n | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 |
| Calibration Error | ||||||||
| 2 | 3 | 4 | 5 | 5 | 4 | 3 | 2 | |
| Adjacent Coupling | ||||||||
| − | ||||||||
| 3 | 5 | 7 | 9 | 7 | 5 | 3 | − | |
| Multiple Coupling | ||||||||
| − | ||||||||
| 3 | 5 | 7 | 9 | 7 | 5 | 3 | − | |
| − | − | |||||||
| − | − | |||||||
| SLL (dB) | BW (u = sinθ) | Δ | ||
|---|---|---|---|---|
| − | ||||
| CIA | ||||
| CS | ||||
| RIA | ||||
| CIA | ||||
| CS | ||||
| RIA | ||||
| CIA | ||||
| CS | ||||
| RIA | ||||
| (i,j) | |||||
|---|---|---|---|---|---|
| SLL (dB) | BW (u = sinθ) | (dB) | |
|---|---|---|---|
| CIA |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Anselmi, N.; Salucci, M.; Rocca, P.; Massa, A. Power Pattern Sensitivity to Calibration Errors and Mutual Coupling in Linear Arrays through Circular Interval Arithmetics. Sensors 2016, 16, 791. https://doi.org/10.3390/s16060791
Anselmi N, Salucci M, Rocca P, Massa A. Power Pattern Sensitivity to Calibration Errors and Mutual Coupling in Linear Arrays through Circular Interval Arithmetics. Sensors. 2016; 16(6):791. https://doi.org/10.3390/s16060791
Chicago/Turabian StyleAnselmi, Nicola, Marco Salucci, Paolo Rocca, and Andrea Massa. 2016. "Power Pattern Sensitivity to Calibration Errors and Mutual Coupling in Linear Arrays through Circular Interval Arithmetics" Sensors 16, no. 6: 791. https://doi.org/10.3390/s16060791
APA StyleAnselmi, N., Salucci, M., Rocca, P., & Massa, A. (2016). Power Pattern Sensitivity to Calibration Errors and Mutual Coupling in Linear Arrays through Circular Interval Arithmetics. Sensors, 16(6), 791. https://doi.org/10.3390/s16060791







