Relaxation Dynamics of Chlorophyll b in the Sub-ps Ultrafast Timescale Measured by 2D Electronic Spectroscopy
Abstract
1. Introduction
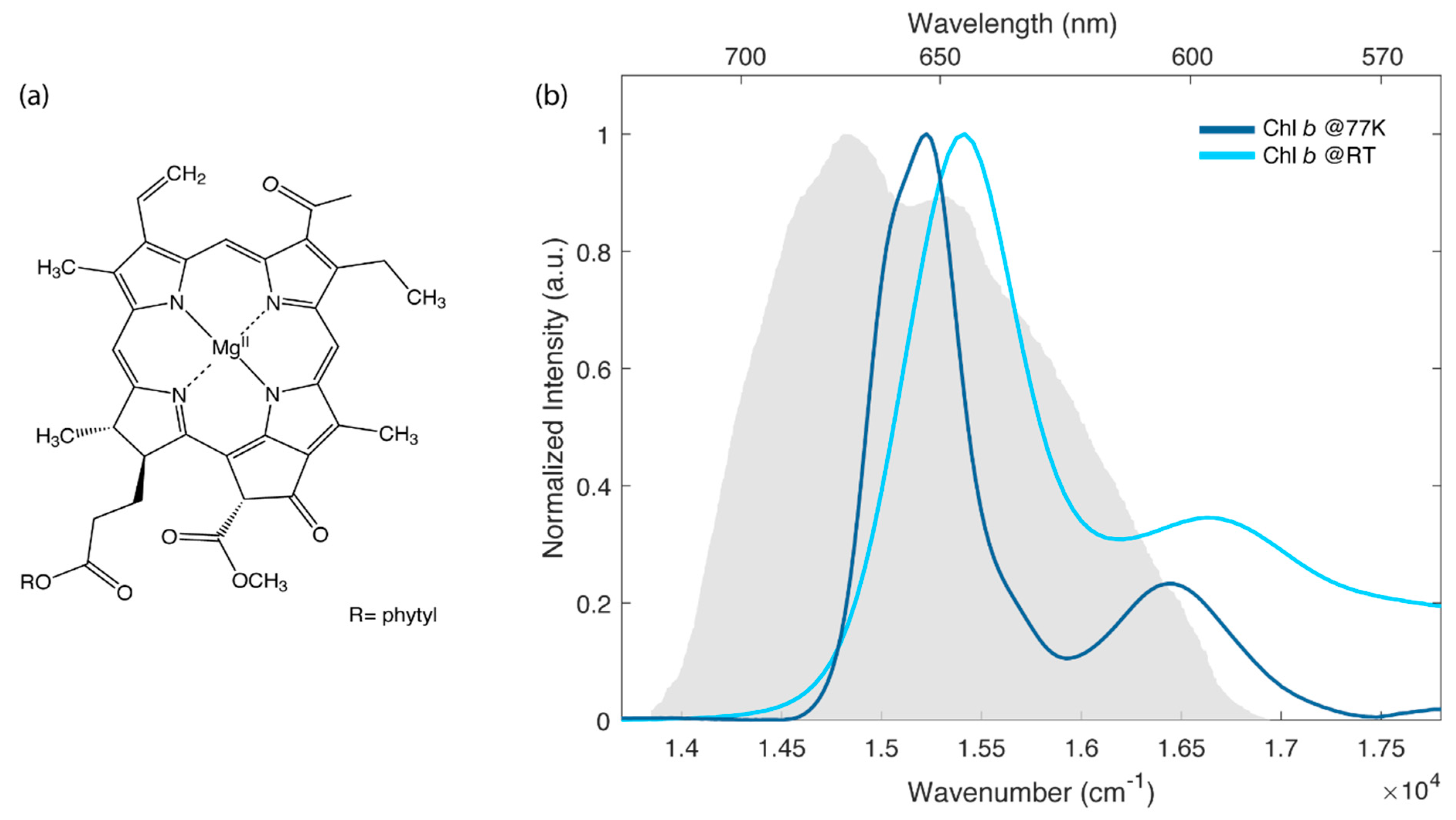
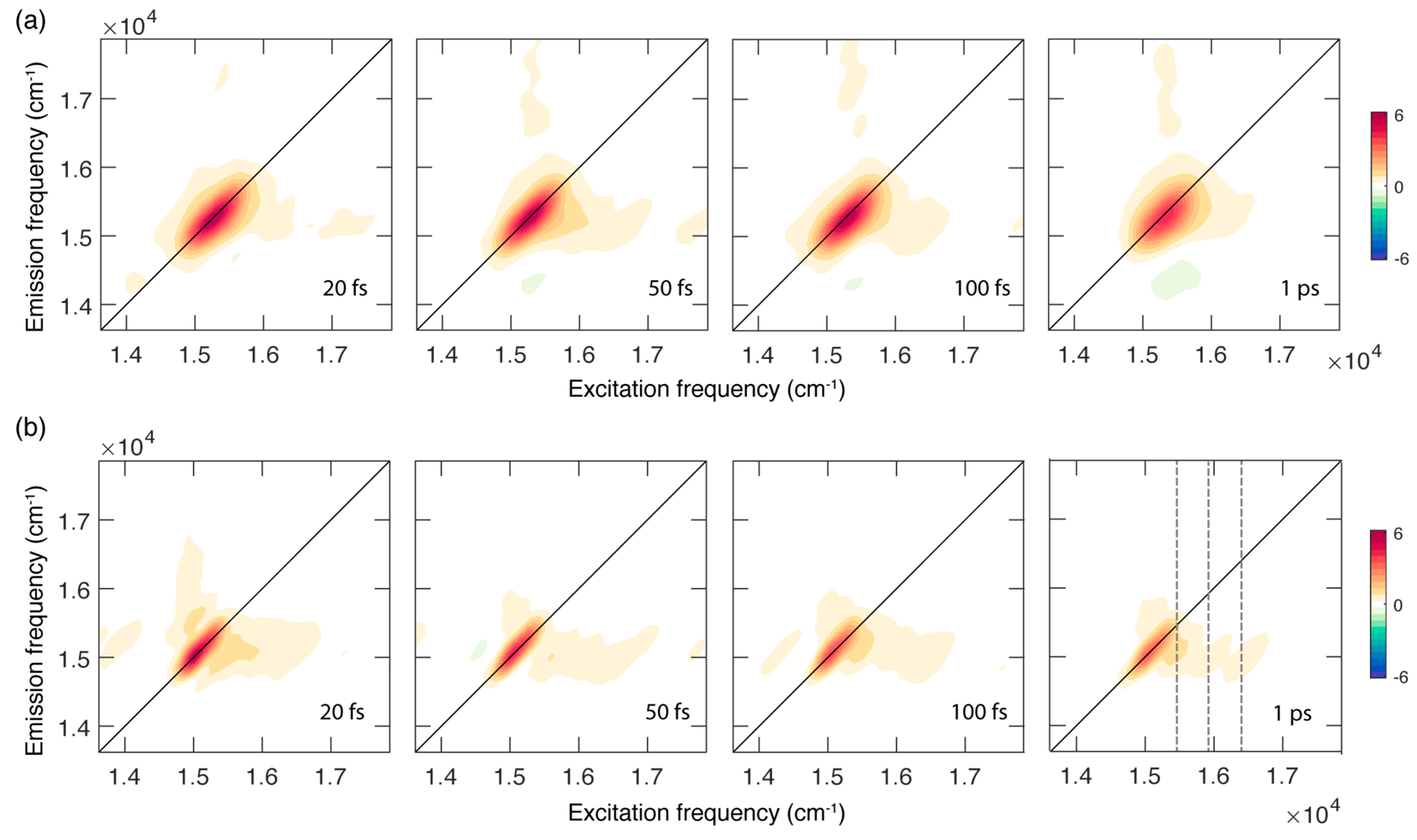
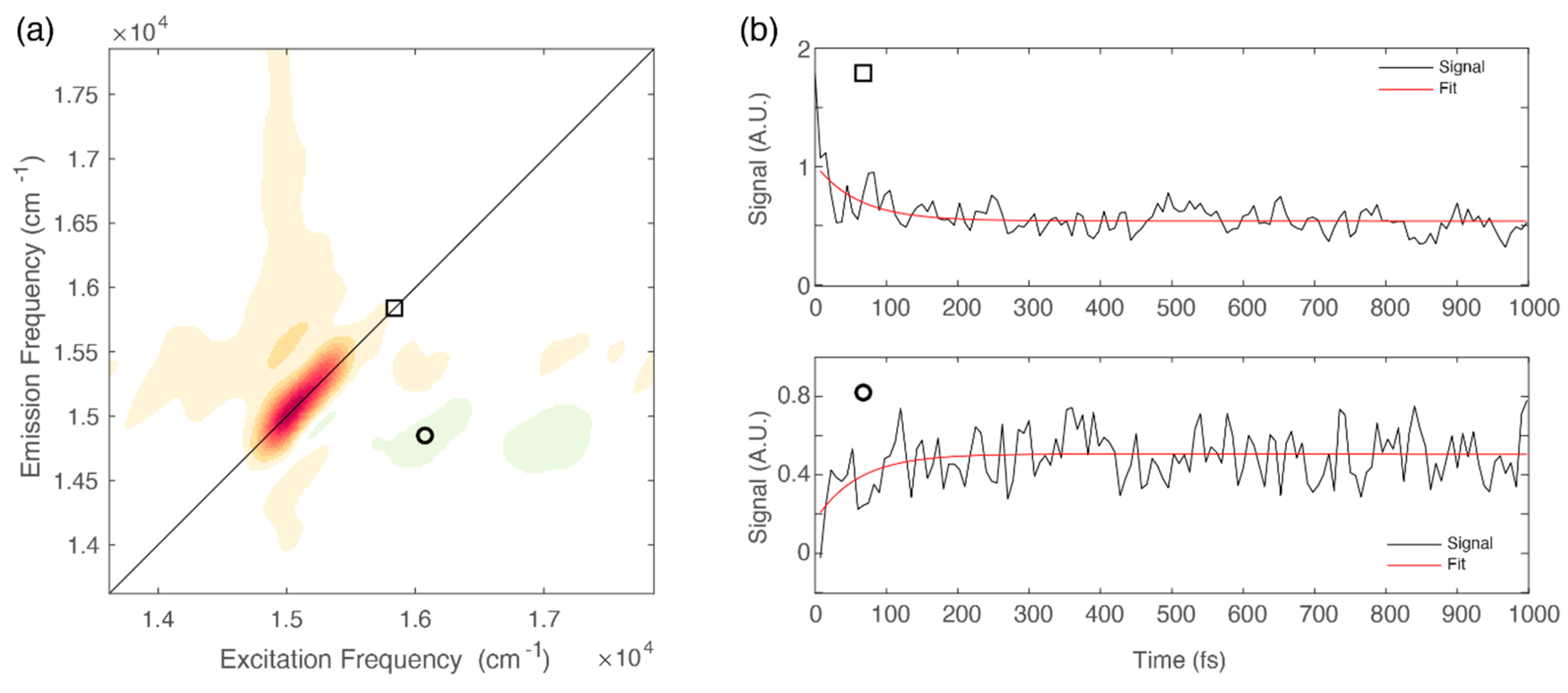
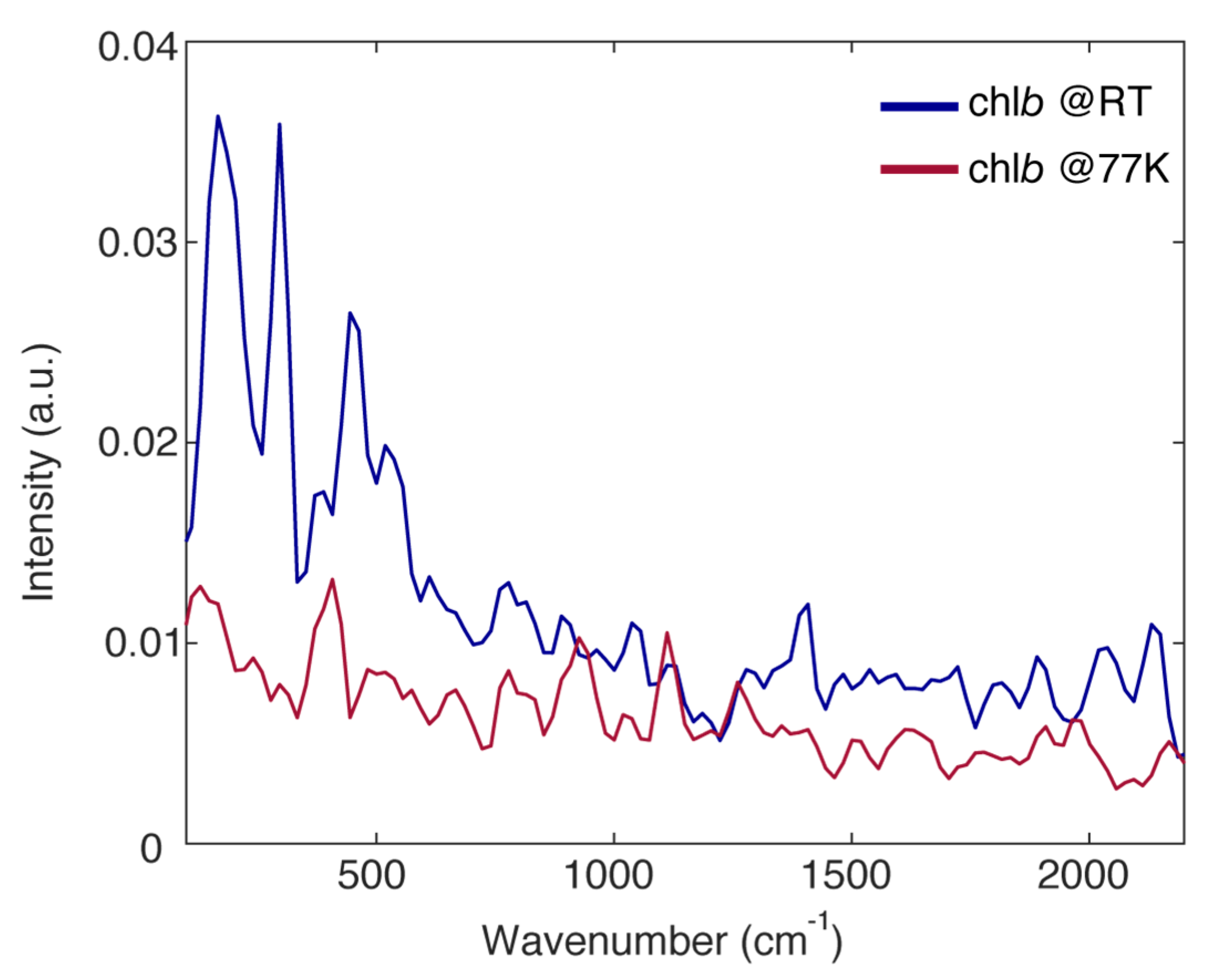
2. Results
3. Discussion
4. Materials and Methods
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Conflicts of Interest
References
- Scholes, G.D.; Fassioli, F.; Dinshaw, R.; Arpin, P.C. Photosynthetic light harvesting: Excitons and coherence. J. R. Soc. Interface 2014, 11, 20130901. [Google Scholar]
- Light-Harvesting Antennas in Photosynthesis; Green, B., Parson, W.W., Eds.; Springer Netherlands: Amsterdam, The Netherlands, 2003. [Google Scholar]
- van Amerogen, H.; Valkunas, L.; van Grondelle, R. Photosynthetic excitons; World Scientific: Singapore, 2000. [Google Scholar]
- Light Harvesting in Photosynthesis; Croce, R., van Grondelle, R., van Amerongen, H., Van Stokkum, I.H.M., Eds.; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Shipman, L.L.; Cotton, T.M.; Norris, J.R.; Katz, J.J. An analysis of the visible absorption spectrum of chlorophyll a monomer, dimer, and oligomers in solution. J. Am. Chem. Soc. 1976, 98, 8222–8230. [Google Scholar] [CrossRef] [PubMed]
- Connolly, J.S.; Janzen, A.F.; Samuel, E.B. Fluorescence lifetimes of chlorophyll a: Solvent, concentration and oxygen dependence. Photochem. Photobiol. 1982, 36, 559–563. [Google Scholar] [CrossRef]
- Renge, I.; Mauring, K. Spectral shift mechanisms of chlorophylls in liquids and proteins. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2013, 102, 301–313. [Google Scholar] [CrossRef] [PubMed]
- Niedzwiedzki, D.M.; Liu, H.; Chen, M.; Blankenship, R.E. Excited state properties of chlorophyll f in organic solvents at ambient and cryogenic temperatures. Photosynth. Res. 2014, 121, 25–34. [Google Scholar] [CrossRef]
- Meneghin, E.; Leonardo, C.; Volpato, A.; Bolzonello, L.; Collini, E. Mechanistic insight into internal conversion process within Q-bands of chlorophyll a. Sci. Rep. 2017, 7, 11389. [Google Scholar] [CrossRef]
- Moca, R.; Meech, S.R.; Heisler, I.A. Two-dimensional electronic spectroscopy of chlorophyll a: Solvent dependent spectral evolution. J. Phys. Chem. B 2015, 119, 8623–8630. [Google Scholar] [CrossRef]
- Scheer, H.; Scheer, H. Chapter 1 An Overview of Chlorophylls and Bacteriochlorophylls: Biochemistry, Biophysics, Functions and Applications. Chlorophylls Bacteriochlorophylls 2006, 1–26. [Google Scholar]
- Hoober, J.K.; Eggink, L.L.; Chen, M. Chlorophylls, ligands and assembly of light-harvesting complexes in chloroplasts. Photosynth. Res. 2007, 94, 387–400. [Google Scholar] [CrossRef]
- Grimm, B.; Porra, R.J.; Rüdiger, W.; Scheer, H. Chlorophylls and Bacteriochlorophylls (Advances in Photosynthesis and Respiration); Springer: Amsterdam, The Netherlands, 2006; ISBN 9781402045158. [Google Scholar]
- Tanaka, R.; Tanaka, A. Chlorophyll cycle regulates the construction and destruction of the light-harvesting complexes. Biochim. Biophys. Acta Bioenerg. 2011, 1807, 968–976. [Google Scholar] [CrossRef]
- Fresch, E.; Meneghin, E.; Agostini, A.; Paulsen, H.; Carbonera, D.; Collini, E. How the Protein Environment Can Tune the Energy, the Coupling, and the Ultrafast Dynamics of Interacting Chlorophylls: The Example of the Water-Soluble Chlorophyll Protein. J. Phys. Chem. Lett. 2020, 1059–1067. [Google Scholar] [CrossRef] [PubMed]
- Oksanen, J.A.I.; Martinsson, P.; Åkesson, E.; Hynninen, P.H.; Sundström, V. Transient hole burning and solvation dynamics of chlorophyll b monomers in various solvent environments. J. Phys. Chem. A 1998, 102, 4328–4336. [Google Scholar] [CrossRef]
- Bricker, W.P.; Shenai, P.M.; Ghosh, A.; Liu, Z.; Enriquez, M.G.M.; Lambrev, P.H.; Tan, H.-S.; Lo, C.S.; Tretiak, S.; Fernandez-Alberti, S.; et al. Non-radiative relaxation of photoexcited chlorophylls: Theoretical and experimental study. Sci. Rep. 2015, 5, 13625. [Google Scholar] [CrossRef] [PubMed]
- Khyasudeen, M.F.; Nowakowski, P.J.; Nguyen, H.L.; Sim, J.H.N.; Do, T.N.; Tan, H.S. Studying the spectral diffusion dynamics of chlorophyll a and chlorophyll b using two-dimensional electronic spectroscopy. Chem. Phys. 2019, 527, 110480. [Google Scholar] [CrossRef]
- Lewis, N.H.C.; Fleming, G.R. Two-Dimensional Electronic-Vibrational Spectroscopy of Chlorophyll a and b. J. Phys. Chem. Lett. 2016, 7, 831–837. [Google Scholar] [CrossRef]
- Gouterman, M. Spectra of porphyrins. J. Mol. Spectrosc. 1961, 6, 138–163. [Google Scholar] [CrossRef]
- Gouterman, M.; Wagnière, G.H.; Snyder, L.C. Spectra of porphyrins. Part II. Four orbital model. J. Mol. Spectrosc. 1963, 11, 108–127. [Google Scholar] [CrossRef]
- Hedayatifar, L.; Irani, E.; Mazarei, M.; Rasti, S.; Azar, Y.T.; Rezakhani, A.T.; Mashaghi, A.; Shayeganfar, F.; Anvari, M.; Heydari, T.; et al. Optical absorption and electronic spectra of chlorophylls a and b. RSC Adv. 2016, 6, 109778–109785. [Google Scholar] [CrossRef]
- Reimers, J.R.; Cai, Z.-L.; Kobayashi, R.; Rätsep, M.; Freiberg, A.; Krausz, E. Assignment of the Q-bands of the chlorophylls: Coherence loss via Qx - Qy mixing. Sci. Rep. 2013, 3, 2761. [Google Scholar] [CrossRef]
- Rätsep, M.; Linnanto, J.; Freiberg, A. Mirror symmetry and vibrational structure in optical spectra of chlorophyll a. J. Chem. Phys. 2009, 130, 1–11. [Google Scholar] [CrossRef]
- Bolzonello, L.; Volpato, A.; Meneghin, E.; Collini, E. Versatile setup for high-quality rephasing, non-rephasing, and double quantum 2D electronic spectroscopy. J. Opt. Soc. Am. B 2017, 34, 1223. [Google Scholar] [CrossRef]
- Collini, E. Spectroscopic signatures of quantum-coherent energy transfer. Chem. Soc. Rev. 2013, 42. [Google Scholar] [CrossRef] [PubMed]
- Brańczyk, A.M.; Turner, D.B.; Scholes, G.D. Crossing disciplines - A view on two-dimensional optical spectroscopy. Ann. Phys. 2014, 526, 31–49. [Google Scholar] [CrossRef]
- Gelzinis, A.; Augulis, R.; Butkus, V.; Robert, B.; Valkunas, L. Two-dimensional spectroscopy for non-specialists. Biochim. Biophys. Acta Bioenerg. 2019, 1860, 271–285. [Google Scholar] [CrossRef] [PubMed]
- Meneghin, E.; Pedron, D.; Collini, E. Raman and 2D electronic spectroscopies: A fruitful alliance for the investigation of ground and excited state vibrations in chlorophyll a. Chem. Phys. 2018, 514, 132–140. [Google Scholar] [CrossRef]
- Meneghin, E.; Pedron, D.; Collini, E. Characterization of the coherent dynamics of bacteriochlorophyll a in solution. Chem. Phys. 2019, 519, 85–91. [Google Scholar] [CrossRef]
- Bolzonello, L.; Fassioli, F.; Collini, E. Correlated Fluctuations and Intraband Dynamics of J-Aggregates Revealed by Combination of 2DES Schemes. J. Phys. Chem. Lett. 2016, 7, 4996–5001. [Google Scholar] [CrossRef]
- Šanda, F.; Perlík, V.; Lincoln, C.N.; Hauer, J. Center Line Slope Analysis in Two-Dimensional Electronic Spectroscopy. J. Phys. Chem. A 2015, 119, 10893–10909. [Google Scholar] [CrossRef]
- Volpato, A.; Bolzonello, L.; Meneghin, E.; Collini, E. Global analysis of coherence and population dynamics in 2D electronic spectroscopy. Opt. Express 2016, 24, 24773–24785. [Google Scholar] [CrossRef]
- Volpato, A. fitko—Global Fit of 2DES data. Available online: https://github.com/MUOSColliniLab/fitko (accessed on 1 April 2020).
- Meneghin, E.; Pedron, D.; Collini, E. Spectroscopy data for the time and frequency characterization of vibrational coherences in bacteriochlorophyll a. Data Br. 2019, 23, 103707. [Google Scholar] [CrossRef]
- Passino, S.A.; Nagasawa, Y.; Joo, T.; Fleming, G.R. Three-Pulse Echo Peak Shift Studies of Polar Solvation Dynamics. J. Phys. Chem. A 1997, 101, 725–731. [Google Scholar] [CrossRef]
- Horng, M.L.; Gardecki, J.A.; Papazyan, A.; Maroncelli, M. Subpicosecond Measurements of Polar Solvation Dynamics: Coumarin 153 Revisited. J. Phys. Chem. 1995, 99, 17311–17337. [Google Scholar] [CrossRef]
- Lee, S.-H.; Lee, J.-H.; Joo, T. Deuterium isotope effect on the solvation dynamics of a dye molecule in methanol and acetonitrile. J. Chem. Phys. 1999, 110, 10969–10977. [Google Scholar] [CrossRef]
- Berg, M.; Walsh, C.A.; Narasimhan, L.R.; Littau, K.A.; Fayer, M.D. Dynamics in low temperature glasses: Theory and experiments on optical dephasing, spectral diffusion, and hydrogen tunneling. J. Chem. Phys. 1988, 88, 1564–1587. [Google Scholar] [CrossRef]
- Kwak, K.; Rosenfeld, D.E.; Fayer, M.D. Taking apart the two-dimensional infrared vibrational echo spectra: More information and elimination of distortions. J. Chem. Phys. 2008, 128, 204505. [Google Scholar] [CrossRef] [PubMed]
- Bolzonello, L.; Polo, A.; Volpato, A.; Meneghin, E.; Cordaro, M.; Trapani, M.; Fortino, M.; Pedone, A.; Castriciano, M.A.; Collini, E. Two-dimensional electronic spectroscopy reveals dynamics and mechanisms of solvent-driven inertial relaxation in polar BODIPY dyes. J. Phys. Chem. Lett. 2018, 9, 1079–1085. [Google Scholar] [CrossRef] [PubMed]
- Jakučionis, M.; Chorošajev, V.; Abramavičius, D. Vibrational damping effects on electronic energy relaxation in molecular aggregates. Chem. Phys. 2018, 515, 193–202. [Google Scholar] [CrossRef]
- Shenai, P.M.; Fernandez-Alberti, S.; Bricker, W.P.; Tretiak, S.; Zhao, Y. Internal Conversion and Vibrational Energy Redistribution in Chlorophyll A. J. Phys. Chem. B 2016, 120, 49–58. [Google Scholar] [CrossRef]
- Kano, H.; Kobayashi, T. Time-resolved fluorescence and absorption spectroscopies of porphyrin J-aggregates. J. Chem. Phys. 2001, 116, 184–195. [Google Scholar] [CrossRef]
- Kosumi, D.; Nishiguchi, T.; Sugisaki, M.; Hashimoto, H. Ultrafast coherent spectroscopic investigation on photosynthetic pigment chlorophyll a utilizing 20fs pulses. J. Photochem. Photobiol. A Chem. 2015, 313, 72–78. [Google Scholar] [CrossRef]
- Dong, L.-Q.; Niu, K.; Cong, S.-L. Theoretical analysis of internal conversion pathways and vibrational relaxation process of chlorophyll-a in ethyl ether solvent. Chem. Phys. Lett. 2007, 440, 150–154. [Google Scholar] [CrossRef]
- Mataga, N.; Shibata, Y.; Chosrowjan, H.; Yoshida, N.; Osuka, A. Internal Conversion and Vibronic Relaxation from Higher Excited Electronic State of Porphyrins: Femtosecond Fluorescence Dynamics Studies. J. Phys. Chem. B 2000, 104, 4001–4004. [Google Scholar] [CrossRef]
- Volpato, A.; Collini, E. Time-frequency methods for coherent spectroscopy. Opt. Express 2015, 23, 20040–20050. [Google Scholar] [CrossRef] [PubMed]
- Pajusalu, M.; Rätsep, M.; Freiberg, A. Temperature dependent electron–phonon coupling in chlorin-doped impurity glass and in photosynthetic FMO protein containing bacteriochlorophyll a. J. Lumin. 2014, 152, 79–83. [Google Scholar] [CrossRef]
- Owrutsky, J.C.; Raftery, D.; Hochstrasser, R.M. Vibrational Relaxation Dynamics in Solutions. Annu. Rev. Phys. Chem. 1994, 45, 519–555. [Google Scholar] [CrossRef]

© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fresch, E.; Collini, E. Relaxation Dynamics of Chlorophyll b in the Sub-ps Ultrafast Timescale Measured by 2D Electronic Spectroscopy. Int. J. Mol. Sci. 2020, 21, 2836. https://doi.org/10.3390/ijms21082836
Fresch E, Collini E. Relaxation Dynamics of Chlorophyll b in the Sub-ps Ultrafast Timescale Measured by 2D Electronic Spectroscopy. International Journal of Molecular Sciences. 2020; 21(8):2836. https://doi.org/10.3390/ijms21082836
Chicago/Turabian StyleFresch, Elisa, and Elisabetta Collini. 2020. "Relaxation Dynamics of Chlorophyll b in the Sub-ps Ultrafast Timescale Measured by 2D Electronic Spectroscopy" International Journal of Molecular Sciences 21, no. 8: 2836. https://doi.org/10.3390/ijms21082836
APA StyleFresch, E., & Collini, E. (2020). Relaxation Dynamics of Chlorophyll b in the Sub-ps Ultrafast Timescale Measured by 2D Electronic Spectroscopy. International Journal of Molecular Sciences, 21(8), 2836. https://doi.org/10.3390/ijms21082836




