Drift Versus Entropic Forces in Overdamped Diffusion Through a Widening Channel
Abstract
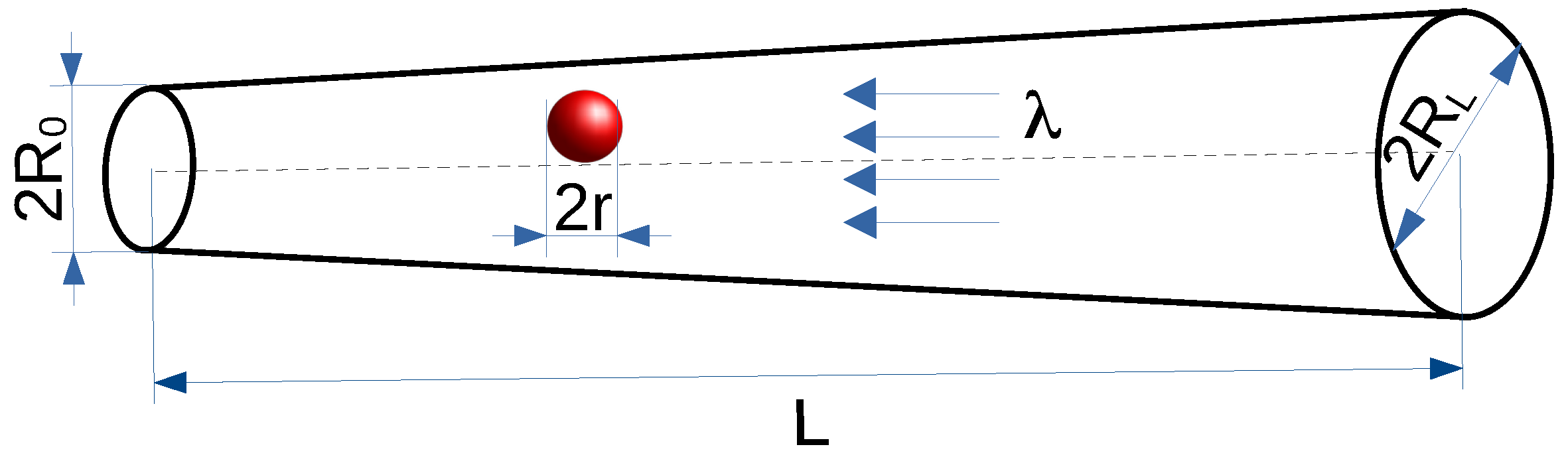
1. Introduction
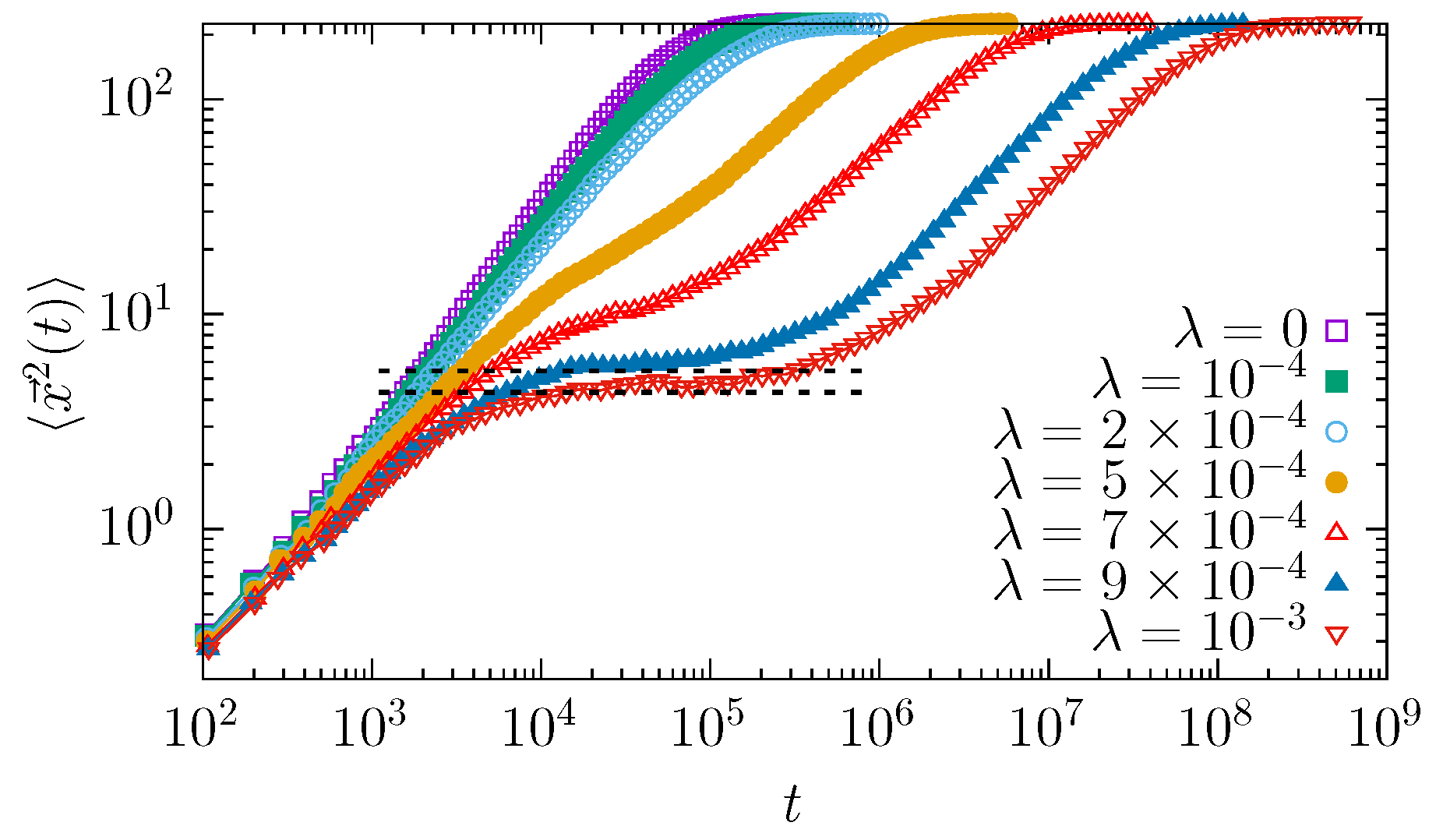
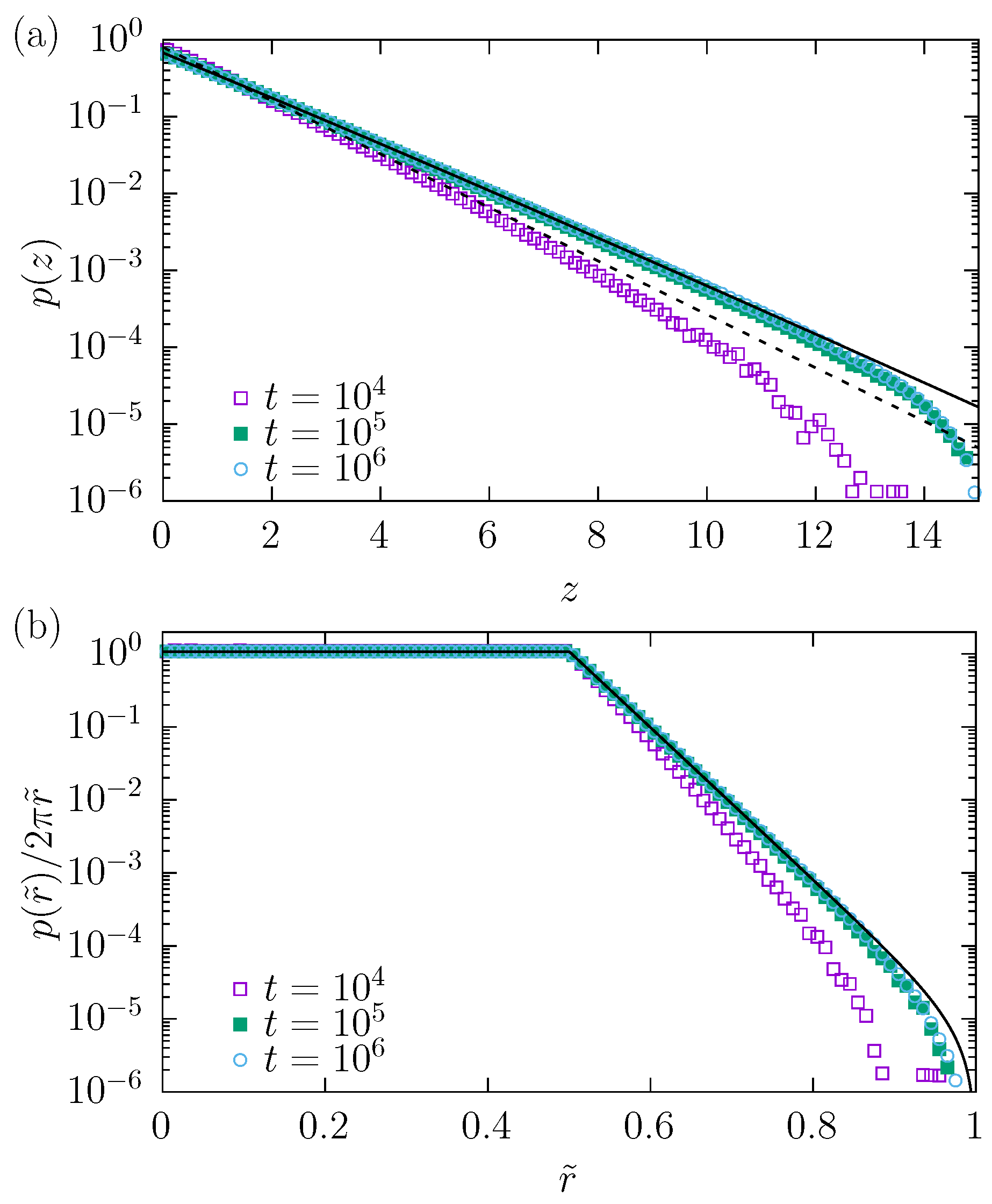
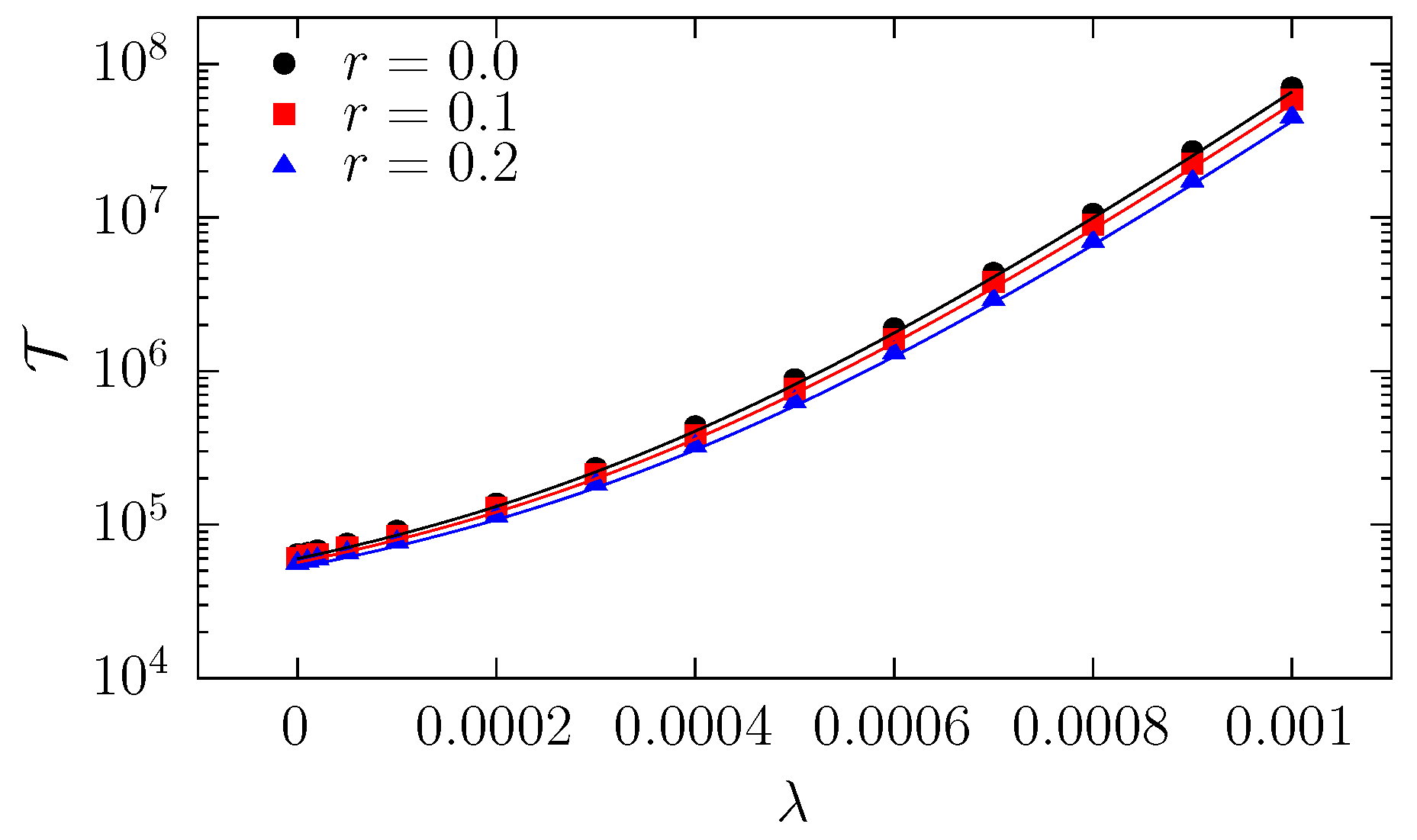
2. Results and Discussion
Mean First Passage Time
3. Materials and Methods
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| MSD | mean squared displacement |
| MFPT | mean first passage time |
Appendix A. Fick–Jacobs Description of Entropic Forces
References
- Kasianowicz, J.J.; Brandin, E.; Branton, D.; Deamer, D.W. Characterization of individual polynucleotide molecules using a membrane channel. Proc. Nat. Acad. Sci. USA 1996, 93, 13770–13773. [Google Scholar] [CrossRef] [PubMed]
- Bayley, H.; Martin, C.R. Resistive-Pulse Sensing: From Microbes to Molecules. Chem. Rev. 2000, 100, 2575–2594. [Google Scholar] [CrossRef] [PubMed]
- Uram, J.D.; Ke, K.; Hunt, A.J.; Mayer, M. Submicrometer Pore-Based Characterization and Quantification of Antibody–Virus Interactions. Small 2006, 2, 967–972. [Google Scholar] [CrossRef] [PubMed]
- Dekker, C. Solid-state nanopores. Nat. Nanotechnol. 2007, 2, 209–215. [Google Scholar] [CrossRef]
- Iqbal, S.M.; Akin, D.; Bashir, R. Solid-state nanopore channels with DNA selectivity. Nat. Nanotechnol. 2007, 2, 243–248. [Google Scholar] [CrossRef]
- Makhnovskii, Y.; Berezhkovskii, A.; Zitserman, V. Diffusion in a tube of alternating diameter. Chem. Phys. 2010, 370, 238–243. [Google Scholar] [CrossRef]
- Bezrukov, S.M.; Schimansky-Geier, L.; Schmid, G. Brownian motion in confined geometries. Eur. Phys. J. Spec. Top. 2014, 223, 3021–3025. [Google Scholar] [CrossRef]
- Liu, L.; Cherstvy, A.G.; Metzler, R. Facilitated Diffusion of Transcription Factor Proteins with Anomalous Bulk Diffusion. J. Phys. Chem. B. 2017, 121, 1284–1289. [Google Scholar] [CrossRef]
- Horne, R.I.; Sandler, S.E.; Vendruscolo, M.; Keyser, U.F. Detection of protein oligomers with nanopores. Nat. Rev. Chem. 2025, 9, 224–240. [Google Scholar] [CrossRef]
- Kalinay, P. Effective dynamics of a particle diffusing in time-dependent confinement. Phys. Rev. E 2025, 111, 014117. [Google Scholar] [CrossRef]
- Hille, B. Ionic channels in excitable membranes. Current problems and biophysical approaches. Biophys. J. 1978, 22, 283–294. [Google Scholar] [CrossRef] [PubMed]
- Hille, B. Ion Channels of Excitable Membranes; Sinauer Associates: Sunderland, MA, USA, 2001. [Google Scholar]
- Thompson, M.J.; Baenziger, J.E. Ion channels as lipid sensors: From structures to mechanisms. Nat. Chem. Biol. 2020, 16, 1331–1342. [Google Scholar] [CrossRef] [PubMed]
- Diederichs, T.; Ahmad, K.; Burns, J.R.; Nguyen, Q.H.; Siwy, Z.S.; Tornow, M.; Coveney, P.V.; Tampé, R.; Howorka, S. Principles of small-molecule transport through synthetic nanopores. ACS Nano 2021, 15, 16194–16206. [Google Scholar] [CrossRef] [PubMed]
- Cherf, G.M.; Lieberman, K.R.; Rashid, H.; Lam, C.E.; Karplus, K.; Akeson, M. Automated forward and reverse ratcheting of DNA in a nanopore at 5-Å precision. Nat. Biotechnol. 2012, 30, 344–348. [Google Scholar] [CrossRef]
- Chien, C.C.; Shekar, S.; Niedzwiecki, D.J.; Shepard, K.L.; Drndić, M. Single-Stranded DNA Translocation Recordings through Solid-State Nanopores on Glass Chips at 10 MHz Measurement Bandwidth. ACS Nano 2019, 13, 10545–10554. [Google Scholar] [CrossRef]
- Venkatesan, B.M.; Bashir, R. Nanopore sensors for nucleic acid analysis. Nat. Nanotechnol. 2011, 6, 615–624. [Google Scholar] [CrossRef]
- Wanunu, M. Nanopores: A journey towards DNA sequencing. Phys. Life Rev. 2012, 9, 125–158. [Google Scholar] [CrossRef]
- Xue, L.; Yamazaki, H.; Ren, R.; Wanunu, M.; Ivanov, A.P.; Edel, J.B. Solid-state nanopore sensors. Nat. Rev. Mater. 2020, 5, 931–951. [Google Scholar] [CrossRef]
- Akhtarian, S.; Miri, S.; Doostmohammadi, A.; Brar, S.K.; Rezai, P. Nanopore sensors for viral particle quantification: Current progress and future prospects. Bioengineered 2021, 12, 9189–9215. [Google Scholar] [CrossRef]
- Tagliazucchi, M.; Szleifer, I. Transport mechanisms in nanopores and nanochannels: Can we mimic nature? Mater. Today 2015, 18, 131–142. [Google Scholar] [CrossRef]
- Sirkin, Y.P.; Tagliazucchi, M.; Szleifer, I. Transport in nanopores and nanochannels: Some fundamental challenges and nature-inspired solutions. Mater. Today Adv. 2020, 5, 100047. [Google Scholar] [CrossRef]
- Gubbiotti, A.; Chinappi, M.; Casciola, C.M. Confinement effects on the dynamics of a rigid particle in a nanochannel. Phys. Rev. E 2019, 100, 053307. [Google Scholar] [CrossRef] [PubMed]
- Berezhkovskii, A.M.; Pustovoit, M.A.; Bezrukov, S.M. Diffusion in a tube of varying cross section: Numerical study of reduction to effective one-dimensional description. J. Chem. Phys. 2007, 126, 134706. [Google Scholar] [CrossRef] [PubMed]
- Rubi, J.M. Entropic diffusion in confined soft-matter and biological systems. Europhys. Lett. 2019, 127, 10001. [Google Scholar] [CrossRef]
- Dagdug, L.; Berezhkovskii, A.M.; Bezrukov, S.M. Diffusion Resistance of Segmented Channels. J. Phys. Chem. B 2023, 127, 7291–7298. [Google Scholar] [CrossRef]
- Yang, X.; Liu, C.; Li, Y.; Marchesoni, F.; Hänggi, P.; Zhang, H.P. Hydrodynamic and entropic effects on colloidal diffusion in corrugated channels. Proc. Natl. Acad. Sci. USA 2017, 114, 9564–9569. [Google Scholar] [CrossRef]
- Li, Y.; Mei, R.; Xu, Y.; Kurths, J.; Duan, J.; Metzler, R. Particle dynamics and transport enhancement in a confined channel with position-dependent diffusivity. New J. Phys. 2020, 22, 053016. [Google Scholar] [CrossRef]
- Kubala, P.; Cieśla, M.; Dybiec, B. Diffusion in crowded environments: Trapped by the drift. Phys. Rev. E 2021, 104, 044127. [Google Scholar] [CrossRef]
- Lançon, P.; Batrouni, G.; Lobry, L.; Ostrowsky, N. Brownian walker in a confined geometry leading to a space-dependent diffusion coefficient. Physica A 2002, 304, 65–76. [Google Scholar] [CrossRef]
- Reguera, D.; Rubí, J.M. Kinetic equations for diffusion in the presence of entropic barriers. Phys. Rev. E 2001, 64, 061106. [Google Scholar] [CrossRef]
- Burada, P.S.; Schmid, G.; Talkner, P.; Hänggi, P.; Reguera, D.; Rubí, J.M. Entropic particle transport in periodic channels. BioSystems 2008, 93, 16–22. [Google Scholar] [CrossRef] [PubMed]
- Riefler, W.; Schmid, G.; Burada, P.S.; Hänggi, P. Entropic transport of finite size particles. J. Condens. Matter Phys. 2010, 22, 454109. [Google Scholar] [CrossRef] [PubMed]
- Reguera, D.; Luque, A.; Burada, P.S.; Schmid, G.; Rubí, J.M.; Hänggi, P. Entropic Splitter for Particle Separation. Phys. Rev. Lett. 2012, 108, 020604. [Google Scholar] [CrossRef] [PubMed]
- Berezhkovskii, A.M.; Bezrukov, S.M. On the applicability of entropy potentials in transport problems. Eur. Phys. J. Spec. Top. 2014, 223, 3063–3077. [Google Scholar] [CrossRef]
- Cieśla, M.; Dybiec, B.; Krasowska, M.; Strzelewicz, A. Effective anomalous diffusion in a conical channel. Chaos 2025, 35, 023143. [Google Scholar] [CrossRef]
- Jacobs, M.H. Diffusion Processes; Springer: Berlin/Heidelberg, Germany, 1967. [Google Scholar] [CrossRef]
- Zwanzig, R. Diffusion past an entropy barrier. J. Phys. Chem. 1992, 96, 3926–3930. [Google Scholar] [CrossRef]
- Kalinay, P.; Percus, J. Projection of two-dimensional diffusion in a narrow channel onto the longitudinal dimension. J. Phys. Chem. 2005, 122, 204701. [Google Scholar] [CrossRef]
- Kalinay, P.; Percus, J. Corrections to the Fick-Jacobs equation. Phys. Rev. E 2006, 74, 041203. [Google Scholar] [CrossRef]
- Mangeat, M.; Guérin, T.; Dean, D.S. Dispersion in two dimensional channels—The Fick–Jacobs approximation revisited. J. Stat. Mech. 2017, 2017, 123205. [Google Scholar] [CrossRef][Green Version]
- Berezhkovskii, A.M.; Dagdug, L.; Bezrukov, S.M. First passage, looping, and direct transition in expanding and narrowing tubes: Effects of the entropy potential. J. Chem. Phys. 2017, 147, 134104. [Google Scholar] [CrossRef]
- Slater, G.W.; Guo, H.L.; Nixon, G.I. Bidirectional transport of polyelectrolytes using self-modulating entropic ratchets. Phys. Rev. Lett. 1997, 78, 1170. [Google Scholar] [CrossRef]
- Berezhkovskii, A.; Dagdug, L.; Bezrukov, S.M. Discriminating between Anomalous Diffusion and Transient Behavior in Microheterogeneous Environments. Biophys. J. 2014, 106, L09–L11. [Google Scholar] [CrossRef] [PubMed]
- Gordon, D.; Chen, R.; Ho, J.; Coote, M.; Chung, S. Rigid Body Brownian Dynamics as a Tool for Studying Ion Channel Blockers. J. Phys. Chem. B 2012, 116, 1933–1941. [Google Scholar] [CrossRef] [PubMed]
- Metzler, R.; Klafter, J. The random walk’s guide to anomalous diffusion: A fractional dynamics approach. Phys. Rep. 2000, 339, 1–77. [Google Scholar] [CrossRef]
- Šiler, M.; Ornigotti, L.; Brzobohatý, O.; Jákl, P.; Ryabov, A.; Holubec, V.; Zemánek, P.; Filip, R. Diffusing up the Hill: Dynamics and Equipartition in Highly Unstable Systems. Phys. Rev. Lett. 2018, 121, 230601. [Google Scholar] [CrossRef]
- Gardiner, C.W. Handbook of Stochastic Methods for Physics, Chemistry and Natural Sciences; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar] [CrossRef]
- Higham, D.J. An algorithmic introduction to numerical simulation of stochastic differential equations. SIAM Rev. 2001, 43, 525–546. [Google Scholar] [CrossRef]
- Mannella, R. Integration of Stochastic Differential Equations on a Computer. Int. J. Mod. Phys. C 2002, 13, 1177–1194. [Google Scholar] [CrossRef]
- Cieśla, M.; Dybiec, B.; Krasowska, M.; Siwy, Z.; Strzelewicz, A. Numerical Modeling of Anisotropic Particle Diffusion through a Cylindrical Channel. Molecules 2024, 29, 3795. [Google Scholar] [CrossRef]
- Strzelewicz, A.; Cieśla, M.; Dybiec, B.; Krasowska, M. Modeling Diffusion of Elongated Particles Through a Narrowing Channel. Entropy 2025, 27, 293. [Google Scholar] [CrossRef]
- Schiel, M.; Siwy, Z.S. Diffusion and trapping of single particles in pores with combined pressure and dynamic voltage. J. Phys. Chem. C 2014, 118, 19214–19223. [Google Scholar] [CrossRef]
- van Kampen, N. Stochastic Processes in Physics and Chemistry, 3rd ed.; Elsevier: Amsterdam, The Netherlands, 2007. [Google Scholar] [CrossRef]

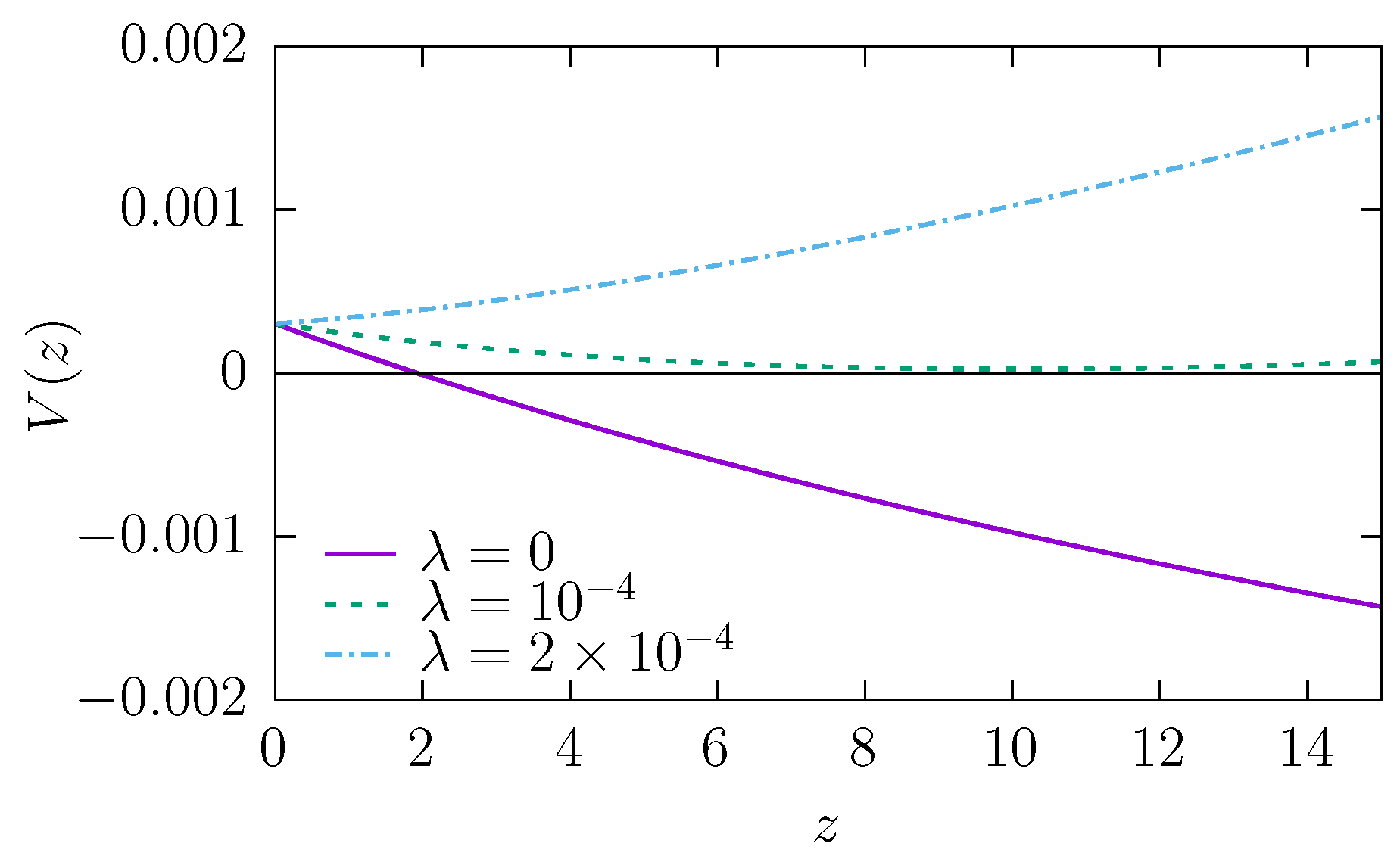
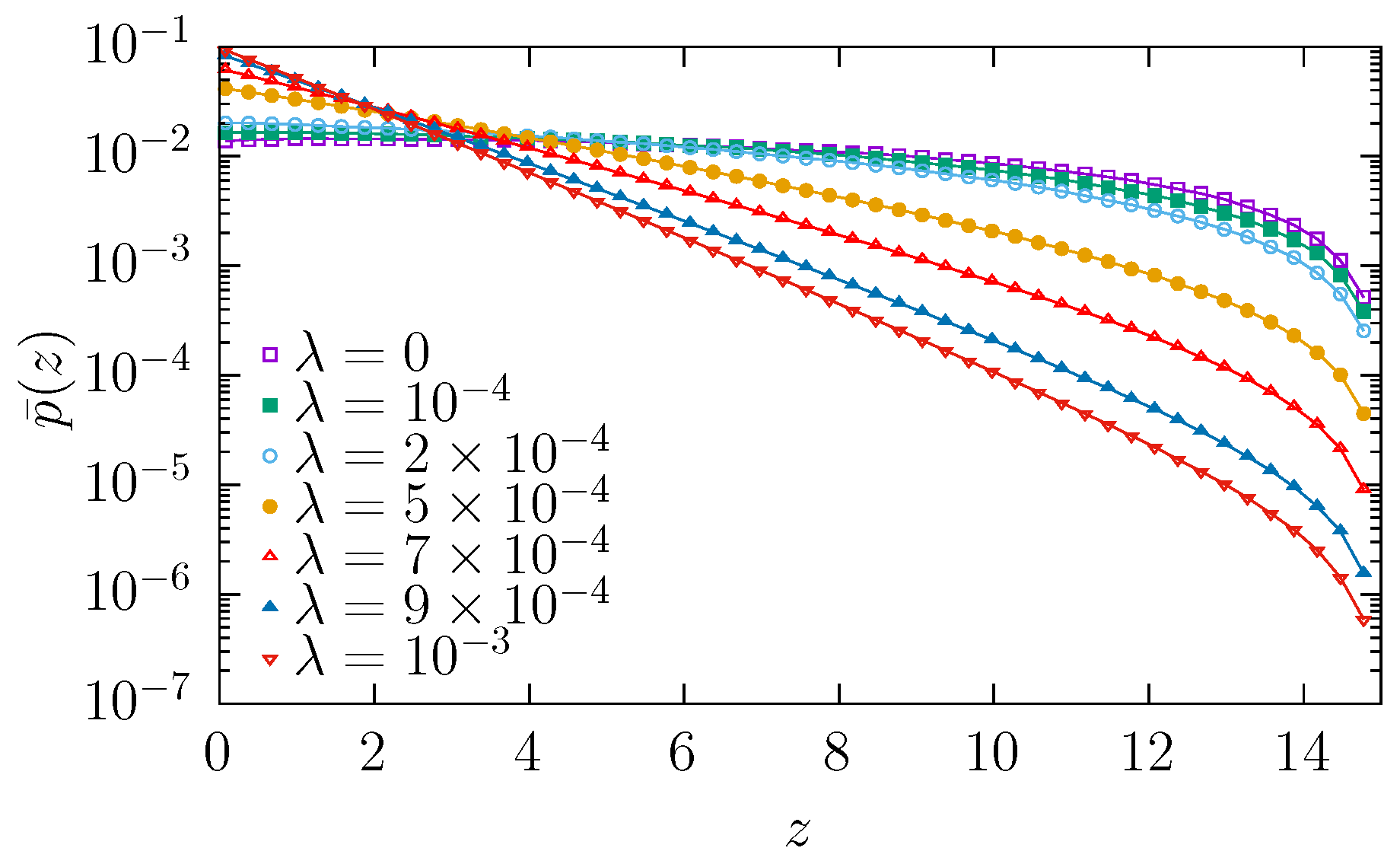
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cieśla, M.; Dybiec, B.; Krasowska, M.; Strzelewicz, A. Drift Versus Entropic Forces in Overdamped Diffusion Through a Widening Channel. Molecules 2025, 30, 2316. https://doi.org/10.3390/molecules30112316
Cieśla M, Dybiec B, Krasowska M, Strzelewicz A. Drift Versus Entropic Forces in Overdamped Diffusion Through a Widening Channel. Molecules. 2025; 30(11):2316. https://doi.org/10.3390/molecules30112316
Chicago/Turabian StyleCieśla, Michał, Bartłomiej Dybiec, Monika Krasowska, and Anna Strzelewicz. 2025. "Drift Versus Entropic Forces in Overdamped Diffusion Through a Widening Channel" Molecules 30, no. 11: 2316. https://doi.org/10.3390/molecules30112316
APA StyleCieśla, M., Dybiec, B., Krasowska, M., & Strzelewicz, A. (2025). Drift Versus Entropic Forces in Overdamped Diffusion Through a Widening Channel. Molecules, 30(11), 2316. https://doi.org/10.3390/molecules30112316





