Enhancing High-Order Harmonic Generation Efficiency Through Molecular Size and Orientation Effects: A Pathway to Ultrafast Chemical Dynamics Studies
Abstract
1. Introduction
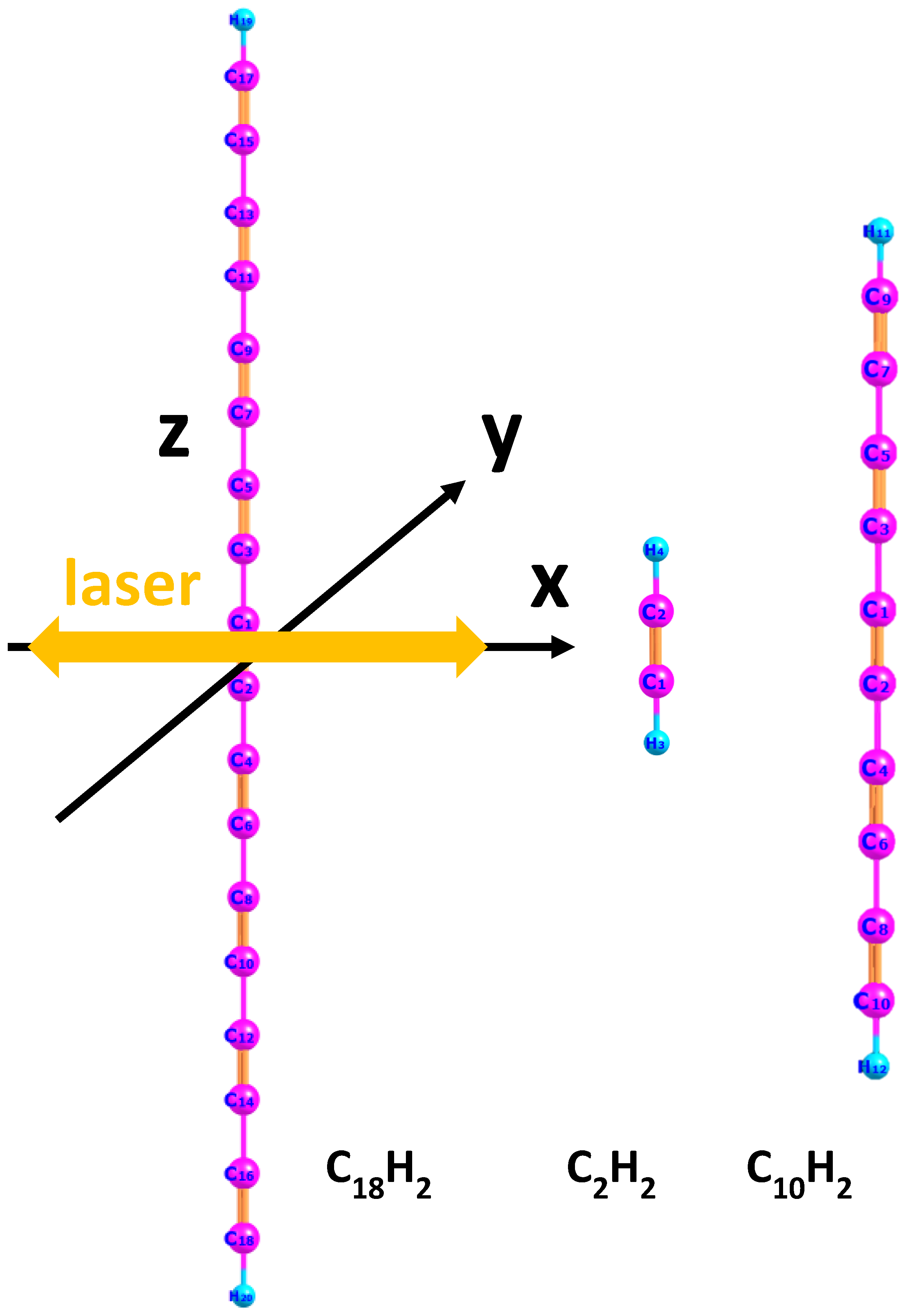
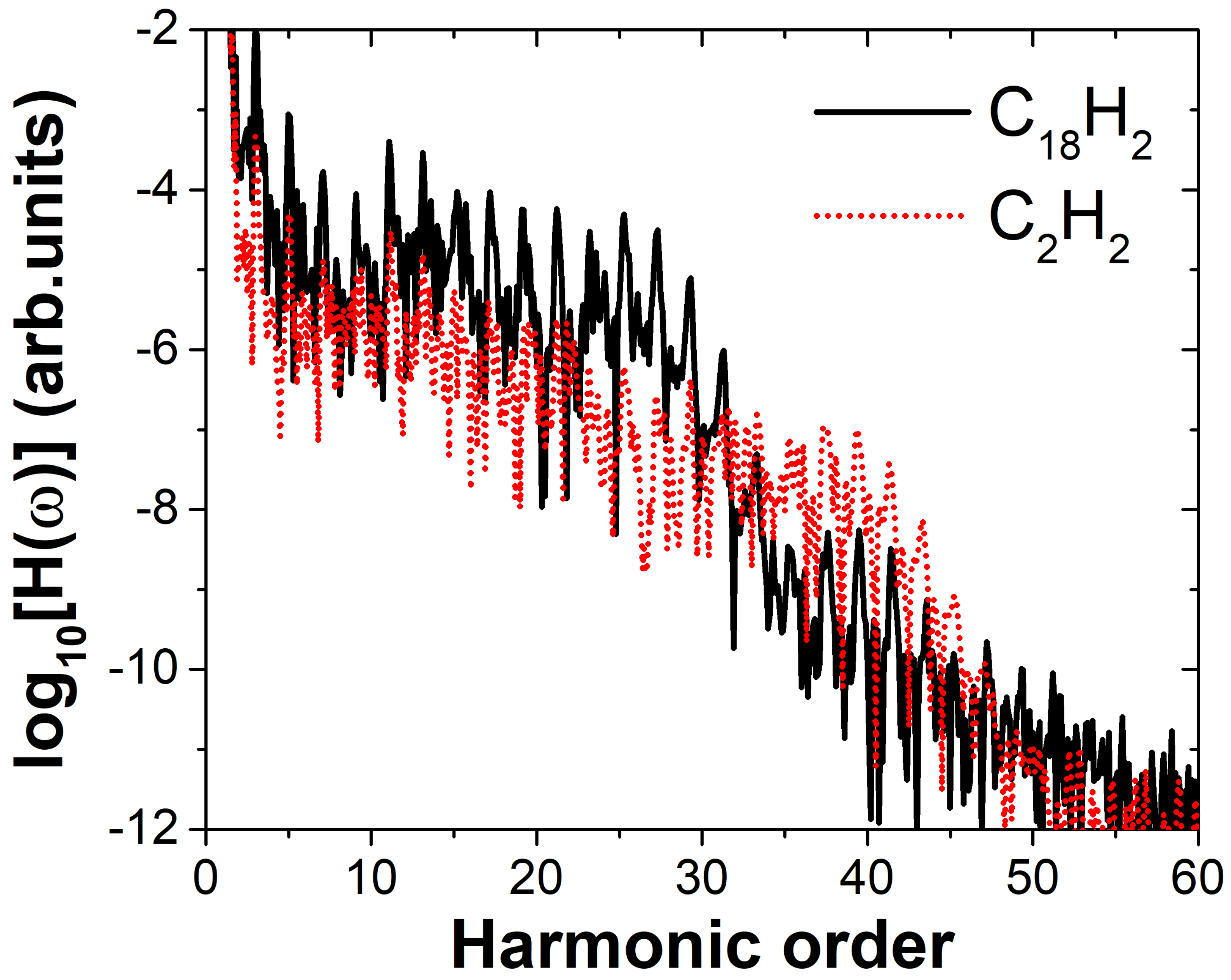
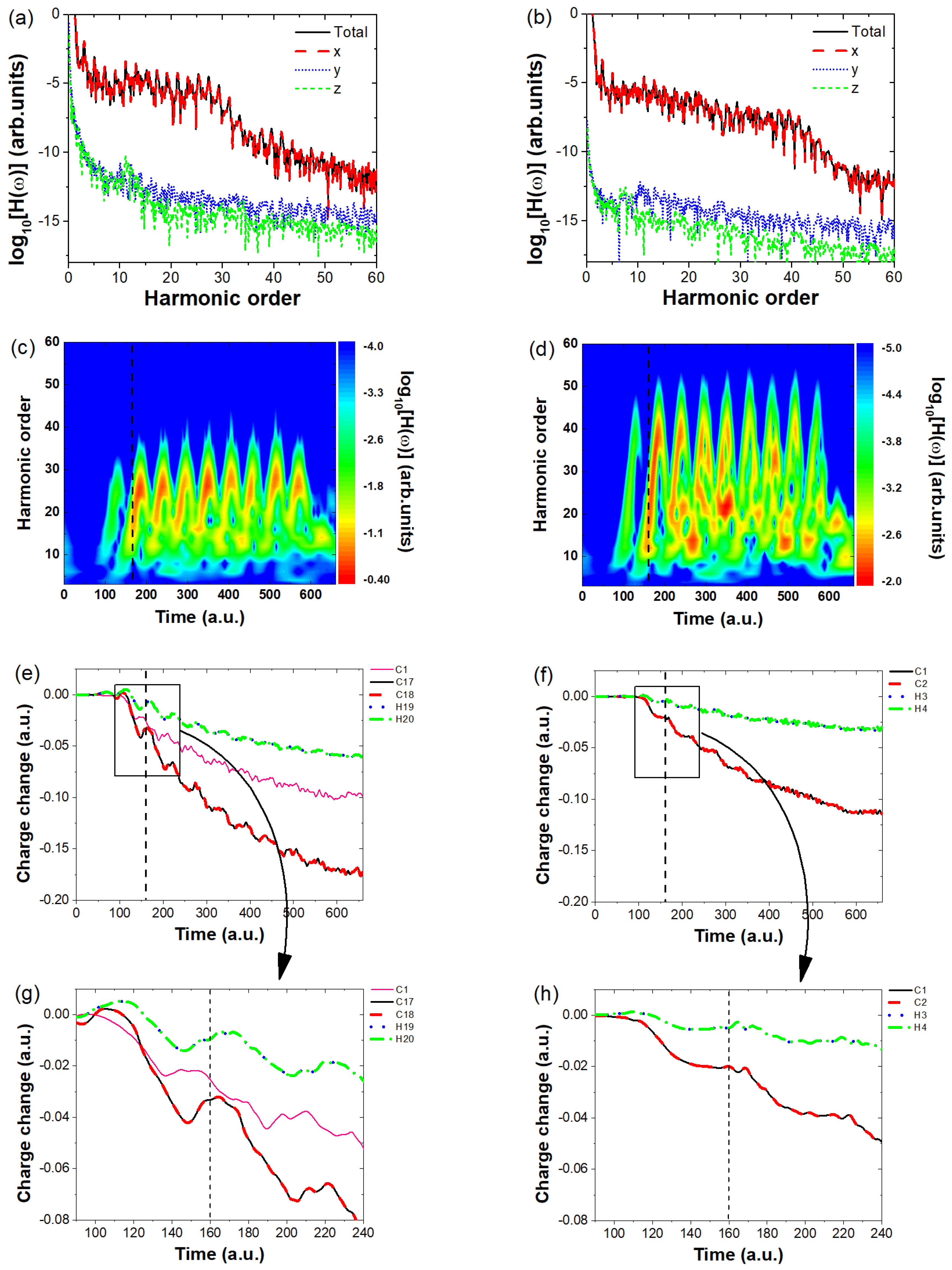
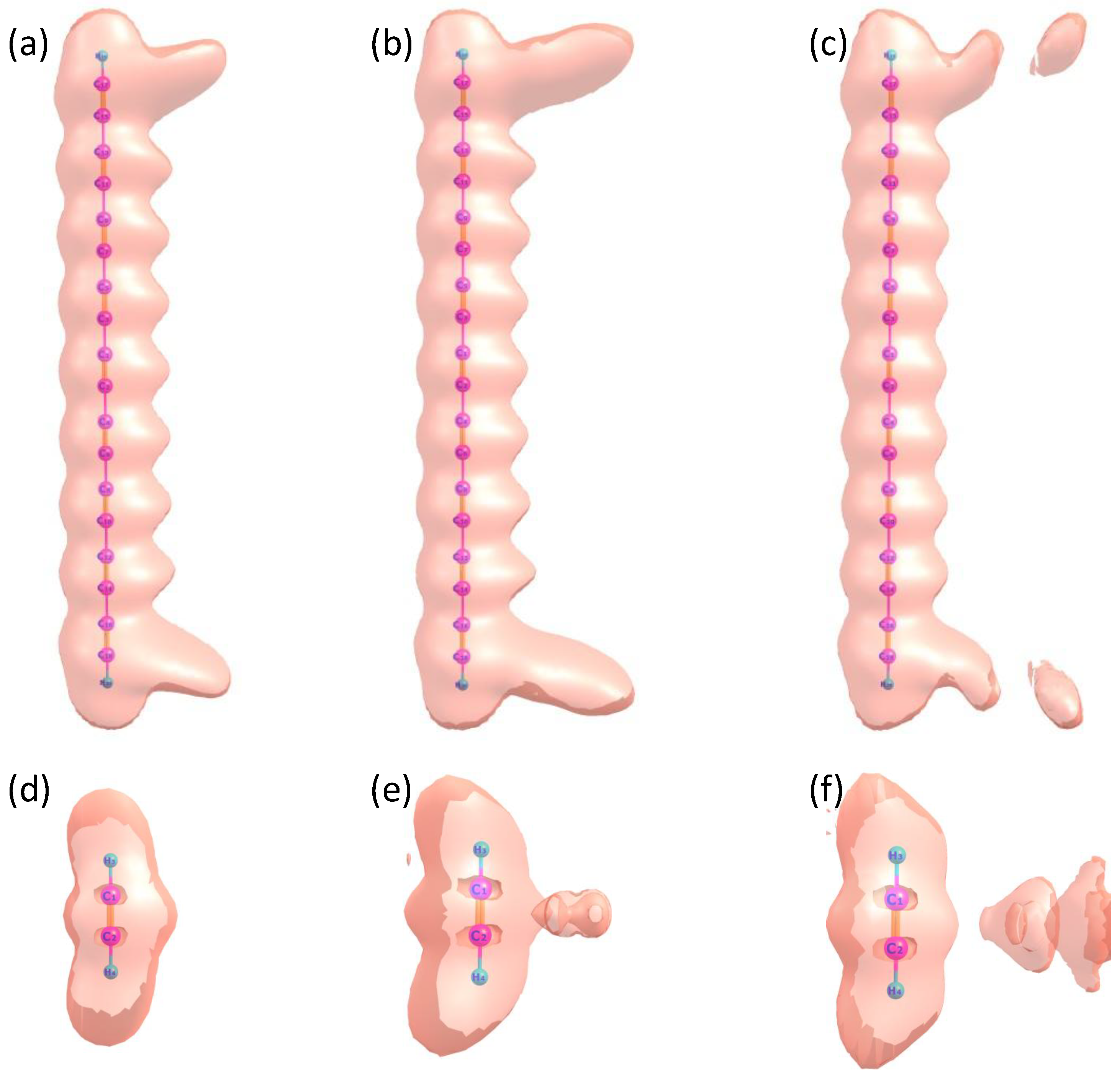
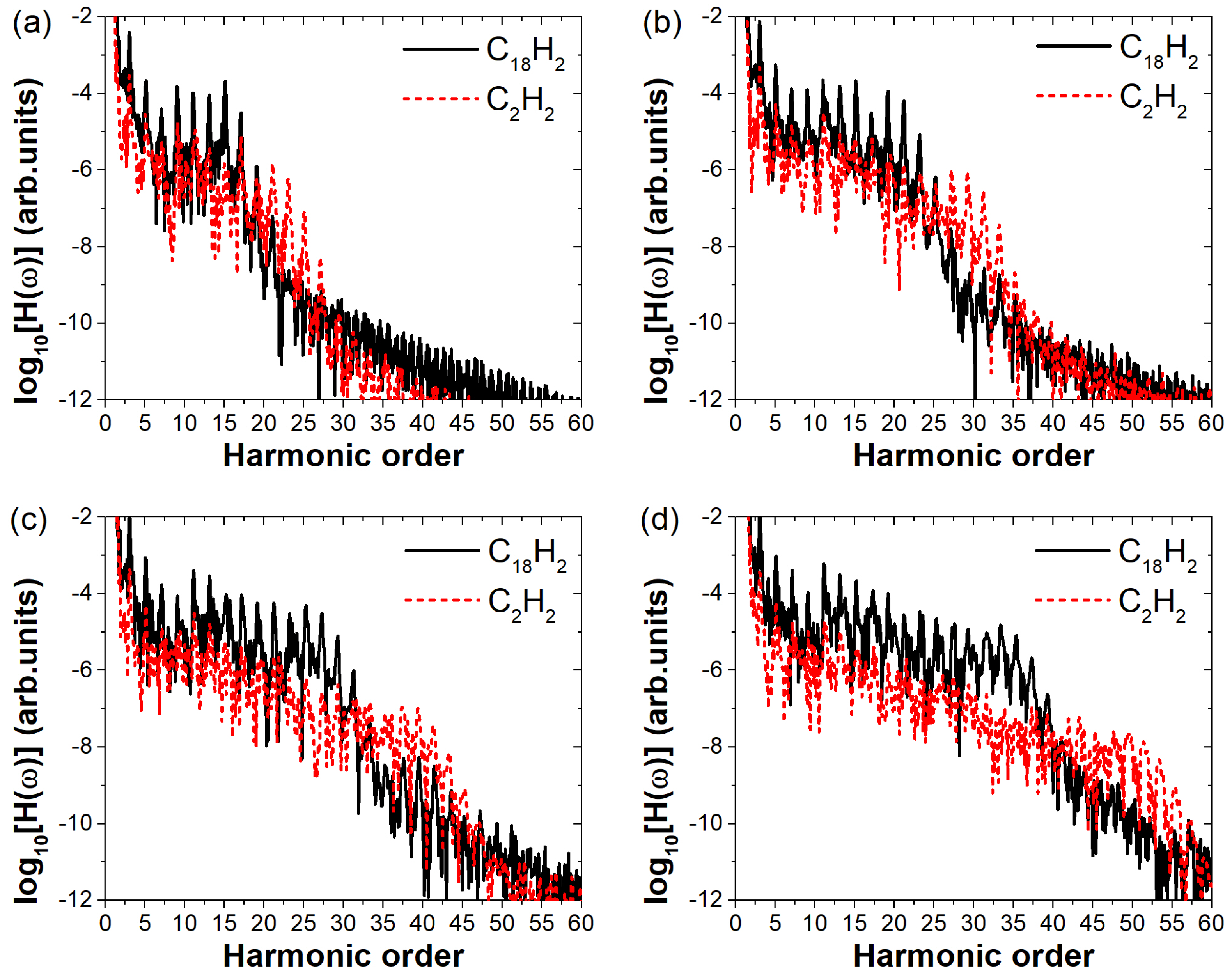
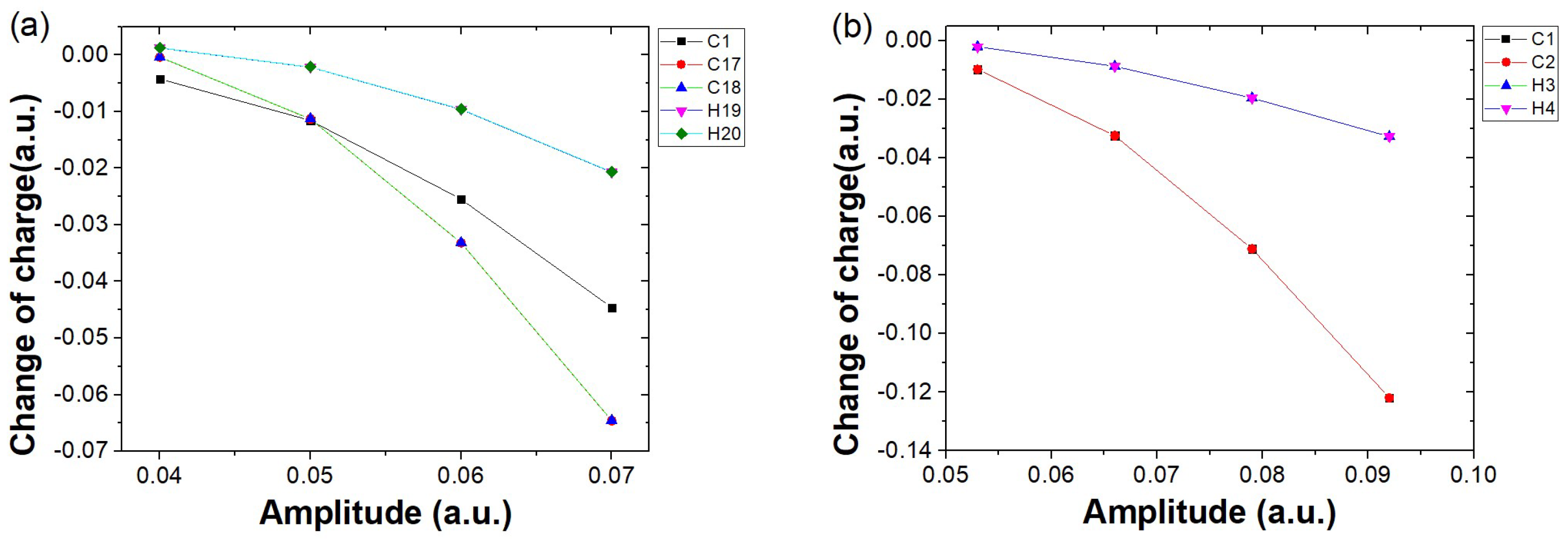
2. Results and Discussion
3. Theory and Method
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Marcos Dantus, M.J.R.; Zewail, A.H. Real-time femtosecond probing of “transition states” in chemical reactions. J. Chem. Phys. 1987, 87, 2395. [Google Scholar] [CrossRef]
- Qiao, Y.; Zhang, S.; Jiang, W.; Guo, F.; Wang, J.; Chen, J.; Yang, Y. Modulation of harmonics from solids by laser pulses with a small chirp. Phys. Rev. A 2025, 111, 013501. [Google Scholar] [CrossRef]
- Xu, N.; Zhou, S.S.; Wang, Y. Regulation of helium atom higher harmonic emission and attosecond pulse angle in inhomogeneous fields. Results Phys. 2025, 72, 108190. [Google Scholar] [CrossRef]
- Peters, M.; Dang, T.N.; Charron, E.; Keller, A.; Atabek, O. Laser-induced electron diffraction: A tool for molecular orbital imaging. Phys. Rev. A 2012, 85, 053417. [Google Scholar] [CrossRef]
- Spielmann, C.; Burnett, N.H.; Sartania, S.; Koppitsch, R.; Schnorer, M.; Kan, C.; Lenzner, M.; Wobrauschek, P.; Krausz, F. Generation of Coherent X-rays in the Water Window Using 5-Femtosecond Laser Pulses. Science 1997, 278, 661–664. [Google Scholar] [CrossRef]
- L’Huillier, A.; Balcou, P. High-order harmonic generation in rare gases with a 1-ps 1053-nm laser. Phys. Rev. Lett. 1993, 70, 774–777. [Google Scholar] [CrossRef] [PubMed]
- Macklin, J.J.; Kmetec, J.D.; Gordon, C.L. High-order harmonic generation using intense femtosecond pulses. Phys. Rev. Lett. 1993, 70, 766–769. [Google Scholar] [CrossRef]
- Popmintchev, T.; Chen, M.C.; Popmintchev, D.; Arpin, P.; Brown, S.; Ališauskas, S.; Andriukaitis, G.; Balčiunas, T.; Mücke, O.D.; Pugzlys, A.; et al. Bright Coherent Ultrahigh Harmonics in the keV X-ray Regime from Mid-Infrared Femtosecond Lasers. Science 2012, 336, 1287–1291. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.; Jiang, W.; Qiao, Y.; Yang, Y.; Chen, J. Generation of Isolated Attosecond Pulses by the Harmonic Spectrum of MgO under a Three-Color Laser Pulse. Chin. Phys. Lett. 2025, 42, 013201. [Google Scholar] [CrossRef]
- Qiao, Y.; Chen, J.; Zhou, S.; Chen, J.; Jiang, S.; Yang, Y. Modulation of High-Order Harmonic Generation from a Monolayer ZnO by Co-rotating Two-Color Circularly Polarized Laser Fields. Chin. Phys. Lett. 2024, 41, 014205. [Google Scholar] [CrossRef]
- Corkum, P.B. Plasma perspective on strong field multiphoton ionization. Phys. Rev. Lett. 1993, 71, 1994–1997. [Google Scholar] [CrossRef] [PubMed]
- Xing, M.; Wang, J.; Zhao, X.; Zhou, S. The Role of Multi-Electron and Multi-Orbital Effects in High-Order Harmonic Generation of Benzonitrile Molecules. Chin. Phys. Lett. 2025, 42, 043201. [Google Scholar] [CrossRef]
- Hentschel, M.; Kienberger, R.; Spielmann, C.; Reider, G.A.; Milosevic, N.; Brabec, T.; Corkum, P.; Heinzmann, U.; Drescher, M.; Krausz, F. Attosecond metrology. Nature 2001, 414, 509–513. [Google Scholar] [CrossRef]
- Paul, P.M.; Toma, E.S.; Breger, P.; Mullot, G.; Augé, F.; Balcou, P.; Muller, H.G.; Agostini, P. Observation of a Train of Attosecond Pulses from High Harmonic Generation. Science 2001, 292, 1689–1692. [Google Scholar] [CrossRef]
- Takahashi, E.J.; Pengfei, L.; Oliver, D.M.; Yasuo, N.; Katsumi, M. Attosecond nonlinear optics using gigawatt-scale isolated attosecond pulses. Nat. Commun. 2013, 4, 2691. [Google Scholar] [CrossRef]
- Ayuso, D.; Jiménez-Galán, A.; Morales, F.; Ivanov, M.; Smirnova, O. Attosecond control of spin polarization in electron–ion recollision driven by intense tailored fields. New J. Phys. 2017, 19, 073007. [Google Scholar] [CrossRef]
- Vozzi, C.; Negro, M.; Calegari, F.; Sansone, G.; Nisoli, M.; Silvestri, S.D.; Stagira, S. Generalized molecular orbital tomography. Nat. Phys. 2011, 7, 822–826. [Google Scholar] [CrossRef]
- Niikura, H.; Dudovich, N.; Villeneuve, D.M.; Corkum, P.B. Mapping Molecular Orbital Symmetry on High-Order Harmonic Generation Spectrum Using Two-Color Laser Fields. Phys. Rev. Lett. 2010, 105, 053003. [Google Scholar] [CrossRef]
- Itatani, J.; Levesque, J.; Zeidler, D.; Hiromichi, N.; Pépin, H.; Kieffer, J.C.; Corkum, P.B.; Villeneuve, D.M. Tomographic imaging of molecular orbitals. Nature 2004, 432, 867–871. [Google Scholar] [CrossRef]
- Sansone, G.; Benedetti, E.; Calegari, F.; Vozzi, C.; Avaldi, L.; Flammini, R.; Poletto, L.; Villoresi, P.; Altucci, C.; Velotta, R.; et al. Isolated Single-Cycle Attosecond Pulses. Science 2006, 314, 443–446. [Google Scholar] [CrossRef]
- Puthumpally-Joseph, R.; Viau-Trudel, J.; Peters, M.; Nguyen-Dang, T.T.; Atabek, O.; Charron, E. Inversion of strong-field photoelectron spectra for molecular orbital imaging. Phys. Rev. A 2016, 94, 023421. [Google Scholar] [CrossRef]
- Li, J.B.; Zhang, X.; Yue, S.J.; Wu, H.M.; Hu, B.T.; Du, H.C. Enhancement of the second plateau in solid high-order harmonic spectra by the two-color fields. Opt. Express 2017, 25, 18603–18613. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.; Guo, C.; Vampa, G.; Zhang, J.L.; Sarmiento, T.; Xiao, M.; Bucksbaum, P.H.; Vučković, J.; Fan, S.; Reis, D.A. Enhanced high-harmonic generation from an all-dielectric metasurface. Nat. Phys. 2018, 14, 1006–1010. [Google Scholar] [CrossRef]
- Franz, D.; Kaassamani, S.; Gauthier, D.; Nicolas, R.; Kholodtsova, M.; Douillard, L.; Gomes, J.T.; Lavoute, L.; Gaponov, D.; Ducros, N.; et al. All semiconductor enhanced high-harmonic generation from a single nanostructured cone. Sci. Rep. 2019, 9, 5663. [Google Scholar] [CrossRef]
- Yao, D.-H.; Bo, C.; Ma, S.-Q.; Chao, Y.; Lu, R.-F. Enhancing high harmonic generation in bilayer MoS2 by interlayer atomic dislocation. Acta Phys. Sin. 2021, 70, 18–24. [Google Scholar] [CrossRef]
- Kamta-Lagmago, G.; Bandrauk, A.D. Phase dependence of enhanced ionization in asymmetric molecules. Phys. Rev. Lett. 2005, 94, 203003. [Google Scholar] [CrossRef]
- Li, Y.-P.; Yu, S.-J.; Chen, Y.-J. Wavelength-dependent perpendicular-harmonics efficiency from oriented CO2 molecule. Acta Phys. Sin. 2015, 64, 234–241. [Google Scholar]
- Shi, Y.Z.; Zhang, B.; Li, W.Y.; Yu, S.J.; Chen, Y.J. Probing degrees of orientation of polar molecules with harmonic emission in ultrashort laser pulses. Phys. Rev. A 2017, 95, 033406. [Google Scholar] [CrossRef]
- Takahashi, E.J.; Kanai, T.; Ishikawa, K.J.; Nabekawa, Y.; Midorikawa, K. Dramatic Enhancement of High-Order Harmonic Generation. Phys. Rev. Lett. 2007, 99, 053904. [Google Scholar] [CrossRef]
- Hu, J.; Li, X. Influence of high harmonic generation of isotopic molecules. High Power Laser Part. Beams 2010, 22, 1348–1350. [Google Scholar]
- Romanov, A.A.; Silaev, A.A.; Frolov, M.V.; Vvedenskii, N.V. Influence of the polarization of a multielectron atom in a strong laser field on high-order harmonic generation. Phys. Rev. A 2020, 101, 013435. [Google Scholar] [CrossRef]
- Runge, E.; Gross, E.K.U. Density-Functional Theory for Time-Dependent Systems. Phys. Rev. Lett. 1984, 52, 997. [Google Scholar] [CrossRef]
- Schirmer, J. Review of the foundations of time-dependent density-functional theory (TDDFT). Phys. Chem. Chem. Phys. 2025, 27, 4992–5005. [Google Scholar] [CrossRef] [PubMed]
- Zhou, S.; Wang, H.; Hu, M.; Sun, Y.; Zhao, X. Review of the Generation, Regulation, and Applications of High-Order Harmonic Generation in Gases Studied Using Time-Dependent Density Functional Theory. Symmetry 2025, 17, 359. [Google Scholar] [CrossRef]
- Castro, A.; Appel, H.; Oliveira, M.; Rozzi, C.A.; Andrade, X.; Lorenzen, F.; Marques, M.A.L.; Gross, E.K.U.; Rubio, A. octopus: A tool for the application of time-dependent density functional theory. Phys. Status Solidi B 2006, 243, 2465–2488. [Google Scholar] [CrossRef]
- Tancogne-Dejean, N.; Mücke, O.D.; Kärtner, F.X.; Rubio, A. llipticity dependence of high-harmonic generation in solids originating from coupled intraband and interband dynamics. Nat. Commun. 2017, 8, 745. [Google Scholar] [CrossRef]
- Keldysh, L.V. Ionization in the Field of a Strong Electromagnetic Wave. J. Exp. Theor. Phys. 1965, 20, 1307–1314. [Google Scholar]
- Krause, J.L.; Schafer, K.J.; Kulander, K.C. High-order harmonic generation from atoms and ions in the high intensity regime. Phys. Rev. Lett. 1992, 68, 3535–3538. [Google Scholar] [CrossRef]
- Graves, C.E.; Reid, A.H.; Wang, T.; Wu, B.; de Jong, S.; Vahaplar, K.; Radu, I.; Bernstein, D.P.; Messerschmidt, M.; Müller, L.; et al. Nanoscale spin reversal by non-local angular momentum transfer following ultrafast laser excitation in ferrimagnetic GdFeCo. Nat. Mater. 2013, 12, 293–298. [Google Scholar] [CrossRef]
- Ofer, N.; Zahra, N.; Nicolas, T.; Angel, R. Ab Initio Cluster Approach for High Harmonic Generation in Liquids. J. Chem. Theory Comput. 2022, 18, 4117–4126. [Google Scholar]
- Angana, M.; Ofer, N.; Zhong, Y.; Zahra, N.; Vít, S.; Angel, R.; Nicolas, T.D.; Jakob, W.H. High-harmonic spectroscopy of low-energy electron-scattering dynamics in liquids. Nat. Phys. 2023, 19, 1813–1820. [Google Scholar]
- Kepceoglu, A.; Gundogdo, Y.; Dereli, O.; Kilic, H.S. Molecular Structure and TD-DFT Study of the Xylene Isomers. Gazi Univ. J. Sci. 2019, 32, 300–308. [Google Scholar]
- Khan, M.F.S.; Wu, J.; Liu, B.; Cheng, C.; Akbar, M.; Chai, Y.; Memon, A. Fluorescence and photophysical properties of xylene isomers in water: With experimental and theoretical approaches. R. Soc. 2018, 5, 171719. [Google Scholar] [CrossRef]
- Romeo-Gella, F.; Corral, I.; Faraji, S. Theoretical investigation of a novel xylene-based light-driven unidirectional molecular motor. J. Chem. Phys. 2021, 154, 064111. [Google Scholar] [CrossRef]
- Zeng, A.W.; Bian, X.B. Impact of Statistical Fluctuations on High Harmonic Generation in Liquids. Phys. Rev. Lett. 2020, 124, 203901. [Google Scholar] [CrossRef] [PubMed]
- Vampa, G.; McDonald, C.; Orlando, G.; Corkum, P.B.; Brabec, T. Semiclassical analysis of high harmonic generation in bulk crystals. Phys. Rev. B Condens. Matter Mater. Phys. 2015, 91, 064302. [Google Scholar] [CrossRef]
- Zhao, Y.T.; Ma, S.Y.; Jiang, S.C.; Yang, Y.J.; Zhao, X.; Chen, J.G. All-optical reconstruction of k-dependent transition dipole moment by solid harmonic spectra from ultrashort laser pulses. Opt. Express 2019, 27, 34392–34404. [Google Scholar] [CrossRef]
- Liu, L.; Zhao, J.; Dong, W.; Liu, J.; Huang, Y.; Zhao, Z. Spatial coherence in high-order-harmonic generation from periodic solid structures. Phys. Rev. A 2017, 96, 053403. [Google Scholar] [CrossRef]
- Jin, J.Z.; Xiao, X.R.; Liang, H.; Wang, M.X.; Chen, S.G.; Gong, Q.; Peng, L.Y. High-order harmonic generation from a two-dimensional band structure. Phys. Rev. A 2018, 97, 043420. [Google Scholar] [CrossRef]
- Ghimire, S.; DiChiara, A.D.; Sistrunk, E.; Agostini, P.; DiMauro, L.F.; Reis, D.A. Observation of high-order harmonic generation in a bulk crystal. Nat. Phys. 2011, 7, 138–141. [Google Scholar] [CrossRef]
- Qiao, Y.; Chen, J.; Li, Z.; Liu, Y.; Jiang, S.; Liu, W.; Yang, Y.; Chen, J. Analysis on the minimum structure of harmonic spectra from MgO crystals. Opt. Lett. 2024, 49, 3986–3989. [Google Scholar] [CrossRef] [PubMed]
- Klemke, N.; Tancogne-Dejean, N.; Rossi, G.; Yang, Y.; Scheiba, F.; Mainz, R.; Sciacca, G.D.; Rubio, A.; Kaertner, F.; Muecke, O. Polarization-state-resolved high-harmonic spectroscopy of solids. Nat. Commun. 2019, 10, 1319. [Google Scholar] [CrossRef] [PubMed]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, S.; Wang, H.; Yu, D.; Xu, N.; Hu, M. Enhancing High-Order Harmonic Generation Efficiency Through Molecular Size and Orientation Effects: A Pathway to Ultrafast Chemical Dynamics Studies. Molecules 2025, 30, 2133. https://doi.org/10.3390/molecules30102133
Zhou S, Wang H, Yu D, Xu N, Hu M. Enhancing High-Order Harmonic Generation Efficiency Through Molecular Size and Orientation Effects: A Pathway to Ultrafast Chemical Dynamics Studies. Molecules. 2025; 30(10):2133. https://doi.org/10.3390/molecules30102133
Chicago/Turabian StyleZhou, Shushan, Hao Wang, Dongming Yu, Nan Xu, and Muhong Hu. 2025. "Enhancing High-Order Harmonic Generation Efficiency Through Molecular Size and Orientation Effects: A Pathway to Ultrafast Chemical Dynamics Studies" Molecules 30, no. 10: 2133. https://doi.org/10.3390/molecules30102133
APA StyleZhou, S., Wang, H., Yu, D., Xu, N., & Hu, M. (2025). Enhancing High-Order Harmonic Generation Efficiency Through Molecular Size and Orientation Effects: A Pathway to Ultrafast Chemical Dynamics Studies. Molecules, 30(10), 2133. https://doi.org/10.3390/molecules30102133






