F-p Hybridization-Induced Ferromagnetism for Ultrathin Two-Dimensional Ferromagnetic Half-Metal (EuN) Monolayer: A First-Principles Study
Abstract
1. Introduction
2. Results and Discussion
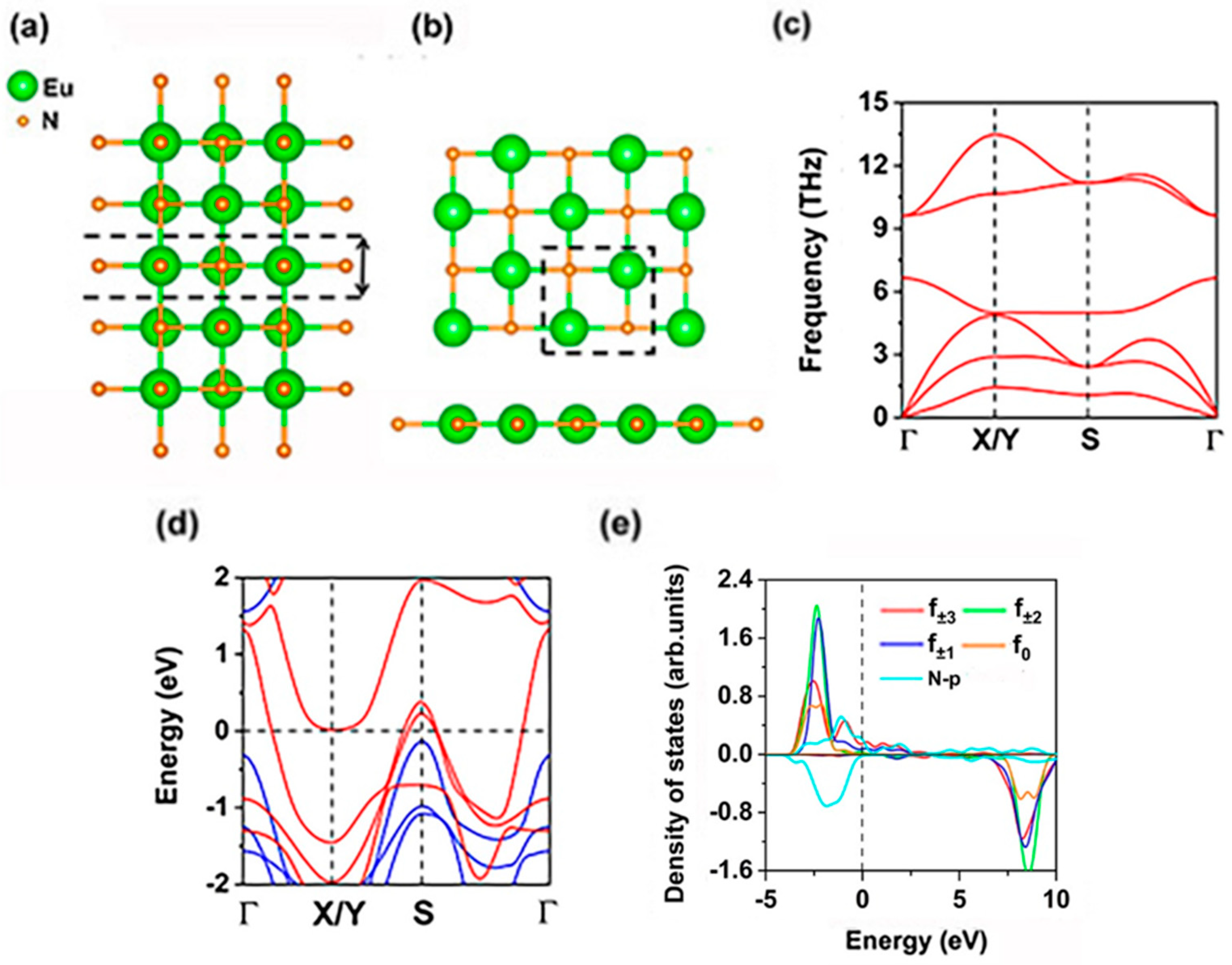
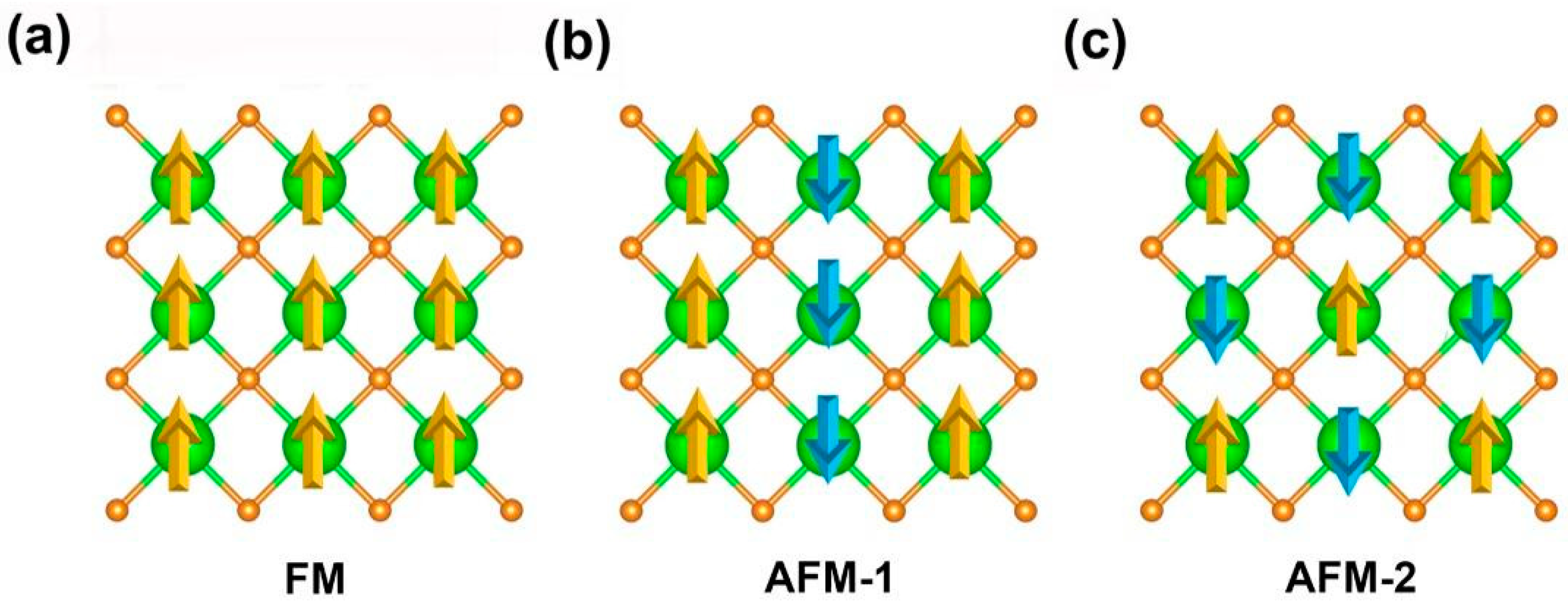
2.1. Ground State, Stability, Electronic and Magnetic Properties of EuN Monolayer
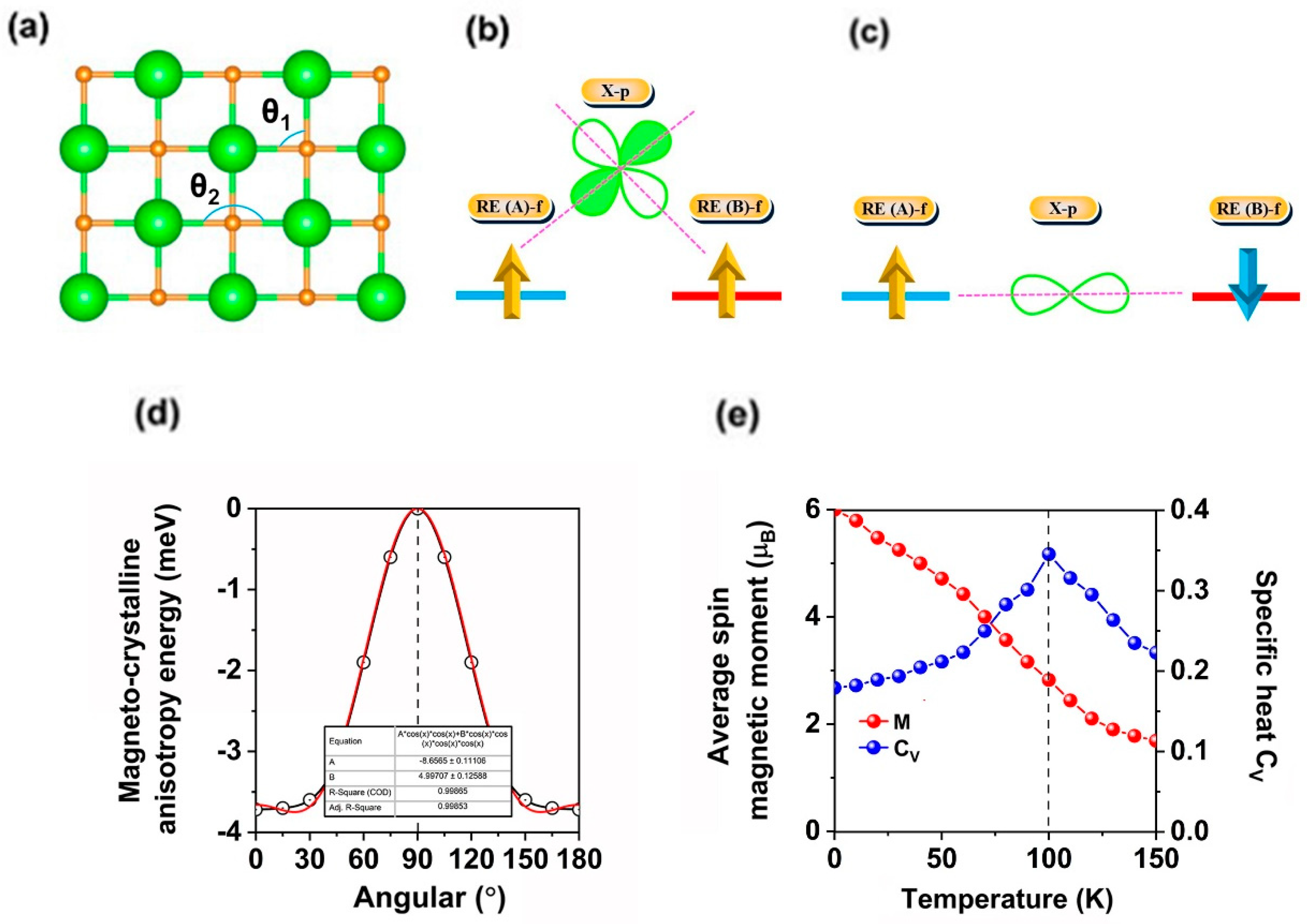
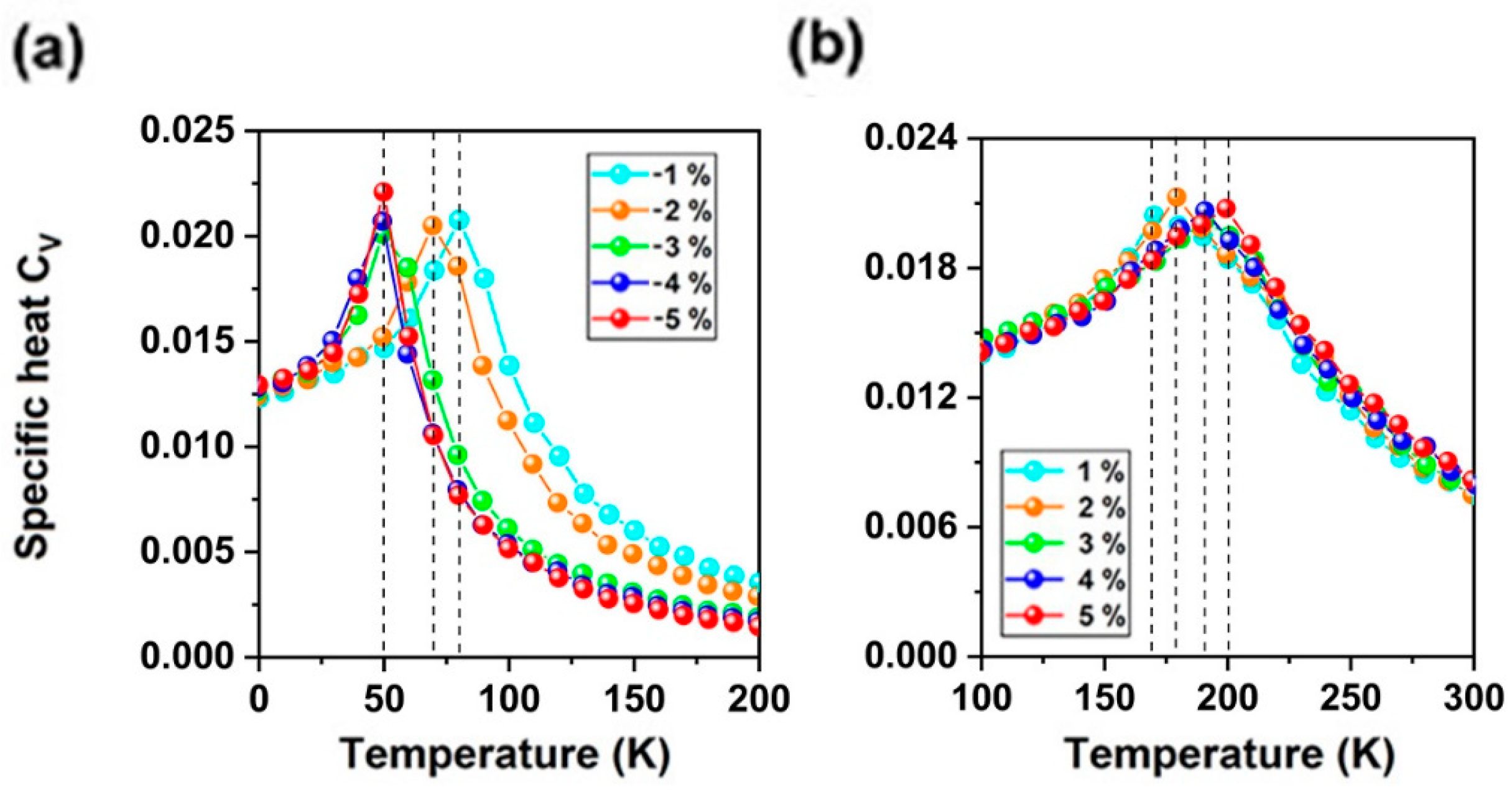
2.2. Curie Temperature and Magnetic Anisotropy Energy of EuN Monolayer
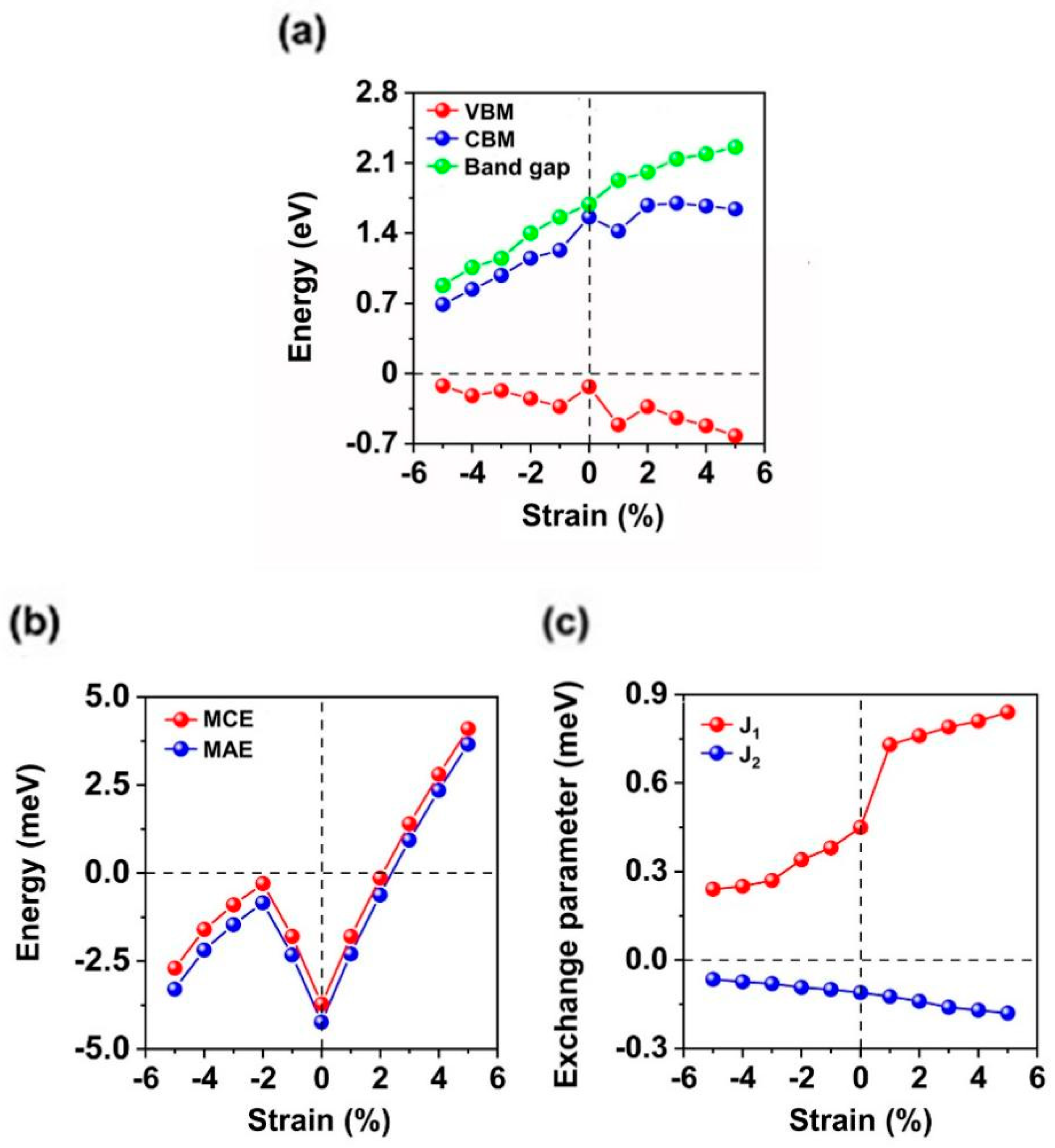
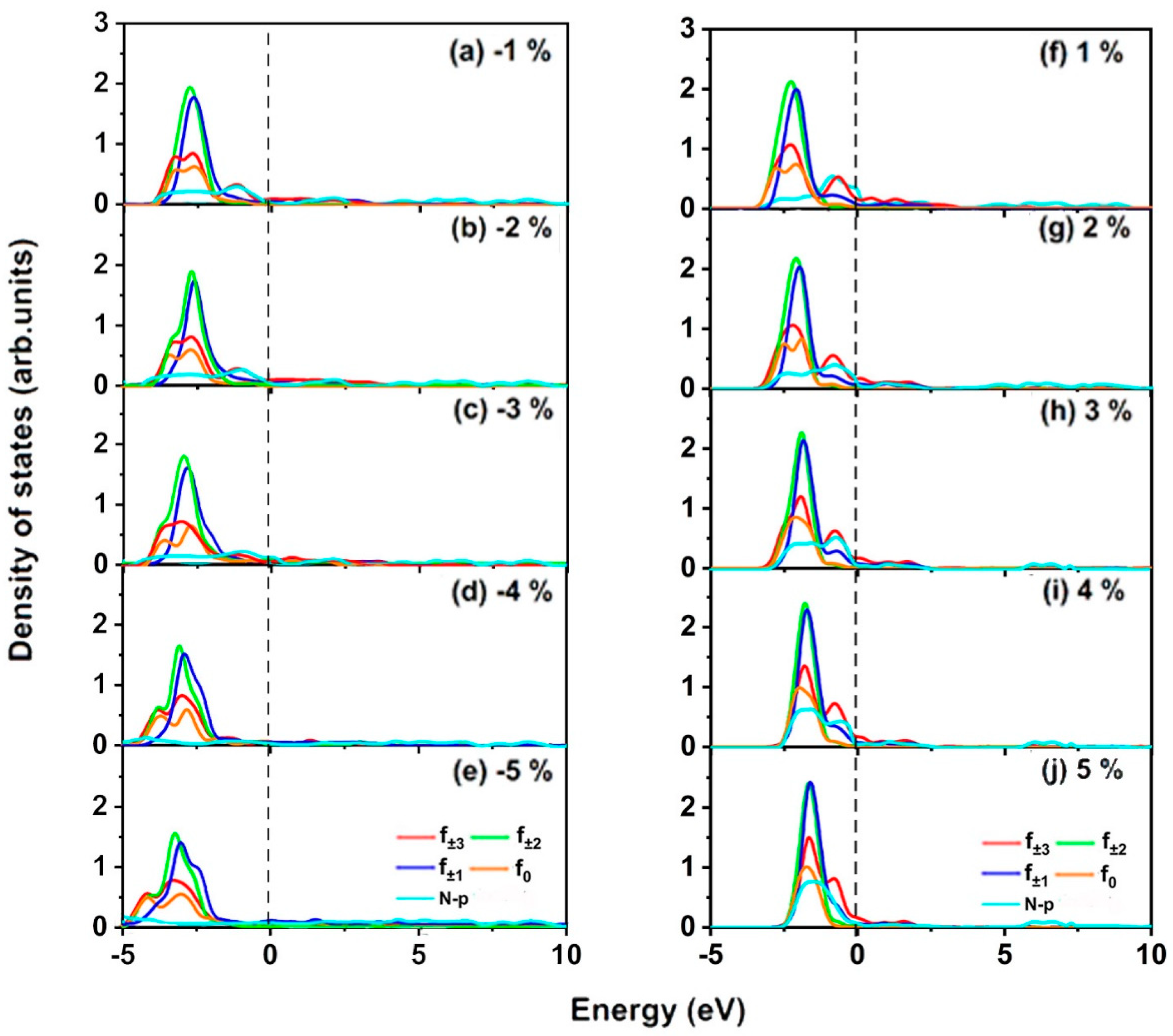
2.3. Tunable Electronic and Magnetic Properties Under Biaxial Strain
2.4. Discussion About Experimental Fabrication of EuN Monolayer
3. Computational Methods
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Fei, Z.Y.; Huang, B.; Malinowski, P.; Wang, W.; Song, T.C.; Sanchez, J.; Yao, W.; Xiao, D.; Zhu, X.Y.; May, A.F.; et al. Two-dimensional itinerant ferromagnetism in atomically thin Fe3GeTe2. Nat Mater. 2008, 17, 778. [Google Scholar] [CrossRef]
- Gong, C.; Zhang, X. Two-dimensional magnetic crystals and emergent heterostructure devices. Science 2019, 363, 706. [Google Scholar] [CrossRef]
- Lu, S.H.; Zhou, Q.H.; Guo, Y.L.; Zhang, Y.H.; Wu, Y.L.; Wang, J.L. Coupling a Crystal Graph multilayer descriptor to active learning for rapid discovery of 2D ferromagnetic semiconductors/half- Metals/metals. Adv Mater. 2020, 32, 2002658. [Google Scholar] [CrossRef] [PubMed]
- Tang, B.J.; Wang, X.W.; Han, M.J.; Xu, X.D.; Zhang, Z.W.; Zhu, C.; Cao, X.; Yang, Y.M.; Fu, Q.D.; Yang, J.Q. Phase engineering of Cr5Te8 with colossal anomalous Hall effect. Nat. Electron. 2022, 5, 224. [Google Scholar] [CrossRef]
- Cui, F.F.; Zhao, X.X.; Xu, J.J.; Tang, B.; Shang, Q.Y.; Xing, J.P.; Huan, Y.H.; Liao, J.H.; Chen, Q.; Hou, Y.L. Controlled growth and thickness-dependent conduction-type transition of 2D ferrimagnetic Cr2 S3 semiconductors. Adv. Mater. 2020, 32, 1905896. [Google Scholar] [CrossRef] [PubMed]
- Pal, R.J.; Abraham, J.J.; Mistonov, A.; Mishra, S.; Stilkerich, N.; Mondal, S.; Mandal, P.; Pal, A.N.; Geck, J.; Buechner, B. Disentangling the unusual magnetic anisotropy of the near-room-temperature ferromagnet Fe4GeTe2. Adv. Funct. Mater. 2024, 34, 2402551. [Google Scholar] [CrossRef]
- Wang, H.; Wen, Y.; Zhao, X.X.; Cheng, R.Q.; Yin, L.; Zhai, B.X.; Jiang, J.; Li, Z.W.; Liu, C.S.; Wu, F.C. Heteroepitaxy of 2D CuCr2Te4 with robust room-temperature ferromagnetism. Adv. Mater. 2023, 35, 2211388. [Google Scholar] [CrossRef] [PubMed]
- Ding, Y.; Wang, Y.L. Intrinsic ferromagnetism and valley polarization in hydrogenated group V transition-metal dinitride (MH2N2, M=V/Nb/Ta) nanosheets: Insights from first-principles. Nanoscale 2020, 12, 1002. [Google Scholar] [CrossRef]
- Hu, X.W.; Yao, D.X.; Cao, K. Tuning magnetic anisotropy in a Fe5GeTe2 monolayer through doping and strain. Phys. Rev. B 2024, 110, 184418. [Google Scholar] [CrossRef]
- Ma, Y.F.; Yao, R.; Wu, J.R.; Gao, Z.S.; Luo, F. Unusual anomalous hall effect in two-dimensional ferromagnetic Cr7Te8. Molecules 2024, 29, 5068. [Google Scholar] [CrossRef]
- Hu, Y.; Liu, X.Y.; Shen, Z.H.; Luo, Z.F.; Chen, Z.G.; Fan, X.L. High Curie temperature and carrier mobility of novel Fe, Co and Ni carbide MXenes. Nanoscale 2020, 12, 11627. [Google Scholar] [CrossRef] [PubMed]
- Hu, Y.; Gong, Y.H.; Zeng, H.H.; Wang, J.H.; Fan, X.L. Two-dimensional stable Fe-based ferromagnetic semiconductors: FeI3 and FeI1.5Cl1.5 monolayers. Phys. Chem. Chem. Phys. 2020, 22, 24506. [Google Scholar] [CrossRef] [PubMed]
- Tokmachev, A.M.; Averyanov, D.V.; Taldenkov, A.N.; Parfenov, O.E.; Karateev, I.A.; Sokolov, I.S.; Storchak, V.G. Lanthanide f7 metalloxenes-a class of intrinsic 2D ferromagnets. Mater. Horiz. 2019, 6, 1488. [Google Scholar] [CrossRef]
- Huang, B.; Clark, G.; Moratalla, E.N.; Klein, D.R.; Cheng, R.; Seyler, K.L.; Zhong, D.; Schmidgall, E.; McGuire, M.A.; Cobben, D.H.; et al. Layer-dependent ferromagnetism in a van der waals crystal down to the monolayer limit. Nature 2017, 546, 270. [Google Scholar] [CrossRef]
- Gong, C.; Li, L.; Li, Z.L.; Ji, H.W.; Stern, A.; Xia, Y.; Cao, T.; Bao, W.; Wang, C.Z.; Wang, Y.; et al. Discovery of intrinsic ferromagnetism in two- dimensional van der waals crystals. Nature 2017, 546, 265. [Google Scholar] [CrossRef]
- Tokmachev, A.M.; Averyanov, D.V.; Parfenov, O.E.; Taldenkov, A.N.; Karateev, I.A.; Sokolov, I.S.; Kondratev, O.A.; Storchak, V.G. Emerging two-dimensional ferromagnetism in silicene materials. Nat. Commun. 2018, 9, 1672. [Google Scholar] [CrossRef]
- Wang, B.; Zhang, X.W.; Zhang, Y.H.; Yuan, S.J.; Guo, Y.L.; Dong, S.; Wang, J.L. Prediction of a two-dimensional high-Tc f-electron ferromagnetic semiconductor. Mater. Horiz. 2020, 7, 1623. [Google Scholar] [CrossRef]
- Zhao, Y.H.; Lin, L.F.; Zhou, Q.H.; Li, Y.H.; Yuan, S.J.; Chen, Q.; Dong, S.; Wang, J.L. Surface vacancy-induced switchable electric polarization and enhanced ferromagnetism in monolayer metal trihalides. Nano Lett. 2018, 18, 2943. [Google Scholar] [CrossRef]
- Zhang, X.W.; Wang, B.; Guo, Y.L.; Zhang, Y.H.; Chen, Y.F.; Wang, J.L. High Curie temperature and intrinsic ferromagnetic half-metallicity in two-dimensional Cr3X4 (X=S, Se, Te) nanosheets. Nanoscale Horiz. 2019, 4, 859. [Google Scholar] [CrossRef]
- Huang, C.X.; Feng, J.S.; Wu, F.; Ahmed, D.; Huang, B.; Xiang, H.J.; Deng, K.M.; Kan, E.J. Toward Intrinsic room-temperature ferromagnetism in two-dimensional semiconductors. J. Am. Chem. Soc. 2018, 140, 11519. [Google Scholar] [CrossRef]
- Deng, Y.J.; Jun, Y.Y.; Chen, S.Y.; Zhao, Z.J.; Zhou, W.N.; Yuan, S.Z.; Fan, Y.Y.; Zheng, W.Y.; Wei, W.S.; Yi, Z.J.; et al. Gate-tunable room-temperature ferromagnetism in two-dimensional Fe3GeTe2. Nature 2018, 563, 94. [Google Scholar] [CrossRef] [PubMed]
- Stöhr, J.; Siegmann, H.C. Magnetism: From Fundamentals to Nanoscale Dynamics; Springer: Berlin/Heidelberg, Germany, 2006; p. 274. [Google Scholar]
- Kumar, H.; Frey, N.C.; Dong, L.; Anasori, B.; Gogotsi, Y.; Shenoy, V.B. Tunable magnetism and transport properties in Nitride MXenes. ACS Nano 2017, 11, 7648. [Google Scholar] [CrossRef]
- Farooq, M.U.; Khan, I.; Moaied, M.; Hong, J. Hydrogen functionalization induced two-dimensional ferromagnetic semiconductor in Mn di-halide systems. Phys. Chem. Chem. Phys. 2017, 19, 29516. [Google Scholar] [CrossRef]
- Hu, Y.; Jin, S.; Luo, Z.F.; Zeng, H.H.; Wang, J.H.; Fan, X.L. Conversation from anti-ferromagnetic MnBr2 to ferromagnetic Mn3Br8 monolayer with large MAE. Nanoscale Res Lett. 2021, 16, 72. [Google Scholar] [CrossRef] [PubMed]
- Gorkan, T.; Vatansever, E.; Aklncl, Ü.; Gökoglu, G.E.; AktÜrk, E.; Ciraci, S. Above room temperature ferromagnetism in Gd2B2 monolayer with high magnetic anisotropy. J. Phys. Chem. C 2020, 124, 12816. [Google Scholar] [CrossRef]
- Gao, Z.H.; Yin, Y.H.; Wang, Y.W.; Cui, Z.C.; Cao, T.F.; Shi, J.Q.; Fan, X.L. Tunable electrical properties and multiple phases of ferromagnetic GdS2, GdSe2 and Janus GdSSe monolayers. Phys. Chem. Chem. Phys. 2023, 25, 22782. [Google Scholar] [CrossRef] [PubMed]
- Li, S.Z.; Si, J.S.; Yang, Z.X.; Zhang, W.B. Weyl nodal loop semimetals and tunable quantum anomalous Hall states in two-dimensional ferromagnetic cerium monohalides. Phys. Rev. B 2024, 109, 115418. [Google Scholar] [CrossRef]
- Li, C.Q.; An, Y.K. Two-dimensional ferromagnetic semiconductors of rare-earth Janus 2H-GdIBr monolayers with large valley polarization. Nanoscale 2023, 15, 8304. [Google Scholar] [CrossRef]
- Zhang, D.H.; Li, A.L.; Zhang, B.; Zhou, W.Z.; Duan, H.M.; Ouyang, F.P. Combined piezoelectricity, valley splitting and Dzyaloshinskii-Moriya interaction in Janus GdXY (X, Y = Cl, Br, I) magnetic semiconductors. Phys. Chem. Chem. Phys. 2023, 12, 8600. [Google Scholar] [CrossRef]
- Ding, F.S.; Ji, S.L.; Li, S.S.; Wang, L.X.; Wu, H.; Hu, Z.F.; Li, F.; Pu, Y. Prediction of intrinsic valley polarization in single-layer GdX2 (X =Br, Cl) from a first-principles study. Phys. Status Solidi B 2021, 258, 2100356. [Google Scholar] [CrossRef]
- Brown, R.C.; Clark, N.J. Composition limits and vaporization behavior of rare earth nitrides. J. Inorg. Nucl. Chem. 1974, 36, 2507. [Google Scholar] [CrossRef]
- Richter, J.H.; Ruck, B.J.; Simpson, M.; Natali, F.; Plank, N.O.V.; Azeem, M.; Trodahl, H.J.; Preston, A.R.H.; Chen, B.; Mcnulty, J.; et al. Electronic structure of EuN: Growth, spectroscopy, and theory. Phys. Rev. B 2011, 84, 235120. [Google Scholar] [CrossRef]
- Bouayed, M.K.; Said, S.G.; Merad, A.E.; Kanoun, M.B. Ab initio calculation of electronic structure and magnetic properties of rare earth nitride using LDA+U approach: EuN and GaEuN. Mater. Sci. Forum. 2009, 609, 167. [Google Scholar] [CrossRef]
- Larson, P.; Lambrecht, W.R.L. Electronic structure of rare-earth nitride using the LSDA+U approach: Importance of allowing 4f orbitals to break the cubic crystal symmetry. Phys. Rev. B 2007, 75, 045114. [Google Scholar] [CrossRef]
- Johannes, M.D.; Pickett, W.E. Magnetic coupling between nonmagnetic ions: Eu3+ in EuN and EuP. Phys. Rev. B 2005, 72, 195116. [Google Scholar] [CrossRef]
- Murugan, A.; Rajeswarapalanichamy, R.; Santhosh, M.; Iyakutti, K. Structural, electronic, mechanical and magnetic properties of rare earth nitrides REN (RE= Pm, Eu and Yb). J. Magn. Magn. Mater. 2015, 385, 441. [Google Scholar] [CrossRef]
- Price, J.; Borland, J.O.; Selbrede, S. Properties of chemical-vapor-deposited titanium nitride. Thin Solid Films 1993, 236, 311. [Google Scholar] [CrossRef]
- Ritala, M.; Leskelä, M.; Rauhala, E.; Jokinen, J. Atomic layer epitaxy growth of TiN thin films from TiI4 and NH3. J. Electrochem. Soc. 1998, 145, 2914. [Google Scholar] [CrossRef]
- Imhoff, L.; Bouteville, A.; Remy, J. Kinetics of the formation of titanium nitride layers by rapid thermal low pressure chemical vapor deposition from TiCl4-NH3-H2. J. Electrochem. Soc. 1998, 145, 1672. [Google Scholar] [CrossRef]
- Pelleg, J.; Zevin, L.; Lungo, S.; Croitoru, N. Reactive-sputter-deposited TiN films on glass substrates. Thin Solid Film. 1991, 197, 117. [Google Scholar] [CrossRef]
- Je, J.; Noh, D.; Kim, H.; Liang, K. Preferred orientation of TiN films studied by a real time synchrotron X-ray scattering. J. Appl. Phys. 1997, 81, 6126. [Google Scholar] [CrossRef][Green Version]
- Sun, Q.; Fu, Z.W. Vanadium nitride as a novel thin film anode material for rechargeable lithium batteries. Electrochim. Acta 2008, 54, 403. [Google Scholar] [CrossRef]
- Zhang, S.H.; Zhou, J.; Wang, Q.; Chen, X.S.; Kawazoe, Y.; Jena, P. You have access penta-graphene: A new carbon allotrope. Proc. Natl. Acad. Sci. USA 2015, 112, 2372. [Google Scholar] [CrossRef]
- Zhang, W.B.; Qu, Q.; Zuna, P.; Lam, C.H. Robust intrinsic ferromagnetism and half semi-conductivity in stable two-dimensional single-layer chromium trihalides. J. Mater. Chem. C 2015, 3, 12457. [Google Scholar] [CrossRef]
- Booth, T.J.; Blake, P.; Nair, R.R.; Jiang, D.; Hill, E.W.; Bangert, U.; Bleloch, A.; Gass, M.; Novoselov, K.S.; Katsnelson, M.I.; et al. Macroscopic graphene membranes and their extraordinary stiffness. Nano Lett. 2008, 8, 2442. [Google Scholar] [CrossRef]
- Miessler, G.L.; Tarr, D.A. Inorganic Chemistry; Prentice-Hall: New York, NY, USA, 1999; p. 358. [Google Scholar]
- Buschow, K.H.J.; Boer, F.R.D. Physics of Magnetism and Magnetic Materials; Kluwer Academic /Plenum Publishers: New York, NY, USA, 2003; p. 92. [Google Scholar]
- Yang, Y.; Wu, S.; Zhu, Z.Z.; Guo, G.Y. Large magneto-optical effects and magnetic anisotropy energy in two-dimensional Cr2Ge2Te6. Phys. Rev. B 2018, 98, 125416. [Google Scholar]
- Guo, G.Y.; Temmerman, W.M.; Ebert, H. 1st-principles determination of the magnetization direction of Fe monolayer in noble-metals. J. Phys. Condens. Matter. 1991, 3, 8205. [Google Scholar] [CrossRef]
- Ma, S.; Bo, X.Y.; Fu, L.; Liu, X.Y.; Wang, S.; Lan, M.X.; Li, S.S.; Huang, T.; Li, F.; Pu, Y. Two-dimensional honeycomb-kagome V2X3 (X=O, S, Se) with half-metallicity, high Curie temperature, and large magnetic anisotropic energy. J. Mater. Chem. C 2024, 12, 14172. [Google Scholar] [CrossRef]
- Bai, Y.H.; Wu, Y.X.; Jia, C.B.; Hou, L.P.; Wang, B. Two-dimensional 4f magnetic EuSn2X2 (X=P, As) monolayers: A first-principles study. Appl. Phys. Lett. 2023, 123, 012401. [Google Scholar] [CrossRef]
- Tung, J.C.; Guo, G.Y. Systematic ab initio study of the magnetic and electronic properties of all 3d transition metal linear and zigzag nanowires. Phys. Rev. B 2007, 76, 094413. [Google Scholar] [CrossRef]
- Ke, L.; Harmon, B.N.; Kramer, M.J. Electronic structure and magnetic properties in T2AlB2 (T=Fe, Mn, Cr, Co and Ni) and their alloys. Phys. Rev. B 2017, 95, 104427. [Google Scholar] [CrossRef]
- Ke, L.; Schilfgaarde, V.M.; Antropov, V. Spin excitations in K2Fe4+xSe5: Linear response approach. Phys. Rev. B 2012, 86, 020402. [Google Scholar] [CrossRef]
- Jiang, Z.; Wang, P.; Xing, J.P.; Jiang, X.; Zhao, J.J. Screening and design of novel 2D ferromagnetic materials with high Curie tem-perature above room temperature. ACS Appl. Mater. Interfaces 2018, 10, 39032. [Google Scholar] [CrossRef]
- Kittel, C.; Kroemer, H. Thermal Physics; W.H. Freeman: New York, NY, USA, 1980; p. 357. [Google Scholar]
- Conley, H.J.; Wang, B.; Ziegler, J.I.; Haglund, R.F.; Pantelides, S.T.; Bolotin, K.I. Bandgap engineering of strained monolayer and bilayer MoS2. Nano Lett. 2013, 13, 3626. [Google Scholar] [CrossRef] [PubMed]
- He, K.; Poole, C.; Mak, K.F.; Shan, J. Experimental demonstration of continuous electronic structure tuning via strain in atomically thin MoS2. Nano Lett. 2013, 13, 2931. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Cong, Y.C.; Yang, W.; Shang, J.; Peimyoo, N.; Chen, Y.; Kang, J.; Huang, W.; Yu, T. Strain-induced direct-indirect bandgap transition and phonon modulation in monolayer WS2. Nano Res. 2015, 8, 2562. [Google Scholar] [CrossRef]
- Hui, Y.Y.; Liu, X.; Jie, W.; Chan, N.Y.; Hao, J.; Hsu, Y.T.; Li, L.J.; Guo, W.; Lau, S.P. Exceptional tunability of band energy in a compressively strained trilayer MoS2 sheet. ACS Nano 2013, 7, 7126. [Google Scholar] [CrossRef]
- Plechinger, G.; Gomez, A.G.; Buscema, M.; Van der Zant, H.S.J.; Steele, G.A.; Kuc, A.; Heine, T.; Schüller, C.; Korn, T. Control of biaxial strain in single-layer molybdenite using local thermal expansion of the substrate. 2D Mater. 2015, 2, 015006. [Google Scholar] [CrossRef]
- Gomez, A.C.; Roldán, R.; Cappelluti, E.; Buscema, M.; Guinea, F.; VanderZant, H.S.J.; Steele, G.A. Local strain engineering in atomically thin MoS2. Nano Lett. 2013, 13, 5361. [Google Scholar] [CrossRef]
- Roldán, R.; Gomez, A.C.; Cappelluti, E.; Guinea, F. Strain engineering in semiconducting two-dimensional crystals. J. Phys. Condens. Matter 2015, 27, 313201. [Google Scholar] [CrossRef]
- Eick, H.A.; Baenziger, N.C.; Eyring, L. The preparation, crystal structure and some properties of SmN, EuN and YbN. J. Am. Chem. Soc. 1956, 78, 5987. [Google Scholar] [CrossRef]
- Inumare, K.; Koyama, K.; Imo-oka, N.; Yamanaka, S. Controlling the structural transition at the Néel point of CrN epitaxial thin films using epitaxial growth. Phys. Rev. B 2007, 75, 054416. [Google Scholar] [CrossRef]
- Kresse, G.; Furthmüller, J. Efficient iterative schemes for ab initio total-energy calculations using a plane-wave basis set. Phys. Rev. B 1996, 54, 11169. [Google Scholar] [CrossRef]
- Blöchl, P.E. Projector augmented-wave method. Phys. Rev. B 1994, 50, 17953. [Google Scholar] [CrossRef] [PubMed]
- Kresse, G.; Joubert, D. From ultrasoft pseudopotentials to the projector augmented-wave method. Phys. Rev. B 1999, 59, 1758. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865. [Google Scholar] [CrossRef]
- Heyd, J.; Scuseria, G.E.; Ernzerhof, M. Hybrid functionals based on a screened Coulomb potentials. J. Chem. Phys. 2003, 118, 8207, Erratum in J. Chem. Phys. 2006, 124, 219906. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188. [Google Scholar] [CrossRef]
- Parlinski, K.; Li, Z.; Kawazoe, Y. First-principles determination of the soft mode in cubic ZrO2. Phys. Rev. Lett. 1997, 78, 4063. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, W.; Hu, Y.; Song, Y.; Huang, Y.; Cao, S. F-p Hybridization-Induced Ferromagnetism for Ultrathin Two-Dimensional Ferromagnetic Half-Metal (EuN) Monolayer: A First-Principles Study. Molecules 2025, 30, 2100. https://doi.org/10.3390/molecules30102100
Sun W, Hu Y, Song Y, Huang Y, Cao S. F-p Hybridization-Induced Ferromagnetism for Ultrathin Two-Dimensional Ferromagnetic Half-Metal (EuN) Monolayer: A First-Principles Study. Molecules. 2025; 30(10):2100. https://doi.org/10.3390/molecules30102100
Chicago/Turabian StyleSun, Wenxue, Yan Hu, Yuling Song, Yuhong Huang, and Shuyao Cao. 2025. "F-p Hybridization-Induced Ferromagnetism for Ultrathin Two-Dimensional Ferromagnetic Half-Metal (EuN) Monolayer: A First-Principles Study" Molecules 30, no. 10: 2100. https://doi.org/10.3390/molecules30102100
APA StyleSun, W., Hu, Y., Song, Y., Huang, Y., & Cao, S. (2025). F-p Hybridization-Induced Ferromagnetism for Ultrathin Two-Dimensional Ferromagnetic Half-Metal (EuN) Monolayer: A First-Principles Study. Molecules, 30(10), 2100. https://doi.org/10.3390/molecules30102100




