Influence of Organic Impurities on Fractional Crystallization of NaCl and Na2SO4 from High-Salinity Coal Chemical Wastewater: Thermodynamics and Nucleation Kinetics Analysis
Abstract
1. Introduction
2. Results and Discussion
2.1. Effect of Dimethoxymethane on Thermodynamics Properties of NaCl and Na2SO4 Crystallization
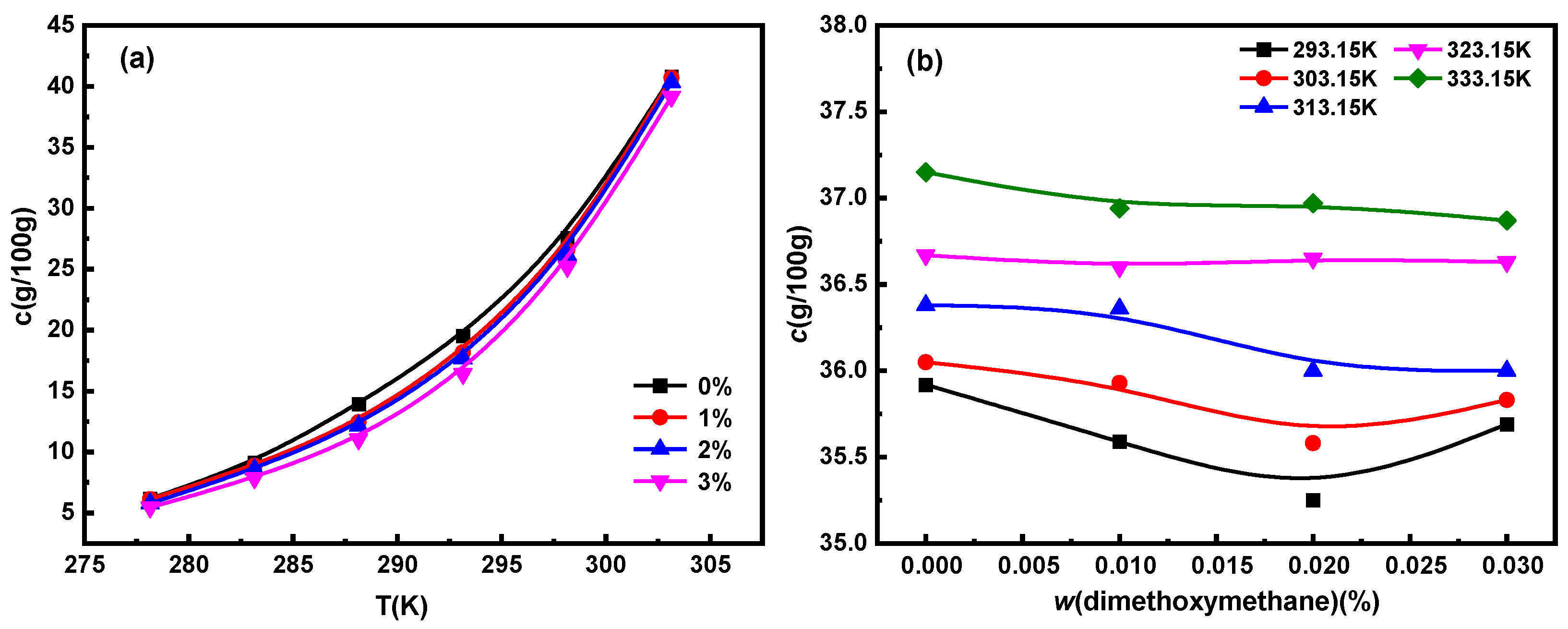
2.1.1. Solubility of Na2SO4 and NaCl
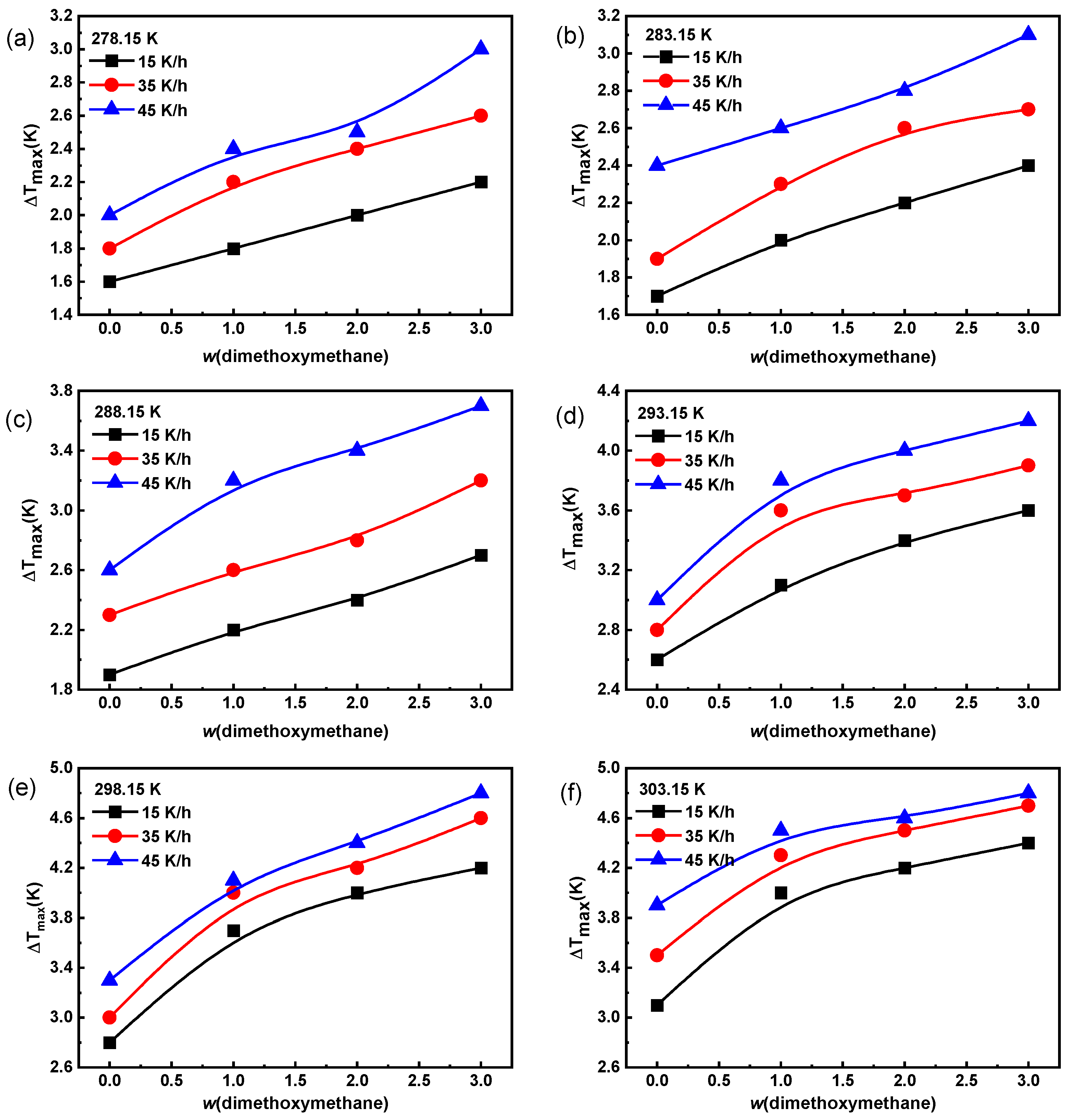
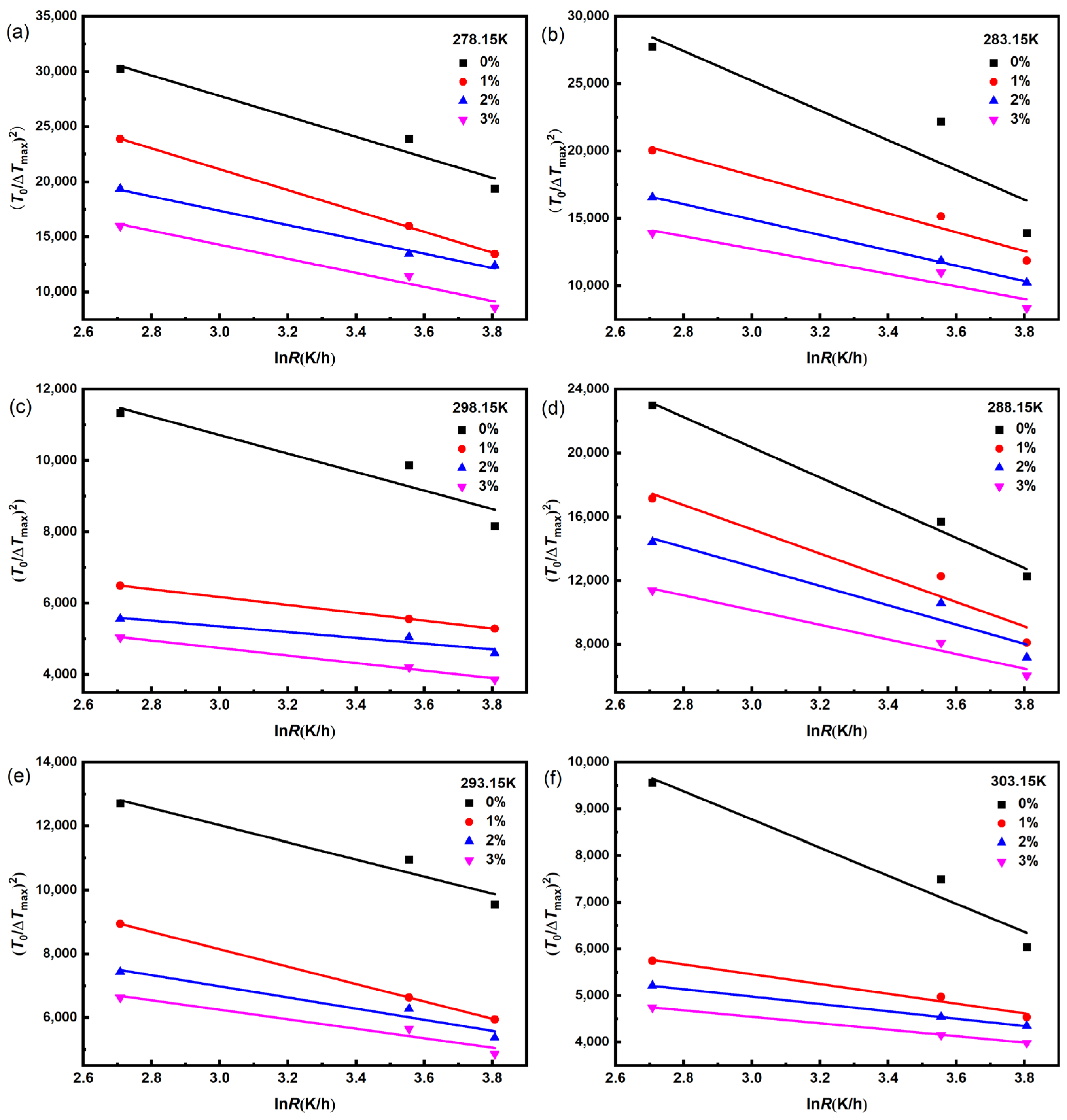
2.1.2. The Metastable Zone Width of Na2SO4 Solution
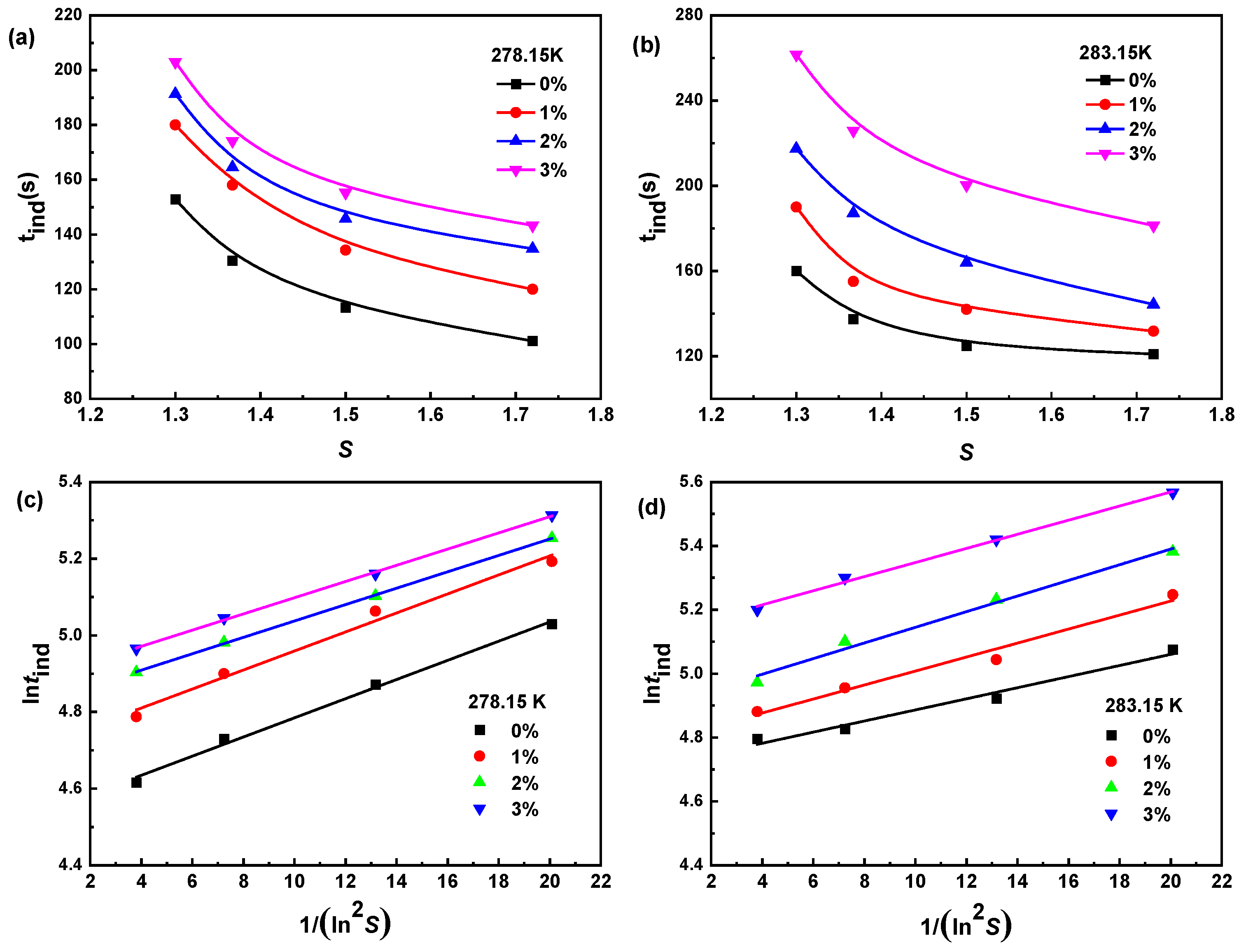
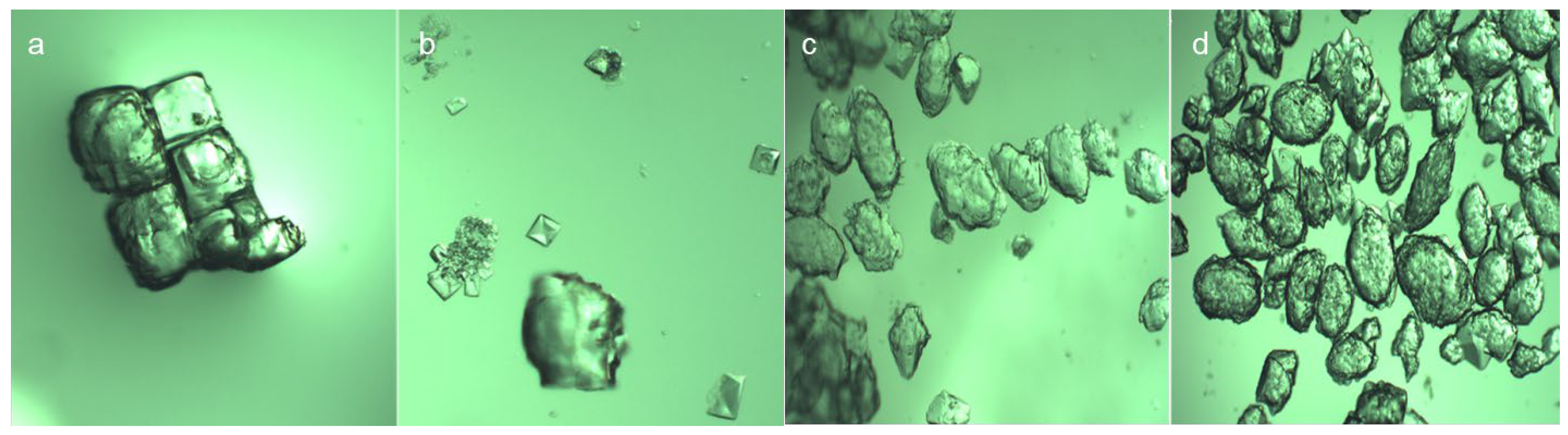
2.1.3. The Induction Period of Na2SO4 Solution
2.2. Phase Equilibrium of Pseudo-Ternary Na2SO4-NaCl-H2O System in Simulated High-Salinity Wastewater
2.3. Crystallization Kinetics of Na2SO4 in Simulated High-Salinity Wastewater
3. Materials and Methods
3.1. Reagents
3.2. Experimental and Analysis Methods
3.3. Theoretical Models
3.3.1. Thermodynamic Models for Solubility [40]
- The Apelblat model
- 2.
- The Van ‘t Hoff model
- 3.
- The polynomial model
3.3.2. Models for Metastable Zone Width
3.3.3. Models for Induction Period
3.3.4. Nucleation Kinetics Models [45]
4. Conclusions
- (1)
- The effects of temperature and organic impurities on the solubility of Na2SO4 and NaCl were investigated. The solubility of Na2SO4 and NaCl increased with a rising temperature. Under certain temperature conditions, the solubility of Na2SO4 decreased with an increasing dimethoxymethane content in the solution.
- (2)
- The metastable zone width and induction period of Na2SO4 increased with an increasing dimethoxymethane content, saturation temperature, and cooling rate. The theoretical reasons for this could be that the increase in the saturation temperature and dimethoxymethane content increased the solid–liquid interface energy and the critical crystal nucleation size, which was unfavorable for the nucleation of Na2SO4.
- (3)
- The phase diagrams of the NaCl-Na2SO4-H2O pseudo-ternary system in the simulated wastewater were plotted in the temperature range of 303.15 to 333.15 K. Under the simulated wastewater conditions, the crystallization zone of Na2SO4 was larger than that of NaCl, and the density of the system was positively correlated with the amount of Na2SO4. The nucleation rate of Na2SO4 was greater than the growth rate due to the salting out effect.
- (4)
- The crystallization kinetics equations of Na2SO4 in the simulated wastewater solution were obtained through kinetic experiments. The crystallization nucleation of Na2SO4 was a secondary nucleation process, controlled by surface reactions. A higher solution temperature and suspension density would be favorable for the crystallization and nucleation of Na2SO4.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Shi, J.; Huang, W.; Han, H.; Xu, C. Pollution control of wastewater from the coal chemical industry in China: Environmental management policy and technical standards. Renew. Sustain. Energy Rev. 2021, 143, 110883. [Google Scholar] [CrossRef]
- Chen, B.; Yang, S.; Cao, Q.; Qian, Y. Life cycle economic assessment of coal chemical wastewater treatment facing the ‘Zero liquid discharge’ industrial water policies in China: Discharge or reuse? Energy Policy 2020, 137, 111107. [Google Scholar] [CrossRef]
- Benaissa, M.; Rouane-Hacene, O.; Boutiba, Z.; Guibbolini-Sabatier, M.E.; Faverney, C.R.D. Ecotoxicological impact assessment of the brine discharges from a desalination plant in the marine waters of the Algerian west coast, using a multibiomarker approach in a limpet, Patella rustica. Environ. Sci. Pollut. Res. 2017, 24, 24521–24532. [Google Scholar] [CrossRef] [PubMed]
- Panagopoulos, A. Brine management (saline water & wastewater effluents): Sustainable utilization and resource recovery strategy through Minimal and Zero Liquid Discharge (MLD & ZLD) desalination systems. Chem. Eng. Process. 2022, 176, 108944. [Google Scholar]
- Panagopoulos, A.; Giannika, V. Decarbonized and circular brine management/valorization for water & valuable resource recovery via minimal/zero liquid discharge (MLD/ZLD) strategies. J. Environ. Manag. 2022, 324, 116239. [Google Scholar]
- Sahu, P. A comprehensive review of saline effluent disposal and treatment: Conventional practices, emerging technologies, and future potential. Water Reuse 2020, 11, 33–65. [Google Scholar] [CrossRef]
- Srivastava, A.; Parida, V.K.; Majumder, A.; Gupta, B.; Gupta, A.K. Treatment of saline wastewater using physicochemical, biological, and hybrid processes: Insights into inhibition mechanisms, treatment efficiencies and performance enhancement. J. Environ. Chem. Eng. 2021, 9, 105775. [Google Scholar] [CrossRef]
- Guo, L.; Xie, Y.; Sun, W.; Xu, Y.; Sun, Y. Research Progress of High-Salinity Wastewater Treatment Technology. Water 2023, 15, 684. [Google Scholar] [CrossRef]
- Dahmardeh, H.; Akhlaghi Amiri, H.A.; Nowee, S.M. Evaluation of mechanical vapor recompression crystallization process for treatment of high salinity wastewater. Chem. Eng. Process. 2019, 145, 107682. [Google Scholar] [CrossRef]
- Lu, H.; Wang, J.; Wang, T.; Wang, N.; Bao, Y.; Hao, H. Crystallization techniques in wastewater treatment: An overview of applications. Chemosphere 2017, 173, 474–484. [Google Scholar] [CrossRef]
- Guo, Y.; Yin, H.; Wu, X.; Deng, T. Metastable Phase Equilibrium in the Aqueous Quaternary System (NaCl + MgCl2 + Na2SO4 + MgSO4 + H2O) at 323.15 K. J. Chem. Eng. Data 2010, 55, 4216–4220. [Google Scholar] [CrossRef]
- Chen, H.; Bian, C.; Bian, J.; Song, X.; Yu, J. Stable Solid—Liquid Equilibrium of the Quaternary System Na+//Cl−, NO3−, and SO42−—H2O at 333.15 K. J. Chem. Eng. Data 2019, 64, 3569–3575. [Google Scholar] [CrossRef]
- Lu, H.; Wang, J.; Yu, J.; Wu, Y.; Wang, T.; Bao, Y.; Ma, D.; Hao, H. Phase equilibria for the pseudo-ternary system (NaCl+ Na2SO4+H2O) of coal gasification wastewater at T= (268.15 to 373.15) K. Chin. J. Chem. Eng. 2017, 25, 955–962. [Google Scholar] [CrossRef]
- Ge, S.-Y.; Ma, Y.-L.; Zhu, L.; Zheng, X.-X.; Ren, Y.-S.; Ma, R.; Gao, J. Study of the Solubility, Supersolubility, and Metastable Zone Width of Ternary System (NaCl + Na2SO4 + H2O) Containing Organic Impurity at 333.15 K. J. Chem. Eng. Data 2019, 64, 5113–5121. [Google Scholar] [CrossRef]
- Bian, C.; Chen, H.; Song, X.; Yu, J. Process Parameters Optimal Investigation on the Na2SO4 Fractional Crystallization from Coal Chemical Industry High-saline Wastewater. Chem. Eng. Trans. 2019, 74, 1117–1122. [Google Scholar]
- Zhang, X.; Ren, Y.; Li, P.; Ma, H.; Ma, W.; Liu, C.; Wang, Y.N.; Kong, L.; Shen, W. Solid–Liquid Equilibrium for the Ternary Systems (Na2SO4 + NaH2PO4 + H2O) and (Na2SO4 + NaCl + H2O) at 313.15 K and Atmospheric Pressure. J. Chem. Eng. Data 2014, 59, 3969–3974. [Google Scholar] [CrossRef]
- Zeng, G.; Li, H.; Huang, S.; Wang, X.; Chen, J. Determination of metastable zone width and the primary nucleation kinetics of sodium sulfate. Theor. Found. Chem. Eng. 2015, 49, 869–876. [Google Scholar] [CrossRef]
- Su, N.; Wang, Y.; Xiao, Y.; Lu, H.; Lou, Y.; Huang, J.; He, M.; Li, Y.; Hao, H. Mechanism of Influence of Organic Impurity on Crystallization of Sodium Sulfate. Ind. Eng. Chem. Res. 2018, 57, 1705–1713. [Google Scholar] [CrossRef]
- Sangwal, K. Effect of impurities on the metastable zone width of solute-solvent systems. J. Cryst. Growth 2009, 311, 4050–4061. [Google Scholar] [CrossRef]
- Hu, R.; Fan, Z.; Liu, Z.; Huang, T.; Wen, G. Experimental study on the effects of different factors on the crystallization rate and products of Mn–Ca co-crystallization. Environ. Sci. Pollut. Res. 2023, 30, 13521–13531. [Google Scholar] [CrossRef]
- Fielitz, T.R.; Phenicie, C.M.; Holmes, R.J. Effects of Additives on Crystallization in Thin Organic Films. Cryst. Growth Des. 2017, 17, 4522–4526. [Google Scholar] [CrossRef]
- Zhu, L.; Ma, Y.; Ge, S.; Ren, Y.; Zhang, S.; Zheng, X.; Wu, D.; Che, C. Determination of NaCl and Na2SO4 Equilibrium Solubility in Cyclohexanol and Cyclohexanone Aqueous Solutions at 323.15 K. J. Chem. Eng. Data 2020, 65, 1617–1626. [Google Scholar] [CrossRef]
- Rajesh, N.P.; Meera, K.; Srinivasan, K.; Raghavan, P.S.; Ramasamy, P. Effect of EDTA on the metastable zone width of ADP. J. Cryst. Growth 2000, 213, 389–394. [Google Scholar] [CrossRef]
- Sasaki, S.; Doki, N.; Kubota, N. The Effect of an Additive on Morphology of Sodium Chloride Crystals in Seeded Batch Cooling Crystallization. In Proceedings of the 12th International Conference on Chemical and Process Engineering (ICheaP), Milano, Italy, 19–22 May 2015; pp. 799–804. [Google Scholar]
- Nanev, C.N. Thermodynamic and molecular-kinetic considerations of the initial growth of newly born crystals; crystal size distribution; Dissolution of small crystals during Ostwald ripening due to temperature changes. Prog. Cryst. Growth Charact. Mater. 2023, 69, 100604. [Google Scholar] [CrossRef]
- Zhang, L.; Ji, X.; Lu, W.; Chen, N. Chemical Bond-Parametric Analysis of Formation Regularities of Salt Hydrates. J. Shanghai Univ. (Nat. Sci.) 2004, 10, 381–385. [Google Scholar]
- Menger, F.M.; Chlebowski, M.E. Is the ether group hydrophilic or hydrophobic? Langmuir 2005, 21, 2689–2695. [Google Scholar] [CrossRef] [PubMed]
- Kadam, S.S.; Kulkarni, S.A.; Ribera, R.C.; Stankiewicz, A.I.; Ter Horst, J.H.; Kramer, H.J.M. A new view on the metastable zone width during cooling crystallization. Chem. Eng. Sci. 2012, 72, 10–19. [Google Scholar] [CrossRef]
- Sangwal, K.; Wójcik, K. Investigation of metastable zone width of ammonium oxalate aqueous solutions. Cryst. Res. Technol. 2009, 44, 363–372. [Google Scholar] [CrossRef]
- Sangwal, K.; Mielniczek-Brzóska, E.; Borc, J. On the induction period for crystallization in solute-solvent systems by polythermal method. Cryst. Res. Technol. 2013, 48, 956–968. [Google Scholar] [CrossRef]
- Flannigan, J.M.; MacIver, D.; Jolliffe, H.; Haw, M.D.; Sefcik, J. Nucleation and Growth Kinetics of Sodium Chloride Crystallization from Water and Deuterium Oxide. Crystals 2023, 13, 1388. [Google Scholar] [CrossRef]
- Custelcean, R.; Sloop, F.V., Jr.; Rajbanshi, A.; Wan, S.; Moyer, B.A. Sodium Sulfate Separation from Aqueous Alkaline Solutions via Crystalline Urea-Functionalized Capsules: Thermodynamics and Kinetics of Crystallization. Cryst. Growth Des. 2015, 15, 517–522. [Google Scholar] [CrossRef]
- Frawley, P.J.; Mitchell, N.A.; O‘Ciardhá, C.T.; Hutton, K.W. The effects of supersaturation, temperature, agitation and seed surface area on the secondary nucleation of paracetamol in ethanol solutions. Chem. Eng. Sci. 2012, 75, 183–197. [Google Scholar] [CrossRef]
- Li, K.; Du, S.; Wu, S.; Cai, D.; Wang, J.; Zhang, D.; Zhao, K.; Yang, P.; Yu, B.; Guo, B.; et al. Determination and correlation of solubility and solution thermodynamics of oxiracetam in three (alcohol + water) binary solvents. J. Chem. Thermodyn. 2016, 96, 12–23. [Google Scholar] [CrossRef]
- GB/T 13025.8-2012; General Test Method in Salt Industry-Determination of Sulfate. General Administration of Quality Supervision, Inspection and Quarantine of the People’s Republic of China, China Standards Press: Beijing, China, 2012.
- GB/T 13025.5-2012; General Test Method in Salt Industry-Determination of Chloride Ion. General Administration of Quality Supervision, Inspection and Quarantine of the People’s Republic of China, China Standards Press: Beijing, China, 2012.
- Kuldipkumar, A.; Kwon, G.S.; Zhang, G.G.Z. Determining the growth mechanism of tolazamide by induction time measurement. Cryst. Growth Des. 2007, 7, 234–242. [Google Scholar] [CrossRef]
- Zhu, L.; Ma, Y.L.; Ge, S.Y.; Wu, D.; Che, C.; Liu, E.; Zhao, K.H. Study of the stable and metastable phase equilibrium of a ternary system (NaCl + Na2SO4+H2O) in coal-to-liquids wastewater at 323.15 K. Desalin. Water Treat. 2020, 197, 121–130. [Google Scholar] [CrossRef]
- Skrivanek, J.; Pekarek, V.; Hostomsky, J. Crystallization studies in thermometric methods. II. Calorimetric determination of crystallization kinetics of sodium sulfate and sodium carbonate decahydrates. Krist. Tech. 1975, 10, 617–620. [Google Scholar] [CrossRef]
- Mirheydari, S.N.; Barzegar-Jalali, M.; Acree, W.E.; Shekaari, H.; Shayanfar, A.; Jouyban, A. Comparison of the Models for Correlation of Drug Solubility in Ethanol plus Water Binary Mixtures. J. Solut. Chem. 2019, 48, 1079–1104. [Google Scholar] [CrossRef]
- Nývlt, J. Kinetics of nucleation in solutions. J. Cryst. Growth 1968, 3, 377–383. [Google Scholar] [CrossRef]
- Maharana, A.; Sarkar, D. Effects of Ultrasound and Its Amplitude on the Metastable Zone Width, Induction Time, and Nucleation Kinetics of Pyrazinamide in Acetone. Ind. Eng. Chem. Res. 2022, 61, 11262–11275. [Google Scholar] [CrossRef]
- Sangwal, K. Recent developments in understanding of the metastable zone width of different solute-solvent systems. J. Cryst. Growth 2011, 318, 103–109. [Google Scholar] [CrossRef]
- Morton, C.W.; Hofmeister, W.H.; Bayuzick, R.J.; Rulison, A.J.; Watkins, J.L. The kinetics of solid nucleation in zirconium. Acta Mater. 1998, 46, 6033–6039. [Google Scholar] [CrossRef]
- Xue, J.; Liu, C.; Luo, M.; Lin, M.; Jiang, Y.; Li, P.; Yu, J.; Rohani, S. Secondary nucleation and growth kinetics of aluminum hydroxide crystallization from potassium aluminate solution. J. Cryst. Growth 2019, 507, 232–240. [Google Scholar] [CrossRef]

| w (Dimethoxymethane) (%) | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| T/K | S | 0 | 1 | 2 | 3 | ||||
| γ | r* | γ | r* | γ | r* | γ | r* | ||
| 278.15 | 1.67 | 5.17 | 2.97 | 5.19 | 3.16 | 5.21 | 3.25 | 5.23 | 3.32 |
| 1.45 | 5.19 | 3.34 | 5.21 | 3.52 | 5.24 | 3.60 | 5.26 | 3.68 | |
| 1.32 | 5.20 | 3.62 | 5.23 | 3.81 | 5.25 | 3.89 | 5.28 | 3.95 | |
| 1.25 | 5.21 | 4.03 | 5.23 | 4.21 | 5.26 | 4.28 | 5.29 | 4.36 | |
| 283.15 | 1.67 | 7.21 | 3.36 | 7.23 | 3.54 | 7.25 | 3.62 | 7.27 | 3.71 |
| 1.45 | 7.23 | 3.72 | 7.24 | 3.91 | 7.26 | 4.01 | 7.29 | 4.11 | |
| 1.32 | 7.24 | 4.01 | 7.25 | 4.20 | 7.27 | 4.28 | 7.30 | 4.35 | |
| 1.25 | 7.26 | 4.45 | 7.27 | 4.63 | 7.28 | 4.71 | 7.31 | 4.81 | |
| Impurities | Concentrations/mg·L−1 |
|---|---|
| sodium nitrate | 100~300 |
| magnesium sulfate | 200~500 |
| calcium chloride | 200~500 |
| diethyl ether | 350~450 |
| dimethoxymethane | 1050~1150 |
| 1,1 dichloroethane | 100~200 |
| dibromomethane | 150 |
| Test Number | Temperature (°C) | Supersaturation (mol·L−1) | Suspension Density (kg·m−3) | Crystallization Period (s) | Nucleation Rate (n·m−3·s−1) | Growth Rate (m·s−1) |
|---|---|---|---|---|---|---|
| 1 | 10 | 0.39 | 20.18 | 300 | 8.11 × 10−8 | 3.45 × 10−7 |
| 2 | 10 | 0.36 | 23.20 | 600 | 3.28 × 10−8 | 1.50 × 10−7 |
| 3 | 10 | 0.33 | 25.68 | 900 | 6.14 × 10−8 | 1.76 × 10−7 |
| 4 | 10 | 0.28 | 27.98 | 1200 | 1.08 × 10−7 | 2.47 × 10−7 |
| 5 | 10 | 0.26 | 30.56 | 1500 | 8.90 × 10−8 | 4.77 × 10−7 |
| 6 | 15 | 0.37 | 24.10 | 300 | 1.59 × 10−8 | 7.07 × 10−8 |
| 7 | 15 | 0.29 | 27.58 | 600 | 6.33 × 10−8 | 2.08 × 10−7 |
| 8 | 15 | 0.19 | 30.70 | 900 | 5.11 × 10−8 | 2.48 × 10−7 |
| 9 | 15 | 0.17 | 33.56 | 1200 | 1.35 × 10−7 | 5.14 × 10−7 |
| 10 | 15 | 0.09 | 36.90 | 1500 | 1.12 × 10−7 | 5.17 × 10−7 |
| 11 | 20 | 0.49 | 68.40 | 300 | 1.09 × 10−6 | 2.35 × 10−7 |
| 12 | 20 | 0.26 | 90.62 | 600 | 7.28 × 10−7 | 2.35 × 10−7 |
| 13 | 20 | 0.15 | 99.56 | 900 | 1.37 × 10−7 | 5.86 × 10−7 |
| 14 | 20 | 0.11 | 111.84 | 1200 | 1.94 × 10−7 | 9.22 × 10−7 |
| 15 | 20 | 0.08 | 116.78 | 1500 | 1.33 × 10−7 | 5.88 × 10−7 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shen, B.; Zhao, B.; Du, H.; Ren, Y.; Tang, J.; Liu, Y.; Hua, Q.; Wang, B. Influence of Organic Impurities on Fractional Crystallization of NaCl and Na2SO4 from High-Salinity Coal Chemical Wastewater: Thermodynamics and Nucleation Kinetics Analysis. Molecules 2024, 29, 1928. https://doi.org/10.3390/molecules29091928
Shen B, Zhao B, Du H, Ren Y, Tang J, Liu Y, Hua Q, Wang B. Influence of Organic Impurities on Fractional Crystallization of NaCl and Na2SO4 from High-Salinity Coal Chemical Wastewater: Thermodynamics and Nucleation Kinetics Analysis. Molecules. 2024; 29(9):1928. https://doi.org/10.3390/molecules29091928
Chicago/Turabian StyleShen, Bo, Bo Zhao, Hai Du, Yongsheng Ren, Jianwei Tang, Yong Liu, Quanxian Hua, and Baoming Wang. 2024. "Influence of Organic Impurities on Fractional Crystallization of NaCl and Na2SO4 from High-Salinity Coal Chemical Wastewater: Thermodynamics and Nucleation Kinetics Analysis" Molecules 29, no. 9: 1928. https://doi.org/10.3390/molecules29091928
APA StyleShen, B., Zhao, B., Du, H., Ren, Y., Tang, J., Liu, Y., Hua, Q., & Wang, B. (2024). Influence of Organic Impurities on Fractional Crystallization of NaCl and Na2SO4 from High-Salinity Coal Chemical Wastewater: Thermodynamics and Nucleation Kinetics Analysis. Molecules, 29(9), 1928. https://doi.org/10.3390/molecules29091928







