Modeling Studies of the Mechanism of Context-Dependent Bidirectional Movements of Kinesin-14 Motors
Abstract
1. Introduction
2. Results
2.1. Dynamics of the KlpA Motor
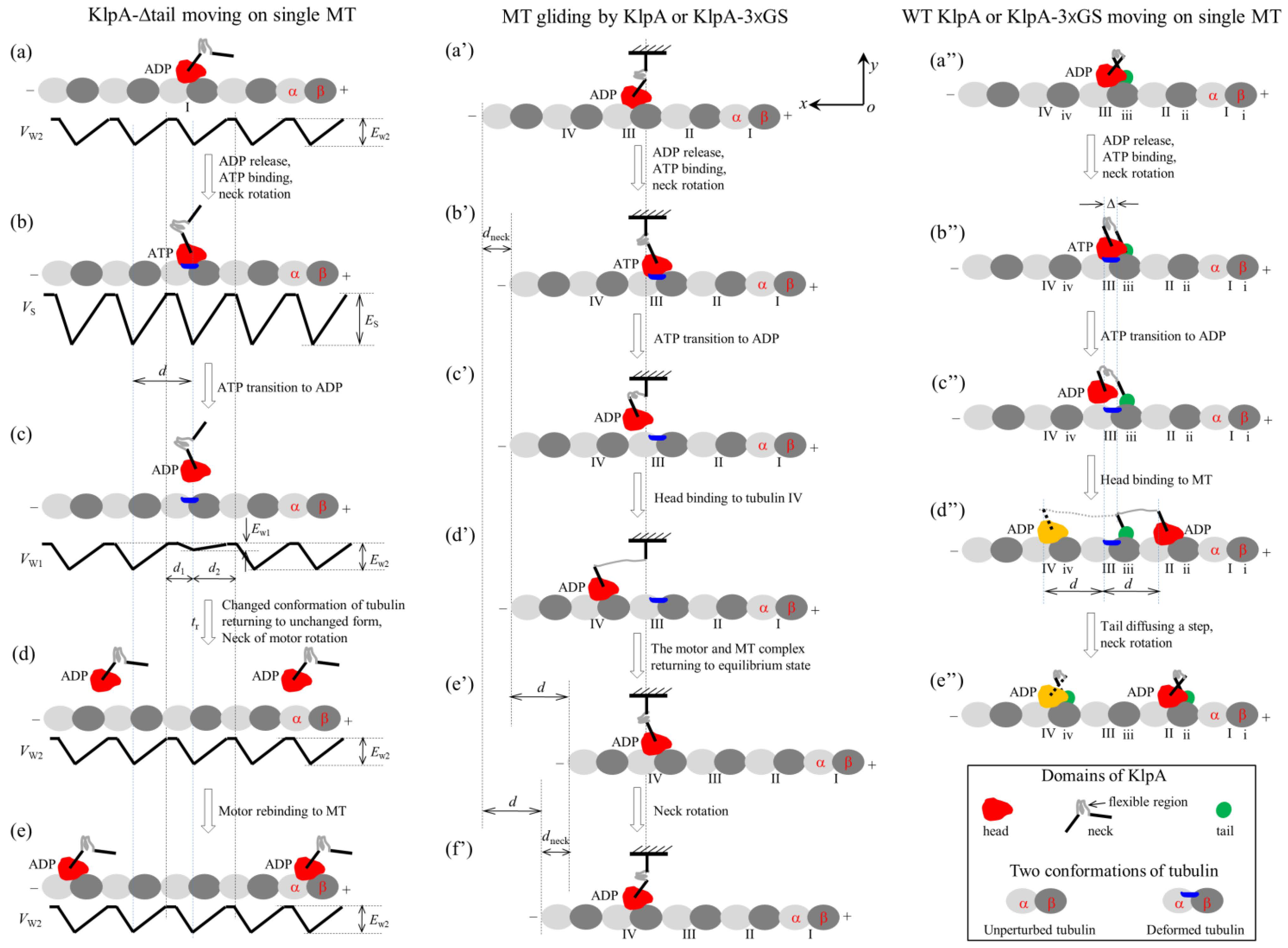
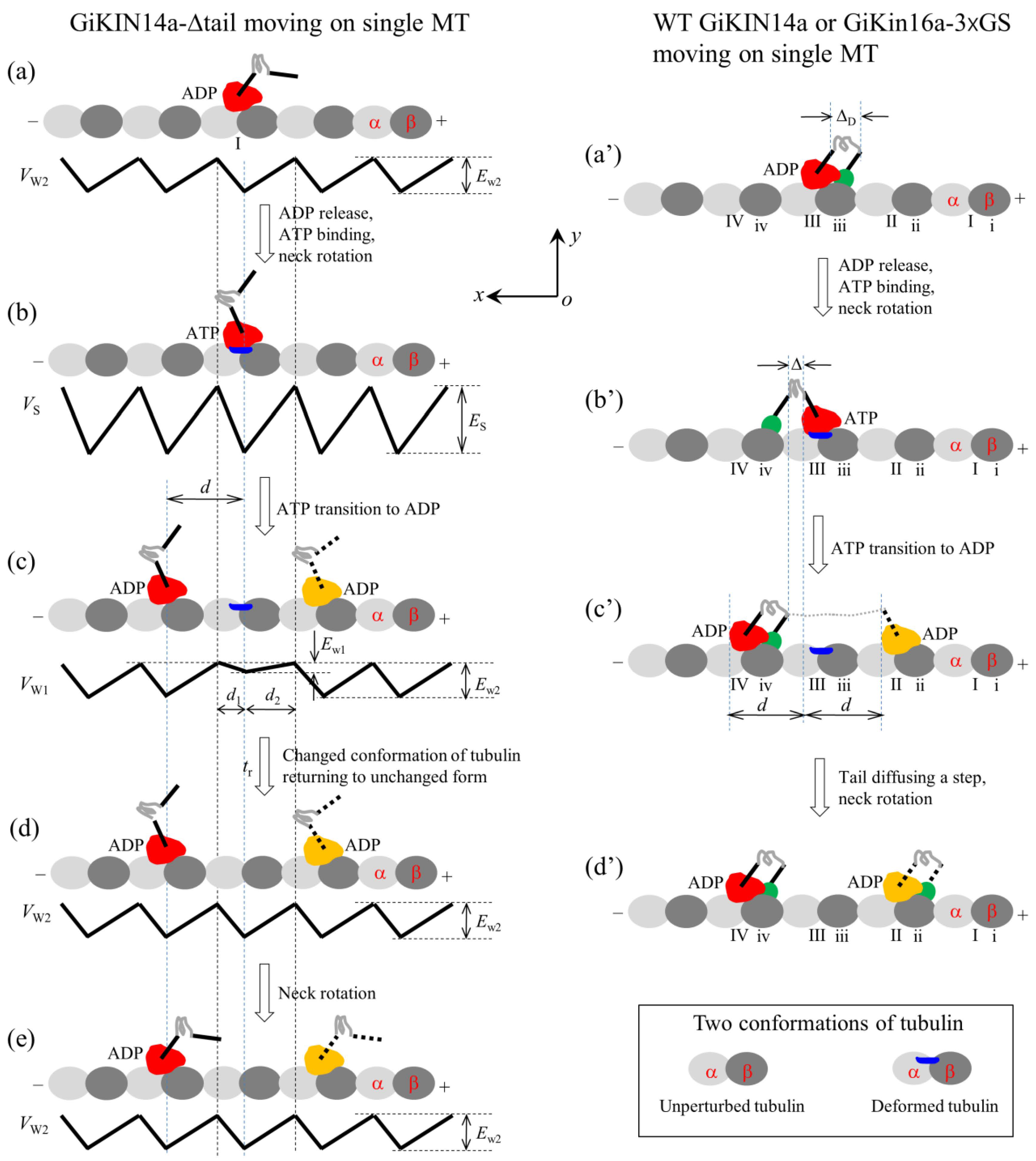
2.1.1. The Single KlpA-ΔTail Motor Moving on a Single MT
2.1.2. MT Gliding by KlpA or the KlpA-3 GS Motor
2.1.3. The Single Full-Length KlpA or KlpA-3 GS Motor Moving on a Single MT
2.1.4. KlpA or KlpA-3 GS Motor Moving Inside Parallel MT Overlap
2.2. Dynamics of the Single GiKIN14a Motor Moving on the Single MT
2.2.1. The Chemo–Mechanical Coupling Efficiency
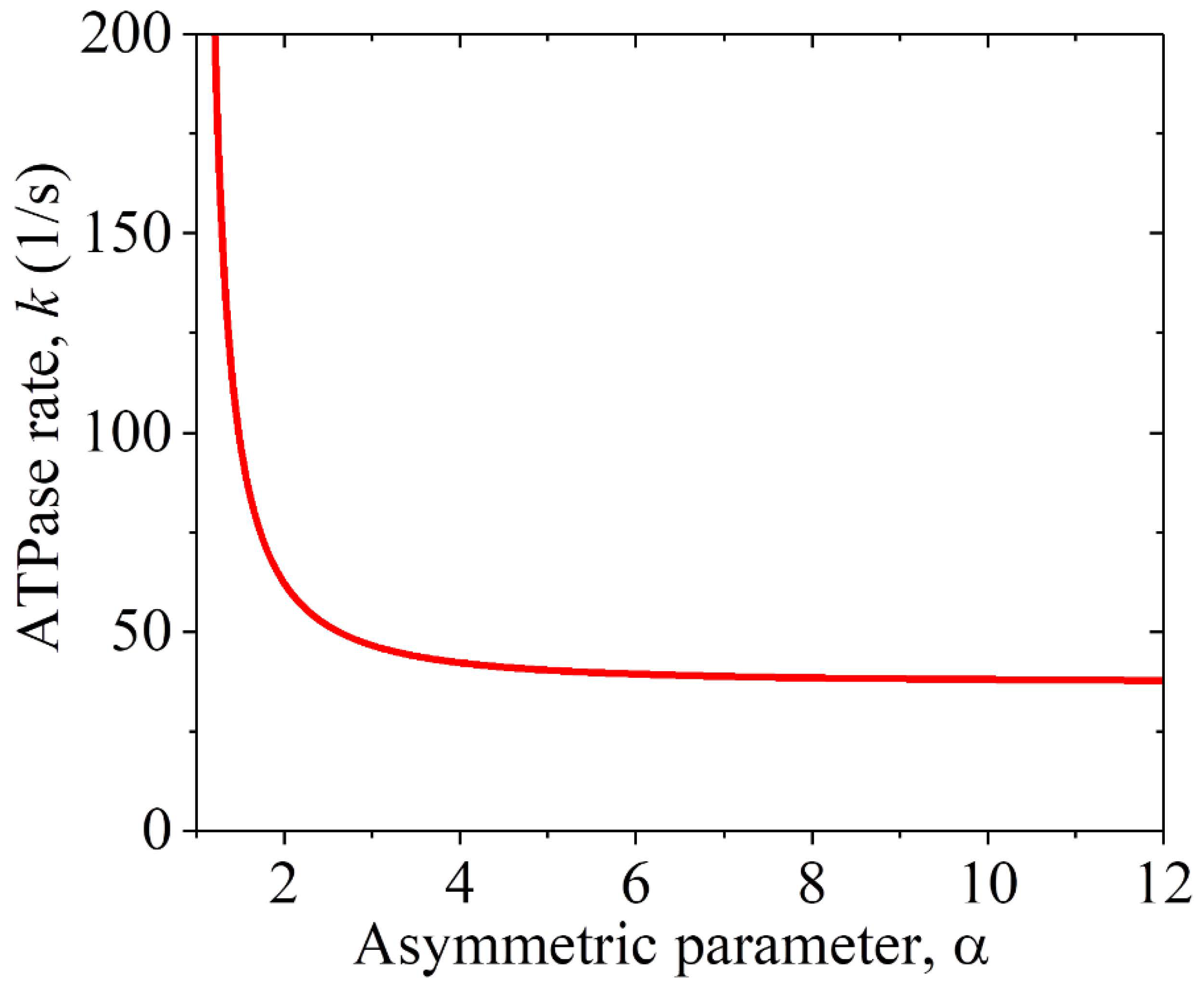
2.2.2. The ATPase Rate
2.2.3. The Velocity
3. Discussion
3.1. Origin of Full-Length Ncd Being Incapable of Diffusing with a Directional Preference Inside Parallel MT Overlaps Contrary to Ncd-3 GS Being Capable of Diffusing with a Directional Preference toward the Minus Ends
3.2. Difference between the Origin of the Bidirectional Movement of Kinesin-14 and That of Kinesin-5
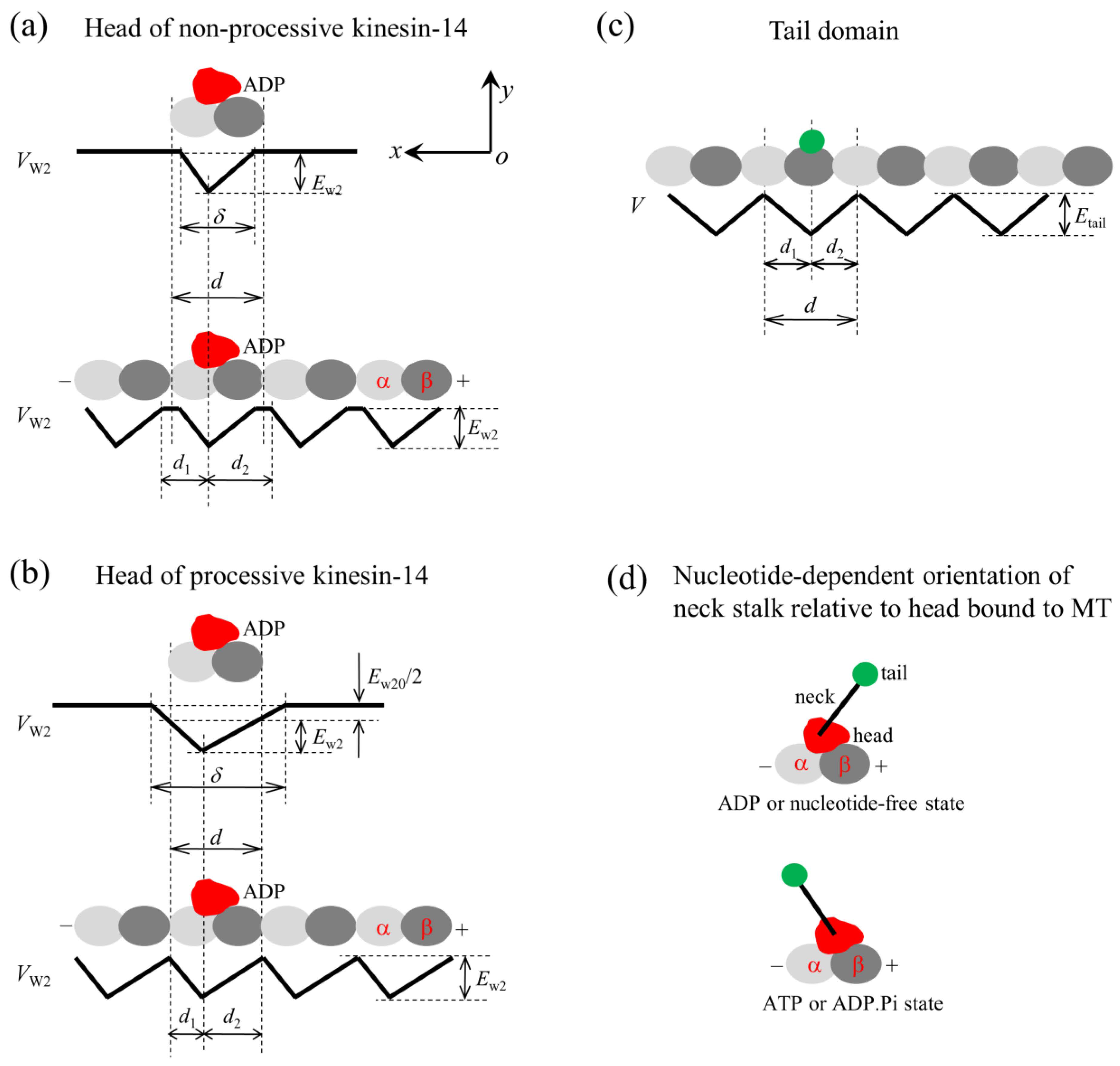
4. The Model
4.1. Interaction Potentials of the Motor with MTs
4.2. Orientations of the Neck Stalk Relative to the Head and Tail Domain
5. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lawrence, C.J.; Dawe, R.K.; Christie, K.R.; Cleveland, D.W.; Dawson, S.C.; Endow, S.A.; Goldstein, L.S.B.; Goodson, H.V.; Nobutaka Hirokawa, N.; Howard, J.; et al. A standardized kinesin nomenclature. J. Cell Biol. 2004, 167, 19–22. [Google Scholar] [CrossRef] [PubMed]
- Hirokawa, N.; Noda, Y.; Tanaka, Y.; Niwa, S. Kinesin superfamily motor proteins and intracellular transport. Nat. Rev. Mol. Cell Biol. 2009, 10, 682–696. [Google Scholar] [CrossRef]
- Wordeman, L. How kinesin motor proteins drive mitotic spindle function: Lessons from molecular assays. Semin. Cell Dev. Biol. 2010, 21, 260–268. [Google Scholar] [CrossRef] [PubMed]
- Cross, R.A.; McAinsh, A. Prime movers: The mechanochemistry of mitotic kinesins. Nat. Rev. Mol. Cell Biol. 2014, 15, 257–271. [Google Scholar] [CrossRef] [PubMed]
- She, Z.-Y.; Yang, W.-X. Molecular mechanisms of kinesin-14 motors in spindle assembly and chromosome segregation. J. Cell Sci. 2017, 130, 2097–2110. [Google Scholar] [CrossRef] [PubMed]
- Matthies, H.J.; McDonald, H.B.; Goldstein, L.S.; Theurkauf, W.E. Anastral meiotic spindle morphogenesis: Role of the non-claret disjunctional kinesin-like protein. J. Cell Biol. 1996, 134, 455–464. [Google Scholar] [CrossRef] [PubMed]
- Endow, S.A.; Chandra, R.; Komma, D.J.; Yamamoto, A.H.; Salmon, E.D. Mutants of the Drosophila ncd microtubule motor protein cause centrosomal and spindle pole defects in mitosis. J. Cell Sci. 1994, 107, 859–867. [Google Scholar] [CrossRef] [PubMed]
- Sharp, D.J.; Yu, K.R.; Sisson, J.C.; Sullivan, W.; Scholey, J.M. Antagonistic microtubule-sliding motors position mitotic centrosomes in Drosophila early embryos. Nat. Cell Biol. 1999, 1, 51–54. [Google Scholar] [CrossRef] [PubMed]
- Saunders, W.S.; Hoyt, M.A. Kinesin-related proteins required for structural integrity of the mitotic spindle. Cell 1992, 70, 451–458. [Google Scholar] [CrossRef]
- Oladipo, A.; Cowan, A.; Rodionov, V. Microtubule motor Ncd induces sliding of microtubules in vivo. Mol. Biol. Cell 2007, 18, 3601–3606. [Google Scholar] [CrossRef]
- O’Connell, M.J.; Meluh, P.B.; Rose, M.D.; Morris, N.R. Suppression of the bimC4 mitotic spindle defect by deletion of klpA, a gene encoding a KAR3-related kinesin-like protein in Aspergillus nidulans. J. Cell Biol. 1993, 120, 153–162. [Google Scholar] [CrossRef] [PubMed]
- Adam, R.D. Biology of Giardia lamblia. Clin. Microbiol. Rev. 2001, 14, 447–475. [Google Scholar] [CrossRef] [PubMed]
- Chandra, R.; Salmon, E.D.; Erickson, H.P.; Lockhart, A.; Endow, S.A. Structural and functional domains of the Drosophila ncd microtubule motor protein. J. Biol. Chem. 1993, 268, 9005–9013. [Google Scholar] [CrossRef] [PubMed]
- Karabay, A.; Walker, R.A. Identification of microtubule binding sites in the Ncd tail domain. Biochemistry 1999, 38, 1838–1849. [Google Scholar] [CrossRef]
- Popchock, A.R.; Tseng, K.-F.; Wang, P.; Karplus, P.A.; Xiang, X.; Qiu, W. The mitotic kinesin-14 KlpA contains a context-dependent directionality switch. Nat. Commun. 2017, 8, 13999. [Google Scholar] [CrossRef] [PubMed]
- Wang, P.; Tseng, K.-F.; Gao, Y.; Cianfrocco, M.; Guo, L.; Qiu, W. The Central Stalk Determines the Motility of Mitotic Kinesin-14 Homodimers. Curr. Biol. 2018, 28, 2302–2308. [Google Scholar] [CrossRef] [PubMed]
- Tseng, K.-F.; Mickolajczyk, K.J.; Feng, G.; Feng, Q.; Kwok, E.S.; Howe, J.; Barbar, E.J.; Dawson, S.C.; Hancock, W.O.; Qiu, W. The Tail of Kinesin-14a in Giardia Is a Dual Regulator of Motility. Curr. Biol. 2020, 30, 3664–3671. [Google Scholar] [CrossRef] [PubMed]
- Reinemann, D.N.; Norris, S.R.; Ohi, R.; Lang, M.J. Processive Kinesin-14 HSET Exhibits Directional Flexibility Depending on Motor Traffic. Curr. Biol. 2018, 28, 2356–2362. [Google Scholar] [CrossRef]
- Shi, X.-X.; Fu, Y.-B.; Guo, S.-K.; Wang, P.-Y.; Chen, H.; Xie, P. Investigating role of conformational changes of microtubule in regulating its binding affinity to kinesin by all-atom molecular dynamics simulation. Proteins 2018, 86, 1127–1139. [Google Scholar] [CrossRef]
- Shi, X.-X.; Wang, P.-Y.; Chen, H.; Xie, P. Studies of conformational changes of tubulin induced by interaction with kinesin using atomistic molecular dynamics simulations. Int. J. Mol. Sci. 2021, 22, 6709. [Google Scholar] [CrossRef]
- Xie, P. Modeling study of the dynamics of kinesin-14 molecular motors. J. Phys. Chem. B 2022, 126, 8720–8734. [Google Scholar] [CrossRef] [PubMed]
- Lansky, Z.; Braun, M.; Ludecke, A.; Schlierf, M.; Ten Wolde, P.R.; Janson, M.E.; Diez, S. Diffusible Crosslinkers Generate Directed Forces in Microtubule Networks. Cell 2015, 160, 1159–1168. [Google Scholar] [CrossRef] [PubMed]
- Wierenga, H.; Ten Wolde, P.R. Diffusible Cross-linkers Cause Superexponential Friction Forces. Phys. Rev. Lett. 2020, 125, 78101. [Google Scholar] [CrossRef] [PubMed]
- Xie, P. Effect of varying load in moving period of a step on dynamics of molecular motors. Eur. Phys. J. E 2022, 45, 28. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Liu, Y.-R.; Wang, P.-Y.; Xie, P. Dynamics of cooperative transport by multiple kinesin motors and diffusing microtubule-associated proteins. Commun. Theory Phys. 2022, 74, 105601. [Google Scholar] [CrossRef]
- Schmiedl, T.; Seifert, U. Efficiency of molecular motors at maximum power. Europhys. Lett. 2008, 83, 30005. [Google Scholar] [CrossRef]
- Wagoner, J.A.; Dill, K.A. Molecular Motors: Power Strokes Outperform Brownian Ratchets. J. Phys. Chem. B 2016, 120, 6327–6336. [Google Scholar] [CrossRef]
- Brown, A.I.; Sivak, D.A. Allocating and Splitting Free Energy to Maximize Molecular Machine Flux. J. Phys. Chem. B 2018, 122, 1387–1393. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, Y.-R.; Wang, P.-Y.; Xie, P. Computational studies reveal how passive cross-linkers regulate anaphase spindle elongation. J. Phys. Chem. B 2024, 128, 1194–1204. [Google Scholar] [CrossRef]
- Endres, N.F.; Yoshioka, C.; Milligan, R.A.; Vale, R.D. A lever-arm rotation drives motility of the minus-end-directed kinesin Ncd. Nature 2006, 439, 875–878. [Google Scholar] [CrossRef]
- Rank, K.C.; Chen, C.J.; Cope, J.; Porche, K.; Hoenger, A.; Gilbert, S.P.; Rayment, I. Kar3Vik1, a member of the kinesin-14 superfamily, shows a novel kinesin microtubule binding pattern. J. Cell Biol. 2012, 197, 957–970. [Google Scholar] [CrossRef] [PubMed]
- deCastro, M.J.; Fondecave, R.M.; Clarke, L.A.; Schmidt, C.F.; Stewart, R.J. Working strokes by single molecules of the kinesin-related microtubule motor ncd. Nat. Cell Biol. 2000, 2, 724–729. [Google Scholar] [CrossRef]
- Shang, Z.; Zhou, K.; Xu, C.; Csencsits, R.; Cochran, J.C.; Sindelar, C.V. High-resolution structures of kinesin on microtubules provide a basis for nucleotide-gated force-generation. eLife 2014, 3, e04686. [Google Scholar] [CrossRef] [PubMed]
- Roostalu, J.; Hentrich, C.; Bieling, P.; Telley, I.A.; Schiebel, E.; Surrey, T. Directional switching of the kinesin cin8 through motor coupling. Science 2011, 332, 94–99. [Google Scholar] [CrossRef] [PubMed]
- Gerson-Gurwitz, A.; Thiede, C.; Movshovich, N.; Fridman, V.; Podolskaya, M.; Danieli, T.; Lakamper, S.; Klopfenstein, D.R.; Schmidt, C.F.; Gheber, L. Directionality of individual kinesin-5 Cin8 motors is modulated by loop 8, ionic strength and microtubule geometry. EMBO J. 2011, 30, 4942–4954. [Google Scholar] [CrossRef] [PubMed]
- Fridman, V.; Gerson-Gurwitz, A.; Shapira, O.; Movshovich, N.; Lakamper, S.; Schmidt, C.F.; Gheber, L. Kinesin-5 Kip1 is a bi-directional motor that stabilizes microtubules and tracks their plus-ends in vivo. J. Cell Sci. 2013, 126, 4147–4159. [Google Scholar] [CrossRef] [PubMed]
- Edamatsu, M. Bidirectional motility of the fission yeast kinesin-5, Cut7. Biochem. Biophys. Res. Commun. 2014, 446, 231–234. [Google Scholar] [CrossRef] [PubMed]
- Singh, S.K.; Pandey, H.; Al-Bassam, J.; Gheber, L. Bidirectional motility of kinesin-5 motor proteins: Structural determinants, cumulative functions and physiological roles. Cell. Mol. Life Sci. 2018, 75, 1757–1771. [Google Scholar] [CrossRef] [PubMed]
- Xie, P. A common ATP-dependent stepping model for kinesin-5 and kinesin-1: Mechanism of bi-directionality of kinesin-5. Biophys. Chem. 2021, 271, 106548. [Google Scholar] [CrossRef]
- Xie, P. Effect of the neck linker on processive stepping of kinesin motor. Biophysica 2023, 3, 46–68. [Google Scholar] [CrossRef]
- Duan, Z.; Xie, P.; Li, W.; Wang, P. Are coiled-coils of dimeric kinesins unwound during their walking on microtubule? PLoS ONE 2012, 7, e36071. [Google Scholar] [CrossRef] [PubMed]
- Liu, S.-X.; Lü, G.; Zhang, H.; Geng, Y.-Z.; Ji, Q. Origin of the Surprising Mechanical Stability of Kinesin’s Neck Coiled Coil. J. Chem. Theory Comput. 2021, 17, 1017–1029. [Google Scholar] [CrossRef] [PubMed]
- Xie, P. Modeling processive motion of kinesin-13 MCAK and kinesin-14 Cik1-Kar3 molecular motors. Protein Sci. 2021, 30, 2092–2105. [Google Scholar] [CrossRef] [PubMed]
- Crevel, I.M.-T.C.; Lockhart, A.; Cross, R.A. Weak and strong states of kinesin and ncd. J. Mol. Biol. 1996, 257, 66–76. [Google Scholar] [CrossRef] [PubMed]
- Sosa, H.; Peterman, E.J.G.; Moerner, W.E.; Goldstein, L.S.B. ADP-induced rocking of the kinesin motor domain revealed by single-molecule fluorescence polarization microscopy. Nat. Struct. Biol. 2001, 8, 540–544. [Google Scholar] [CrossRef] [PubMed]
- Hoenger, A.; Sablin, E.P.; Vale, R.D.; Fletterick, R.J.; Milligan, R.A. Three-dimensional structure of a tubulin-motor-protein complex. Nature 1995, 376, 271–274. [Google Scholar] [CrossRef] [PubMed]
- Hoenger, A.; Milligan, R.A. Motor domains of kinesin and ncd interact with microtubule protofilaments with the same binding geometry. J. Mol. Biol. 1997, 265, 553–564. [Google Scholar] [CrossRef] [PubMed]
- Hirose, K.; Amos, W.B.; Lockhart, A.; Cross, R.A.; Amos, L.A. Three-dimensional cryoelectron microscopy of 16-protofilament microtubules: Structure, polarity, and interaction with motor proteins. J. Struct. Biol. 1997, 118, 140–148. [Google Scholar] [CrossRef]
- Krebs, A.; Goldie, K.N.; Hoenger, A. Complex formation with kinesin motor domains affects the structure of microtubules. J. Mol. Biol. 2004, 335, 139–153. [Google Scholar] [CrossRef]
- Morikawa, M.; Yajima, H.; Nitta, R.; Inoue, S.; Ogura, T.; Sato, C.; Hirokawa, N. X-ray and Cryo-EM structures reveal mutual conformational changes of Kinesin and GTP-state microtubules upon binding. EMBO J. 2015, 34, 1270–1286. [Google Scholar] [CrossRef]
- Verhey, K.J.; Ohi, R. Causes, costs and consequences of kinesin motors communicating through the microtubule lattice. J. Cell Sci. 2023, 136, jcs260735. [Google Scholar] [CrossRef] [PubMed]
- Braun, M.; Lansky, Z.; Szuba, A.; Schwarz, F.W.; Mitra, A.; Gao, M.; Ludecke, A.; Ten Wolde, P.R.; Diez, S. Changes in microtubule overlap length regulate kinesin-14-driven microtubule sliding. Nat. Chem. Biol. 2017, 13, 1245–1252. [Google Scholar] [CrossRef] [PubMed]



| Form of KlpA | Dynamical Feature | Reference |
|---|---|---|
| Tailless KlpA (KlpA-Δtail) | Unbiased diffusion on a single MT | Ref. [15] |
| Full-length KlpA (KlpA) | Minus-end-directed motion in MT gliding | Ref. [15] |
| Full-length KlpA (KlpA) | Minus-end-directed motion inside parallel MTs | Ref. [15] |
| Full-length KlpA (KlpA) | Plus-end-directed motion on a single MT | Ref. [15] |
| KlpA with the insertion of 3 GS (KlpA-3 GS) | Minus-end-directed motion on a single MT | Ref. [16] |
| Parameter | Value | Description |
|---|---|---|
| 4 | Determined theoretically | |
| 2 nm | Adjustable |
| Parameter | Value | Description |
|---|---|---|
| 4 | Determined theoretically | |
| 0.5 nm | Adjustable | |
| 3.5 nm | Determined from | |
| 0.8 kBT | Adjustable |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xie, P. Modeling Studies of the Mechanism of Context-Dependent Bidirectional Movements of Kinesin-14 Motors. Molecules 2024, 29, 1792. https://doi.org/10.3390/molecules29081792
Xie P. Modeling Studies of the Mechanism of Context-Dependent Bidirectional Movements of Kinesin-14 Motors. Molecules. 2024; 29(8):1792. https://doi.org/10.3390/molecules29081792
Chicago/Turabian StyleXie, Ping. 2024. "Modeling Studies of the Mechanism of Context-Dependent Bidirectional Movements of Kinesin-14 Motors" Molecules 29, no. 8: 1792. https://doi.org/10.3390/molecules29081792
APA StyleXie, P. (2024). Modeling Studies of the Mechanism of Context-Dependent Bidirectional Movements of Kinesin-14 Motors. Molecules, 29(8), 1792. https://doi.org/10.3390/molecules29081792






