Study on the Effects of Wettability and Pressure in Shale Matrix Nanopore Imbibition during Shut-in Process by Molecular Dynamics Simulations
Abstract
1. Introduction
2. Results
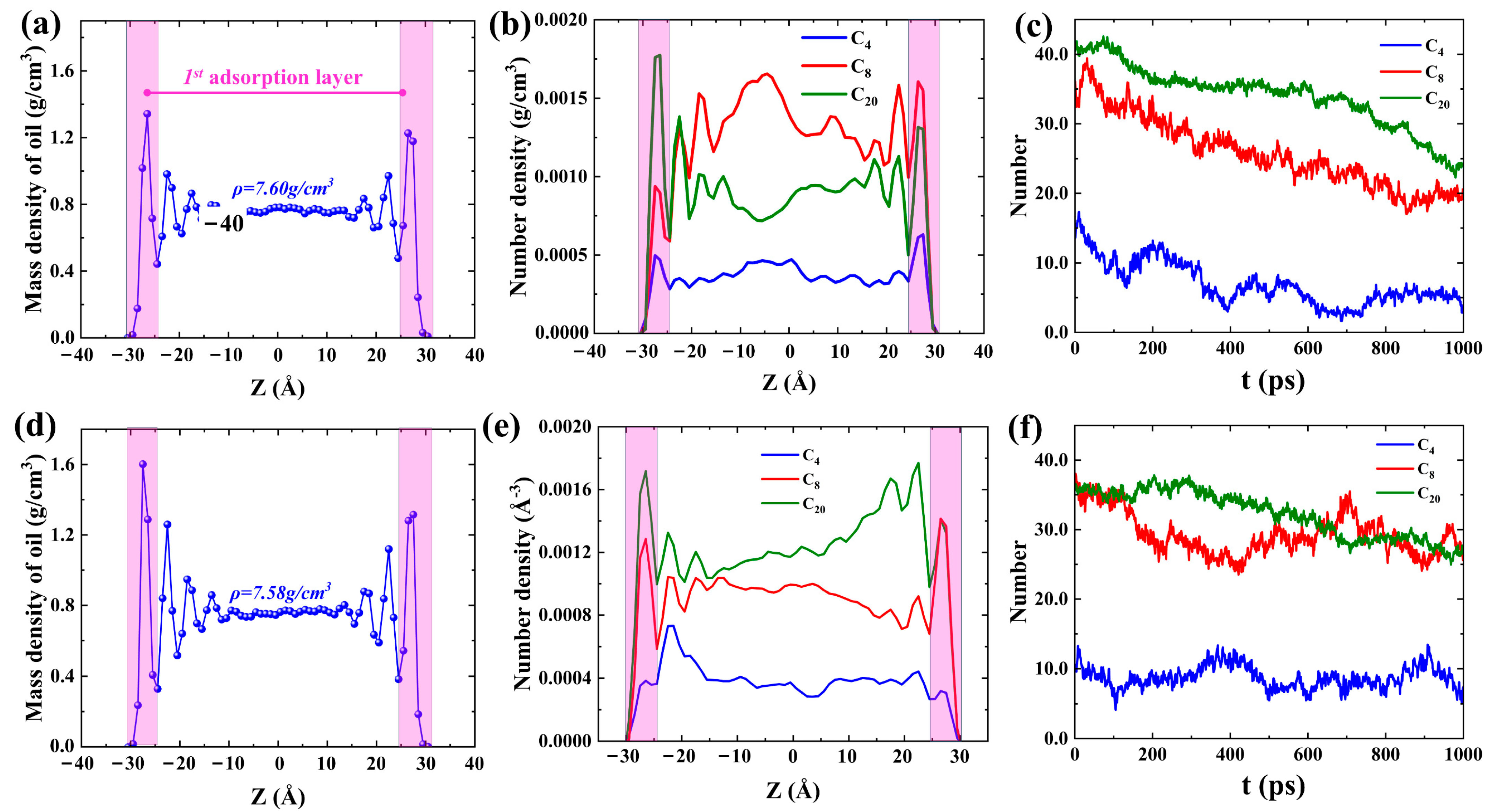
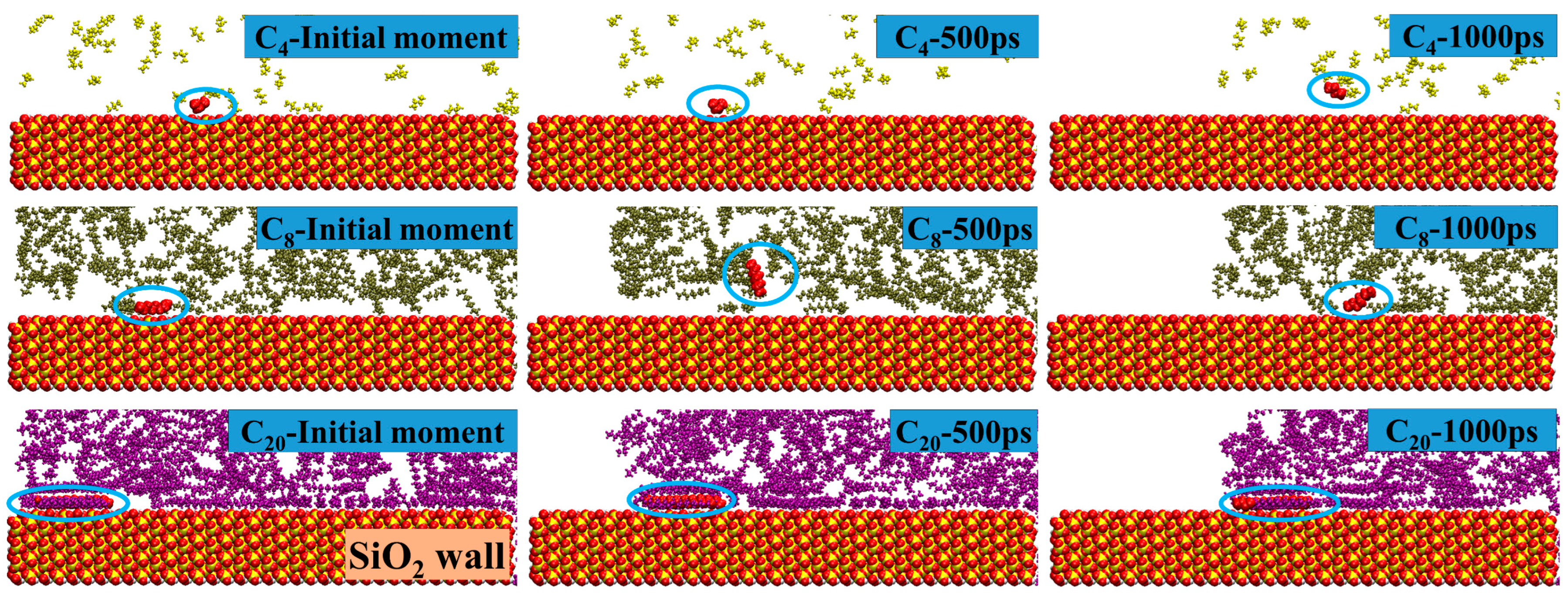
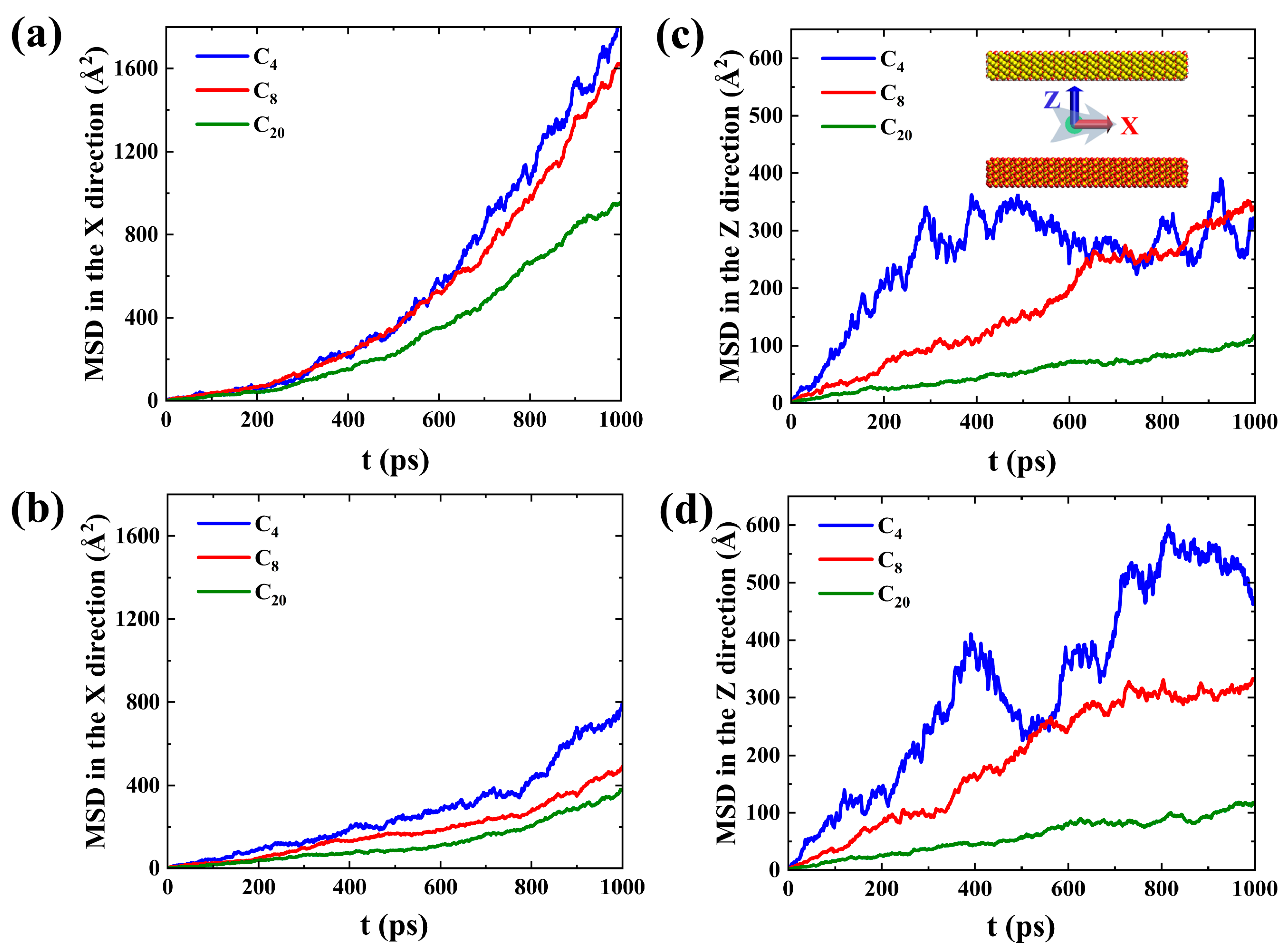
2.1. Shale Oil Migration in Different Hydrophilic Nanopores during Spontaneous Imbibition
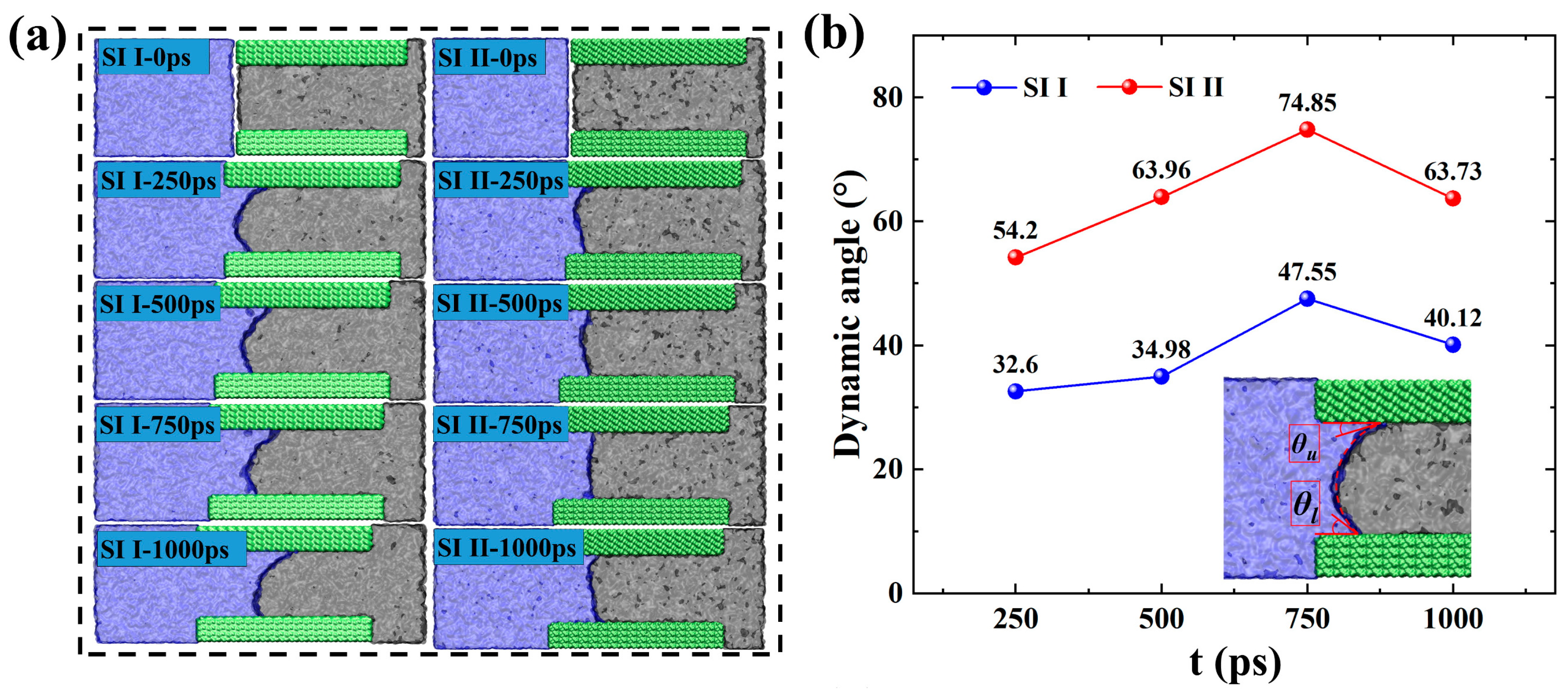
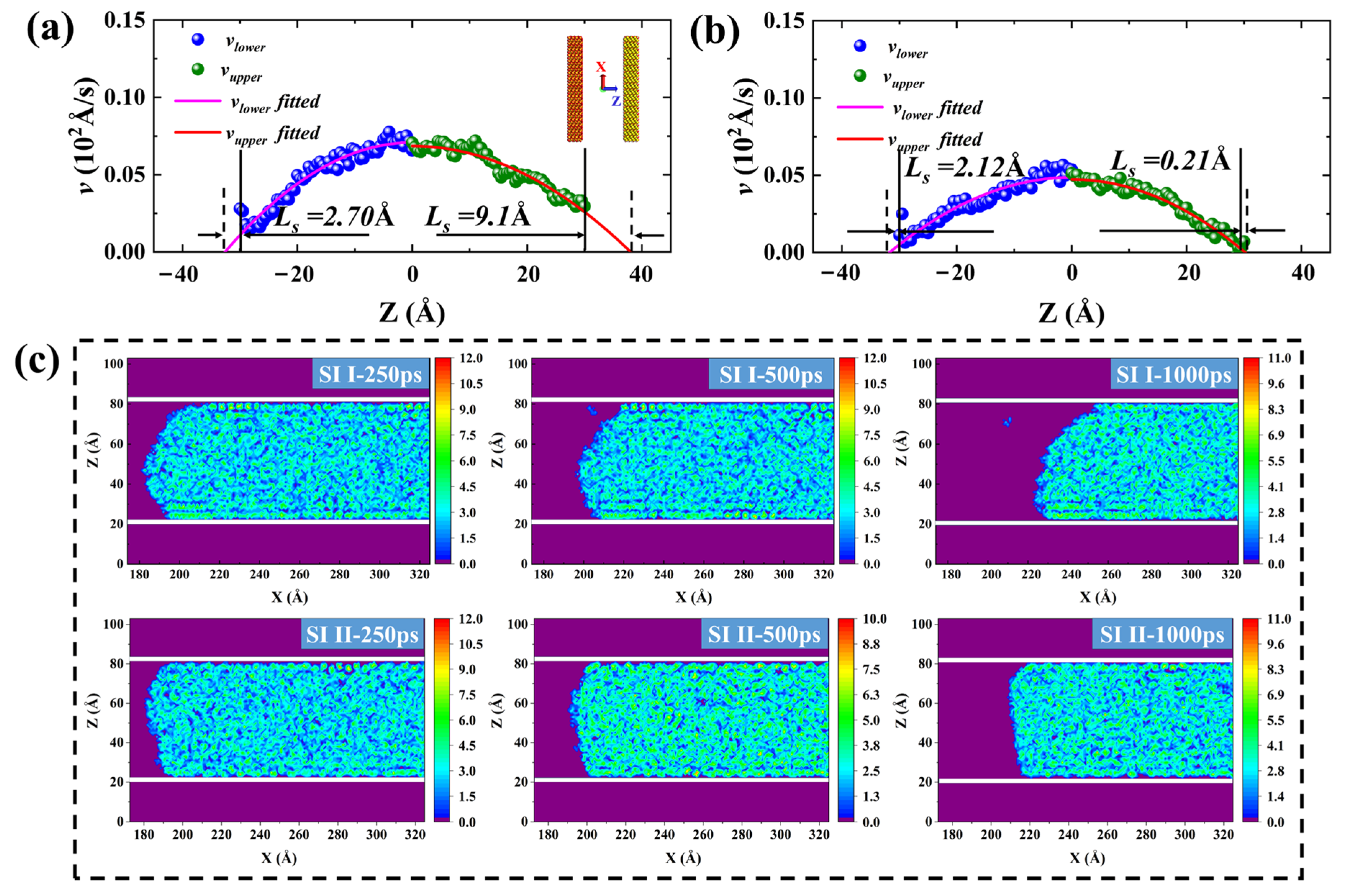
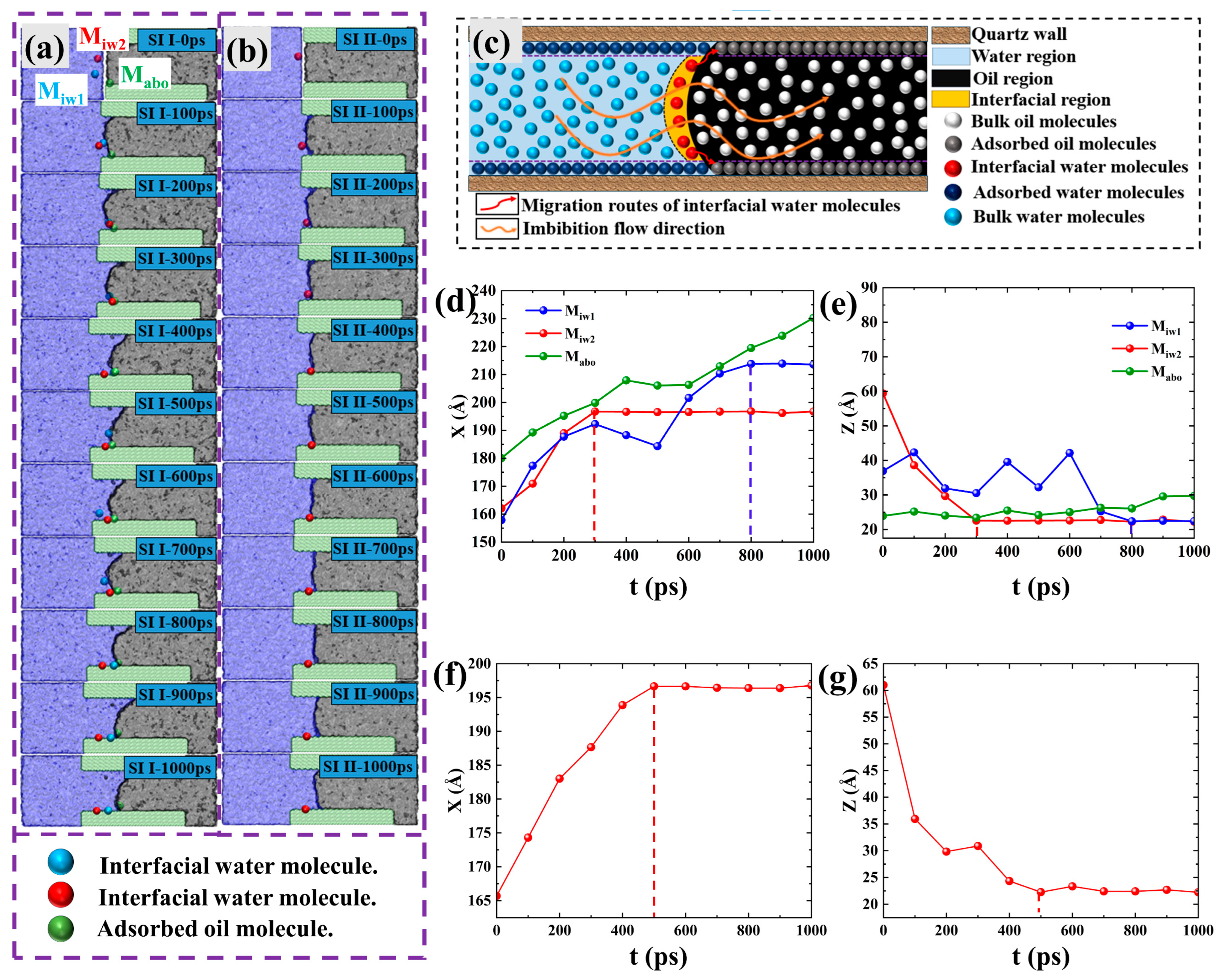
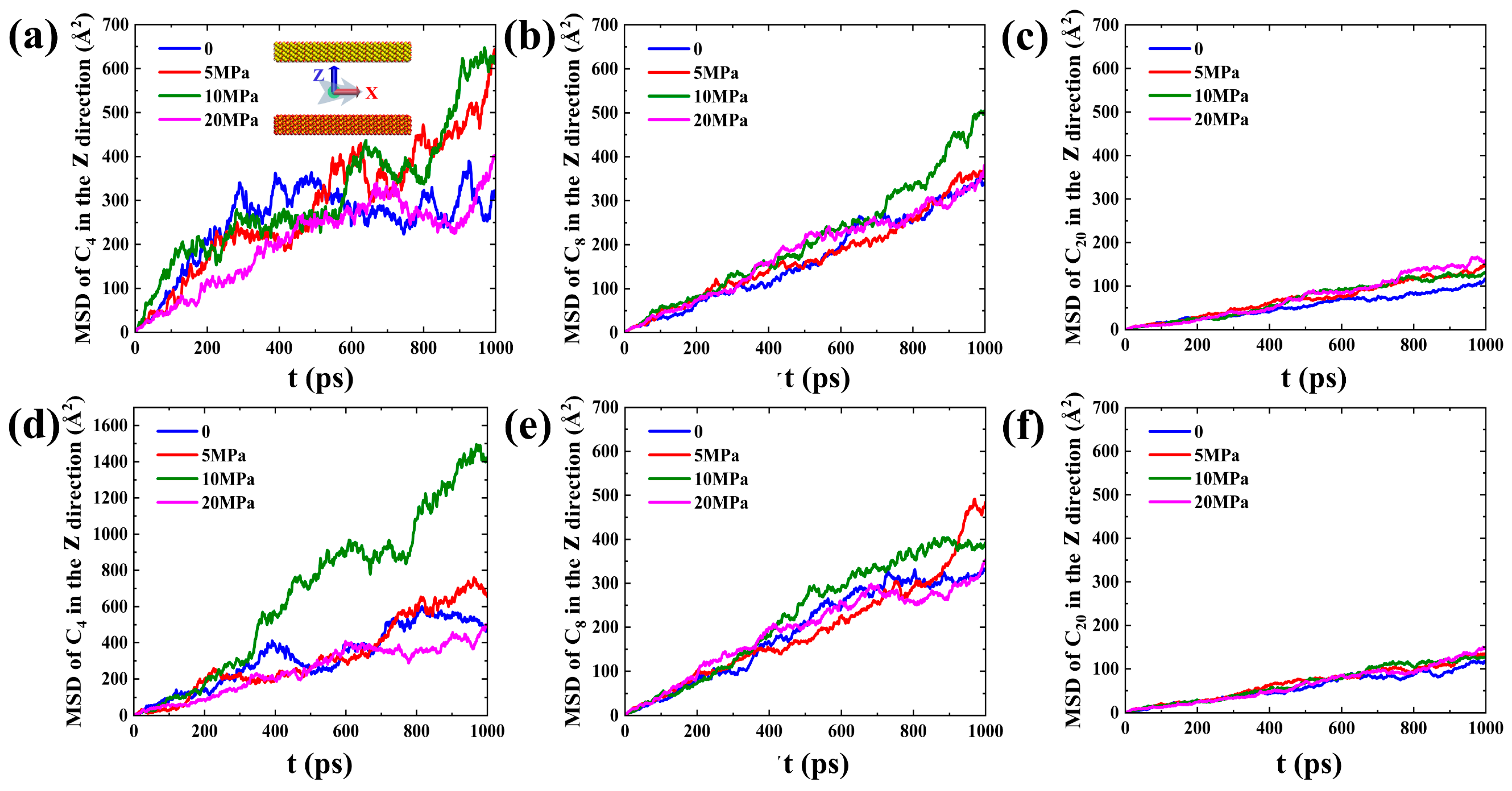
2.2. Microscopic Advancement Mechanism of Imbibition Front
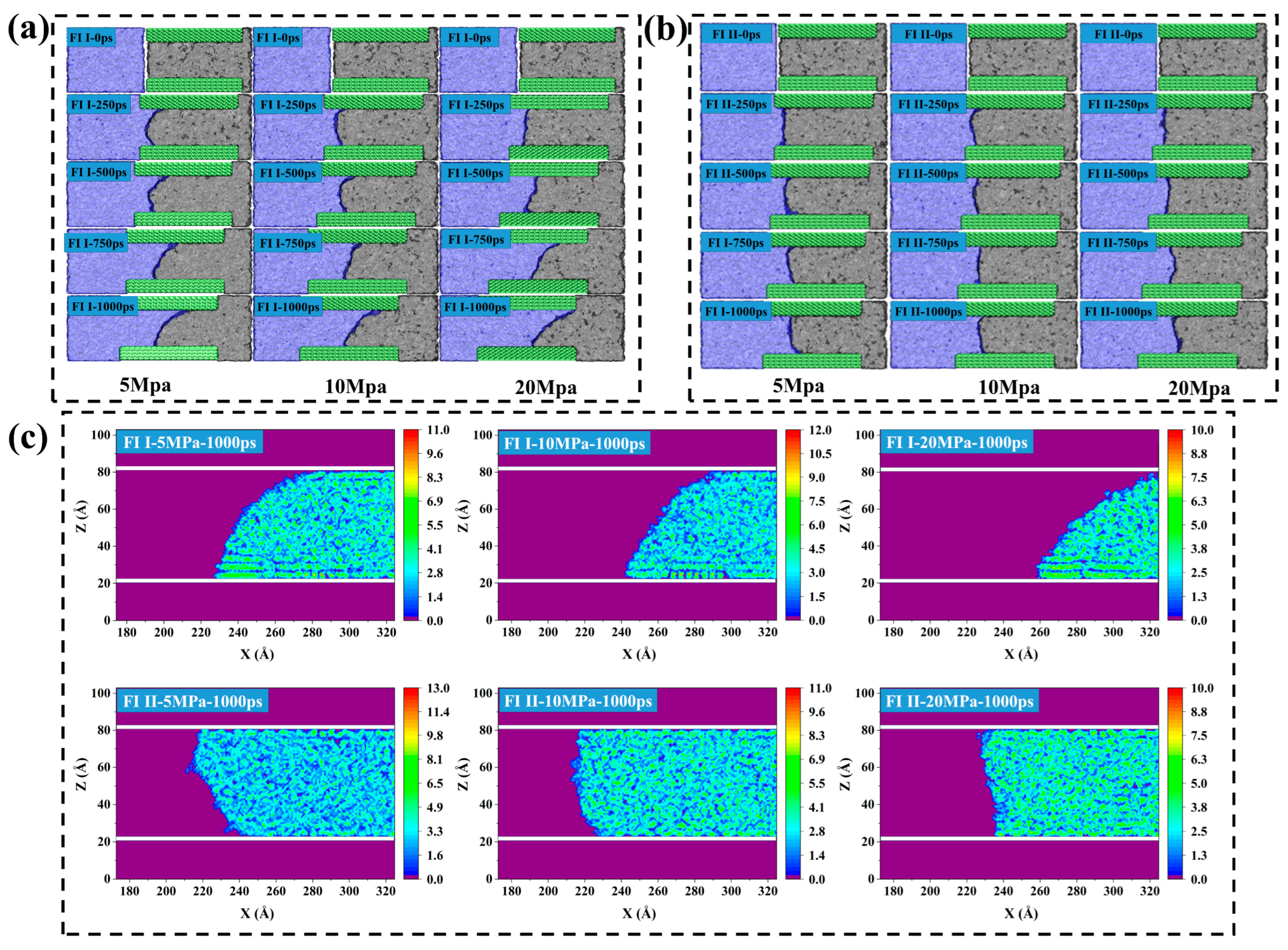
2.3. Forced Imbibition under Pressure Difference
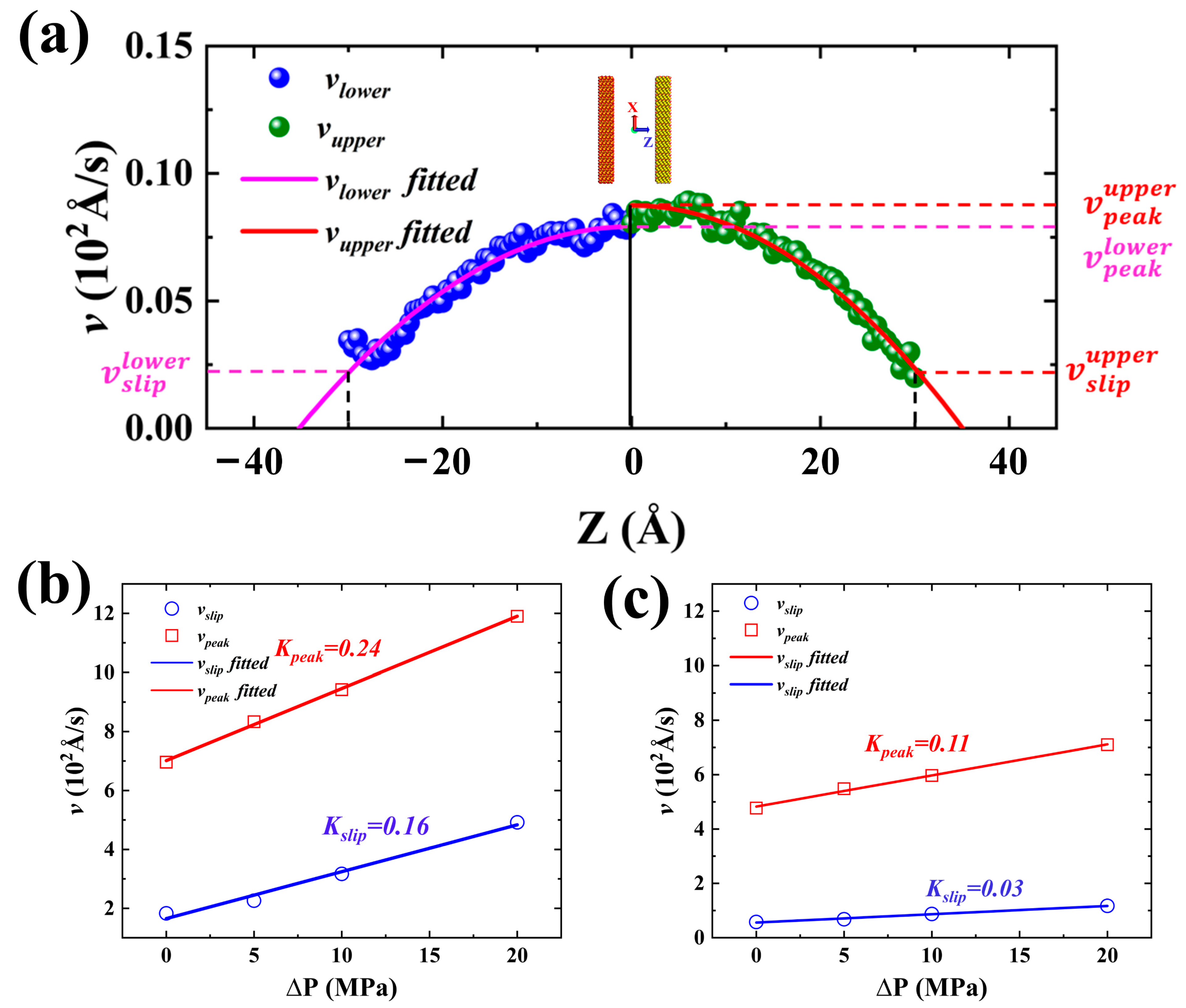
3. Models and Methodology
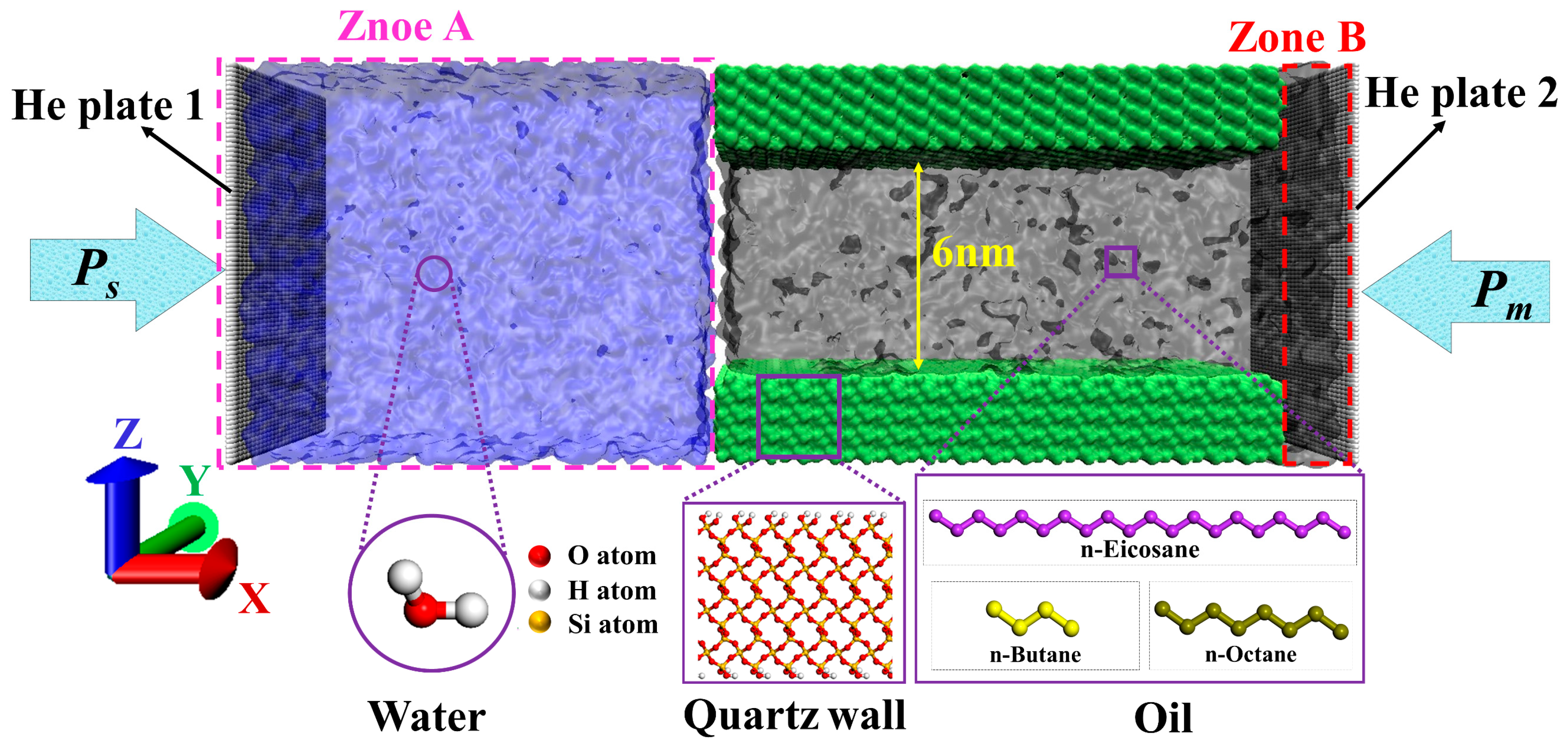
3.1. Model System
3.2. Simulation Details
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Xia, D.; Yang, Z.; Gao, T.; Li, H.; Lin, W. Characteristics of micro-and nano-pores in shale oil reservoirs. J. Pet. Explor. Prod. 2021, 11, 157–169. [Google Scholar] [CrossRef]
- Dai, C.; Wang, K.; Liu, Y.; Fang, J.; Zhao, M. Study on the reutilization of clear fracturing flowback fluids in surfactant flooding with additives for Enhanced Oil Recovery (EOR). PLoS ONE 2014, 9, e113723. [Google Scholar] [CrossRef]
- Shaibu, R.; Guo, B. The dilemma of soaking a hydraulically fractured horizontal shale well prior to flowback—A decade literature review. J. Nat. Gas Sci. Eng. 2021, 94, 104084. [Google Scholar] [CrossRef]
- Hu, Y.; Ren, F.; Li, J.; Wu, Z.; Peng, H.; Hou, J. Effect of dynamic imbibition on the development of ultralow permeability reservoir. Geofluids 2021, 2021, 5544484. [Google Scholar] [CrossRef]
- Sobhani, A.; Ghasemi Dehkordi, M. The effect of nanoparticles on spontaneous imbibition of brine into initially oil-wet sandstones. Energy Sources Part A Recovery Util. Environ. Eff. 2019, 41, 2746–2756. [Google Scholar] [CrossRef]
- Morrow, N.R.; Mason, G. Recovery of oil by spontaneous imbibition. Curr. Opin. Colloid Interface Sci. 2001, 6, 321–337. [Google Scholar] [CrossRef]
- Nguyen, D.; Wang, D.; Oladapo, A.; Zhang, J.; Sickorez, J.; Butler, R.; Mueller, B. Evaluation of Surfactants for Oil Recovery Potential in Shale Reservoirs; SPE Improved Oil Recovery Symposium: Tulsa, OK, USA, 2014. [Google Scholar]
- Hatiboglu, C.U.; Babadagli, T. Experimental and visual analysis of co-and counter-current spontaneous imbibition for different viscosity ratios, interfacial tensions, and wettabilities. J. Pet. Sci. Eng. 2010, 70, 214–228. [Google Scholar] [CrossRef]
- Sukee, A.; Nunta, T.; Haruna, M.A.; Kalantariasl, A.; Tangparitkul, S. Influence of sequential changes in the crude oil-water interfacial tension on spontaneous imbibition in oil-wet sandstone. J. Pet. Sci. Eng. 2022, 210, 110032. [Google Scholar] [CrossRef]
- Darvishi, H.; Goudarznia, I.; Esmaeilzadeh, F. Effects of rock permeability on capillary imbibition oil recovery from carbonate cores. Trans. C Chem. Chem. Eng. 2010, 17, 185–190. [Google Scholar]
- Strand, S.; Puntervold, T.; Austad, T. Effect of temperature on enhanced oil recovery from mixed-wet chalk cores by spontaneous imbibition and forced displacement using seawater. Energy Fuels 2008, 22, 3222–3225. [Google Scholar] [CrossRef]
- Zhao, H.; Kang, W.; Yang, H.; Zhang, H.; Zhu, T.; Wang, F.; Li, X.; Zhou, B.; Sarsenbekuly, B.; Aidarova, S. Imbibition enhancing oil recovery mechanism of the two surfactants. Phys. Fluids 2020, 32, 047103. [Google Scholar] [CrossRef]
- Wang, F.; Wang, L.; Jiao, L.; Liu, Z.; Yang, K. Experimental Mechanism for Enhancing Oil Recovery by Spontaneous Imbibition with Surfactants in a Tight Sandstone Oil Reservoir. Energy Fuels 2023, 37, 8180–8189. [Google Scholar] [CrossRef]
- Wang, Y.; Xu, H.; Yu, W.; Bai, B.; Song, X.; Zhang, J. Surfactant induced reservoir wettability alteration: Recent theoretical and experimental advances in enhanced oil recovery. Pet. Sci. 2011, 8, 463–476. [Google Scholar] [CrossRef]
- Pal, N.; Saxena, N.; Laxmi, K.D.; Mandal, A. Interfacial behaviour, wettability alteration and emulsification characteristics of a novel surfactant: Implications for enhanced oil recovery. Chem. Eng. Sci. 2018, 187, 200–212. [Google Scholar] [CrossRef]
- Haghighi, O.M.; Zargar, G.; Khaksar Manshad, A.; Ali, M.; Takassi, M.A.; Ali, J.A.; Keshavarz, A. Effect of environment-friendly non-ionic surfactant on interfacial tension reduction and wettability alteration; implications for enhanced oil recovery. Energies 2020, 13, 3988. [Google Scholar] [CrossRef]
- Meng, Q.; Liu, H.; Wang, J. A critical review on fundamental mechanisms of spontaneous imbibition and the impact of boundary condition, fluid viscosity and wettability. Adv. Geo-Energy Res. 2017, 1, 1–17. [Google Scholar] [CrossRef]
- Meng, M.; Ge, H.; Ji, W.; Shen, Y.; Su, S. Monitor the process of shale spontaneous imbibition in co-current and counter-current displacing gas by using low field nuclear magnetic resonance method. J. Nat. Gas Sci. Eng. 2015, 27, 336–345. [Google Scholar] [CrossRef]
- Chakraborty, N.; Karpyn, Z.; Liu, S.; Yoon, H. Permeability evolution of shale during spontaneous imbibition. J. Nat. Gas Sci. Eng. 2017, 38, 590–596. [Google Scholar] [CrossRef]
- Zhou, Z.; Abass, H.; Li, X.; Bearinger, D.; Frank, W. Mechanisms of imbibition during hydraulic fracturing in shale formations. J. Pet. Sci. Eng. 2016, 141, 125–132. [Google Scholar] [CrossRef]
- Jing, W.; Huiqing, L.; Genbao, Q.; Yongcan, P.; Yang, G. Investigations on spontaneous imbibition and the influencing factors in tight oil reservoirs. Fuel 2019, 236, 755–768. [Google Scholar] [CrossRef]
- Chang, Q.; Huang, L.; Wu, X. A Molecular Dynamics Study on Low-Pressure Carbon Dioxide in the Water/Oil Interface for Enhanced Oil Recovery. SPE J. 2023, 28, 643–652. [Google Scholar] [CrossRef]
- Goodarzi, F.; Zendehboudi, S. Effects of salt and surfactant on interfacial characteristics of water/oil systems: Molecular dynamic simulations and dissipative particle dynamics. Ind. Eng. Chem. Res. 2019, 58, 8817–8834. [Google Scholar] [CrossRef]
- Boldon, L.; Laliberte, F.; Liu, L. Review of the fundamental theories behind small angle X-ray scattering, molecular dynamics simulations, and relevant integrated application. Nano Rev. 2015, 6, 25661. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Xiao, S.; Zhang, Z.; He, J. Effect of nanoparticles on spontaneous imbibition of water into ultraconfined reservoir capillary by molecular dynamics simulation. Energies 2017, 10, 506. [Google Scholar] [CrossRef]
- Yang, S.; Dehghanpour, H.; Binazadeh, M.; Dong, P. A molecular dynamics explanation for fast imbibition of oil in organic tight rocks. Fuel 2017, 190, 409–419. [Google Scholar] [CrossRef]
- Karna, N.K.; Oyarzua, E.; Walther, J.H.; Zambrano, H.A. Effect of the meniscus contact angle during early regimes of spontaneous imbibition in nanochannels. Phys. Chem. Chem. Phys. 2016, 18, 31997–32001. [Google Scholar] [CrossRef] [PubMed]
- Sang, Q.; Zhao, X.-Y.; Liu, H.-M.; Dong, M.-Z. Analysis of imbibition of n-alkanes in kerogen slits by molecular dynamics simulation for characterization of shale oil rocks. Pet. Sci. 2022, 19, 1236–1249. [Google Scholar] [CrossRef]
- Wang, S.; Wang, J.; Liu, H.; Liu, F. Impacts of polar molecules of crude oil on spontaneous imbibition in calcite nanoslit: A molecular dynamics simulation study. Energy Fuels 2021, 35, 13671–13686. [Google Scholar] [CrossRef]
- Shun, W.; Jing, W.; Huiqing, L. Forced Imbibition to Enhanced Tight/Shale Oil Recovery: A Molecular Dynamics Simulation Study. In Proceedings of the International Conference on Applied Energy, Bangkok, Thailand, 29 November–5 December 2021. [Google Scholar]
- Kang, W.-L.; Zhou, B.-B.; Issakhov, M.; Gabdullin, M. Advances in enhanced oil recovery technologies for low permeability reservoirs. Pet. Sci. 2022, 19, 1622–1640. [Google Scholar] [CrossRef]
- Wei, B.; Gao, K.; Song, T.; Zhang, X.; Pu, W.; Xu, X.; Wood, C. Nuclear-magnetic-resonance monitoring of mass exchange in a low-permeability matrix/fracture model during CO2 cyclic injection: A mechanistic study. SPE J. 2020, 25, 440–450. [Google Scholar] [CrossRef]
- Tu, J.; Sheng, J.J. Experimental and numerical study of surfactant solution spontaneous imbibition in shale oil reservoirs. J. Taiwan Inst. Chem. Eng. 2020, 106, 169–182. [Google Scholar] [CrossRef]
- Wang, S.; Javadpour, F.; Feng, Q. Molecular dynamics simulations of oil transport through inorganic nanopores in shale. Fuel 2016, 171, 74–86. [Google Scholar] [CrossRef]
- Yuan, L.; Zhang, Y.; Liu, S.; Zhang, Y.; Chen, C.; Song, Y. Study on the slip behavior of CO2-crude oil on nanopore surfaces with different wettability. Int. J. Heat Mass Transf. 2024, 218, 124787. [Google Scholar] [CrossRef]
- Sui, H.; Zhang, F.; Wang, Z.; Wang, D.; Wang, Y. Molecular simulations of oil adsorption and transport behavior in inorganic shale. J. Mol. Liq. 2020, 305, 112745. [Google Scholar] [CrossRef]
- Fang, T.; Wang, M.; Gao, Y.; Zhang, Y.; Yan, Y.; Zhang, J. Enhanced oil recovery with CO2/N2 slug in low permeability reservoir: Molecular dynamics simulation. Chem. Eng. Sci. 2019, 197, 204–211. [Google Scholar] [CrossRef]
- Zhang, C.; Dai, H.; Lu, P.; Wu, L.; Zhou, B.; Yu, C. Molecular dynamics simulation of distribution and diffusion behaviour of oil–water interfaces. Molecules 2019, 24, 1905. [Google Scholar] [CrossRef]
- Wang, Z.; Yu, C.; Zhao, J.; Guo, P.; Liu, H. Molecular dynamics simulation for quantitative characterization of wettability transition on silica surface. J. Mater. Res. Technol. 2022, 19, 4371–4380. [Google Scholar] [CrossRef]
- Li, G.; Su, Y.; Guo, Y.; Hao, Y.; Li, L. Frontier enhanced oil recovery (EOR) research on the application of imbibition techniques in high-pressure forced soaking of hydraulically fractured shale oil reservoirs. Geofluids 2021, 2021, 6634357. [Google Scholar] [CrossRef]
- Dong, H.; Zhu, Q.; Wang, L.; Yue, X.; Fang, H.; Wang, Z.; Liu, S.; Wei, S.; Lu, X. Effects of Shale Pore Size and Connectivity on scCO2 Enhanced Oil Recovery: A Molecular Dynamics Simulation Investigation. Langmuir 2023, 39, 6287–6299. [Google Scholar] [CrossRef] [PubMed]
- Shi, P.; Luo, H.; Tan, X.; Lu, Y.; Zhang, H.; Yang, X. Molecular dynamics simulation study of adsorption of anionic–nonionic surfactants at oil/water interfaces. RSC Adv. 2022, 12, 27330–27343. [Google Scholar] [CrossRef] [PubMed]
- Xu, G.; Shi, Y.; Jiang, Y.; Jia, C.; Gao, Y.; Han, X.; Zeng, X. Characteristics and influencing factors for forced imbibition in tight sandstone based on low-field nuclear magnetic resonance measurements. Energy Fuels 2018, 32, 8230–8240. [Google Scholar] [CrossRef]
- Albers, B. Modeling the hysteretic behavior of the capillary pressure in partially saturated porous media: A review. Acta Mech. 2014, 225, 2163–2189. [Google Scholar] [CrossRef]
- Wu, P.; Nikolov, A.D.; Wasan, D.T. Capillary rise: Validity of the dynamic contact angle models. Langmuir 2017, 33, 7862–7872. [Google Scholar] [CrossRef]
- Zhang, L.; Ping, J.; Tang, B.; Kang, L.; Imani, G.; Yang, Y.; Sun, H.; Zhong, J.; Yao, J.; Fan, D. Mathematical Model of Two-Phase Spontaneous Imbibition with Dynamic Contact Angle. Transp. Porous Media 2023, 148, 157–172. [Google Scholar] [CrossRef]
- Martic, G.; Gentner, F.; Seveno, D.; Coulon, D.; De Coninck, J.; Blake, T. A molecular dynamics simulation of capillary imbibition. Langmuir 2002, 18, 7971–7976. [Google Scholar] [CrossRef]
- Sun, E.W.-H.; Bourg, I.C. Molecular dynamics simulations of mineral surface wettability by water versus CO2: Thin films, contact angles, and capillary pressure in a silica nanopore. J. Phys. Chem. C 2020, 124, 25382–25395. [Google Scholar] [CrossRef]
- Wu, P.; Nikolov, A.D.; Wasan, D.T. Two-phase displacement dynamics in capillaries-nanofluid reduces the frictional coefficient. J. Colloid Interface Sci. 2018, 532, 153–160. [Google Scholar] [CrossRef] [PubMed]
- Rehfeld, S.J. Adsorption of sodium dodecyl sulfate at various hydrocarbon-water interfaces. J. Phys. Chem. 1967, 71, 738–745. [Google Scholar] [CrossRef]
- Caineng, Z.; Zhi, Y.; Jingwei, C.; Rukai, Z.; Lianhua, H.; Shizhen, T.; Xuanjun, Y.; Songtao, W.; Senhu, L.; Lan, W. Formation mechanism, geological characteristics and development strategy of nonmarine shale oil in China. Pet. Explor. Dev. 2013, 40, 15–27. [Google Scholar]
- Stevens, S.H.; Moodhe, K.D.; Kuuskraa, V.A. China Shale Gas and Shale Oil Resource Evaluation and Technical Challenges. In Proceedings of the SPE Asia Pacific Oil and Gas Conference and Exhibition, Jakarta, Indonesia, 22–24 October 2013. [Google Scholar]
- Zou, C.; Dong, D.; Wang, S.; Li, J.; Li, X.; Wang, Y.; Li, D.; Cheng, K. Geological characteristics and resource potential of shale gas in China. Pet. Explor. Dev. 2010, 37, 641–653. [Google Scholar] [CrossRef]
- Zhu, D.-Y.; Zhao, Y.-H.; Zhang, H.-J.; Zhao, Q.; Shi, C.-Y.; Qin, J.-H.; Su, Z.-H.; Wang, G.-Q.; Liu, Y.; Hou, J.-R. Combined imbibition system with black nanosheet and low-salinity water for improving oil recovery in tight sandstone reservoirs. Pet. Sci. 2022, 20, 1562–1571. [Google Scholar] [CrossRef]
- Wei, B.; Zhang, X.; Wu, R.; Zou, P.; Gao, K.; Xu, X.; Pu, W.; Wood, C. Pore-scale monitoring of CO2 and N2 flooding processes in a tight formation under reservoir conditions using nuclear magnetic resonance (NMR): A case study. Fuel 2019, 246, 34–41. [Google Scholar] [CrossRef]
- Fei, W.; Yingqi, R.; Qiaoyun, C.; Zhang, S. A pressure drop model of post-fracturing shut-in considering the effect of fracturing-fluid imbibition and oil replacement. Pet. Explor. Dev. 2021, 48, 1440–1449. [Google Scholar]
- Lu, M.; Cao, H.; Sun, W.; Yan, X.; Yang, Z.; Xu, Y.; Wang, Z.; Ouyang, M. Quantitative prediction of seismic rock physics of hybrid tight oil reservoirs of the Permian Lucaogou Formation, Junggar Basin, Northwest China. J. Asian Earth Sci. 2019, 178, 216–223. [Google Scholar] [CrossRef]
- Pan, L.; Dai, F.; Li, G.; Liu, S. A TGA/DTA-MS investigation to the influence of process conditions on the pyrolysis of Jimsar oil shale. Energy 2015, 86, 749–757. [Google Scholar] [CrossRef]
- Dong, X.; Xu, W.; Liu, H.; Chen, Z.; Lu, N.; Wang, W. On the replacement behavior of CO2 in nanopores of shale oil reservoirs: Insights from wettability tests and molecular dynamics simulations. Geoenergy Sci. Eng. 2023, 223, 211528. [Google Scholar] [CrossRef]
- Huang, P.; Shen, L.; Gan, Y.; Maggi, F.; El-Zein, A.; Pan, Z. Atomistic study of dynamic contact angles in CO2–water–silica system. Langmuir 2019, 35, 5324–5332. [Google Scholar] [CrossRef]
- Le, T.T.B.; Striolo, A.; Gautam, S.S.; Cole, D.R. Propane–water mixtures confined within cylindrical silica nanopores: Structural and dynamical properties probed by molecular dynamics. Langmuir 2017, 33, 11310–11320. [Google Scholar] [CrossRef]
- Xu, J.; Zhan, S.; Wang, W.; Su, Y.; Wang, H. Molecular dynamics simulations of two-phase flow of n-alkanes with water in quartz nanopores. Chem. Eng. J. 2022, 430, 132800. [Google Scholar] [CrossRef]
- Liu, L.; Ye, Z.; Lai, N. Characterization Method for Non-uniform Wettability of Shale Oil Reservoir. Int. Core J. Eng. 2022, 8, 179–186. [Google Scholar]
- Koretsky, C.M.; Sverjensky, D.A.; Sahai, N. A model of surface site types on oxide and silicate minerals based on crystal chemistry; implications for site types and densities, multi-site adsorption, surface infrared spectroscopy, and dissolution kinetics. Am. J. Sci. 1998, 298, 349–438. [Google Scholar] [CrossRef]
- Su, Y.; Zha, M.; Ding, X.; Qu, J.; Wang, X.; Yang, C.; Iglauer, S. Pore type and pore size distribution of tight reservoirs in the Permian Lucaogou Formation of the Jimsar Sag, Junggar Basin, NW China. Mar. Pet. Geol. 2018, 89, 761–774. [Google Scholar] [CrossRef]
- Jin, J.; Yang, Z.; Chen, X.; Li, L.; Yang, H.; Ju, Y.; Qiao, P.; Sun, Y. Characteristics of Micro/Nano Pores and Hydrocarbon Accumulation in a Continental Shale Oil Reservoir—A Case Study of the Lucaogou Formation in the Jimsar Sag, Junggar Basin, Northwest China. J. Nanosci. Nanotechnol. 2021, 21, 262–273. [Google Scholar] [CrossRef] [PubMed]
- Wang, X.; Song, Y.; Guo, X.; Chang, Q.; Kong, Y.; Zheng, M.; Qin, Z.; Yang, X. Pore-throat structure characteristics of tight reservoirs of the Middle Permian Lucaogou formation in the Jimsar Sag, Junggar Basin, northwest China. J. Pet. Sci. Eng. 2022, 208, 109245. [Google Scholar] [CrossRef]
- Cygan, R.T.; Liang, J.-J.; Kalinichev, A.G. Molecular models of hydroxide, oxyhydroxide, and clay phases and the development of a general force field. J. Phys. Chem. B 2004, 108, 1255–1266. [Google Scholar] [CrossRef]
- Nie, X.; Chen, J.; Sheng, N.; Zeng, L.; Yang, H.; Wang, C. Effect of water molecules on nanoscale wetting behaviour of molecular ethanol on hydroxylated SiO2. Mol. Simul. 2017, 43, 1377–1384. [Google Scholar] [CrossRef]
- Hong, X.; Yu, H.; Xu, H.; Wang, X.; Jin, X.; Wu, H.; Wang, F. Competitive adsorption of asphaltene and n-heptane on quartz surfaces and its effect on crude oil transport through nanopores. J. Mol. Liq. 2022, 359, 119312. [Google Scholar] [CrossRef]
- Zhan, S.; Su, Y.; Jin, Z.; Wang, W.; Li, L. Effect of water film on oil flow in quartz nanopores from molecular perspectives. Fuel 2020, 262, 116560. [Google Scholar] [CrossRef]
- Deng, Y.; Wu, Q.; Li, Z.; Huang, X.; Rao, S.; Liang, Y.; Lu, H. Crystal face dependent wettability of α-quartz: Elucidation by time-of-flight secondary ion mass spectrometry techniques combined with molecular dynamics. J. Colloid Interface Sci. 2022, 607, 1699–1708. [Google Scholar] [CrossRef]
- Mayo, S.L.; Olafson, B.D.; Goddard, W.A. DREIDING: A generic force field for molecular simulations. J. Phys. Chem. 1990, 94, 8897–8909. [Google Scholar] [CrossRef]
- Jorgensen, W.L.; Maxwell, D.S.; Tirado-Rives, J. Development and testing of the OPLS all-atom force field on conformational energetics and properties of organic liquids. J. Am. Chem. Soc. 1996, 118, 11225–11236. [Google Scholar] [CrossRef]
- Wang, S.; Feng, Q.; Javadpour, F.; Xia, T.; Li, Z. Oil adsorption in shale nanopores and its effect on recoverable oil-in-place. Int. J. Coal Geol. 2015, 147, 9–24. [Google Scholar] [CrossRef]
- Berendsen, H.; Grigera, J.; Straatsma, T. The missing term in effective pair potentials. J. Phys. Chem. 1987, 91, 6269–6271. [Google Scholar] [CrossRef]
- Zhao, J.; Yao, G.; Ramisetti, S.B.; Hammond, R.B.; Wen, D. Molecular dynamics investigation of substrate wettability alteration and oil transport in a calcite nanopore. Fuel 2019, 239, 1149–1161. [Google Scholar] [CrossRef]
- Quezada, G.R.; Jeldres, M.; Toro, N.; Robles, P.; Toledo, P.G.; Jeldres, R.I. Understanding the flocculation mechanism of quartz and kaolinite with polyacrylamide in seawater: A molecular dynamics approach. Colloids Surf. A Physicochem. Eng. Asp. 2021, 608, 125576. [Google Scholar] [CrossRef]
- Shen, X.; Bourg, I.C. Molecular dynamics simulations of the colloidal interaction between smectite clay nanoparticles in liquid water. J. Colloid Interface Sci. 2021, 584, 610–621. [Google Scholar] [CrossRef] [PubMed]
- Meng, J.; Li, C.; Yan, S.; Zhang, S.; Zhang, H.; Wang, G.; Yang, X. Atomic-level insights into the mechanism of saline-regulated montmorillonite (001)-salt droplet interface wetting: A molecular dynamics study. Appl. Clay Sci. 2022, 224, 106513. [Google Scholar] [CrossRef]
- Plimpton, S. Fast parallel algorithms for short-range molecular dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef]
- Humphrey, W.; Dalke, A.; Schulten, K. VMD: Visual molecular dynamics. J. Mol. Graph. 1996, 14, 33–38. [Google Scholar] [CrossRef] [PubMed]
- Guo, L.; Tang, G.; Kumar, S. Droplet morphology and mobility on lubricant-impregnated surfaces: A molecular dynamics study. Langmuir 2019, 35, 16377–16387. [Google Scholar] [CrossRef] [PubMed]
- Chen, J.; Mi, J.-G.; Chan, K.-Y. Comparison of different mixing rules for prediction of density and residual internal energy of binary and ternary Lennard–Jones mixtures. Fluid Phase Equilibria 2001, 178, 87–95. [Google Scholar] [CrossRef]
- Wang, L.; Lyu, W.; Ji, Z.; Wang, L.; Liu, S.; Fang, H.; Yue, X.; Wei, S.; Liu, S.; Wang, Z. Molecular Dynamics Insight into the CO2 Flooding Mechanism in Wedge-Shaped Pores. Molecules 2022, 28, 188. [Google Scholar] [CrossRef] [PubMed]
- Liu, B.; Liu, W.; Pan, Z.; Yu, L.; Xie, Z.; Lv, G.; Zhao, P.; Chen, D.; Fang, W. Supercritical CO2 breaking through a water bridge and enhancing shale oil recovery: A molecular dynamics simulation study. Energy Fuels 2022, 36, 7558–7568. [Google Scholar] [CrossRef]


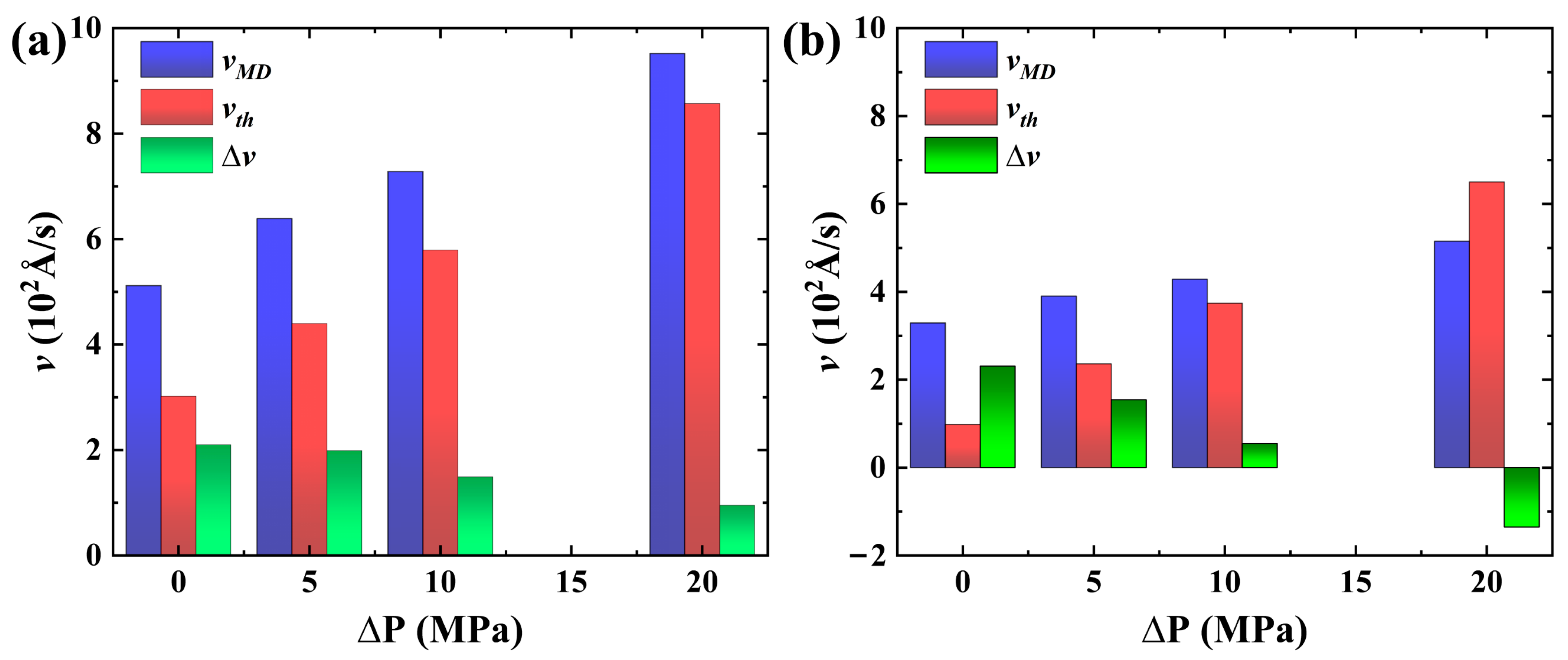
| ΔP (Mpa) | (102 Å/s) | R | ||
|---|---|---|---|---|
| System I | System II | System I | System II | |
| 0 | 5.12 | 3.29 | 1.00 | 1.00 |
| 5 | 6.39 | 3.90 | 1.24 | 1.19 |
| 10 | 7.28 | 4.29 | 1.42 | 1.30 |
| 20 | 9.52 | 5.15 | 1.85 | 1.56 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiang, W.; Lv, W.; Jia, N.; Lu, X.; Wang, L.; Wang, K.; Mei, Y. Study on the Effects of Wettability and Pressure in Shale Matrix Nanopore Imbibition during Shut-in Process by Molecular Dynamics Simulations. Molecules 2024, 29, 1112. https://doi.org/10.3390/molecules29051112
Jiang W, Lv W, Jia N, Lu X, Wang L, Wang K, Mei Y. Study on the Effects of Wettability and Pressure in Shale Matrix Nanopore Imbibition during Shut-in Process by Molecular Dynamics Simulations. Molecules. 2024; 29(5):1112. https://doi.org/10.3390/molecules29051112
Chicago/Turabian StyleJiang, Wen, Weifeng Lv, Ninghong Jia, Xiaoqing Lu, Lu Wang, Kai Wang, and Yuhao Mei. 2024. "Study on the Effects of Wettability and Pressure in Shale Matrix Nanopore Imbibition during Shut-in Process by Molecular Dynamics Simulations" Molecules 29, no. 5: 1112. https://doi.org/10.3390/molecules29051112
APA StyleJiang, W., Lv, W., Jia, N., Lu, X., Wang, L., Wang, K., & Mei, Y. (2024). Study on the Effects of Wettability and Pressure in Shale Matrix Nanopore Imbibition during Shut-in Process by Molecular Dynamics Simulations. Molecules, 29(5), 1112. https://doi.org/10.3390/molecules29051112









