Eclipsed and Twisted Excimers of Pyrene and 2-Azapyrene: How Nitrogen Substitution Impacts Excimer Emission
Abstract
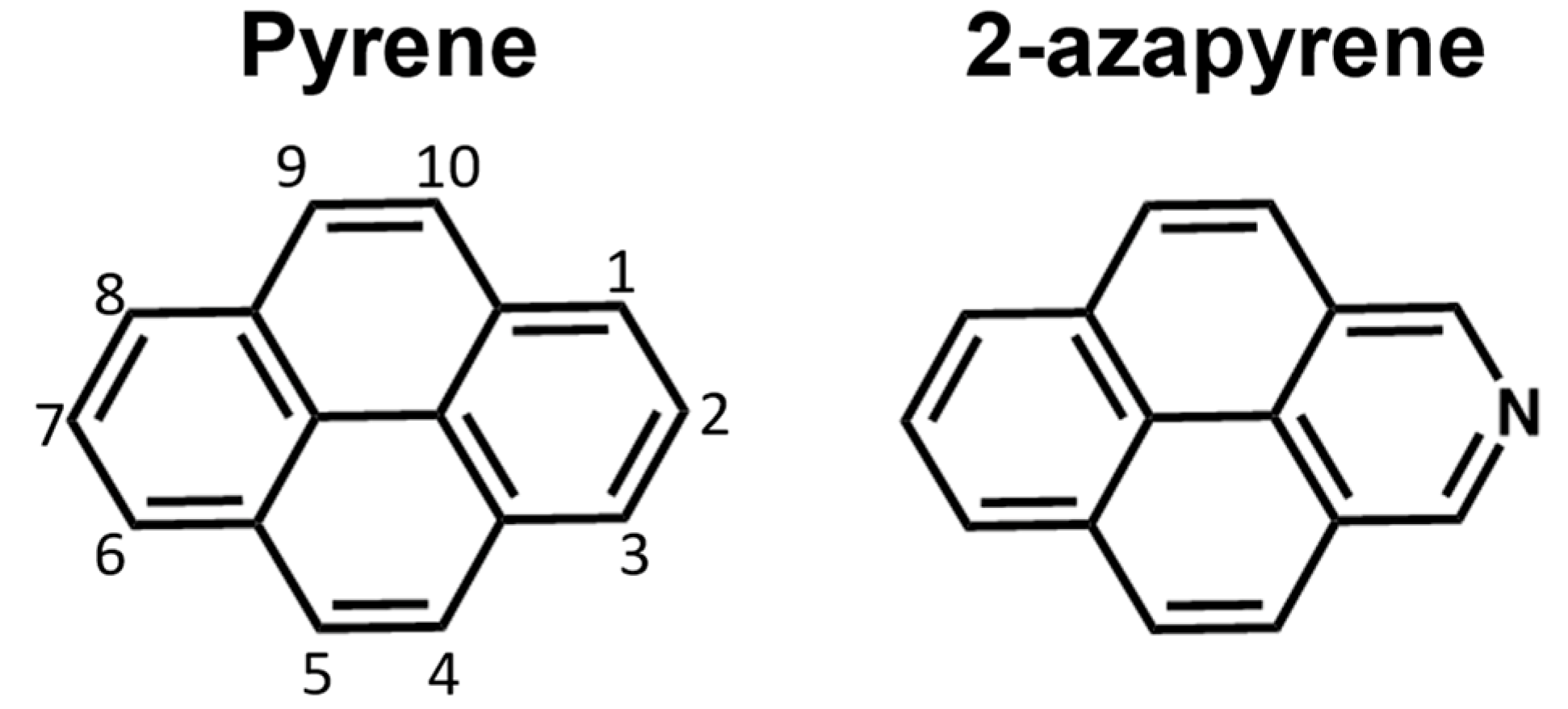
1. Introduction
2. Results and Discussion
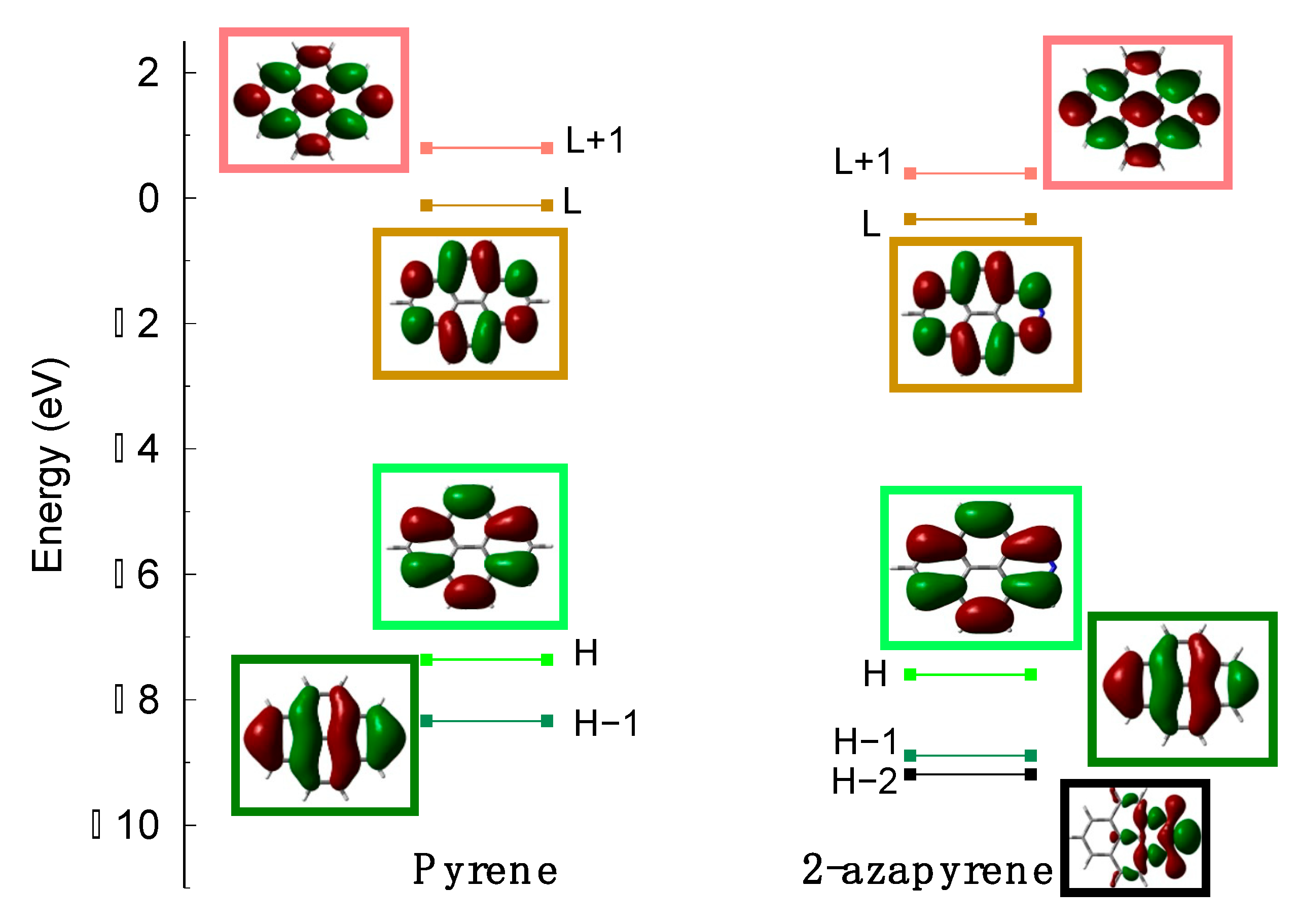
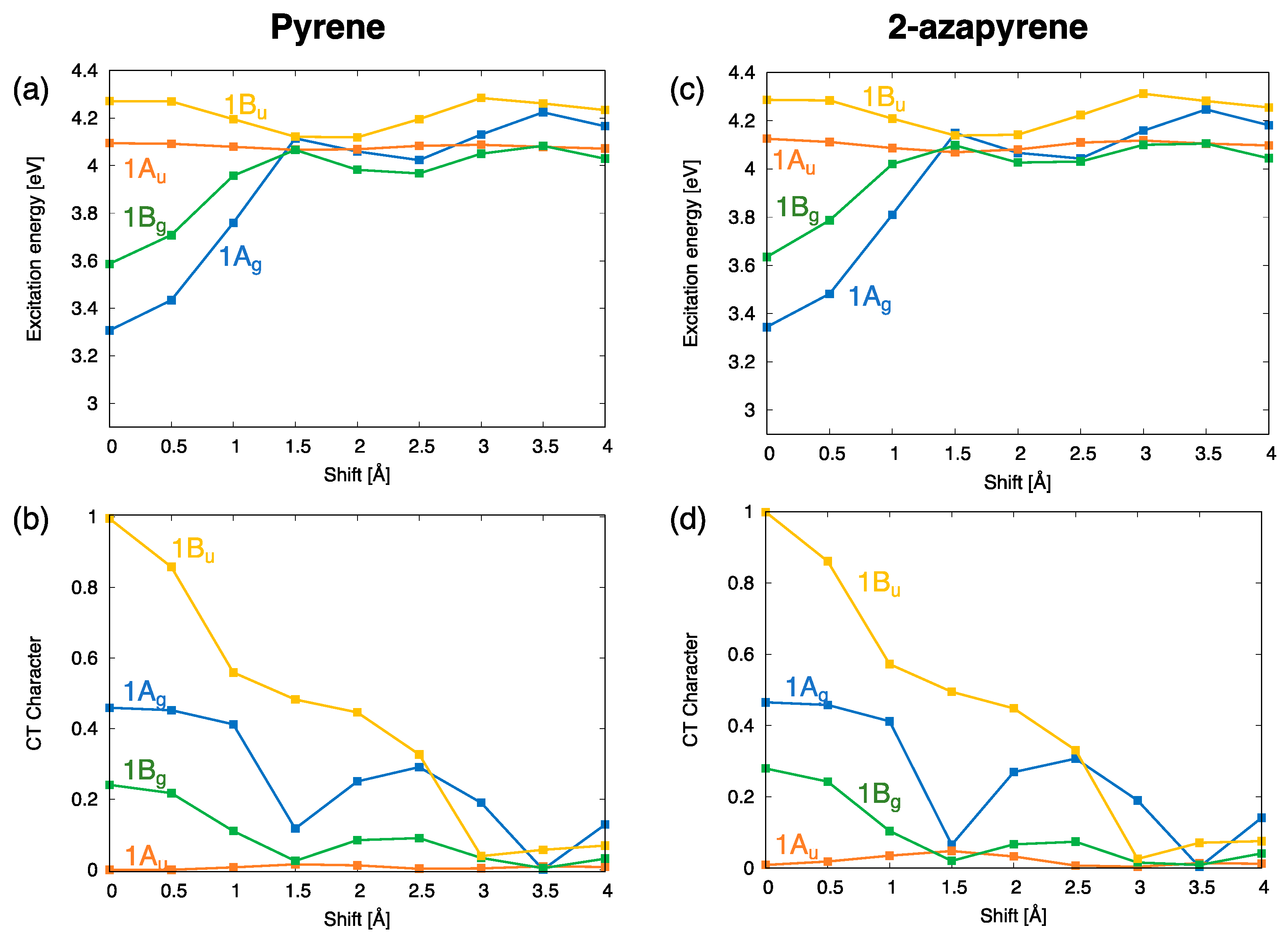
2.1. Orbital Nature of Low-Lying Excited States of Pyrene and 2-Azapyrene
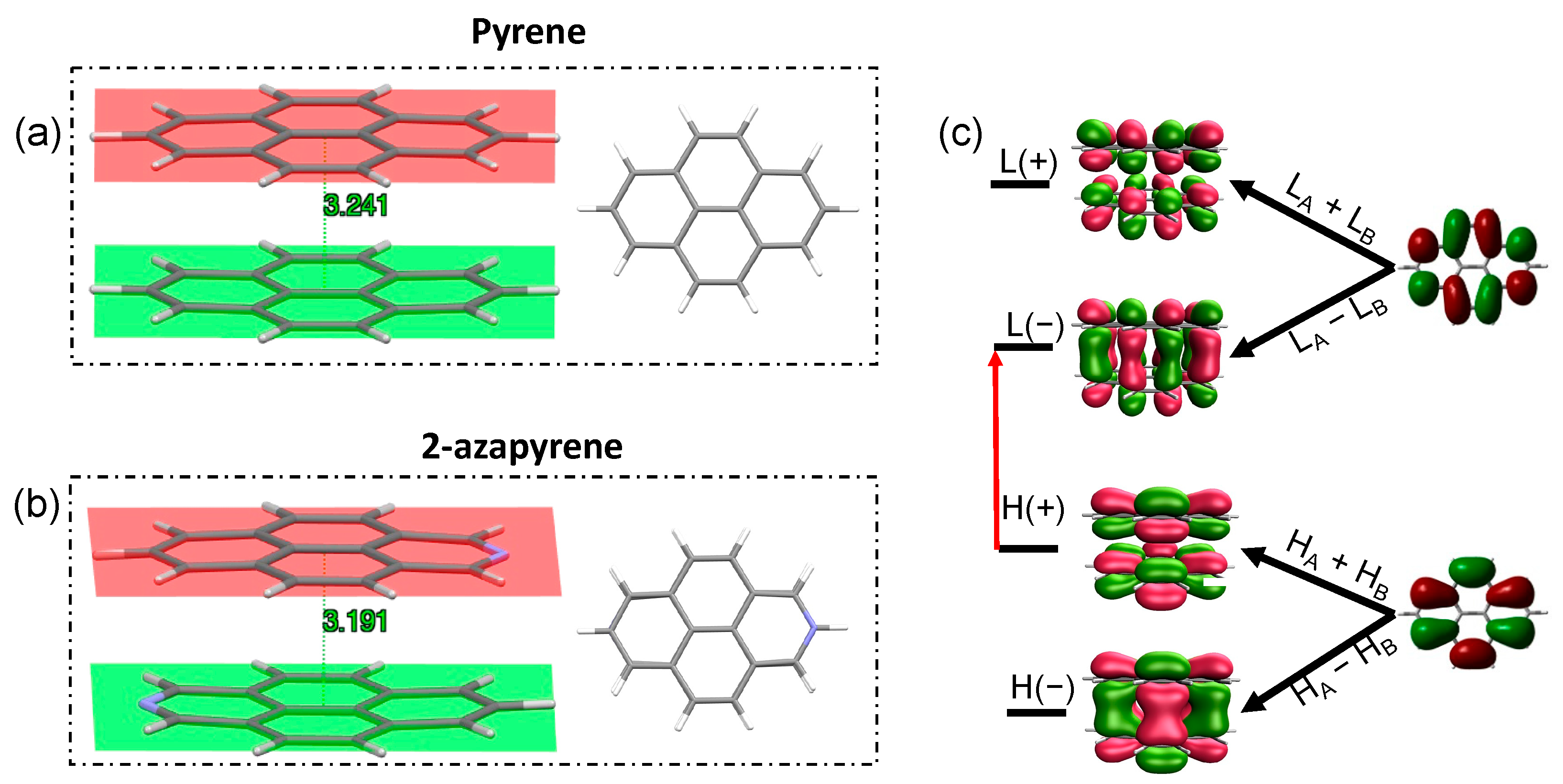
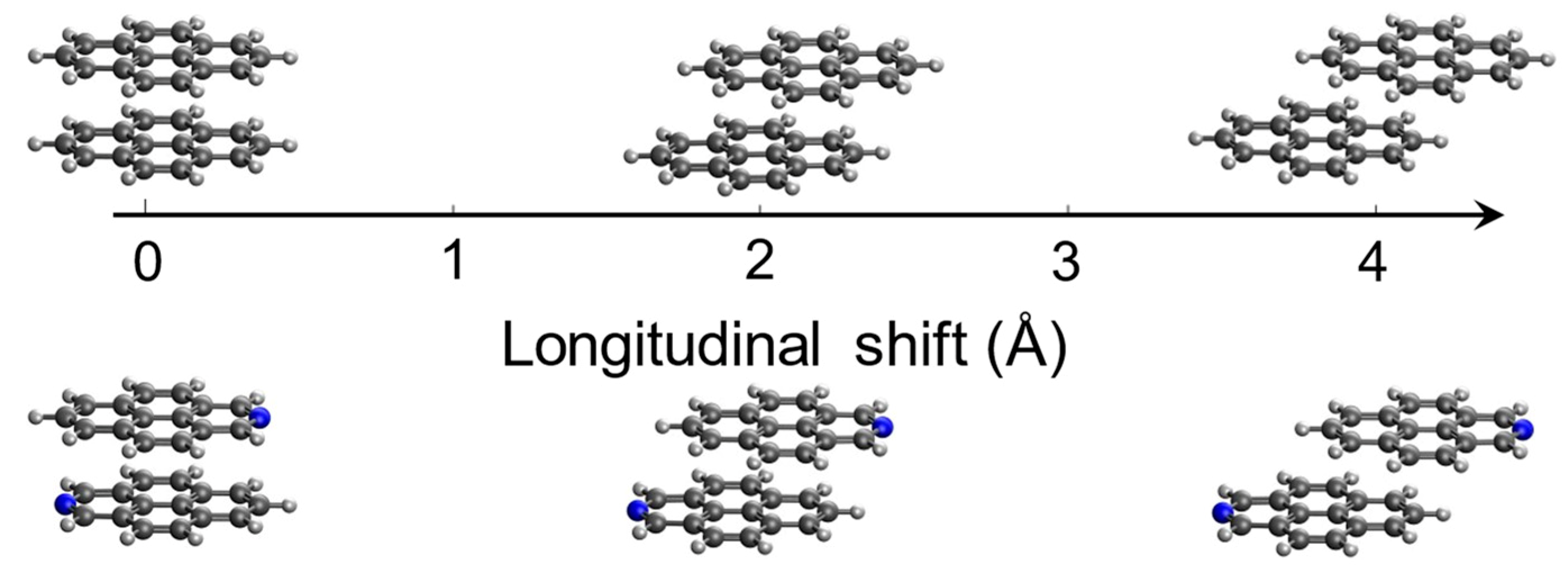
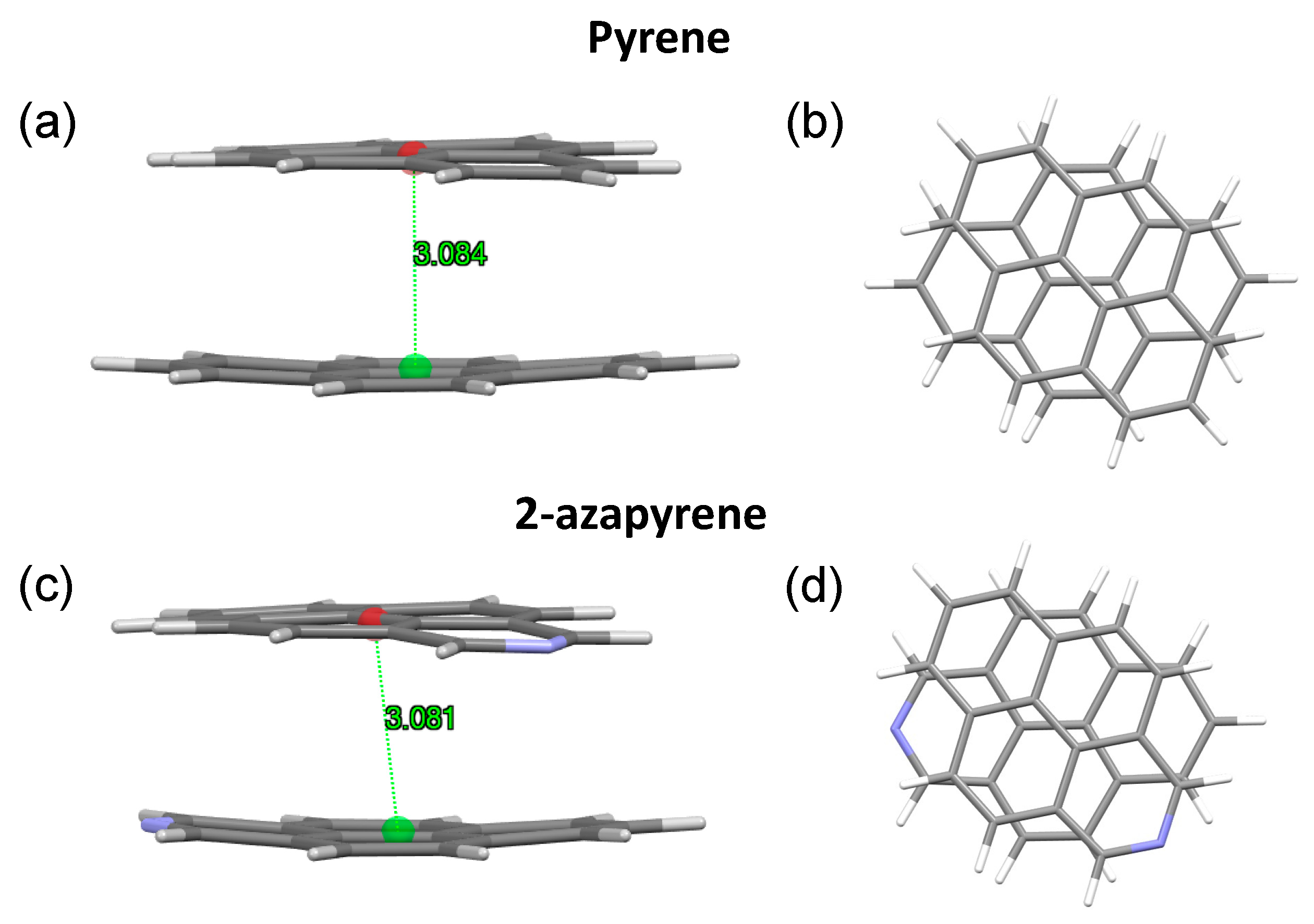
2.2. Ground-State Dimer Structures
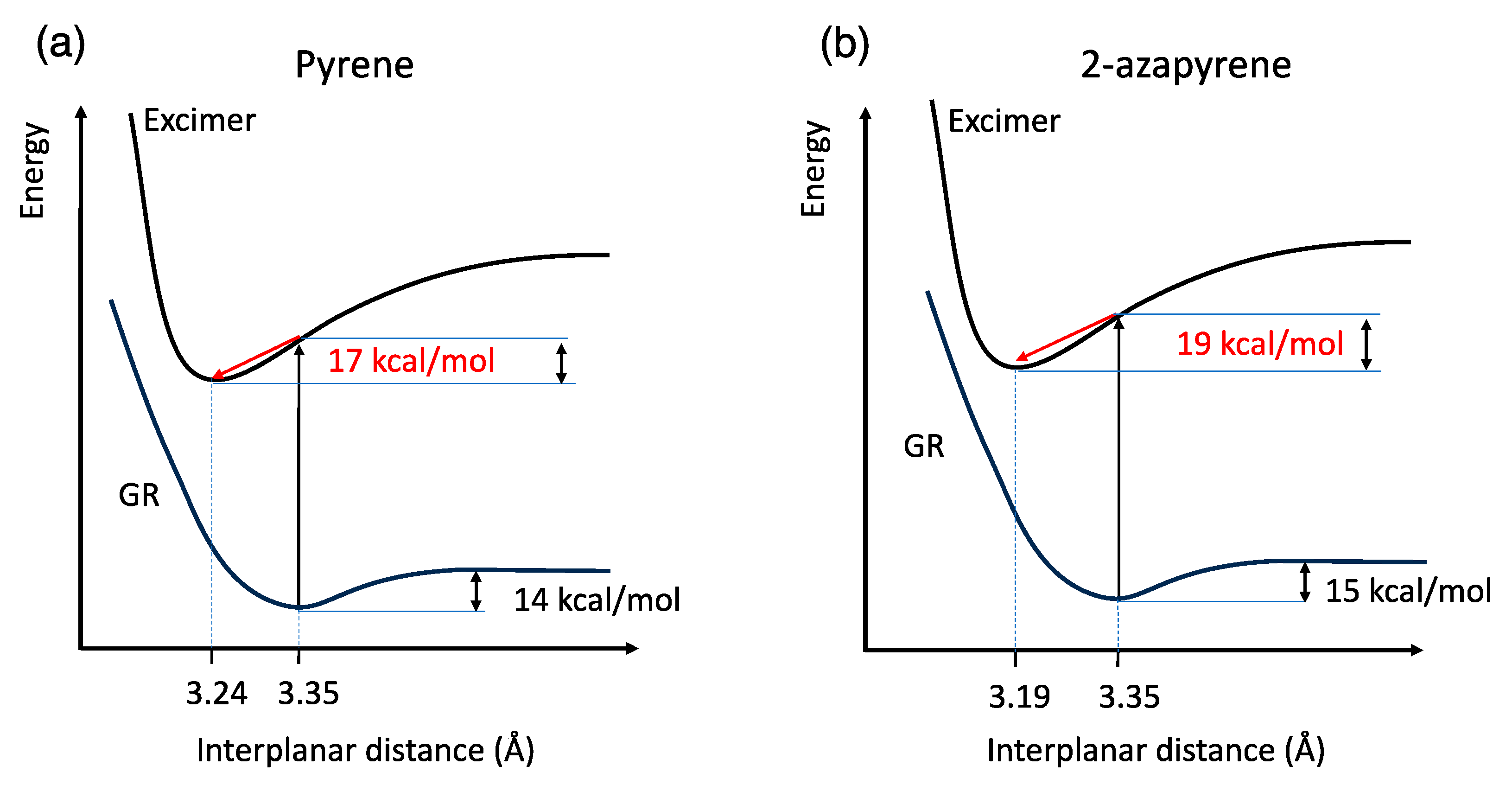
2.3. Eclipsed Excimer Structures
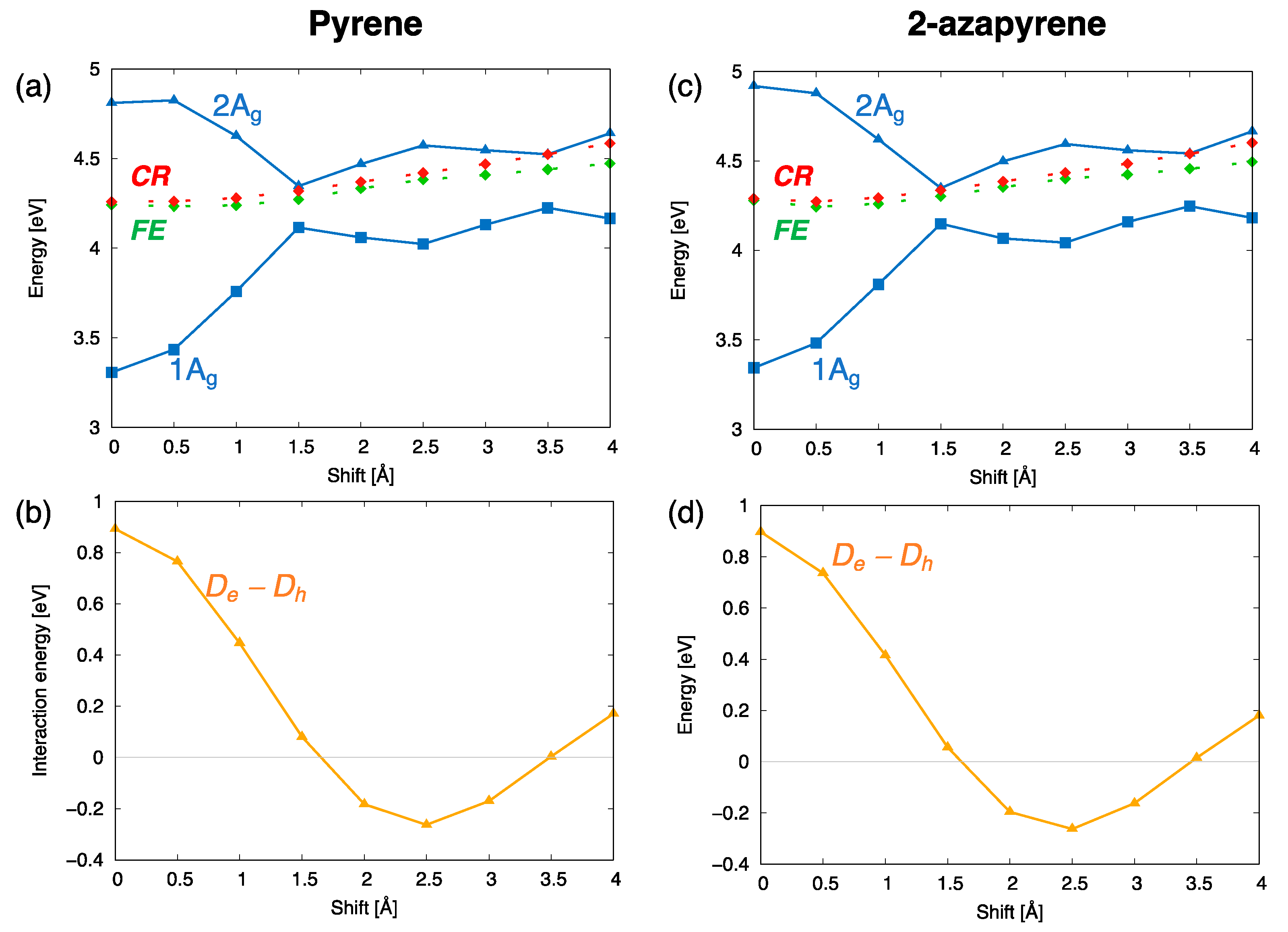
2.4. Exciton States and Excimer Formation: Evidence from Diabatization
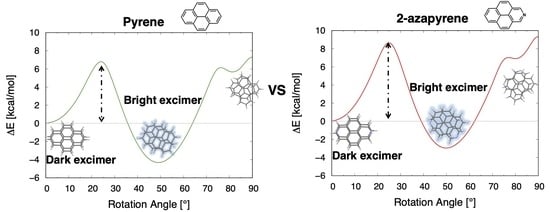
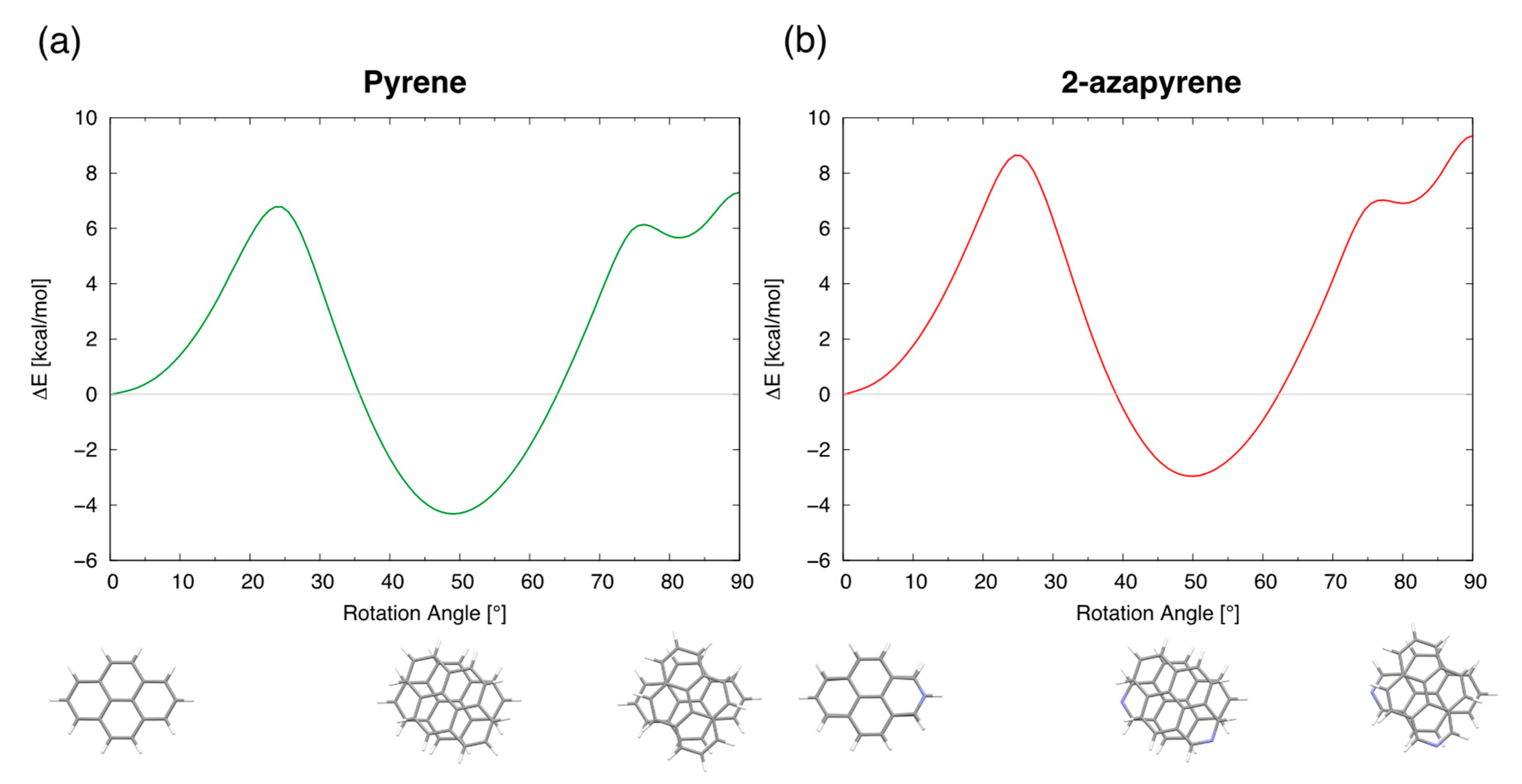
2.5. Rotated Excimer Structures and Interconversion from Eclipsed Excimers
3. Computational Details
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Patel, A.B.; Shaikh, S.; Jain, K.R.; Desai, C.; Madamwar, D. Polycyclic Aromatic Hydrocarbons: Sources, Toxicity, and Remediation Approaches. Front. Microbiol. 2020, 11, 562813. [Google Scholar] [CrossRef] [PubMed]
- Kopacz, N.; Corazzi, M.A.; Poggiali, G.; von Essen, A.; Kofman, V.; Fornaro, T.; van Ingen, H.; Camprubi, E.; King, H.E.; Brucato, J.; et al. The photochemical evolution of polycyclic aromatic hydrocarbons and nontronite clay on early Earth and Mars. Icarus 2023, 394, 115437. [Google Scholar] [CrossRef]
- Wang, C.; Dong, H.; Hu, W.; Liu, Y.; Zhu, D. Semiconducting π-Conjugated Systems in Field-Effect Transistors: A Material Odyssey of Organic Electronics. Chem. Rev. 2012, 112, 2208–2267. [Google Scholar] [CrossRef] [PubMed]
- Wang, C.; Dong, H.; Jiang, L.; Hu, W. Organic semiconductor crystals. Chem. Soc. Rev. 2018, 47, 422–500. [Google Scholar] [CrossRef] [PubMed]
- Jiang, H.; Hu, W. The Emergence of Organic Single-Crystal Electronics. Angew. Chem. Int. Ed. 2020, 59, 1408–1428. [Google Scholar] [CrossRef] [PubMed]
- Ahmad, S. Organic semiconductors for device applications: Current trends and future prospects. J. Polym. Eng. 2014, 34, 279–338. [Google Scholar] [CrossRef]
- Shirota, Y.; Kageyama, H. Charge carrier transporting molecular materials and their applications in devices. Chem. Rev. 2007, 107, 953–1010. [Google Scholar] [CrossRef] [PubMed]
- Friend, R. Organic Materials for Large Area Electronics. Mater. Sci. Forum 2008, 608, 159–179. [Google Scholar] [CrossRef]
- Figueira-Duarte, T.M.; Müllen, K. Pyrene-based materials for organic electronics. Chem. Rev. 2011, 111, 7260–7314. [Google Scholar] [CrossRef]
- Karpovich, D.S.; Blanchard, G.J. Relating the polarity-dependent fluorescence response of pyrene to vibronic coupling. Achieving a fundamental understanding of the py polarity scale. J. Phys. Chem. 1995, 99, 3951–3958. [Google Scholar] [CrossRef]
- Kalyanasundaram, K.; Thomas, J.K. Environmental effects on vibronic band intensities in pyrene monomer fluorescence and their application in studies of micellar systems. J. Am. Chem. Soc. 1977, 99, 2039–2044. [Google Scholar] [CrossRef]
- Förster, T.; Kasper, K. Ein Konzentrationsumschlag der Fluoreszenz. Z. Phys. Chem. 1954, 1, 275–277. [Google Scholar] [CrossRef]
- Sahoo, D.; Narayanaswami, V.; Kay, C.M.; Ryan, R.O. Pyrene Excimer Fluorescence: A Spatially Sensitive Probe to Monitor Lipid-Induced Helical Rearrangement of Apolipophorin III. Biochemistry 2000, 39, 6594–6601. [Google Scholar] [CrossRef] [PubMed]
- Bains, G.K.; Kim, S.H.; Sorin, E.J.; Narayanaswami, V. The Extent of Pyrene Excimer Fluorescence Emission Is a Reflector of Distance and Flexibility: Analysis of the Segment Linking the LDL Receptor-Binding and Tetramerization Domains of Apolipoprotein E3. Biochemistry 2012, 51, 6207–6219. [Google Scholar] [CrossRef] [PubMed]
- Babu, B.; Ali, T.A.; Ochappan, T.; Mack, J.; Nyokong, T.; Sethuraman, M.G. Photocytotoxicity of heavy-atom-free thiobarbituric acid functionalized pyrene derivatives against MCF-7 cancer cells. Photodiagnosis Photodyn. Ther. 2021, 33, 102102. [Google Scholar] [CrossRef] [PubMed]
- Takimiya, K.; Bulgarevich, K.; Abbas, M.; Horiuchi, S.; Ogaki, T.; Kawabata, K.; Ablat, A. “Manipulation” of Crystal Structure by Methylthiolation Enabling Ultrahigh Mobility in a Pyrene-Based Molecular Semiconductor. Adv. Mater. 2021, 33, 2102914. [Google Scholar] [CrossRef] [PubMed]
- Ju, H.; Wang, K.; Zhang, J.; Geng, H.; Liu, Z.; Zhang, G.; Zhao, Y.; Zhang, D. 1,6- and 2,7-trans-β-Styryl Substituted Pyrenes Exhibiting Both Emissive and Semiconducting Properties in the Solid State. Chem. Mater. 2017, 29, 3580–3588. [Google Scholar] [CrossRef]
- Cho, H.; Lee, S.; Cho, N.S.; Jabbour, G.E.; Kwak, J.; Hwang, D.-H.; Lee, C. High-Mobility Pyrene-Based Semiconductor for Organic Thin-Film Transistors. ACS Appl. Mater. Interfaces 2013, 5, 3855–3860. [Google Scholar] [CrossRef] [PubMed]
- Han, Y.; Hu, Z.; Liu, M.; Li, M.; Wang, T.; Chen, Y. Synthesis, Characterization, and Properties of Diazapyrenes via Bischler-Napieralski Reaction. J. Org. Chem. 2019, 84, 3953–3959. [Google Scholar] [CrossRef]
- Molenda, R.; Boldt, S.; Villinger, A.; Ehlers, P.; Langer, P. Synthesis of 2-Azapyrenes and Their Photophysical and Electrochemical Properties. J. Org. Chem. 2020, 85, 12823–12842. [Google Scholar] [CrossRef]
- Taniya, O.S.; Khasanov, A.F.; Varaksin, M.V.; Starnovskaya, E.S.; Krinochkin, A.P.; Savchuk, M.I.; Kopchuk, D.S.; Kovalev, I.S.; Kim, G.A.; Nosova, E.V.; et al. Azapyrene-based fluorophores: Synthesis and photophysical properties. New J. Chem. 2021, 45, 20955–20971. [Google Scholar] [CrossRef]
- Mukherjee, A.; Akulov, A.A.; Santra, S.; Varaksin, M.V.; Kim, G.A.; Kopchuk, D.S.; Taniya, O.S.; Zyryanov, G.V.; Chupakhin, O.N. 2,7-Diazapyrenes: A brief review on synthetic strategies and application opportunities. RSC Adv. 2022, 12, 9323–9341. [Google Scholar] [CrossRef] [PubMed]
- Zhu, Z.; Zhang, D.; Xiao, T.; Fang, Y.H.; Xiao, X.; Wang, X.G.; Jiang, S.D.; Zhao, D. Rational Design of an Air-Stable, High-Spin Diradical with Diazapyrene. Angew. Chem. Int. Ed. 2023, 135, e202314900. [Google Scholar] [CrossRef]
- Vardanyan, A.; Boldt, S.; Villinger, A.; Ehlers, P.; Langer, P. Synthesis and Properties of 1-Azapyrenes. J. Org. Chem. 2022, 87, 11296–11308. [Google Scholar] [CrossRef] [PubMed]
- Fan, G.; Yu, X.; Han, X.; Zhao, Z.; Liu, S. Tunable White-Light Emissions of Azapyrene Derivatives with Cucurbit[n]uril Hosts in Aqueous Solution. Org. Lett. 2021, 23, 6633–6637. [Google Scholar] [CrossRef] [PubMed]
- Tucker, S.A.; Acree, W.E.; Tanga, M.J. Polycyclic aromatic nitrogen heterocycles. Part II. Effect of solvent polarity on the fluorescence emission fine structure of three azapyrene compounds. Appl. Spectrosc. 1991, 45, 57–60. [Google Scholar] [CrossRef]
- Kirchlechner, R.; Jutz, C. 2-Azapyrene. Angew. Chem. Int. Ed. Engl. 1968, 7, 376–377. [Google Scholar] [CrossRef]
- Kołaski, M.; Arunkumar, C.R.; Kim, K.S. Aromatic Excimers: Ab Initio and TD-DFT Study. J. Chem. Theory Comput. 2013, 9, 847–856. [Google Scholar] [CrossRef]
- Hoche, J.; Schmitt, H.C.; Humeniuk, A.; Fischer, I.; Mitrić, R.; Röhr, M.I.S. The mechanism of excimer formation: An experimental and theoretical study on the pyrene dimer. Phys. Chem. Chem. Phys. 2017, 19, 25002–25015. [Google Scholar] [CrossRef]
- Huenerbein, R.; Grimme, S. Time-dependent density functional study of excimers and exciplexes of organic molecules. Chem. Phys. 2008, 343, 362–371. [Google Scholar] [CrossRef]
- Reiter, S.; Roos, M.K.; de Vivie-Riedle, R. Excited State Conformations of Bridged and Unbridged Pyrene Excimers. ChemPhotoChem 2019, 3, 881–888. [Google Scholar] [CrossRef]
- do Casal, M.T.; Cardozo, T.M. Impact of low-cost methods in the description of excimer and exciplex formation: Pyrene–pyrene and pyrene–naphthalene case studies. Theor. Chem. Acc. 2020, 139, 144. [Google Scholar] [CrossRef]
- Shi, B.; Nachtigallová, D.; Aquino, A.J.A.; Machado, F.B.C.; Lischka, H. Excited states and excitonic interactions in prototypic polycyclic aromatic hydrocarbon dimers as models for graphitic interactions in carbon dots. Phys. Chem. Chem. Phys. 2019, 21, 9077–9088. [Google Scholar] [CrossRef] [PubMed]
- Hancock, A.C.; Goerigk, L. Noncovalently bound excited-state dimers: A perspective on current time-dependent density functional theory approaches applied to aromatic excimer models. RSC Adv. 2023, 13, 35964–35984. [Google Scholar] [CrossRef] [PubMed]
- Shao, X.; Aquino, A.J.A.; Otyepka, M.; Nachtigallová, D.; Lischka, H. Tuning the UV spectrum of PAHs by means of different N-doping types taking pyrene as paradigmatic example: Categorization: Via valence bond theory and high-level computational approaches. Phys. Chem. Chem. Phys. 2020, 22, 22003–22015. [Google Scholar] [CrossRef] [PubMed]
- Bardeen, C.J. The Structure and Dynamics of Molecular Excitons. Annu. Rev. Phys. Chem. 2014, 65, 127–148. [Google Scholar] [CrossRef] [PubMed]
- Mewes, S.A.; Dreuw, A. Density-based descriptors and exciton analyses for visualizing and understanding the electronic structure of excited states. Phys. Chem. Chem. Phys. 2019, 21, 2843–2856. [Google Scholar] [CrossRef]
- Darghouth, A.A.M.H.M.; Correa, G.C.; Juillard, S.; Casida, M.E.; Humeniuk, A.; Mitrić, R. Davydov-type excitonic effects on the absorption spectra of parallel-stacked and herringbone aggregates of pentacene: Time-dependent density-functional theory and time-dependent density-functional tight binding. J. Chem. Phys. 2018, 149, 134111. [Google Scholar] [CrossRef]
- Casanova, D. Theoretical investigations of the perylene electronic structure: Monomer, dimers, and excimers. Int. J. Quantum Chem. 2015, 115, 442–452. [Google Scholar] [CrossRef]
- Zubiria-Ulacia, M.; Matxain, J.M.; Casanova, D. The role of CT excitations in PDI aggregates. Phys. Chem. Chem. Phys. 2020, 22, 15908–15918. [Google Scholar] [CrossRef]
- Mao, Y.; Montoya-Castillo, A.; Markland, T.E. Accurate and efficient DFT-based diabatization for hole and electron transfer using absolutely localized molecular orbitals. J. Chem. Phys. 2019, 151, 164114. [Google Scholar] [CrossRef] [PubMed]
- Mao, Y.; Montoya-Castillo, A.; Markland, T.E. Excited state diabatization on the cheap using DFT: Photoinduced electron and hole transfer. J. Chem. Phys. 2020, 153, 244111. [Google Scholar] [CrossRef] [PubMed]
- Mewes, S.A.; Plasser, F.; Krylov, A.; Dreuw, A. Benchmarking Excited-State Calculations Using Exciton Properties. J. Chem. Theory Comput. 2018, 14, 710–725. [Google Scholar] [CrossRef]
- Jurinovich, S.; Cupellini, L.; Guido, C.A.; Mennucci, B. EXAT: EXcitonic analysis tool. J. Comput. Chem. 2018, 39, 279–286. [Google Scholar] [CrossRef] [PubMed]
- Walter, C.; Krämer, V.; Engels, B. On the applicability of time-dependent density functional theory (TDDFT) and semiempirical methods to the computation of excited-state potential energy surfaces of perylene-based dye-aggregates. Int. J. Quantum Chem. 2017, 117, e25337. [Google Scholar] [CrossRef]
- Accomasso, D.; Persico, M.; Granucci, G. Diabatization by Localization in the Framework of Configuration Interaction Based on Floating Occupation Molecular Orbitals (FOMO−CI). ChemPhotoChem 2019, 3, 933–944. [Google Scholar] [CrossRef]
- Tamura, H. Diabatization for Time-Dependent Density Functional Theory: Exciton Transfers and Related Conical Intersections. J. Phys. Chem. A 2016, 120, 9341–9347. [Google Scholar] [CrossRef]
- Liu, W.; Lunkenheimer, B.; Settels, V.; Engels, B.; Fink, R.F.; Köhn, A. A general ansatz for constructing quasi-diabatic states in electronically excited aggregated systems. J. Chem. Phys. 2015, 143, 084106. [Google Scholar] [CrossRef]
- Carreras, A.; Uranga-Barandiaran, O.; Castet, F.; Casanova, D. Photophysics of Molecular Aggregates from Excited State Diabatization. J. Chem. Theory Comput. 2019, 15, 2320–2330. [Google Scholar] [CrossRef]
- Shirai, S.; Iwata, S.; Tani, T.; Inagaki, S. Ab Initio Studies of Aromatic Excimers Using Multiconfiguration Quasi-Degenerate Perturbation Theory. J. Phys. Chem. A 2011, 115, 7687–7699. [Google Scholar] [CrossRef]
- Diaz-Andres, A.; Casanova, D. Benzene Excimer and Excited Multimers: Electronic Character, Interaction Nature, and Aromaticity. J. Phys. Chem. Lett. 2021, 12, 7400–7408. [Google Scholar] [CrossRef]
- Krishnan, A.; Diaz-Andres, A.; Sudhakaran, K.P.; John, A.T.; Hariharan, M.; Casanova, D. Deciphering the role of (anti)aromaticity in cofacial excimers of linear acenes. J. Phys. Org. Chem. 2022, 369, e4438. [Google Scholar] [CrossRef]
- East, A.L.L.; Lim, E.C. Naphthalene dimer: Electronic states, excimers, and triplet decay. J. Chem. Phys. 2000, 113, 8981–8994. [Google Scholar] [CrossRef]
- Platt, J.R. Classification of Spectra of Cata-Condensed Hydrocarbons. J. Chem. Phys. 1949, 17, 484–495. [Google Scholar] [CrossRef]
- Dai, Y.; Calzolari, A.; Zubiria-Ulacia, M.; Casanova, D.; Negri, F. Intermolecular Interactions and Charge Resonance Contributions to Triplet and Singlet Exciton States of Oligoacene Aggregates. Molecules 2023, 28, 119. [Google Scholar] [CrossRef] [PubMed]
- Becker, R.S.; Singh, I.S.; Jackson, E.A. Comprehensive spectroscopic investigation of polynuclear aromatic hydrocarbons. I. Absorption spectra and state assignments for the tetracyclic hydrocarbons and their alkyl-substituted derivatives. J. Chem. Phys. 1963, 38, 2144–2171. [Google Scholar] [CrossRef]
- Thulstrup, E.W.; Downing, J.W.; Michl, J. Excited singlet states of pyrene. Polarization directions and magnetic circular dichroism of azapyrenes. Chem. Phys. 1977, 23, 307–319. [Google Scholar] [CrossRef]
- Macrae, C.F.; Bruno, I.J.; Chisholm, J.A.; Edgington, P.R.; McCabe, P.; Pidcock, E.; Rodriguez-Monge, L.; Taylor, R.; van de Streek, J.; Wood, P.A. Mercury CSD 2.0—New features for the visualization and investigation of crystal structures. J. Appl. Crystallogr. 2008, 41, 466–470. [Google Scholar] [CrossRef]
- Camerman, A.; Trotter, J. The crystal and molecular structure of pyrene. Acta Crystallogr. 1965, 18, 636–643. [Google Scholar] [CrossRef]
- Birks, J.B. Excimers. Reports Prog. Phys. 1975, 38, 903–974. [Google Scholar] [CrossRef]
- Henrichsmeyer, J.; Thelen, M.; Bröckel, M.; Fadel, M.; Behnle, S.; Sekkal-Rahal, M.; Fink, R.F. Rationalizing Aggregate Structures with Orbital Contributions to the Exchange-Repulsion Energy. ChemPhysChem 2023, 24, e202300097. [Google Scholar] [CrossRef]
- Boys, S.F.; Bernardi, F. The calculation of small molecular interactions by the differences of separate total energies. Some procedures with reduced errors. Mol. Phys. 1970, 19, 553–566. [Google Scholar] [CrossRef]
- Valenti, G.; Fiorani, A.; Di Motta, S.; Bergamini, G.; Gingras, M.; Ceroni, P.; Negri, F.; Paolucci, F.; Marcaccio, M. Molecular Size and Electronic Structure Combined Effects on the Electrogenerated Chemiluminescence of Sulfurated Pyrene-Cored Dendrimers. Chem. Eur. J. 2015, 21, 2936–2947. [Google Scholar] [CrossRef] [PubMed]
- Bao, P.; Hettich, C.P.; Shi, Q.; Gao, J. Block-Localized Excitation for Excimer Complex and Diabatic Coupling. J. Chem. Theory Comput. 2021, 17, 240–254. [Google Scholar] [CrossRef] [PubMed]
- Shirai, S.; Kurashige, Y.; Yanai, T. Computational Evidence of Inversion of 1La and 1Lb-Derived Excited States in Naphthalene Excimer Formation from ab Initio Multireference Theory with Large Active Space: DMRG-CASPT2 Study. J. Chem. Theory Comput. 2016, 12, 2366–2372. [Google Scholar] [CrossRef] [PubMed]
- Liu, W.; Canola, S.; Köhn, A.; Engels, B.; Negri, F.; Fink, R.F. A model hamiltonian tuned toward high level ab initio calculations to describe the character of excitonic states in perylenebisimide aggregates. J. Comput. Chem. 2018, 39, 1979–1989. [Google Scholar] [CrossRef] [PubMed]
- Canola, S.; Bagnara, G.; Dai, Y.; Ricci, G.; Calzolari, A.; Negri, F. Addressing the Frenkel and charge transfer character of exciton states with a model Hamiltonian based on dimer calculations: Application to large aggregates of perylene bisimide. J. Chem. Phys. 2021, 154, 124101. [Google Scholar] [CrossRef] [PubMed]
- Dai, Y.; Zubiria-Ulacia, M.; Casanova, D.; Negri, F. Impact of Charge-Resonance Excitations on CT-Mediated J-Type Aggregation in Singlet and Triplet Exciton States of Perylene Di-Imide Aggregates: A TDDFT Investigation. Computation 2022, 10, 18. [Google Scholar] [CrossRef]
- Plasser, F.; Lischka, H. Analysis of Excitonic and Charge Transfer Interactions from Quantum Chemical Calculations. J. Chem. Theory Comput. 2012, 8, 2777–2789. [Google Scholar] [CrossRef]
- Casanova, D.; Krylov, A.I. Quantifying local exciton, charge resonance, and multiexciton character in correlated wave functions of multichromophoric systems. J. Chem. Phys. 2016, 144, 014102. [Google Scholar] [CrossRef]
- Andriessen, R.; Ameloot, M.; Boens, N.; De Schryver, F.C. Non a priori analysis of fluorescence decay surfaces of excited-state processes. 3. Intermolecular excimer formation of pyrene quenched by iodomethane. J. Phys. Chem. 1992, 96, 314–326. [Google Scholar] [CrossRef]
- Birks, J.B.; Dyson, D.J.; Munro, I.H. ‘Excimer’ fluorescence II. Lifetime studies of pyrene solutions. Proc. R. Soc. London. Ser. A. Math. Phys. Sci. 1963, 275, 575–588. [Google Scholar] [CrossRef]
- Kim, J.J.; Beardslee, R.A.; Phillips, D.T.; Offen, H.W. Fluorescence Lifetimes of Pyrene Monomer and Excimer at High Pressures. J. Chem. Phys. 1969, 51, 2761–2762. [Google Scholar] [CrossRef]
- Liu, W.; Settels, V.; Harbach, P.H.P.; Dreuw, A.; Fink, R.F.; Engels, B. Assessment of TD-DFT- and TD-HF-based approaches for the prediction of exciton coupling parameters, potential energy curves, and electronic characters of electronically excited aggregates. J. Comput. Chem. 2011, 32, 1971–1981. [Google Scholar] [CrossRef] [PubMed]
- Hirata, S.; Head-Gordon, M. Time-dependent density functional theory within the Tamm–Dancoff approximation. Chem. Phys. Lett. 1999, 314, 291–299. [Google Scholar] [CrossRef]
- Chai, J.-D.; Head-Gordon, M. Long-range corrected hybrid density functionals with damped atom–atom dispersion corrections. Phys. Chem. Chem. Phys. 2008, 10, 6615. [Google Scholar] [CrossRef] [PubMed]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16; Gaussian, Inc.: Wallingford CT, USA, 2016. [Google Scholar]
- Norton, J.E.; Brédas, J.-L. Theoretical characterization of titanyl phthalocyanine as a p-type organic semiconductor: Short intermolecular π-π interactions yield large electronic couplings and hole transport bandwidths. J. Chem. Phys. 2008, 128, 034701. [Google Scholar] [CrossRef]
- Kim, D. A Theoretical Analysis of the Excited State of Oligoacene Aggregates: Local Excitation vs. Charge-Transfer Transition. Bull. Korean Chem. Soc. 2015, 36, 2284–2289. [Google Scholar] [CrossRef]
- Nottoli, M.; Jurinovich, S.; Cupellini, L.; Gardiner, A.T.; Cogdell, R.; Mennucci, B. The role of charge-transfer states in the spectral tuning of antenna complexes of purple bacteria. Photosynth. Res. 2018, 137, 215–226. [Google Scholar] [CrossRef]
- Yamagata, H.; Pochas, C.M.; Spano, F.C. Designing J- and H-Aggregates through Wave Function Overlap Engineering: Applications to Poly(3-hexylthiophene). J. Phys. Chem. B 2012, 116, 14494–14503. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dai, Y.; Rambaldi, F.; Negri, F. Eclipsed and Twisted Excimers of Pyrene and 2-Azapyrene: How Nitrogen Substitution Impacts Excimer Emission. Molecules 2024, 29, 507. https://doi.org/10.3390/molecules29020507
Dai Y, Rambaldi F, Negri F. Eclipsed and Twisted Excimers of Pyrene and 2-Azapyrene: How Nitrogen Substitution Impacts Excimer Emission. Molecules. 2024; 29(2):507. https://doi.org/10.3390/molecules29020507
Chicago/Turabian StyleDai, Yasi, Filippo Rambaldi, and Fabrizia Negri. 2024. "Eclipsed and Twisted Excimers of Pyrene and 2-Azapyrene: How Nitrogen Substitution Impacts Excimer Emission" Molecules 29, no. 2: 507. https://doi.org/10.3390/molecules29020507
APA StyleDai, Y., Rambaldi, F., & Negri, F. (2024). Eclipsed and Twisted Excimers of Pyrene and 2-Azapyrene: How Nitrogen Substitution Impacts Excimer Emission. Molecules, 29(2), 507. https://doi.org/10.3390/molecules29020507