An Investigation into the Stability Source of Collagen Fiber Modified Using Cr(III): An Adsorption Isotherm Study
Abstract
1. Introduction
2. Results and Discussion
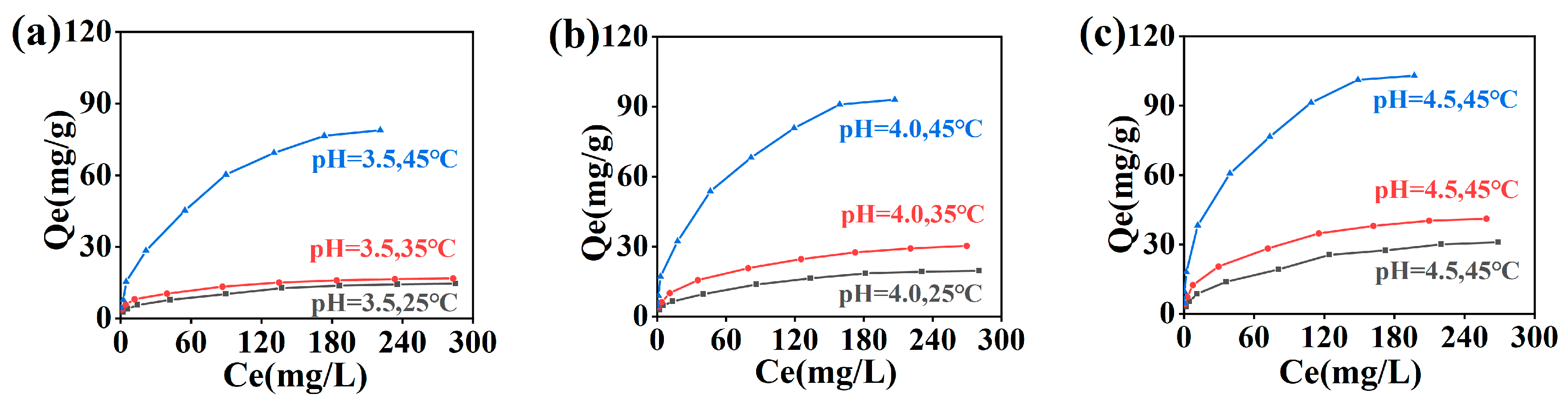
2.1. Adsorption Isotherm of Cr(III) onto Collagen Fibers
2.2. Adsorption Isotherm Fitting
2.3. Adsorption Thermodynamics
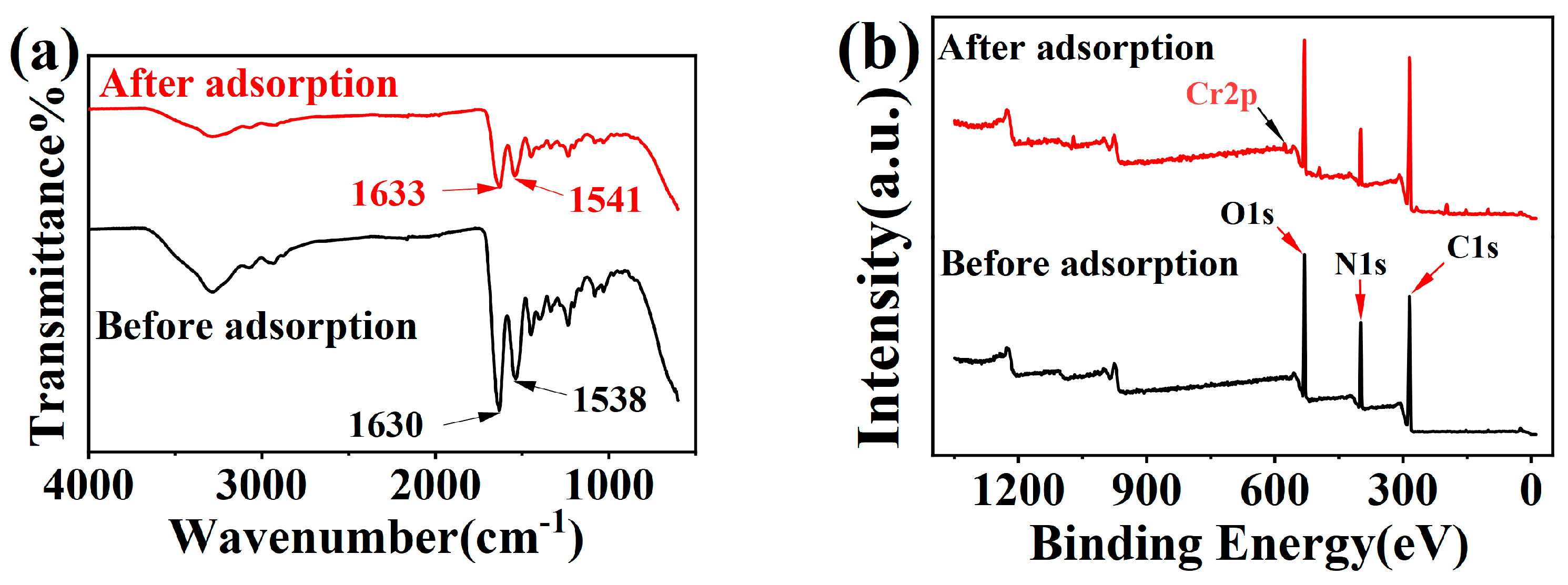
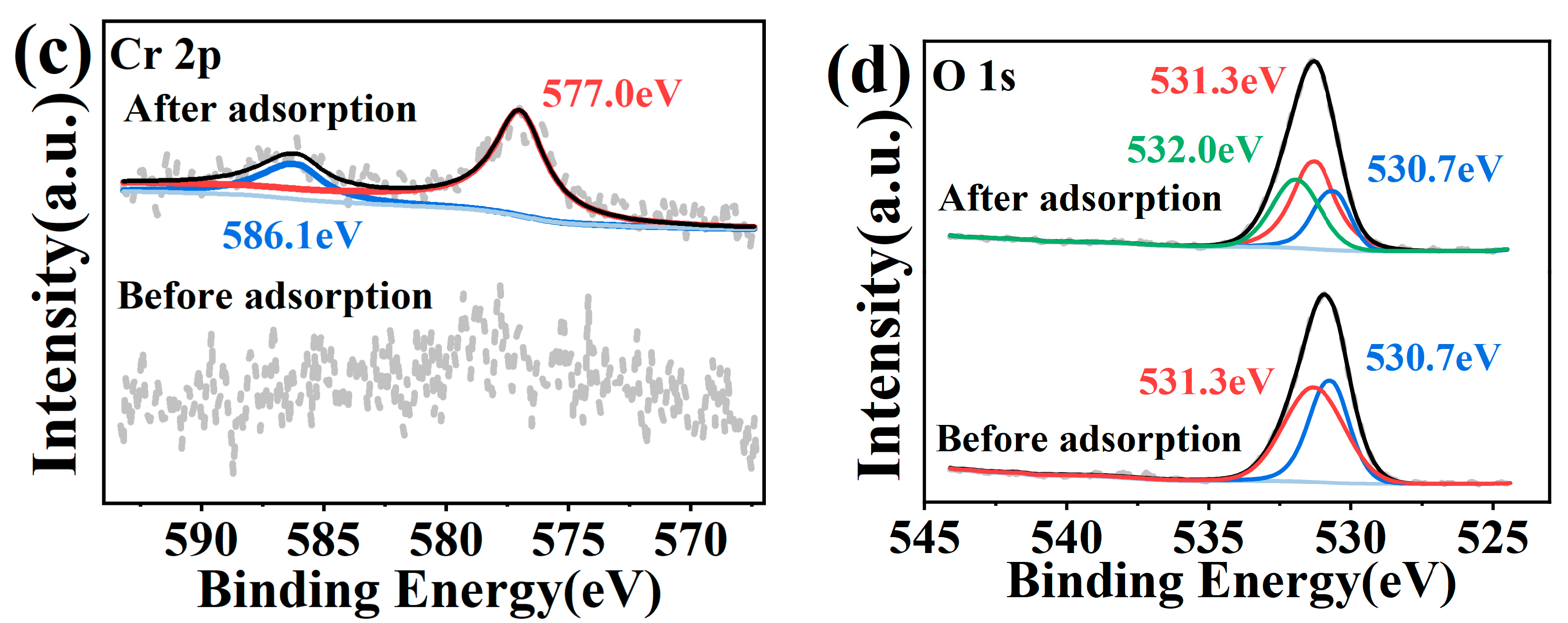
2.4. Material Characterization
3. Materials and Methods
3.1. Adsorption Isotherm of Cr(III) onto Collagen Fibers
3.2. Zeta Potential Measurement of Collagen Fibers
3.3. Characterization
3.4. Adsorption Isotherm Model
3.5. Statistical Parameters
3.6. Adsorption Thermodynamics
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Covington, A.D. Tanning Chemistry: The Science of Leather; RSC Publishing: Cambridge, UK, 2009. [Google Scholar]
- Long, W.; Peng, L.; Jiang, X.; He, F.; Zhang, W. Long-Term Releasing Kinetics of Chromium from Leather. J. Am. Leather Chem. Assoc. 2021, 116, 435–442. [Google Scholar] [CrossRef]
- Zhang, Y.; Mansel, B.W.; Rafea, N.; Cheong, S.; Yao, Y.; Holmes, G.; Chen, H.L.; Prabakar, S. Revealing molecular level indicators of collagen stability: Minimizing chrome usage in leather processing. ACS Sustain. Chem. Eng. 2018, 6, 7096–7104. [Google Scholar] [CrossRef]
- Brown, E.M.; Taylor, M.M. Essential chromium? J. Am. Leather Chem. Assoc. 2003, 98, 408–414. [Google Scholar]
- He, D.; Liu, J.; Chen, H.; Ding, K. Solid state nuclear magnetic resonance analysis of tanned leather powder with aluminum, silicon, and phosphorus tanning agents before and after hydrothermal denaturation. Leather Sci. Eng. 2021, 31, 6–10, 16. (In Chinese) [Google Scholar] [CrossRef]
- Covington, A.D.; Song, L.; Suparno, O.; Koon, H.E.C.; Collins, M. Link-lock: An explanation of the chemical stabilisation of collagen. J. Soc. Leather Technol. Chem. 2008, 92, 17. [Google Scholar]
- Liao, H.; Yu, J.; Zhu, W.; Kuang, M.; Duan, T.; Zhang, Y.; Lin, X.; Luo, X.; Zhou, J. Nano-zero-valent Fe/Ni particles loaded on collagen fibers immobilized by bayberry tannin as an effective reductant for uranyl in aqueous solutions. Appl. Surf. Sci. 2020, 507, 145075. [Google Scholar] [CrossRef]
- Xiao, H.; Wang, Y.; Hao, B.; Cao, Y.; Cui, Y.; Huang, X.; Shi, B. Collagen Fiber-Based Advanced Separation Materials: Recent Developments and Future Perspectives. Adv. Mater. 2022, 34, 2107891. [Google Scholar] [CrossRef]
- Mozaffari Majd, M.; Kordzadeh-Kermani, V.; Ghalandari, V.; Askari, A.; Sillanpää, M. Adsorption isotherm models: A comprehensive and systematic review (2010−2020). Sci. Total Environ. 2022, 812, 151334. [Google Scholar] [CrossRef]
- Tran, H.N.; Lima, E.C.; Juang, R.S.; Bollinger, J.C.; Chao, H.P. Thermodynamic parameters of liquid–phase adsorption process calculated from different equilibrium constants related to adsorption isotherms: A comparison study. J. Environ. Chem. Eng. 2021, 9, 106674. [Google Scholar] [CrossRef]
- Al-Ghouti, M.A.; Da’ana, D.A. Guidelines for the use and interpretation of adsorption isotherm models: A review. J. Hazard. Mater. 2020, 393, 122383. [Google Scholar] [CrossRef]
- Hu, Q.; Lan, R.; He, L.; Liu, H.; Pei, X. A critical review of adsorption isotherm models for aqueous contaminants: Curve characteristics, site energy distribution and common controversies. J. Environ. Manag. 2023, 329, 117104. [Google Scholar] [CrossRef] [PubMed]
- Ayawei, N.; Ebelegi, A.N.; Wankasi, D. Modelling and Interpretation of Adsorption Isotherms. J. Chem. 2017, 2017, 3039817. [Google Scholar] [CrossRef]
- Koble, R.A.; Corrigan, T.E. Adsorption isotherms for pure hydrocarbons. Ind. Eng. Chem. 1952, 44, 383–387. [Google Scholar] [CrossRef]
- Selmi, T.; Seffen, M.; Sammouda, H.; Mathieu, S.; Jagiello, J.; Celzard, A. Physical meaning of the parameters used in fractal kinetic and generalised adsorption models of Brouers–Sotolongo. Adsorption 2018, 24, 11–27. [Google Scholar] [CrossRef]
- Jin, Y.; Li, Y.; Du, Q.; Chen, B.; Chen, K.; Zhang, Y.; Wang, M.; Sun, Y.; Zhao, S.; Jing, Z.; et al. Efficient adsorption of Congo red by MIL-53(Fe)/chitosan composite hydrogel spheres. Microporous Mesoporous Mater. 2023, 348, 112404. [Google Scholar] [CrossRef]
- Nakhjiri, M.T.; Marandi, G.B.; Kurdtabar, M. Poly(AA-co-VPA) hydrogel cross-linked with N-maleyl chitosan as dye adsorbent: Isotherms, kinetics and thermodynamic investigation. Int. J. Biol. Macromol. 2018, 117, 152–166. [Google Scholar] [CrossRef]
- Lima, E.C.; Gomes, A.A.; Tran, H.N. Comparison of the nonlinear and linear forms of the van’t Hoff equation for calculation of adsorption thermodynamic parameters (∆S° and ∆H°). J. Mol. Liq. 2020, 311, 113315. [Google Scholar] [CrossRef]
- Atkins, P.W. Physical Chemistry, 8th ed.; Oxford University Press: Oxford, UK, 2006. [Google Scholar]
- Zhu, S.; Gu, Z.; Xiong, S.; An, Y.; Liu, Y.; Yin, T.; You, J.; Hu, Y. Fabrication of a novel bio-inspired collagen–polydopamine hydrogel and insights into the formation mechanism for biomedical applications. RSC Adv. 2016, 6, 66180–66190. [Google Scholar] [CrossRef]
- He, X.; Huang, Y.; Xiao, H.; Xu, X.; Wang, Y.; Huang, X.; Shi, B. Tanning agent free leather making enabled by the dispersity of collagen fibers combined with superhydrophobic coating. Green Chem. 2021, 23, 3581–3587. [Google Scholar] [CrossRef]
- Wang, Y.; Hu, L. Essential role of isoelectric point of skin/leather in leather processing. J. Leather Sci. Eng. 2022, 4, 25. [Google Scholar] [CrossRef]
- Wang, D.; He, S.; Shan, C.; Ye, Y.; Ma, H.; Zhang, X.; Zhang, W.; Pan, B. Chromium speciation in tannery effluent after alkaline precipitation: Isolation and characterization. J. Hazard. Mater. 2016, 316, 169–177. [Google Scholar] [CrossRef] [PubMed]
- Ma, H.; Wang, Q.; Hao, Y.; Zhu, C.; Chen, X.; Wang, C.; Yang, Y. Fenton reaction induced in-situ redox and re-complexation of polyphenol-Cr complex and their products. Chemosphere 2020, 250, 126214. [Google Scholar] [CrossRef] [PubMed]
- Bertagnolli, C.; Uhart, A.; Dupin, J.C.; da Silva, M.G.C.; Guibal, E.; Desbrieres, J. Biosorption of chromium by alginate extraction products from Sargassum filipendula: Investigation of adsorption mechanisms using X-ray photoelectron spectroscopy analysis. Bioresour. Technol. 2014, 164, 264–269. [Google Scholar] [CrossRef] [PubMed]
- Zou, L.; Shao, P.; Zhang, K.; Yang, L.; You, D.; Shi, H.; Pavlostathis, S.G.; Lai, W.; Liang, D.; Luo, X. Tannic acid-based adsorbent with superior selectivity for lead(II) capture: Adsorption site and selective mechanism. Chem. Eng. J. 2019, 364, 160–166. [Google Scholar] [CrossRef]
- Liao, X.; Zhang, M.; Shi, B. Collagen-ffber-immobilized tannins and their adsorption of Au(III). Ind. Eng. Chem. Res. 2004, 43, 2222. [Google Scholar] [CrossRef]
- Langmuir, I. The constitution and fundamental properties of solids and liquids. J. Frankl. Inst. 1917, 183, 102–105. [Google Scholar] [CrossRef]
- Freundlich, H. Over the adsorption in solution. J. Phys. Chem. 1906, 57, 1100–1107. [Google Scholar]
- Halsey, G. Physical adsorption on non-uniform surfaces. J. Chem. Phys. 1948, 16, 931–937. [Google Scholar] [CrossRef]
- Brouers, F.; Sotolongo, O.; Marquez, F.; Pirard, J.P. Microporous and heterogeneous surface adsorption isotherms arising from levy distributions. Phys. A 2005, 349, 271–282. [Google Scholar] [CrossRef]
- Fritz, W.; Schluender, E.U. Simultaneous adsorption equilibria of organic solutes in dilute aqueous solutions on activated carbon. Chem. Eng. Sci. 1974, 29, 1279–1282. [Google Scholar] [CrossRef]
- Jossens, L.; Prausnitz, J.M.; Fritz, W.; Schlünder, E.U.; Myers, A.L. Thermodynamics of multi-solute adsorption from dilute aqueous solutions. Chem. Eng. Sci. 1978, 33, 1097–1106. [Google Scholar] [CrossRef]
- Radke, C.; Prausnitz, J. Adsorption of organic solutes from dilute aqueous solution of activated carbon. Ind. Eng. Chem. Fundam. 1972, 11, 445–451. [Google Scholar] [CrossRef]
- Redlich, O.; Peterson, D.L. A useful adsorption isotherm. J. Phys. Chem. 1959, 63, 1024. [Google Scholar] [CrossRef]
- Sips, R. On the structure of a catalyst surface. J. Chem. Phys. 1948, 16, 490–495. [Google Scholar] [CrossRef]
- Vieth, W.R.; Sladek, K. A model for diffusion in a glassy polymer. J. Colloid Sci. 1965, 20, 1014–1033. [Google Scholar] [CrossRef]
- Wang, J.; Guo, X. Adsorption isotherm models: Classification, physical meaning, application and solving method. Chemosphere 2020, 258, 127279. [Google Scholar] [CrossRef]
- Li, J.; Peng, L.; Guo, L.; Zhang, W. Solid liquid adsorption isotherm model and thermodynamic parameter calculation. Leather Sci. Eng. 2023, 33, 36. (In Chinese) [Google Scholar] [CrossRef]

| Unit | pH | pH | pH | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 3.5 | 4.0 | 4.5 | ||||||||
| Temperature | Temperature | Temperature | ||||||||
| 25 °C | 35 °C | 45 °C | 25 °C | 35 °C | 45 °C | 25 °C | 35 °C | 45 °C | ||
| Qmax | mg/g | 14.63 | 16.88 | 78.88 | 19.65 | 30.34 | 93.04 | 31.00 | 41.17 | 103.02 |
| 1. Langmuir model | ||||||||||
| QL | mg/g | 15.5736 | 16.4671 | 97.7820 | 21.9845 | 32.3725 | 113.4898 | 36.3115 | 44.4526 | 115.9466 |
| KL | L/mg | 0.0334 | 0.0870 | 0.0186 | 0.0249 | 0.0313 | 0.0213 | 0.0187 | 0.0341 | 0.0341 |
| R2 | - | 0.9434 | 0.9156 | 0.9853 | 0.9634 | 0.9598 | 0.9792 | 0.9751 | 0.9687 | 0.9760 |
| Adj-R2 | - | 0.9353 | 0.9036 | 0.9832 | 0.9582 | 0.9541 | 0.9762 | 0.9716 | 0.9642 | 0.9726 |
| χ2 | - | 1.3949 | 2.3425 | 14.9666 | 1.8249 | 4.7002 | 29.6171 | 3.3698 | 7.5685 | 42.8851 |
| 2. Freundlich model | ||||||||||
| KF | 2.2787 | 4.1842 | 7.7367 | 2.5306 | 4.4278 | 10.4484 | 3.1041 | 6.2129 | 14.7199 | |
| nF | - | 0.3375 | 0.2542 | 0.4415 | 0.3736 | 0.3502 | 0.4206 | 0.4200 | 0.3504 | 0.3800 |
| R2 | - | 0.9910 | 0.9930 | 0.9906 | 0.9907 | 0.9964 | 0.9929 | 0.9913 | 0.9913 | 0.9916 |
| Adj-R2 | - | 0.9897 | 0.9920 | 0.9892 | 0.9894 | 0.9959 | 0.9919 | 0.9900 | 0.9900 | 0.9903 |
| χ2 | - | 0.2211 | 0.1948 | 9.6026 | 0.4618 | 0.4225 | 10.1384 | 1.1858 | 2.1138 | 15.1076 |
| 3. Halsey model | ||||||||||
| KHL | 0.0872 | 0.0036 | 0.0097 | 0.0835 | 0.0143 | 0.0038 | 0.0675 | 0.0055 | 0.0008 | |
| nHL | - | 2.9628 | 3.9337 | 2.2648 | 2.6760 | 2.8549 | 2.3772 | 2.3809 | 2.8533 | 2.6313 |
| R2 | - | 0.9910 | 0.9930 | 0.9906 | 0.9907 | 0.9964 | 0.9929 | 0.9913 | 0.9913 | 0.9916 |
| Adj-R2 | - | 0.9897 | 0.9920 | 0.9892 | 0.9894 | 0.9959 | 0.9919 | 0.9900 | 0.9900 | 0.9903 |
| χ2 | - | 0.2211 | 0.1948 | 9.6026 | 0.4618 | 0.4225 | 10.1384 | 1.1858 | 2.1138 | 15.1075 |
| 4. Brouers–Sotolongo model | ||||||||||
| QBS | mg/g | 25.3768 | 25.1269 | 108.2579 | 29.4429 | 52.4230 | 152.0294 | 46.7217 | 55.5578 | 145.9117 |
| KBS | 0.0779 | 0.1584 | 0.0485 | 0.0670 | 0.0736 | 0.0552 | 0.0494 | 0.0887 | 0.0833 | |
| αBS | - | 0.4328 | 0.3514 | 0.6193 | 0.5061 | 0.4444 | 0.5425 | 0.5593 | 0.4982 | 0.5184 |
| R2 | - | 0.9935 | 0.9971 | 0.9965 | 0.9952 | 0.9988 | 0.9959 | 0.9955 | 0.9978 | 0.9969 |
| Adj-R2 | - | 0.9914 | 0.9961 | 0.9953 | 0.9936 | 0.9984 | 0.9946 | 0.9940 | 0.9970 | 0.9959 |
| χ2 | - | 0.1862 | 0.0937 | 4.2077 | 0.2805 | 0.1671 | 6.7728 | 0.7145 | 0.6325 | 6.3839 |
| 5. Fritz–Shluender model | ||||||||||
| QFS | mg/g | −1.17 × 1045 | −4.04 × 1044 | 1.55 × 1045 | 2.04 × 1045 | −3.25 × 1044 | 3.35 × 1045 | −1.90 × 1045 | −7.61 × 1045 | 1.20 × 1045 |
| KFS | L/mg | 2.2747 | 4.1859 | 7.7597 | 2.5351 | 4.4209 | 10.4362 | 3.0912 | 6.1900 | 14.7328 |
| mFS | - | 0.6622 | 0.7459 | 0.5591 | 0.6267 | 0.6495 | 0.5792 | 0.5792 | 0.6489 | 0.6202 |
| R2 | - | 0.9910 | 0.9930 | 0.9906 | 0.9907 | 0.9964 | 0.9929 | 0.9913 | 0.9913 | 0.9916 |
| Adj-R2 | - | 0.9880 | 0.9906 | 0.9874 | 0.9877 | 0.9952 | 0.9905 | 0.9883 | 0.9883 | 0.9887 |
| χ2 | - | 0.2579 | 0.2273 | 11.2042 | 0.5388 | 0.4929 | 11.8282 | 1.3837 | 2.4666 | 17.6258 |
| 6. Jossens model | ||||||||||
| iJS | L/g | 5.3860 | 13.0283 | 6.8210 | 3.2684 | 12.6969 | 22.2457 | 2.9425 | 8.2542 | 26.5524 |
| jJS | L/mg | 2.0077 | 2.6862 | 0.4882 | 0.9403 | 2.4578 | 1.6347 | 0.5863 | 0.9268 | 1.2653 |
| mJS | - | 0.6911 | 0.7731 | 0.6617 | 0.6811 | 0.6772 | 0.6274 | 0.6618 | 0.7143 | 0.6874 |
| R2 | - | 0.9920 | 0.9958 | 0.9942 | 0.9931 | 0.9974 | 0.9946 | 0.9944 | 0.9954 | 0.9961 |
| Adj-R2 | - | 0.9894 | 0.9944 | 0.9923 | 0.9908 | 0.9965 | 0.9928 | 0.9925 | 0.9939 | 0.9948 |
| χ2 | - | 0.2293 | 0.1371 | 6.8754 | 0.4031 | 0.3591 | 8.9419 | 0.8864 | 1.2966 | 8.1405 |
| 7. Koble–Corrigan model | ||||||||||
| AKC | 2.0052 | 4.0776 | 5.3229 | 1.9977 | 3.8928 | 8.5429 | 2.3060 | 4.9782 | 12.3130 | |
| KKC | 0.0486 | 0.1105 | 0.0327 | 0.0440 | 0.0465 | 0.0347 | 0.0324 | 0.0620 | 0.0573 | |
| nKC | - | 0.4377 | 0.3665 | 0.6331 | 0.5163 | 0.4521 | 0.5468 | 0.5736 | 0.5188 | 0.5361 |
| R2 | - | 0.9933 | 0.9970 | 0.9960 | 0.9948 | 0.9987 | 0.9957 | 0.9953 | 0.9974 | 0.9968 |
| Adj-R2 | - | 0.9911 | 0.9959 | 0.9947 | 0.9931 | 0.9982 | 0.9943 | 0.9937 | 0.9966 | 0.9957 |
| χ2 | - | 0.1925 | 0.0989 | 4.7479 | 0.3025 | 0.1827 | 7.1497 | 0.7473 | 0.7259 | 6.7105 |
| 8. Radke–Prausnitz model | ||||||||||
| aRP | L/g | 1.21 × 1045 | −2.41 × 1045 | 1.29 × 1046 | −8.26 × 1044 | −1.28 × 1045 | 1.76 × 1046 | −1.98 × 1045 | 8.2416 | 4.56 × 1045 |
| bRP | 2.2771 | 4.1894 | 7.7306 | 2.5346 | 4.4219 | 10.4164 | 3.1114 | 8.9131 | 14.7149 | |
| mRP | - | 0.3376 | 0.2539 | 0.4416 | 0.3733 | 0.3505 | 0.4212 | 0.4195 | 0.2856 | 0.3800 |
| R2 | - | 0.9910 | 0.9930 | 0.9906 | 0.9907 | 0.9964 | 0.9929 | 0.9913 | 0.9954 | 0.9916 |
| Adj-R2 | - | 0.9880 | 0.9906 | 0.9874 | 0.9877 | 0.9952 | 0.9905 | 0.9883 | 0.9939 | 0.9887 |
| χ2 | - | 0.2579 | 0.2273 | 11.2031 | 0.5388 | 0.4929 | 11.8292 | 1.3835 | 1.2966 | 17.6255 |
| 9. Redlich–Peterson model | ||||||||||
| K(RP) | L/g | 5.3860 | 13.0283 | 6.8210 | 3.2684 | 12.6969 | 22.2457 | 2.9425 | 8.2542 | 26.5524 |
| a(RP) | 2.0077 | 2.6862 | 0.4882 | 0.9403 | 2.4578 | 1.6347 | 0.5863 | 0.9268 | 1.2653 | |
| n(RP) | - | 0.6911 | 0.7731 | 0.6617 | 0.6811 | 0.6772 | 0.6274 | 0.6618 | 0.7143 | 0.6874 |
| R2 | - | 0.9920 | 0.9958 | 0.9942 | 0.9931 | 0.9974 | 0.9946 | 0.9944 | 0.9954 | 0.9961 |
| Adj-R2 | - | 0.9894 | 0.9944 | 0.9923 | 0.9908 | 0.9965 | 0.9928 | 0.9925 | 0.9939 | 0.9948 |
| χ2 | - | 0.2293 | 0.1371 | 6.8754 | 0.4031 | 0.3591 | 8.9419 | 0.8864 | 1.2966 | 8.1405 |
| 10. Sips model | ||||||||||
| Qsips | mg/g | 41.2450 | 36.8928 | 162.5875 | 45.4424 | 83.6429 | 246.3362 | 71.2752 | 80.2749 | 214.9686 |
| Ksips | 0.0486 | 0.1105 | 0.0327 | 0.0440 | 0.0465 | 0.0347 | 0.0324 | 0.0620 | 0.0573 | |
| nsips | - | 0.4377 | 0.3665 | 0.6331 | 0.5163 | 0.4521 | 0.5468 | 0.5736 | 0.5188 | 0.5360 |
| R2 | - | 0.9933 | 0.9970 | 0.9960 | 0.9948 | 0.9987 | 0.9957 | 0.9953 | 0.9974 | 0.9968 |
| Adj-R2 | - | 0.9911 | 0.9959 | 0.9947 | 0.9931 | 0.9982 | 0.9943 | 0.9937 | 0.9966 | 0.9957 |
| χ2 | - | 0.1925 | 0.0989 | 4.7479 | 0.3025 | 0.1827 | 7.1497 | 0.7473 | 0.7259 | 6.7105 |
| 11. Vieth–Sladek model | ||||||||||
| QVS | mg/g | 9.2344 | 11.3718 | 58.1386 | 12.8445 | 18.9478 | 56.9334 | 19.5955 | 28.0660 | 66.2991 |
| KVS | L/g | 0.0228 | 0.0231 | 0.1309 | 0.0300 | 0.0490 | 0.2125 | 0.0513 | 0.0618 | 0.2266 |
| βVS | L/mg | 0.1154 | 0.2619 | 0.0456 | 0.0785 | 0.1093 | 0.0864 | 0.0609 | 0.1004 | 0.1261 |
| R2 | - | 0.9777 | 0.9743 | 0.9889 | 0.9821 | 0.9864 | 0.9854 | 0.9886 | 0.9863 | 0.9881 |
| Adj-R2 | - | 0.9703 | 0.9657 | 0.9852 | 0.9762 | 0.9819 | 0.9806 | 0.9848 | 0.9818 | 0.9841 |
| χ2 | - | 0.6405 | 0.8339 | 13.2171 | 1.0386 | 1.8516 | 24.2260 | 1.8071 | 3.8488 | 24.8572 |
| T (K) | pH | KModel | Van’t Hoff Equation | ∆G° | ∆H° | ∆S° | |
|---|---|---|---|---|---|---|---|
| (kJ·mol−1) | (kJ·mol−1) | (J·mol−1·K−1) | |||||
| 1. Langmuir model | |||||||
| 298 | 3.5 | 0.0334 | 1738.88 | y = 2619.09x − 0.92 R2 =0.12632 | −18.49 | −21.78 | −11.06 |
| 308 | 3.5 | 0.0870 | 4521.92 | −21.55 | −0.74 | ||
| 318 | 3.5 | 0.0186 | 968.24 | −18.18 | −11.33 | ||
| 298 | 4.0 | 0.0249 | 1295.32 | y = 727.10x + 4.83 R2 = 0.1618 | −17.76 | −6.05 | 39.28 |
| 308 | 4.0 | 0.0313 | 1625.00 | −18.93 | 41.82 | ||
| 318 | 4.0 | 0.0213 | 1107.08 | −18.53 | 39.25 | ||
| 298 | 4.5 | 0.0187 | 972.92 | y = −2872.68x + 16.61 R2 = 0.7661 | −17.05 | 23.88 | 137.34 |
| 308 | 4.5 | 0.0341 | 1774.24 | −19.16 | 139.73 | ||
| 318 | 4.5 | 0.0341 | 1775.28 | −19.78 | 137.30 | ||
| 2. Fritz–Shluender model | |||||||
| 298 | 3.5 | 2.2747 | 1.18 × 105 | y = −5825.44x + 31.22 R2 = 0.9995 | −28.94 | 48.43 | 259.63 |
| 308 | 3.5 | 4.1859 | 2.18 × 105 | −31.47 | 259.43 | ||
| 318 | 3.5 | 7.7597 | 4.04 × 105 | −34.13 | 259.61 | ||
| 298 | 4.0 | 2.5351 | 1.32 × 105 | y = −6663.68x + 34.10 R2 = 0.9814 | −29.21 | 55.40 | 283.92 |
| 308 | 4.0 | 4.4209 | 2.30 × 105 | −31.61 | 282.51 | ||
| 318 | 4.0 | 10.4362 | 5.43 × 105 | −34.91 | 283.99 | ||
| 298 | 4.5 | 3.0912 | 1.61 × 105 | y = −7379.79x + 36.72 R2 = 0.9928 | −29.70 | 61.36 | 305.57 |
| 308 | 4.5 | 6.1900 | 3.22 × 105 | −32.47 | 304.66 | ||
| 318 | 4.5 | 14.7328 | 7.66 × 105 | −35.82 | 305.60 | ||
| 3. Jossens model | |||||||
| 298 | 3.5 | 5.3860 | 2.80 × 105 | y = −1215.69x + 16.87 R2 = 0.0776 | −31.08 | 10.11 | 138.21 |
| 308 | 3.5 | 13.0283 | 6.77 × 105 | −34.38 | 144.45 | ||
| 318 | 3.5 | 6.8210 | 3.55 × 105 | −33.79 | 138.04 | ||
| 298 | 4.0 | 3.2684 | 1.70 × 105 | y = −9135.17x + 42.81 R2 = 0.9535 | −29.84 | 75.95 | 354.99 |
| 308 | 4.0 | 12.6969 | 6.60 × 105 | −34.31 | 358.00 | ||
| 318 | 4.0 | 22.2457 | 1.16 × 106 | −36.91 | 354.91 | ||
| 298 | 4.5 | 2.9425 | 1.53 × 105 | y = −10413.24x + 36.72 R2 = 0.9969 | −29.58 | 86.58 | 389.79 |
| 308 | 4.5 | 8.2542 | 4.29 × 105 | −33.21 | 388.93 | ||
| 318 | 4.5 | 26.5524 | 1.38 × 106 | −37.38 | 389.81 | ||
| 4. Redlich–Peterson model | |||||||
| 298 | 3.5 | 2.0077 | 1.43 × 105 | y = 9764.62x − 20.46 R2 = 0.6366 | −29.40 | −81.18 | −173.76 |
| 308 | 3.5 | 2.6862 | 1.87 × 105 | −31.08 | −162.66 | ||
| 318 | 3.5 | 0.4882 | 1.76 × 104 | −25.84 | −174.03 | ||
| 298 | 4.0 | 0.9403 | 4.75 × 104 | y = −4221.75x + 25.25 R2 = 0.38729 | −26.68 | 35.10 | 207.32 |
| 308 | 4.0 | 2.4578 | 1.96 × 105 | −31.21 | 215.29 | ||
| 318 | 4.0 | 1.6347 | 1.14 × 105 | −30.78 | 207.17 | ||
| 298 | 4.5 | 0.5863 | 2.32 × 104 | y = −5459.84x + 28.41 R2 = 0.9888 | −24.90 | 45.39 | 235.87 |
| 308 | 4.5 | 0.9268 | 4.67 × 104 | −27.53 | 236.75 | ||
| 318 | 4.5 | 1.2653 | 7.32 × 104 | −29.61 | 235.85 | ||
| 5. Sips model | |||||||
| 298 | 3.5 | 0.0486 | 51.97 | y = −7167.05x + 28.04 R2 = 0.9916 | −9.79 | 59.59 | 232.82 |
| 308 | 3.5 | 0.1105 | 127.63 | −12.42 | 233.80 | ||
| 318 | 3.5 | 0.0327 | 234.63 | −14.43 | 232.77 | ||
| 298 | 4.0 | 0.0440 | 122.42 | y = 544.37x + 2.76 R2 = 0.0205 | −11.91 | −4.53 | 24.77 |
| 308 | 4.0 | 0.0465 | 58.83 | −10.43 | 19.16 | ||
| 318 | 4.0 | 0.0347 | 111.15 | −12.45 | 24.91 | ||
| 298 | 4.5 | 0.0324 | 131.20 | y = −3062.14x + 15.25 R2 = 0.7889 | −12.08 | 25.46 | 125.97 |
| 308 | 4.5 | 0.0620 | 244.54 | −14.08 | 128.38 | ||
| 318 | 4.5 | 0.0573 | 250.62 | −14.60 | 125.97 | ||
| 6. Vieth–Sladek model | |||||||
| 298 | 3.5 | 0.1154 | 5998.20 | y = 4273.29x − 5.22 R2 = 0.2653 | −21.55 | −35.53 | −46.90 |
| 308 | 3.5 | 0.2619 | 1.36 × 104 | −24.38 | −36.22 | ||
| 318 | 3.5 | 0.0456 | 2369.64 | −20.54 | −47.13 | ||
| 298 | 4.0 | 0.0785 | 4084.08 | y = −503.38x + 10.09 R2 = 0.0924 | −20.60 | 4.19 | 83.19 |
| 308 | 4.0 | 0.1093 | 5684.64 | −22.14 | 85.48 | ||
| 318 | 4.0 | 0.0864 | 4490.72 | −22.23 | 83.09 | ||
| 298 | 4.5 | 0.0609 | 3166.80 | y = −3471.52x + 19.75 R2 = 0.9637 | −19.97 | 28.86 | 163.86 |
| 308 | 4.5 | 0.1004 | 5221.84 | −21.92 | 164.87 | ||
| 318 | 4.5 | 0.1261 | 6557.72 | −23.24 | 163.82 | ||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Long, W.; Peng, L.; Guo, L.; Zhang, W. An Investigation into the Stability Source of Collagen Fiber Modified Using Cr(III): An Adsorption Isotherm Study. Molecules 2024, 29, 300. https://doi.org/10.3390/molecules29020300
Li J, Long W, Peng L, Guo L, Zhang W. An Investigation into the Stability Source of Collagen Fiber Modified Using Cr(III): An Adsorption Isotherm Study. Molecules. 2024; 29(2):300. https://doi.org/10.3390/molecules29020300
Chicago/Turabian StyleLi, Jiheng, Wenjun Long, Liangqiong Peng, Lijun Guo, and Wenhua Zhang. 2024. "An Investigation into the Stability Source of Collagen Fiber Modified Using Cr(III): An Adsorption Isotherm Study" Molecules 29, no. 2: 300. https://doi.org/10.3390/molecules29020300
APA StyleLi, J., Long, W., Peng, L., Guo, L., & Zhang, W. (2024). An Investigation into the Stability Source of Collagen Fiber Modified Using Cr(III): An Adsorption Isotherm Study. Molecules, 29(2), 300. https://doi.org/10.3390/molecules29020300





