Caffeine—Legal Natural Stimulant with Open Research Perspective: Spectroscopic and Theoretical Characterization
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. NMR Measurements
2.3. Theoretical Methods
3. Results
3.1. 17O NMR Spectrum of Caffeine in CDCl3
3.2. Theoretical Nuclear Magnetic Shielding Parameters of Caffeine
3.3. Nuclear Magnetic Shieldings of Reference Molecules
3.4. 1H, 13C, 15N and 17O Chemical Shifts in Caffeine
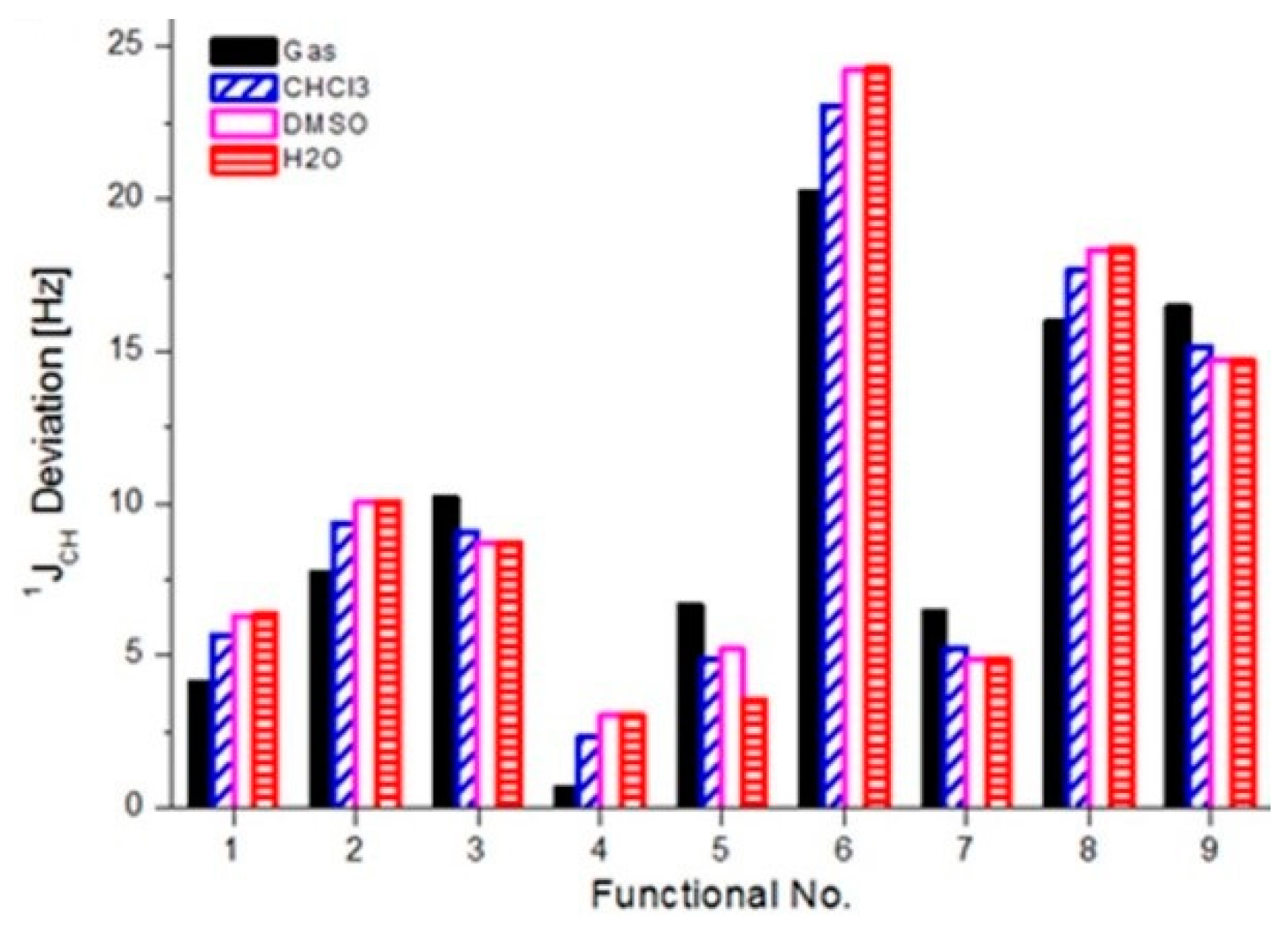
3.5. Indirect Spin–Spin Coupling Constants (SSCCs) of Caffeine in Vacuum and Solution
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Egawa, T.; Kamiya, A.; Takeuchi, H.; Konaka, S. Molecular structure of caffeine as determined by gas electron diffraction aided by theoretical calculations. J. Mol. Struct. 2006, 825, 151–157. [Google Scholar] [CrossRef]
- Fredholm, B.B.; Bättig, K.; Holmén, J.; Nehlig, A.; Zvartau, E.E. Actions of Caffeine in the Brain with Special Reference to Factors That Contribute to Its Widespread Use. Pharmacol. Rev. 1999, 51, 83–133. [Google Scholar] [PubMed]
- Bae, I.K.; Ham, H.M.; Jeong, M.H.; Kim, D.H.; Kim, H.J. Simultaneous determination of 15 phenolic compounds and caffeine in teas and mate using RP-HPLC/UV detection: Method development and optimization of extraction process. Food Chem. 2015, 172, 469–475. [Google Scholar] [CrossRef] [PubMed]
- Daily Rituals: How Artists Work; Knopf: New York, NY, USA, 2013; p. 278.
- Suzuki, J. A History of Mathematics, 1st ed.; Pearson: London, UK, 2001; p. 832. [Google Scholar]
- Hadad, G.M.; Abdel Salam, R.A.; Soliman, R.M.; Mesbah, M.K. Rapid and simultaneous determination of antioxidant markers and caffeine in commercial teas and dietary supplements by HPLC-DAD. Talanta 2012, 101, 38–44. [Google Scholar] [CrossRef]
- Rahim, A.A.; Nofrizal, S.; Saad, B. Rapid tea catechins and caffeine determination by HPLC using microwave-assisted extraction and silica monolithic column. Food Chem. 2014, 147, 262–268. [Google Scholar] [CrossRef]
- Fraser, K.; Harrison, S.J.; Lane, G.A.; Otter, D.E.; Hemar, Y.; Quek, S.-Y.; Rasmussen, S. HPLC–MS/MS profiling of proanthocyanidins in teas: A comparative study. J. Food Compos. Anal. 2012, 26, 43–51. [Google Scholar] [CrossRef]
- Wu, C.; Xu, H.; Héritier, J.; Andlauer, W. Determination of catechins and flavonol glycosides in Chinese tea varieties. Food Chem. 2012, 132, 144–149. [Google Scholar] [CrossRef]
- Shadkami, F.; Estevez, S.; Helleur, R. Analysis of catechins and condensed tannins by thermally assisted hydrolysis/methylation-GC/MS and by a novel two step methylation. J. Anal. Appl. Pyrol. 2009, 85, 54–65. [Google Scholar] [CrossRef]
- Castro, J.; Pregibon, T.; Chumanov, K.; Marcus, R. Determination of catechins and caffeine in proposed green tea standard reference materials by liquid chromatography-particle beam/electron ionization mass spectrometry (LC-PB/EIMS). Talanta 2010, 82, 1687–1695. [Google Scholar] [CrossRef]
- Chen, Q.; Zhao, J.; Chaitep, S.; Guo, Z. Simultaneous analysis of main catechins contents in green tea (Camellia sinensis (L.)) by Fourier transform near infrared reflectance (FT-NIR) spectroscopy. Food Chem. 2009, 113, 1272–1277. [Google Scholar] [CrossRef]
- Wang, Y.; Xian, J.; Xi, X.; Wei, X. Multi-fingerprint and quality control analysis of tea polysaccharides. Carbohydrate Polym. 2013, 92, 583–590. [Google Scholar] [CrossRef] [PubMed]
- Wahyuni, D.S.C.; Kristanti, M.W.; Putri, R.K.; Rinanto, Y. NMR Metabolic profiling of green tea (Camellia sinensis L.) leaves grown at Kemuning, Indonesia. J. Phys. Conf. Ser. 2017, 795, 012013. [Google Scholar] [CrossRef]
- Bartoszek, M.; Polak, J.; Chorążewski, M. Comparison of antioxidant capacities of different types of tea using the spectroscopy methods and semi-empirical mathematical model. Eur. Food Res. Technol. 2018, 244, 595–601. [Google Scholar] [CrossRef]
- Srivastava, S.K.; Singh, V.B. Ab initio and DFT studies of the structure and vibrational spectra of anhydrous caffeine. Spectrochim. Acta A Mol. Biomol. Spectrosc. 2013, 115, 45–50. [Google Scholar] [CrossRef]
- Tomasi, J.; Mennucci, B.; Cammi, R. Quantum mechanical continuum solvation models. Chem. Rev. 2005, 105, 2999–3093. [Google Scholar] [CrossRef]
- Miertus, S.; Scrocco, E.; Tomasi, J. Electrostatic Interaction of a Solute with a Continuum. A Direct Utilization of ab initio Molecular Potentials for the Prevision of Solvent Effects. Chem. Phys. 1981, 55, 117–129. [Google Scholar] [CrossRef]
- Gibson, C.M.; Fowler, P.W. Aromaticity of caffeine, xanthine and the dimethyl xanthines. Tetrahedron Lett. 2014, 55, 2078–2081. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Inhomogeneous Electron Gas. Phys. Rev. 1964, 136, B864–B871. [Google Scholar] [CrossRef]
- Kohn, W.; Sham, L.J. Self-Consistent Equations Including Exchange and Correlation Effects. Phys. Rev. 1965, 140, A1133–A1138. [Google Scholar] [CrossRef]
- Rijal, R.; Sah, M.; Lamichhane, H.P.; Mallik, H.S. Quantum chemical calculations of nicotine and caffeine molecule in gas phase and solvent using DFT methods. Heliyon 2022, 8, e12494. [Google Scholar] [CrossRef]
- Gómez, S.; Rojas-Valencia, N.; Restrepo, A. Analysis of Conformational Preferences in Caffeine. Molecules 2022, 27, 1937. [Google Scholar] [CrossRef] [PubMed]
- Liakos, D.G.; Sparta, M.; Kesharwani, M.K.; Martin, J.M.L.; Neese, F. Exploring the Accuracy Limits of Local Pair Natural Orbital Coupled-Cluster Theory. J. Chem. Theory Comput. 2015, 11, 1525–1539. [Google Scholar] [CrossRef] [PubMed]
- Sitkowski, J.; Stefaniak, L.; Nicol, L.; Martin, M.L.; Martin, G.J.; Webb, G.A. Complete assignments of the 1H, 13C and 15N NMR spectra of caffeine. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 1995, 51, 839–841. [Google Scholar] [CrossRef]
- Krivdin, L.B. 17O nuclear magnetic resonance: Recent advances and applications. Magn. Reson. Chem. 2023, 61, 507–529. [Google Scholar] [CrossRef]
- Boykin, D.W. 17O NMR Spectroscopy in Organic Chemistry; CRC: Boca Raton, FL, USA, 1991; p. 161. [Google Scholar]
- Gerothanassis, I.P. Oxygen-17 NMR spectroscopy: Basic principles and applications (Part I). Prog. Nucl. Magn. Reson. Spectrosc. 2010, 56, 95–197. [Google Scholar] [CrossRef]
- Gerothanassis, I.P. Oxygen-17 NMR spectroscopy: Basic principles and applications (part II). Prog. Nucl. Magn. Reson. Spectrosc. 2010, 57, 1–110. [Google Scholar] [CrossRef]
- Gerothanassis, J.P.; Lauterwein, J.; Sheppard, N. Natural-abundance 17O NMR of monosaccharides. J. Magn. Reson. 1982, 48, 431–446. [Google Scholar] [CrossRef]
- Gerothanassis, J.P.; Sheppard, N. Natural-abundance 17O NMR spectra of some inorganic and biologically important phosphates. J. Magn. Reson. 1982, 46, 423–439. [Google Scholar] [CrossRef]
- Gerothanassis, I.P.; Vacca, C.; Troganis, A. 17O NMR studies of the solvation state of cis/trans isomers of amides and model protected peptides. J. Magn. Reson. B 1996, 111, 220–229. [Google Scholar] [CrossRef]
- Theodorou, V.; Troganis, A.N.; Gerothanassis, I.P. On the detection of both carbonyl and hydroxyl oxygens in amino acid derivatives: A 17O NMR reinvestigation. Tetrahedron Lett. 2004, 45, 2243–2245. [Google Scholar] [CrossRef]
- Gerothanassis, I.P.; Vakka, C. 17O NMR Chemical Shifts as a Tool to Study Specific Hydration Sites of Amides and Peptides: Correlation with the IR Amide I Stretching Vibration. J. Org. Chem. 1994, 59, 2341–2348. [Google Scholar] [CrossRef]
- Ielo, L.; Pace, V.; Holzer, W.; Rahman, M.; Meng, G.; Szostak, R.; Szostak, M. Electrophilicity Scale of Activated Amides: 17O NMR and 15N NMR Chemical Shifts of Acyclic Twisted Amides in N−C(O) Cross-Coupling. Chem.—Eur. J. 2020, 26, 16246–16250. [Google Scholar] [CrossRef] [PubMed]
- Ruchmann, A.; Lauterwein, J.; Bäcker, T.; Klessinger, M. 17O NMR of L-Ascorbic Acid. New Spectroscopy of an Old Molecule. Magn. Reson. Chem. MRC 1996, 34, 116–122. [Google Scholar] [CrossRef]
- Schattenberg, C.J.; Kaupp, M. Extended Benchmark Set of Main-Group Nuclear Shielding Constants and NMR Chemical Shifts and Its Use to Evaluate Modern DFT Methods. J. Chem. Theory Comput. 2021, 17, 7602–7621. [Google Scholar] [CrossRef]
- Faber, R.; Buczek, A.; Kupka, T.; Sauer, S.P.A. On the convergence of zero-point vibrational corrections to nuclear shieldings and shielding anisotropies towards the complete basis set limit in water. Mol. Phys. 2017, 115, 144–160. [Google Scholar] [CrossRef]
- Aktaş, A.; Nassif, W.; Sayin, K. Investigations of structural, spectral (IR, 1H-, 9F-, 11B-, 13C-, 15N-, 17O-NMR) and anticancer properties of 5FU@B12N12 complexes. Chem. Pap. 2021, 75, 1727–1737. [Google Scholar] [CrossRef]
- Colherinhas, G.; Ludwig, V.; da Costa Ludwig, Z.M. GIAO-NMR spectroscopy of the xanthine’s structures in water solution using S-MC/QM methodology: An evaluation of the DFT-functionals’ efficiency. J. Mol. Liq. 2022, 347, 117955. [Google Scholar] [CrossRef]
- Ditchfield, R. Self-consistent perturbation theory of diamagnetism I. A gauge-invariant LCAO method for N.M.R. chemical shifts. Mol. Phys. 1974, 27, 789–807. [Google Scholar] [CrossRef]
- Wolinski, K.; Hinton, J.F.; Pulay, P. Efficient implementation of the gauge-independent atomic orbital method for NMR chemical shift calculations. J. Am. Chem. Soc. 1990, 112, 8251–8260. [Google Scholar] [CrossRef]
- Ulrich, E.L.; Akutsu, H.; Doreleijers, J.F.; Harano, Y.; Ioannidis, Y.E.; Lin, J.; Livny, M.; Mading, S.; Maziuk, D.; Miller, Z.; et al. BioMagResBank. Nucleic Acids Res. 2008, 36 (Suppl. 1), D402–D408. [Google Scholar] [CrossRef]
- Kupka, T.; Ruscic, B.; Botto, R.E. Toward Hartree-Fock- and Density Functional Complete Basis-Set Predicted NMR Parameters. J. Phys. Chem. A 2002, 106, 10396–10407. [Google Scholar] [CrossRef]
- Kupka, T.; Buczek, A.; Broda, M.A.; Mnich, A.; Kar, T. Performance of polarization-consistent vs. correlation-consistent basis sets for CCSD(T) prediction of water dimer interaction energy. J. Mol. Model. 2019, 25, 313. [Google Scholar] [CrossRef] [PubMed]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16 Rev. C.01; Gaussian Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Foresman, J.B.; Frisch, A. Exploring Chemistry with Electronic Structure Methods, 2nd ed.; Gaussian Inc.: Pittsburg, PA, USA, 1996. [Google Scholar]
- Becke, A.D. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A 1988, 38, 3098–3100. [Google Scholar] [CrossRef] [PubMed]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti Correlation-Energy Formula into a Functional of the Electron Density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef]
- Miehlich, B.; Savin, A.; Stoll, H.; Preuss, H. Results obtained with the correlation-energy density functionals of Becke and Lee, Yang and Parr. Chem. Phys. Lett. 1989, 157, 200–206. [Google Scholar] [CrossRef]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Yanai, T.; Tew, D.P.; Handy, N.C. A new hybrid exchange–correlation functional using the Coulomb-attenuating method (CAM-B3LYP). Chem. Phys. Lett. 2004, 393, 51–57. [Google Scholar] [CrossRef]
- Iikura, H.; Tsuneda, T.; Yanai, T.; Hirao, K. A long-range correction scheme for generalized-gradient-approximation exchange functionals. J. Chem. Phys. 2001, 115, 3540–3544. [Google Scholar] [CrossRef]
- Zhao, V.; Truhlar, D.G. The M06 Suite of Density Functionals for Main Group Thermochemistry, Thermochemical Kinetics, Noncovalent interactions, Excited States, and Transition Elements: Two New Functionals and Systematic Testing of Four M06 Functionals and Twelve Other Functionals. Theor. Chem. Acc. 2008, 120, 215–241. [Google Scholar] [CrossRef]
- Adamo, C.; Barone, V. Toward reliable density functional methods without adjustable parameters: The PBE0 model. J. Chem. Phys. 1999, 110, 6158–6170. [Google Scholar] [CrossRef]
- Tao, J.; Perdew, J.P.; Staroverov, V.N.; Scuseria, G.E. Climbing the Density Functional Ladder: Nonempirical Meta–Generalized Gradient Approximation Designed for Molecules and Solids. Phys. Rev. Lett. 2003, 91, 146401. [Google Scholar] [CrossRef] [PubMed]
- Chai, J.-D.; Head-Gordon, M. Long-range corrected hybrid density functionals with damped atom-atom dispersion corrections. Phys. Chem. Chem. Phys. 2008, 10, 6615–6620. [Google Scholar] [CrossRef] [PubMed]
- Barone, V.; Cossi, M.; Tomasi, J. Geometry optimization of molecular structures in solution by the polarizable continuum model. J. Comp. Chem. 1998, 19, 404–417. [Google Scholar] [CrossRef]
- Semenov, V.A.; Krivdin, L.B. Computational NMR of natural products. Russ. Chem. Rev. 2022, 91, RCR5027. [Google Scholar] [CrossRef]
- Rzepiela, K.; Buczek, A.; Kupka, T.; Broda, M.A. Factors Governing the Chemical Stability and NMR Parameters of Uracil Tautomers and Its 5-Halogen Derivatives. Molecules 2020, 25, 3931. [Google Scholar] [CrossRef]
- Kupka, T.; Mnich, A.; Broda, M.A. Performance of revised STO(1M)-3G basis set for prediction of 5-fluorocytosine chemical shifts. Magn. Reson. Chem. 2019, 57, 489–498. [Google Scholar] [CrossRef]
- Makulski, W.; Wilczek, M.; Jackowski, K. 17O and 1H NMR spectral parameters in isolated water molecules. Phys. Chem. Chem. Phys. 2018, 20, 22468–22476. [Google Scholar] [CrossRef]
- Hansen, P.E.; Saeed, B.A.; Rutu, R.S.; Kupka, T. One-bond 1J(15N,H) coupling constants at sp2-hybridized nitrogen of Schiff bases, enaminones and similar compounds: A theoretical study. Magn. Reson. Chem. 2020, 58, 750–762. [Google Scholar] [CrossRef]
- Kupka, T.; Nieradka, M.; Stachow, M.; Pluta, T.; Nowak, P.; Kjaer, H.; Kongsted, J.; Kaminsky, J. Basis Set Convergence of Indirect Spin-Spin Coupling Constants in the Kohn-Sham Limit for Several Small Molecules. J. Phys. Chem. A 2012, 116, 3728–3738. [Google Scholar] [CrossRef]
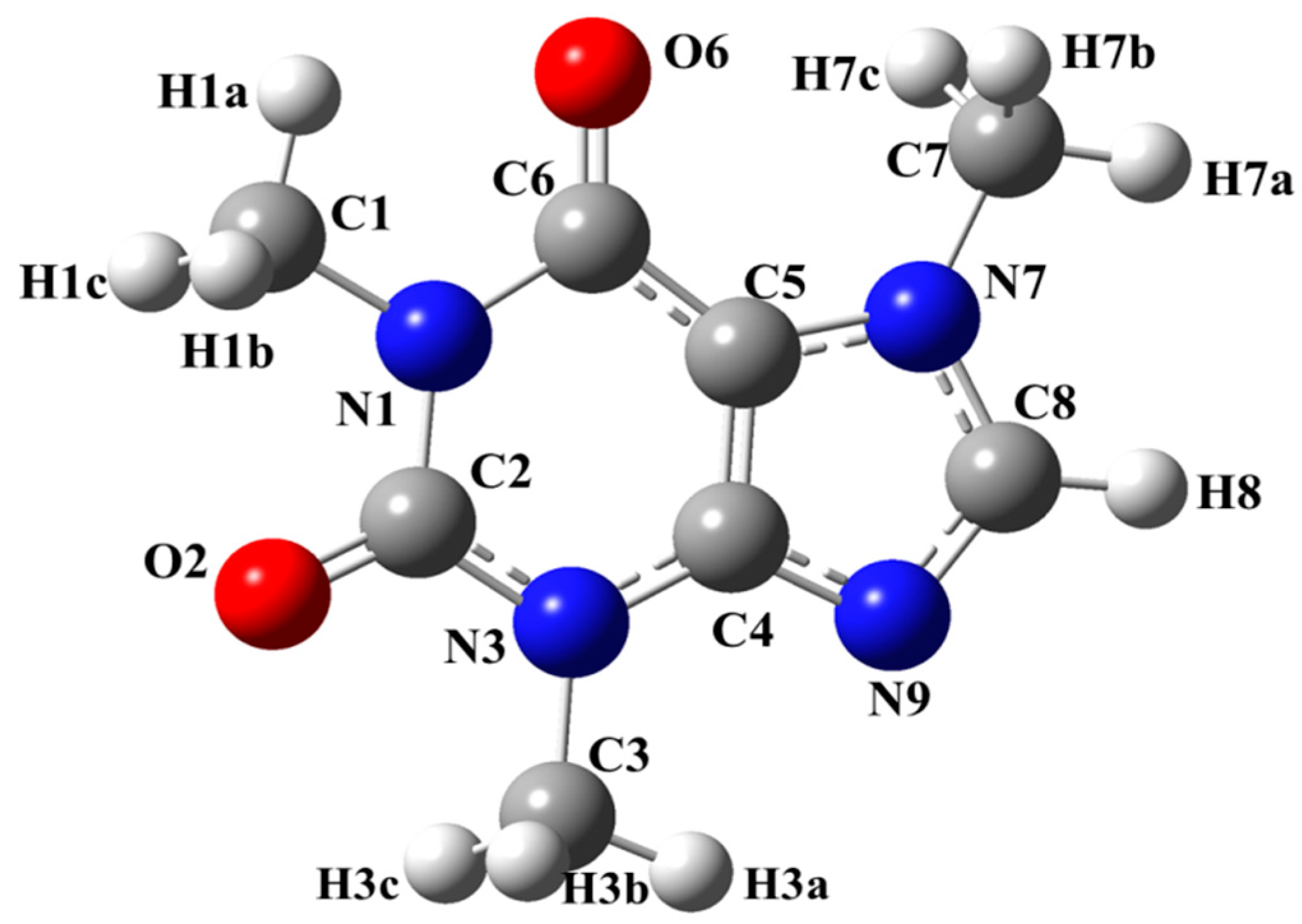
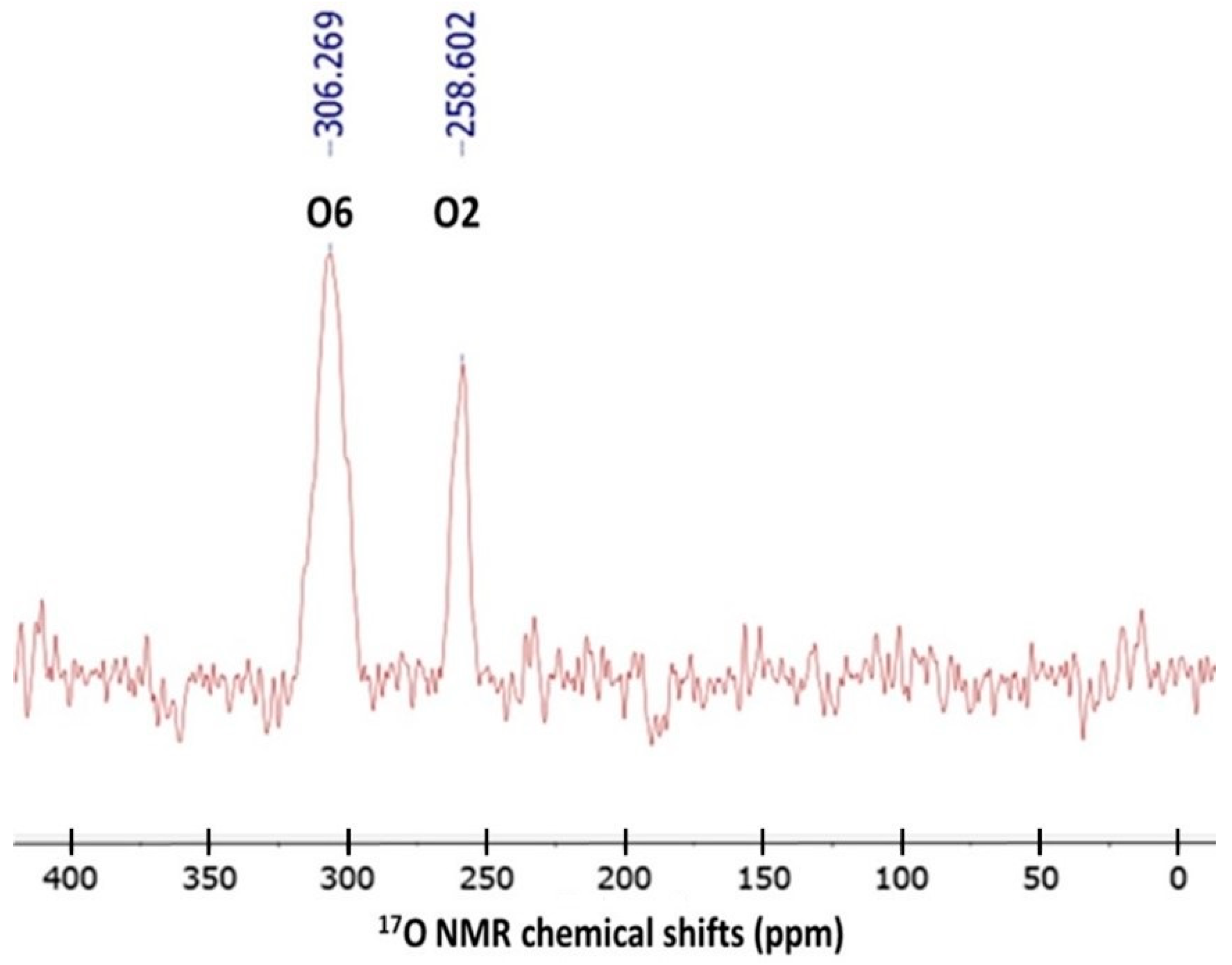


| Atom | B3LYP | BLYP | BP86 | CAM-B3LYP | LC-BLYP | M06 | PBE0 | TPSSh | wB97X | Exp. a |
|---|---|---|---|---|---|---|---|---|---|---|
| Vacuum | ||||||||||
| C1 | 1.080 | 1.692 | 0.811 | 0.346 | −0.605 | 0.334 | 0.581 | 1.804 | 0.295 | 27.5 |
| C2 | 6.253 | 4.532 | 1.563 | 9.001 | 13.998 | 6.524 | 5.334 | 1.716 | 7.398 | 151.3 |
| C3 | 1.627 | 2.548 | 1.696 | 0.807 | −0.346 | 3.116 | 1.445 | 2.351 | 0.683 | 29.3 |
| C4 | 8.287 | 6.597 | 3.678 | 10.365 | 14.488 | 10.207 | 7.109 | 2.623 | 8.213 | 148.3 |
| C5 | 7.573 | 8.659 | 6.354 | 7.168 | 8.305 | 6.351 | 6.285 | 3.425 | 5.000 | 107.1 |
| C6 | 6.281 | 4.020 | 1.092 | 9.857 | 15.942 | 5.605 | 5.693 | 1.063 | 8.666 | 154.9 |
| C7 | 3.510 | 4.627 | 3.802 | 2.606 | 1.201 | 3.683 | 3.045 | 4.100 | 2.276 | 33.2 |
| C8 | 3.713 | 1.160 | −0.899 | 6.479 | 11.815 | 7.554 | 3.344 | −1.436 | 4.948 | 141.2 |
| Chloroform | ||||||||||
| C1 | 1.530 | 2.270 | 1.322 | 0.913 | −10.130 | 2.070 | 0.921 | 2.206 | 0.608 | 27.5 |
| C2 | 7.818 | 6.102 | 2.987 | 10.780 | 2.853 | 8.672 | 6.776 | 3.083 | 8.939 | 151.3 |
| C3 | 2.249 | 3.340 | 2.394 | 1.511 | −10.136 | 3.647 | 1.952 | 2.904 | 1.127 | 29.3 |
| C4 | 8.903 | 7.305 | 4.320 | 11.117 | −0.817 | 11.548 | 7.618 | 3.166 | 8.710 | 148.3 |
| C5 | 8.276 | 9.601 | 7.222 | 7.898 | −5.067 | 6.046 | 6.792 | 4.046 | 5.394 | 107.1 |
| C6 | 7.570 | 5.320 | 2.247 | 11.370 | 3.379 | 8.788 | 6.842 | 2.125 | 9.883 | 154.9 |
| C7 | 4.213 | 5.523 | 4.607 | 3.384 | −8.568 | 4.819 | 3.631 | 4.841 | 2.837 | 33.2 |
| C8 | 6.613 | 4.141 | 2.042 | 9.510 | 0.988 | 11.309 | 6.146 | 1.336 | 7.701 | 141.2 |
| Atom | B3LYP | BLYP | BP86 | CAM-B3LYP | LC-BLYP | M06 | PBE0 | TPSSh | wB97X | Exp. a |
|---|---|---|---|---|---|---|---|---|---|---|
| Vacuum | ||||||||||
| H1 | 0.034 | 0.032 | 0.016 | 0.060 | 0.074 | −0.027 | −0.712 | 0.032 | 0.008 | 3.37 |
| H3 | 0.012 | 0.018 | 0.001 | 0.026 | 0.026 | 0.034 | −0.018 | 0.002 | −0.018 | 3.55 |
| H7 | −0.114 | −0.128 | −0.146 | −0.100 | −0.098 | −0.103 | −0.138 | −0.144 | −0.142 | 4.01 |
| H8 | −0.272 | −0.388 | −0.428 | −0.200 | −0.116 | −0.125 | −0.229 | −0.305 | −0.213 | 7.58 |
| Chloroform | ||||||||||
| H1 | 0.037 | 0.046 | 0.027 | 0.058 | −0.093 | −0.021 | −0.001 | 0.030 | 0.004 | 3.37 |
| H3 | 0.030 | 0.048 | 0.028 | 0.039 | −0.143 | −0.008 | −0.001 | 0.017 | −0.009 | 3.55 |
| H7 | −0.068 | −0.075 | −0.096 | −0.055 | −0.209 | −0.058 | −0.092 | −0.101 | −0.102 | 4.01 |
| H8 | −0.031 | −0.146 | −0.181 | 0.044 | −0.033 | 0.103 | 0.016 | −0.063 | 0.030 | 7.58 |
| Atom | B3LYP | BLYP | BP86 | CAM-B3LYP | LC-BLYP | M06 | PBE0 | TPSSh | wB97X | Exp. a |
|---|---|---|---|---|---|---|---|---|---|---|
| Vacuum | ||||||||||
| N1 | −1.509 | 20.745 | 27.929 | −12.802 | −24.166 | −11.534 | 6.479 | 12.379 | −9.465 | −231.3 |
| N3 | −3.515 | 18.243 | 26.339 | −15.655 | −27.967 | −13.999 | 4.612 | 12.752 | −11.581 | −268.4 |
| N7 | −4.194 | 11.793 | 19.855 | −12.064 | −19.942 | −9.574 | 4.264 | 8.604 | −7.714 | −227.2 |
| N9 | 0.372 | 14.039 | 20.120 | −7.034 | −13.512 | −0.371 | 7.832 | 7.450 | −5.791 | −151.2 |
| Chloroform | ||||||||||
| N1 | −9.994 | 11.984 | 19.668 | −21.070 | −44.037 | −20.328 | −1.438 | 4.479 | −17.412 | −231.3 |
| N3 | −10.168 | 11.338 | 19.897 | −22.139 | −44.485 | −20.723 | −1.531 | 6.626 | −17.865 | −268.4 |
| N7 | −9.950 | 6.074 | 14.688 | −17.768 | −39.357 | −15.345 | −0.915 | 3.471 | −13.224 | −227.2 |
| N9 | −14.033 | −0.196 | 6.729 | −21.667 | −45.876 | −15.719 | −5.836 | −5.758 | −20.195 | −151.2 |
| Atom | B3LYP | BLYP | BP86 | CAM-B3LYP | LC-BLYP | M06 | PBE0 | TPSSh | wB97X | Exp. a |
|---|---|---|---|---|---|---|---|---|---|---|
| Vacuum | ||||||||||
| O2 | 37.302 | 41.158 | 37.013 | 33.858 | 31.265 | 38.317 | 35.085 | 22.308 | 27.429 | 258.602 |
| O6 | 16.501 | 21.502 | 15.523 | 13.015 | 11.691 | 8.316 | 16.318 | 1.443 | 7.288 | 306.269 |
| Chloroform | ||||||||||
| O2 | 23.767 | 28.623 | 25.079 | 19.778 | −4.859 | 23.993 | 22.112 | 10.400 | 13.724 | 258.602 |
| O6 | 4.838 | 10.454 | 5.225 | 1.438 | −21.545 | 6.837 | 5.040 | −8.855 | −4.287 | 306.269 |
| Label | Solvent | Vacuum | Chloroform | DMSO | Water |
|---|---|---|---|---|---|
| Functional | 13C | ||||
| 1 | B3LYP | 5.414 | 6.472 | 6.783 | 6.951 |
| 2 | BLYP | 4.839 | 5.863 | 6.298 | 6.485 |
| 3 | BP86 | 3.089 | 3.835 | 4.219 | 4.273 |
| 4 | CAM-B3LYP | 6.937 | 8.153 | 8.591 | 8.633 |
| 5 | LC-BLYP | 10.431 | 6.380 | 12.241 | 5.643 |
| 6 | M06 | 6.113 | 7.835 | 6.229 | 7.798 |
| 7 | PBE0 | 4.661 | 5.618 | 6.224 | 6.260 |
| 8 | TPSSh | 2.508 | 3.141 | 3.504 | 3.514 |
| 9 | wB97X | 5.618 | 6.629 | 7.282 | 7.314 |
| 1H | |||||
| 1 | B3LYP | 0.149 | 0.044 | 0.049 | 0.049 |
| 2 | BLYP | 0.205 | 0.089 | 0.052 | 0.050 |
| 3 | BP86 | 0.226 | 0.104 | 0.059 | 0.060 |
| 4 | CAM-B3LYP | 0.117 | 0.050 | 0.083 | 0.084 |
| 5 | LC-BLYP | 0.085 | 0.136 | 0.128 | 0.285 |
| 6 | M06 | 0.084 | 0.060 | 0.119 | 0.115 |
| 7 | PBE0 | 0.381 | 0.047 | 0.070 | 0.072 |
| 8 | TPSSh | 0.170 | 0.062 | 0.049 | 0.050 |
| 9 | wB97X | 0.128 | 0.053 | 0.079 | 0.080 |
| 15N | |||||
| 1 | B3LYP | 2.844 | 11.171 | 14.716 | 14.896 |
| 2 | BLYP | 16.578 | 8.791 | 7.038 | 6.989 |
| 3 | BP86 | 23.837 | 16.153 | 13.603 | 13.477 |
| 4 | CAM-B3LYP | 12.288 | 20.732 | 24.126 | 24.296 |
| 5 | LC-BLYP | 22.059 | 43.508 | 33.519 | 47.007 |
| 6 | M06 | 10.257 | 18.202 | 21.817 | 21.997 |
| 7 | PBE0 | 5.974 | 3.135 | 6.434 | 6.604 |
| 8 | TPSSh | 10.552 | 5.224 | 5.958 | 6.043 |
| 9 | wB97X | 8.898 | 17.357 | 20.737 | 20.910 |
| 17O | |||||
| 1 | B3LYP | 28.842 | 17.150 | 12.361 | 12.129 |
| 2 | BLYP | 32.835 | 21.547 | 16.381 | 16.105 |
| 3 | BP86 | 28.381 | 18.114 | 13.725 | 13.503 |
| 4 | CAM-B3LYP | 25.649 | 14.022 | 9.844 | 9.675 |
| 5 | LC-BLYP | 23.603 | 15.617 | 7.785 | 20.971 |
| 6 | M06 | 27.616 | 18.756 | 15.124 | 15.564 |
| 7 | PBE0 | 24.921 | 16.228 | 12.572 | 12.385 |
| 8 | TPSSh | 15.807 | 9.658 | 10.394 | 10.512 |
| 9 | wB97X | 20.068 | 10.166 | 8.627 | 8.646 |
| Functional | Vacuum | Chloroform | DMSO | Water |
|---|---|---|---|---|
| B3LYP | 4.096 | 5.667 | 6.323 | 6.357 |
| BLYP | 7.727 | 9.364 | 10.043 | 10.078 |
| BP86 | 10.178 | 9.084 | 8.724 | 8.708 |
| CAM-B3LYP | 0.658 | 2.305 | 3.012 | 3.048 |
| LC-BLYP | 6.637 | 4.904 | 5.206 | 3.571 |
| M06 | 20.233 | 23.050 | 24.232 | 24.290 |
| PBE0 | 6.421 | 5.239 | 4.882 | 4.867 |
| TPSSh | 15.948 | 17.640 | 18.334 | 18.369 |
| wB97X | 16.443 | 15.164 | 14.696 | 14.675 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kupka, T.; Makieieva, N.; Jewgiński, M.; Witek, M.; Blicharska, B.; Rahmonov, O.; Doležal, K.; Pospíšil, T. Caffeine—Legal Natural Stimulant with Open Research Perspective: Spectroscopic and Theoretical Characterization. Molecules 2024, 29, 4382. https://doi.org/10.3390/molecules29184382
Kupka T, Makieieva N, Jewgiński M, Witek M, Blicharska B, Rahmonov O, Doležal K, Pospíšil T. Caffeine—Legal Natural Stimulant with Open Research Perspective: Spectroscopic and Theoretical Characterization. Molecules. 2024; 29(18):4382. https://doi.org/10.3390/molecules29184382
Chicago/Turabian StyleKupka, Teobald, Natalina Makieieva, Michał Jewgiński, Magdalena Witek, Barbara Blicharska, Oimahmad Rahmonov, Karel Doležal, and Tomáš Pospíšil. 2024. "Caffeine—Legal Natural Stimulant with Open Research Perspective: Spectroscopic and Theoretical Characterization" Molecules 29, no. 18: 4382. https://doi.org/10.3390/molecules29184382
APA StyleKupka, T., Makieieva, N., Jewgiński, M., Witek, M., Blicharska, B., Rahmonov, O., Doležal, K., & Pospíšil, T. (2024). Caffeine—Legal Natural Stimulant with Open Research Perspective: Spectroscopic and Theoretical Characterization. Molecules, 29(18), 4382. https://doi.org/10.3390/molecules29184382









