Thermoelectric Properties Regulated by Quantum Size Effects in Quasi-One-Dimensional γ-Graphdiyne Nanoribbons
Abstract
1. Introduction
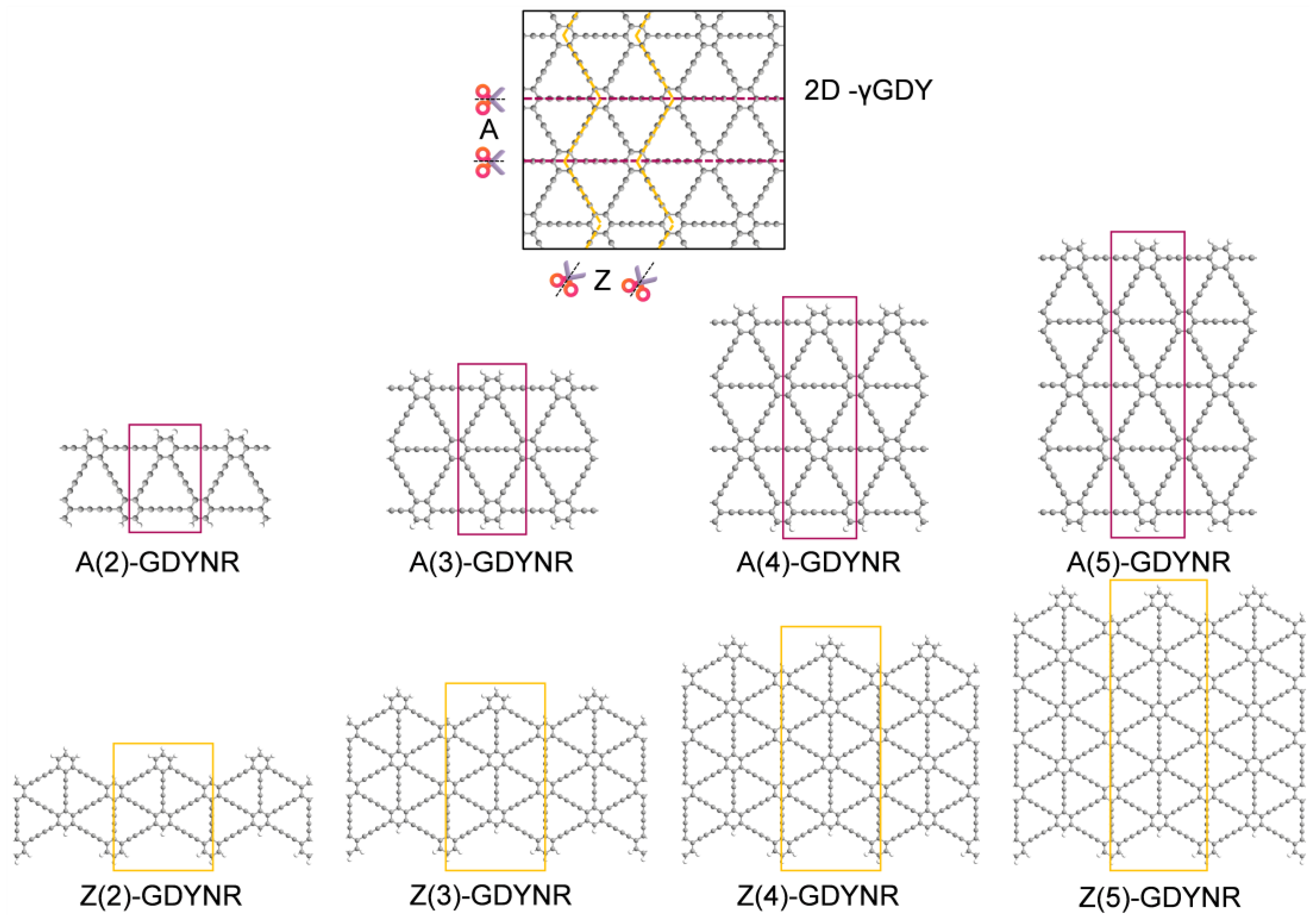
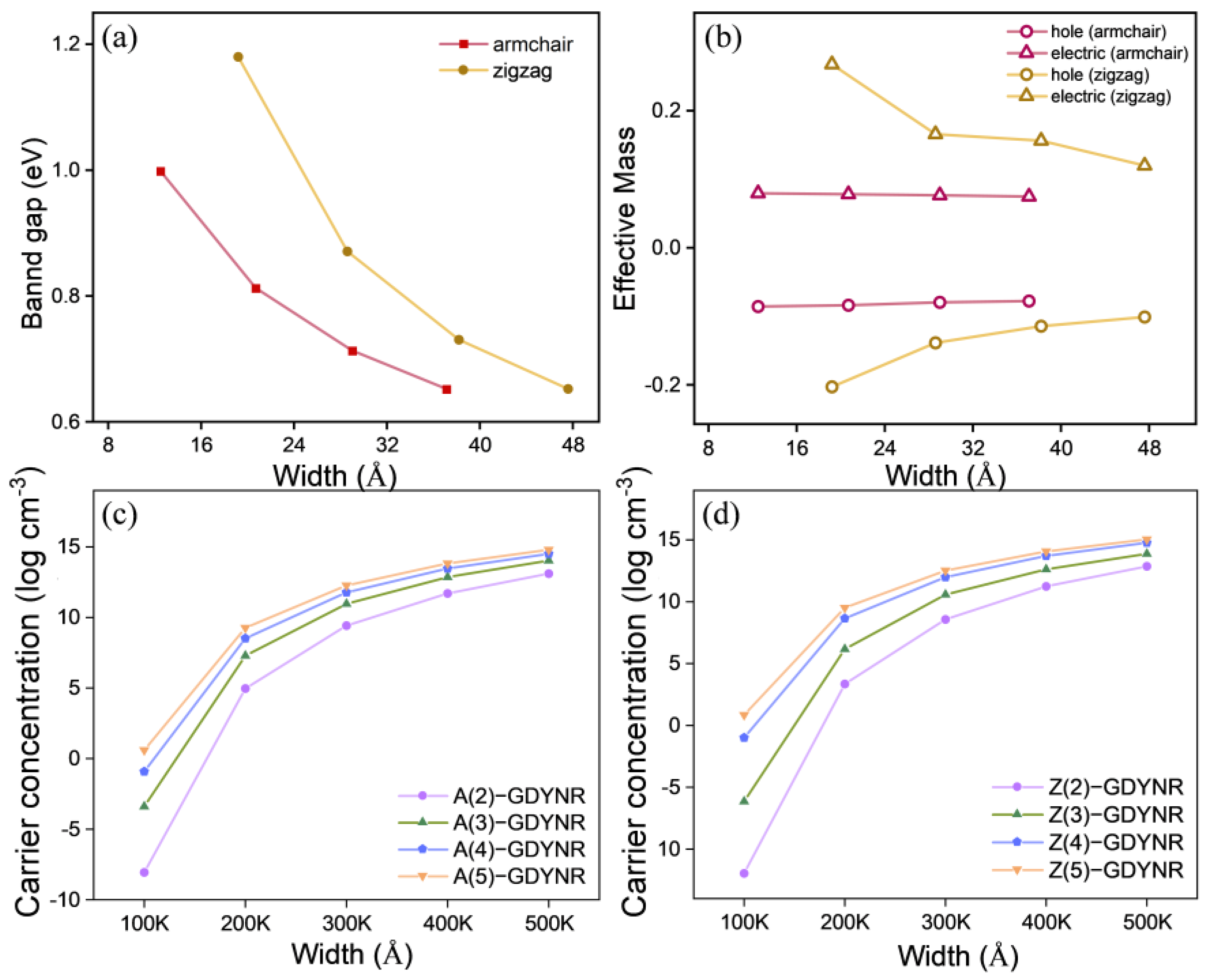
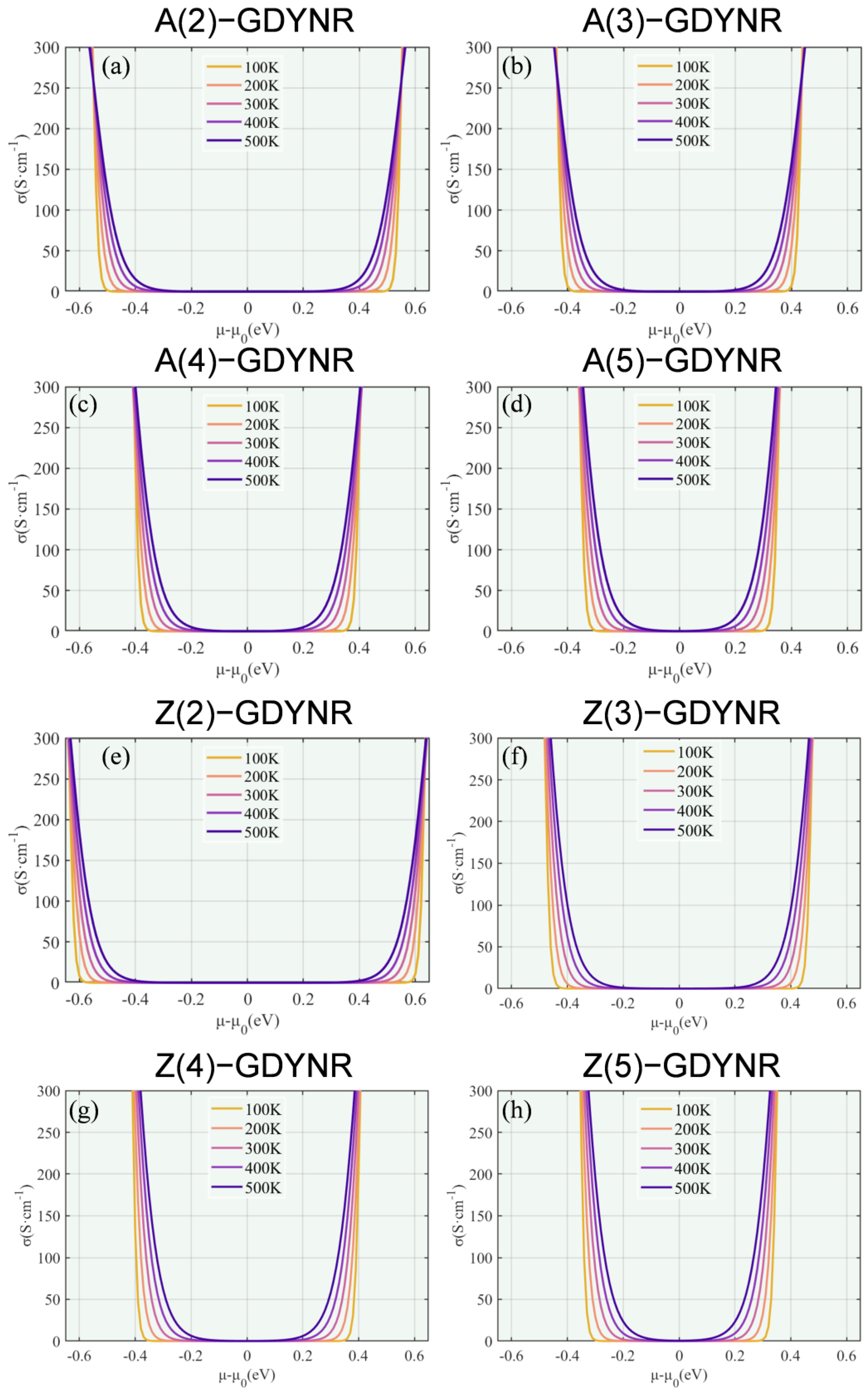
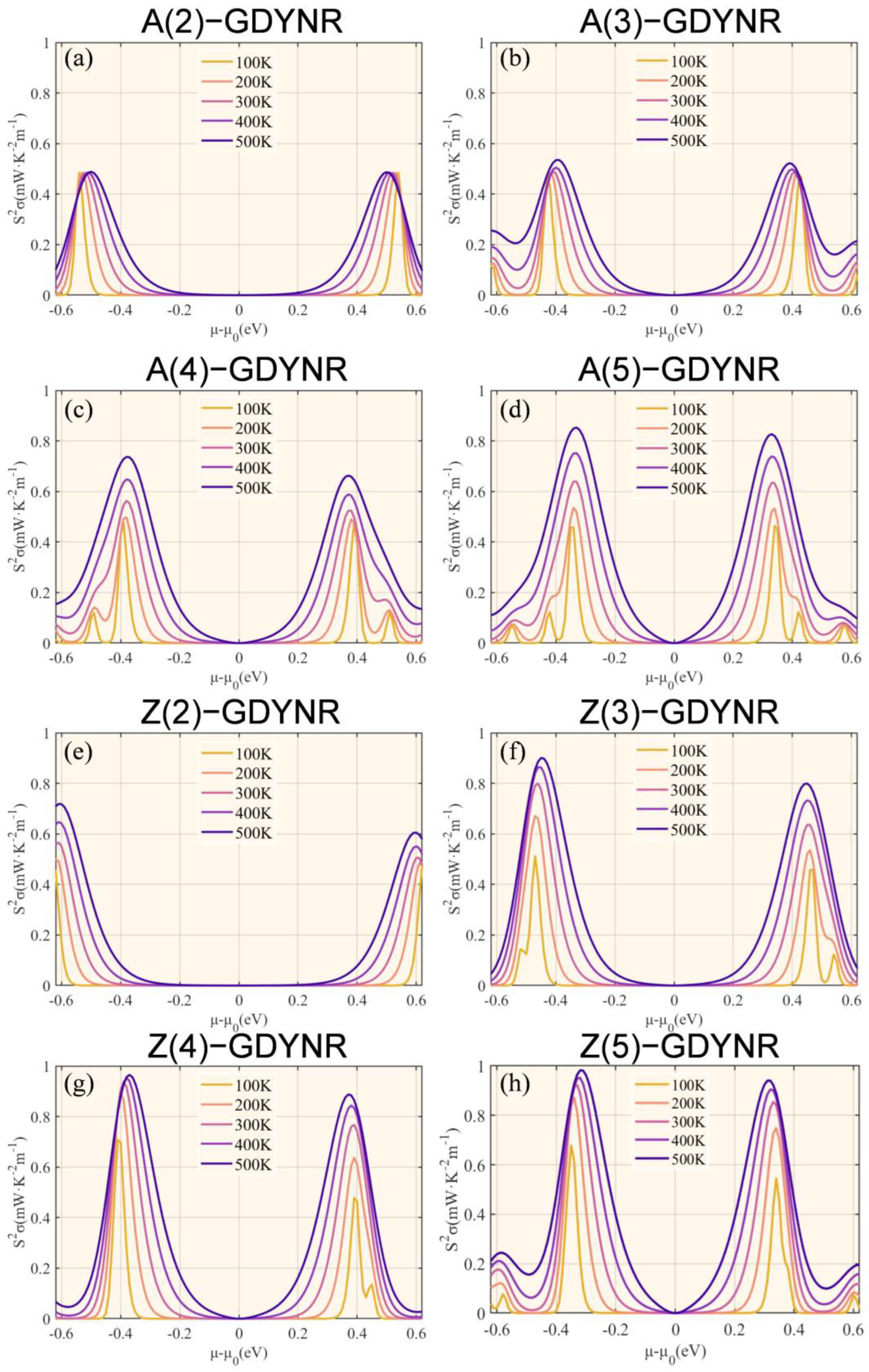
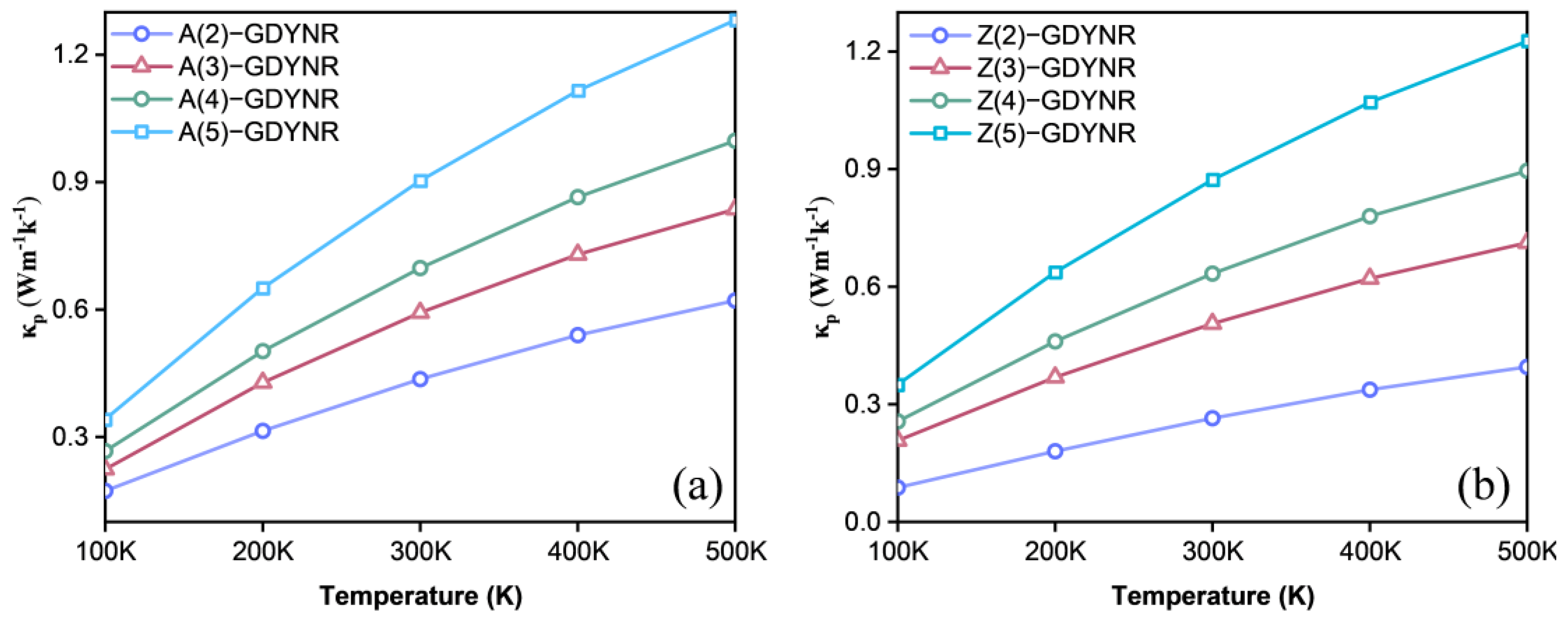
2. Results and Discussions
3. Method
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xi, J.; Nakamura, Y.; Nakamura, Y.; Zhao, T.; Wang, D.; Shuai, Z.G. Theoretical Studies on the Deformation Potential, Electron-Phonon Coupling, and Carrier Transports of Layered Systems. Acta Phys.-Chim. Sin. 2018, 34, 961–976. [Google Scholar] [CrossRef]
- Han, C.; Sun, Q.; Li, Z.; Dou, S.X. Thermoelectric Enhancement of Different Kinds of Metal Chalcogenides. Adv. Energy Mater. 2016, 6, 1600498. [Google Scholar] [CrossRef]
- Mao, J.; Chen, G.; Ren, Z. Thermoelectric Cooling Materials. Nat. Mater. 2021, 20, 454–461. [Google Scholar] [CrossRef]
- Lin, T.; Zhang, Y.H.; Li, H.; Li, P.C. Synthesis and Thermoelectric Properties of Dithieno [3,2-b:2′,3′-d] pyrrole-Based D-A Conjugated Polymers. J. Petrochem. Univ. 2024, 37, 1–10. [Google Scholar]
- Mojdeh, G.; Mohammad, P.; Mozhgan, G.; Azadeh, T. A computational insight into the intrinsic, Si-decorated and vacancy-defected γ-graphyne nanoribbon towards adsorption of CO2 and O2 molecules. Appl. Surf. Sci. 2023, 610, 155510. [Google Scholar]
- Hung, N.T.; Hasdeo, E.H.; Nugraha, A.R.; Dresselhaus, M.S.; Saito, R. Quantum Effects in the Thermoelectric Power Factor of Low-Dimensional Semiconductors. Phys. Rev. Lett. 2016, 117, 036602. [Google Scholar] [CrossRef]
- Slack, G.A. CRC Handbook of Thermo-Electrics; CRC Press: Boca Raton, FL, USA, 1995; Volume 2, p. 407. [Google Scholar]
- Hicks, L.D.; Dresselhaus, M.S. Thermoelectric figure of merit of a one-dimensional conductor. Phys. Rev. B 1993, 47, 12727. [Google Scholar] [CrossRef]
- Fang, Y.; Liu, Y.; Qi, L.; Xue, Y.; Li, Y. 2D graphdiyne: An emerging carbon material. Chem. Soc. Rev. 2022, 51, 2681–2709. [Google Scholar] [CrossRef]
- Li, Y.; Xu, L.; Liu, H.; Li, Y. Graphdiyne and graphyne: From theoretical predictions to practical construction. Chem. Soc. Rev. 2014, 43, 2572–2586. [Google Scholar] [CrossRef]
- Zhou, W.; Shen, H.; Zeng, Y.; Yi, Y.; Zuo, Z.; Li, J.; Li, Y. Controllable Synthesis of Graphdiyne Nanoribbons. Ange. Chem. Int. Ed. 2020, 59, 4908–4913. [Google Scholar] [CrossRef]
- Haskins, J.; Kınacı, A.; Sevik, C.; Sevinçli, H.; Cuniberti, G.; Cağın, T. Control of thermal and electronic transport in defect-engineered graphene nanoribbons. ACS Nano 2011, 5, 3779–3787. [Google Scholar] [CrossRef]
- Yi, Y.; Jian, H.; Zhan, L.; Sheng, C.; Zhen, Z. Inducing abundant magnetic phases and enhancing magnetic stability by edge modifications and physical regulations for NiI2 nanoribbons. Phys. Chem. Chem. Phys. 2024, 26, 5045–5058. [Google Scholar] [CrossRef]
- Yan, P.; Ouyang, T.; He, C.; Li, J.; Zhang, C.; Tang, C.; Zhong, J. Newly discovered graphyne allotrope with rare and robust Dirac node loop. Nanoscale 2021, 13, 3564–3571. [Google Scholar] [CrossRef]
- Ouyang, T.; Cui, C.; Shi, X.; He, C.; Li, J.; Zhang, C.; Tang, C.; Zhong, J. Systematic Enumeration of Low Energy Graphyne Allotropes Based on a Coordination-Constrained Searching Strategy. Phys. Status Solidi. Rapid Res. Lett. 2020, 14, 2000437. [Google Scholar] [CrossRef]
- Sun, L.; Jiang, P.H.; Liu, H.J.; Fan, D.D.; Liang, J.H.; Wei, J.; Cheng, L.; Zhang, J. Graphdiyne: A two-dimensional thermoelectric material with high figure of merit. Carbon 2015, 90, 255–259. [Google Scholar] [CrossRef]
- Wang, C.; Ouyang, T.; Chen, Y.; Zhou, B.; Zhong, J. Thermoelectric properties of gamma-graphyne nanoribbon incorporating diamond-like quantum dots. J. Phys. D Appl. Phys. 2016, 49, 135303. [Google Scholar] [CrossRef]
- Huang, C.; Li, Y.; Wang, N.; Xue, Y.; Zuo, Z.; Liu, H.; Li, Y. Progress in research into 2D graphdiyne-based materials. Chem. Rev. 2018, 118, 7744–7803. [Google Scholar] [CrossRef]
- Tian, W.; Cheng, C.; Wang, C.; Li, W. Research Progress on Thermal Conductivity of Graphdiyne Nanoribbons and its Defects: A Review. Recent Pat. Nano 2020, 14, 294–306. [Google Scholar] [CrossRef]
- Cui, C.; Ouyang, T.; Tang, C.; He, C.; Li, J.; Zhang, C.; Zhong, J. Bayesian optimization-based design of defect gamma-graphyne nanoribbons with high thermoelectric conversion efficiency. Carbon 2021, 176, 52–60. [Google Scholar] [CrossRef]
- Liu, Q.; Wang, X.; Jing, Y.; Wang, J. Graphyne and graphdiyne nanoribbons: From their structures and properties to potential applications. Phys. Chem. Chem. Phys. 2024, 26, 1541. [Google Scholar] [CrossRef]
- Liu, Q.; Feng, N.; Zou, Y.; Fan, C.; Wang, J. Exploring the impact of stress on the electronic structure and optical properties of graphdiyne nanoribbons for advanced optoelectronic applications. Sci. Rep. 2024, 14, 6051. [Google Scholar] [CrossRef]
- Pan, L.D.; Zhang, L.Z.; Song, B.Q.; Du, S.X.; Gao, H.J. Graphyne- and graphdiyne-based nanoribbons: Density functional theory calculations of electronic structures. Appl. Phys. Lett. 2011, 98, 173102. [Google Scholar] [CrossRef]
- Heremans, J.P. Low-Dimensional Thermoelectricity. Acta Phys. Polym. 2005, 108, 609–634. [Google Scholar] [CrossRef]
- Bai, H.C.; Zhu, Y.; Qiao, W.Y.; Huang, Y.H. Structures, stabilities and electronic properties of graphdiyne nanoribbons. RSC Adv. 2011, 1, 768–775. [Google Scholar] [CrossRef]
- Lu, J.; Cui, C.; Ouyang, T.; Li, J.; He, C.; Tang, C.; Zhong, J. Adaptive genetic algorithm-based design of gamma-graphyne nanoribbon incorporating diamond-shaped segment with high thermoelectric conversion efficiency. Chin. Phys. B 2023, 32, 048401. [Google Scholar] [CrossRef]
- Tan, X.; Shao, H.; Hu, T.; Liu, G.; Jiang, J.; Jiang, H. High thermoelectric performance in two-dimensional graphyne sheets predicted by first-principles calculations. Phys. Chem. Chem. Phys. 2015, 17, 22872–22881. [Google Scholar] [CrossRef]
- Rodrigues, D.C.; Lage, L.L.; Venezuela, P.; Latgé, A. Exploring the enhancement of the thermoelectric properties of bilayer graphyne nanoribbons. Phys. Chem. Chem. Phys. 2022, 24, 9324–9332. [Google Scholar] [CrossRef]
- Pan, C.N.; Chen, X.K.; Tang, L.M.; Chen, K.Q. Orientation dependent thermal conductivity in graphyne nanoribbons. Phys. E 2014, 64, 129–133. [Google Scholar] [CrossRef]
- Ouyang, T.; Chen, Y.; Liu, L.; Xie, Y.; Wei, X.; Zhong, J. Thermal transport in graphyne nanoribbons. Phys. Rev. B 2012, 85, 235436. [Google Scholar] [CrossRef]
- Wei, N.; Xu, L.; Wang, H.Q.; Zheng, J.C. Strain engineering of thermal conductivity in graphene sheets and nanoribbons: A demonstration of magic flexibility. Nanotechnology 2011, 22, 105705. [Google Scholar] [CrossRef]
- Li, J.Q.; Han, Y. Artificial carbon allotrope γ-graphyne Synthesis, properties, and applications. Giant 2023, 13, 100140. [Google Scholar] [CrossRef]
- Wang, J.; Liu, Q.; Miao, W.; Sun, M. Simulation on Stress-Related Anisotropy of qTP C60 and qHP C60: Implications for Optoelectronic Nanodevices. ACS Appl. Nano Mater. 2024, 7, 5912–5921. [Google Scholar] [CrossRef]
- Shen, J.J.; Fang, T.; Fu, T.Z. Lattice thermal conductivity in thermoelectric materials. J. Inorg. Mater. 2019, 34, 260–268. [Google Scholar]
- vanSetten, M.J.; Giantomassi, M.; Bousquet, E.; Verstraete, M.J.; Hamann, D.R.; Gonze, V.; Rignanese, G.M. The PseudoDojo: Training and grading a 85 element optimized norm-conserving pseudopotential table. Comput. Phys. Commun. 2018, 226, 39–54. [Google Scholar] [CrossRef]
- Smidstrup, S.; Markussen, T.; Vancraeyveld, P.; Wellendorff, J.; Schneider, J.; Gunst, T.; Verstichel, B.; Stradi, D.; Homyakov, P.A.; Vej-Hansen, U.G.; et al. QuantumATK: An integrated platform of electronic and atomic-scale modelling tools. J. Phys. Condens. Matter 2019, 32, 015901. [Google Scholar] [CrossRef]
- Grimme, S.; Antony, J.; Ehrlich, S.; Krieg, H. A consistent and accurate ab initio parametrization of density functional dispersion correction (DFT-D) for the 94 elements H-Pu. J. Chem. Phys. 2010, 132, 154104. [Google Scholar] [CrossRef]
- For Details of Nanodcal Software Package. Available online: http://www.hzwtech.com (accessed on 10 November 2023).
- Hughes, J.L.; Sipe, J. Calculation of second-order optical response in semi-conductors. Phys. Rev. B 1996, 53, 10751. [Google Scholar] [CrossRef]
- Levine, Z.H.; Allan, D.C. Linear optical response in silicon and germanium including self-energy effects. Phys. Rev. Lett. 1989, 63, 1719. [Google Scholar] [CrossRef]
- Zhang, J.; Xie, Y.; Hu, Y.; Shao, H. Remarkable intrinsic ZT in the 2D PtX2(X = O, S, Se, Te) monolayers at room temperature. Appl. Surf. Sci. 2020, 532, 147387. [Google Scholar] [CrossRef]
- Song, J.; Sun, M. Modulating thermoelectric properties of the MoSe2/WSe2 superlattice heterostructure by twist angles. ACS Appl. Mater. Inter. 2024, 16, 3325–3333. [Google Scholar] [CrossRef]
- Mott, N. The electrical conductivity of transition metals. Proceedings of the Royal Society of London. Proc. Math. Phys. Eng. Sci. 1936, 153, 699–717. [Google Scholar]
- Trachenko, K. Heat capacity of liquids: An approach from the solid phase. Phys. Rev. B Condens. 2008, 78, 104201. [Google Scholar] [CrossRef]
- Jonson, M.; Mahan, G. Mott’s Formula for the Thermopower and the Wiedemann-Franz Law. Phys. Rev. B 1980, 21, 4223. [Google Scholar] [CrossRef]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, M.; Liu, Q.; Zou, Y.; Wang, J.; Fan, C. Thermoelectric Properties Regulated by Quantum Size Effects in Quasi-One-Dimensional γ-Graphdiyne Nanoribbons. Molecules 2024, 29, 3312. https://doi.org/10.3390/molecules29143312
Li M, Liu Q, Zou Y, Wang J, Fan C. Thermoelectric Properties Regulated by Quantum Size Effects in Quasi-One-Dimensional γ-Graphdiyne Nanoribbons. Molecules. 2024; 29(14):3312. https://doi.org/10.3390/molecules29143312
Chicago/Turabian StyleLi, Mi, Qiaohan Liu, Yi Zou, Jingang Wang, and Chuanqiang Fan. 2024. "Thermoelectric Properties Regulated by Quantum Size Effects in Quasi-One-Dimensional γ-Graphdiyne Nanoribbons" Molecules 29, no. 14: 3312. https://doi.org/10.3390/molecules29143312
APA StyleLi, M., Liu, Q., Zou, Y., Wang, J., & Fan, C. (2024). Thermoelectric Properties Regulated by Quantum Size Effects in Quasi-One-Dimensional γ-Graphdiyne Nanoribbons. Molecules, 29(14), 3312. https://doi.org/10.3390/molecules29143312







