Renewing Interest in Zeolites as Adsorbents for Capture of Cationic Dyes from Aqueous and Ethanolic Solutions: A Simulation-Based Insight into the Efficiency of Dye Adsorption in View of Wastewater Treatment and Valorization of Post-Sorption Materials
Abstract
1. Introduction
2. Results
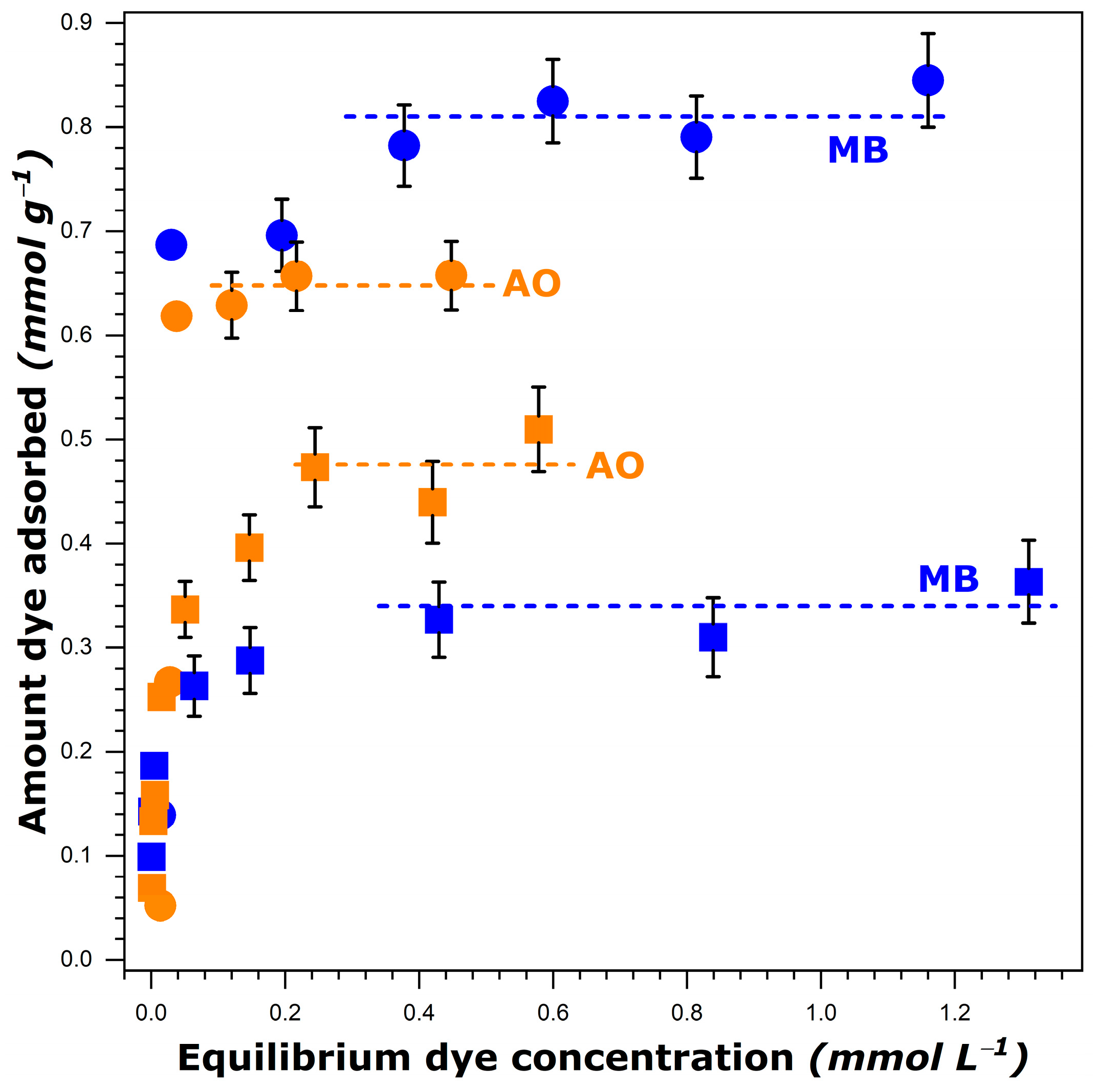
2.1. Experimental Adsorption Isotherms
2.2. Preliminary Molecular Simulations
2.2.1. Model of FAU-Y
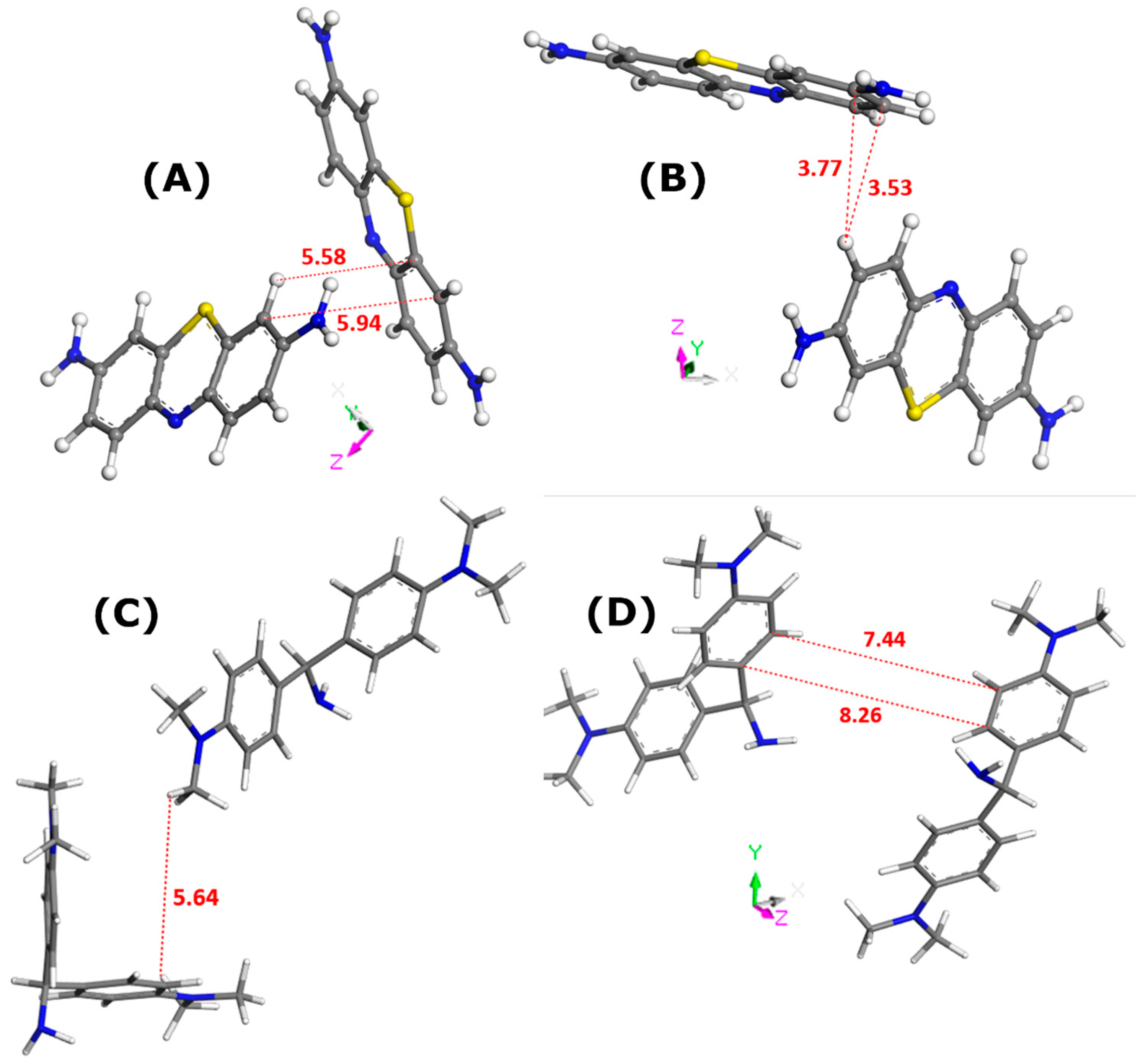
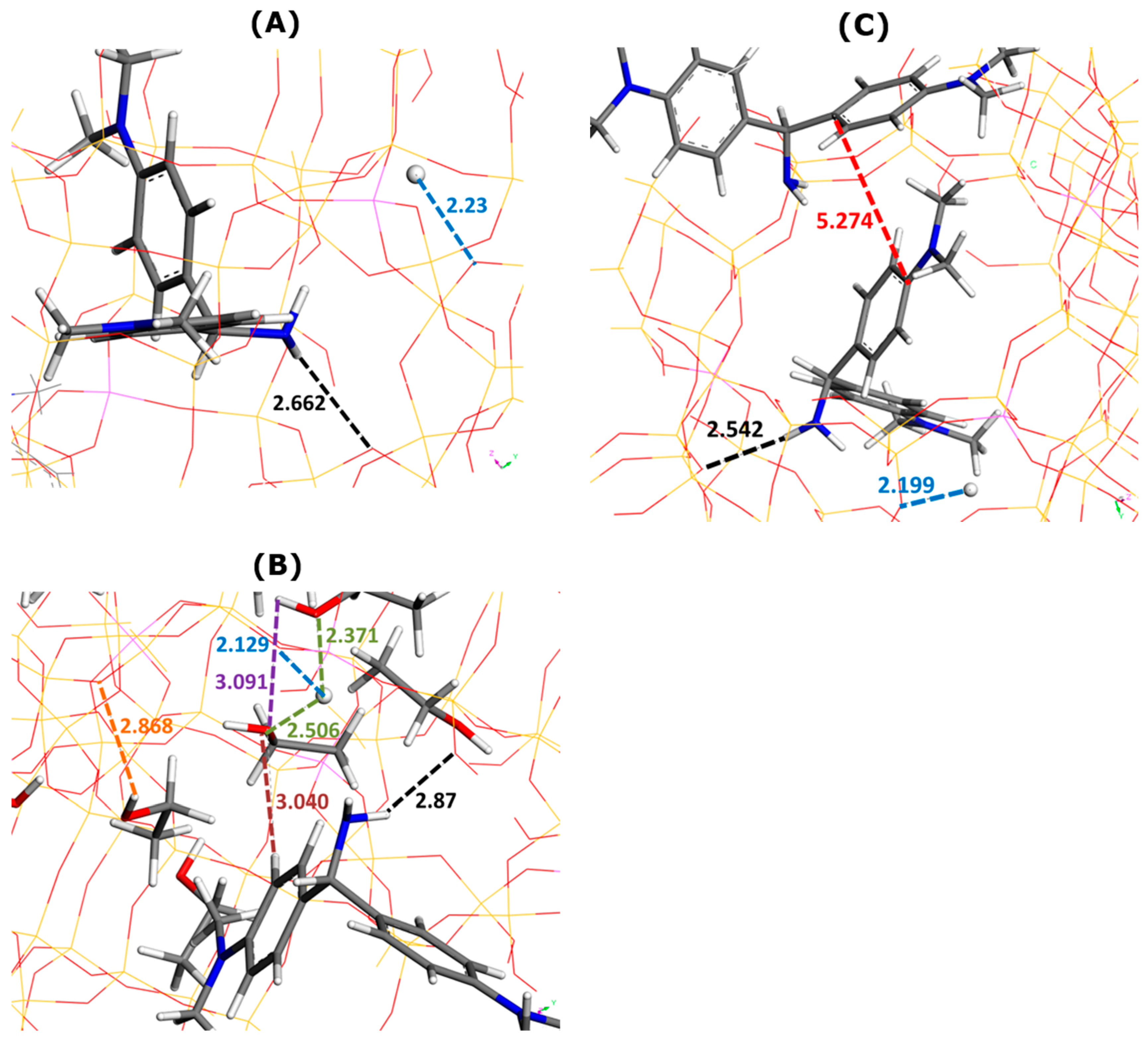
2.2.2. Adsorption Enthalpies and Pi-Stacking in Both Solvents
2.3. Monte Carlo Simulations Involving the Presence of Solvent
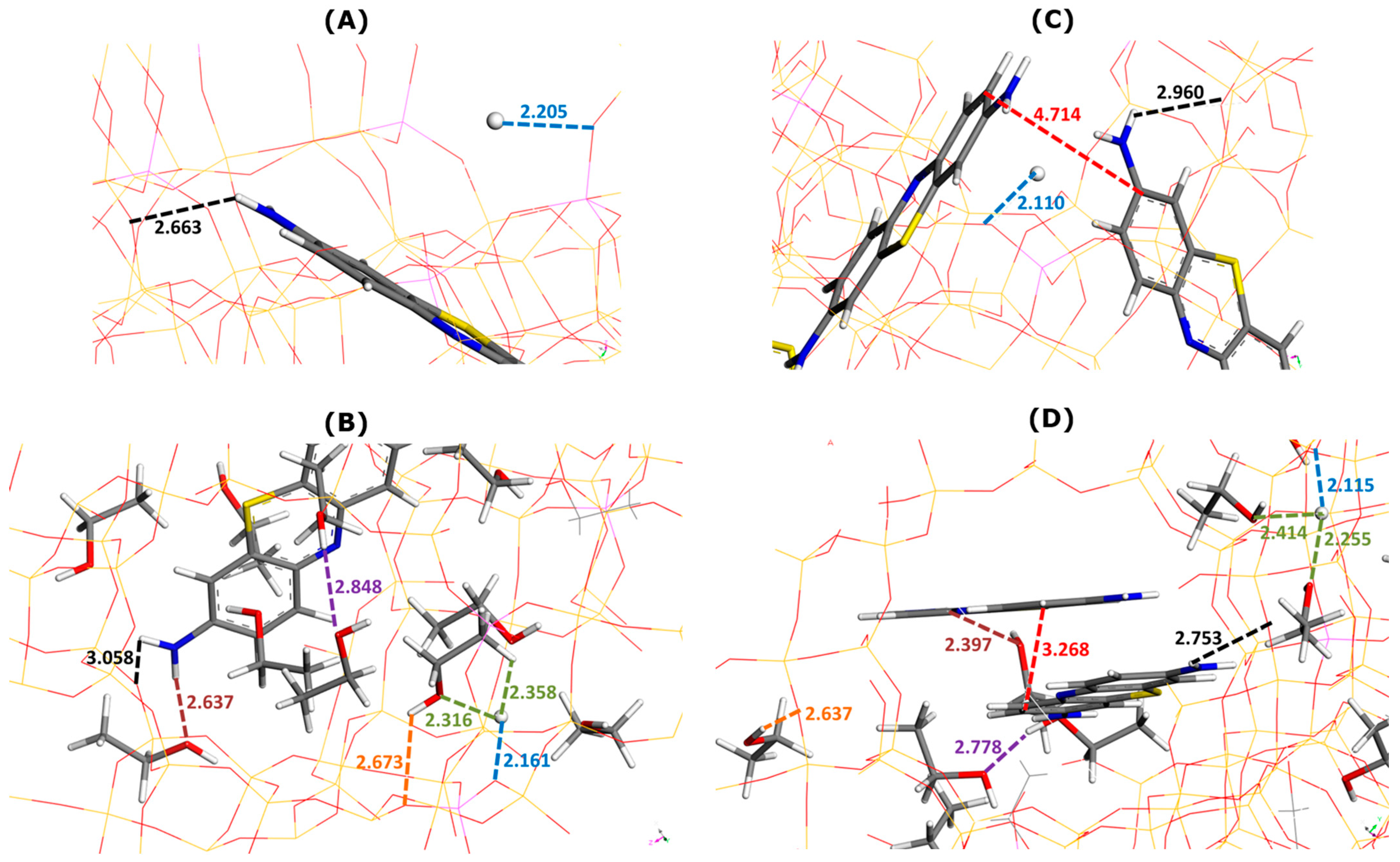
2.3.1. MB–Zeolite System at Saturation in the Presence of Water or Ethanol
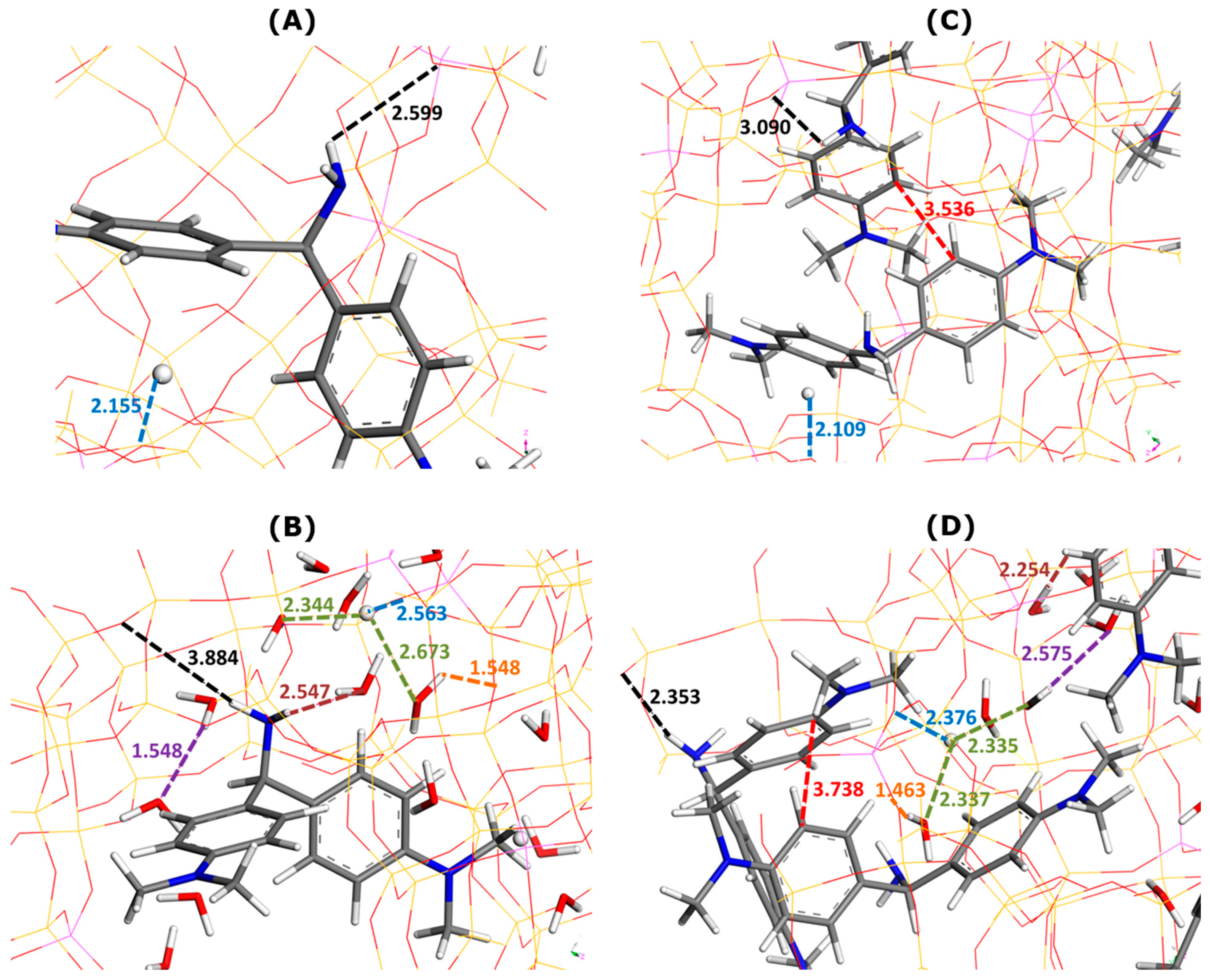
2.3.2. AO–Zeolite System at Saturation in the Presence of Water or Ethanol
3. Discussion
4. Materials and Methods
4.1. Materials
4.2. Dye Adsorption Isotherms
4.3. Strategy of Molecular Simulations
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Lellis, B.; Fávaro-Polonio, C.Z.; Pamphile, J.A.; Polonio, J.C. Effects of textile dyes on health and the environment and bioremediation potential of living organisms. Biotechnol. Res. Innov. 2019, 3, 275–290. [Google Scholar] [CrossRef]
- Pereira, L.; Alves, M. Dyes—Environmental Impact and Remediation. In Environmental Protection Strategies for Sustainable Development; Malik, A., Grohmann, E., Eds.; Springer: Dordrecht, The Netherlands, 2012; pp. 111–162. [Google Scholar]
- Singh, N.B.; Nagpal, G.; Agrawal, S.; Rachna. Water purification by using Adsorbents: A Review. Environ. Technol. Innovation 2018, 11, 187–240. [Google Scholar] [CrossRef]
- Osagie, C.; Othmani, A.; Ghosh, S.; Malloum, A.; Kashitarash Esfahani, Z.; Ahmadi, S. Dyes adsorption from aqueous media through the nanotechnology: A review. J. Mater. Res. Technol. 2021, 14, 2195–2218. [Google Scholar] [CrossRef]
- Rai, P.K. Novel adsorbents in remediation of hazardous environmental pollutants: Progress, selectivity, and sustainability prospects. Cleaner Mater. 2022, 3, 100054. [Google Scholar] [CrossRef]
- Xia, L.; Wang, A.; Zhang, C.; Liu, Y.; Guo, H.; Ding, C.; Wang, Y.; Xu, W. Environmentally friendly dyeing of cotton in an ethanol–water mixture with excellent exhaustion. Green Chem. 2018, 20, 4473–4483. [Google Scholar] [CrossRef]
- Valdes-Aguilera, O.; Neckers, D.C. Aggregation phenomena in xanthene dyes. Acc. Chem. Res. 1989, 22, 171–177. [Google Scholar] [CrossRef]
- Moshoeshoe, M.N.; Nadiye-Tabbiruka, M.S.; Obuseng, V.C. A Review of the Chemistry, Structure, Properties and Applications of Zeolites. Am. J. Mater. Sci. 2017, 7, 196–221. [Google Scholar] [CrossRef]
- Li, Y.; Li, L.; Yu, J. Applications of Zeolites in Sustainable Chemistry. Chem 2017, 3, 928–949. [Google Scholar] [CrossRef]
- Hammood, Z.A.; Chyad, T.F.; Al-Saedi, R. Adsorption Performance of Dyes Over Zeolite for Textile Wastewater Treatment. Ecol. Chem. Eng. S 2021, 28, 329–337. [Google Scholar] [CrossRef]
- de Magalhães, L.F.; da Silva, G.R.; Peres, A.E.C.; Kooh, M.R.R. Zeolite Application in Wastewater Treatment. Adsorpt. Sci. Technol. 2022, 2022, 4544104. [Google Scholar] [CrossRef]
- Radoor, S.; Karayil, J.; Jayakumar, A.; Nandi, D.; Parameswaranpillai, J.; Lee, J.; Shivanna, J.M.; Nithya, R.; Siengchin, S. Adsorption of Cationic Dye onto ZSM-5 Zeolite-Based Bio Membrane: Characterizations, Kinetics and Adsorption Isotherm. J. Polym. Environ. 2022, 30, 3279–3292. [Google Scholar] [CrossRef]
- Velarde, L.; Nabavi, M.S.; Escalera, E.; Antti, M.-L.; Akhtar, F. Adsorption of heavy metals on natural zeolites: A review. Chemosphere 2023, 328, 138508. [Google Scholar] [CrossRef]
- Díez, E.; Redondo, C.; Gómez, J.M.; Miranda, R.; Rodríguez, A. Zeolite Adsorbents for Selective Removal of Co(II) and Li(I) from Aqueous Solutions. Water 2023, 15, 270. [Google Scholar] [CrossRef]
- Fischer, M. Simulation-based evaluation of zeolite adsorbents for the removal of emerging contaminants. Mater. Adv. 2020, 1, 86–98. [Google Scholar] [CrossRef]
- Johan, E.; Yamada, T.; Munthali, M.W.; Kabwadza-Corner, P.; Aono, H.; Matsue, N. Natural Zeolites as Potential Materials for Decontamination of Radioactive Cesium. Procedia Environ. Sci. 2015, 28, 52–56. [Google Scholar] [CrossRef]
- Karadag, D.; Akgul, E.; Tok, S.; Erturk, F.; Kaya, M.A.; Turan, M. Basic and Reactive Dye Removal Using Natural and Modified Zeolites. J. Chem. Eng. Data 2007, 52, 2436–2441. [Google Scholar] [CrossRef]
- Han, R.; Zhang, J.; Han, P.; Wang, Y.; Zhao, Z.; Tang, M. Study of equilibrium, kinetic and thermodynamic parameters about methylene blue adsorption onto natural zeolite. Chem. Eng. J. 2009, 145, 496–504. [Google Scholar] [CrossRef]
- Ozdemir, O.; Armagan, B.; Turan, M.; Çelik, M.S. Comparison of the adsorption characteristics of azo-reactive dyes on mezoporous minerals. Dyes Pigm. 2004, 62, 49–60. [Google Scholar] [CrossRef]
- Rakanović, M.; Vukojević, A.; Savanović, M.M.; Armaković, S.; Pelemiš, S.; Živić, F.; Sladojević, S.; Armaković, S.J. Zeolites as Adsorbents and Photocatalysts for Removal of Dyes from the Aqueous Environment. Molecules 2022, 27, 6582. [Google Scholar] [CrossRef]
- Hu, G.; Yang, J.; Duan, X.; Farnood, R.; Yang, C.; Yang, J.; Liu, W.; Liu, Q. Recent developments and challenges in zeolite-based composite photocatalysts for environmental applications. Chem. Eng. J. 2021, 417, 129209. [Google Scholar] [CrossRef]
- Kusiak-Nejman, E.; Sienkiewicz, A.; Wanag, A.; Rokicka-Konieczna, P.; Morawski, A.W. The Role of Adsorption in the Photocatalytic Decomposition of Dyes on APTES-Modified TiO2 Nanomaterials. Catalysts 2021, 11, 172. [Google Scholar] [CrossRef]
- Xue, G.; Liu, H.; Chen, Q.; Hills, C.; Tyrer, M.; Innocent, F. Synergy between surface adsorption and photocatalysis during degradation of humic acid on TiO2/activated carbon composites. J. Hazard. Mater. 2011, 186, 765–772. [Google Scholar] [CrossRef] [PubMed]
- Pan, Y.; Zhou, Q.; Meng, F.; Cao, Q.; Bai, J.; Zhang, Z.; Liu, Q.; Zhang, H. (1 1 1) Facet-dominant peracetic acid activation by octahedral CoO anchored hollow carbon microspheres for tetracycline degradation. Chem. Eng. J. 2024, 493, 152446. [Google Scholar] [CrossRef]
- Pan, Y.; Meng, F.; Bai, J.; Song, B.; Cao, Q. Highly efficient peroxymonosulfate activation by CoFe2O4@attapulgite–biochar composites: Degradation properties and mechanism insights. J. Environ. Chem. Eng. 2024, 12, 112579. [Google Scholar] [CrossRef]
- Harikishore Kumar Reddy, D.; Vijayaraghavan, K.; Kim, J.A.; Yun, Y.-S. Valorisation of post-sorption materials: Opportunities, strategies, and challenges. Adv. Colloid Interface Sci. 2017, 242, 35–58. [Google Scholar] [CrossRef]
- Binder, F.; Calzaferri, G.; Gfeller, N. Dye molecules in zeolites as artificial antenna. Sol. Energy Mater. Sol. Cells 1995, 38, 175–186. [Google Scholar] [CrossRef]
- Zanjanchi, M.A.; Sohrabnezhad, S. Evaluation of methylene blue incorporated in zeolite for construction of an optical humidity sensor. Sens. Actuators B 2005, 105, 502–507. [Google Scholar] [CrossRef]
- Calzaferri, G.; Li, H.; Brühwiler, D. Dye-Modified Nanochannel Materials for Photoelectronic and Optical Devices. Chem. Eur. J. 2008, 14, 7442–7449. [Google Scholar] [CrossRef]
- Schulz-Ekloff, G.; Wöhrle, D.; van Duffel, B.; Schoonheydt, R.A. Chromophores in porous silicas and minerals: Preparation and optical properties. Microporous Mesoporous Mater. 2002, 51, 91–138. [Google Scholar] [CrossRef]
- Gigli, L.; Arletti, R.; Fois, E.; Tabacchi, G.; Quartieri, S.; Dmitriev, V.; Vezzalini, G. Unravelling the High-Pressure Behaviour of Dye-Zeolite L Hybrid Materials. Crystals 2018, 8, 79. [Google Scholar] [CrossRef]
- Ismail, A.M.; Menazea, A.A.; Ali, H. Selective adsorption of cationic azo dyes onto zeolite nanorod-based membranes prepared via laser ablation. J. Mater. Sci. Mater. Electron. 2021, 32, 19352–19367. [Google Scholar] [CrossRef]
- Oladoye, P.O.; Kadhom, M.; Khan, I.; Hama Aziz, K.H.; Alli, Y.A. Advancements in adsorption and photodegradation technologies for Rhodamine B dye wastewater treatment: Fundamentals, applications, and future directions. Green Chem. Eng. [CrossRef]
- Gould, N.S.; Li, S.; Cho, H.J.; Landfield, H.; Caratzoulas, S.; Vlachos, D.; Bai, P.; Xu, B. Understanding solvent effects on adsorption and protonation in porous catalysts. Nat. Commun. 2020, 11, 1060. [Google Scholar] [CrossRef] [PubMed]
- Ottewill, R.H.; Rochester, C.H.; Smith, A.L. (Eds.) Adsorption from Solution; Academic Press: London, UK, 1983. [Google Scholar]
- Koopal, L.K. Adsorption from solution on solids. In Interface Science, 2nd ed.; Koopal, L.K., Ed.; Wageningen University: Wageningen, The Netherlands, 2008. [Google Scholar]
- Prélot, B.; Zając, J. Chapter 2. Contribution of isothermal titration calorimetry to elucidate the mechanism of adsorption from dilute aqueous solutions on solid surfaces: Data processing, analysis, and interpretation. In Thermal Analysis and Calorimetry; Auroux, A., Damjanovic-Vasilic, L., Eds.; De Gruyter: Berlin, Germany; Boston, MA, USA, 2023; pp. 47–90. [Google Scholar]
- Aydan, T.; Yang, J.-J.; Muhammad, T.; Gao, F.; Yang, X.-X.; Hu, Y.-T. In-situ measurement of Auramine-O adsorption on macroporous adsorption resins at low temperature using fiber-optic sensing. Desalin. Water Treat. 2021, 213, 240–247. [Google Scholar] [CrossRef]
- Green, F.J. The Sigma-Aldrich Handbook of Stains Dyes and Indicators; Aldrich Chemical: Milwaukee, WI, USA, 1990. [Google Scholar]
- Salimi, A.; Roosta, A. Experimental solubility and thermodynamic aspects of methylene blue in different solvents. Thermochim. Acta 2019, 675, 134–139. [Google Scholar] [CrossRef]
- Adedokun, O.; Sanusi, Y.K.; Awodugba, A.O. Solvent dependent natural dye extraction and its sensitization effect for dye sensitized solar cells. Optik 2018, 174, 497–507. [Google Scholar] [CrossRef]
- Di Lella, A.; Desbiens, N.; Boutin, A.; Demachy, I.; Ungerer, P.; Bellat, J.-P.; Fuchs, A.H. Molecular simulation studies of water physisorption in zeolites. Phys. Chem. Chem. Phys. 2006, 8, 5396–5406. [Google Scholar] [CrossRef] [PubMed]
- Abrioux, C.; Coasne, B.; Maurin, G.; Henn, F.; Jeffroy, M.; Boutin, A. Cation Behavior in Faujasite Zeolites upon Water Adsorption: A Combination of Monte Carlo and Molecular Dynamics Simulations. J. Phys. Chem. C 2009, 113, 10696–10705. [Google Scholar] [CrossRef]
- Ferrage, E.; Sakharov, B.A.; Michot, L.J.; Delville, A.; Bauer, A.; Lanson, B.; Grangeon, S.; Frapper, G.; Jiménez-Ruiz, M.; Cuello, G.J. Hydration Properties and Interlayer Organization of Water and Ions in Synthetic Na-Smectite with Tetrahedral Layer Charge. Part 2. Toward a Precise Coupling between Molecular Simulations and Diffraction Data. J. Phys. Chem. C 2011, 115, 1867–1881. [Google Scholar] [CrossRef]
- Saidi, M.; Ho, P.H.; Yadav, P.; Salles, F.; Charnay, C.; Girard, L.; Boukli-Hacene, L.; Trens, P. Zirconium-Based Metal Organic Frameworks for the Capture of Carbon Dioxide and Ethanol Vapour. A Comparative Study. Molecules 2021, 26, 7620. [Google Scholar] [CrossRef]
- Pillai, R.S.; Peter, S.A.; Jasra, R.V. CO2 and N2 adsorption in alkali metal ion exchanged X-Faujasite: Grand canonical Monte Carlo simulation and equilibrium adsorption studies. Microporous Mesoporous Mater. 2012, 162, 143–151. [Google Scholar] [CrossRef]
- Assaf, M.; Martin-Gassin, G.; Prelot, B.; Gassin, P.-M. Driving Forces of Cationic Dye Adsorption, Confinement, and Long-Range Correlation in Zeolitic Materials. Langmuir 2022, 38, 1296–1303. [Google Scholar] [CrossRef] [PubMed]
- Alby, D.; Salles, F.; Fullenwarth, J.; Zajac, J. On the use of metal cation-exchanged zeolites in sorption thermochemical storage: Some practical aspects in reference to the mechanism of water vapor adsorption. Sol. Energy Mater. Sol. Cells 2018, 179, 223–230. [Google Scholar] [CrossRef]
- Zhu, L.; Seff, K. Reinvestigation of the Crystal Structure of Dehydrated Sodium Zeolite X. J. Phys. Chem. B 1999, 103, 9512–9518. [Google Scholar] [CrossRef]
- Heard, C.J.; Grajciar, L.; Nachtigall, P. The effect of water on the validity of Löwenstein’s rule. Chem. Sci. 2019, 10, 5705–5711. [Google Scholar] [CrossRef] [PubMed]
- Halasz, I.; Kim, S.; Marcus, B. Hydrophilic and hydrophobic adsorption on Y zeolites. Mol. Phys. 2002, 100, 3123–3132. [Google Scholar] [CrossRef]
- Wang, C.; Guo, H.; Leng, S.; Yu, J.; Feng, K.; Cao, L.; Huang, J. Regulation of hydrophilicity/hydrophobicity of aluminosilicate zeolites: A review. Crit. Rev. Solid State Mater. Sci. 2021, 46, 330–348. [Google Scholar] [CrossRef]
- Patil, K.; Pawar, R.; Talap, P. Self-aggregation of Methylene Blue in aqueous medium and aqueous solutions of Bu4NBr and urea. Phys. Chem. Chem. Phys. 2000, 2, 4313–4317. [Google Scholar] [CrossRef]
- Calero, S.; Dubbeldam, D.; Krishna, R.; Smit, B.; Vlugt, T.J.H.; Denayer, J.F.M.; Martens, J.A.; Maesen, T.L.M. Understanding the Role of Sodium during Adsorption: A Force Field for Alkanes in Sodium-Exchanged Faujasites. J. Am. Chem. Soc. 2004, 126, 11377–11386. [Google Scholar] [CrossRef] [PubMed]
- BIOVIA. Dassault Systèmes, Materials Studio; BIOVIA: Paris, France, 2020. [Google Scholar]
- Abascal, J.L.F.; Vega, C. A general purpose model for the condensed phases of water: TIP4P/2005. J. Chem. Phys. 2005, 123, 234505. [Google Scholar] [CrossRef] [PubMed]
- Wells, B.A.; Chaffee, A.L. Ewald Summation for Molecular Simulations. J. Chem. Theory Comput. 2015, 11, 3684–3695. [Google Scholar] [CrossRef]
- Salcedo-Abraira, P.; Biglione, C.; Vilela, S.M.F.; Svensson Grape, E.; Ureña, N.; Salles, F.; Pérez-Prior, M.T.; Willhammar, T.; Trens, P.; Várez, A.; et al. High Proton Conductivity of a Bismuth Phosphonate Metal–Organic Framework with Unusual Topology. Chem. Mater. 2023, 35, 4329–4337. [Google Scholar] [CrossRef]
- Kramer, G.J.; Farragher, N.P.; Vanbeest, B.W.H.; Vansanten, R.A. Interatomic force fields for silicas, aluminophosphates, and zeolites: Derivation based on ab initio calculations. Phys. Rev. B 1991, 43, 5068–5080. [Google Scholar] [CrossRef] [PubMed]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Boudjema, L.; Assaf, M.; Salles, F.; Gassin, P.-M.; Martin-Gassin, G.; Zajac, J. Renewing Interest in Zeolites as Adsorbents for Capture of Cationic Dyes from Aqueous and Ethanolic Solutions: A Simulation-Based Insight into the Efficiency of Dye Adsorption in View of Wastewater Treatment and Valorization of Post-Sorption Materials. Molecules 2024, 29, 2952. https://doi.org/10.3390/molecules29132952
Boudjema L, Assaf M, Salles F, Gassin P-M, Martin-Gassin G, Zajac J. Renewing Interest in Zeolites as Adsorbents for Capture of Cationic Dyes from Aqueous and Ethanolic Solutions: A Simulation-Based Insight into the Efficiency of Dye Adsorption in View of Wastewater Treatment and Valorization of Post-Sorption Materials. Molecules. 2024; 29(13):2952. https://doi.org/10.3390/molecules29132952
Chicago/Turabian StyleBoudjema, Lotfi, Marwa Assaf, Fabrice Salles, Pierre-Marie Gassin, Gaelle Martin-Gassin, and Jerzy Zajac. 2024. "Renewing Interest in Zeolites as Adsorbents for Capture of Cationic Dyes from Aqueous and Ethanolic Solutions: A Simulation-Based Insight into the Efficiency of Dye Adsorption in View of Wastewater Treatment and Valorization of Post-Sorption Materials" Molecules 29, no. 13: 2952. https://doi.org/10.3390/molecules29132952
APA StyleBoudjema, L., Assaf, M., Salles, F., Gassin, P.-M., Martin-Gassin, G., & Zajac, J. (2024). Renewing Interest in Zeolites as Adsorbents for Capture of Cationic Dyes from Aqueous and Ethanolic Solutions: A Simulation-Based Insight into the Efficiency of Dye Adsorption in View of Wastewater Treatment and Valorization of Post-Sorption Materials. Molecules, 29(13), 2952. https://doi.org/10.3390/molecules29132952






