Recovering Phosphate from Complex Wastewater Using Macroporous Cryogel Composited Calcium Silicate Hydrate Nanoparticles
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Preparation and Characterization of the Adsorption Column
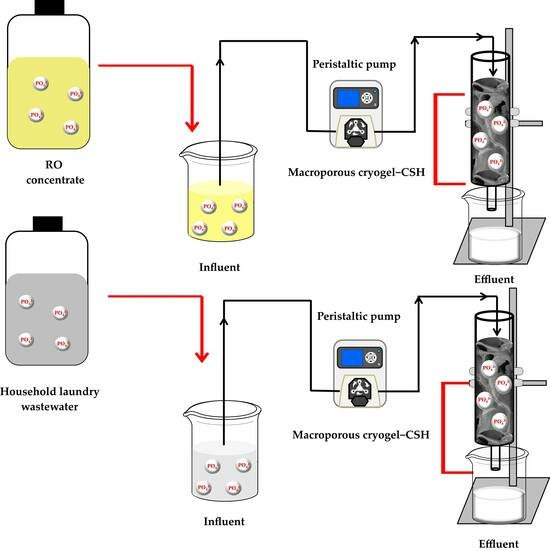
2.3. Continuous Flow Adsorption System
2.4. Adsorption Data Analysis
2.5. Influence of Interferences
2.6. Real Sample Application
3. Results and Discussion
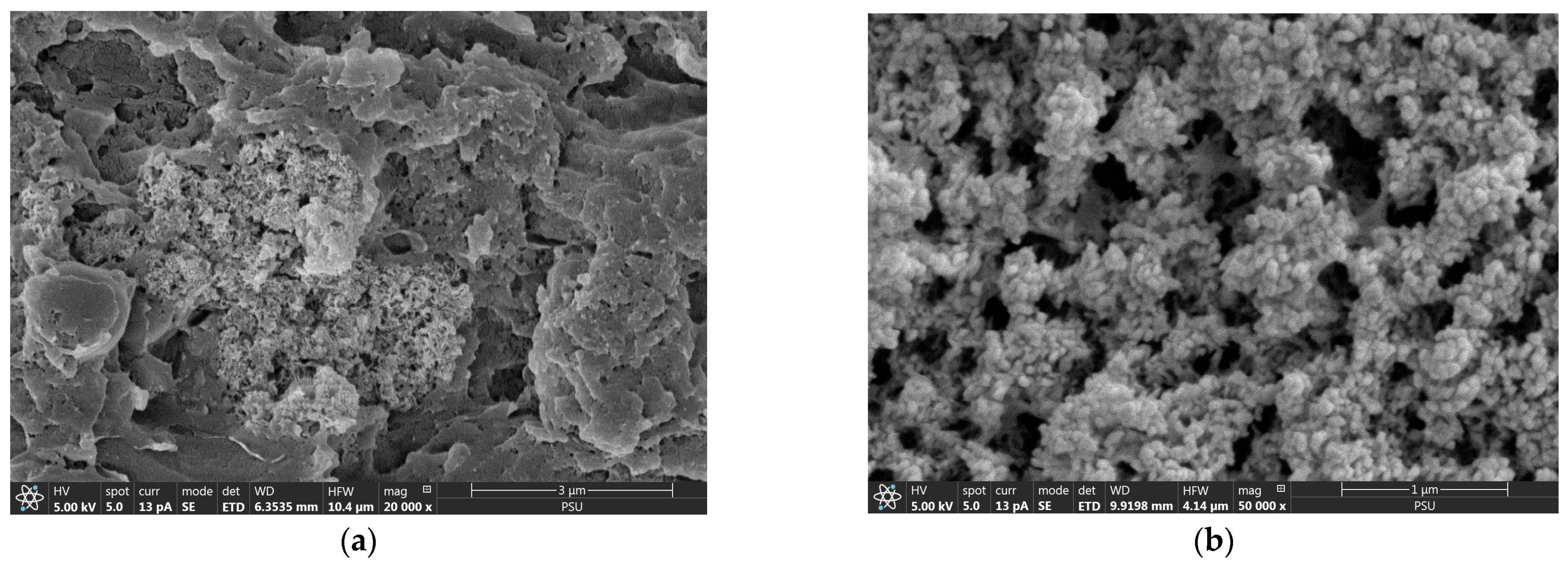
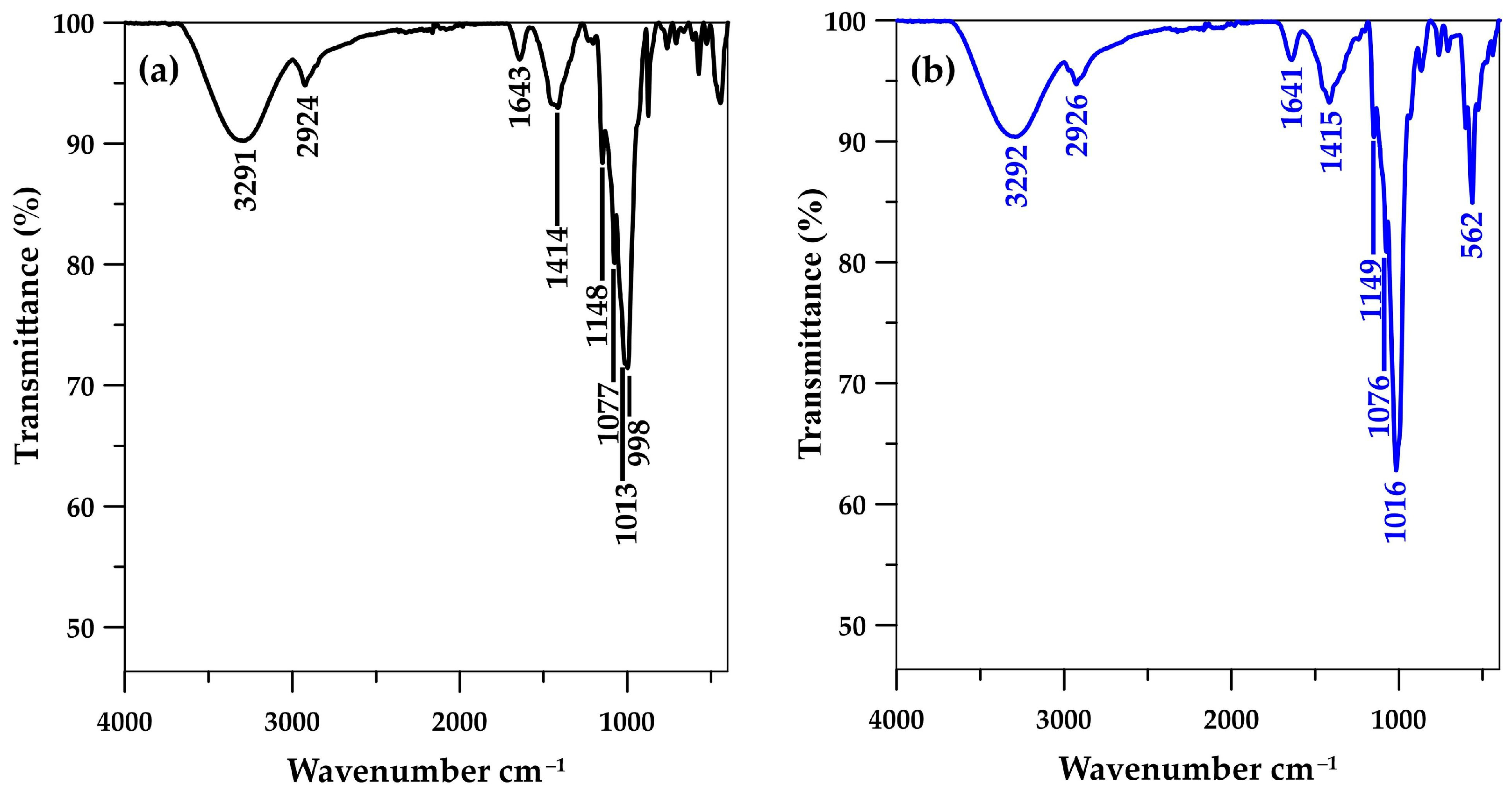
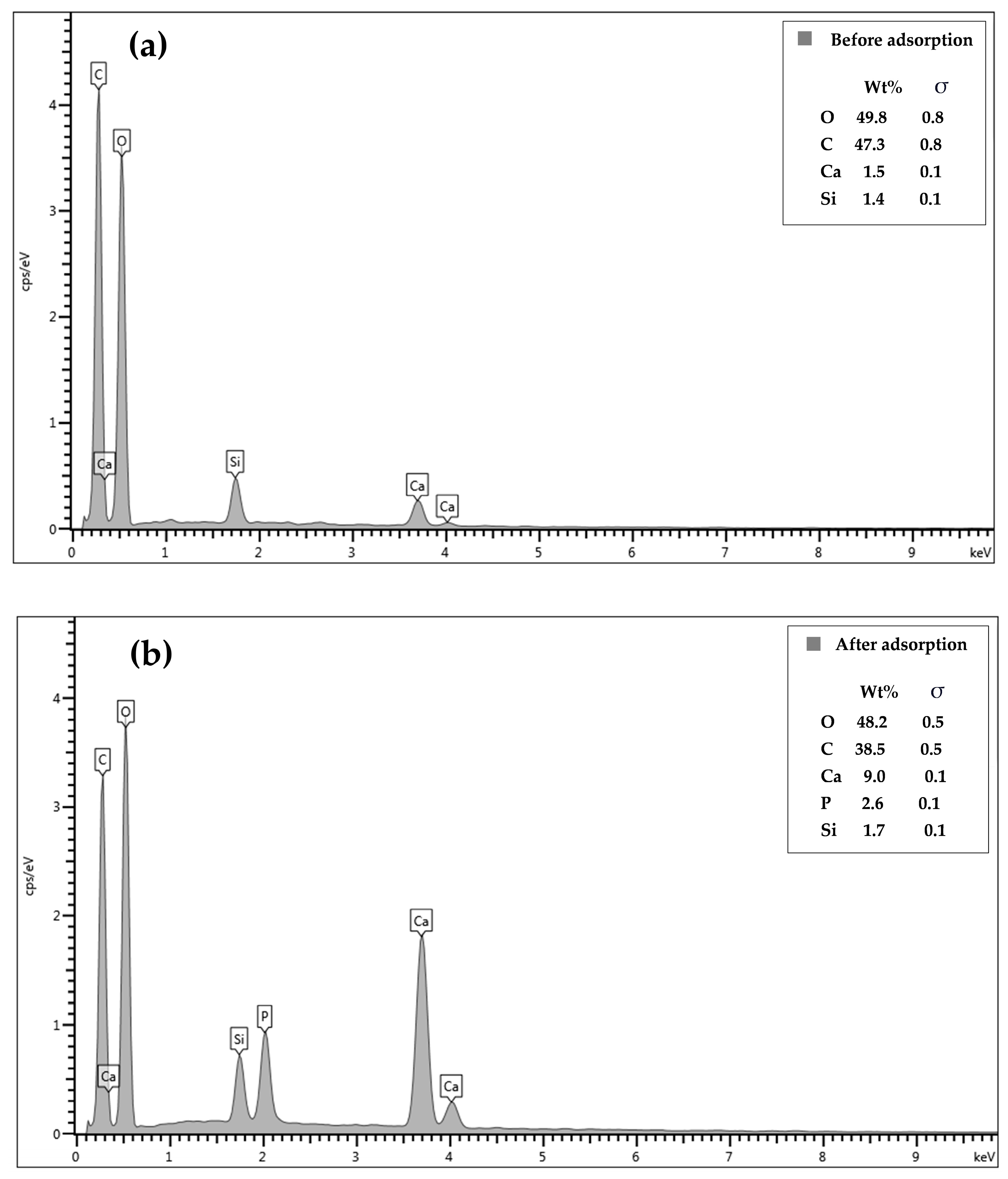
3.1. Characterization of Phosphate Adsorption on CSH Column
3.2. Influence of Continuous-Flow Parameters on Phosphate Adsorption
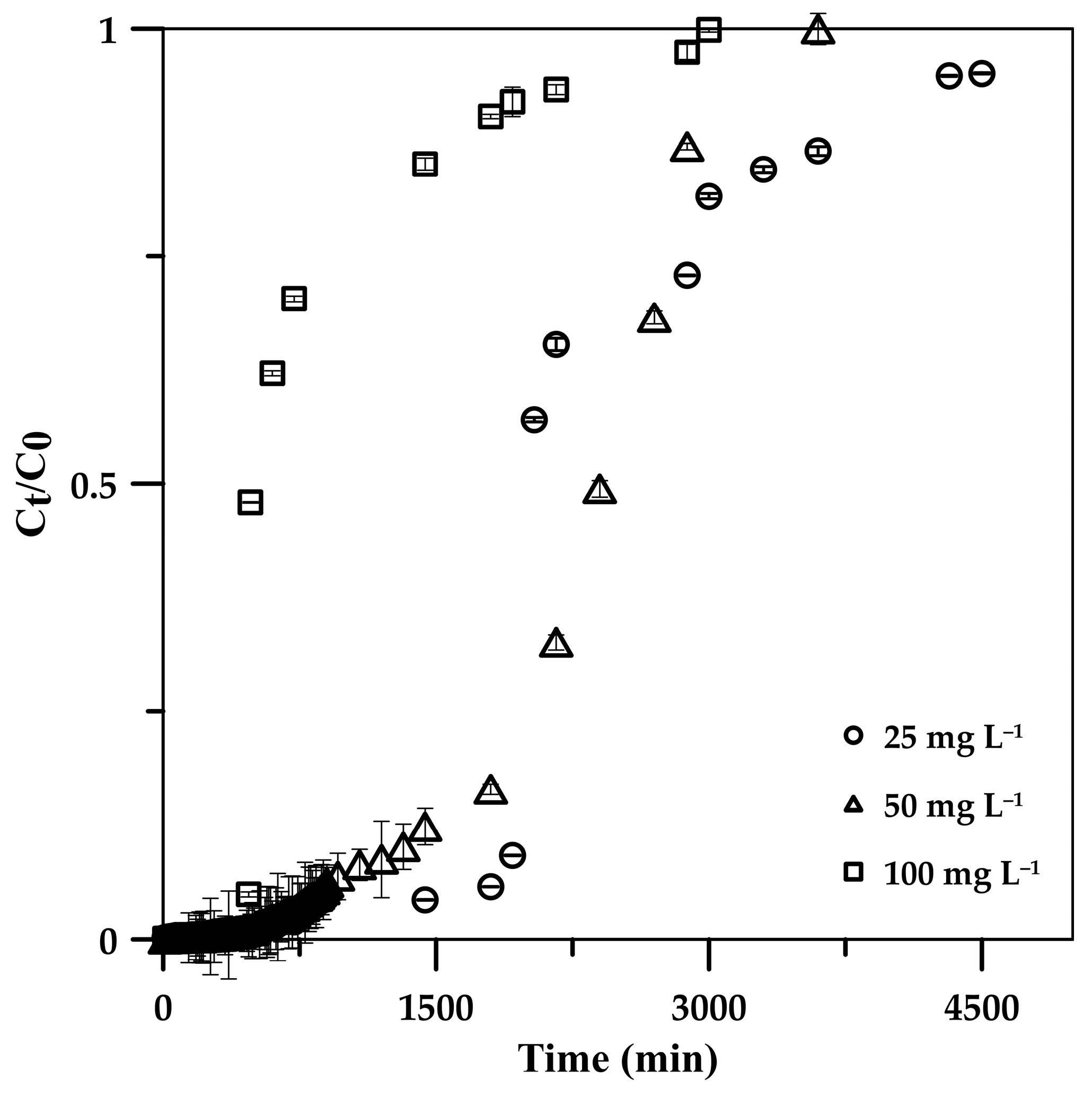
3.2.1. Initial Concentration
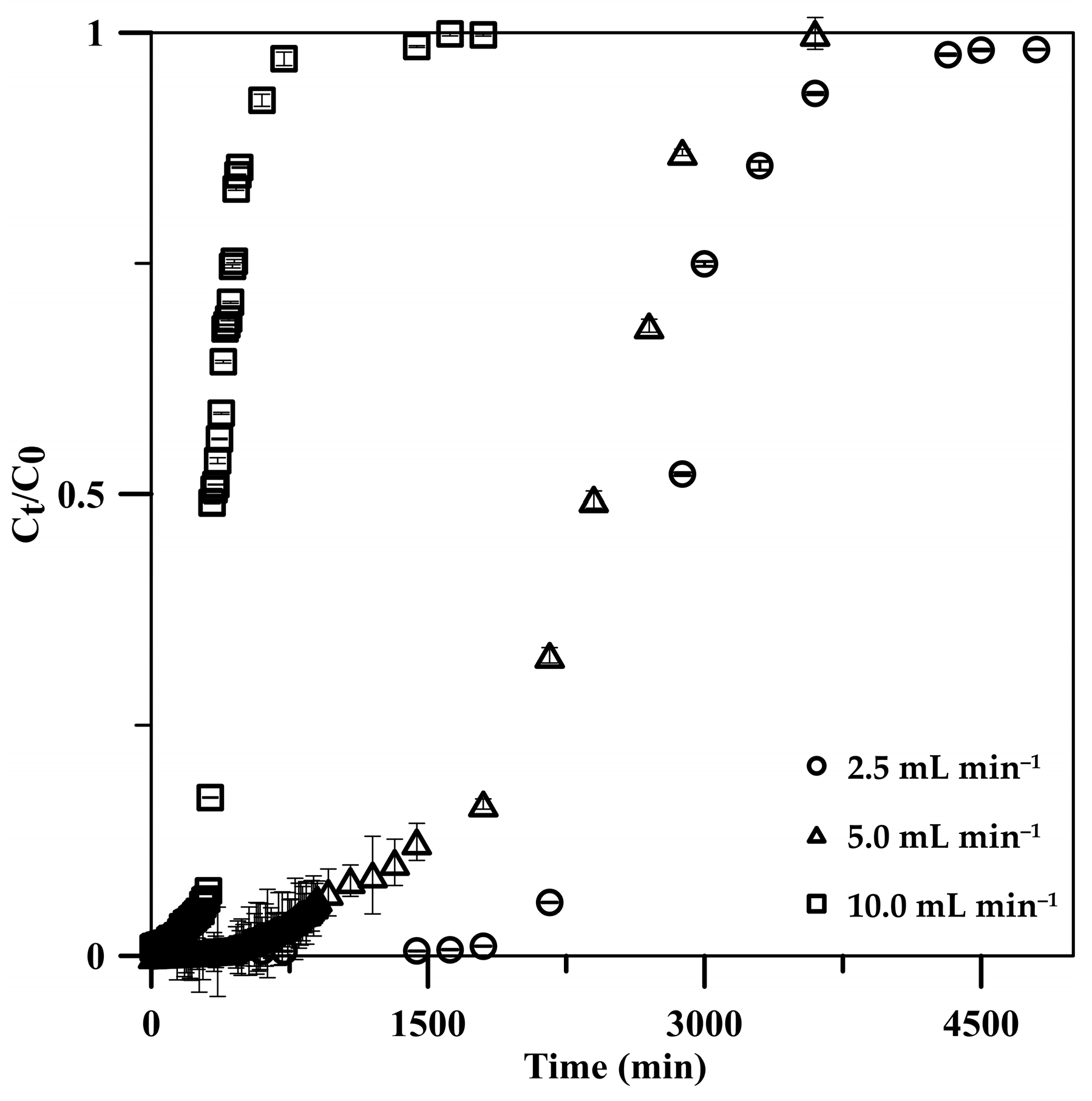
3.2.2. Influent Flow Rate
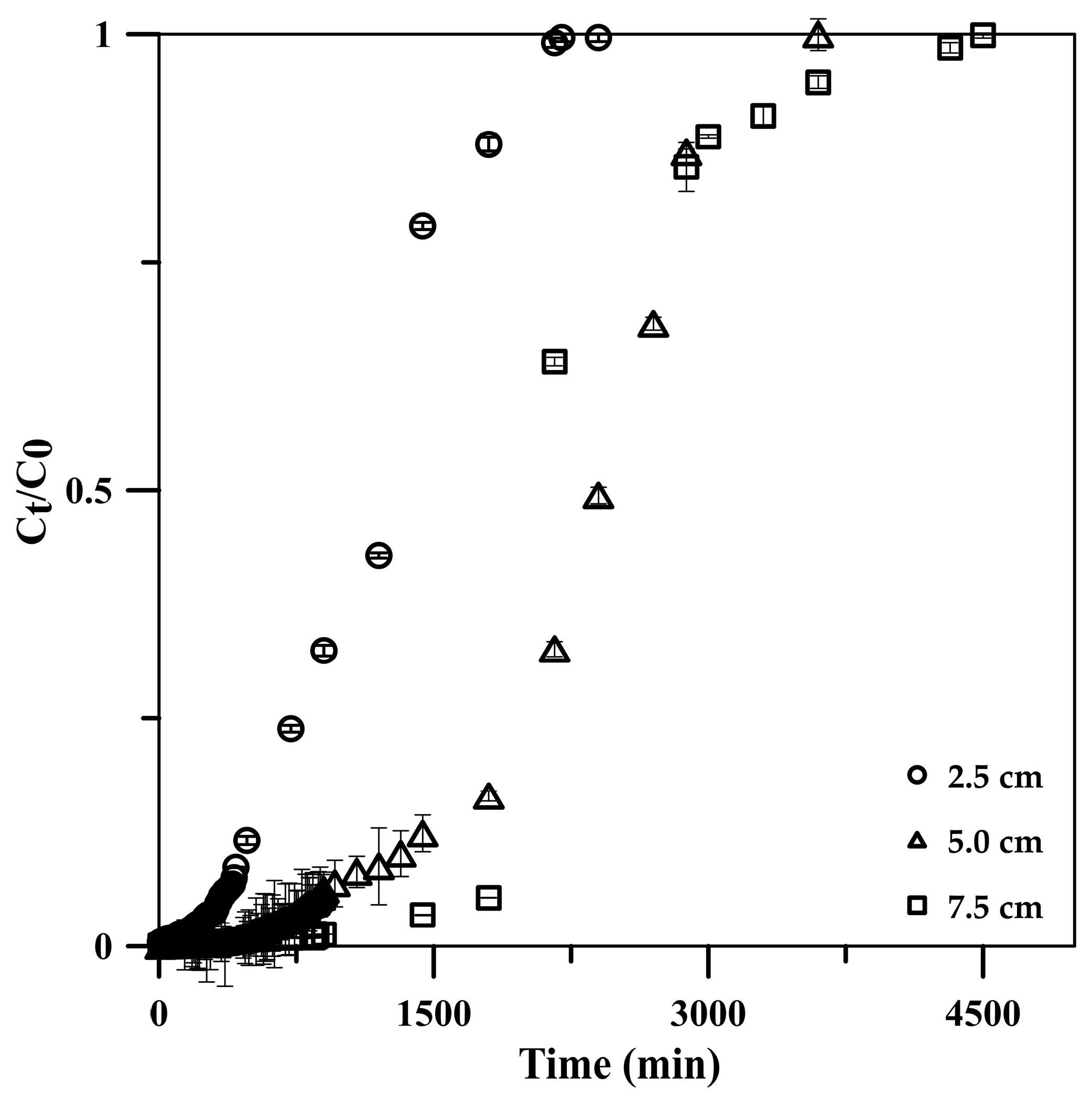
3.2.3. Column Height
3.3. Column Adsorption Dynamics
3.3.1. Adams–Bohart Model Application
3.3.2. Yoon–Nelson Model Application
3.4. Influence of Competing Ions on Phosphate Adsorption
3.5. Phosphate Recovery from Complex Wastewater Samples
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Alewell, C.; Ringeval, B.; Ballabio, C.; Robinson, D.A.; Panagos, P.; Borrelli, P. Global phosphorus shortage will be aggravated by soil erosion. Nat. Commun. 2020, 11, 4546. [Google Scholar] [CrossRef] [PubMed]
- Geissler, B.; Mew, M.C.; Weber, O.; Steiner, G. Efficiency performance of the world’s leading corporations in phosphate rock mining. Resour. Conserv. Recycl. 2015, 105, 246–258. [Google Scholar] [CrossRef]
- Panagos, P.; Köningner, J.; Ballabio, C.; Liakos, L.; Muntwyler, A.; Borrelli, P.; Lugato, E. Improving the phosphorus budget of European agricultural soils. Sci. Total Environ. 2022, 853, 158706. [Google Scholar] [CrossRef] [PubMed]
- Chrispim, M.C.; Scholz, M.; Nolasco, M.A. Phosphorus recovery from municipal wastewater treatment: Critical review of challenges and opportunities for developing countries. J. Environ. Manag. 2019, 248, 109268. [Google Scholar] [CrossRef] [PubMed]
- Cordell, D.; White, S. Tracking phosphorus security: Indicators of phosphorus vulnerability in the global food system. Food Secur. 2015, 7, 337–350. [Google Scholar] [CrossRef]
- Blackwell, M.; Darch, T.; Haslam, R. Phosphorus use efficiency and fertilizers: Future opportunities for improvements. Front. Agric. Sci. Eng. 2019, 6, 332–340. [Google Scholar] [CrossRef]
- Cordell, D.; White, S. Life’s Bottleneck: Sustaining the World’s Phosphorus for a Food Secure Future. Annu. Rev. Environ. Resour. 2014, 39, 161–188. [Google Scholar] [CrossRef]
- Reijnders, L. Phosphorus resources, their depletion and conservation, a review. Resour. Conserv. Recycl. 2014, 93, 32–49. [Google Scholar] [CrossRef]
- Cornel, P.; Schaum, C. Phosphorus recovery from wastewater: Needs, technologies and costs. Water Sci. Technol. 2009, 59, 1069–1076. [Google Scholar] [CrossRef]
- Peng, L.; Dai, H.; Wu, Y.; Peng, Y.; Lu, X. A comprehensive review of phosphorus recovery from wastewater by crystallization processes. Chemosphere 2018, 197, 768–781. [Google Scholar] [CrossRef]
- Yesigat, A.; Worku, A.; Mekonnen, A.; Bae, W.; Feyisa, G.L.; Gatew, S.; Han, J.-L.; Liu, W.; Wang, A.; Guadie, A. Phosphorus recovery as K-struvite from a waste stream: A review of influencing factors, advantages, disadvantages and challenges. Environ. Res. 2022, 214, 114086. [Google Scholar] [CrossRef] [PubMed]
- Sniatala, B.; Kurniawan, T.A.; Sobotka, D.; Makinia, J.; Othman, M.H.D. Macro-nutrients recovery from liquid waste as a sustainable resource for production of recovered mineral fertilizer: Uncovering alternative options to sustain global food security cost-effectively. Sci. Total Environ. 2023, 856, 159283. [Google Scholar] [CrossRef] [PubMed]
- Khaing, K.T.; Polprasert, C.; Mahasandana, S.; Pimpeach, W.; Patthanaissaranukool, W.; Polprasert, S. Phosphorus Recovery and Bioavailability from Chemical Extraction of Municipal Wastewater Treatment’s Waste Activated Sludge: A Case of Bangkok Metropolis, Thailand. Environ. Nat. Resour. J. 2022, 22, 369–378. [Google Scholar] [CrossRef]
- Cañas, J.; Álvarez-Torrellas, S.; Hermana, B.; García, J. Phosphorus Recovery from Sewage Sludge as Struvite. Water 2023, 15, 2382. [Google Scholar] [CrossRef]
- Atienza–Martínez, M.; Gea, G.; Arauzo, J.; Kersten, S.R.A.; Kootstra, A.M.J. Phosphorus recovery from sewage sludge char ash. Biomass-Bioenergy 2014, 65, 42–50. [Google Scholar] [CrossRef]
- McConville, J.R.; Kvarnström, E.; Jönsson, H.; Kärrman, E.; Johansson, M. Source separation: Challenges & opportunities for transition in the swedish wastewater sector. Resour. Conserv. Recycl. 2017, 120, 144–156. [Google Scholar] [CrossRef]
- Santiviago, C.; Peralta, J.; López, I. Phosphorus removal from wastewater through struvite crystallization in a continuous fluidized-bed reactor: An improved comprehensive model. Chem. Eng. J. 2022, 430, 132903. [Google Scholar] [CrossRef]
- Chen, X.; Kong, H.; Wu, D.; Wang, X.; Lin, Y. Phosphate removal and recovery through crystallization of hydroxyapatite using xonotlite as seed crystal. J. Environ. Sci. 2009, 21, 575–580. [Google Scholar] [CrossRef]
- Fang, C.; Zhang, T.; Jiang, R.; Ohtake, H. Phosphate enhance recovery from wastewater by mechanism analysis and optimization of struvite settleability in fluidized bed reactor. Sci. Rep. 2016, 6, 32215. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, X.; Zhao, J. Phosphate recovery from wastewater using calcium silicate hydrate (C-S-H): Sonochemical synthesis and properties. Environ. Sci. Water Res. Technol. 2019, 5, 131–139. [Google Scholar] [CrossRef]
- Okano, K.; Uemoto, M.; Kagami, J.; Miura, K.; Aketo, T.; Toda, M.; Honda, K.; Ohtake, H. Novel technique for phosphorus recovery from aqueous solutions using amorphous calcium silicate hydrates (A-CSHs). Water Res. 2013, 47, 2251–2259. [Google Scholar] [CrossRef] [PubMed]
- Liu, X.; Shen, F.; Qi, X. Adsorption recovery of phosphate from aqueous solution by CaO-biochar composites prepared from eggshell and rice straw. Sci. Total Environ. 2019, 666, 694–702. [Google Scholar] [CrossRef] [PubMed]
- Taweekarn, T.; Wongniramaikul, W.; Choodum, A. Removal and recovery of phosphate using a novel calcium silicate hydrate composite starch cryogel. J. Environ. Manag. 2022, 301, 113923. [Google Scholar] [CrossRef] [PubMed]
- Phawachalotorn, C.; Wongniramaikul, W.; Taweekarn, T.; Kleangklao, B.; Pisitaro, W.; Limsakul, W.; Sriprom, W.; Towanlong, W.; Choodum, A. Continuous phosphate removal and recovery using a calcium silicate hydrate composite monolithic cryogel column. Polymers 2023, 15, 539. [Google Scholar] [CrossRef] [PubMed]
- Miyazato, T.; Nuryono, N.; Kobune, M.; Rusdiarso, B.; Otomo, R.; Kamiya, Y. Phosphate recovery from an aqueous solution through adsorption-desorption cycle over thermally treated activated carbon. J. Water Process Eng. 2020, 36, 101302. [Google Scholar] [CrossRef]
- Martin, E.; Lalley, J.; Wang, W.; Nadagouda, M.N.; Sahle-Demessie, E.; Chae, S.-R. Phosphate recovery from water using cellulose enhanced magnesium carbonate pellets: Kinetics, isotherms, and desorption. Chem. Eng. J. 2018, 352, 612–624. [Google Scholar] [CrossRef]
- Ogata, F.; Nagai, N.; Iijima, S.; Toda, M.; Otani, M.; Nakamura, T.; Kawasaki, N. Exploiting the different parameters on the adsorption of phosphate ions and its subsequent recovery using complex nickel–aluminum–zirconium hydroxide. Chem. Pharm. Bull. 2021, 69, 789–795. [Google Scholar] [CrossRef]
- Peng, L.; Dai, H.; Wu, Y.; Dai, Z.; Li, X.; Lu, X. Performance and adsorption mechanism of a magnetic calcium silicate hydrate composite for phosphate removal and recovery. Water Sci. Technol. 2018, 2017, 578–591. [Google Scholar] [CrossRef]
- Guan, W.; Ji, F.; Chen, Q.; Yan, P.; Zhang, Q. Preparation and phosphorus recovery performance of porous calcium–silicate–hydrate. Ceram. Int. 2013, 39, 1385–1391. [Google Scholar] [CrossRef]
- American Public Health, APHA. Ascorbic acid method. In Standard Method for the Examination of Water and Wastewater, 20th ed.; American Public Health Association, American Water Works Association and Water Environmental Federation: Washington, DC, USA, 1998; Available online: https://www.scirp.org/reference/ReferencesPapers?ReferenceID=1909322 (accessed on 29 November 2023).
- Boonkanon, C.; Phatthanawiwat, K.; Chuenchom, L.; Lamthornkit, N.; Taweekarn, T.; Wongniramaikul, W.; Choodum, A. Preparation and characterization of calcium cross-linked starch monolithic cryogels and their application as cost-effective green filters. Polymers 2021, 13, 3975. [Google Scholar] [CrossRef]
- Taweekarn, T.; Wongniramaikul, W.; Sriprom, W.; Limsakul, W.; Choodum, A. Continuous-flow system for methylene blue removal using a green and cost-effective starch single-rod column. Polymers 2023, 15, 3989. [Google Scholar] [CrossRef] [PubMed]
- Thomas, H.C. Heterogeneous Ion Exchange in a Flowing System. J. Am. Chem. Soc. 1944, 66, 1664–1666. [Google Scholar] [CrossRef]
- Patel, H. Fixed-bed column adsorption study: A comprehensive review. Appl. Water Sci. 2019, 9, 45. [Google Scholar] [CrossRef]
- Bohart, G.S.; Adams, E.Q. Some aspects of the behavior of charcoal with respect to chlorine. J. Am. Chem. Soc. 1920, 42, 523–544. [Google Scholar] [CrossRef]
- Yoon, Y.H.; Nelson, J.H. Application of gas adsorption kinetics I. A theoretical model for respirator cartridge service life. Am. Ind. Hyg. Assoc. J. 1984, 45, 509–516. [Google Scholar] [CrossRef] [PubMed]
- Yagub, M.T.; Sen, T.K.; Afroze, S.; Ang, H.M. Fixed-bed dynamic column adsorption study of methylene blue (MB) onto pine cone. Desalination Water Treat. 2015, 55, 1026–1039. [Google Scholar] [CrossRef]
- Kapur, M.; Mondal, M.K. Design and model parameters estimation for fixed–bed column adsorption of Cu(II) and Ni(II) ions using magnetized saw dust. Desalination Water Treat. 2016, 57, 12192–12203. [Google Scholar] [CrossRef]
- Esmaeili, H.; Foroutan, R.; Jafari, D.; Rezaei, M.A. Effect of interfering ions on phosphate removal from aqueous media using magnesium oxide@ferric molybdate nanocomposite. Korean J. Chem. Eng. 2020, 37, 804–814. [Google Scholar] [CrossRef]
- Guan, W.; Zhao, X. Fluoride recovery using porous calcium silicate hydrates via spontaneous Ca2+ and OH− release. Sep. Purif. Technol. 2016, 165, 71–77. [Google Scholar] [CrossRef]
- Ding, S.; Fang, D.; Pang, Z.; Luo, B.; Kuang, L.; Wang, H.; Zhang, Q.; Shen, Q.; Ji, F. Immobilization of powdery calcium silicate hydrate via PVA covalent cross-linking process for phosphorus removal. Sci. Total Environ. 2018, 645, 937–945. [Google Scholar] [CrossRef]
- Boonkanon, C.; Phatthanawiwat, K.; Wongniramaikul, W.; Choodum, A. Curcumin nanoparticle doped starch thin film as a green colorimetric sensor for detection of boron. Spectrochim. Acta Part A Mol. Biomol. Spectrosc. 2020, 224, 117351. [Google Scholar] [CrossRef] [PubMed]
- Wongniramaikul, W.; Limsakul, W.; Choodum, A. A biodegradable colorimetric film for rapid low-cost field determination of formaldehyde contamination by digital image colorimetry. Food Chem. 2018, 249, 154–161. [Google Scholar] [CrossRef] [PubMed]
- Woumfo, E.D.; Siéwé, J.M.; Njopwouo, D. A fixed-bed column for phosphate removal from aqueous solutions using an andosol-bagasse mixture. J. Environ. Manag. 2015, 151, 450–460. [Google Scholar] [CrossRef] [PubMed]
- Afroze, S.; Sen, T.K.; Ang, H.M. Adsorption performance of continuous fixed bed column for the removal of methylene blue (MB) dye using Eucalyptus sheathiana bark biomass. Res. Chem. Intermed. 2016, 42, 2343–2364. [Google Scholar] [CrossRef]
- Ahmad, A.A.; Hameed, B.H. Fixed-bed adsorption of reactive azo dye onto granular activated carbon prepared from waste. J. Hazard. Mater. 2010, 175, 298–303. [Google Scholar] [CrossRef] [PubMed]
- Ramirez, A.; Giraldo, S.; García-Nunez, J.; Flórez, E.; Acelas, N. Phosphate removal from water using a hybrid material in a fixed-bed column. J. Water Process Eng. 2018, 26, 131–137. [Google Scholar] [CrossRef]
- Sadaf, S.; Bhatti, H.N. Evaluation of peanut husk as a novel, low cost biosorbent for the removal of indosol orange RSN dye from aqueous solutions: Batch and fixed bed studies. Clean Technol. Environ. Policy 2014, 16, 527–544. [Google Scholar] [CrossRef]
- Aksu, Z.; Gönen, F. Biosorption of phenol by immobilized activated sludge in a continuous packed bed: Prediction of breakthrough curves. Process Biochem. 2004, 39, 599–613. [Google Scholar] [CrossRef]
- Lv, N.; Li, X. Phosphorus removal from wastewater using Ca-modified attapulgite: Fixed-bed column performance and breakthrough curves analysis. J. Environ. Manag. 2023, 328, 116905. [Google Scholar] [CrossRef]
- Auta, M.; Hameed, B.H. Chitosan–clay composite as highly effective and low-cost adsorbent for batch and fixed-bed adsorption of methylene blue. Chem. Eng. J. 2014, 237, 352–361. [Google Scholar] [CrossRef]
- Calero, M.; Hernáinz, M.; Blázquez, G.; Tenorio, G.; Martín-Lara, M.A. Study of Cr (III) biosorption in a fixed-bed column. J. Hazard. Mater. 2009, 171, 886–893. [Google Scholar] [CrossRef] [PubMed]
- Cheng, J.; Zhan, C.; Wu, J.; Cui, Z.; Si, J.; Wang, Q.; Peng, X.; Turng, L.-S. Highly efficient removal of methylene blue dye from an aqueous solution using cellulose acetate nanofibrous membranes modified by polydopamine. ACS Omega 2020, 5, 5389–5400. [Google Scholar] [CrossRef] [PubMed]
- Mouni, L.; Belkhiri, L.; Bollinger, J.-C.; Bouzaza, A.; Assadi, A.; Tirri, A.; Dahmoune, F.; Madani, K.; Remini, H. Removal of methylene blue from aqueous solutions by adsorption on kaolin: Kinetic and equilibrium studies. Appl. Clay Sci. 2018, 153, 38–45. [Google Scholar] [CrossRef]
- Lee, C.-G.; Alvarez, P.J.J.; Kim, H.-G.; Jeong, S.; Lee, S.; Lee, K.B.; Lee, S.-H.; Choi, J.-W. Phosphorous recovery from sewage sludge using calcium silicate hydrates. Chemosphere 2018, 193, 1087–1093. [Google Scholar] [CrossRef]

| No. | Equation | Definition |
|---|---|---|
| (1) | Veff is the effluent volume (mL), Q is the volumetric flow rate (mL min−1), and Ttotal is the total flow time (min); | |
| (2) | qtotal is the total mass of adsorbed, and Cad is the concentration of phosphate removal (mg L−1); | |
| (3) | qe is the equilibrium uptake or maximum adsorption capacity at equilibrium, and M is the dry weight of adsorbent in the column (g); | |
| (4) | mtotal is total number of phosphate ions entering column; | |
| (5) | R% is the recovery percentage (%); | |
| (6) | KAB (L mg−1 min−1) is the Bohart–Adams rate constant, N0 (mg L−1) is saturation concentration in column, Z (cm) is the height of monolithic column, and U (cm min−1) is linear rate and is calculated by dividing the flow rate (mL min−1) by cross sectional area of the column (cm2). The values of KAB and N0 are calculated from linear plot of vs. time, which are obtained from the slope and the intercept, respectively. | |
| (7) | KYN is Yoon–Nelson of rate constant (min−1), and τ is the time required for 50% adsorbate breakthrough (min). The values of kYN and τ can be estimated from the slope and the intercept from the linear plot of vs. time. |
| Condition/Parameter | Tb * (min) | Ttotal (min) | Veff (mL) | qtotal (mg) | qe (mg g−1) | RE (%) |
|---|---|---|---|---|---|---|
| Initial conc. (mg L−1) ** | ||||||
| 25 | 1920 | 4500 | 22,500 | 561.6 | 115.2 | 99.84 |
| 50 | 1320 | 3600 | 18,000 | 899.8 | 185.7 | 99.98 |
| 100 | 480 | 3000 | 15,000 | 1499.2 | 308.5 | 99.94 |
| Flow rate (mL min−1) *** | ||||||
| 2.5 | 2160 | 4800 | 12,000 | 600.0 | 123.7 | 99.99 |
| 5.0 | 1320 | 3600 | 18,000 | 899.8 | 185.7 | 99.98 |
| 10.0 | 320 | 1620 | 16,200 | 802.9 | 165.4 | 99.13 |
| Column height (cm) **** | ||||||
| 2.5 | 720 | 2400 | 12,000 | 599.9 | 164.2 | 99.99 |
| 5.0 | 1320 | 3600 | 18,000 | 899.8 | 185.7 | 99.98 |
| 7.5 | 2160 | 4800 | 24,000 | 1199.7 | 180.7 | 99.98 |
| Parameter/Model | Adams–Bohart | Yoon–Nelson | ||||
|---|---|---|---|---|---|---|
| KAB × 10−5 (L mg−1 min−1) | N0 (mg L−1) | R2 | KYN (min−1) | τ (min) | R2 | |
| Initial conc. (mg L−1) | ||||||
| 25 | 8.4 | 13,646 | 0.8899 | 0.0021 | 2669 | 0.9634 |
| 50 | 8.0 | 17,276 | 0.8543 | 0.0035 | 1870 | 0.8965 |
| 100 | 7.6 | 20,282 | 0.7994 | 0.0046 | 1443 | 0.8608 |
| Flow rate (mL min−1) | ||||||
| 2.5 | 4.0 | 34,936 | 0.6320 | 0.0024 | 2915 | 0.9235 |
| 5.0 | 8.0 | 17,276 | 0.8543 | 0.0035 | 1870 | 0.8965 |
| 10 | 16.4 | 12,231 | 0.8599 | 0.0072 | 546 | 0.7723 |
| Column height (cm) | ||||||
| 2.5 | 13.4 | 16,510 | 0.6916 | 0.0039 | 1232 | 0.8821 |
| 5.0 | 8.0 | 17,276 | 0.8543 | 0.0035 | 1870 | 0.8965 |
| 7.5 | 3.8 | 22,528 | 0.8794 | 0.0024 | 2701 | 0.9643 |
| Sample | Tb (min) | Ttotal (min) | Veff (mL) | qtotal (mg) | mtotal (mg) | qe (mg g−1) | RE (%) |
|---|---|---|---|---|---|---|---|
| PO43− (50 mg L−1) | 1320 | 3600 | 18,000 | 899.8 | 900.0 | 185.0 | 99.99 |
| PO43− + aCO32− | 10 | 90 | 450 | 20.8 | 22.5 | 4.4 | 92.46 |
| PO43− + bCO32− | 30 | 180 | 900 | 43.1 | 45.0 | 9.2 | 96.15 |
| PO43− + cCO32− | 420 | 1000 | 5000 | 246.4 | 250.0 | 52.4 | 98.57 |
| PO43− + SO42− + dNO3− | 440 | 3600 | 18,000 | 890.8 | 900.0 | 184.45 | 98.99 |
| Sample | PO43− Conc. (mg L−1) | Tb (min) | Ttotal (min) | Veff (mL) | qtotal (mg) | qe (mg g−1) | RE (%) |
|---|---|---|---|---|---|---|---|
| Synthetic laundry wastewater | 4.01 | 10 | 200 | 1000 | 3.37 | 0.70 | 84.04 |
| Household laundry wastewater | 1.84 | 10 | 450 | 2250 | 3.42 | 0.71 | 82.82 |
| Household + PO43− | 51.84 | 30 | 360 | 1800 | 87.42 | 18.02 | 91.74 |
| RO concentrate | 26.46 | 10 | 120 | 600 | 15.49 | 3.20 | 97.58 |
| RO concentrate + PO43− | 51.46 | 540 | 1620 | 8100 | 404.63 | 86.09 | 99.91 |
| PO43− | 50 | 1320 | 3600 | 18,000 | 899.8 | 185.0 | 98.99 |
| Temperature (°C) | Tb (min) | Ttotal (min) | Veff (mL) | qtotal (mg) | mtotal (mg) | qe (mg g−1) | RE (%) |
|---|---|---|---|---|---|---|---|
| 25 | 10 | 450 | 2250 | 3.42 | 4.13 | 0.71 | 82.82 |
| 35 | 30 | 480 | 2400 | 5.06 | 6.00 | 1.07 | 84.35 |
| 45 | 30 | 480 | 2400 | 5.29 | 6.12 | 1.12 | 86.42 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Taweekarn, T.; Wongniramaikul, W.; Roop-o, P.; Towanlong, W.; Choodum, A. Recovering Phosphate from Complex Wastewater Using Macroporous Cryogel Composited Calcium Silicate Hydrate Nanoparticles. Molecules 2024, 29, 228. https://doi.org/10.3390/molecules29010228
Taweekarn T, Wongniramaikul W, Roop-o P, Towanlong W, Choodum A. Recovering Phosphate from Complex Wastewater Using Macroporous Cryogel Composited Calcium Silicate Hydrate Nanoparticles. Molecules. 2024; 29(1):228. https://doi.org/10.3390/molecules29010228
Chicago/Turabian StyleTaweekarn, Tarawee, Worawit Wongniramaikul, Pariyaporn Roop-o, Wanchitra Towanlong, and Aree Choodum. 2024. "Recovering Phosphate from Complex Wastewater Using Macroporous Cryogel Composited Calcium Silicate Hydrate Nanoparticles" Molecules 29, no. 1: 228. https://doi.org/10.3390/molecules29010228
APA StyleTaweekarn, T., Wongniramaikul, W., Roop-o, P., Towanlong, W., & Choodum, A. (2024). Recovering Phosphate from Complex Wastewater Using Macroporous Cryogel Composited Calcium Silicate Hydrate Nanoparticles. Molecules, 29(1), 228. https://doi.org/10.3390/molecules29010228