Coalescence and Break-Up Behaviors of Nanodroplets under AC Electric Field
Abstract
1. Introduction
2. Molecular Simulation Details
3. Results and Discussion
3.1. Dynamic Coalescence Process of Nanodroplets
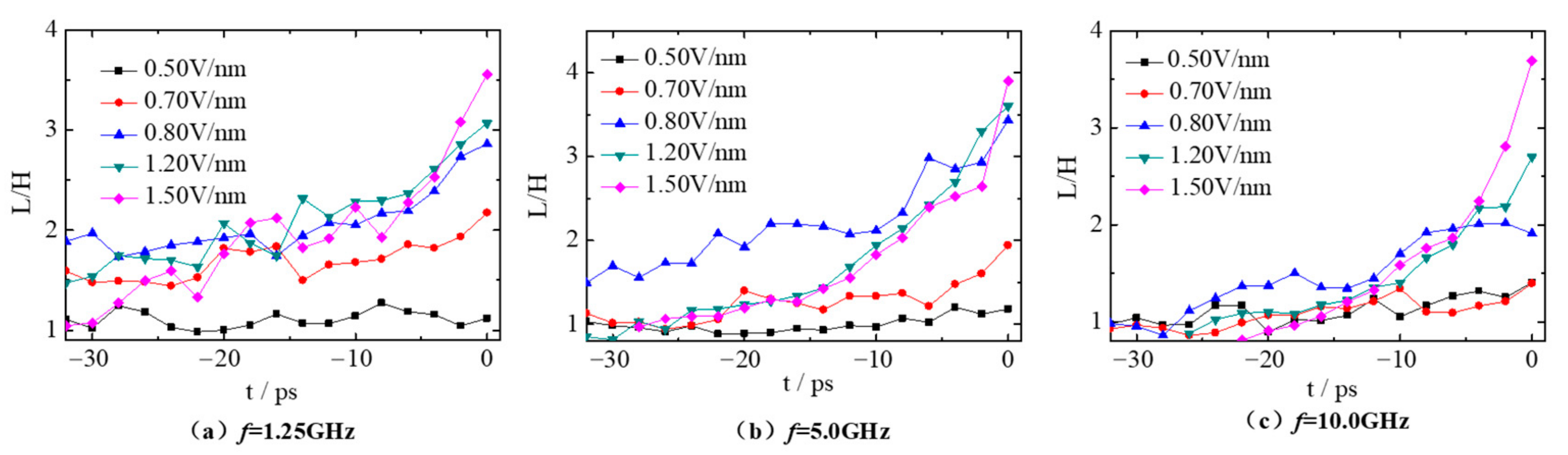
3.2. Parameter Analysis
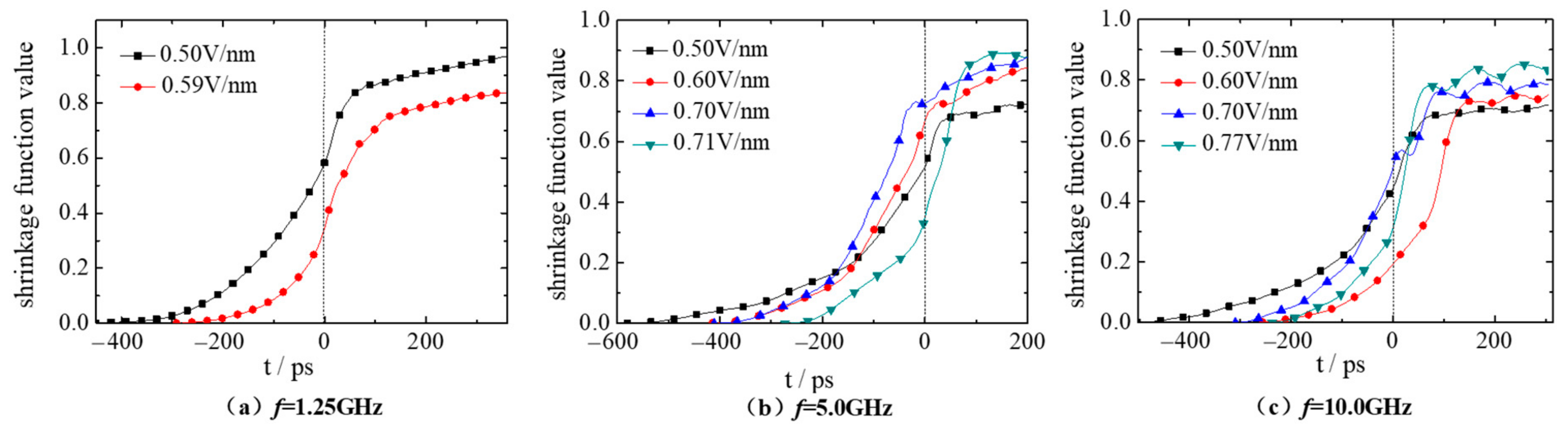
3.3. Oscillation Phenomenon after Coalescence
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gong, H.; Li, W.; Zhang, X.; Peng, Y.; Yu, B.; Mou, Y. Simulation of the coalescence and breakup of water-in-oil emulsion in a separation device strengthened by coupling electric and swirling centrifugal fields. Sep. Purif. Technol. 2020, 238, 116397. [Google Scholar] [CrossRef]
- Luo, X.; Gong, H.; Cao, J.; Yin, H.; Yan, Y.; He, L. Enhanced separation of water-in-oil emulsions using ultrasonic standing waves. Chem. Eng. Sci. 2019, 203, 285–292. [Google Scholar] [CrossRef]
- Binner, E.R.; Robinson, J.P.; Silvester, S.A.; Kingman, S.W.; Lester, E.H. Investigation into the mechanisms by which microwave heating enhances separation of water-in-oil emulsions. Fuel 2014, 116, 516–521. [Google Scholar] [CrossRef]
- Aryafar, H.; Kavehpour, H.P. Electrocoalescence: Effects of DC electric fields on coalescence of drops at planar interfaces. Langmuir 2009, 25, 12460–124465. [Google Scholar] [CrossRef]
- Alberini, F.; Dapelo, D.; Enjalbert, R.; Crombrugge, Y.V.; Simmons, J.H. Influence of DC electric field upon the production of oil-in-water-in-oil double emulsions in upwards mm-scale channels at low electric field strength. Exp. Therm. Fluid Sci. 2017, 81, 265–276. [Google Scholar] [CrossRef]
- Chen, G.; Tan, P.; Chen, S.; Wen, W.; Xu, L. Coalescence of Pickering emulsion droplets induced by an electric field. Phys. Rev. Lett. 2013, 110, 064502. [Google Scholar] [CrossRef]
- He, L.M.; Yang, D.H.; Gong, R.N.; Ye, T.; Lu, Y.; Luo, X. An investigation into the deformation, movement and coalescence characteristics of water-in-oil droplets in an AC electric field. Pet. Sci. 2013, 10, 548–561. [Google Scholar] [CrossRef]
- Suhwan, C.; Saveliev, A.V. Oscillatory coalescence of droplets in an alternating electric field. Phys. Rev. Fluids 2017, 2, 063603. [Google Scholar]
- Zhang, Y.Z.; Liu, Y.H.; Ji, R. Dehydration efficiency of high-frequency pulsed DC electrical fields on water-in-oil emulsion. Colloids Surf. A Physicochem. Eng. Asp. 2011, 373, 130–137. [Google Scholar] [CrossRef]
- Abdullah, M.; Ezzat, A.O.; Al-Lohedan, H.A.; Aldalbahi, A.; Atta, A.M. New amphiphilic ionic liquids for the demulsification of water-in-heavy crude oil emulsion. Molecules 2022, 27, 3238. [Google Scholar] [CrossRef]
- Abdullah, M.; Al-Lohedan, H.A.; Atta, A.M. Fabrication of new demulsifiers employing the waste polyethylene terephthalate and their demulsification efficiency for heavy crude oil emulsions. Molecules 2021, 26, 589. [Google Scholar] [CrossRef]
- Liu, L.; Nicholson, D.; Bhatia, S.K. Exceptionally high performance of charged carbon nanotube arrays for CO2 separation from flue gas. Carbon 2017, 125, 245–257. [Google Scholar] [CrossRef]
- Amooey, A.A.; Amiri, E.O. Computational fluid dynamic simulation of dispersed oil-water flow with new drop coalescence model. J. Fluid Mech. 2019, 12, 119–126. [Google Scholar] [CrossRef]
- Kelbaliev, G.I.; Rasulov, S.R.; Mustafaeva, G.R. Modeling of phenomena of drop coalescence in oil emulsion breaking processes. Chem. Technol. Fuels Oil 2018, 54, 158–165. [Google Scholar] [CrossRef]
- Raj, M.D.; Rengaswamy, R. Interacting coalescence avalanches in a 2D droplet assembly. AIChE J. 2019, 65, 1111–1118. [Google Scholar]
- Bird, J.C.; Ristenpart, W.D.; Belmonte, A.; Stone, H.A. Critical angle for electrically driven coalescence of two conical droplets. Phys. Rev. Lett. 2009, 103, 164502. [Google Scholar] [CrossRef]
- Hamlin, B.S.; Creasey, J.C.; Ristenpart, W.D. Electrically tunable partial coalescence of oppositely charged drops. Phys. Rev. Lett. 2012, 109, 094501. [Google Scholar] [CrossRef]
- Guo, C.H.; He, L.H. Coalescence behaviour of two large water-drops in viscous oil under a DC electric field. J. Electrostat. 2014, 72, 470–476. [Google Scholar] [CrossRef]
- Guo, C.H.; He, L.H.; Xin, Y.C. Deformation and breakup of aqueous drops in viscous oil under a uniform AC electric field. J. Electrostat. 2015, 77, 27–34. [Google Scholar] [CrossRef]
- Muhammad, S.A.; Husnain, A.; Ali, H.K.; Tariq, N.C.; Muhammad, U.; Sheraz, A.; Muhammad, U.; Douhadji, A. Emulsion droplet pair coalescence under a direct current electric field. Math. Probl. Eng. 2022, 1382, 1–9. [Google Scholar]
- Lei, G.P.; Liu, C.; Xie, H.; Liu, J.F. Removal of hydrogen sulfide from natural gas by the graphene-nanotube hybrid structure: A molecular simulation. Chem. Phys. Lett. 2014, 616, 232–236. [Google Scholar] [CrossRef]
- Lin, Z.Z.; Liu, C.; Liu, L.; He, D.L.; Zhang, Y. Unprecedentedly high selective adsorption of Xe/Kr mixtures in carbon nanotubes: A molecular simulation study. Chem. Phys. Lett. 2020, 393, 124744. [Google Scholar] [CrossRef]
- Wang, T.; Liu, X.; Xu, J.; Afzal, W.; He, M. Simulation of softwood lignin gasification in supercritical carbon dioxide. J. CO2 Util. 2022, 59, 101959. [Google Scholar] [CrossRef]
- Liu, L.; Nicholson, D.; Bhatia, S.K. Adsorption of CH4 and CH4/CO2 mixtures in carbon nanotubes and disordered carbons: A molecular simulation study. Chem. Eng. Sci. 2015, 121, 268–278. [Google Scholar] [CrossRef]
- Lei, G.P.; Liu, C.; Li, Q.B.; Xu, X.X. Graphyne nanostructure as a potential adsorbent for separation of H2S/CH4 mixture: Combining grand canonical Monte Carlo simulations with ideal adsorbed solution theory. Fuel 2016, 182, 210–219. [Google Scholar] [CrossRef]
- Li, B.; Ju, M.D.; Dou, X.H.; Dou, X.H.; Li, N.; Zhang, W.; Xu, H.; Sun, Z.Q.; Yu, K.; Wang, J.F.; et al. Microscopic mechanism for nanoparticle-laden droplet–droplet electrocoalescence: A molecular dynamics study. Sep. Purif. Technol. 2022, 299, 121768. [Google Scholar] [CrossRef]
- Li, B.; Dou, X.H.; Yu, K.; Li, N.; Zhang, W.; Xu, H.j.; Sun, Z.Q.; Wang, Z.T.; Wang, J.F. Molecular dynamics simulations of nanoparticle-laden drop–interface electrocoalescence behaviors under direct and alternating current electric fields. J. Mol. Liq. 2021, 344, 117875. [Google Scholar] [CrossRef]
- Chen, Q.C.; Lu, K.; Zhang, Y.J.; Qin, D.; Xu, H.M.; Yang, C.; He, N. Effect of DC electric field on coalescence and breakup behaviors of binary emulsion nanodroplets. J. Mol. Liq. 2021, 323, 114939. [Google Scholar] [CrossRef]
- Li, S.; Yuan, S.; Zhang, Y.; Guo, H.; Liu, S.; Wang, D.; Wang, Y. Molecular dynamics study on the demulsification mechanism of water-in-oil emulsion with SDS surfactant under a DC electric field. Langmuir 2022, 38, 12717–12730. [Google Scholar] [CrossRef]
- Song, F.H.; Li, B.Q.; Liu, C. Molecular dynamics simulation of nanosized water droplet spreading in an electric field. Langmuir 2018, 29, 4266–4274. [Google Scholar] [CrossRef]
- Liu, X.; Zong, X.; Xue, S.; He, M. Regulating structure and flow of ionic liquid confined in nanochannel using water and electric field. J. Mol. Liq. 2022, 351, 118612. [Google Scholar] [CrossRef]
- Song, F.H.; Xue, J.Y.; Ma, B.; Fan, J.; Wang, Y.C.; Jiang, Y.H. Wetting and electro-wetting behaviors of [Bmim] [Bf4] ionic liquid droplet on lyophobic and lyophilic solid substrates. J. Mol. Liq. 2022, 347, 118405. [Google Scholar] [CrossRef]
- Liu, L.; Nicholson, D.; Bhatia, S.K. Effects of flange adsorption affinity and membrane porosity on interfacial resistance in carbon nanotube membranes. ACS Appl. Mater. Int. 2018, 10, 34706–34717. [Google Scholar] [CrossRef]
- Thompson, A.P.; Aktulga, H.M.; Berger, R.; Bolintineanu, D.S.; Brown, W.M.; Crozier, P.S.; in’tVeld, P.J.; Kohlmeyer, A.; Moore, S.G.; Nguyen, T.D.; et al. LAMMPS—A flexible simulation tool for particle-based materials modeling at the atomic, meso, and continuum scales. Comput. Phys. Commun. 2022, 271, 10817. [Google Scholar] [CrossRef]
- Beckers, J.V.L.; Lowe, C.P.; Leeuw, S.W. An Iterative PPPM method for simulating coulombic systems on distributed memory parallel computers. Mol. Simul. 1998, 20, 369–383. [Google Scholar] [CrossRef]
- Li, Q.; Ren, J.; Liu, Y.; Zhou, Y. Prediction of critical properties and boiling point of fluorine/chlorine-containing refrigerants. Int. J. Refrig. 2022, 143, 28–36. [Google Scholar] [CrossRef]
- Song, F.H.; Niu, H.; Fan, J.; Chen, Q.C.; Wang, G.; Liu, L. Molecular dynamics study on the coalescence and break-up behaviors of ionic droplets under DC electric field. J. Mol. Liq. 2020, 312, 113195. [Google Scholar] [CrossRef]
- Wang, Z.; Dong, K.; Tian, L.; Wang, J.; Tu, J. Numerical study on coalescence behavior of suspended drop pair in viscous liquid under uniform electric field. AIP Adv. 2018, 8, 085215. [Google Scholar] [CrossRef]
- Liao, M.L.; Ju, S.P.; Yang, S.H. Coalescence behavior of water nanoclusters: Temperature and size effects. J. Phys. Chem. C 2007, 111, 6927–6932. [Google Scholar] [CrossRef]
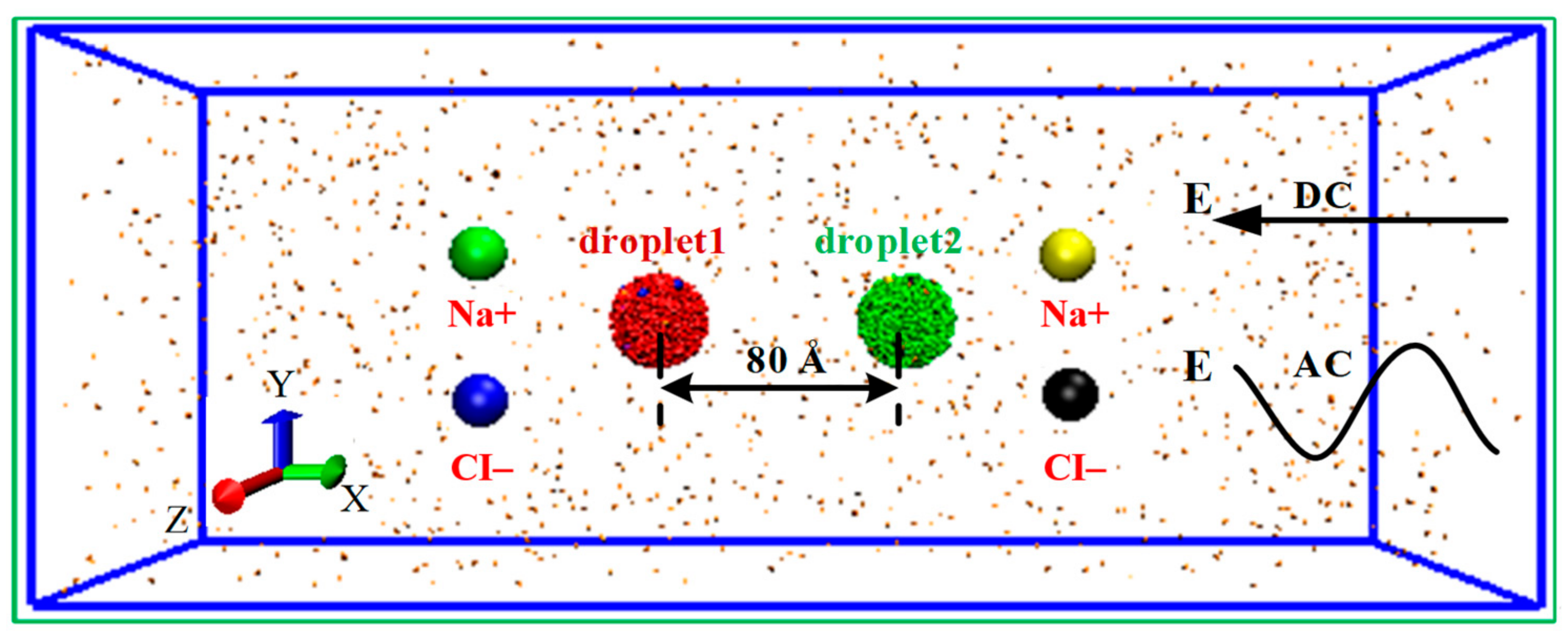



| Atom | σ (nm) | ε (kJ/mol) | q (e) |
|---|---|---|---|
| O | 0.316 | 0.6502 | −0.8476 |
| H | 0 | 0 | 0.4238 |
| Na | 0.258 | 0.6173 | +1.0000 |
| Cl | 0.440 | 1.0512 | −1.0000 |
| N | 0.331 | 0.7908 | +0.0000 |
| Electric Field Frequency f/GHz | Critical Electric Field Strength Ec/V·nm−1 | Time Required for Contacing t0/ps |
|---|---|---|
| 0 [33] | 0.52 | 130 |
| 1.25 | 0.59 | 292 |
| 5.0 | 0.71 | 248 |
| 10.0 | 0.77 | 237 |
| Electric Field Strength Ec V·nm−1 | Electric Field Frequency f = 5.0 GHz | Electric Field Frequency f = 10.0 GHz |
|---|---|---|
| 0.50 | 0.73 | 0.71 |
| 0.60 | 0.85 | 0.72 |
| 0.70 | 0.88 | 0.79 |
| 0.71 (f = 5.0 GHz) 0.77 (f = 10.0 GHz) | 0.88 | 0.81 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, F.; Chen, R.; Wang, G.; Fan, J.; Niu, H. Coalescence and Break-Up Behaviors of Nanodroplets under AC Electric Field. Molecules 2023, 28, 3064. https://doi.org/10.3390/molecules28073064
Song F, Chen R, Wang G, Fan J, Niu H. Coalescence and Break-Up Behaviors of Nanodroplets under AC Electric Field. Molecules. 2023; 28(7):3064. https://doi.org/10.3390/molecules28073064
Chicago/Turabian StyleSong, Fenhong, Ruifeng Chen, Gang Wang, Jing Fan, and Hu Niu. 2023. "Coalescence and Break-Up Behaviors of Nanodroplets under AC Electric Field" Molecules 28, no. 7: 3064. https://doi.org/10.3390/molecules28073064
APA StyleSong, F., Chen, R., Wang, G., Fan, J., & Niu, H. (2023). Coalescence and Break-Up Behaviors of Nanodroplets under AC Electric Field. Molecules, 28(7), 3064. https://doi.org/10.3390/molecules28073064







