Crystallization of Amorphous Nimesulide: The Relationship between Crystal Growth Kinetics and Liquid Dynamics
Abstract
1. Introduction
2. Experimental Section
2.1. Materials
2.2. Measurement of the Crystal Growth of Nimesulide
2.3. Raman Microscopy
2.4. Thermal Analysis
2.5. Broadband Dielectric Spectroscopy
2.6. Shear Viscosity Measurement
3. Results and Discussion
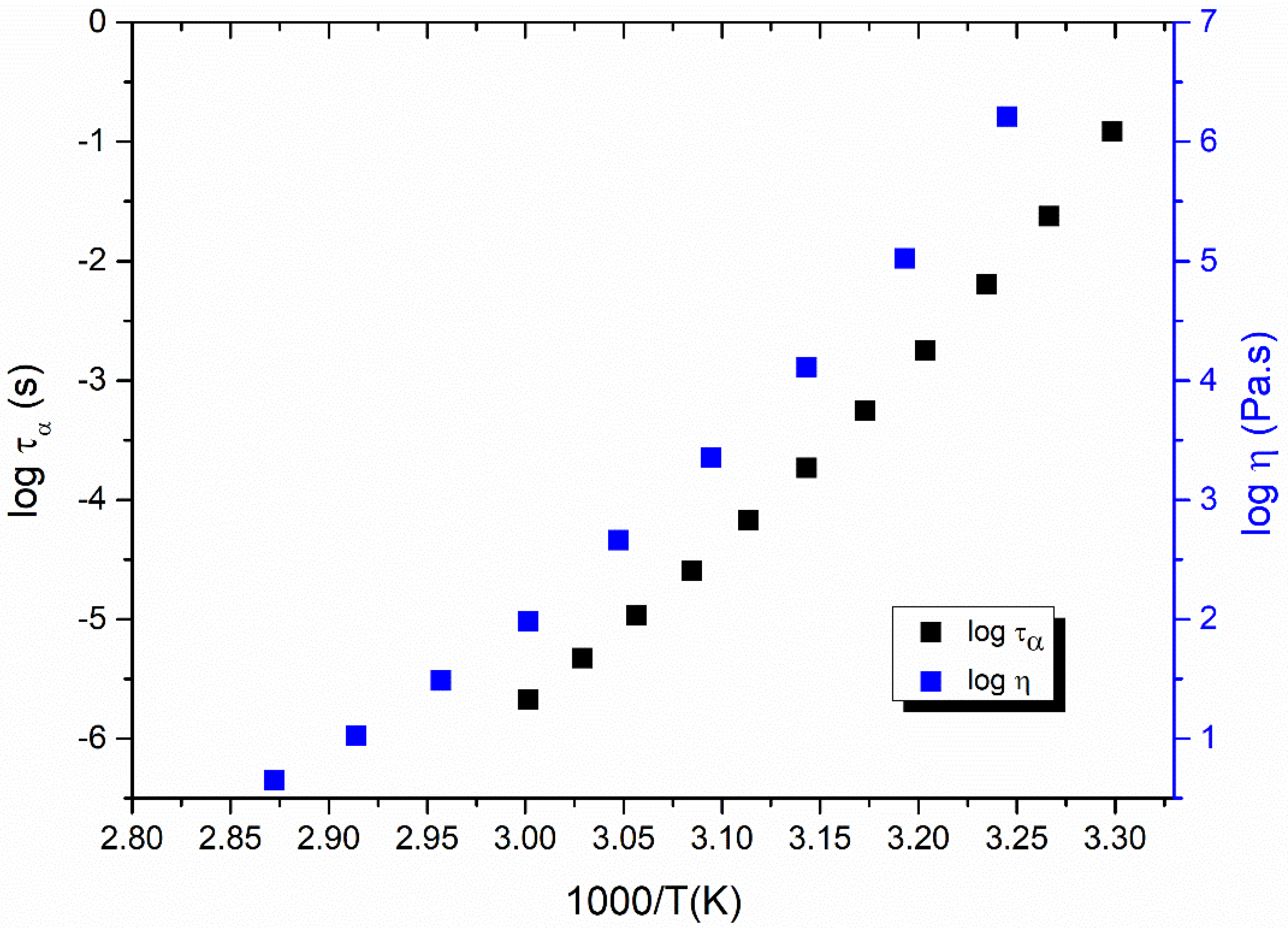
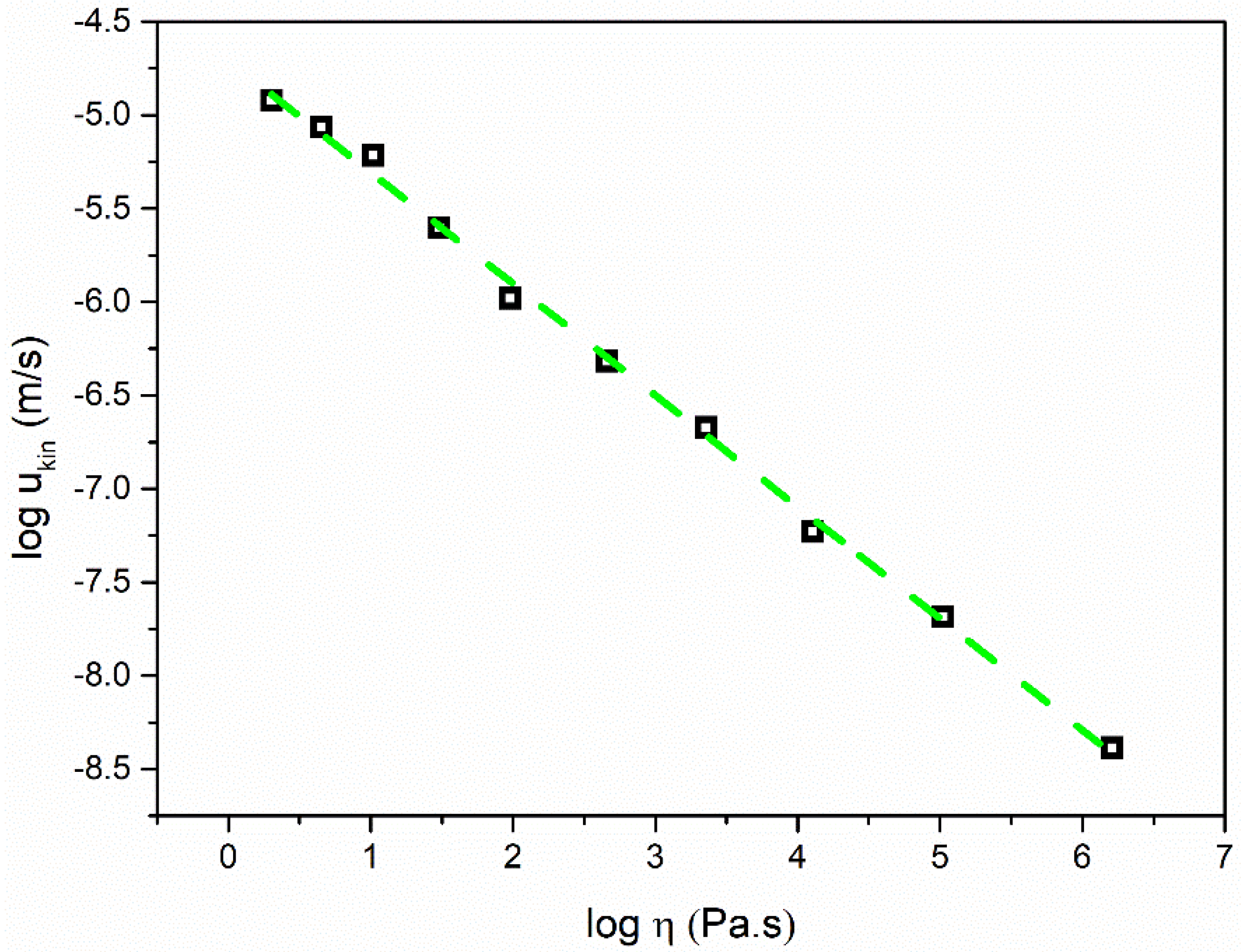
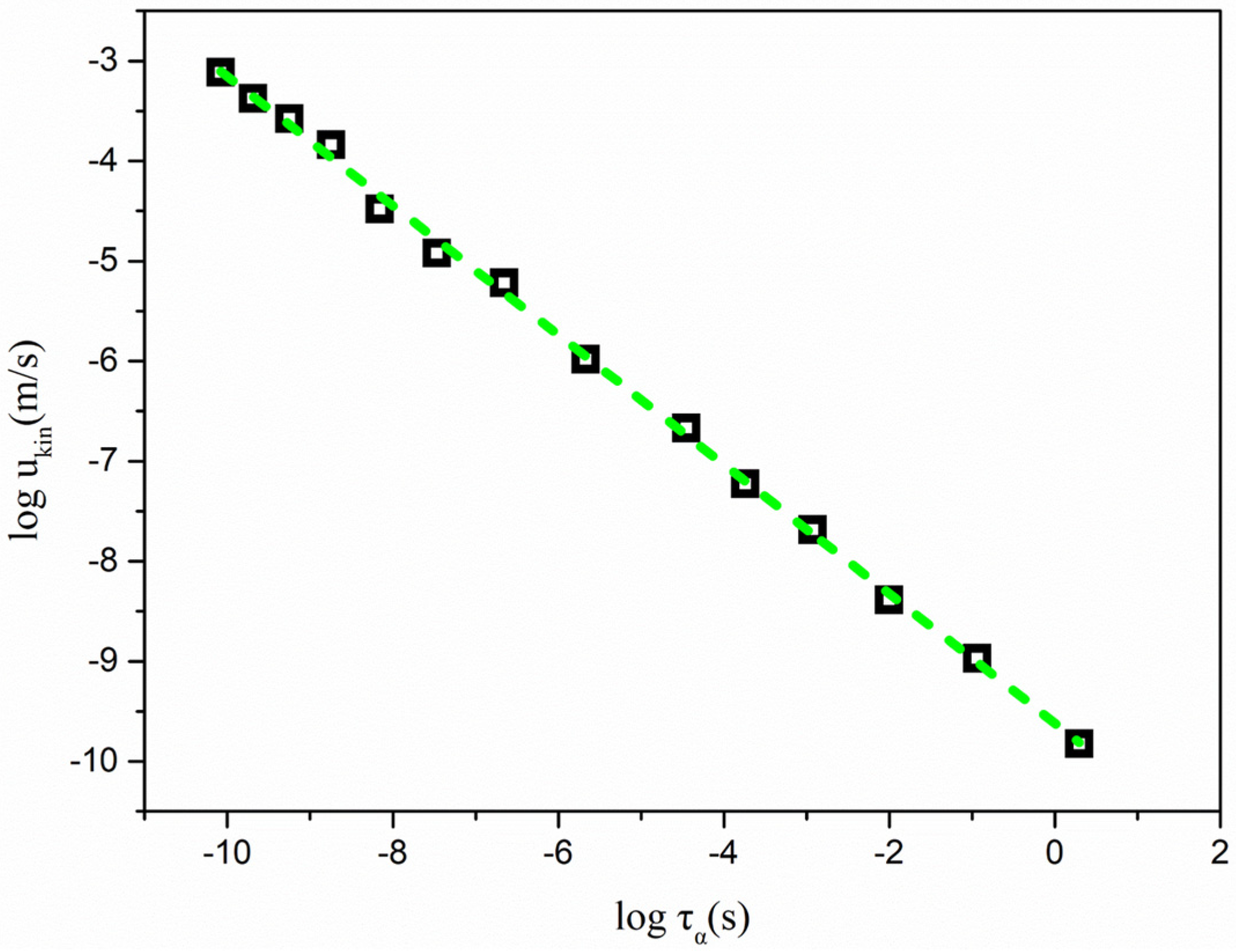
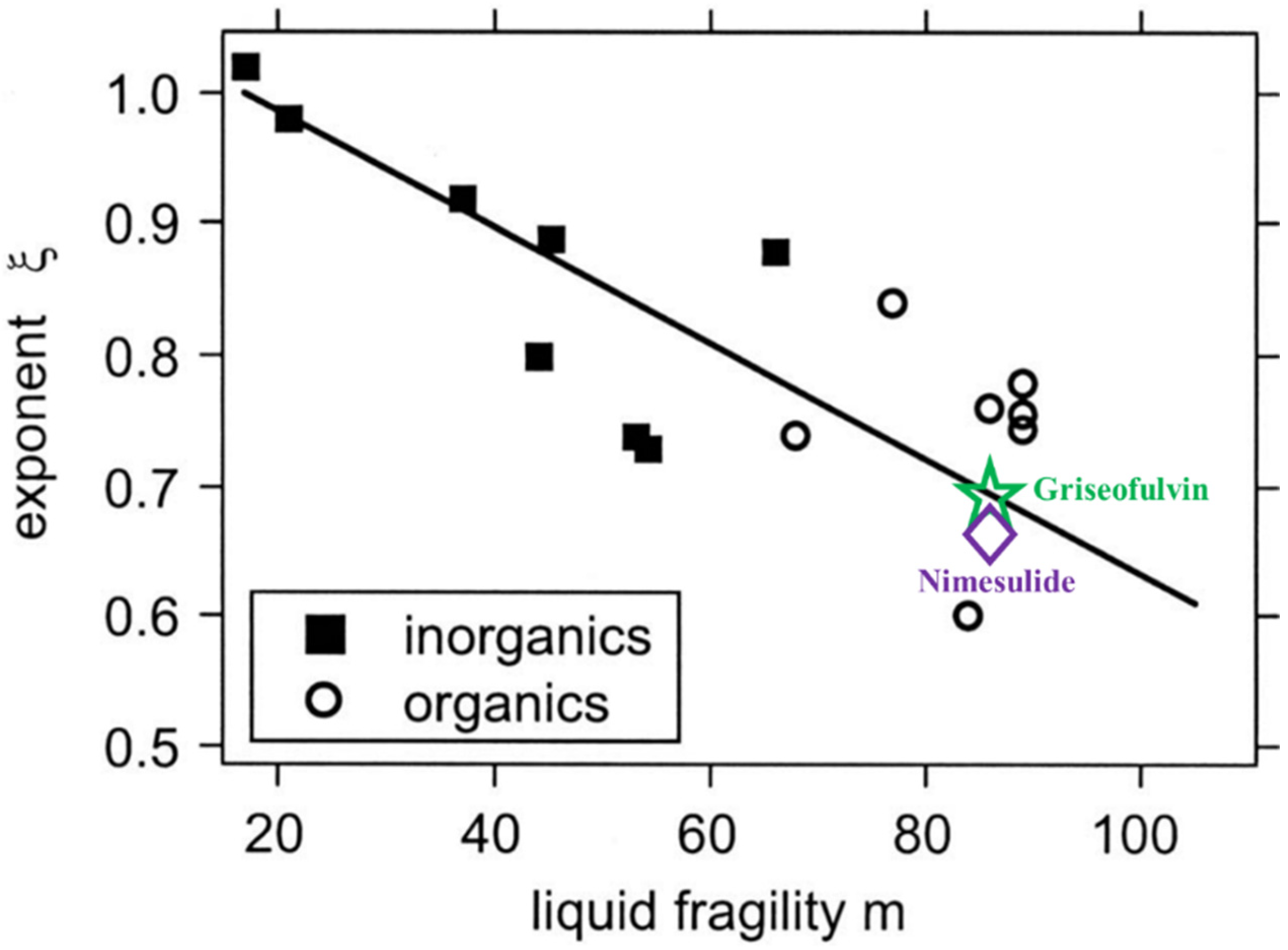
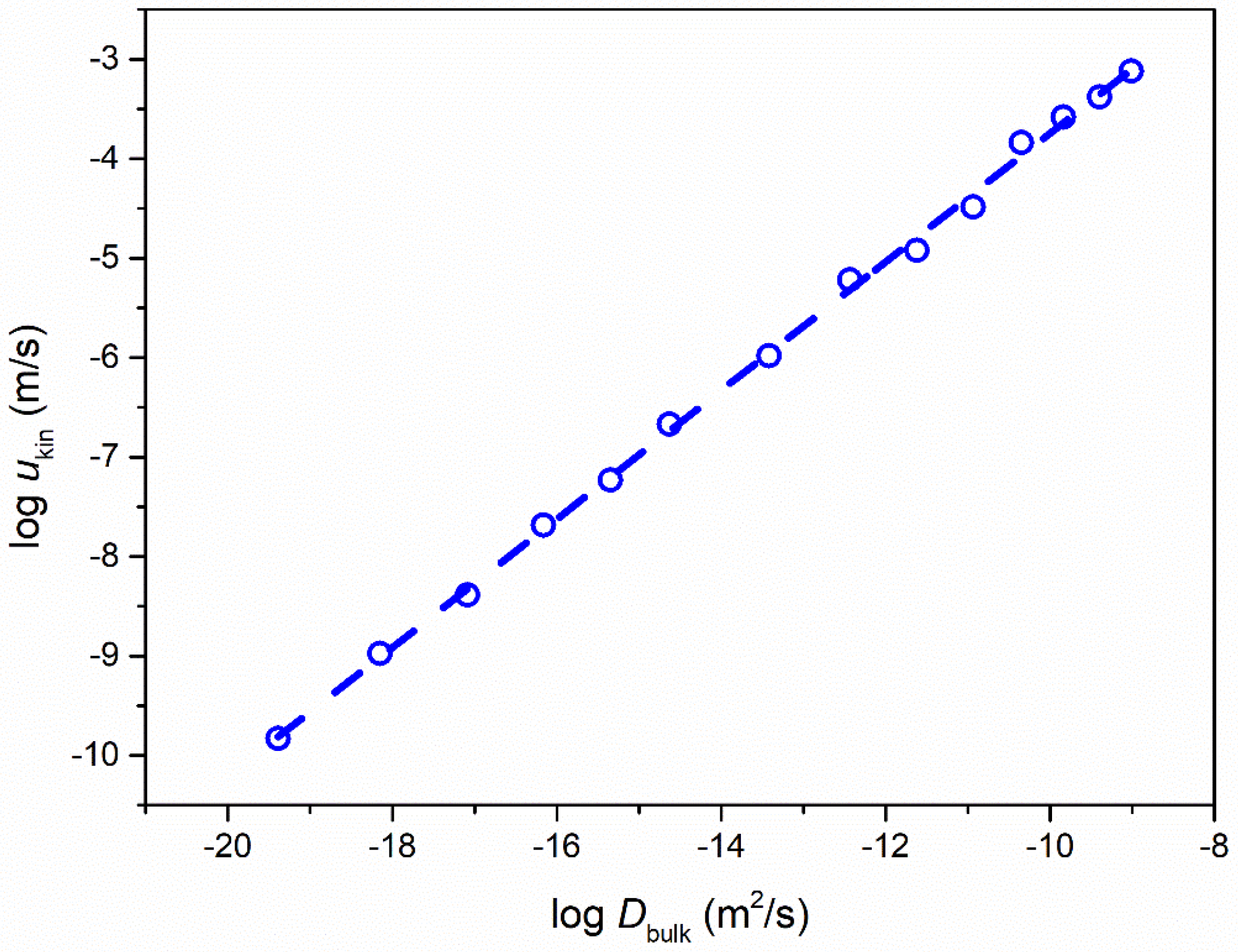
3.1. Correlations of Crystal Growth Kinetics and the Molecular Mobility of Nimesulide in the Supercooled Liquid
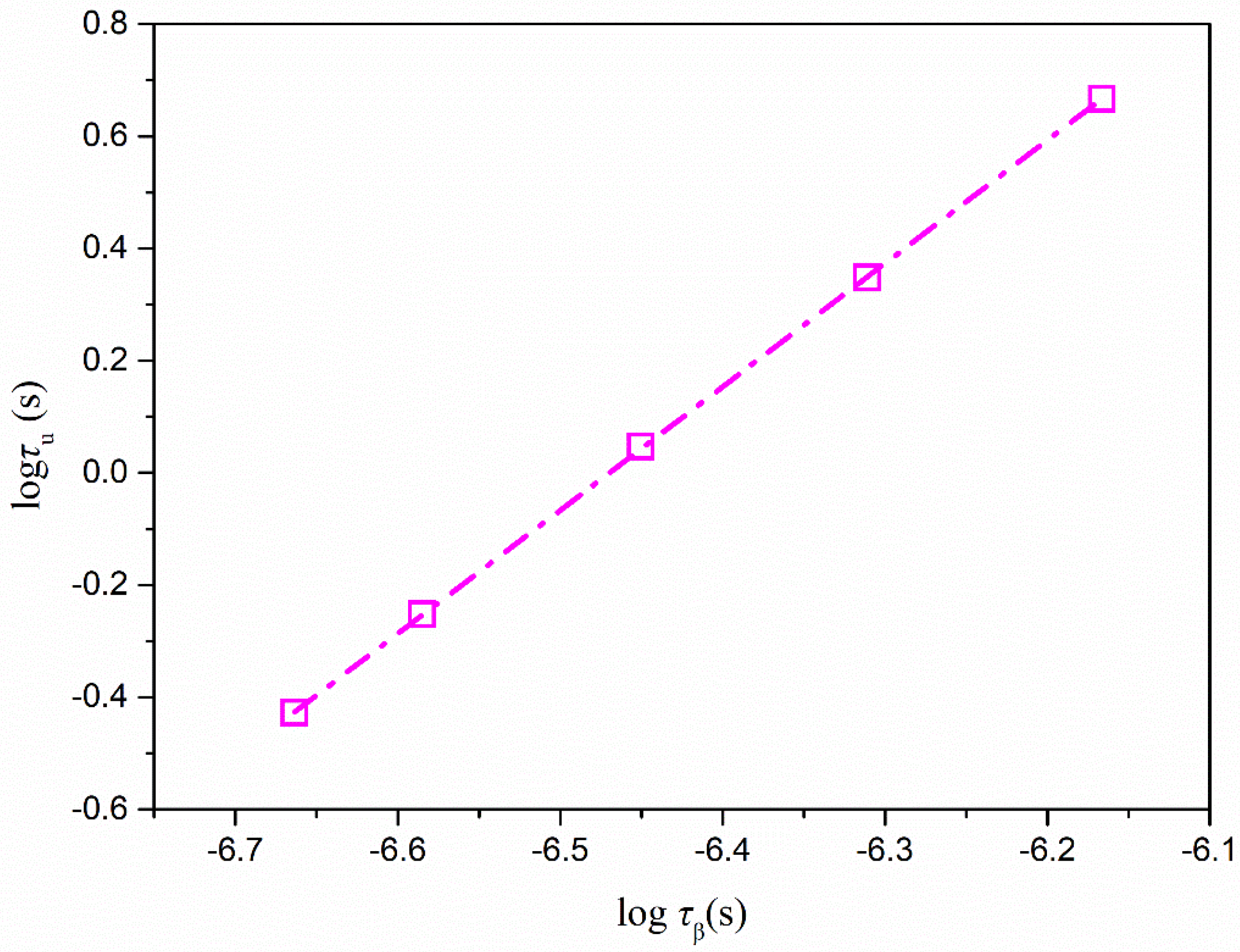
3.2. Correlations of Crystal Growth Kinetics and Molecular Mobility of Nimesulide in the Glassy State
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Shi, Q.; Li, F.; Yeh, S.; Wang, Y.; Xin, J. Physical stability of amorphous pharmaceutical solids: Nucleation, crystal growth, phase separation and effects of the polymers. Int. J. Pharm. 2020, 590, 119925. [Google Scholar] [CrossRef] [PubMed]
- Yu, L. Surface mobility of molecular glasses and its importance in physical stability. Adv. Drug Deliv. Rev. 2016, 100, 3–9. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.; Zhu, L.; Wu, T.; Cai, T.; Gunn, E.M.; Yu, L. Stability of amorphous pharmaceutical solids: Crystal growth mechanisms and effect of polymer additives. AAPS J. 2012, 14, 380–388. [Google Scholar] [CrossRef] [PubMed]
- Huang, C.; Powell, C.T.; Sun, Y.; Cai, T.; Yu, L. Effect of low-concentration polymers on crystal growth in molecular glasses: A controlling role for polymer segmental mobility relative to host dynamics. J. Phys. Chem. B 2017, 121, 1963–1971. [Google Scholar] [CrossRef]
- Wang, K.; Sun, C.C. Crystal growth of celecoxib from amorphous state: Polymorphism, growth mechanism, and kinetics. Cryst. Growth Des. 2019, 19, 3592–3600. [Google Scholar] [CrossRef]
- Shi, Q.; Tao, J.; Zhang, J.; Su, Y.; Cai, T. Crack- and bubble-induced fast crystal growth of amorphous griseofulvin. Cryst. Growth Des. 2019, 20, 24–28. [Google Scholar] [CrossRef]
- Swallen, S.F.; Ediger, M.D. Self-diffusion of the amorphous pharmaceutical indomethacin near Tg. Soft Matter 2011, 7, 10339–10344. [Google Scholar] [CrossRef]
- Shi, Q.; Wang, Y.; Xu, J.; Liu, Z.; Chin, C. Fast crystal growth of amorphous nimesulide: Implication of surface effects. Acta Crystallogr. Sect. B Struct. Sci. Cryst. Eng. Mater. 2022, 78, 33–39. [Google Scholar] [CrossRef]
- Shi, Q.; Moinuddin, S.M.; Wang, Y.; Ahsan, F.; Li, F. Physical stability and dissolution behaviors of amorphous pharmaceutical solids: Role of surface and interface effects. Int. J. Pharm. 2022, 625, 122098. [Google Scholar] [CrossRef]
- Powell, C.T.; Xi, H.; Sun, Y.; Gunn, E.; Chen, Y.; Ediger, M.D.; Yu, L. Fast crystal growth in o-terphenyl glasses: A possible role for fracture and surface mobility. J. Phys. Chem. B 2015, 119, 10124–10130. [Google Scholar] [CrossRef]
- Sun, Y.; Xi, H.; Ediger, M.D.; Yu, L. Diffusionless crystal growth from glass has precursor in equilibrium liquid. J. Phys. Chem. B 2008, 112, 661–664. [Google Scholar] [CrossRef] [PubMed]
- Sun, Y.; Xi, H.; Chen, S.; Ediger, M.D.; Yu, L. Crystallization near glass transition, transition from diffusion-controlled to diffusionless crystal growth studied with seven polymorphs. J. Phys. Chem. B 2008, 112, 5594–5601. [Google Scholar] [CrossRef]
- Huang, C.; Ruan, S.; Cai, T.; Yu, L. Fast surface diffusion and crystallization of amorphous griseofulvin. J. Phys. Chem. B 2017, 121, 9463–9468. [Google Scholar] [CrossRef] [PubMed]
- Li, Y.; Annamareddy, A.; Morgan, D.; Yu, Z.; Wang, B.; Cao, C.; Perepezko, J.H.; Ediger, M.D.; Voyles, P.M.; Yu, L. Surface diffusion is controlled by bulk fragility across all glass types. Phys. Rev. Lett. 2022, 128, 075501. [Google Scholar] [CrossRef] [PubMed]
- Havriliak, S.; Negami, S. A complex plane representation of dielectric and mechanical relaxation processes in some polymers. Polymer 1967, 8, 161–210. [Google Scholar] [CrossRef]
- Iglesias, T.P.; Carballo, M.; Fernandez, J.P. Broadband Dielectric Spectroscopy; Springer: Berlin/Heidelberg, Germany, 2003. [Google Scholar]
- Grzybowska, K.; Capaccioli, S.; Paluch, M. Recent developments in the experimental investigations of relaxations in pharmaceuticals by dielectric techniques at ambient and elevated pressure. Adv. Drug Deliv. Rev. 2016, 100, 158–182. [Google Scholar] [CrossRef]
- Li, F.; Xin, J.; Shi, Q. Diffusion-controlled and ‘diffusionless’ crystal growth: Relation between liquid dynamics and growth kinetics of griseofulvin. J. Appl. Crystallogr. 2021, 54, 142–147. [Google Scholar] [CrossRef]
- Ediger, M.D.; Harrowell, P.; Yu, L. Crystal growth kinetics exhibit a fragility-dependent decoupling from viscosity. J. Chem. Phys. 2008, 128, 034709. [Google Scholar] [CrossRef]
- Baird, J.A.; Van Eerdenbrugh, B.; Taylor, L.S. A classification system to assess the crystallization tendency of organic molecules from undercooled melts. J. Pharm. Sci. 2010, 99, 3787–3806. [Google Scholar] [CrossRef]
- Knapik-Kowalczuk, J.; Rams-Baron, M.; Paluch, M. Current research trends in dielectric relaxation studies of amorphous pharmaceuticals: Physical stability, tautomerism, and the role of hydrogen bonding. TrAC-Trend Anal. Chem. 2021, 134, 116097. [Google Scholar] [CrossRef]
- Svoboda, R.; Košťálová, D.; Krbal, M.; Komersová, A. Indomethacin: The interplay between structural relaxation, viscous flow and crystal growth. Molecules 2022, 27, 5668. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Wang, Y.; Cheng, J.; Chen, H.; Xu, J.; Liu, Z.; Shi, Q.; Zhang, C. Recent advances in the application of characterization techniques for studying physical stability of amorphous pharmaceutical solids. Crystals 2021, 11, 1440. [Google Scholar] [CrossRef]
- Zhang, J.; Shi, Q.; Qu, T.; Zhou, D.; Cai, T. Crystallization kinetics and molecular dynamics of binary coamorphous systems of nimesulide and profen analogs. Int. J. Pharm. 2021, 610, 121235. [Google Scholar] [CrossRef] [PubMed]
- Böhmer, R.; Ngai, K.L.; Angell, C.A.; Plazek, D.J. Nonexponential relaxations in strong and fragile glass formers. J. Chem. Phys. 1993, 99, 4201–4209. [Google Scholar] [CrossRef]
- Knapik, J.; Wojnarowska, Z.; Grzybowska, K.; Tajber, L.; Mesallati, H.; Paluch, K.J.; Paluch, M. Molecular dynamics and physical stability of amorphous nimesulide drug and its binary drug-polymer systems. Mol. Pharm. 2016, 13, 1937–1946. [Google Scholar] [CrossRef] [PubMed]
- Kawakami, K.; Harada, T.; Yoshihashi, Y.; Yonemochi, E.; Terada, K.; Moriyama, H. Correlation between glass-forming ability and fragility of pharmaceutical compounds. J. Phys. Chem. B 2015, 119, 4873–4880. [Google Scholar] [CrossRef]
- Knapik, J.; Wojnarowska, Z.; Grzybowska, K.; Jurkiewicz, K.; Tajber, L.; Paluch, M. Molecular dynamics and physical stability of coamorphous ezetimib and indapamide mixtures. Mol. Pharm. 2015, 12, 3610–3619. [Google Scholar] [CrossRef]
- Tanaka, H. Relationship among glass-forming ability, fragility, and short-range bond ordering of liquids. J.Non-Cryst. Solids 2005, 351, 678–690. [Google Scholar] [CrossRef]
- Mirigian, S.; Schweizer, K.S. Theory of activated glassy relaxation, mobility gradients, surface diffusion, and vitrification in free standing thin films. J. Chem. Phys. 2015, 143, 244705. [Google Scholar] [CrossRef] [PubMed]
- Bhattacharya, S.; Suryanarayanan, R. Local mobility in amorphous pharmaceuticals—Haracterization and implications on stability. J. Pharm. Sci. 2009, 98, 2935–2953. [Google Scholar] [CrossRef]
- Sun, Y.X.; Xi, H.; Ediger, M.D.; Richert, R.; Yu, L. Diffusion-controlled and diffusionless crystal growth near the glass transition temperature: Relation between liquid dynamics and growth kinetics of seven ROY polymorphs. J. Chem. Phys. 2009, 131, 074506. [Google Scholar] [CrossRef]
- Shi, Q.; Zhang, C.; Su, Y.; Zhang, J.; Zhou, D.; Cai, T. Acceleration of crystal growth of amorphous griseofulvin by low-concentration poly(ethylene oxide): Aspects of crystallization kinetics and molecular mobility. Mol. Pharm. 2017, 14, 2262–2272. [Google Scholar] [CrossRef]
- Kothari, K.; Ragoonanan, V.; Suryanarayanan, R. Influence of molecular mobility on the physical stability of amorphous pharmaceuticals in the supercooled and glassy States. Mol. Pharm. 2014, 11, 3048–3055. [Google Scholar] [CrossRef] [PubMed]
- Priemel, P.A.; Laitinen, R.; Barthold, S.; Grohganz, H.; Lehto, V.P.; Rades, T.; Strachan, C.J. Inhibition of surface crystallisation of amorphous indomethacin particles in physical drug-polymer mixtures. Int. J. Pharm. 2013, 456, 301–306. [Google Scholar] [CrossRef]
- Zhang, W.; Yu, L. Surface diffusion of polymer glasses. Macromolecules 2016, 49, 731–735. [Google Scholar] [CrossRef]
- Chen, Y.; Zhang, W.; Yu, L. Hydrogen bonding slows down surface diffusion of molecular glasses. J. Phys. Chem. B 2016, 120, 8007–8015. [Google Scholar] [CrossRef] [PubMed]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, Q.; Wang, Y.; Kong, J. Crystallization of Amorphous Nimesulide: The Relationship between Crystal Growth Kinetics and Liquid Dynamics. Molecules 2023, 28, 2919. https://doi.org/10.3390/molecules28072919
Shi Q, Wang Y, Kong J. Crystallization of Amorphous Nimesulide: The Relationship between Crystal Growth Kinetics and Liquid Dynamics. Molecules. 2023; 28(7):2919. https://doi.org/10.3390/molecules28072919
Chicago/Turabian StyleShi, Qin, Yanan Wang, and Jianfei Kong. 2023. "Crystallization of Amorphous Nimesulide: The Relationship between Crystal Growth Kinetics and Liquid Dynamics" Molecules 28, no. 7: 2919. https://doi.org/10.3390/molecules28072919
APA StyleShi, Q., Wang, Y., & Kong, J. (2023). Crystallization of Amorphous Nimesulide: The Relationship between Crystal Growth Kinetics and Liquid Dynamics. Molecules, 28(7), 2919. https://doi.org/10.3390/molecules28072919





