Slow Magnetic Relaxation and Modulated Photoluminescent Emission of Coordination Polymer Based on 3-Amino-4-hydroxybenzoate Zn and Co Metal Ions
Abstract
1. Introduction
2. Results
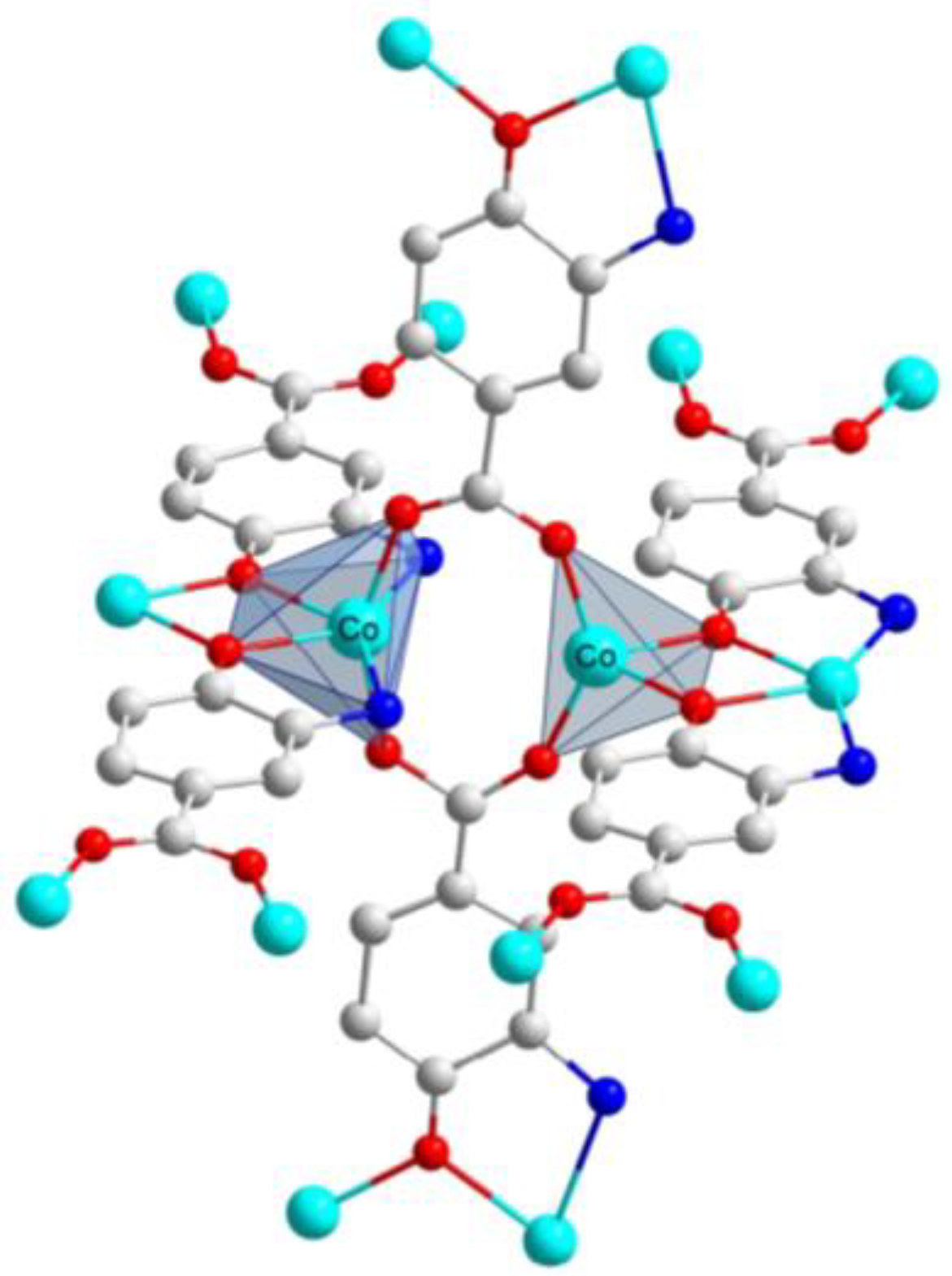
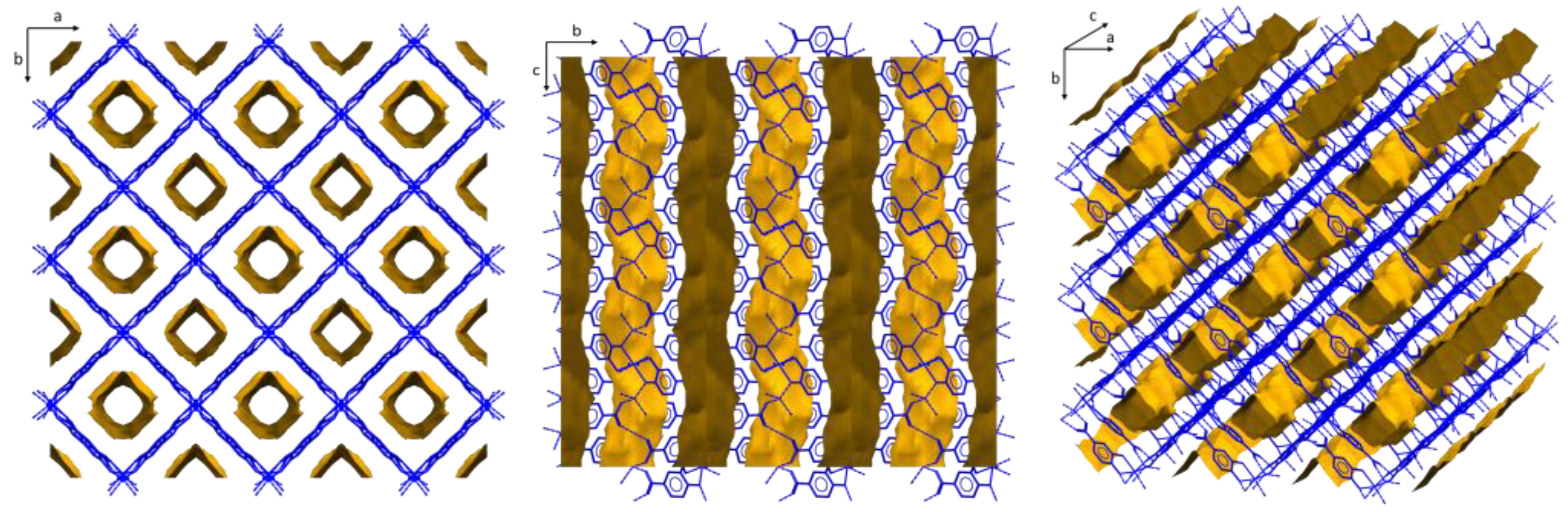
2.1. Crystal Structure Description
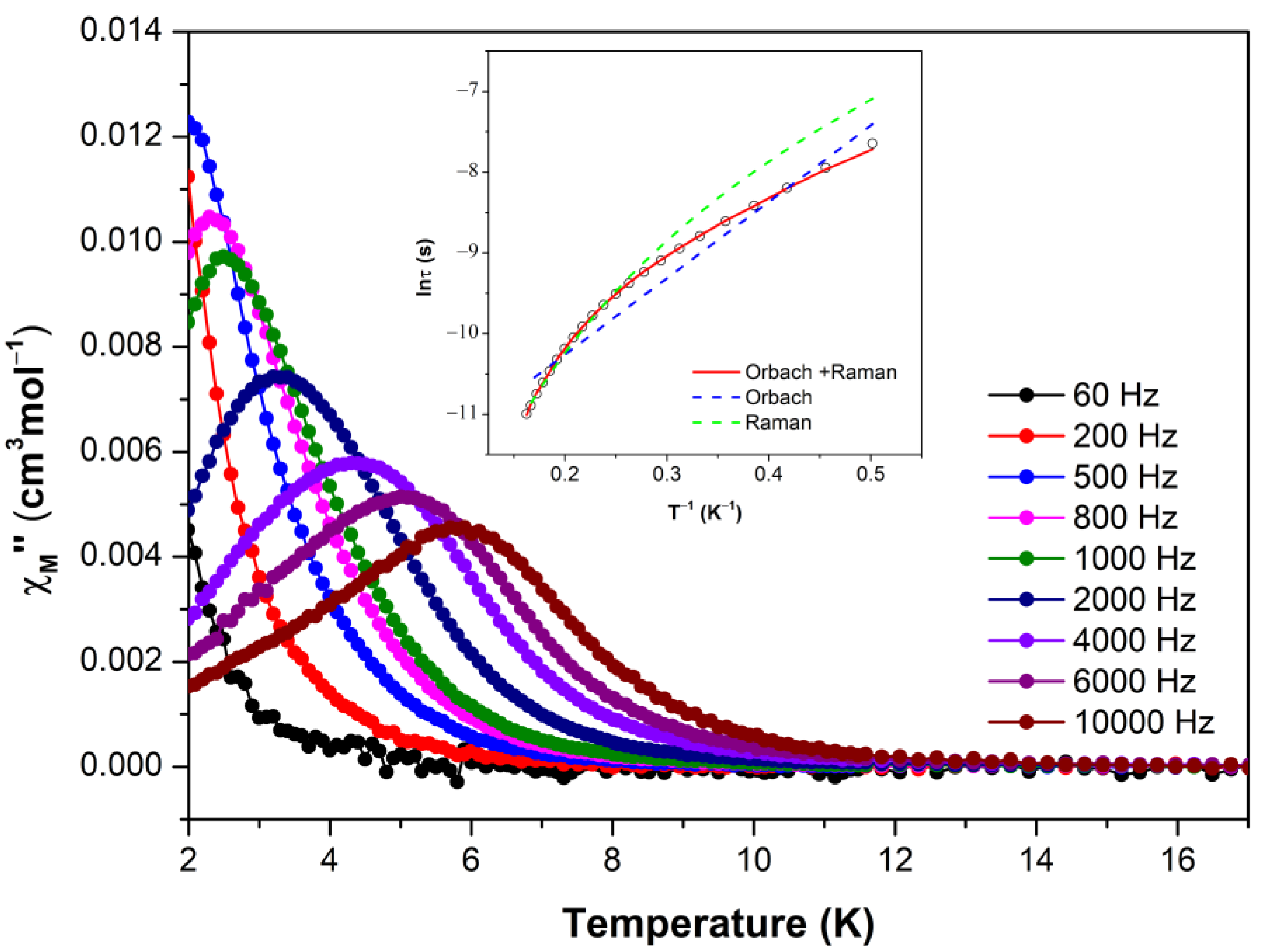
2.2. Magnetic Properties
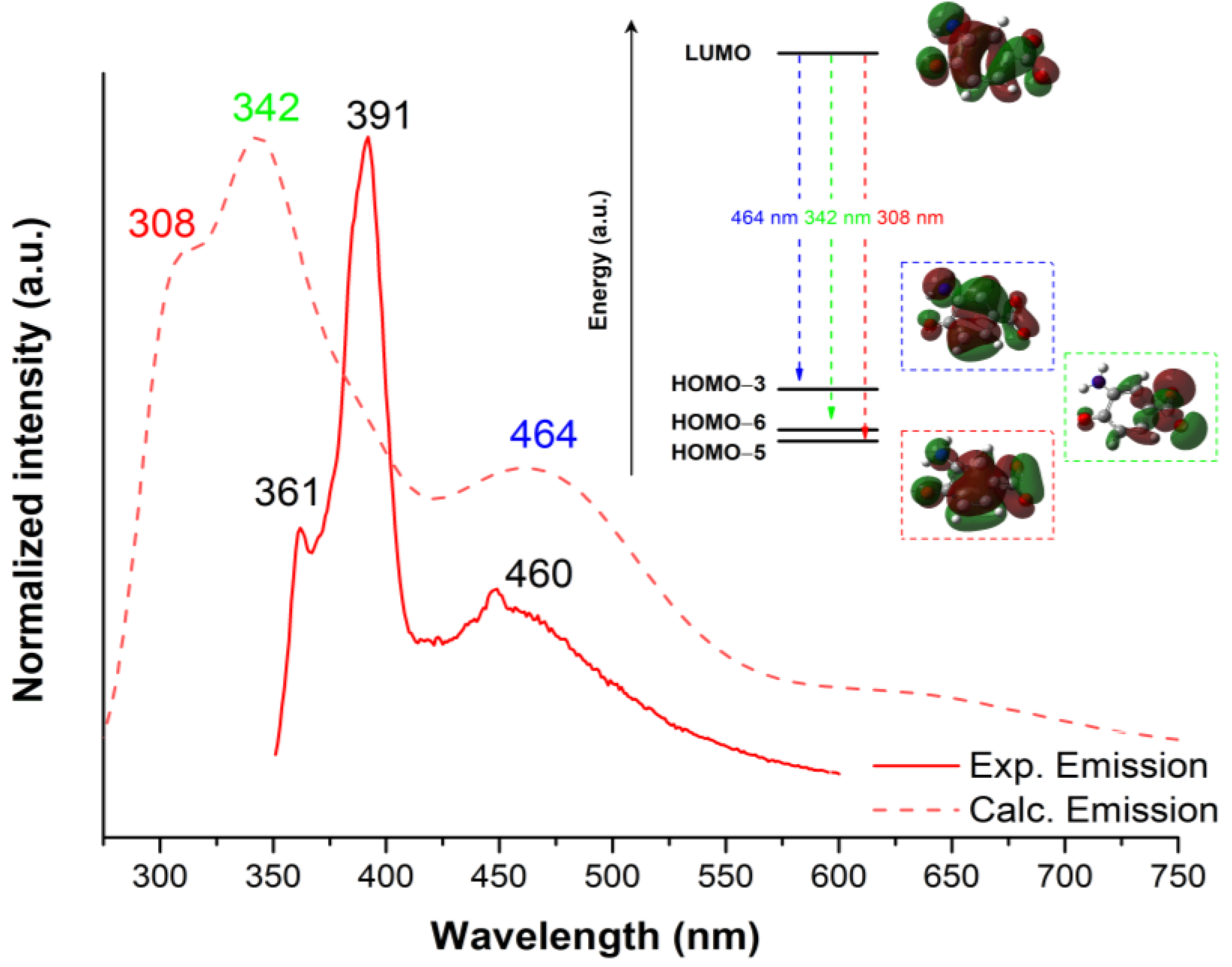
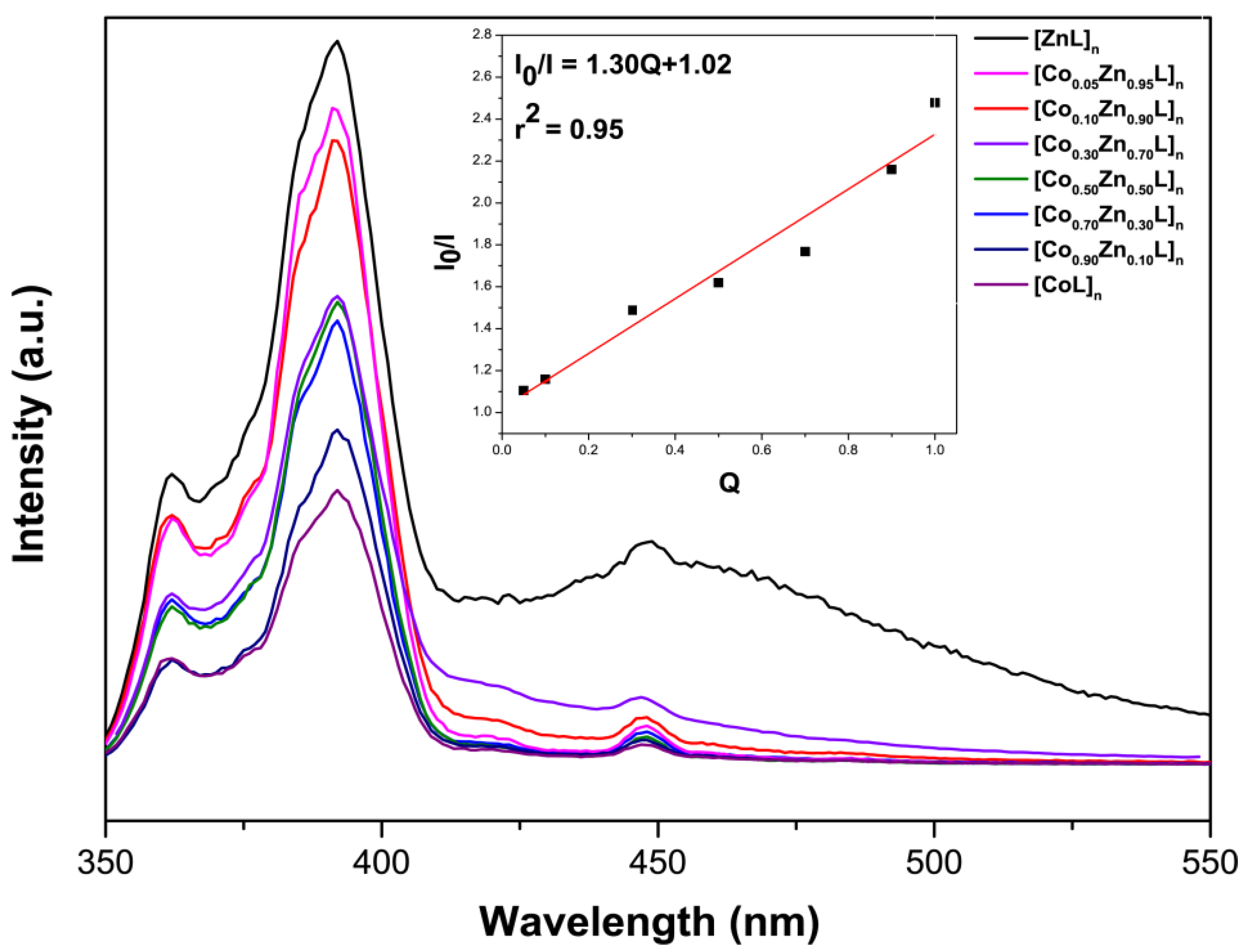
2.3. Photoluminescent Properties
3. Materials and Methods
3.1. Preparation of Complexes
- Synthetic pathway to obtain single crystals
- Synthetic pathway to obtain polycrystalline powder
3.2. Physical Measurements
3.3. Single-Crystal X-ray Diffraction (SCXRD)
3.4. Computational Details
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ouahab, L. Multifunctional Molecular Materials; Jenny Stanford Publishing: New York, NY, USA, 2012. [Google Scholar]
- Pajuelo-Corral, O.; Zabala-Lekuona, A.; San Sebastian, E.; Rodríguez-Diéguez, A.; García, J.A.; Lezama, L.; Colacio, E.; Seco, J.M.; Cepeda, J. Modulating Magnetic and Photoluminescence Properties in 2-Aminonicotinate-Based Bifunctional Coordination Polymers by Merging 3d Metal Ions. Chem. A Eur. J. 2020, 26, 13484–13498. [Google Scholar] [CrossRef] [PubMed]
- Warrier, M.; Bhardwaj, U.; Bukkuru, S. Multifunctional Materials Related content Rays, Waves and Photons: Mirrors-through one darkly-Multi-scale Modeling of Radiation Damage: Large Scale Data Analysis. Multifunct. Mater. 2018, 1, 010201. [Google Scholar] [CrossRef]
- Chen, J.; Cheng, F.; Luo, D.; Huang, J.; Ouyang, J.; Nezamzadeh-Ejhieh, A.; Khan, M.S.; Liu, J.; Peng, Y. Recent advances in Ti-based MOFs in biomedical applications. Dalton Trans. 2022, 51, 14817–14832. [Google Scholar] [CrossRef] [PubMed]
- Zheng, M.; Chen, J.; Zhang, L.; Cheng, Y.; Lu, C.; Liu, Y.; Singh, A.; Trivedi, M.; Kumar, A.; Liu, J. Metal organic frameworks as efficient adsorbents for drugs from wastewater. Mater. Today Commun. 2022, 31, 103514. [Google Scholar] [CrossRef]
- Qin, L.; Li, Y.; Liang, F.; Li, L.; Lan, Y.; Li, Z.; Lu, X.; Yang, M.; Ma, D. A microporous 2D cobalt-based MOF with pyridyl sites and open metal sites for selective adsorption of CO2. Microporous Mesoporous Mater. 2022, 341, 112098. [Google Scholar] [CrossRef]
- Qin, L.; Liang, F.; Li, Y.; Wu, J.; Guan, S.; Wu, M.; Xie, S.; Luo, M.; Ma, D. A 2D Porous Zinc-Organic Framework Platform for Loading of 5-Fluorouracil. Inorganics 2022, 10, 202. [Google Scholar] [CrossRef]
- Li, M.; Yin, S.; Lin, M.; Chen, X.; Pan, Y.; Peng, Y.; Sun, J.; Kumar, A.; Liu, J. Current status and prospects of metal–organic frameworks for bone therapy and bone repair. J. Mater. Chem. B 2022, 10, 5105–5128. [Google Scholar] [CrossRef]
- Li, Y.-W.; Liu, S.-J.; Hu, T.-L.; Bu, X.-H. Doping cobalt into a [Zn7] cluster-based MOF to tune magnetic behaviour and induce fluorescence signal mutation. Dalton Trans. 2014, 43, 11470–11473. [Google Scholar] [CrossRef]
- Caskey, S.R.; Matzger, A.J. Selective Metal Substitution for the Preparation of Heterobimetallic Microporous Coordination Polymers. Inorg. Chem. 2008, 47, 7942–7944. [Google Scholar] [CrossRef]
- Zhang, L.; Jiang, K.; Li, L.; Xia, Y.P.; Hu, T.L.; Yang, Y.; Cui, Y.; Li, B.; Chen, B.; Qian, G. Efficient separation of C2H2 from C2H2/CO2 mixtures in an acid-base resistant metal-organic framework. Chem. Commun. 2018, 54, 4846–4849. [Google Scholar] [CrossRef]
- Fischer, M.; Hoffmann, F.; Fröba, M. New Microporous Materials for Acetylene Storage and C2H2/CO2 Separation: Insights from Molecular Simulations. ChemPhysChem 2010, 11, 2220–2229. [Google Scholar] [CrossRef] [PubMed]
- Li, P.; He, Y.; Zhao, Y.; Weng, L.; Wang, H.; Krishna, R.; Wu, H.; Zhou, W.; O’Keeffe, M.; Han, Y.; et al. A Rod-Packing Microporous Hydrogen-Bonded Organic Framework for Highly Selective Separation of C2H2/CO2 at Room Temperature. Angew. Chem. 2015, 127, 584–587. [Google Scholar] [CrossRef]
- Cui, X.; Chen, K.; Xing, H.; Yang, Q.; Krishna, R.; Bao, Z.; Wu, H.; Zhou, W.; Dong, X.; Han, Y.; et al. Pore chemistry and size control in hybrid porous materials for acetylene capture from ethylene. Science 2016, 353, 141–144. [Google Scholar] [CrossRef]
- Luo, F.; Yan, C.; Dang, L.; Krishna, R.; Zhou, W.; Wu, H.; Dong, X.; Han, Y.; Hu, T.-L.; O’Keeffe, M.; et al. UTSA-74: A MOF-74 Isomer with Two Accessible Binding Sites per Metal Center for Highly Selective Gas Separation. J. Am. Chem. Soc. 2016, 138, 5678–5684. [Google Scholar] [CrossRef]
- Sessoli, R.; Gatteschi, D.; Caneschi, A.; Novak, M.A. Magnetic bistability in a metal-ion cluster. Nature 1993, 365, 141–143. [Google Scholar] [CrossRef]
- Mínguez Espallargas, G.; Coronado, E. Magnetic functionalities in MOFs: From the framework to the pore. Chem. Soc. Rev. 2018, 47, 533–557. [Google Scholar] [CrossRef]
- Craig, G.A.; Murrie, M. 3D Single-Ion Magnets. Chem. Soc. Rev. 2015, 44, 2135–2147. [Google Scholar] [CrossRef] [PubMed]
- Bar, A.K.; Pichon, C.; Sutter, J.P. Magnetic anisotropy in two- to eight-coordinated transition–metal complexes: Recent developments in molecular magnetism. Coord. Chem. Rev. 2016, 308, 346–380. [Google Scholar] [CrossRef]
- Murrie, M. Cobalt(II) single-molecule magnets. Chem. Soc. Rev. 2010, 39, 1986–1995. [Google Scholar] [CrossRef]
- Villa-Pérez, C.; Oyarzabal, I.; Echeverría, G.A.; Valencia-Uribe, G.C.; Seco, J.M.; Soria, D.B. Single-Ion Magnets Based on Mononuclear Cobalt(II) Complexes with Sulfadiazine. Eur. J. Inorg. Chem. 2016, 2016, 4835–4841. [Google Scholar] [CrossRef]
- Peng, Y.; Bodenstein, T.; Fink, K.; Mereacre, V.; Anson, C.E.; Powell, A.K. Magnetic anisotropy of a CoII single ion magnet with distorted trigonal prismatic coordination: Theory and experiment. Phys. Chem. Chem. Phys. 2016, 18, 30135–30143. [Google Scholar] [CrossRef]
- Pamei, M.; Puzari, A. Luminescent transition metal–organic frameworks: An emerging sensor for detecting biologically essential metal ions. Nano Struct. Nano Objects 2019, 19, 100364. [Google Scholar] [CrossRef]
- Majee, P.; Singha, D.K.; Mondal, S.K.; Mahata, P. Effect of charge transfer and structural rigidity on divergent luminescence response of a metal organic framework towards different metal ions: Luminescence lifetime decay experiments and DFT calculations. Photochem. Photobiol. Sci. 2019, 18, 1110–1121. [Google Scholar] [CrossRef] [PubMed]
- Cui, Y.; Yue, Y.; Qian, G.; Chen, B. Luminescent functional metal-organic frameworks. Chem. Rev. 2012, 112, 1126–1162. [Google Scholar] [CrossRef] [PubMed]
- García-Valdivia, A.A.; Pérez-Yáñez, S.; García, J.A.; Fernández, B.; Cepeda, J.; Rodríguez-Diéguez, A. Magnetic and Photoluminescent Sensors Based on Metal-Organic Frameworks Built up from 2-aminoisonicotinate. Sci. Rep. 2020, 10, 8843. [Google Scholar] [CrossRef] [PubMed]
- Echenique-Errandonea, E.; Mendes, R.F.; Figueira, F.; Choquesillo-Lazarte, D.; Beobide, G.; Cepeda, J.; Ananias, D.; Rodríguez-Diéguez, A.; Almeida Paz, F.A.; Seco, J.M. Multifunctional Lanthanide-Based Metal-Organic Frameworks Derived from 3-Amino-4-hydroxybenzoate: Single-Molecule Magnet Behavior, Luminescent Properties for Thermometry, and CO2Adsorptive Capacity. Inorg. Chem. 2022, 61, 12977–12990. [Google Scholar] [CrossRef] [PubMed]
- Llunell, M.; Casanova, D.; Cirera, J.; Bofill, J.M.; Alemany, P.; Alvarez, S.; Pinsky, M.; Avnir, D. Program for the Stereochemical Analysis of Molecular Fragments by Means of Continuous Shape Measures and Associated Tools. In SHAPE, v1.1b; University of Barcelona: Barcelona, Spain, 2005; pp. 1–35. [Google Scholar]
- Blatov, V.A.; Shevchenko, A.P.; Proserpio, D.M. Applied topological analysis of crystal structures with the program package topospro. Cryst. Growth Des. 2014, 14, 3576–3586. [Google Scholar] [CrossRef]
- Singh Pannu, A.P.; Kapoor, P.; Hundal, G.; Kapoor, R.; Corbella, M.; Aliaga-Alcalde, N.; Singh Hundal, M. Magneto-structural studies of two new cobalt(II)-N, N-diisobutylisonicotinamide compounds: [ColCl2]n and [Co(L)2(H2O)4][CoLBr3]2·2H2O. J. Chem. Soc. Dalton Trans. 2011, 40, 12560–12569. [Google Scholar] [CrossRef] [PubMed]
- Zhao, J.P.; Song, W.C.; Zhao, R.; Yang, Q.; Hu, B.W.; Bu, X.H. Tuning the subunits and topologies in cluster-based cobalt-organic frameworks by varying the reaction conditions. Cryst. Growth Des. 2013, 13, 2858–2865. [Google Scholar] [CrossRef]
- Xiao, D.R.; Zhang, G.J.; Liu, J.L.; Fan, L.L.; Yuan, R.; Tong, M.L. Enantiopure chiral coordination polymers of tetrahedral and octahedral cobalt(II) alternate chains exhibiting slow magnetic relaxation behavior. Dalton Trans. 2011, 40, 5680–5683. [Google Scholar] [CrossRef]
- Oyarzabal, I.; Ruiz, J.; Mota, A.J.; Rodríguez-Diéguez, A.; Seco, J.M.; Colacio, E. An experimental and theoretical magneto-structural study of polynuclear NiII complexes assembled from a versatile bis(salicylaldehyde)diamine polytopic ligand. Dalton Trans. 2015, 44, 6825–6838. [Google Scholar] [CrossRef]
- Yao, B.; Singh, M.K.; Deng, Y.F.; Wang, Y.N.; Dunbar, K.R.; Zhang, Y.Z. Trigonal Prismatic Cobalt(II) Single-Ion Magnets: Manipulating the Magnetic Relaxation through Symmetry Control. Inorg. Chem. 2020, 59, 8505–8513. [Google Scholar] [CrossRef] [PubMed]
- Razquin-Bobillo, L.; Pajuelo-Corral, O.; Artetxe, B.; Zabala-Lekuona, A.; Choquesillo-Lazarte, D.; Rodríguez-Diéguez, A.; Sebastian, E.S.; Cepeda, J. Combined experimental and theoretical investigation on the magnetic properties derived from the coordination of 6-methyl-2-oxonicotinate to 3d-metal ions. Dalton Trans. 2022, 51, 9780–9792. [Google Scholar] [CrossRef]
- Rodriguez-Dieguez, A.; Perez-Yanez, S.; Ruiz-Rubio, L.; Seco, J.M.; Cepeda, J. From isolated to 2D Coordination Polymers based on 6-aminonicotinate and 3d-Metal Ions: Towards Field-Induced Single-Ion-Magnets. CrystEngComm 2017, 19, 2229–2242. [Google Scholar] [CrossRef]
- Zhao, X.Q.; Wang, J.; Bao, D.X.; Xiang, S.; Liu, Y.J.; Li, Y.C. The ferromagnetic [Ln2Co6] heterometallic complexes. Dalton Trans. 2017, 46, 2196–2203. [Google Scholar] [CrossRef] [PubMed]
- Zadrozny, J.M.; Telser, J.; Long, J.R. Slow magnetic relaxation in the tetrahedral cobalt(II) complexes [Co(EPh)4]2− (Edouble bondO, S, Se). Polyhedron 2013, 64, 209–217. [Google Scholar] [CrossRef]
- Cole, K.S.; Cole, R.H. Dispersion and Absorption in Dielectrics I. Alternating Current Characteristics. J. Chem. Phys. 2004, 9, 341–351. [Google Scholar] [CrossRef]
- Zadrozny, J.M.; Long, J.R. Slow Magnetic Relaxation at Zero Field in the Tetrahedral Complex [Co(SPh)4]2–. J. Am. Chem. Soc. 2011, 133, 20732–20734. [Google Scholar] [CrossRef]
- Rechkemmer, Y.; Breitgoff, F.D.; van der Meer, M.; Atanasov, M.; Hakl, M.; Orlita, M.; Neugebauer, P.; Neese, F.; Sarkar, B.; van Slageren, J. A four-coordinate cobalt(II) single-ion magnet with coercivity and a very high energy barrier. Nat. Commun. 2016, 7, 10467. [Google Scholar] [CrossRef]
- Novikov, V.V.; Pavlov, A.A.; Nelyubina, Y.V.; Boulon, M.-E.; Varzatskii, O.A.; Voloshin, Y.Z.; Winpenny, R.E.P. A Trigonal Prismatic Mononuclear Cobalt(II) Complex Showing Single-Molecule Magnet Behavior. J. Am. Chem. Soc. 2015, 137, 9792–9795. [Google Scholar] [CrossRef]
- Yao, B.; Deng, Y.-F.; Li, T.; Xiong, J.; Wang, B.-W.; Zheng, Z.; Zhang, Y.-Z. Construction and Magnetic Study of a Trigonal-Prismatic Cobalt(II) Single-Ion Magnet. Inorg. Chem. 2018, 57, 14047–14051. [Google Scholar] [CrossRef] [PubMed]
- Cepeda, J.; García-Valdivia, A.A.; Echenique-Errandonea, E.; Ramírez-Rodríguez, G.B.; Delgado-López, J.M.; Fernández, B.; Rojas, S.; Cepeda, J.; Rodríguez-Diéguez, A. Photoluminescent Coordination Polymers Based on Group 12 Metals and 1H-Indazole-6-Carboxylic Acid. Inorganics 2021, 9, 20–32. [Google Scholar] [CrossRef]
- Cepeda, J.; Rodríguez-Diéguez, A. Tuning the luminescence performance of metal-organic frameworks based on d10metal ions: From an inherent versatile behaviour to their response to external stimuli. CrystEngComm 2016, 18, 8556–8573. [Google Scholar] [CrossRef]
- Barbieri, A.; Accorsi, G.; Armaroli, N. Luminescent complexes beyond the platinum group: The d10 avenue. Chem. Commun. 2008, 252, 2185–2193. [Google Scholar] [CrossRef] [PubMed]
- Echenique-Errandonea, E.; Pérez, J.M.; Rojas, S.; Cepeda, J.; Seco, J.M.; Fernández, I.; Rodríguez-Diéguez, A. A novel yttrium-based metal-organic framework for the efficient solvent-free catalytic synthesis of cyanohydrin silyl ethers. Dalton Trans. 2021, 50, 11720–11724. [Google Scholar] [CrossRef]
- Earnshaw, A. Introduction to Magnetochemistry; Academic Press: London, UK, 1968. [Google Scholar]
- Bruker APEX2 (software). Bruker Corporation: Madison, WI, USA, 2012.
- Sheldrick, G.M. SADABS Empirical Absorption Program; University of Göttingen: Göttingen, Germany, 1996. [Google Scholar]
- Sheldrick, G.M. SHELXTL, Version 2014/7; Bruker AXS, Inc.: Madison, WI, USA, 2014. [Google Scholar]
- Farrugia, L.J. WinGX and ORTEP for Windows: An update. J. Appl. Crystallogr. 2012, 45, 849–854. [Google Scholar] [CrossRef]
- Rodríguez-Carvajal, J. FULLPROF 2000, Version 2.5d; Lab. Léon Brillouin (CEA-CNRS), Cent. d’Études Saclay: Gif sur Yvette, France, 2000. [Google Scholar]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Petersson, G.A.; Nakatsuji, H.; et al. Gaussian 16 Revision C.01; Gaussian Inc.: Wallingford, CT, USA, 2016. [Google Scholar]
- Becke, A.D. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A 1988, 38, 3098–3100. [Google Scholar] [CrossRef]
- Schäfer, A.; Horn, H.; Ahlrichs, R. Fully optimized contracted Gaussian basis sets for atoms Li to Kr. J. Chem. Phys. 1992, 97, 2571–2577. [Google Scholar] [CrossRef]
- Rassolov, V.A.; Pople, J.A.; Ratner, M.A.; Windus, T.L. 6-31G* basis set for atoms K through Zn. J. Chem. Phys. 1998, 109, 1223–1229. [Google Scholar] [CrossRef]
- Ruiz, E.; Cano, J.; Alvarez, S.; Alemany, P. Broken symmetry approach to calculation of exchange coupling constants for homobinuclear and heterobinuclear transition metal complexes. J. Comput. Chem. 1999, 20, 1391–1400. [Google Scholar] [CrossRef]
- Noodleman, L. Valence bond description of antiferromagnetic coupling in transition metal dimers. J. Chem. Phys. 1981, 74, 5737–5743. [Google Scholar] [CrossRef]
- Neese, F.; Wennmohs, F.; Becker, U.; Riplinger, C. The ORCA quantum chemistry program package. J. Chem. Phys. 2020, 152, 224108. [Google Scholar] [CrossRef] [PubMed]
- Neese, F. The ORCA program system. WIREs Comput. Mol. Sci. 2012, 2, 73–78. [Google Scholar] [CrossRef]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- van Wüllen, C. Molecular density functional calculations in the regular relativistic approximation: Method, application to coinage metal diatomics, hydrides, fluorides and chlorides, and comparison with first-order relativistic calculations. J. Chem. Phys. 1998, 109, 392–399. [Google Scholar] [CrossRef]
- Weigend, F.; Ahlrichs, R. Balanced basis sets of split valence, triple zeta valence and quadruple zeta valence quality for H to Rn: Design and assessment of accuracy. Phys. Chem. Chem. Phys. 2005, 7, 3297–3305. [Google Scholar] [CrossRef]
- Weigend, F. Accurate Coulomb-fitting basis sets for H to Rn. Phys. Chem. Chem. Phys. 2006, 8, 1057–1065. [Google Scholar] [CrossRef]
- Hellweg, A.; Hättig, C.; Höfener, S.; Klopper, W. Optimized accurate auxiliary basis sets for RI-MP2 and RI-CC2 calculations for the atoms Rb to Rn. Theor. Chem. Acc. 2007, 117, 587–597. [Google Scholar] [CrossRef]
- Deng, Y.-F.; Singh, M.K.; Gan, D.; Xiao, T.; Wang, Y.; Liu, S.; Wang, Z.; Ouyang, Z.; Zhang, Y.-Z.; Dunbar, K.R. Probing the Axial Distortion Effect on the Magnetic Anisotropy of Octahedral Co(II) Complexes. Inorg. Chem. 2020, 59, 7622–7630. [Google Scholar] [CrossRef]
- Angeli, C.; Borini, S.; Cestari, M.; Cimiraglia, R. A quasidegenerate formulation of the second order n-electron valence state perturbation theory approach. J. Chem. Phys. 2004, 121, 4043–4049. [Google Scholar] [CrossRef] [PubMed]
- Maganas, D.; Sottini, S.; Kyritsis, P.; Groenen, E.J.J.; Neese, F. Theoretical Analysis of the Spin Hamiltonian Parameters in Co(II)S4 Complexes, Using Density Functional Theory and Correlated ab initio Methods. Inorg. Chem. 2011, 50, 8741–8754. [Google Scholar] [CrossRef] [PubMed]
- Ungur, L.; Chibotaru, L.F. Ab Initio Crystal Field for Lanthanides. Chem. A Eur. J. 2017, 23, 3708–3718. [Google Scholar] [CrossRef] [PubMed]
- Chibotaru, L.F.; Ungur, L. Ab initio calculation of anisotropic magnetic properties of complexes. I. Unique definition of pseudospin Hamiltonians and their derivation. J. Chem. Phys. 2012, 137, 64112. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Echenique-Errandonea, E.; Rojas, S.; Cepeda, J.; Choquesillo-Lazarte, D.; Rodríguez-Diéguez, A.; Seco, J.M. Slow Magnetic Relaxation and Modulated Photoluminescent Emission of Coordination Polymer Based on 3-Amino-4-hydroxybenzoate Zn and Co Metal Ions. Molecules 2023, 28, 1846. https://doi.org/10.3390/molecules28041846
Echenique-Errandonea E, Rojas S, Cepeda J, Choquesillo-Lazarte D, Rodríguez-Diéguez A, Seco JM. Slow Magnetic Relaxation and Modulated Photoluminescent Emission of Coordination Polymer Based on 3-Amino-4-hydroxybenzoate Zn and Co Metal Ions. Molecules. 2023; 28(4):1846. https://doi.org/10.3390/molecules28041846
Chicago/Turabian StyleEchenique-Errandonea, Estitxu, Sara Rojas, Javier Cepeda, Duane Choquesillo-Lazarte, Antonio Rodríguez-Diéguez, and José M. Seco. 2023. "Slow Magnetic Relaxation and Modulated Photoluminescent Emission of Coordination Polymer Based on 3-Amino-4-hydroxybenzoate Zn and Co Metal Ions" Molecules 28, no. 4: 1846. https://doi.org/10.3390/molecules28041846
APA StyleEchenique-Errandonea, E., Rojas, S., Cepeda, J., Choquesillo-Lazarte, D., Rodríguez-Diéguez, A., & Seco, J. M. (2023). Slow Magnetic Relaxation and Modulated Photoluminescent Emission of Coordination Polymer Based on 3-Amino-4-hydroxybenzoate Zn and Co Metal Ions. Molecules, 28(4), 1846. https://doi.org/10.3390/molecules28041846








