Probing Methyl Group Tunneling in [(CH3)2NH2][Zn(HCOO)3] Hybrid Perovskite Using Co2+ EPR
Abstract
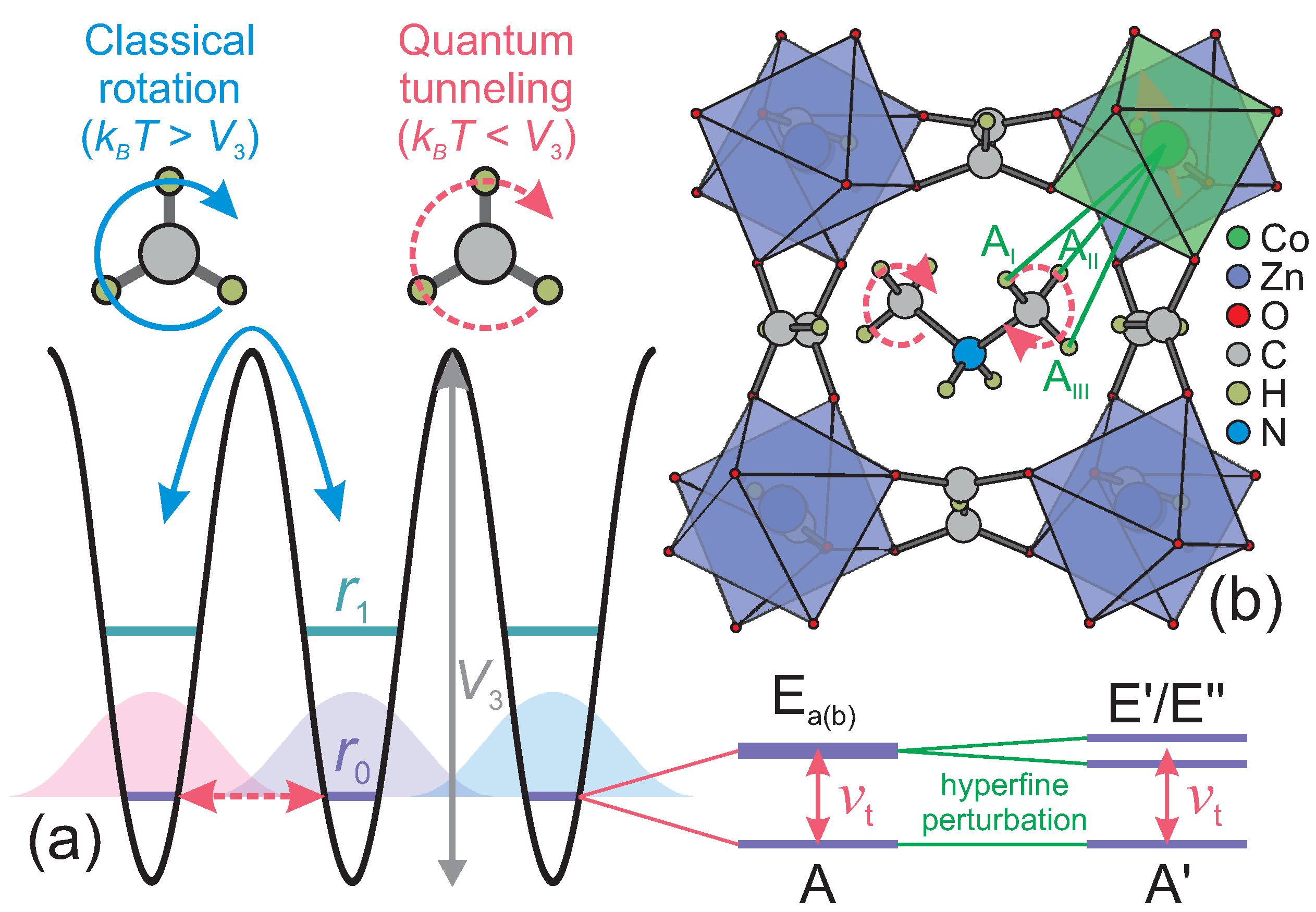
1. Introduction
2. Results and Discussion
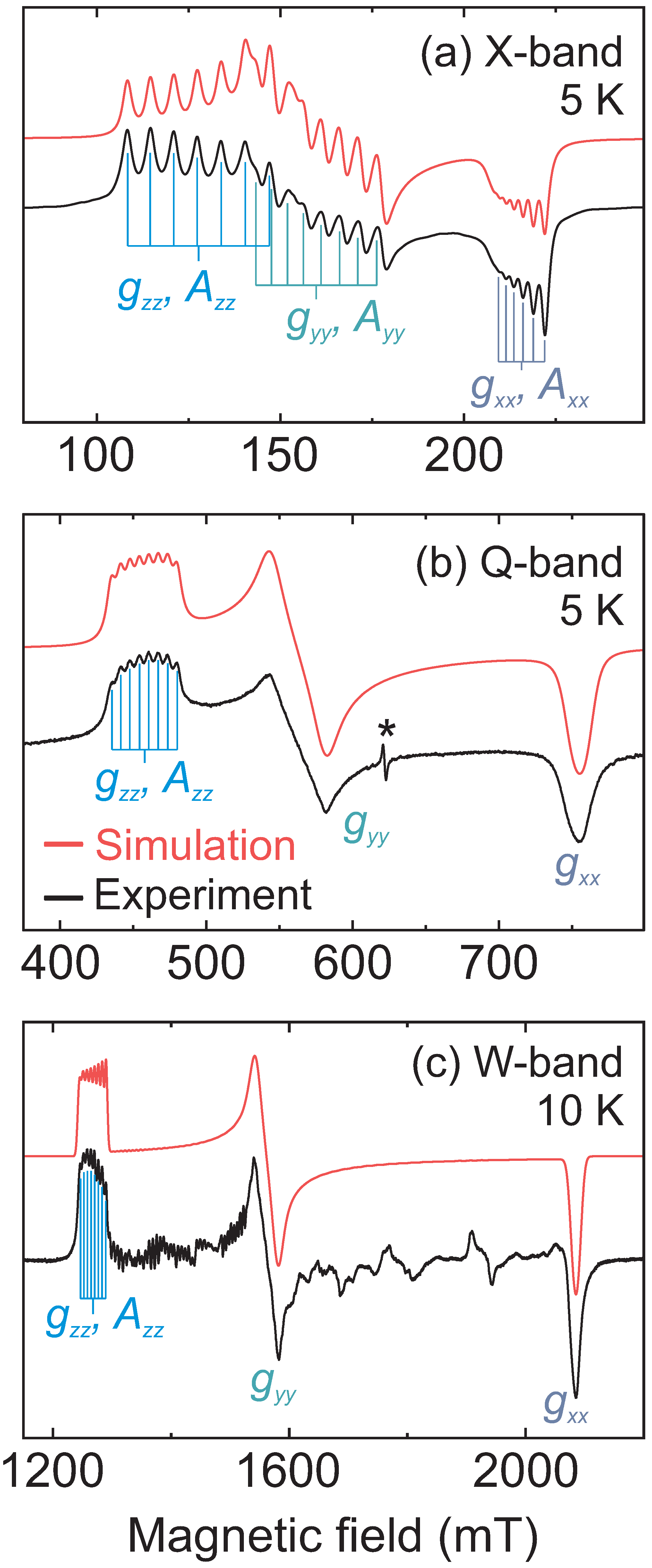
2.1. Spin Hamiltonian
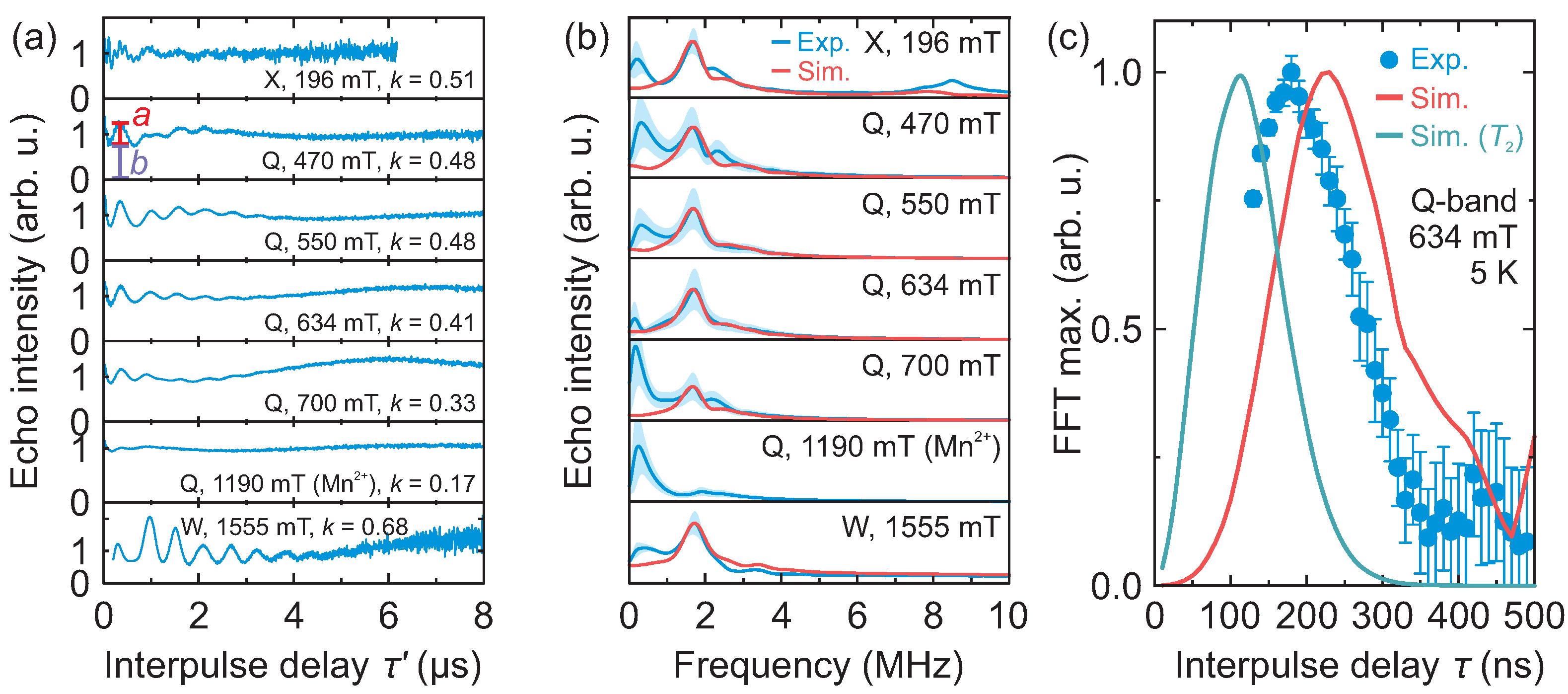
2.2. Relaxation Properties
2.3. Methyl Group Tunneling
3. Experimental and Simulation Details
3.1. Sample Synthesis and Characterization
3.2. EPR Spectroscopy
3.3. Simulation Details
4. Summary and Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| DMAZn | Dimethylammonium zinc formate |
| DMACo | Dimethylammonium cobalt formate |
| DMAMn | Dimethylammonium manganese formate |
| 3p ESEEM | Three pulse electron spin echo envelope modulation |
| EPR | Electron paramagnetic resonance |
| CW | Continuous wave |
| ENDOR | Electron nuclear double resonance |
| NMR | Nuclear magnetic resonance |
| DFT | Density functional theory |
References
- Horsewill, A. Quantum tunnelling aspects of methyl group rotation studied by NMR. Prog. Nucl. Magn. Reson. Spectrosc. 1999, 35, 359–389. [Google Scholar] [CrossRef]
- Dimeo, R.M. Visualization and measurement of quantum rotational dynamics. Am. J. Phys. 2003, 71, 885–893. [Google Scholar] [CrossRef]
- Latanowicz, L. NMR relaxation study of methyl groups in solids from low to high temperatures. Concepts Magn. Reson. Part A 2005, 27A, 38–53. [Google Scholar] [CrossRef]
- Prager, M.; Heidemann, A. Rotational Tunneling and Neutron Spectroscopy: A Compilation. Chem. Rev. 1997, 97, 2933–2966. [Google Scholar] [CrossRef] [PubMed]
- Neumann, M.; Johnson, M.R. Methyl group tunneling - A quantitative probe of atom-atom potentials. J. Chem. Phys. 1997, 107, 1725–1731. [Google Scholar] [CrossRef]
- Spangler, L.H. Structural information from methyl internal rotation spectroscopy. Annu. Rev. Phys. Chem. 1997, 48, 481–510. [Google Scholar] [CrossRef] [PubMed]
- Peksa, P.; Nowok, A.; Formalik, F.; Zaręba, J.K.; Trzmiel, J.; Gągor, A.; Mączka, M.; Sieradzki, A. More complex than originally thought: Revisiting the origins of the relaxation processes in dimethylammonium zinc formate. J. Mater. Chem. C 2022, 10, 6866–6877. [Google Scholar] [CrossRef]
- Inaba, A. Calorimetric studies of tunneling phenomena. Phys. B 1994, 202, 325–331. [Google Scholar] [CrossRef]
- Trapani, A.P.; Strauss, H.L. Infrared structural holeburning and orientational tunneling of NH3D+ ions in ammonium cobalt sulfate. J. Chem. Phys. 1987, 87, 1899–1900. [Google Scholar] [CrossRef]
- Clough, S.; Hill, J.; Poldy, F. Tunnelling sidebands of methyl group hyperfine structure. J. Phys. C Solid State Phys. 1972, 5, 1739. [Google Scholar] [CrossRef]
- Geoffroy, M.; Kispert, L.D.; Hwang, J.S. An ESR, ENDOR, and ELDOR study of tunneling rotation of a hindered methyl group in x-irradiated 2,2,5-trimethyl-1,3-dioxane-4,6-dione crystals. J. Chem. Phys. 1979, 70, 4238–4242. [Google Scholar] [CrossRef]
- Clough, S.; Poldy, F. Study of Tunneling Rotation of Methyl Groups by Electron Spin Resonance and Electron Nuclear Double Resonance. J. Chem. Phys. 1969, 51, 2076–2084. [Google Scholar] [CrossRef]
- Clough, S.; Hill, J.; Poldy, F. Methyl group tunnelling rotation frequency in γ-irradiated methyl malonic acid crystals. J. Phys. C Solid State Phys. 1972, 5, 518. [Google Scholar] [CrossRef]
- Bonon, F.; Brustolon, M.; Maniero, A.; Segre, U. An ENDOR study of the temperature dependence of methyl tunnelling. Chem. Phys. 1992, 161, 257–263. [Google Scholar] [CrossRef]
- Martínez, J.I.; Alonso, P.J.; García-Rubio, I.; Medina, M. Methyl rotors in flavoproteins. Phys. Chem. Chem. Phys. 2014, 16, 26203–26212. [Google Scholar] [CrossRef]
- Šimėnas, M.; Macalik, L.; Aidas, K.; Kalendra, V.; Klose, D.; Jeschke, G.; Mączka, M.; Völkel, G.; Banys, J.; Pöppl, A. Pulse EPR and ENDOR Study of Manganese Doped [(CH3)2NH2][Zn(HCOO)3] Hybrid Perovskite Framework. J. Phys. Chem. C 2017, 121, 27225–27232. [Google Scholar] [CrossRef]
- Šimėnas, M.; Klose, D.; Ptak, M.; Aidas, K.; Mączka, M.; Banys, J.; Pöppl, A.; Jeschke, G. Magnetic excitation and readout of methyl group tunnel coherence. Sci. Adv. 2020, 6, eaba1517. [Google Scholar] [CrossRef] [PubMed]
- Deligiannakis, Y.; Louloudi, M.; Hadjiliadis, N. Electron spin echo envelope modulation (ESEEM) spectroscopy as a tool to investigate the coordination environment of metal centers. Coord. Chem. Rev. 2000, 204, 1–112. [Google Scholar] [CrossRef]
- Šimėnas, M.; Ciupa, A.; Usevičius, G.; Aidas, K.; Klose, D.; Jeschke, G.; Mączka, M.; Völkel, G.; Pöppl, A.; Banys, J. Electron paramagnetic resonance of a copper doped [(CH3)2NH2][Zn(HCOO)3] hybrid perovskite framework. Phys. Chem. Chem. Phys. 2018, 20, 12097–12105. [Google Scholar] [CrossRef]
- Lien, S.Y.; Wang, C.W.; Chen, W.R.; Liu, C.H.; Kang, C.C.; Huang, C.J. The Influence of Oxygen Plasma on Methylammonium Lead Iodide (MAPbI3) Film Doped with Lead Cesium Triiodide (CsPbI3). Molecules 2021, 26, 5133. [Google Scholar] [CrossRef] [PubMed]
- Ptak, M.; Sieradzki, A.; Šimėnas, M.; Maczka, M. Molecular Spectroscopy of Hybrid Organic-Inorganic Perovskites and Related Compounds. Coord. Chem. Rev. 2021, 448, 214180. [Google Scholar] [CrossRef]
- Šimėnas, M.; Balciunas, S.; Wilson, J.N.; Svirskas, S.; Kinka, M.; Garbaras, A.; Kalendra, V.; Gagor, A.; Szewczyk, D.; Sieradzki, A.; et al. Suppression of Phase Transitions and Glass Phase Signatures in Mixed Cation Halide Perovskites. Nat. Commun. 2020, 11, 5103. [Google Scholar] [CrossRef]
- Šimėnas, M.; Balčiūnas, S.; Gągor, A.; Pieniążek, A.; Tolborg, K.; Kinka, M.; Klimavicius, V.; Svirskas, S.; Kalendra, V.; Ptak, M.; et al. Mixology of MA1-xEAxPbI3 Hybrid Perovskites: Phase Transitions, Cation Dynamics, and Photoluminescence. Chem. Mat. 2022, 34, 10104–10112. [Google Scholar] [CrossRef]
- Jain, P.; Dalal, N.S.; Toby, B.H.; Kroto, H.W.; Cheetham, A.K. Order-Disorder Antiferroelectric Phase Transition in a Hybrid Inorganic-Organic Framework with the Perovskite Architecture. J. Am. Chem. Soc. 2008, 130, 10450–10451. [Google Scholar] [CrossRef]
- Šimėnas, M.; Ciupa, A.; Mączka, M.; Pöppl, A.; Banys, J. EPR Study of Structural Phase Transition in Manganese-Doped [(CH3)2NH2][Zn(HCOO)3] Metal-Organic Framework. J. Phys. Chem. C 2015, 119, 24522–24528. [Google Scholar] [CrossRef]
- Šimėnas, M.; Kultaeva, A.; Balčiūnas, S.; Trzebiatowska, M.; Klose, D.; Jeschke, G.; Mączka, M.; Banys, J.; Pöppl, A. Single Crystal Electron Paramagnetic Resonance of Dimethylammonium and Ammonium Hybrid Formate Frameworks: Influence of External Electric Field. J. Phys. Chem. C 2017, 121, 16533–16540. [Google Scholar] [CrossRef]
- Šimėnas, M.; Balčiūnas, S.; Ciupa, A.; Vilčiauskas, L.; Jablonskas, D.; Kinka, M.; Sieradzki, A.; Samulionis, V.; Mączka, M.; Banys, J. Elucidation of Dipolar Dynamics and the Nature of Structural Phases in the [(CH3)2NH2][Zn(HCOO)3] Hybrid Perovskite Framework. J. Mater. Chem. C 2019, 7, 6779–6785. [Google Scholar] [CrossRef]
- Banci, L.; Bencini, A.; Benelli, C.; Gatteschi, D.; Zanchini, C. Spectral-Structural Correlations in High-Spin Cobalt(II) Complexes; Springer: Berlin/Heidelberg, Germany, 1982; pp. 37–86. [Google Scholar]
- Makinen, M.W.; Kuo, L.C.; Yim, M.B.; Wells, G.B.; Fukuyama, J.M.; Kim, J.E. Ground term splitting of high-spin cobalt(2+) ion as a probe of coordination structure. 1. Dependence of the splitting on coordination geometry. J. Am. Chem. Soc. 1985, 107, 5245–5255. [Google Scholar] [CrossRef]
- Pilbrow, J. Transition Ion Electron Paramagnetic Resonance; Oxford Science Publications, Clarendon Press: Oxford, UK, 1990. [Google Scholar]
- Lässig, D.; Lincke, J.; Griebel, J.; Kirmse, R.; Krautscheid, H. Synthesis, Crystal Structure, and Electron Paramagnetic Resonance Investigations of Heteronuclear CoII/ZnII and CoII/CdII Coordination Polymers. Inorg. Chem. 2011, 50, 213–219. [Google Scholar] [CrossRef]
- Misochko, E.Y.; Akimov, A.V.; Korchagin, D.V.; Nehrkorn, J.; Ozerov, M.; Palii, A.V.; Clemente-Juan, J.M.; Aldoshin, S.M. Purely Spectroscopic Determination of the Spin Hamiltonian Parameters in High-Spin Six-Coordinated Cobalt(II) Complexes with Large Zero-Field Splitting. Inorg. Chem. 2019, 58, 16434–16444. [Google Scholar] [CrossRef]
- Hughey, K.D.; Clune, A.J.; Yokosuk, M.O.; Li, J.; Abhyankar, N.; Ding, X.; Dalal, N.S.; Xiang, H.; Smirnov, D.; Singleton, J.; et al. Structure-Property Relations in Multiferroic [(CH3)2NH2]M(HCOO)3 (M = Mn, Co, Ni). Inorg. Chem. 2018, 57, 11569–11577. [Google Scholar] [CrossRef]
- Šimėnas, M.; Ptak, M.; Khan, A.H.; Dagys, L.; Balevičius, V.; Bertmer, M.; Völkel, G.; Mączka, M.; Pöppl, A.; Banys, J. Spectroscopic Study of [(CH3)2NH2][Zn(HCOO)3] Hybrid Perovskite Containing Different Nitrogen Isotopes. J. Phys. Chem. C 2018, 122, 10284–10292. [Google Scholar] [CrossRef]
- Orio, M.; Bindra, J.K.; van Tol, J.; Giorgi, M.; Dalal, N.S.; Bertaina, S. Quantum dynamics of Mn2+ in dimethylammonium magnesium formate. J. Chem. Phys. 2021, 154, 154201. [Google Scholar] [CrossRef]
- Abragam, A.; Bleaney, B. Electron Paramagnetic Resonance of Transition Ions; Oxford University Press: Oxford, UK, 2012. [Google Scholar]
- Mabbs, F.E.; Collison, D. (Eds.) Electron Paramagnetic Resonance of d Transition Metal Compounds; Elsevier Science: Amsterdam, The Netherlands, 1992. [Google Scholar]
- Pilbrow, J. Effective g values for S = 3/2 and S = 5/2. J. Magn. Reson. (1969) 1978, 31, 479–490. [Google Scholar] [CrossRef]
- Fu, D.W.; Zhang, W.; Cai, H.L.; Zhang, Y.; Ge, J.Z.; Xiong, R.G.; Huang, S.D.; Nakamura, T. A Multiferroic Perdeutero Metal-Organic Framework. Angew. Chem. Int. Ed. 2011, 50, 11947–11951. [Google Scholar] [CrossRef]
- Völkel, G.; Müller, H.E.; Flohrer, W. Critical Anomaly of the Electron Spin-Lattice Relaxation in X-Irradiated Rochelle Salt. Phys. Status Solidi B 1981, 108, 501–506. [Google Scholar] [CrossRef]
- Šimėnas, M.; Balčiūnas, S.; Trzebiatowska, M.; Ptak, M.; Mączka, M.; Völkel, G.; Pöppl, A.; Banys, J. Electron paramagnetic resonance and electric characterization of a [CH3NH2NH2][Zn(HCOO)3] perovskite metal formate framework. J. Mater. Chem. C 2017, 5, 4526–4536. [Google Scholar] [CrossRef]
- Orbach, R. Spin-lattice relaxation in rare-earth salts. Proc. R. Soc. Lond. Ser. A 1961, 264, 458–484. [Google Scholar]
- Finn, C.B.P.; Orbach, R.; Wolf, W.P. Spin-Lattice Relaxation in Cerium Magnesium Nitrate at Liquid Helium Temperature: A New Process. Proc. Phys. Soc. Lond. 1961, 77, 261. [Google Scholar] [CrossRef]
- Tyryshkin, A.M.; Tojo, S.; Morton, J.J.L.; Riemann, H.; Abrosimov, N.V.; Becker, P.; Pohl, H.J.; Schenkel, T.; Thewalt, M.L.W.; Itoh, K.M.; et al. Electron spin coherence exceeding seconds in high-purity silicon. Nat. Mater. 2012, 11, 143–147. [Google Scholar] [CrossRef]
- Boström, H.L.B.; Kieslich, G. Influence of Metal Defects on the Mechanical Properties of ABX3 Perovskite-Type Metal-formate Frameworks. J. Phys. Chem. C 2021, 125, 1467–1471. [Google Scholar] [CrossRef]
- Guo, Z.; Wang, J.; Yin, W.J. Atomistic origin of lattice softness and its impact on structural and carrier dynamics in three dimensional perovskites. Energy Environ. Sci. 2022, 15, 660–671. [Google Scholar] [CrossRef]
- Mączka, M.; Ptak, M.; Macalik, L. Infrared and Raman Studies of Phase Transitions in Metal-Organic Frameworks of [(CH3)2NH2][M(HCOO)3] with M=Zn, Fe. Vib. Spectrosc. 2014, 71, 98–104. [Google Scholar] [CrossRef]
- Van Doorslaer, S.; Sierra, G.; Schweiger, A. Dead Time-Dependent Line Distortions in Absolute-Value Electron Spin Echo Envelope Modulation Spectra. J. Magn. Reson. 1999, 136, 152–158. [Google Scholar] [CrossRef] [PubMed]
- Stoll, S.; Schweiger, A. EasySpin, a Comprehensive Software Package for Spectral Simulation and Analysis in EPR. J. Magn. Reson. 2006, 178, 42–55. [Google Scholar] [CrossRef] [PubMed]
- Jeschke, G. Rotational Coupling in Methyl-Tunneling Electron Spin Echo Envelope Modulation. Appl. Magn. Reson. 2022, 53, 635–651. [Google Scholar] [CrossRef] [PubMed]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Usevičius, G.; Eggeling, A.; Pocius, I.; Kalendra, V.; Klose, D.; Mączka, M.; Pöppl, A.; Banys, J.; Jeschke, G.; Šimėnas, M. Probing Methyl Group Tunneling in [(CH3)2NH2][Zn(HCOO)3] Hybrid Perovskite Using Co2+ EPR. Molecules 2023, 28, 979. https://doi.org/10.3390/molecules28030979
Usevičius G, Eggeling A, Pocius I, Kalendra V, Klose D, Mączka M, Pöppl A, Banys J, Jeschke G, Šimėnas M. Probing Methyl Group Tunneling in [(CH3)2NH2][Zn(HCOO)3] Hybrid Perovskite Using Co2+ EPR. Molecules. 2023; 28(3):979. https://doi.org/10.3390/molecules28030979
Chicago/Turabian StyleUsevičius, Gediminas, Andrea Eggeling, Ignas Pocius, Vidmantas Kalendra, Daniel Klose, Mirosław Mączka, Andreas Pöppl, Jūras Banys, Gunnar Jeschke, and Mantas Šimėnas. 2023. "Probing Methyl Group Tunneling in [(CH3)2NH2][Zn(HCOO)3] Hybrid Perovskite Using Co2+ EPR" Molecules 28, no. 3: 979. https://doi.org/10.3390/molecules28030979
APA StyleUsevičius, G., Eggeling, A., Pocius, I., Kalendra, V., Klose, D., Mączka, M., Pöppl, A., Banys, J., Jeschke, G., & Šimėnas, M. (2023). Probing Methyl Group Tunneling in [(CH3)2NH2][Zn(HCOO)3] Hybrid Perovskite Using Co2+ EPR. Molecules, 28(3), 979. https://doi.org/10.3390/molecules28030979









