Solubility and Thermodynamics Data of Cabozantinib Malate in Various Aqueous Solutions of Dimethyl Sulfoxide at Different Temperatures
Abstract
:1. Introduction
2. Results and Discussion
2.1. CBZM Solid-Phase Characterization and Measured Solubility Data
2.2. Assessment of Hansen Solubility Parameters (HSPs)
2.3. Ideal Solubility (xidl) and Activity Coefficients (γi) Data to Derive Molecular Interactions
2.4. Correlation of CBZM Solubility Data
2.5. Thermodynamic Data for CBZM Dissolution
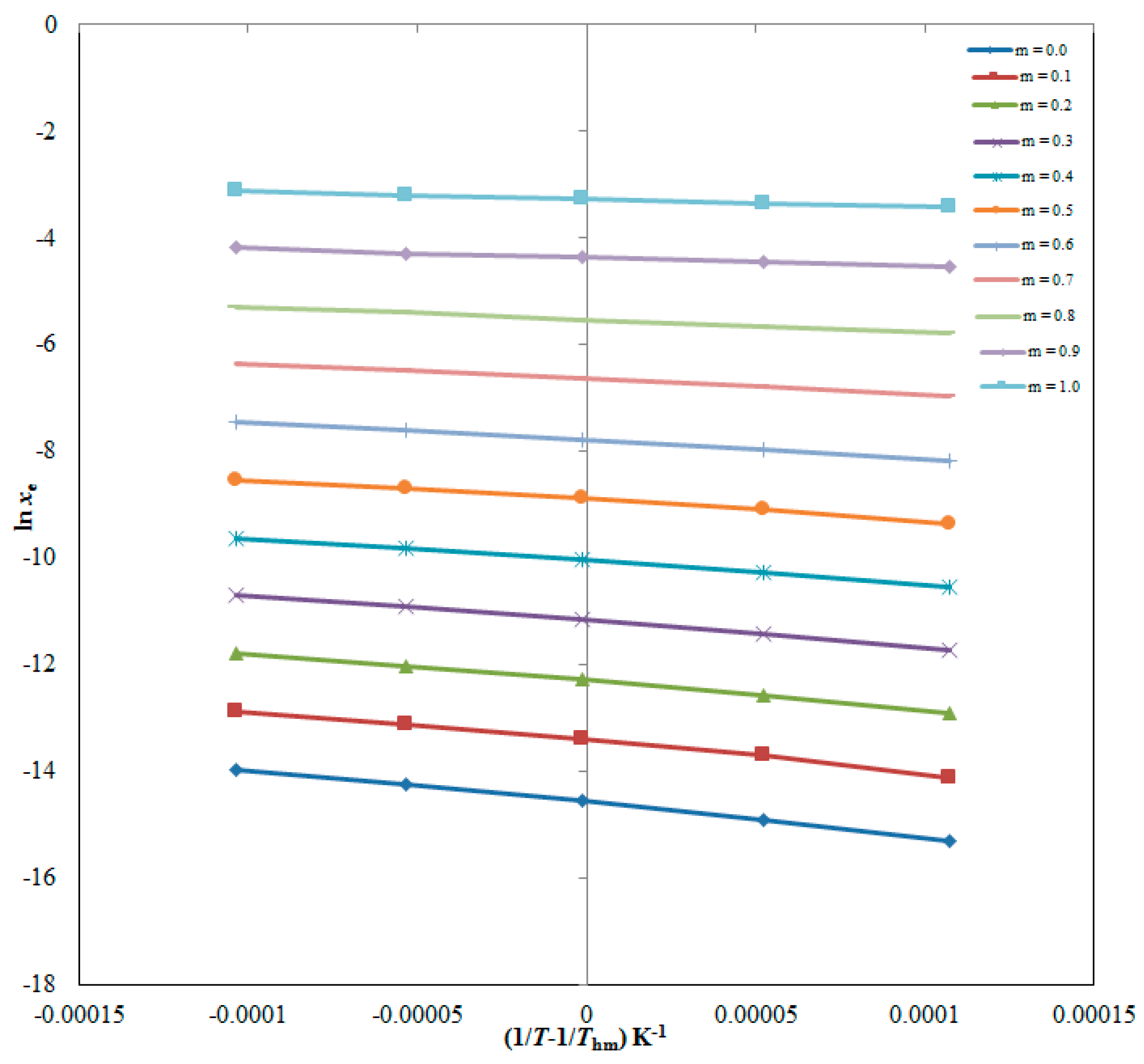
2.6. Enthalpy–Entropy Compensation Analyses
3. Materials and Methods
3.1. Materials
3.2. Measurement of CBZM Solubility in DMSO Aqueous Solutions and Pure Solvents
3.3. HSPs of CBZM and Various DMSO Aqueous Solutions
3.4. CBZM xidl and γi Data to Derive Molecular Interactions
3.5. Computational Analysis
3.6. Apparent Thermodynamic Analyses
3.7. Enthalpy-Entropy Compensation Analyses
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| CBZM | Cabozantinib malate |
| DMSO | Dimethyl sulfoxide |
| H2O | Water |
| smTKI | Small molecule tyrosine kinase inhibitor |
| ARC | Advanced renal carcinoma |
| CRPC | Castration-resistant prostate carcinoma |
| MTC | Medullary thyroid cancer |
| HCC | Hepatocellular carcinoma |
| DSC | Differential scanning calorimetry |
| m | DMSO mass fraction in {DMSO + H2O} mixtures |
| T | Absolute temperature (K) |
| u(T) | Uncertainty in temperature (K) |
| u(m) | Uncertainty in DMSO mass fraction |
| u(p) | Uncertainty in atmospheric pressure (kPa) |
| xe | Experimental mole fraction solubility of CBZM |
| U(xe) | Relative uncertainty in CBZM solubility |
| xidl | Ideal solubility of CBZM in mole fraction |
| HSP | Hansen solubility parameter |
| δt | Total HSP for CBZM (MPa1/2) |
| δ1 | HSP of neat DMSO (MPa1/2) |
| δ2 | HSP of neat water (MPa1/2) |
| δmix | HSP for {DMSO + H2O} mixtures free of CBZM (MPa1/2) |
| α | DMSO volume fraction in {DMSO + H2O} mixtures |
| γi | Activity coefficient of CBZM |
| R2 | Correlation coefficient for CBZM |
| RMSD | Root mean square deviations (%) |
| a and b | Parameters of the van’t Hoff model |
| A, B, and C | Parameters of the Apelblat model |
| λ and h | Parameters of the Buchowski–Ksiazaczak λh model |
| Ji | Parameter of the Jouyban–Acree model |
| A1, B1, A2 and B2 | Parameter of the Jouyban–Acree-Van’t Hoff model |
| xVan’t | Van’t Hoff model solubility of CBZM |
| xApl | Apelblat model solubility of CBZM |
| xYal | Yalkowsky model solubility of CBZM |
| xm,T | Jouyban–Acree model solubility of CBZM |
| ΔsolH0 | Apparent standard enthalpy of CBZM (kJ mol−1) |
| ΔsolG0 | Apparent standard Gibbs energy of CBZM (kJ mol−1) |
| ΔsolS0 | Apparent standard entropy of CBZM (J mol−1 K−1) |
| u(ΔsolH0) | Relative uncertainty in ΔsolH0 |
| u(ΔsolG0) | Relative uncertainty in ΔsolG0 |
| u(ΔsolS0) | Relative uncertainty in ΔsolS0 |
| Thm | Mean harmonic temperature (K) |
| Tfus | CBZM fusion temperature (K) |
| R | Universal gas constant (J mol−1 K−1) |
| ΔHfus | CBZM molar fusion enthalpy (kJ mol−1) |
| ΔCp | Difference in molar heat capacity of CBZM (J mol−1 K−1) |
| x1 | Mole fraction of CBZM in neat DMSO |
| x2 | Mole fraction of CBZM in neat H2O |
| w1 | DMSO mass fraction |
| w2 | H2O mass fraction |
References
- Williams, H.D.; Ford, L.; Han, S.; Tangso, K.J.; Lim, S.; Shackleford, D.M.; Vodak, D.T.; Benameur, H.; Pouton, C.W.; Scammells, P.J.; et al. Enhancing the oral absorption of kinase inhibitors using lipophilic salts and lipid-based formulations. Mol. Pharm. 2018, 15, 5678–5696. [Google Scholar] [CrossRef] [PubMed]
- US. Department of Health and Human Services; Food and Drug Administration (FDA); Center for Drug Evaluation and Research (CDER). Cabometyx® (Cabozantinib) Tablets, for Oral Use. Guidance for Industry; Draft guidance; Food and Drug Administration (FDA): Washington, DC, USA, 2017.
- Cohen, P. Protein kinases-the major drug targets of the twenty first century? Nat. Rev. Drug Discov. 2002, 1, 309–315. [Google Scholar] [CrossRef] [PubMed]
- Levitzki, A.; Gazit, A. Tyrosine kinase inhibition: An approach to drug development. Science 1995, 267, 1782. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Yang, P.L.; Gray, N.S. Targeting cancer with small molecule kinase inhibitors. Nat. Rev. Cancer 2009, 9, 28–39. [Google Scholar] [CrossRef] [PubMed]
- Ibrahim, N. Cabozantinib: Cabometyx versus cometriq. Are they interchangeable? Glob. J. Med. Ther. 2019, 1, 5–7. [Google Scholar] [CrossRef]
- Di, L.; Fish, P.V.; Mano, T. Bridging solubility between drug discovery and development. Drug Discov. Today 2012, 17, 486–495. [Google Scholar] [CrossRef] [PubMed]
- Rezaei, H.; Rahimpour, E.; Zhao, H.; Martinez, F.; Barzegar-Jalali, M.; Jouyban, A. Solubility of baclofen in some neat and mixed solvents at different temperatures. J. Mol. Liq. 2022, 347, 118352. [Google Scholar] [CrossRef]
- Barrett, J.A.; Yang, W.; Skolnik, S.M.; Belliveau, L.M.; Patros, K.M. Discovery solubility measurement and assessment of small molecules with drug development in mind. Drug Discov. Today 2022, 27, 1315–1325. [Google Scholar] [CrossRef]
- Soliman, M.E.; Adewumi, A.T.; Akawa, O.B.; Subair, T.I.; Okunlola, F.O.; Akinsuku, A.E.; Khan, S. Simulation models for prediction of bioavailability of medicinal drugs-the interface between experiment and computation. AAPS PharmSciTech 2022, 23, 86. [Google Scholar] [CrossRef]
- Yadav, K.; Sachan, A.K.; Kumar, S.; Dubey, A. Techniques for increasing solubility: A review of conventional and new strategies. Asian J. Pharm. Res. Dev. 2022, 10, 144–153. [Google Scholar] [CrossRef]
- Jouyban, A. Review of the cosolvency models for predicting drug solubility in solvent mixtures: An update. J. Pharm. Pharm. Sci. 2019, 22, 466–485. [Google Scholar] [CrossRef]
- Bolla, G.; Nangia, A. Pharmaceutical cocrystals: Walking the talk. Chem. Commun. 2016, 52, 8342–8360. [Google Scholar] [CrossRef] [PubMed]
- Bolla, G.; Sarma, B.; Nangia, A.K. Crystal engineering of pharmaceutical cocrystals in the discovery and development of improved drugs. Chem. Rev. 2022, 122, 11514–11603. [Google Scholar] [CrossRef] [PubMed]
- Duggirala, N.K.; Perry, M.L.; Almarsson, O.; Zaworotko, M.J. Pharmaceutical cocrystals: Along with the path to improve medicines. Chem. Commun. 2016, 52, 640–655. [Google Scholar] [CrossRef] [PubMed]
- Paus, R.; Hart, E.; Ji, Y.; Sadowski, G. Solubility and caloric properties of cinnarizine. J. Chem. Eng. Data 2015, 60, 2256–2261. [Google Scholar] [CrossRef]
- Ruether, F.; Sadowski, G. Modeling the solubility of pharmaceuticals in pure solvents and solvent mixtures for drug process design. J. Pharm. Sci. 2009, 98, 4205–4215. [Google Scholar] [CrossRef] [PubMed]
- Alyamani, M.; Alshehri, S.; Alam, P.; Wani, S.U.D.; Ghoneim, M.M.; Shakeel, F. Solubility and solution thermodynamics of raloxifene hydrochloride in various (DMSO + water) compositions. Alexand. Eng. J. 2022, 61, 9119–9128. [Google Scholar] [CrossRef]
- Yang, Q.; Moulder, R.K.; Cohen, M.S.; Cai, S.; Forrest, L.M. Cabozantinib loaded DSPE-PEG2000 micelles as delivery systems: Formulation, characterization and cytotoxicity evaluation. BAOJ Pharm. Sci. 2015, 1, E001. [Google Scholar]
- Shakeel, F.; Haq, N.; Alsarra, I.A. Equilibrium solubility determination, Hansen solubility parameters and solution thermodynamics of cabozantinib malate in different monosolvents of pharmaceutical importance. J. Mol. Liq. 2021, 324, E115146. [Google Scholar] [CrossRef]
- Alsenz, J.; Kansy, M. High throughput solubility measurement in drug discovery and development. Adv. Drug Deliv. Rev. 2007, 59, 546–567. [Google Scholar] [CrossRef]
- Wernersson, S.; Birgersson, S.; Akke, M. Cosolvent dimethyl sulfoxide influences protein-ligand binding kinetics via solvent viscosity effects: Revealing the success rate of complex formation following diffusive protein-ligand encounter. Biochemistry 2023, 62, 44–52. [Google Scholar] [CrossRef] [PubMed]
- Novales, N.A.; Schwans, J.P. Comparing the effects of organic cosolvents on acetylcholinesterase and butyrylcholinesterase activity. Anal. Biochem. 2022, 654, 114796. [Google Scholar] [CrossRef] [PubMed]
- Stenstrom, O.; Diehl, C.; Modig, K.; Nilsson, U.J.; Akke, M. Mapping the energy landscape of protein-ligand binding via linear free energy relationships determined by protein NMR relaxation dispersion. RSC Chem. Biol. 2021, 2, 259–265. [Google Scholar] [CrossRef] [PubMed]
- Shakeel, F.; Haq, N.; Salem-Bekhit, M.M.; Raish, M. Solubility and dissolution thermodynamics of sinapic acid in (DMSO + water) binary solvent mixtures at different temperatures. J. Mol. Liq. 2017, 225, 833–839. [Google Scholar] [CrossRef]
- Shakeel, F.; Alshehri, S.; Imran, M.; Haq, N.; Alanazi, A.; Anwer, M.K. Experimental and computational approaches for solubility measurement of pyridazinone derivative in binary (DMSO + water) systems. Molecules 2019, 25, 171. [Google Scholar] [CrossRef] [PubMed]
- Alshahrani, S.M.; Shakeel, F. Solubility data and computational modeling of baricitinib in various (DMSO + water) mixtures. Molecules 2020, 26, 2124. [Google Scholar] [CrossRef] [PubMed]
- Tinjaca, D.A.; Martinez, F.; Almanza, O.A.; Pena, M.A.; Jouyban, A.; Acree, W.E., Jr. Increasing the equilibrium solubility of meloxicam in aqueous media by using dimethyl sulfoxide as a cosolvent: Correlation, dissolution thermodynamics and preferential solvation. Liquids 2022, 2, 161–182. [Google Scholar] [CrossRef]
- Yao, X.; Wang, Z.; Geng, Y.; Zhao, H.; Rahimpour, E.; Acree, W.E., Jr.; Jouyban, A. Hirshfeld surface and electrostatic potential surface analysis of clozapine and its solubility and molecular interactions in aqueous blends. J. Mol. Liq. 2022, 360, 119328. [Google Scholar] [CrossRef]
- Shakeel, F.; Haq, N.; Alshehri, S.; Alenazi, M.; Alwhaibi, A.; Alsarra, I.A. Solubility and thermodynamic analysis of isotretinoin in different (DMSO + water) mixtures. Molecules 2023, 28, 7110. [Google Scholar] [CrossRef]
- Zhu, Q.N.; Wang, Q.; Hu, Y.B.; Abliz, X. Practical determination of the solubility parameters of 1-alkyl-3-methylimidazolium bromide ([CnC1im]Br, n = 5, 6, 7, 8) ionic liquids by inverse gas chromatography and the Hansen solubility parameter. Molecules 2019, 24, 1346. [Google Scholar] [CrossRef]
- Vay, K.; Scheler, S.; Frieß, W. Application of Hansen solubility parameters for understanding and prediction of drug distribution in microspheres. Int. J. Pharm. 2011, 416, 202–209. [Google Scholar] [CrossRef]
- Zhang, Y.; Shi, X.; Yu, Y.; Zhao, S.; Song, H.; Chen, A.; Shang, Z. Preparation and characterization of vanillin cross-linked chitosan microspheres of pterostilbene. Int. J. Polym. Anal. Charact. 2014, 19, 83–93. [Google Scholar] [CrossRef]
- Mohammadian, E.; Rahimpour, E.; Martinez, F.; Jouyban, A. Budesonide solubility in polyethylene glycol 400 + water at different temperatures: Experimental measurement and mathematical modelling. J. Mol. Liq. 2019, 274, 418–425. [Google Scholar] [CrossRef]
- Apelblat, A.; Manzurola, E. Solubilities of o-acetylsalicylic, 4-aminosalicylic, 3,5-dinitrosalicylic and p-toluic acid and magnesium-DL-aspartate in water from T = (278–348) K. J. Chem. Thermodyn. 1999, 31, 85–91. [Google Scholar] [CrossRef]
- Manzurola, E.; Apelblat, A. Solubilities of L-glutamic acid, 3-nitrobenzoic acid, acetylsalicylic, p-toluic acid, calcium-L-lactate, calcium gluconate, magnesium-DL-aspartate, and magnesium-L-lactate in water. J. Chem. Thermodyn. 2002, 34, 1127–1136. [Google Scholar] [CrossRef]
- Ksiazczak, A.; Moorthi, K.; Nagata, I. Solid-solid transition and solubility of even n-alkanes. Fluid Phase Equilib. 1994, 95, 15–29. [Google Scholar] [CrossRef]
- Tong, Y.; Wang, Z.; Yang, E.; Pan, B.; Jiang, J.; Dang, P.; Wei, H. Determination and correlation of solubility and solution thermodynamics of ethenzamide in different pure solvents. Fluid Phase Equilib. 2016, 427, 549–556. [Google Scholar] [CrossRef]
- Shakeel, F.; Alshehri, S. Solubilization, Hansen solubility parameters, solution thermodynamics and solvation behavior of flufenamic acid in (Carbitol + water) mixtures. Processes 2020, 8, 1204. [Google Scholar] [CrossRef]
- Yalkowsky, S.H.; Roseman, T.J. Solubilization of Drugs by Cosolvents. In Techniques of Solubilization of Drugs; Yalkowsky, S.H., Ed.; Marcel Dekker Inc.: New York, NY, USA, 1981; pp. 91–134. [Google Scholar]
- Jouyban, A.; Acree, W.E., Jr. Mathematical derivation of the Jouyban-Acree model to represent solute solubility data in mixed solvents at various temperatures. J. Mol. Liq. 2018, 256, 541–547. [Google Scholar] [CrossRef]
- Higuchi, T.; Connors, K.A. Phase-solubility techniques. Adv. Anal. Chem. Instr. 1965, 4, 117–122. [Google Scholar]
- Wan, Y.; He, H.; Huang, Z.; Zhang, P.; Sha, J.; Li, T.; Ren, B. Solubility, thermodynamic modeling and Hansen solubility parameter of 5-norbornene-2,3-dicarboximide in three binary solvents (methanol, ethanol, ethyl acetate + DMF) from 278.15 K to 323.15 K. J. Mol. Liq. 2020, 300, 112097. [Google Scholar] [CrossRef]
- Ruidiaz, M.A.; Delgado, D.R.; Martínez, F.; Marcus, Y. Solubility and preferential solvation of indomethacin in 1,4-dioxane + water solvent mixtures. Fluid Phase Equilib. 2010, 299, 259–265. [Google Scholar] [CrossRef]
- Hildebrand, J.H.; Prausnitz, J.M.; Scott, R.L. Regular and Related Solutions; Van Nostrand Reinhold: New York, NY, USA, 1970. [Google Scholar]
- Manrique, Y.J.; Pacheco, D.P.; Martínez, F. Thermodynamics of mixing and solvation of ibuprofen and naproxen in propylene glycol + water cosolvent mixtures. J. Sol. Chem. 2008, 37, 165–181. [Google Scholar] [CrossRef]
- Shakeel, F.; Bhat, M.A.; Haq, N.; Fathi-Azarbayjani, A.; Jouyban, A. Solubility and thermodynamic parameters of a novel anti-cancer drug (DHP-5) in polyethylene glycol 400 + water mixtures. J. Mol. Liq. 2017, 229, 241–245. [Google Scholar] [CrossRef]
- Cong, Y.; Du, C.; Xing, K.; Bian, Y.; Li, X.; Wang, M. Research on dissolution of actarit in aqueous mixtures: Solubilitydetermination and correlation, preferential solvation, solvent effectand thermodynamics. J. Mol. Liq. 2022, 355, 119141. [Google Scholar] [CrossRef]
- Tinjaca, D.A.; Martinez, F.; Almanza, O.A.; Jouyban, A.; Acree, W.E., Jr. Solubility, correlation, dissolution thermodynamics and preferential solvation of meloxicam in aqueous mixtures of 2-propanol. Pharm. Sci. 2022, 28, 130–144. [Google Scholar] [CrossRef]
- Krug, R.R.; Hunter, W.G.; Grieger, R.S. Enthalpy-entropy compensation. 2. Separation of the chemical from the statistic effect. J. Phys. Chem. 1976, 80, 2341–2351. [Google Scholar] [CrossRef]
- Holguín, A.R.; Rodríguez, G.A.; Cristancho, D.M.; Delgado, D.R.; Martínez, F. Solution thermodynamics of indomethacin in propylene glycol + water mixtures. Fluid Phase Equilib. 2012, 314, 134–139. [Google Scholar] [CrossRef]
- Behboudi, E.; Soleymani, J.; Martinez, F.; Jouyban, A. Solubility of amlodipine besylate in binary mixtures of polyethylene glycol 400 + water at various temperatures: Measurement and modelling. J. Mol. Liq. 2022, 347, 118394. [Google Scholar] [CrossRef]
- Mohammadian, E.; Foroumadi, A.; Hasanvand, Z.; Rahimpour, E.; Zhao, H.; Jouyban, A. Simulation of mesalazine solubility in the binary solvents at various temperatures. J. Mol. Liq. 2022, 357, 119160. [Google Scholar] [CrossRef]
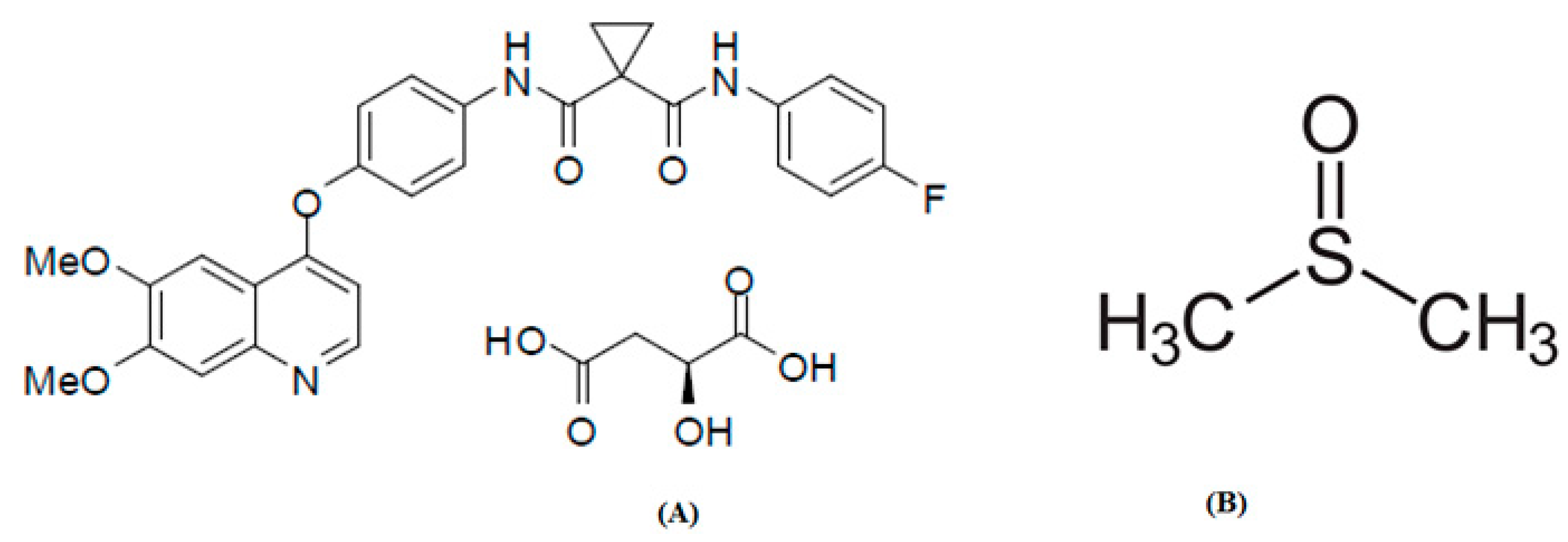
 indicates the stated mole fraction solubilities of CBZM in (A) neat H2O and (B) neat DMSO, and the symbol
indicates the stated mole fraction solubilities of CBZM in (A) neat H2O and (B) neat DMSO, and the symbol  indicates the reported solubilities of CBZM in (A) neat H2O and (B) neat DMSO retrieved from Ref. [20].
indicates the reported solubilities of CBZM in (A) neat H2O and (B) neat DMSO retrieved from Ref. [20].
 indicates the stated mole fraction solubilities of CBZM in (A) neat H2O and (B) neat DMSO, and the symbol
indicates the stated mole fraction solubilities of CBZM in (A) neat H2O and (B) neat DMSO, and the symbol  indicates the reported solubilities of CBZM in (A) neat H2O and (B) neat DMSO retrieved from Ref. [20].
indicates the reported solubilities of CBZM in (A) neat H2O and (B) neat DMSO retrieved from Ref. [20].
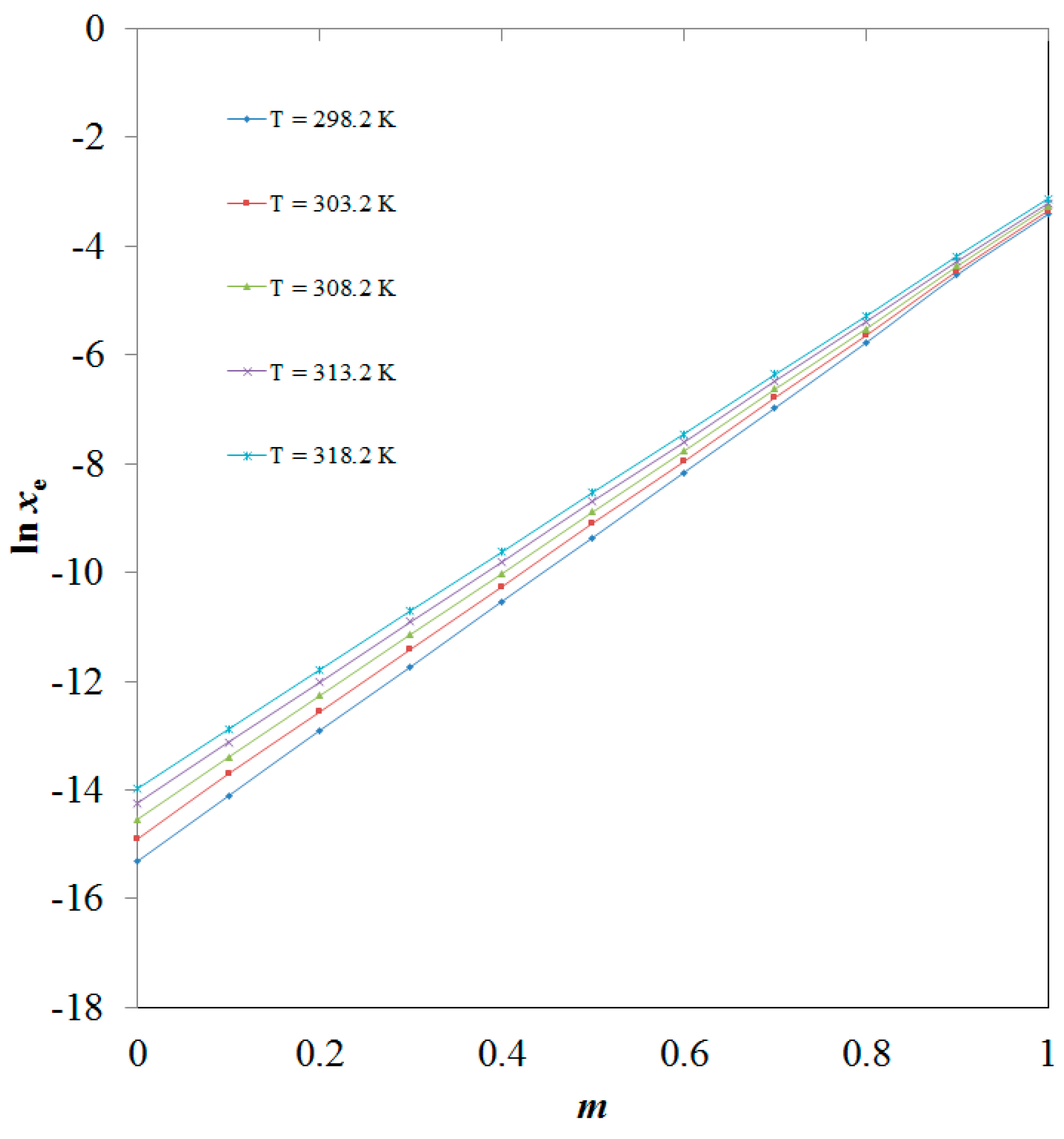


| ma | xeb | ||||
|---|---|---|---|---|---|
| T = 298.2 K | T = 303.2 K | T = 308.2 K | T = 313.2 K | T = 318.2 K | |
| 0.0 | 2.24 × 10−7 | 3.37 × 10−7 | 4.85 × 10−7 | 6.55 × 10−7 | 8.53 × 10−7 |
| 0.1 | 7.46 × 10−7 | 1.12 × 10−6 | 1.52 × 10−6 | 2.01 × 10−6 | 2.55 × 10−6 |
| 0.2 | 2.48 × 10−6 | 3.48 × 10−6 | 4.69 × 10−6 | 6.00 × 10−6 | 7.57 × 10−6 |
| 0.3 | 8.00 × 10−6 | 1.11 × 10−5 | 1.46 × 10−5 | 1.83 × 10−5 | 2.25 × 10−5 |
| 0.4 | 2.66 × 10−5 | 3.48 × 10−5 | 4.42 × 10−5 | 5.49 × 10−5 | 6.59 × 10−5 |
| 0.5 | 8.61 × 10−5 | 1.12 × 10−4 | 1.38 × 10−4 | 1.69 × 10−4 | 1.98 × 10−4 |
| 0.6 | 2.48 × 10−4 | 3.47 × 10−4 | 4.21 × 10−4 | 4.95 × 10−4 | 5.79 × 10−4 |
| 0.7 | 9.33 × 10−4 | 1.12 × 10−3 | 1.31 × 10−3 | 1.53 × 10−3 | 1.72 × 10−3 |
| 0.8 | 3.09 × 10−3 | 3.53 × 10−3 | 3.99 × 10−3 | 4.55 × 10−3 | 5.04 × 10−3 |
| 0.9 | 1.07 × 10−2 | 1.15 × 10−2 | 1.26 × 10−2 | 1.38 × 10−2 | 1.52 × 10−2 |
| 1.0 | 3.29 × 10−2 | 3.50 × 10−2 | 3.78 × 10−2 | 4.02 × 10−2 | 4.38 × 10−2 |
| xidl | 1.50 × 10−3 | 1.91 × 10−3 | 2.44 × 10−3 | 3.10 × 10−3 | 3.92 × 10−3 |
| m | γi | ||||
|---|---|---|---|---|---|
| T = 298.2 K | T = 303.2 K | T = 308.2 K | T = 313.2 K | T = 318.2 K | |
| 0.0 | 6703 | 5689 | 5044 | 4739 | 4597 |
| 0.1 | 2011 | 1712 | 1608 | 1542 | 1538 |
| 0.2 | 605.5 | 550.8 | 521.3 | 517.5 | 518.0 |
| 0.3 | 187.7 | 173.5 | 167.9 | 169.7 | 174.4 |
| 0.4 | 56.40 | 55.14 | 55.29 | 56.55 | 59.49 |
| 0.5 | 17.42 | 17.07 | 17.69 | 18.40 | 19.80 |
| 0.6 | 5.281 | 5.524 | 5.800 | 6.266 | 6.776 |
| 0.7 | 1.609 | 1.699 | 1.853 | 2.023 | 2.267 |
| 0.8 | 0.4851 | 0.5430 | 0.6120 | 0.6808 | 0.7771 |
| 0.9 | 0.1392 | 0.1658 | 0.1932 | 0.2245 | 0.2572 |
| 1.0 | 0.0455 | 0.0547 | 0.0646 | 0.0771 | 0.0894 |
| m | a | b | R2 | Overall RMSD (%) |
|---|---|---|---|---|
| 0.0 | 6.0050 | –6345.1 | 0.9951 | |
| 0.1 | 5.3286 | –5781.7 | 0.9928 | |
| 0.2 | 4.8131 | –5275.2 | 0.9961 | |
| 0.3 | 4.6768 | –4884.5 | 0.9946 | |
| 0.4 | 3.9428 | –4311.2 | 0.9972 | |
| 0.5 | 3.8641 | –3935.2 | 0.9949 | 1.94 |
| 0.6 | 3.1637 | –3375.2 | 0.9984 | |
| 0.7 | 2.8568 | –2927.5 | 0.9961 | |
| 0.8 | 2.0735 | –2340.7 | 0.9991 | |
| 0.9 | 0.99780 | –1651.9 | 0.9946 | |
| 1.0 | 1.0960 | –1346.7 | 0.9952 |
| m | A | B | C | R2 | Overall RMSD (%) |
|---|---|---|---|---|---|
| 0.0 | 1171.2 | –59,844 | –173.03 | 0.9996 | |
| 0.1 | 1292.7 | –64,886 | –191.17 | 0.9995 | |
| 0.2 | 854.93 | –44,308 | –126.23 | 0.9995 | |
| 0.3 | 945.45 | –48,077 | –139.70 | 0.9995 | |
| 0.4 | 617.15 | –32,468 | –91.057 | 0.9999 | |
| 0.5 | 758.16 | –38,566 | –112.00 | 0.9998 | 1.11 |
| 0.6 | 337.58 | –18,734 | –49.657 | 0.9996 | |
| 0.7 | 497.02 | –25,617 | –73.381 | 0.9999 | |
| 0.8 | 132.99 | –8356.9 | –19.439 | 0.9995 | |
| 0.9 | –314.18 | 12,808 | 46,808 | 0.9998 | |
| 1.0 | –217.67 | 8689.8 | 32.489 | 0.9989 |
| m | λ | h | R2 | Overall RMSD (%) |
|---|---|---|---|---|
| 0.0 | 6.7074 | 946.01 | 0.9951 | |
| 0.1 | 6.1660 | 937.72 | 0.9928 | |
| 0.2 | 5.8575 | 900.57 | 0.9961 | |
| 0.3 | 4.8790 | 1001.1 | 0.9945 | |
| 0.4 | 4.3741 | 985.64 | 0.9972 | |
| 0.5 | 3.6405 | 1080.9 | 0.9949 | 4.26 |
| 0.6 | 3.1306 | 1078.1 | 0.9984 | |
| 0.7 | 2.4700 | 1185.2 | 0.9961 | |
| 0.8 | 1.9852 | 1179.0 | 0.9991 | |
| 0.9 | 1.5723 | 1050.6 | 0.9946 | |
| 1.0 | 0.81440 | 1653.4 | 0.9952 |
| m | Log xYal | Overall RMSD (%) | ||||
|---|---|---|---|---|---|---|
| T = 298.2 K | T = 303.2 K | T = 308.2 K | T = 313.2 K | T = 318.2 K | ||
| 0.1 | −6.13 | −5.97 | −5.82 | −5.70 | −5.59 | |
| 0.2 | −5.61 | −5.46 | −5.33 | −5.22 | −5.12 | |
| 0.3 | −5.09 | −4.96 | −4.84 | −4.74 | −4.65 | |
| 0.4 | −4.58 | −4.46 | −4.35 | −4.26 | −4.18 | |
| 0.5 | −4.06 | −3.96 | −3.86 | −3.78 | −3.71 | 2.98 |
| 0.6 | −3.54 | −3.46 | −3.37 | −3.30 | −3.24 | |
| 0.7 | −3.03 | −2.96 | −2.89 | −2.82 | −2.77 | |
| 0.8 | −2.51 | −2.45 | −2.40 | −2.34 | −2.30 | |
| 0.9 | −1.99 | −1.95 | −1.91 | −1.86 | −1.82 | |
| System | Jouyban–Acree | Jouyban–Acree-Van’t Hoff |
|---|---|---|
| A1 1.0960 B1 –1346.7 A2 6.0050 B2 –6345.1 Ji 38,476 1.19 | ||
| {DMSO + H2O} | Ji 40,476 | |
RMSD (%) | 1.12 |
| m | ΔsolH0/kJ mol−1 | ΔsolG0/kJ mol−1 | ΔsolS0/J mol−1 K−1 | R2 |
|---|---|---|---|---|
| 0.0 | 52.71 | 37.36 | 49.82 | 0.9952 |
| 0.1 | 48.03 | 34.41 | 44.21 | 0.9929 |
| 0.2 | 43.82 | 31.52 | 39.92 | 0.9961 |
| 0.3 | 40.57 | 28.62 | 38.80 | 0.9946 |
| 0.4 | 35.81 | 25.74 | 32.71 | 0.9973 |
| 0.5 | 32.78 | 22.81 | 32.35 | 0.9946 |
| 0.6 | 28.02 | 19.95 | 26.18 | 0.9985 |
| 0.7 | 24.18 | 17.03 | 23.21 | 0.9963 |
| 0.8 | 19.48 | 14.15 | 17.30 | 0.9991 |
| 0.9 | 31.98 | 11.19 | 9.05 | 0.9957 |
| 1.0 | 11.43 | 8.38 | 9.91 | 0.9973 |
| Material | Molecular Formula | Molar Mass (g mol−1) | CAS RN | Purification Method | Mass Fraction Purity | Analysis Method | Source |
|---|---|---|---|---|---|---|---|
| CBZM | C32H30FN3O10 | 635.60 | 4759-48-2 | None | >0.99 | HPLC | Beijing Mesochem |
| DMSO | C2H6OS | 78.13 | 67-68-5 | None | >0.99 | GC | E-Merck |
| Water | H2O | 18.07 | 7732-18-5 | None | - | - | Milli-Q |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shakeel, F.; Haq, N.; Alshehri, S.; Alsarra, I.A. Solubility and Thermodynamics Data of Cabozantinib Malate in Various Aqueous Solutions of Dimethyl Sulfoxide at Different Temperatures. Molecules 2023, 28, 7805. https://doi.org/10.3390/molecules28237805
Shakeel F, Haq N, Alshehri S, Alsarra IA. Solubility and Thermodynamics Data of Cabozantinib Malate in Various Aqueous Solutions of Dimethyl Sulfoxide at Different Temperatures. Molecules. 2023; 28(23):7805. https://doi.org/10.3390/molecules28237805
Chicago/Turabian StyleShakeel, Faiyaz, Nazrul Haq, Sultan Alshehri, and Ibrahim A. Alsarra. 2023. "Solubility and Thermodynamics Data of Cabozantinib Malate in Various Aqueous Solutions of Dimethyl Sulfoxide at Different Temperatures" Molecules 28, no. 23: 7805. https://doi.org/10.3390/molecules28237805
APA StyleShakeel, F., Haq, N., Alshehri, S., & Alsarra, I. A. (2023). Solubility and Thermodynamics Data of Cabozantinib Malate in Various Aqueous Solutions of Dimethyl Sulfoxide at Different Temperatures. Molecules, 28(23), 7805. https://doi.org/10.3390/molecules28237805









