Mechanical and Electronic Properties of Al(111)/6H-SiC Interfaces: A DFT Study
Abstract
1. Introduction
2. Results and Discussion
2.1. Simulation Models and the Effects of Characteristic Defects
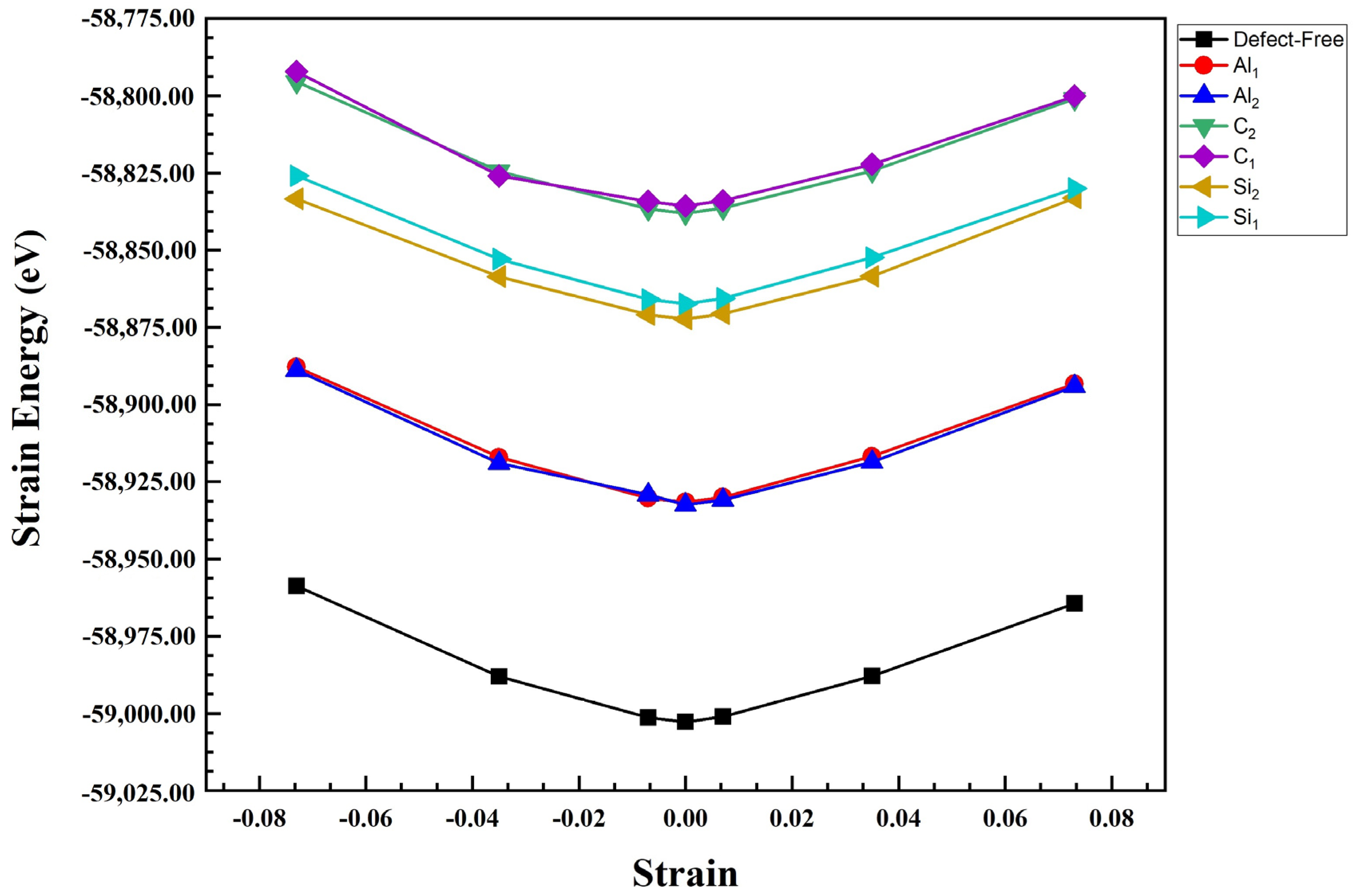
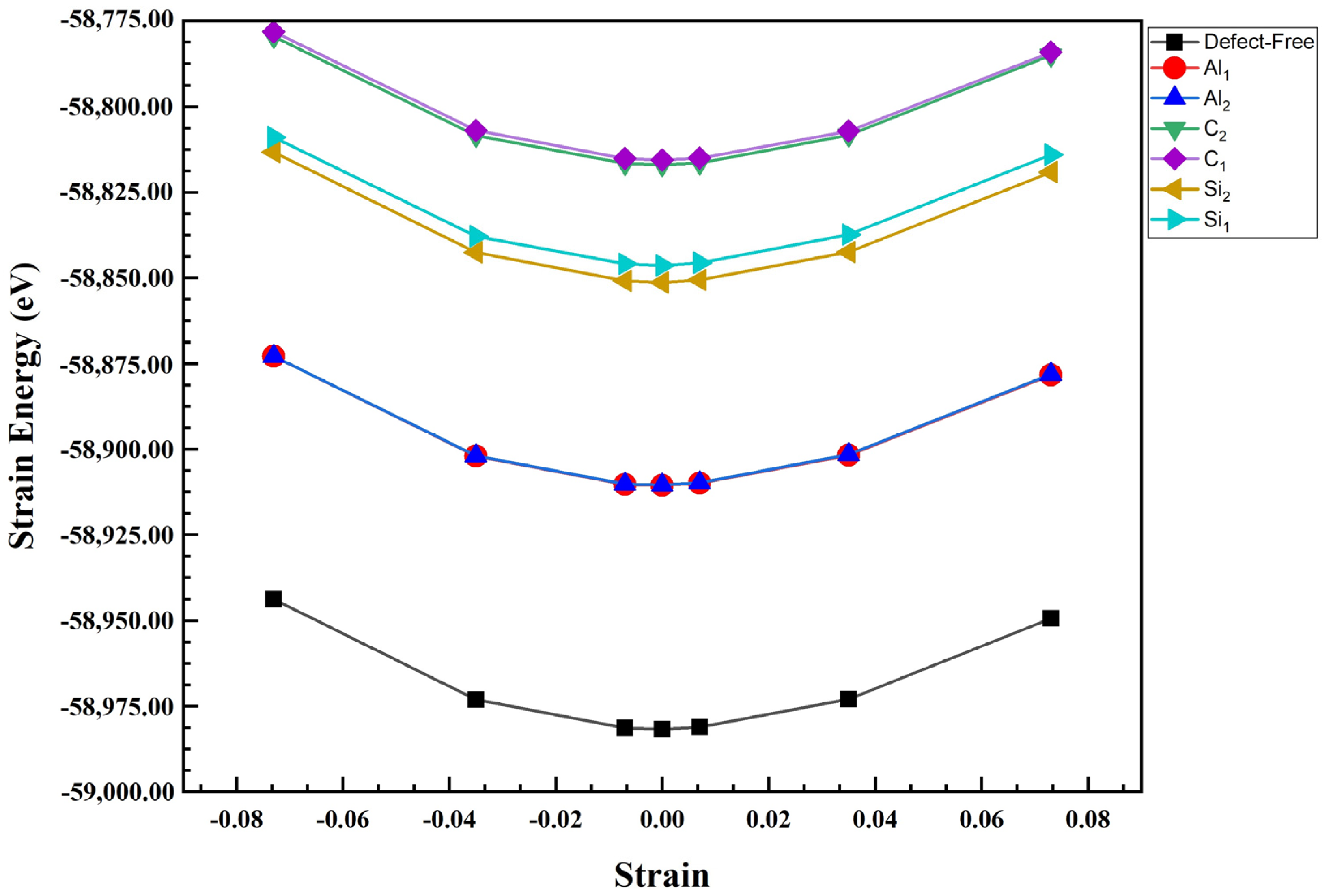
2.2. Formation Energy and Work of Adhesion Energy
2.3. Young’s Modulus
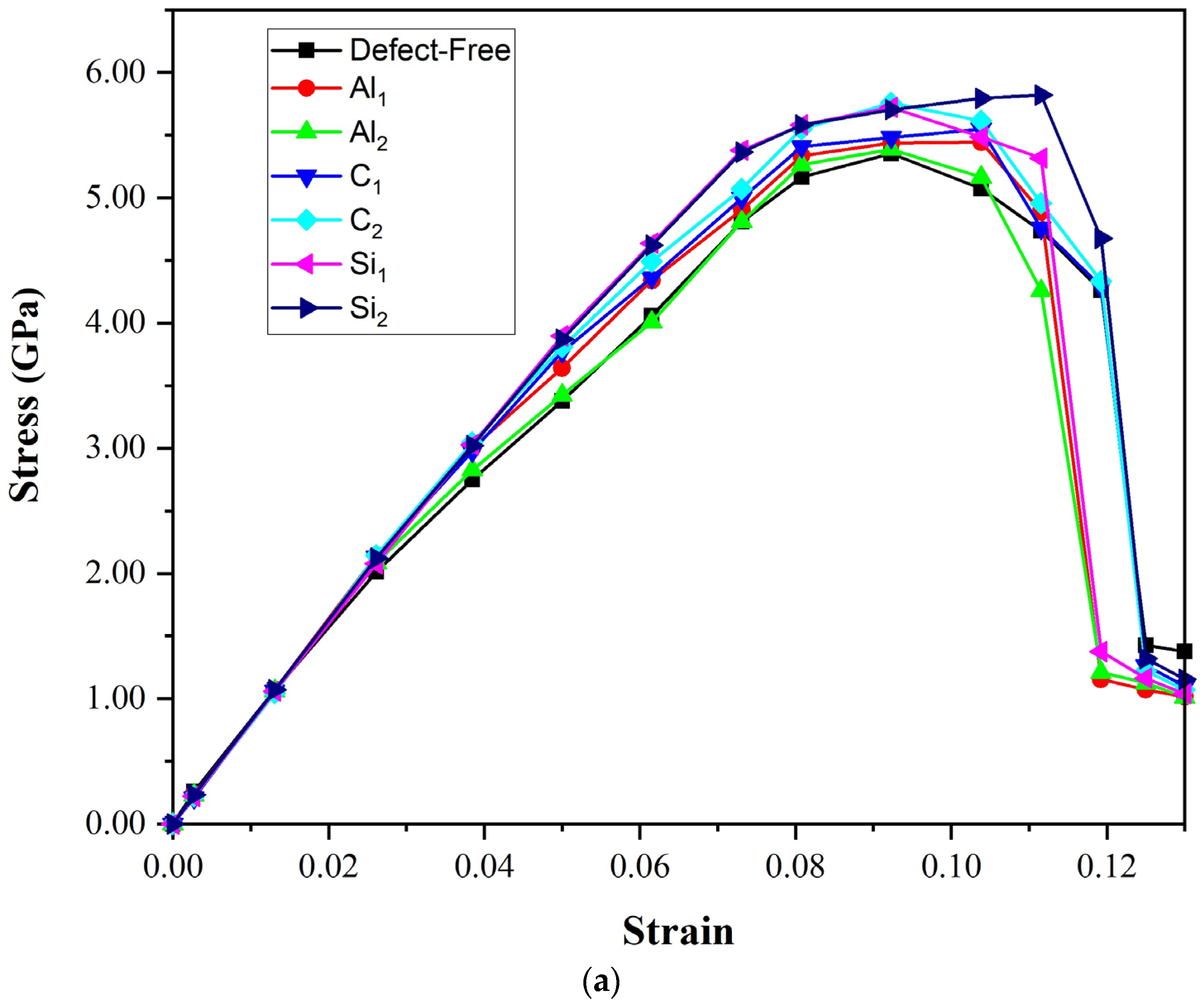
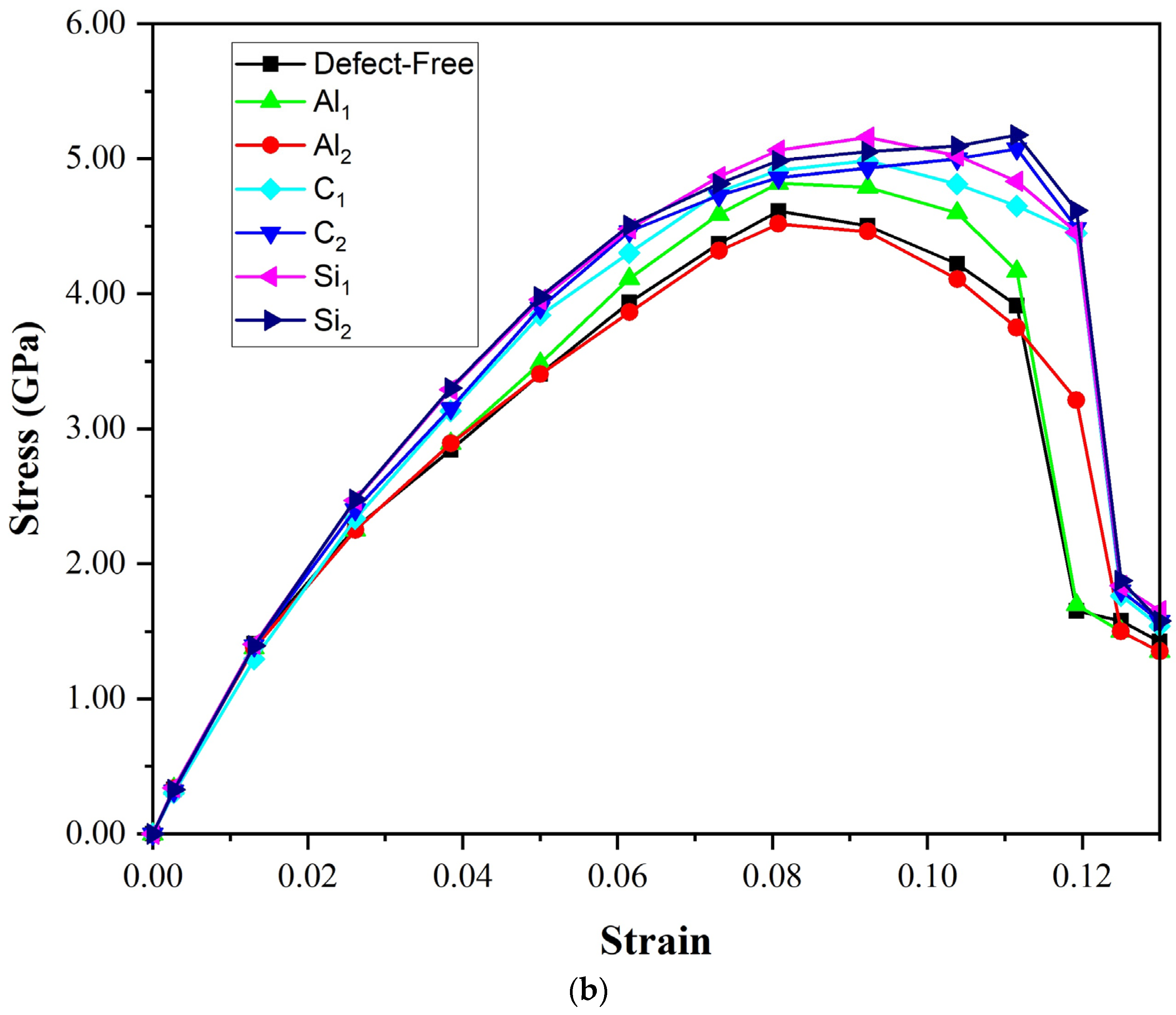
2.4. Investigation of Tensile Test Simulation Behavior
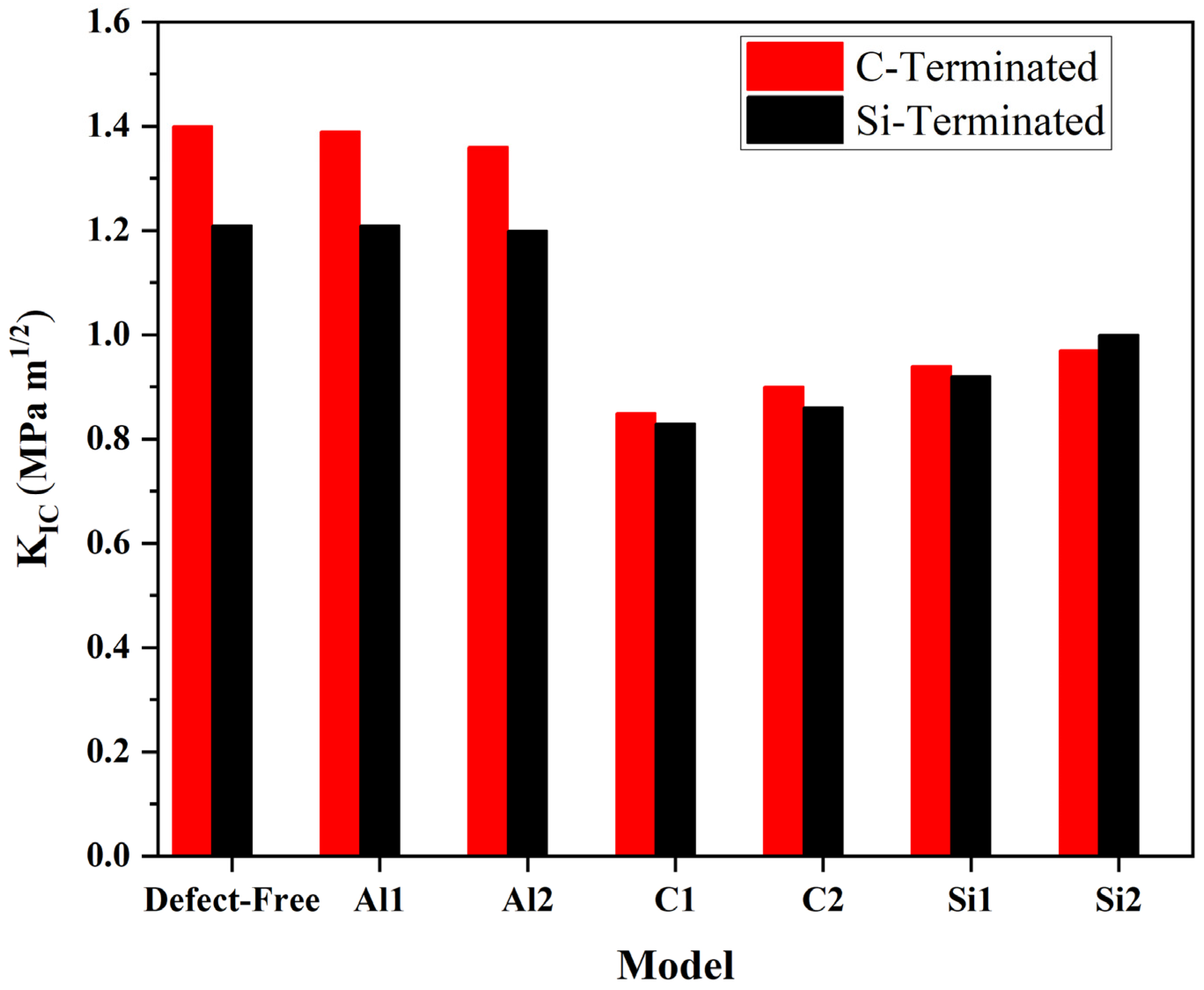
2.5. Surface Energy and Fracture Toughness
2.6. Density of States (DOS)
3. Methodology
4. Conclusions
- The vacancies significantly impact interface adhesion. Most vacancies weaken interfacial adhesion, though the weakening effects reduce with the depth of the vacancy from the interface to the inner.
- Although Al/SiC composites have lower adhesion energy due to defects at the interface, their tensile strength increases. It is because combining Al with SiC produces a much stronger compound than pure.
- The presence of Al vacancies at the interface may somewhat raise the material’s tensile properties by restoring Al atoms at the interface. According to the tensile strength results, the tensile properties of the configurations are better when point vacancies are placed on the SiC side than the point vacancies could be placed on the Al side.
- Vacancies on the SiC side significantly affect Young’s modulus, especially in the C1 model. Furthermore, Si-terminated configurations have a significantly lower Young’s modulus than C-terminated configurations. It is notable that Young’s modulus decreases significantly in the presence of carbon vacancies at the interface.
- C and Si vacancies affect fracture toughness more than Al vacancies in the interface. In the perfect model, the fracture toughness is 1.40 MPa m1/2 and 1.21 MPa m1/2, respectively, for the C-terminated and Si-terminated configurations. The lowest fracture toughness was found in the C1 model with C- and Si-terminated configurations (0.85 MPa m1/2 and 0.83 MPa m1/2, respectively). Likewise, the lowest Young’s modulus was observed in C1 for C- and Si-terminated configurations (145.1 GPa and 138.5 GPa, respectively).
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Sample Availability
References
- Senthil, S.; Raguraman, M.; Thamarai Manalan, D. Manufacturing processes & recent applications of aluminium metal matrix composite materials: A review. Mater. Today Proc. 2021, 45, 5934–5938. [Google Scholar] [CrossRef]
- Chintada, S.; Dora, S.P.; Kare, D. Mechanical Behavior and Metallographic Characterization of Microwave Sintered Al/SiC Composite Materials—An Experimental Approach. Silicon 2022, 14, 7341–7352. [Google Scholar] [CrossRef]
- Mayuet Ares, P.F.; Rodríguez-Parada, L.; Gómez-Parra, Á.; Batista Ponce, M. Characterization and Defect Analysis of Machined Regions in Al-SiC Metal Matrix Composites Using an Abrasive Water Jet Machining Process. Appl. Sci. 2020, 10, 1512. [Google Scholar] [CrossRef]
- Brandes, E.A.; Brook, G.B. Simithells Light Metals Handbook, 7th ed.; Elsevier & Butterwort-Heinemann: Oxford, UK, 1998. [Google Scholar]
- Somashekhar, S.; Siva Shanmugam, N.; Ramesh Bapu, B. Advances in Manufacturing Technology. Proceedings of ICAMT; Springer: Singapore, 2018. [Google Scholar] [CrossRef]
- Sharma, P.; Dwivedi, P.; Sharma, R.; Dabra, V.; Sharma, N. Microstructural and mechanical behavior of aluminium alloy reinforced with TiC. Mater. Today Proc 2020, 25, 934–937. [Google Scholar] [CrossRef]
- Sijo, M.T.; Jayadevan, K.R. Analysis of Stir Cast Aluminium Silicon Carbide Metal Matrix Composite: A Comprehensive Review. Proc. Technol. 2016, 24, 379–385. [Google Scholar] [CrossRef]
- Faisal, N.; Kumar, K. Mechanical and tribological behaviour of nano scaled silicon carbide reinforced aluminium composites. J. Exp. Nanosci. 2018, 13, S1–S13. [Google Scholar] [CrossRef]
- Sadhu, K.K.; Mandal, N.; Sahoo, R.R. SiC/graphene reinforced aluminum metal matrix composites prepared by powder metallurgy: A review. J. Manuf. Process. 2023, 91, 10–43. [Google Scholar] [CrossRef]
- Miracle, D.B. Metal matrix composites—From science to technological significance. Compos. Sci. Technol. 2005, 65, 2526–2540. [Google Scholar] [CrossRef]
- Sulaiman, S.; Marjom, Z.; Ismail, M.I.S.; Ariffin, M.K.A.; Ashrafi, N. Effect of Modifier on Mechanical Properties of Aluminium Silicon Carbide (Al-SiC) Composites. Procedia Eng. 2017, 184, 773–777. [Google Scholar] [CrossRef]
- Singh, Y.T.; Patra, P.K.; Hieu, N.N.; Rai, D.P. Study of electronic and mechanical properties of single walled Carbon nanotube (SWCNT) via substitutional Boron doping in zigzag and armchair pattern. Surf. Interfaces 2022, 29, 101815. [Google Scholar] [CrossRef]
- Liu, B.; Yang, J. Mg on adhesion of Al(111)/3C-SiC(111) interfaces from first principles study. J. Alloys Compd. 2019, 791, 530–539. [Google Scholar] [CrossRef]
- Wu, Q.; Xie, J.; Wang, A.; Ma, D.; Wang, C. First-principles calculations on the structure of 6H-SiC/Al interface. Mater. Res. Express 2019, 6, 065015. [Google Scholar] [CrossRef]
- Tahani, M.; Postek, E.; Motevalizadeh, L.; Sadowski, T. Molecular Dynamics Study of Interdiffusion for Cubic and Hexagonal SiC/Al Interfaces. Crystals 2023, 13, 46. [Google Scholar] [CrossRef]
- Tahani, M.; Postek, E.; Sadowski, T. Effect of Vacancy Defect Content on the Interdiffusion of Cubic and Hexagonal SiC/Al Interfaces: A Molecular Dynamics Study. Molecules 2023, 28, 744. [Google Scholar] [CrossRef]
- Goswami, R.; Pande, C.S.; Bernstein, N.; Johannes, M.D.; Baker, C.; Villalobos, G. A high degree of enhancement of strength of sputter deposited Al/Al2O3 multilayers upon post-annealing. Acta Mater. 2015, 95, 378–385. [Google Scholar] [CrossRef]
- Zhang, X.; Zhang, B.; Mu, Y.; Shao, S.; Wick, C.; Ramu Ramachandran, B.; Meng, W.J. Mechanical failure of metal/ceramic interfacial regions under shear loading. Acta Mater. 2017, 138, 224–236. [Google Scholar] [CrossRef]
- Passerone, A.; Luigia Muolo, L.; Novakovic, R.; Passerone, D. Liquid metal/ceramic interactions in the (Cu, Ag, Au)/ZrB2 systems. J. Eur. Ceram. Soc. 2007, 27, 3277–3285. [Google Scholar] [CrossRef]
- Qi, Y.; Hector, L.G. Hydrogen effect on adhesion and adhesive transfer at aluminum/diamond interfaces. Phys. Rev. B 2003, 68, 201403. [Google Scholar] [CrossRef]
- Qi, Y.; Hector, L.G. Adhesion and adhesive transfer at aluminum/diamond interfaces: A first-principles study. Phys. Rev. B 2004, 69, 235401. [Google Scholar] [CrossRef]
- Xu, X.-Y.; Wang, H.-Y.; Zha, M.; Wang, C.; Yang, Z.-Z.; Jiang, Q.-C. Effects of Ti, Si, Mg and Cu additions on interfacial properties and electronic structure of Al(111)/4H-SiC(0001) interface: A first-principles study. Appl. Surf. Sci. 2018, 437, 103–109. [Google Scholar] [CrossRef]
- Chen, D.; Ma, X.L.; Wang, Y.M. First-principles study of the interfacial structures of Au/MgO(001). Phys. Rev. B 2007, 75, 125409. [Google Scholar] [CrossRef]
- Lin, X.L.; Pan, F.C. The electronic structures and magnetism in Al doped 4H-SiC: The first-principles calculation. J. Supercond. Nov. Magn. 2014, 27, 1513–1516. [Google Scholar] [CrossRef]
- Matsunaka, D.; Shibutani, Y. Electronic states and adhesion properties at metal/MgO incoherent interfaces: First-principles calculations. Phys. Rev. B 2008, 77, 165435. [Google Scholar] [CrossRef]
- Matsunaka, D.; Shibutani, Y. Effects of oxygen vacancy on adhesion of incoherent metal/oxide interface by first-principles calculations. Surf. Sci. 2010, 604, 196–200. [Google Scholar] [CrossRef]
- Gdoutos, E.E. Fracture Mechanics. Solid Mech. Its Appl. 2005, 123. [Google Scholar] [CrossRef]
- Song, L.; Tian, X.; Jiang, H.; Yu, W.; Zaho, Z.; Zheng, H.; Qin, J.; Lin, X. Vacancies effect on the mechanical properties in B2 FeAl intermetallic by the first-principles study. Part A Mater. Sci. 2019, 21, 99. [Google Scholar] [CrossRef]
- Rao, K.; Jena, P. Molecular view of the interfacial adhesion in aluminum-silicon carbide metal-matrix composites. Appl. Phys. Lett. 1990, 57, 2285. [Google Scholar] [CrossRef]
- Janisch, R.; Ahmed, N.; Hartmaier, A. Ab initiotensile tests of Al bulk crystals and grain boundaries: The universality of mechanical behavior. Phys. Rev. B 2010, 81, 184108. [Google Scholar] [CrossRef]
- Lazar, P.; Podloucky, R.; Wolf, W. Correlating elasticity and cleavage. Appl. Phys. Lett. 2005, 87, 261910. [Google Scholar] [CrossRef]
- Xu, C.; Jiang, Y.; Yi, D.; Zhang, H.; Peng, S.; Liang, J. Interface-level thermodynamic stability diagram for in situ internal oxidation of Ag(SnO2)p composites. J. Mater. Sci. 2014, 50, 1646–1654. [Google Scholar] [CrossRef]
- Wu, Q.; Xie, J.; Wang, A.; Wang, C.; Mao, A. Effects of vacancies at Al(1 1 1)/6H-SiC(0001) interfaces on deformation behavior: A first-principle study. Comput. Mater. Sci. 2019, 158, 110–116. [Google Scholar] [CrossRef]
- Finnis, M.W. The theory of metal-ceramic interfaces. J. Phys. Condens. Matter. 1996, 8, 5811. [Google Scholar] [CrossRef]
- Ganji, M.D.; Sharifi, N.; Fereidoon, A.; Ghorbanzadeh Ahangari, M. Epoxy monomer adsorption on Group III (B, Al, Ga) nitride nanotubes: vdW-DF studies on mechanical and electronic properties. Superlattices Microstruct 2014, 67, 127–143. [Google Scholar] [CrossRef]
- Ghorbanzadeh Ahangari, M.; Ganji, M.D.; Montazar, F. Mechanical and electronic properties of carbon nanobuds: First-principles study. Solid State Commun. 2015, 203, 58–62. [Google Scholar] [CrossRef]
- Hamed Mashhadzadeh, A.; Ghorbanzadeh Ahangari, M.; Dadrasi, A.; Fathalian, M. Theoretical studies on the mechanical and electronic properties of 2D and 3D structures of Beryllium-Oxide graphene and graphene nanobud. Appl. Surf. Sci. 2019, 476, 36–48. [Google Scholar] [CrossRef]
- Chorfi, H.; Lobato, A.; Boudjada, F.; Salvadó, M. Computational Modeling of Tensile Stress Effects on the Structure and Stability of Prototypical Covalent and Layered Materials. Nanomaterials 2019, 9, 1483. [Google Scholar] [CrossRef]
- Wu, Q.; Xie, J.; Wang, C.; Li, L.; Wang, A.; Mao, A. First-principles study of the structure properties of Al(111)/6H-SiC(0001) interfaces. Surf. Sci. 2018, 670, 1–7. [Google Scholar] [CrossRef]
- Zhou, G.; Duan, W.; Gu, B. First-principles study on morphology and mechanical properties of single-walled carbon nanotube. Chem. Phys. Lett. 2001, 33, 344–349. [Google Scholar] [CrossRef]
- Wang, C.; Chen, W.; Jia, Y.; Xie, J. Calculating Study on Properties of Al(111)/6H-SiC(0001) Interfaces. Metals 2020, 10, 1197. [Google Scholar] [CrossRef]
- Pen, H.; Guo, J.; Cao, Z.; Wang, X.; Wang, Z. Finite element simulation of the micromachining of nanosized-silicon-carbide-particle reinforced composite materials based on the cohesive zone model. Nanotechnol. Precis. Eng. 2018, 1, 242–247. [Google Scholar] [CrossRef]
- Knuth, F.; Carbogno, C.; Atalla, V.; Blum, V.; Blum, V. All-electron formalism for total energy strain derivatives and stress tensor components for numeric atom-centered orbitals. Comput. Phys. Commun. 2015, 190, 33–50. [Google Scholar] [CrossRef]
- Fiorentini, V.; Methfessel, M. Extracting convergent surface energies from slab calculations. J. Phys. Condens. Matter. 1996, 8, 6525. [Google Scholar] [CrossRef]
- Sigumonrong, D.P.; Music, D.; Schneider, J.M. Efficient supercell design for surface and interface calculations of hexagonal phases: α-Al2O3 case study. Comput. Mater. Sci. 2011, 50, 1197–1201. [Google Scholar] [CrossRef]
- Liu, Z.; Wang, B.; Cazorla, C. Mechanical and electronic properties of CeO2 under uniaxial tensile loading: A DFT study. Materialia 2021, 15, 101050. [Google Scholar] [CrossRef]
- Chen, K.; Bielawski, M. Interfacial fracture toughness of transition metal nitrides. Surf. Coat. Technol. 2008, 203, 598–601. [Google Scholar] [CrossRef]
- Ding, Z.; Zhou, S.; Zhao, Y. Hardness and fracture toughness of brittle materials: A density functional theory study. Phys. Rev. B 2004, 70, 184117. [Google Scholar] [CrossRef]
- Chen, K.; Bielawski, M. Ab initio study on fracture toughness of Ti0.75X0.25C ceramic. J. Mater. Sci. 2007, 42, 9713–9716. [Google Scholar] [CrossRef]
- Bickelhaupt, F.M.; Baerends, E.J. Kohn-Sham DFT: Predicting and understanding chemistry. Rev. Comput. Chem. 2010, 15, 1–86. [Google Scholar] [CrossRef]
- Fonseca Guerra, C.; Snijders, J.G.; Velde, G.; Baerends, E.J. Towards an order-N DFT method. Theor. Chem. Acc. 1998, 99, 391–403. [Google Scholar] [CrossRef]
- Hohenberg, P.; Kohn, W. Inhomogeneous Electron Gas. Phys. Rev. 1964, 136, B864–B871. [Google Scholar] [CrossRef]
- Kohn, W. Nobel Lecture: Electronic structure of matter—Wave functions and density functionals. Rev. Mod. Phys. 1999, 71, 1253–1266. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Perdew, Burke, and Ernzerhof reply. Phys. Rev. Lett. 1998, 80, 891. [Google Scholar] [CrossRef]
- Burke, K.; Perdew, J.P.; Ernzerhof, M. Why semilocal functionals work: Accuracy of the on-top pair density and importance of system averaging. J. Chem. Phys. 1998, 109, 3760–3771. [Google Scholar] [CrossRef]
- Soler, J.M.; Artacho, E.; D.Gale, J.; García, A.; Junquera, J.; Ordejón, P.; Sánchez-Portal, D. The SIESTA method for ab initio order- N materials simulation. J. Phys. Condens. Matter. 2002, 14, 2745. [Google Scholar] [CrossRef]
- Monkhorst, H.J.; Pack, J.D. Special points for Brillouin-zone integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- Blöchl, E.; Jepsen, O.; Andersen, O.K. Improved tetrahedron method for brillouin-zone integrations. Phys. Rev. B Condens. Matter. 1994, 49, 16223. [Google Scholar] [CrossRef] [PubMed]
- Hamed Mashhadzadeha, A.; Fathalian, M.; Ghorbanzadeh Ahangaric, M.; Shahavi, M.H. DFT study of Ni, Cu, Cd, and Ag heavy metal atom adsorption onto the surface of the zinc-oxide nanotube and zinc-oxide graphene-like structure. Mater. Chem. Phys. 2018, 220, 366–373. [Google Scholar] [CrossRef]
- Ghorbanzadeh Ahangari, M.; Hamed Mashhadzadeh, A.; Fathalian, M.; Dadrasi, A.; Rostamiyan, Y.; Mallahi, A. Effect of various defects on mechanical and electronic properties of zinc-oxide graphene-like structure: A DFT study. Vacuum 2019, 165, 26–34. [Google Scholar] [CrossRef]

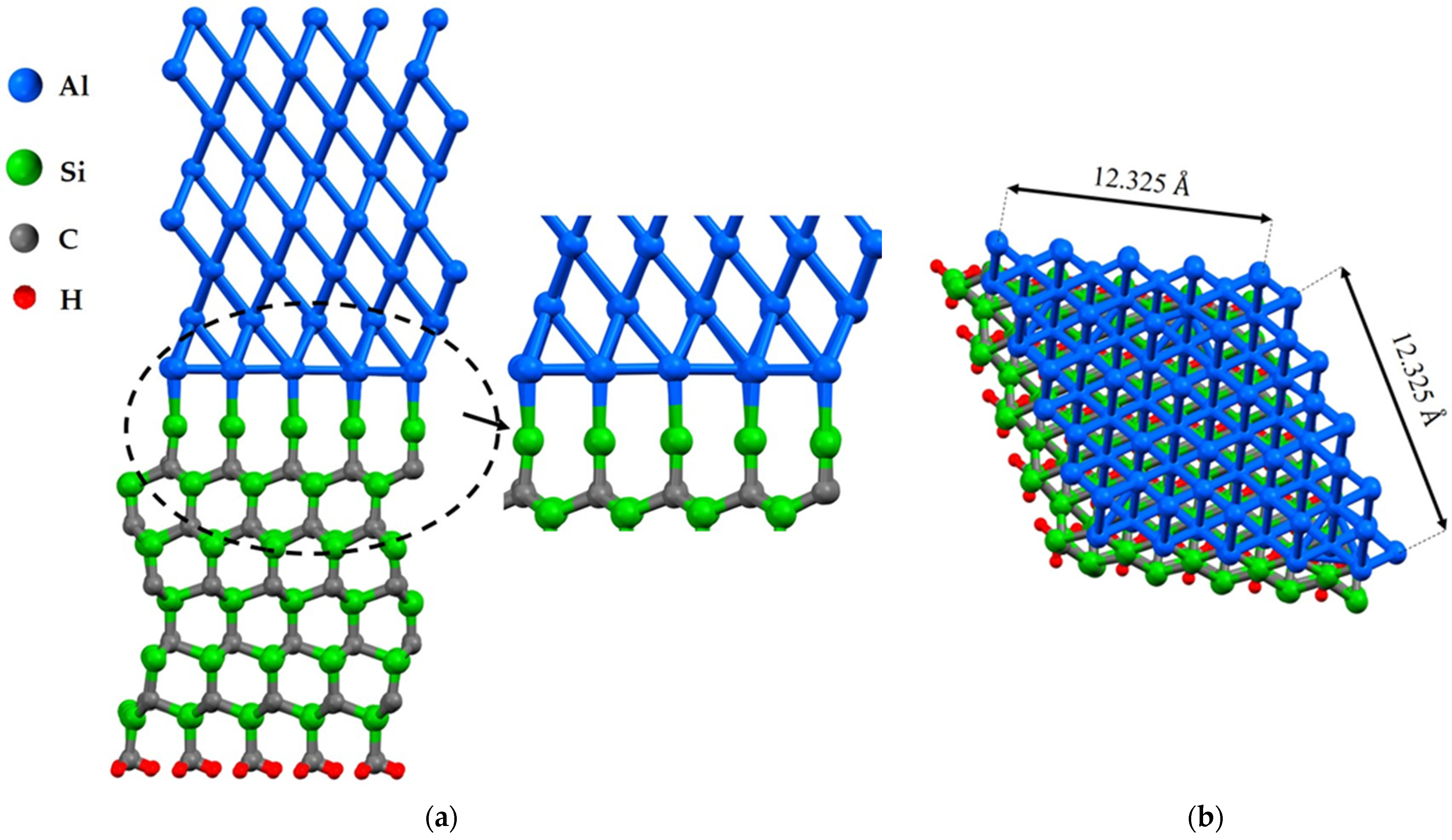
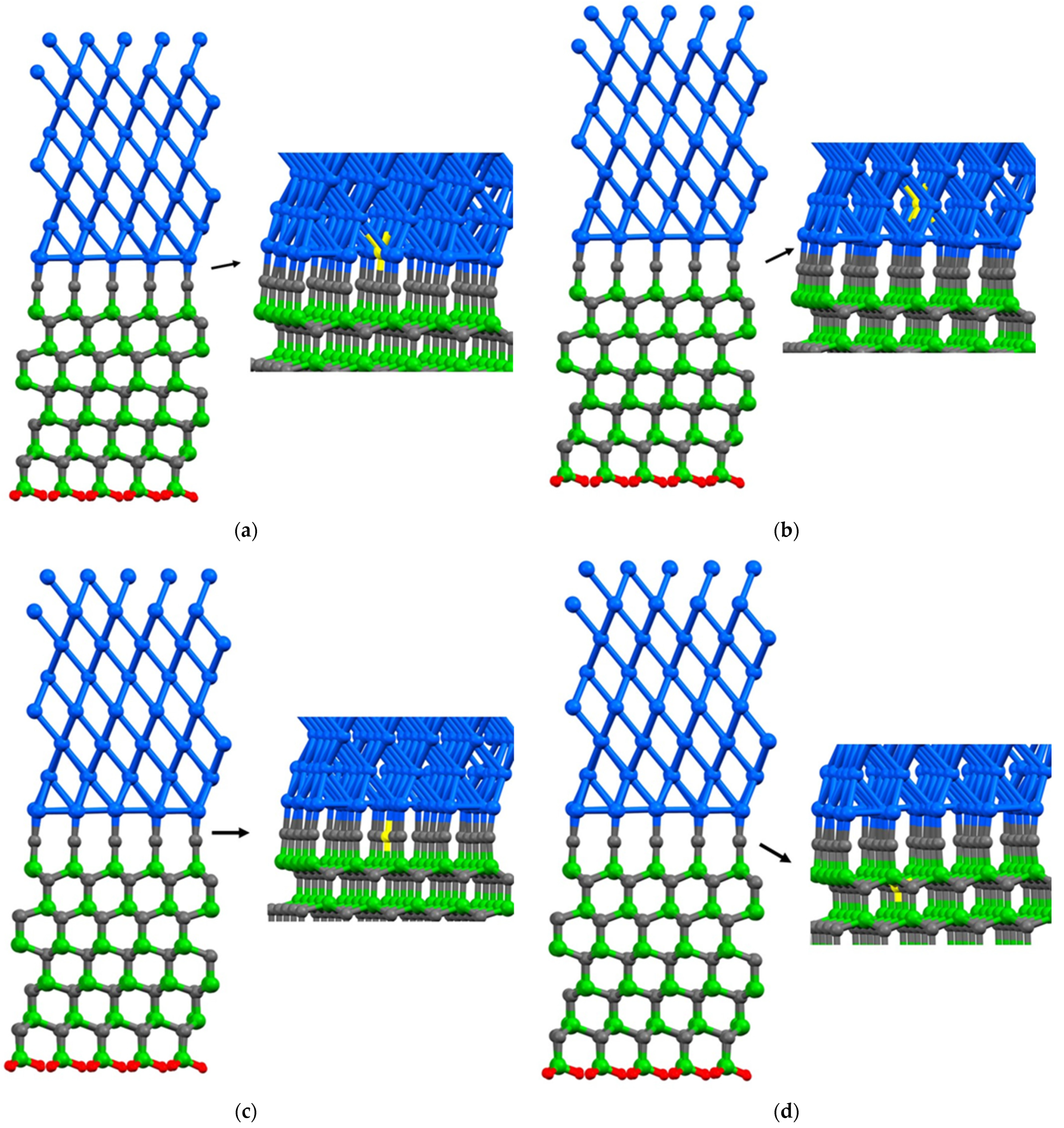
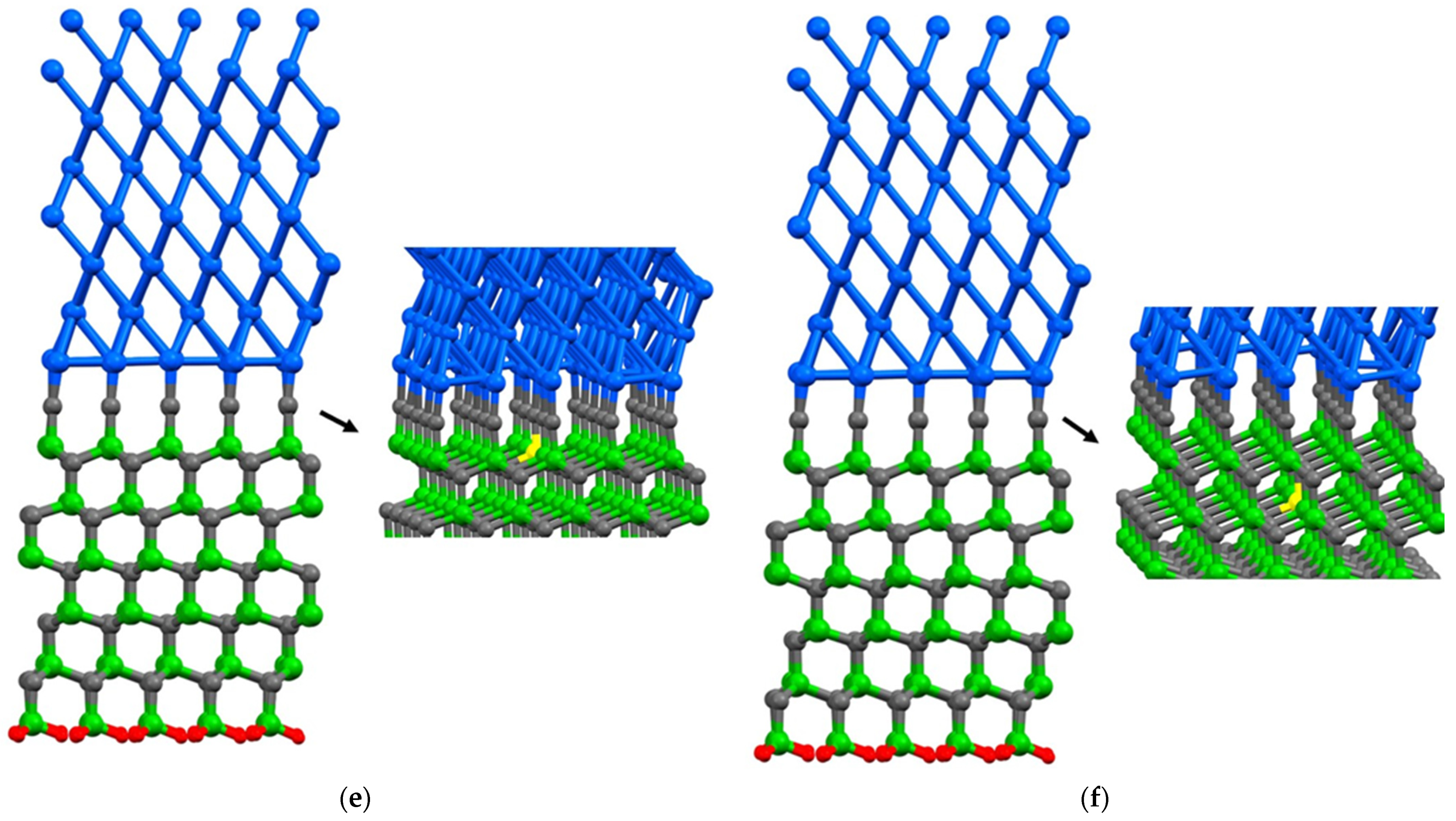
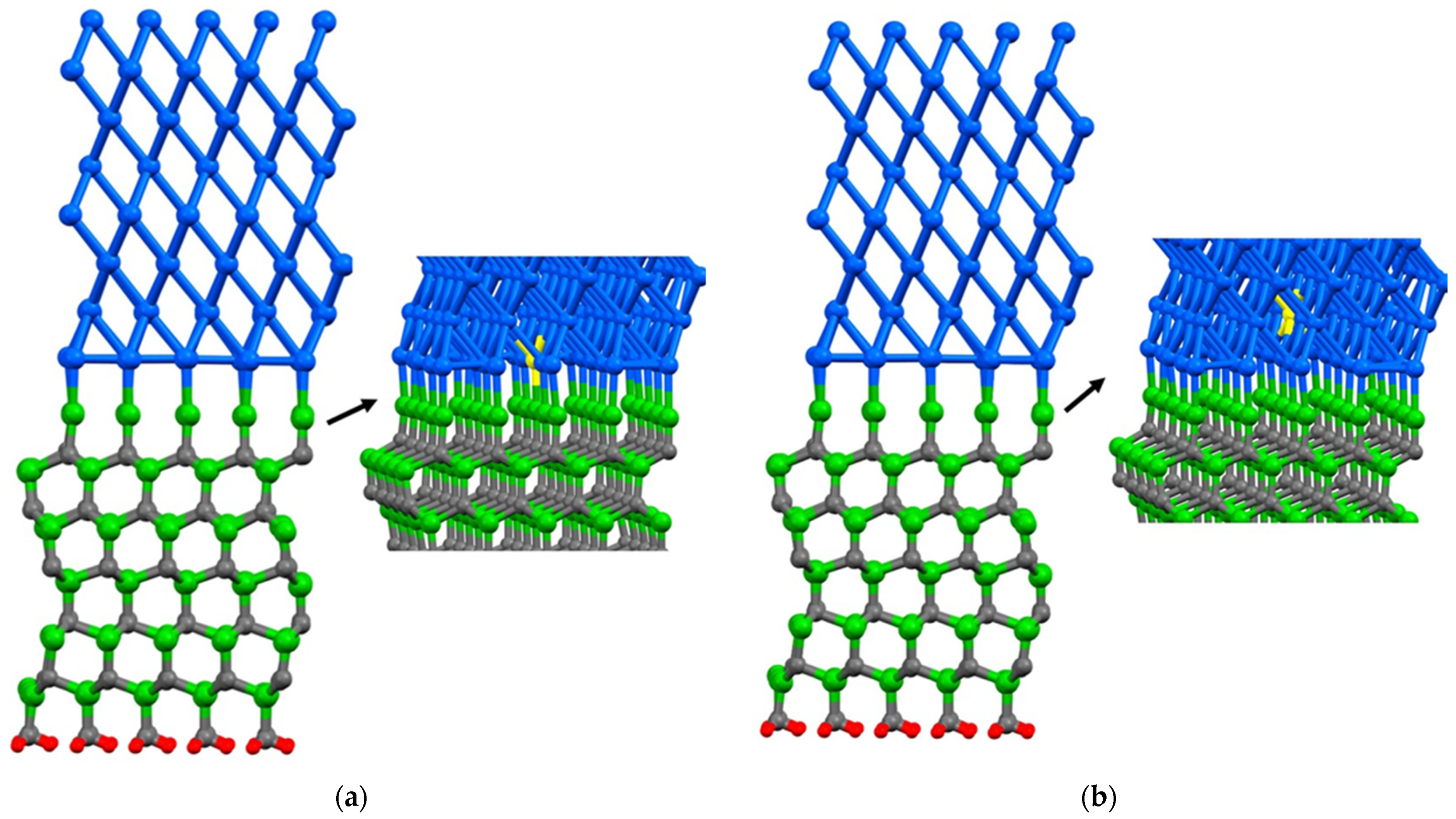
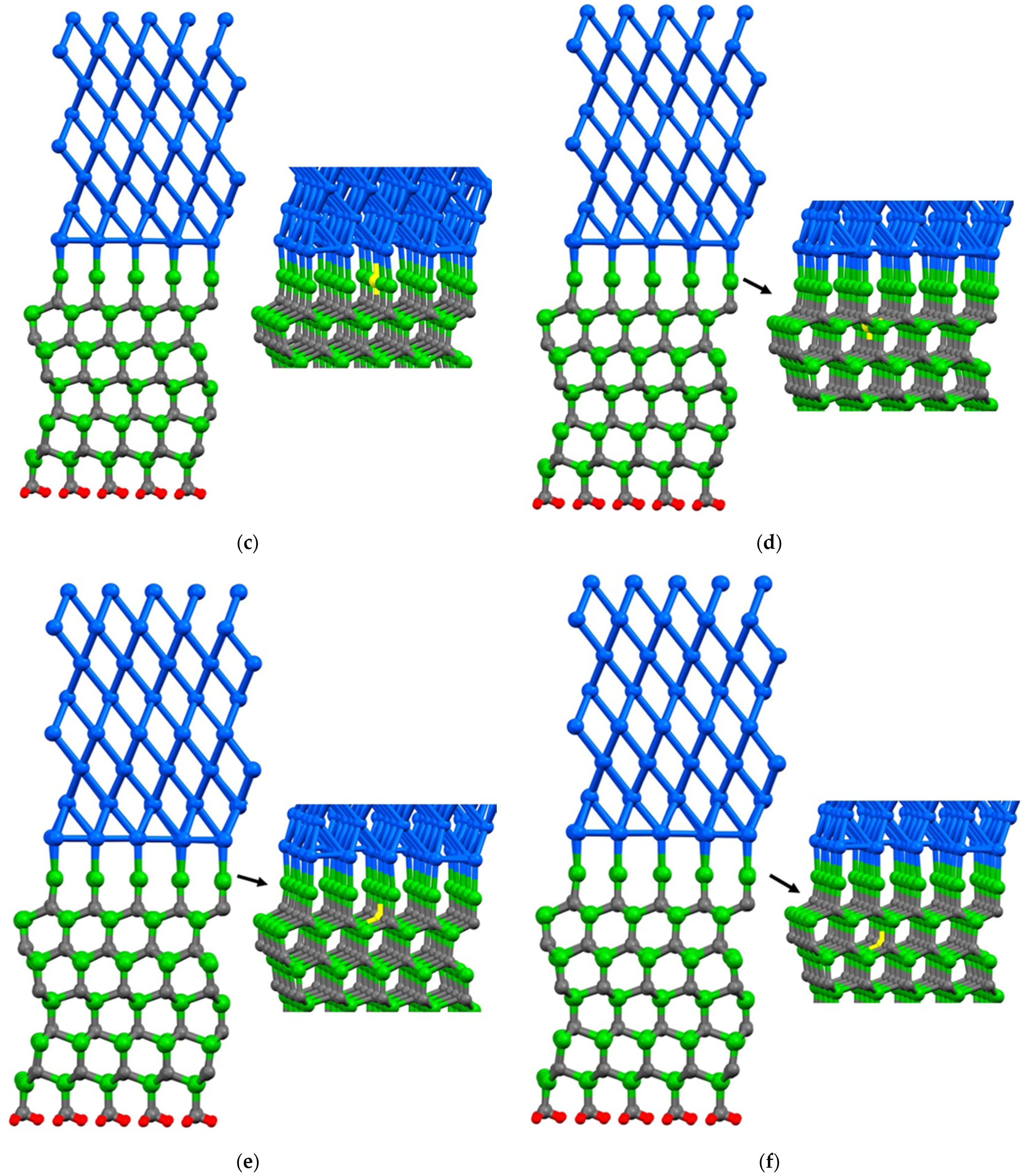



| Model | (J/m2) | Ef (J/m2) | d (Å) | |
|---|---|---|---|---|
| C-Terminated | No Vacancy | 2.991 | - | 1.95 |
| Al1 | 2.870 | 1.740 | 1.92 | |
| Al2 | 2.981 | 1.511 | 1.93 | |
| Si1 | 1.132 | 2.212 | 1.98 | |
| Si2 | 1.200 | 2.592 | 1.98 | |
| C1 | 0.853 | 2.641 | 2.01 | |
| C2 | 0.992 | 2.977 | 2.00 | |
| Si-Terminated | No Vacancy | 2.109 | - | 2.42 |
| Al1 | 2.085 | 1.702 | 2.52 | |
| Al2 | 2.108 | 1.518 | 2.53 | |
| Si1 | 1.036 | 2.161 | 2.54 | |
| Si2 | 1.310 | 2.407 | 2.53 | |
| C1 | 0.753 | 2.649 | 2.53 | |
| C2 | 0.828 | 2.956 | 2.53 |
| Model | Ɣ (J/m2) | KIC (MPa m1/2) | |
|---|---|---|---|
| No Vacancy | 3.29 | 1.40 | |
| Al1 | 3.28 | 1.39 | |
| Al2 | 3.17 | 1.36 | |
| C-Terminated | Si1 | 1.53 | 0.94 |
| Si2 | 1.60 | 0.97 | |
| C1 | 1.25 | 0.85 | |
| C2 | 1.39 | 0.90 | |
| No Vacancy | 2.60 | 1.21 | |
| Al1 | 2.60 | 1.21 | |
| Al2 | 2.58 | 1.20 | |
| Si- Terminated | Si1 | 1.53 | 0.92 |
| Si2 | 1.81 | 1.00 | |
| C1 | 1.25 | 0.83 | |
| C2 | 1.32 | 0.86 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fathalian, M.; Postek, E.; Sadowski, T. Mechanical and Electronic Properties of Al(111)/6H-SiC Interfaces: A DFT Study. Molecules 2023, 28, 4345. https://doi.org/10.3390/molecules28114345
Fathalian M, Postek E, Sadowski T. Mechanical and Electronic Properties of Al(111)/6H-SiC Interfaces: A DFT Study. Molecules. 2023; 28(11):4345. https://doi.org/10.3390/molecules28114345
Chicago/Turabian StyleFathalian, Mostafa, Eligiusz Postek, and Tomasz Sadowski. 2023. "Mechanical and Electronic Properties of Al(111)/6H-SiC Interfaces: A DFT Study" Molecules 28, no. 11: 4345. https://doi.org/10.3390/molecules28114345
APA StyleFathalian, M., Postek, E., & Sadowski, T. (2023). Mechanical and Electronic Properties of Al(111)/6H-SiC Interfaces: A DFT Study. Molecules, 28(11), 4345. https://doi.org/10.3390/molecules28114345







