Samarium Diiodide Acting on Acetone—Modeling Single Electron Transfer Energetics in Solution
Abstract
1. Introduction
2. Results
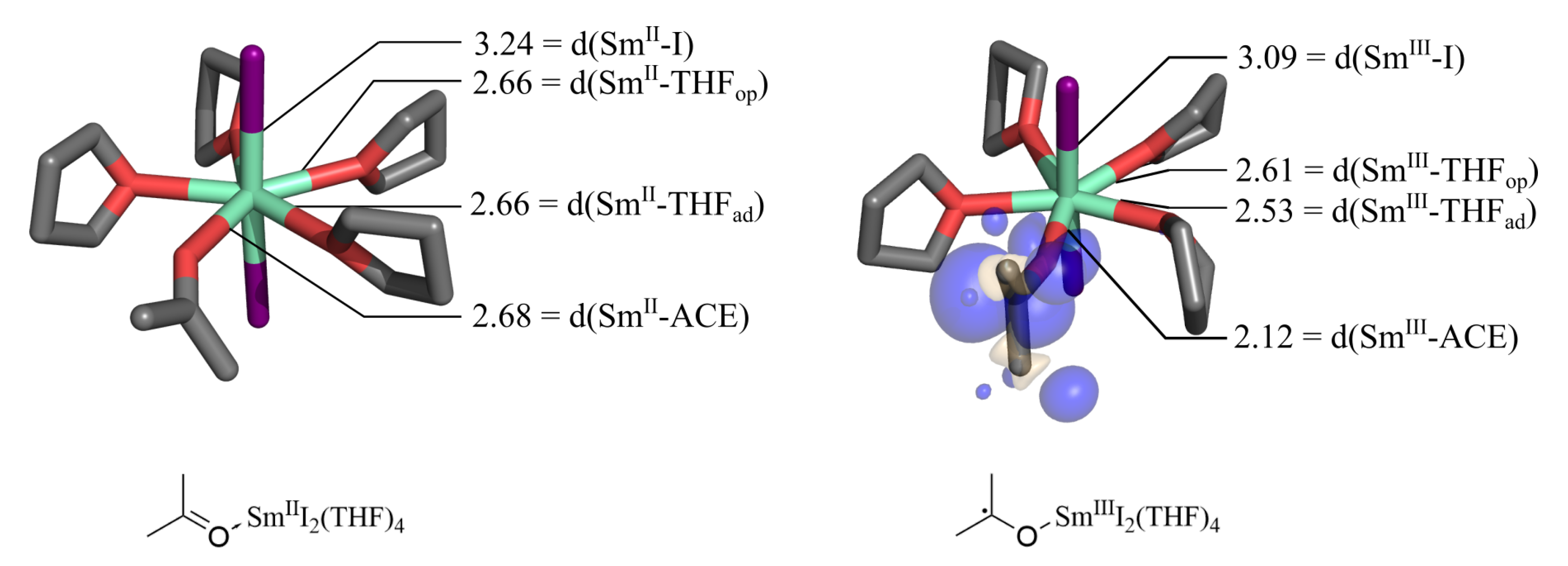
2.1. Optimized Structures
2.2. Electron Transfer Reaction Energy
2.3. Comparison of Quintet and Septet Spin State
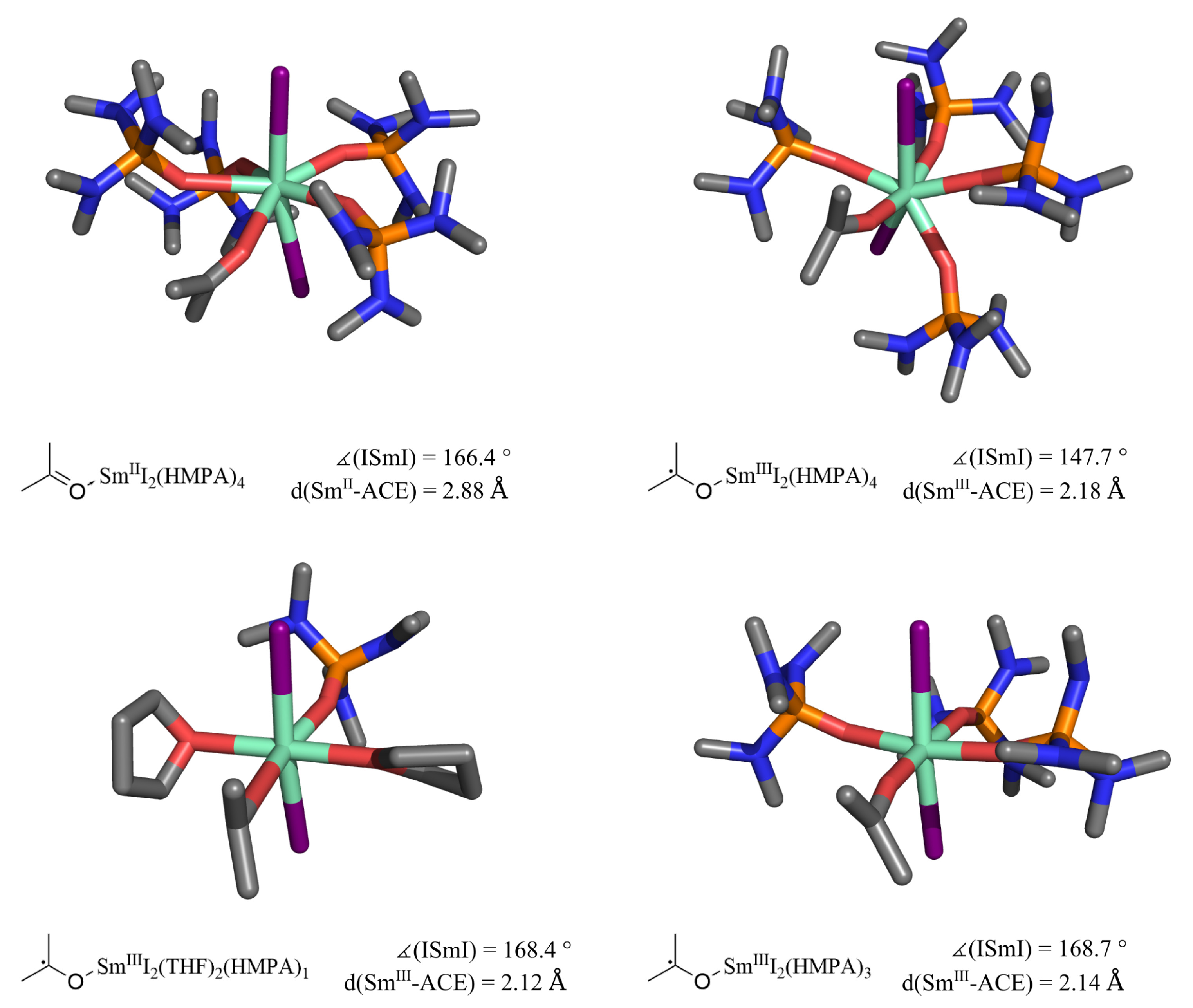
2.4. Effects of HMPA as Cosolvent
3. Methodology
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| DFT | Density functional theory |
| SET | Single electron transfer |
| ACE | Acetone |
| ACE | Ketyl radical anion |
| ECP | Electron core potential |
References
- Namy, J.L.; Girard, P.; Kagan, H. A new preparation of some divalent lanthanide iodides and their usefulness in organic synthesis. 1977. Available online: https://pascal-francis.inist.fr/vibad/index.php?action=getRecordDetail&idt=PASCAL7760218279 (accessed on 6 December 2022).
- Girard, P.; Namy, J.; Kagan, H. Divalent lanthanide derivatives in organic synthesis. 1. Mild preparation of samarium iodide and ytterbium iodide and their use as reducing or coupling agents. J. Am. Chem. Soc. 1980, 102, 2693–2698. [Google Scholar] [CrossRef]
- Molander, G.A.; McKie, J.A. Samarium (II) iodide-induced reductive cyclization of unactivated olefinic ketones. Sequential radical cyclization/intermolecular nucleophilic addition and substitution reactions. J. Org. Chem. 1992, 57, 3132–3139. [Google Scholar] [CrossRef]
- Molander, G.A.; Harris, C.R. Sequencing reactions with samarium II iodide. Chem. Rev. 1996, 96, 307–338. [Google Scholar] [CrossRef] [PubMed]
- Szostak, M.; Fazakerley, N.J.; Parmar, D.; Procter, D.J. Cross-coupling reactions using samarium II iodide. Chem. Rev. 2014, 114, 5959–6039. [Google Scholar] [CrossRef] [PubMed]
- Edmonds, D.J.; Johnston, D.; Procter, D.J. Samarium(II)-iodide-mediated cyclizations in natural product synthesis. Chem. Rev. 2004, 35, 3371–3404. [Google Scholar] [CrossRef] [PubMed]
- Gopalaiah, K.; Kagan, H.B. Recent developments in samarium diiodide promoted organic reactions. Chem. Rec. 2013, 13, 187–208. [Google Scholar] [CrossRef] [PubMed]
- Berndt, M.; Gross, S.; Hölemann, A.; Reissig, H.U. New samarium diiodide-induced ketyl couplings - From analogous reactions to serendipitously discovered processes. Synlett 2004, 422–438. [Google Scholar] [CrossRef]
- Procter, D.J.; Flowers, R.A.; Skrydstrup, T. Organic synthesis using samarium diiodide; Royal Society of Chemistry: London, UK, 2010. [Google Scholar] [CrossRef]
- Beemelmanns, C.; Reissig, H.U. Samarium diiodide induced ketyl-het arene cyclisations towards novel N-heterocycles. Chem. Soc. Rev. 2011, 40, 2199–2210. [Google Scholar] [CrossRef]
- Szostak, M.; Spain, M.; Procter, D.J. Recent advances in the chemoselective reduction of functional groups mediated by samarium II iodide: A single electron transfer approach. Chem. Soc. Rev. 2013, 42, 9155–9183. [Google Scholar] [CrossRef]
- Ashida, Y.; Arashiba, K.; Nakajima, K.; Nishibayashi, Y. Molybdenum-catalysed ammonia production with samarium diiodide and alcohols or water. Nature 2019, 568, 536–540. [Google Scholar] [CrossRef]
- Gong, J.; Chen, H.; Liu, X.Y.; Wang, Z.X.; Nie, W.; Qin, Y. Total synthesis of atropurpuran. Nat. Commun. 2016, 7. [Google Scholar] [CrossRef] [PubMed]
- Leung, J.C.; Bedermann, A.A.; Njardarson, J.T.; Spiegel, D.A.; Murphy, G.K.; Hama, N.; Twenter, B.M.; Dong, P.; Shirahata, T.; McDonald, I.M.; et al. Total synthesis of (A)-phomoidride D. Angew. Chem. Int. Ed. 2018, 57, 1991–1994. [Google Scholar] [CrossRef] [PubMed]
- Boekell, N.G.; Flowers, R.A. Coordination-induced bond weakening. Chem. Rev. 2022, 122, 13447–13477. [Google Scholar] [CrossRef]
- Achazi, A.J.; Dirk, A.; Reissig, H.U.; Paulus, B. A computational study of samarium diiodide-induced cyclizations of N-oxoalkyl-substituted methyl indole-3-carboxylates—A rationale of the diastereoselectivity. J. Comput. Chem. 2017, 38, 2693–2700. [Google Scholar] [CrossRef] [PubMed]
- Kefalidis, C.E.; Essafi, S.; Perrin, L.; Maron, L. Qualitative estimation of the single-electron transfer step energetics mediated by samarium (II) complexes: A “SOMO–LUMO Gap” Approach. Inorg. Chem. 2014, 53, 3427–3433. [Google Scholar] [CrossRef] [PubMed]
- Kefalidis, C.E.; Castro, L.; Perrin, L.; Del Rosal, I.; Maron, L. New perspectives in organolanthanide chemistry from redox to bond metathesis: Insights from theory. Chem. Soc. Rev. 2016, 45, 2516–2543. [Google Scholar] [CrossRef] [PubMed]
- Ramírez-Solís, A.; Boekell, N.G.; León-Pimentel, C.I.; Saint-Martin, H.; Bartulovich, C.O.; Flowers, R.A. Ammonia solvation vs aqueous solvation of samarium diiodide. A theoretical and experimental approach to understanding bond activation upon coordination to Sm (II). J. Org. Chem. 2021, 87, 1689–1697. [Google Scholar] [CrossRef]
- Dyer, H.E.; Huijser, S.; Susperregui, N.; Bonnet, F.; Schwarz, A.D.; Duchateau, R.; Maron, L.; Mountford, P. Ring-opening polymerization of rac-lactide by bis (phenolate) amine-supported samarium borohydride complexes: An experimental and DFT study. Organometallics 2010, 29, 3602–3621. [Google Scholar] [CrossRef]
- Perrin, L.; Kirillov, E.; Carpentier, J.F.; Maron, L. DFT Investigation of the tacticity control during styrene polymerization catalyzed by single-component allyl ansa-lanthanidocenes {(C5H4CMe2 (9-C13H8)} Ln (C3H5). Macromolecules 2010, 43, 6330–6336. [Google Scholar] [CrossRef]
- Dolg, M.; Stoll, H.; Savin, A.; Preuss, H. Energy-adjusted pseudopotentials for the rare earth elements. Theor. Chim. Acta 1989, 75, 173–194. [Google Scholar] [CrossRef]
- Dolg, M.; Stoll, H.; Preuss, H. A combination of quasirelativistic pseudopotential and ligand field calculations for lanthanoid compounds. Theor. Chim. Acta 1993, 85, 441–450. [Google Scholar] [CrossRef]
- Kelly, R.P.; Toniolo, D.; Tirani, F.F.; Maron, L.; Mazzanti, M. A tetranuclear samarium (ii) inverse sandwich from direct reduction of toluene by a samarium (ii) siloxide. Chem. Commun. 2018, 54, 10268–10271. [Google Scholar] [CrossRef] [PubMed]
- Agasti, S.; Beattie, N.A.; McDouall, J.J.W.; Procter, D.J. SmI2-catalyzed intermolecular coupling of cyclopropyl ketones and alkynes: A link between ketone conformation and reactivity. J. Am. Chem. Soc. 2021, 143, 3655–3661. [Google Scholar] [CrossRef] [PubMed]
- Dolg, M.; Stoll, H.; Preuss, H. Energy-adjusted abinitio pseudopotentials for the rare earth elements. J. Chem. Phys. 1989, 90, 1730–1734. [Google Scholar] [CrossRef]
- Beemelmanns, C.; Blot, V.; Gross, S.; Lentz, D.; Reissig, H.U. Highly diastereoselective samarium diiodide induced ketyl cyclisations of indole and pyrrole derivatives – scope and limitations. Eur. J. Org. Chem. 2010, 2010, 2716–2732. [Google Scholar] [CrossRef]
- Curran, D.P.; Fevig, T.L.; Jasperse, C.P.; Totleben, M.J. New mechanistic insights into reductions of halides and radicals with samarium (II) iodide. Synlett 1992, 1992, 943–961. [Google Scholar] [CrossRef]
- Wefelscheid, U.K.; Berndt, M.; Reißig, H.U. Samarium diiodide mediated ketyl–aryl coupling reactions – influence of substituents and trapping experiments. Eur. J. Org. Chem. 2008, 2008, 3635–3646. [Google Scholar] [CrossRef]
- Shabangi, M.; Sealy, J.M.; Fuchs, J.R.; Flowers, R.A., II. The effect of cosolvent on the reducing power of SmI2 in tetrahydrofuran. Tetrahedron Lett. 1998, 39, 4429–4432. [Google Scholar] [CrossRef]
- Hou, Z.; Zhang, Y.; Wakatsuki, Y. Molecular structures of HMPA-coordinated samarium (II) and ytterbium (II) iodide complexes. A structural basis for the HMPA effects in SmI2-promoted reactions. Bull. Chem. Soc. Jpn. 1997, 70, 149–153. [Google Scholar] [CrossRef]
- Shotwell, J.B.; Sealy, J.M.; Flowers, R.A. Structure and Energetics of the Samarium Diiodide HMPA Complex in Tetrahydrofuran. J. Org. Chem. 1999, 64, 5251–5255. [Google Scholar] [CrossRef]
- Enemærke, R.J.; Hertz, T.; Skrydstrup, T.; Daasbjerg, K. Evidence for ionic samarium (II) species in THF/HMPA solution and investigation of their electron-donating properties. Chem.- Eur. J. 2000, 6, 3747–3754. [Google Scholar] [CrossRef] [PubMed]
- Shabangi, M.; Flowers, R.A., II. Electrochemical investigation of the reducing power of SmI2 in THF and the effect of HMPA cosolvent. Tetrahedron Lett. 1997, 38, 1137–1140. [Google Scholar] [CrossRef]
- Kefalidis, C.E.; Perrin, L.; Maron, L. Preliminary theoretical insights into SmI2-mediated reactions: Activation of ketones in THF. Eur. J. Inorg. Chem. 2013, 2013, 4042–4049. [Google Scholar] [CrossRef]
- Hoz, S. Samarium iodide showcase: Unraveling the mechanistic puzzle. Acc. Chem. Res. 2020, 53, 2680–2691. [Google Scholar] [CrossRef] [PubMed]
- Inanaga, J.; Ishikawa, M.; Yamaguchi, M. A mild and convenient method for the reduction of organic halides by using a SmI2-THF solution in the presence of hexamethylphosphoric triamide (HMPA). Chem. Lett. 1987, 16, 1485–1486. [Google Scholar] [CrossRef]
- Enemærke, R.J.; Daasbjerg, K.; Skrydstrup, T. Is samarium diiodide an inner-or outer-sphere electron donating agent? Chem. Commun. 1999, 343–344. [Google Scholar] [CrossRef]
- Sadasivam, D.V.; Teprovich, J.A.J.; Procter, D.J.; Flowers, R.A.I. Dynamic ligand exchange in reactions of samarium diiodide. Org. Lett. 2010, 12, 4140–4143. [Google Scholar] [CrossRef]
- Yang, J.; Dolg, M. Valence basis sets for lanthanide 4f-in-core pseudopotentials adapted for crystal orbital ab initio calculations. Theor. Chem. Acc. 2005, 113, 212–224. [Google Scholar] [CrossRef]
- Weigand, A.; Cao, X.; Yang, J.; Dolg, M. Quasirelativistic f-in-core pseudopotentials and core-polarization potentials for trivalent actinides and lanthanides: Molecular test for trifluorides. Theor. Chem. Acc. 2010, 126, 117–127. [Google Scholar] [CrossRef]
- Dirac, P.A.M.; Fowler, R.H. Quantum mechanics of many-electron systems. Proc. R. Soc. Lond. Ser. A Contain. Pap. A Math. Phys. Character 1929, 123, 714–733. [Google Scholar] [CrossRef]
- Slater, J.C. A Simplification of the Hartree-Fock Method. Phys. Rev. 1951, 81, 385–390. [Google Scholar] [CrossRef]
- Perdew, J.P.; Wang, Y. Erratum: Accurate and simple analytic representation of the electron-gas correlation energy [Phys. Rev. B 45, 13244 (1992)]. Phys. Rev. B 2018, 98, 079904. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized gradient approximation made simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed]
- Perdew, J.P.; Ernzerhof, M.; Burke, K. Rationale for mixing exact exchange with density functional approximations. J. Chem. Phys. 1996, 105, 9982–9985. [Google Scholar] [CrossRef]
- Vosko, S.H.; Wilk, L.; Nusair, M. Accurate spin-dependent electron liquid correlation energies for local spin density calculations: A critical analysis. Can. J. Phys. 1980, 58, 1200–1211. [Google Scholar] [CrossRef]
- Becke, A.D. Density-functional exchange-energy approximation with correct asymptotic behavior. Phys. Rev. A 1988, 38, 3098–3100. [Google Scholar] [CrossRef]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Becke, A.D. A new mixing of Hartree–Fock and local density-functional theories. J. Chem. Phys. 1993, 98, 1372–1377. [Google Scholar] [CrossRef]
- Tao, J.; Perdew, J.P.; Staroverov, V.N.; Scuseria, G.E. Climbing the density functional ladder: Nonempirical meta–generalized gradient approximation designed for molecules and solids. Phys. Rev. Lett. 2003, 91, 146401. [Google Scholar] [CrossRef]
- Staroverov, V.N.; Scuseria, G.E.; Tao, J.; Perdew, J.P. Comparative assessment of a new nonempirical density functional: Molecules and hydrogen-bonded complexes. J. Chem. Phys. 2003, 119, 12129–12137. [Google Scholar] [CrossRef]
- Schäfer, A.; Klamt, A.; Sattel, D.; Lohrenz, J.C.; Eckert, F. COSMO Implementation in TURBOMOLE: Extension of an efficient quantum chemical code towards liquid systems. Phys. Chem. Chem. Phys. 2000, 2, 2187–2193. [Google Scholar] [CrossRef]
- Fdez. Galván, I.; Vacher, M.; Alavi, A.; Angeli, C.; Aquilante, F.; Autschbach, J.; Bao, J.J.; Bokarev, S.I.; Bogdanov, N.A.; Carlson, R.K.; et al. OpenMolcas: From source code to insight. J. Chem. Theory Comput. 2019, 15, 5925–5964. [Google Scholar] [CrossRef]
- Roos, B.O.; Lindh, R.; Malmqvist, P.Å.; Veryazov, V.; Widmark, P.O. Main group atoms and dimers studied with a new relativistic ANO basis set. J. Phys. Chem. A 2003, 108, 2851–2858. [Google Scholar] [CrossRef]
- Roos, B.O.; Lindh, R.; Malmqvist, P.Å.; Veryazov, V.; Widmark, P.O.; Borin, A.C. New relativistic atomic natural orbital basis sets for lanthanide atoms with applications to the Ce diatom and LuF3. J. Phys. Chem. A 2008, 112, 11431–11435. [Google Scholar] [CrossRef]
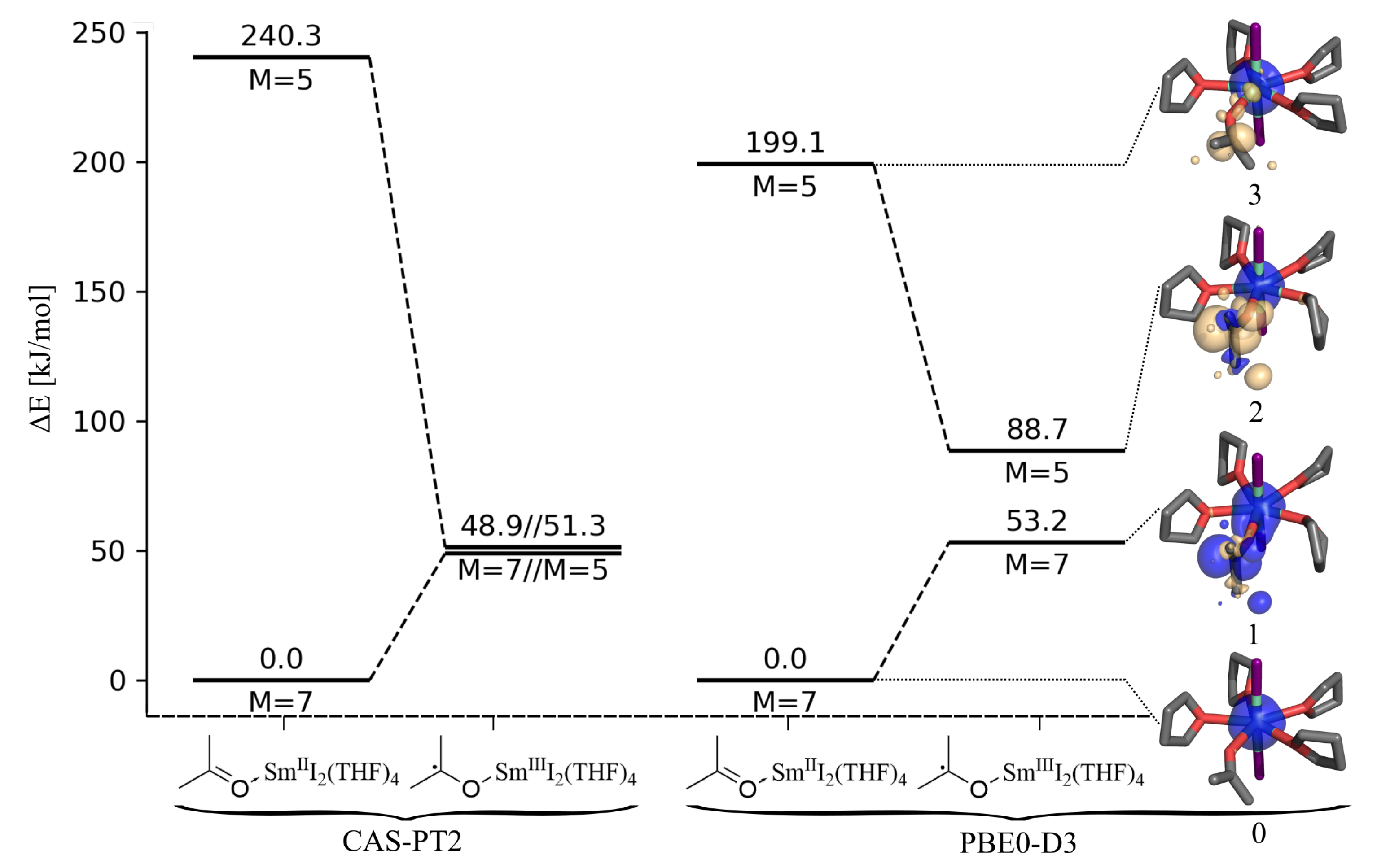
| CASSCF (6,13) | 51.94 |
| CASPT2 (6,13) | 48.94 |
| TPSS-D3 | 12.76 |
| PBE-D3 | 28.91 |
| TPSSH-D3 | 27.28 |
| PBE0-D3 | 53.19 |
| B3LYP-D3 | 55.40 |
| BHLYP-D3 | 38.42 |
| B3PW91-D3 | 69.66 |
| B3PW91 | 40.02 |
| Molecule | ||||||
|---|---|---|---|---|---|---|
| 53.19 | 66.92 | 84.61 | −331.54 | −335.73 | −359.60 | |
| 52.74 | 65.80 | 77.70 | −333.64 | −336.55 | −357.36 | |
| 37.50 | 52.74 | 58.14 | −262.95 | −262.06 | −292.57 | |
| 47.90 | 51.56 | 63.26 | −270.83 | −245.93 | −271.51 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Steiner, L.; Achazi, A.J.; Vlaisavljevich, B.; Miro, P.; Paulus, B.; Kelterer, A.-M. Samarium Diiodide Acting on Acetone—Modeling Single Electron Transfer Energetics in Solution. Molecules 2022, 27, 8673. https://doi.org/10.3390/molecules27248673
Steiner L, Achazi AJ, Vlaisavljevich B, Miro P, Paulus B, Kelterer A-M. Samarium Diiodide Acting on Acetone—Modeling Single Electron Transfer Energetics in Solution. Molecules. 2022; 27(24):8673. https://doi.org/10.3390/molecules27248673
Chicago/Turabian StyleSteiner, Luca, Andreas J. Achazi, Bess Vlaisavljevich, Pere Miro, Beate Paulus, and Anne-Marie Kelterer. 2022. "Samarium Diiodide Acting on Acetone—Modeling Single Electron Transfer Energetics in Solution" Molecules 27, no. 24: 8673. https://doi.org/10.3390/molecules27248673
APA StyleSteiner, L., Achazi, A. J., Vlaisavljevich, B., Miro, P., Paulus, B., & Kelterer, A.-M. (2022). Samarium Diiodide Acting on Acetone—Modeling Single Electron Transfer Energetics in Solution. Molecules, 27(24), 8673. https://doi.org/10.3390/molecules27248673






