Exploring Word-Adjacency Networks with Multifractal Time Series Analysis Techniques
Abstract
1. Introduction
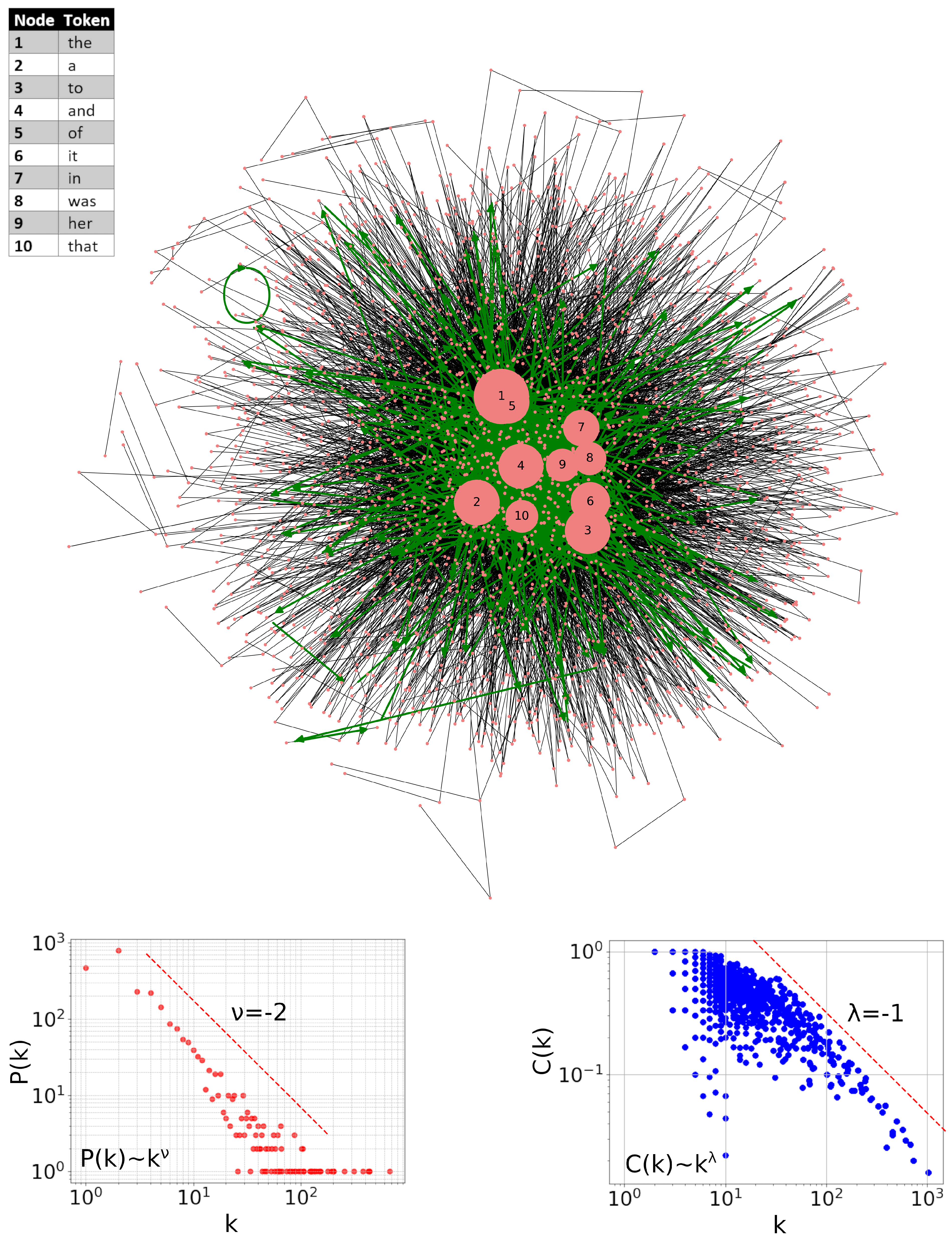
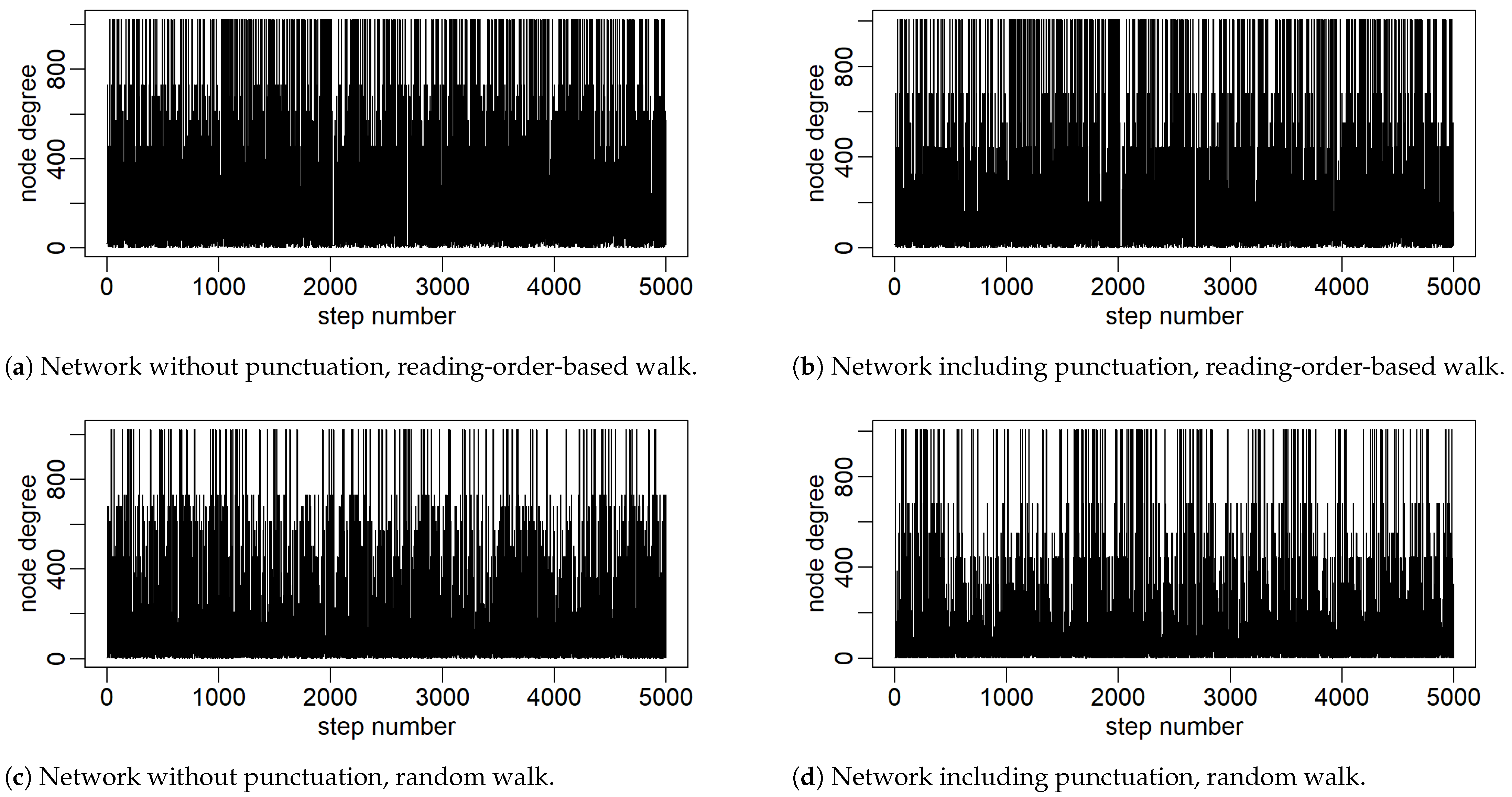
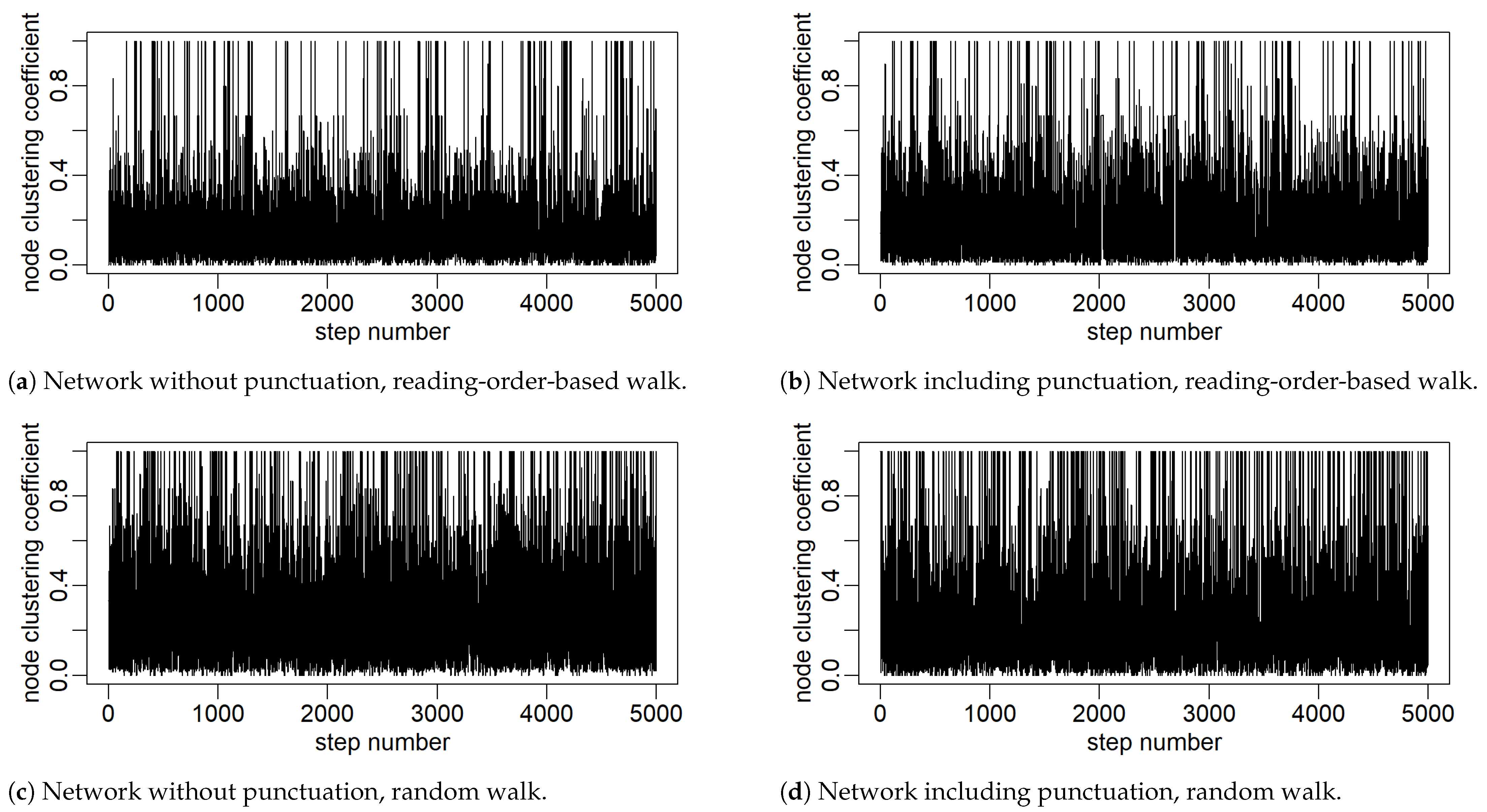
2. From Word-Adjacency Networks to Time Series
3. Detrended Fluctuation Analysis
3.1. Multifractal Formalism
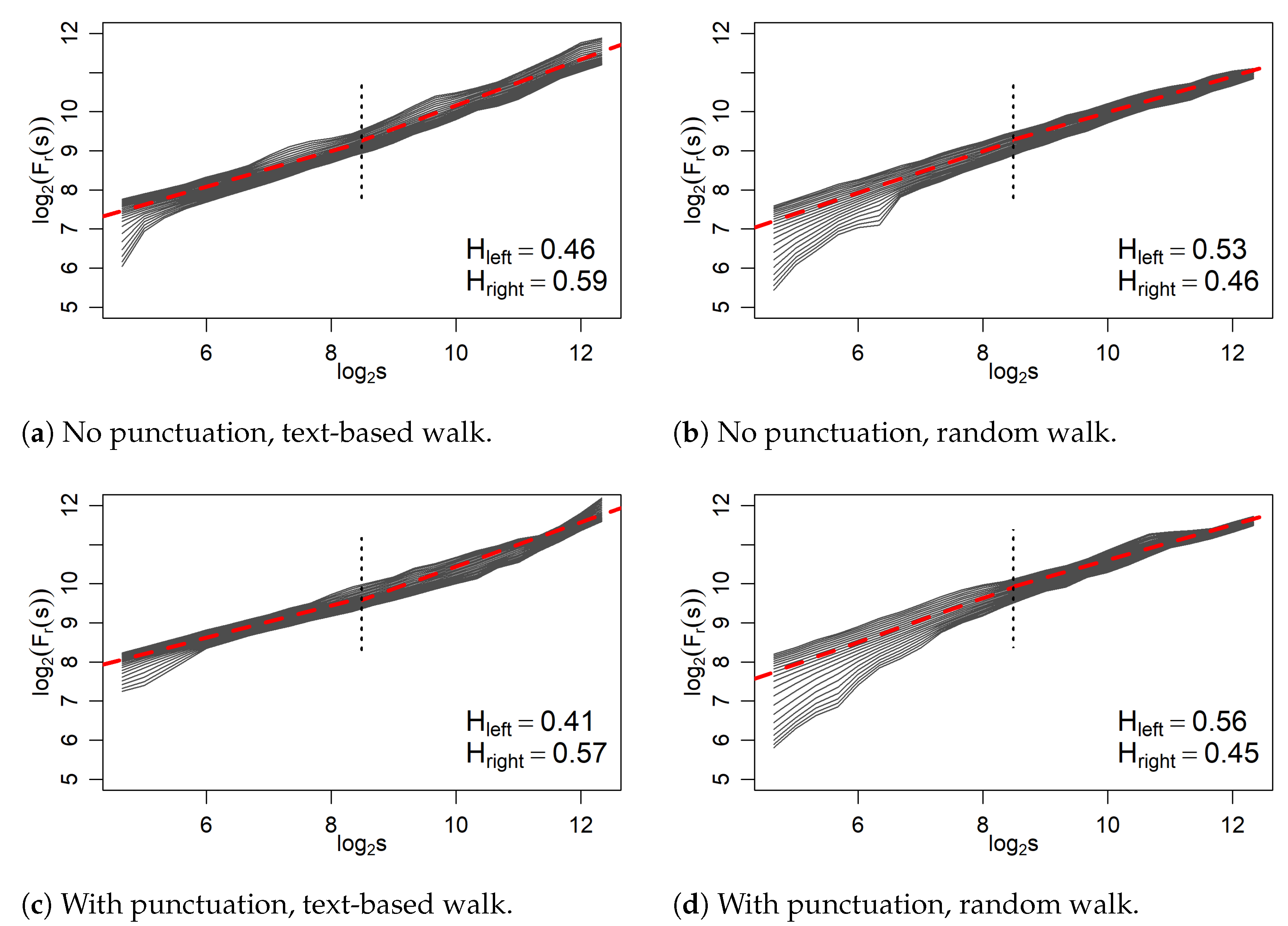
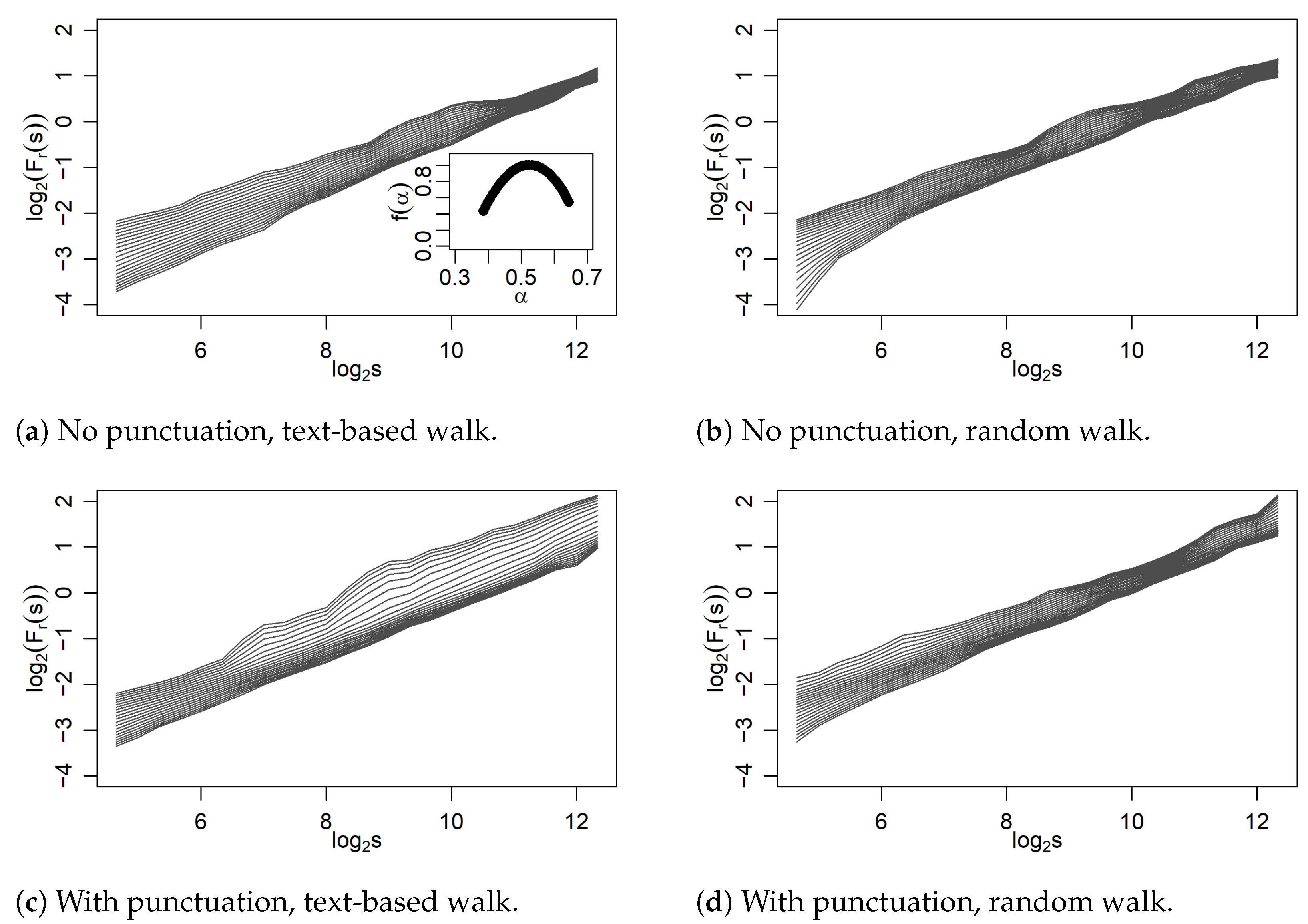
3.2. Fluctuation Functions and Their Scaling Characteristics
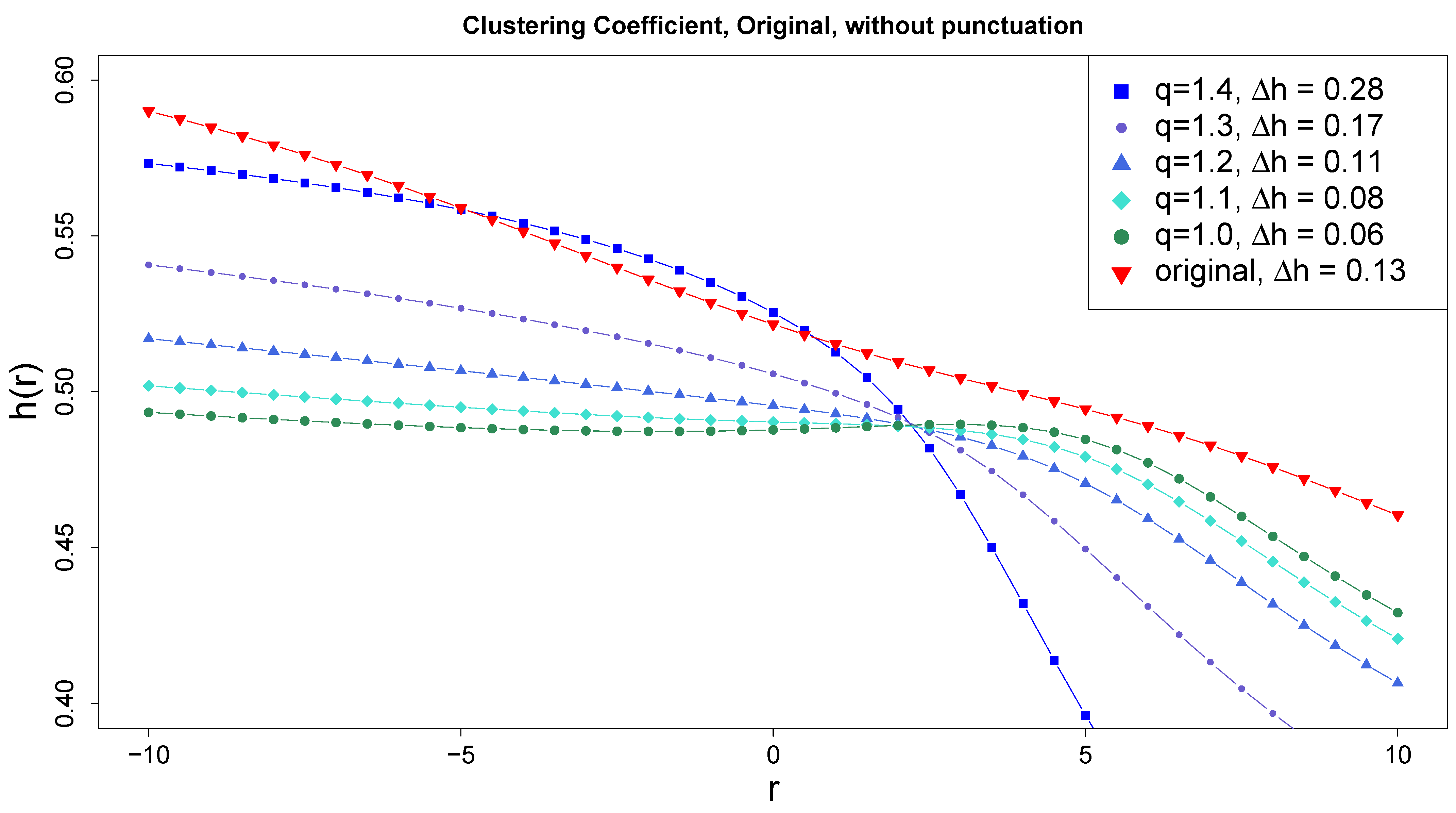
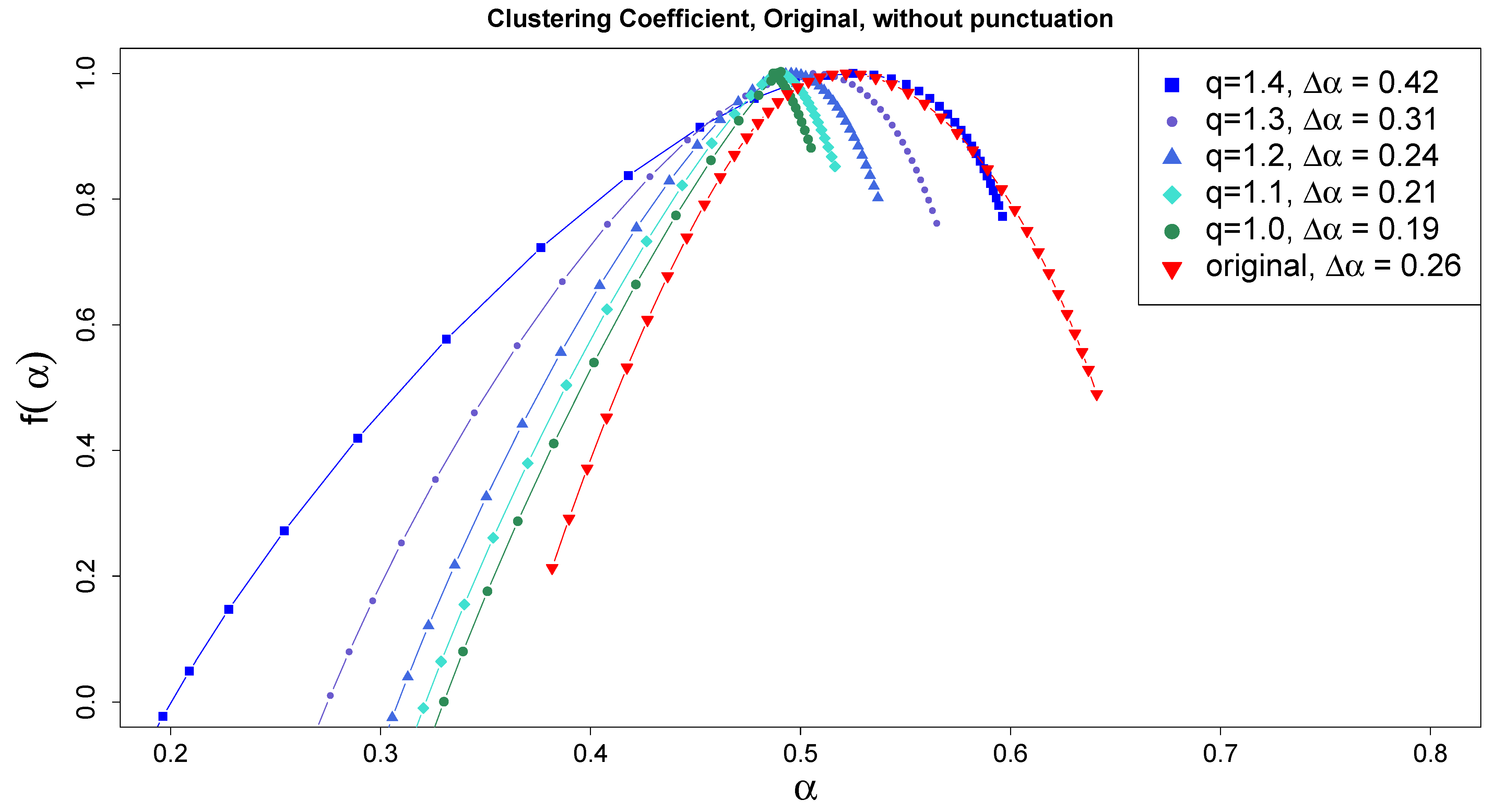
3.3. Testing Significance of Multifractality Signals
4. Summary
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Albert, R.; Barabási, A.L. Statistical mechanics of complex networks. Rev. Mod. Phys. 2002, 74, 47–97. [Google Scholar] [CrossRef]
- Dorogovtsev, S.N.; Mendes, J.F. Evolution of networks. Adv. Phys. 2002, 51, 1079–1187. [Google Scholar] [CrossRef]
- Dorogovtsev, S.N.; Goltsev, A.V.; Mendes, J.F. Critical phenomena in complex networks. Rev. Mod. Phys. 2008, 80, 1275–1335. [Google Scholar] [CrossRef]
- Boccaletti, S.; Latora, V.; Moreno, Y.; Chavez, M.; Hwang, D.U. Complex networks: Structure and dynamics. Phys. Rep. 2006, 424, 175–308. [Google Scholar] [CrossRef]
- Ji, P.; Ye, J.; Mu, Y.; Lin, W.; Tian, Y.; Hens, C.; Perc, M.; Tang, Y.; Sun, J.; Kurths, J. Signal propagation in complex networks. Phys. Rep. 2023, 1017, 1–96. [Google Scholar] [CrossRef]
- Amancio, D.R.; Antiqueira, L.; Pardo, T.A.S.; Da, L.; Costa, F.; Oliveira, O.N.; Nunes, M.G.V. Complex networks analysis of manual and machine translations. Int. J. Mod. Phys. C 2008, 19, 583–598. [Google Scholar]
- Choudhury, M.; Mukherjee, A. The Structure and Dynamics of Linguistic Networks; Birkhäuser: Basel, Switzerland, 2009; pp. 145–166. [Google Scholar] [CrossRef]
- Liu, H.T.; Li, W.W. Language clusters based on linguistic complex networks. Chin. Sci. Bull. 2010, 55, 3458–3465. [Google Scholar] [CrossRef]
- Araújo, T.; Banisch, S. Multidimensional Analysis of Linguistic Networks; Springer: Berlin/Heidelberg, Germany, 2015; pp. 107–131. [Google Scholar] [CrossRef]
- Ramirez-Arellano, A. Classification of Literary Works: Fractality and Complexity of the Narrative, Essay, and Research Article. Entropy 2020, 22, 904. [Google Scholar] [CrossRef]
- Stanisz, T.; Drożdż, S.; Kwapień, J. Complex systems approach to natural language. Phys. Rep. 2024, 1053, 1–84. [Google Scholar] [CrossRef]
- Antiqueira, L.; Nunes, M.G.; Oliveira, O.N.; da F. Costa, L. Strong correlations between text quality and complex networks features. Phys. A 2007, 373, 811–820. [Google Scholar] [CrossRef]
- Borge-Holthoefer, J.; Arenas, A. Semantic networks: Structure and dynamics. Entropy 2010, 12, 1264–1302. [Google Scholar] [CrossRef]
- de Arruda, H.F.; Silva, F.N.; Marinho, V.Q.; Amancio, D.R.; da Fontoura Costa, L. Representation of texts as complex networks: A mesoscopic approach. J. Complex Netw. 2018, 6, 125–144. [Google Scholar] [CrossRef]
- Oliveira, D.A.; de Barros Pereira, H.B. Modeling texts with networks: Comparing five approaches to sentence representation. Eur. Phys. J. B 2024, 97, 77. [Google Scholar] [CrossRef]
- Dorogovtsev, S.N.; Mendes, J.F. Language as an evolving word web. Proc. R. Soc. B Biol. Sci. 2001, 268, 2603–2606. [Google Scholar] [CrossRef]
- Segarra, S.; Eisen, M.; Ribeiro, A. Authorship attribution through function word adjacency networks. IEEE Trans. Signal Process. 2015, 63, 5464–5478. [Google Scholar] [CrossRef]
- Amancio, D.R. Probing the topological properties of complex networks modeling short written texts. PLoS ONE 2015, 10, e0118394. [Google Scholar] [CrossRef]
- Kulig, A.; Drożdż, S.; Kwapień, J.; Oświęcimka, P. Modeling the average shortest-path length in growth of word-adjacency networks. Phys. Rev. E 2015, 91, 032810. [Google Scholar] [CrossRef]
- Campanharo, A.S.L.O.; Sirer, M.I.; Malmgren, R.D.; Ramos, F.M.; Amaral, L.A.N. Duality between Time Series and Networks. PLoS ONE 2011, 6, e23378. [Google Scholar] [CrossRef]
- Hou, L.; Small, M.; Lao, S. Dynamical systems induced on networks constructed from time series. Entropy 2015, 17, 6433–6446. [Google Scholar] [CrossRef]
- Khor, A.; Small, M. Examining k-nearest neighbour networks: Superfamily phenomena and inversion. Chaos 2016, 26, 043101. [Google Scholar] [CrossRef]
- McCullough, M.; Sakellariou, K.; Stemler, T.; Small, M. Regenerating time series from ordinal networks. Chaos 2017, 27, 035814. [Google Scholar] [CrossRef] [PubMed]
- Lacasa, L.; Luque, B.; Ballesteros, F.; Luque, J.; Nuño, J.C. From time series to complex networks: The visibility graph. Proc. Natl. Acad. Sci. USA 2008, 105, 4972–4975. [Google Scholar] [CrossRef] [PubMed]
- Nuñez, A.M.; Lacasa, L.; Gomez, J.P.; Luque, B. Visibility Algorithms: A Short Review; InTech: Houston TX, USA, 2012; pp. 119–152. [Google Scholar]
- Donner, R.V.; Small, M.; Donges, J.F.; Marwan, N.; Zou, Y.; Xiang, R.; Kurths, J. Recurrence-based time series analysis by means of complex network methods. Int. J. Bifurc. Chaos 2011, 21, 1019–1046. [Google Scholar] [CrossRef]
- Nicosia, V.; De Domenico, M.; Latora, V. Characteristic exponents of complex networks. Europhys. Lett. 2014, 106, 58005. [Google Scholar] [CrossRef]
- Noh, J.D.; Rieger, H. Random walks on complex networks. Phys. Rev. Lett. 2004, 92, 118701. [Google Scholar] [CrossRef]
- Masuda, N.; Porter, M.A.; Lambiotte, R. Random walks and diffusion on networks. Phys. Rep. 2017, 716–717, 1–58. [Google Scholar] [CrossRef]
- Oświęcimka, P.; Livi, L.; Drożdż, S. Multifractal cross-correlation effects in two-variable time series of complex network vertex observables. Phys. Rev. E 2016, 94, 042307. [Google Scholar] [CrossRef]
- Oświęcimka, P.; Livi, L.; Drożdż, S. Right-side-stretched multifractal spectra indicate small-worldness in networks. Commun. Nonlinear Sci. Numer. Simul. 2018, 57, 231–245. [Google Scholar] [CrossRef]
- Ausloos, M. Generalized Hurst exponent and multifractal function of original and translated texts mapped into frequency and length time series. Phys. Rev. E 2012, 86, 031108. [Google Scholar] [CrossRef]
- Kulig, A.; Kwapień, J.; Stanisz, T.; Drożdż, S. In narrative texts punctuation marks obey the same statistics as words. Inf. Sci. 2017, 375, 98–113. [Google Scholar] [CrossRef]
- Zipf, G.K. Human Behavior and the Principle of Least Effort: An Introduction to Human Ecology; Addison-Wesley Press: Cambridge, UK, 1949. [Google Scholar] [CrossRef]
- Piantadosi, S.T. Zipf’s word frequency law in natural language: A critical review and future directions. Psychon. Bull. Rev. 2014, 21, 1112–1130. [Google Scholar] [CrossRef] [PubMed]
- Ravasz, E.; Barabasi, A.L. Hierarchical organization in complex networks. Phys. Rev. E 2003, 67, 026112. [Google Scholar] [CrossRef]
- Eckmann, J.P.; Moses, E.; Sergi, D. Entropy of dialogues creates coherent structures in e-mail traffic. Proc. Natl. Acad. Sci. USA 2004, 101, 14333–14337. [Google Scholar] [CrossRef]
- Kwapień, J.; Drożdż, S. Physical approach to complex systems. Phys. Rep. 2012, 515, 115–226. [Google Scholar] [CrossRef]
- Jimenez, J. Intermittency and cascades. J. Fluid Mech. 2000, 409, 99–120. [Google Scholar]
- Kantelhardt, J.W.; Zschiegner, S.A.; Koscielny-Bunde, E.; Havlin, S.; Bunde, A.; Stanley, H.E. Multifractal detrended fluctuation analysis of nonstationary time series. Phys. A 2002, 316, 87–114. [Google Scholar] [CrossRef]
- Oświęcimka, P.; Kwapień, J.; Drożdż, S. Wavelet versus detrended fluctuation analysis of multifractal structures. Phys. Rev. E 2006, 74, 016103. [Google Scholar] [CrossRef]
- Ausloos, M. Measuring complexity with multifractals in texts: Translation effects. Chaos Solitons Fractals 2012, 45, 1349–1357. [Google Scholar] [CrossRef]
- Drożdż, S.; Oświęcimka, P.; Kulig, A.; Kwapień, J.; Bazarnik, K.; Grabska-Gradzińska, I.; Rybicki, J.; Stanuszek, M. Quantifying origin and character of long-range correlations in narrative texts. Inf. Sci. 2016, 331, 32–44. [Google Scholar] [CrossRef]
- Dec, J.; Dolina, M.; Drożdż, S.; Kwapień, J.; Stanisz, T. Multifractal Hopscotch in Hopscotch by Julio Cortázar. Entropy 2024, 26, 716. [Google Scholar] [CrossRef]
- Bartnicki, K.; Drożdż, S.; Kwapień, J.; Stanisz, T. Punctuation patterns in Finnegans Wake by James Joyce are largely translation-invariant. Entropy 2025, 27, 177. [Google Scholar] [CrossRef] [PubMed]
- Peng, C.K.; Buldyrev, S.V.; Havlin, S.; Simons, M.; Stanley, H.E.; Goldberger, A.L. Mosaic organization of DNA nucleotides. Phys. Rev. E 1994, 49, 1685. [Google Scholar] [CrossRef]
- Hurst, H. Long-term storage capacity of reservoirs. Trans. Am. Soc. Civ. Eng. 1951, 116, 770–799. [Google Scholar]
- Heneghan, C.; McDarby, G. Establishing the relation between detrended fluctuation analysis and power spectral density analysis for stochastic processes. Phys. Rev. E 2000, 62, 6103–6110. [Google Scholar]
- Halsey, T.C.; Jensen, M.H.; Kadanoff, L.P.; Procaccia, I.; Shraimant, B.I. Fractal measures and their singularities: The characterization of strange sets. Phys. Rev. A 1986, 33, 1141–1151. [Google Scholar]
- Ohashi, K.; Amaral, L.A.; Natelson, B.H.; Yamamoto, Y. Asymmetrical singularities in real-world signals. Phys. Rev. E 2003, 68, 065204. [Google Scholar] [CrossRef]
- Cao, G.; Cao, J.; Xu, L. Asymmetric multifractal scaling behavior in the Chinese stock market: Based on asymmetric MF-DFA. Phys. A 2013, 392, 797–807. [Google Scholar] [CrossRef]
- Drożdż, S.; Oświęcimka, P. Detecting and interpreting distortions in hierarchical organization of complex time series. Phys. Rev. E 2015, 91, 030902. [Google Scholar] [CrossRef]
- Gómez-Gómez, J.; Carmona-Cabezas, R.; Ariza-Villaverde, A.B.; Gutiérrez de Ravé, E.; Jiménez-Hornero, F.J. Multifractal detrended fluctuation analysis of temperature in Spain (1960–2019). Phys. A 2021, 578, 126118. [Google Scholar] [CrossRef]
- Gomes, L.F.; Gomes, T.F.; Rempel, E.L.; Gama, S. Origin of multifractality in solar wind turbulence: The role of current sheets. Mon. Not. R. Astron. Soc. 2023, 519, 3623–3634. [Google Scholar] [CrossRef]
- Kwapień, J.; Watorek, M.; Bezbradica, M.; Crane, M.; Mai, T.T.; Drożdż, S. Analysis of inter-transaction time fluctuations in the cryptocurrency market. Chaos 2022, 32, 083142. [Google Scholar] [CrossRef] [PubMed]
- Kwapień, J.; Blasiak, P.; Drożdż, S.; Oświęcimka, P. Genuine multifractality in time series is due to temporal correlations. Phys. Rev. E 2023, 107, 034139. [Google Scholar] [CrossRef] [PubMed]
- Drożdż, S.; Kwapień, J.; Oświęcimka, P.; Rak, R. Quantitative features of multifractal subtleties in time series. EPL 2009, 88, 60003. [Google Scholar] [CrossRef]
- Kluszczyński, R.; Drożdż, S.; Kwapień, J.; Stanisz, T.; Wątorek, M. Disentangling sources of multifractality in time series. Mathematics 2025, 13, 205. [Google Scholar] [CrossRef]
- Umarov, S.; Tsallis, C.; Steinberg, S. On a q-Central Limit Theorem Consistent with Nonextensive Statistical Mechanics. Milan J. Math. 2008, 76, 307–328. [Google Scholar] [CrossRef]
- Tsallis, C.; Levy, S.V.F.; Souza, A.M.C.; Maynard, R. Statistical-Mechanical Foundation of the Ubiquity of Lévy Distributions in Nature. Phys. Rev. Lett. 1995, 75, 3589–3593. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dec, J.; Dolina, M.; Drożdż, S.; Kluszczyński, R.; Kwapień, J.; Stanisz, T. Exploring Word-Adjacency Networks with Multifractal Time Series Analysis Techniques. Entropy 2025, 27, 356. https://doi.org/10.3390/e27040356
Dec J, Dolina M, Drożdż S, Kluszczyński R, Kwapień J, Stanisz T. Exploring Word-Adjacency Networks with Multifractal Time Series Analysis Techniques. Entropy. 2025; 27(4):356. https://doi.org/10.3390/e27040356
Chicago/Turabian StyleDec, Jakub, Michał Dolina, Stanisław Drożdż, Robert Kluszczyński, Jarosław Kwapień, and Tomasz Stanisz. 2025. "Exploring Word-Adjacency Networks with Multifractal Time Series Analysis Techniques" Entropy 27, no. 4: 356. https://doi.org/10.3390/e27040356
APA StyleDec, J., Dolina, M., Drożdż, S., Kluszczyński, R., Kwapień, J., & Stanisz, T. (2025). Exploring Word-Adjacency Networks with Multifractal Time Series Analysis Techniques. Entropy, 27(4), 356. https://doi.org/10.3390/e27040356








