A Differential-Geometric Approach to Quantum Ignorance Consistent with Entropic Properties of Statistical Mechanics
Abstract
1. Introduction
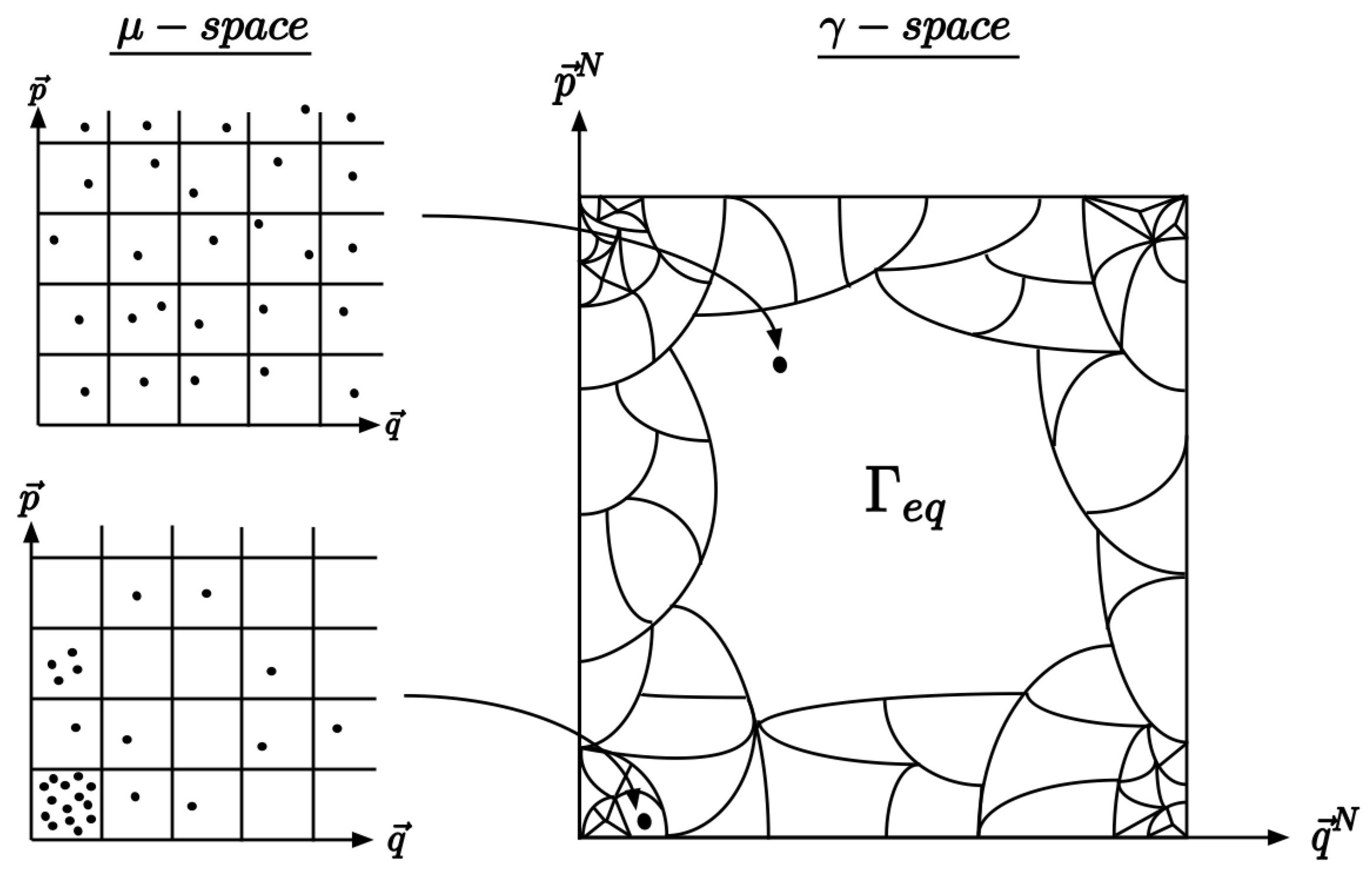
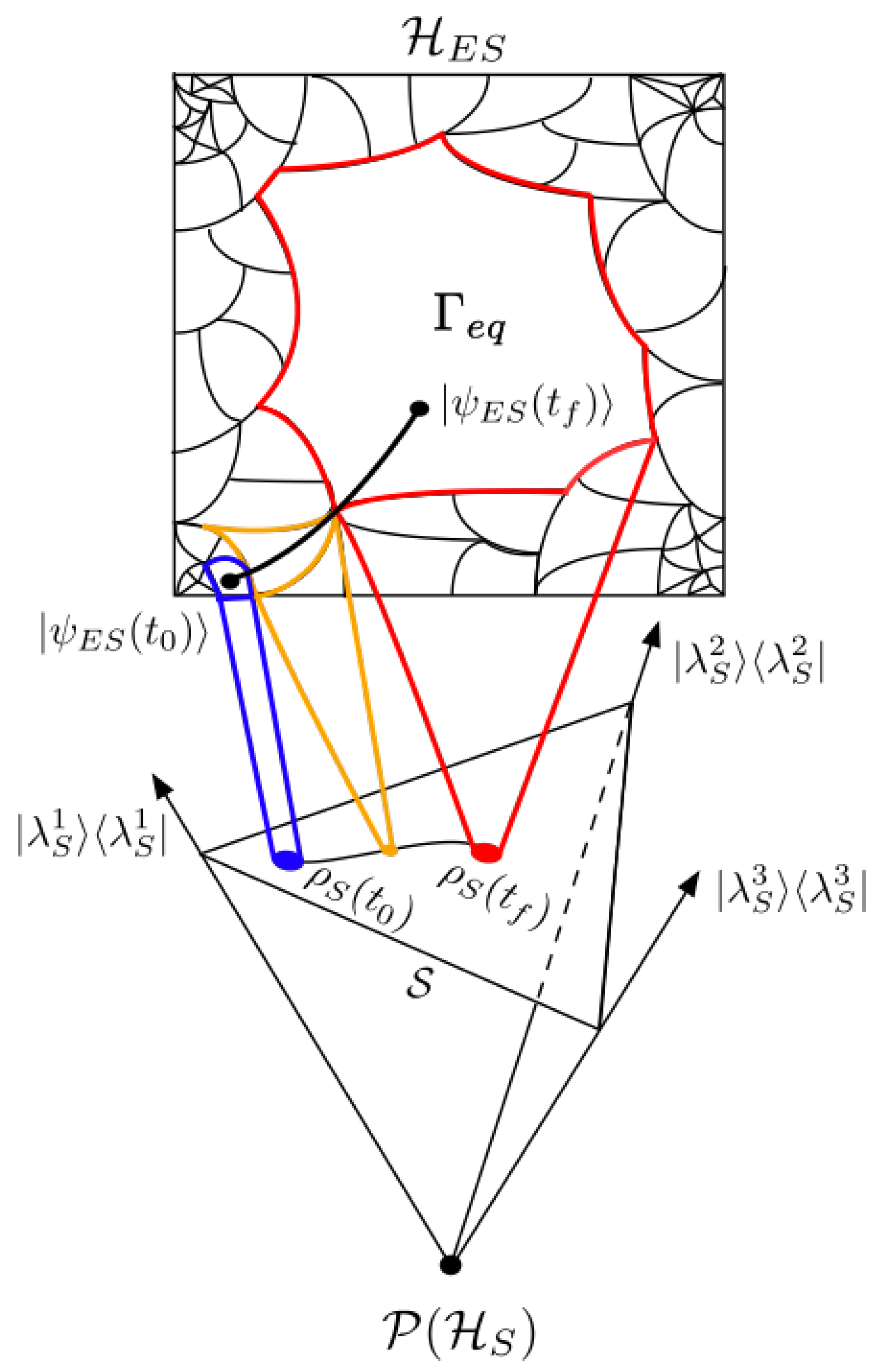
2. Methods: Entanglement Coarse-Graining and the Surfaces of Ignorance
2.1. Macro and Microstates
2.2. Surfaces of Ignorance: Metric Components and Volume
3. Results: Volume Examples
3.1. Arbitrary N-Dimensional Unitary Transformations
3.2. Example:
3.3. Example:
3.3.1. Computing Volume
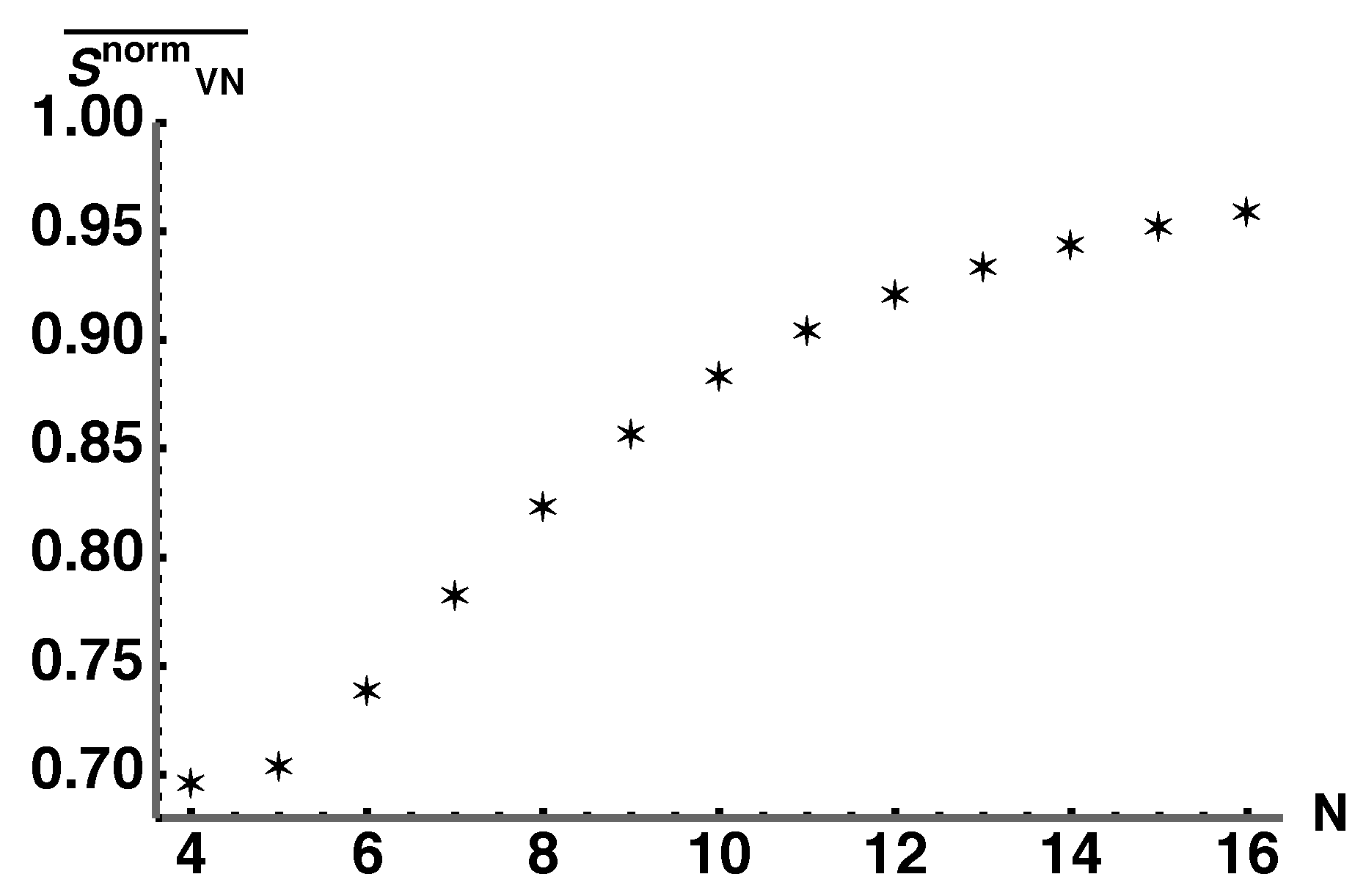
3.3.2. Analyzing the Entanglement Entropy of Macrostates
3.4. Example:
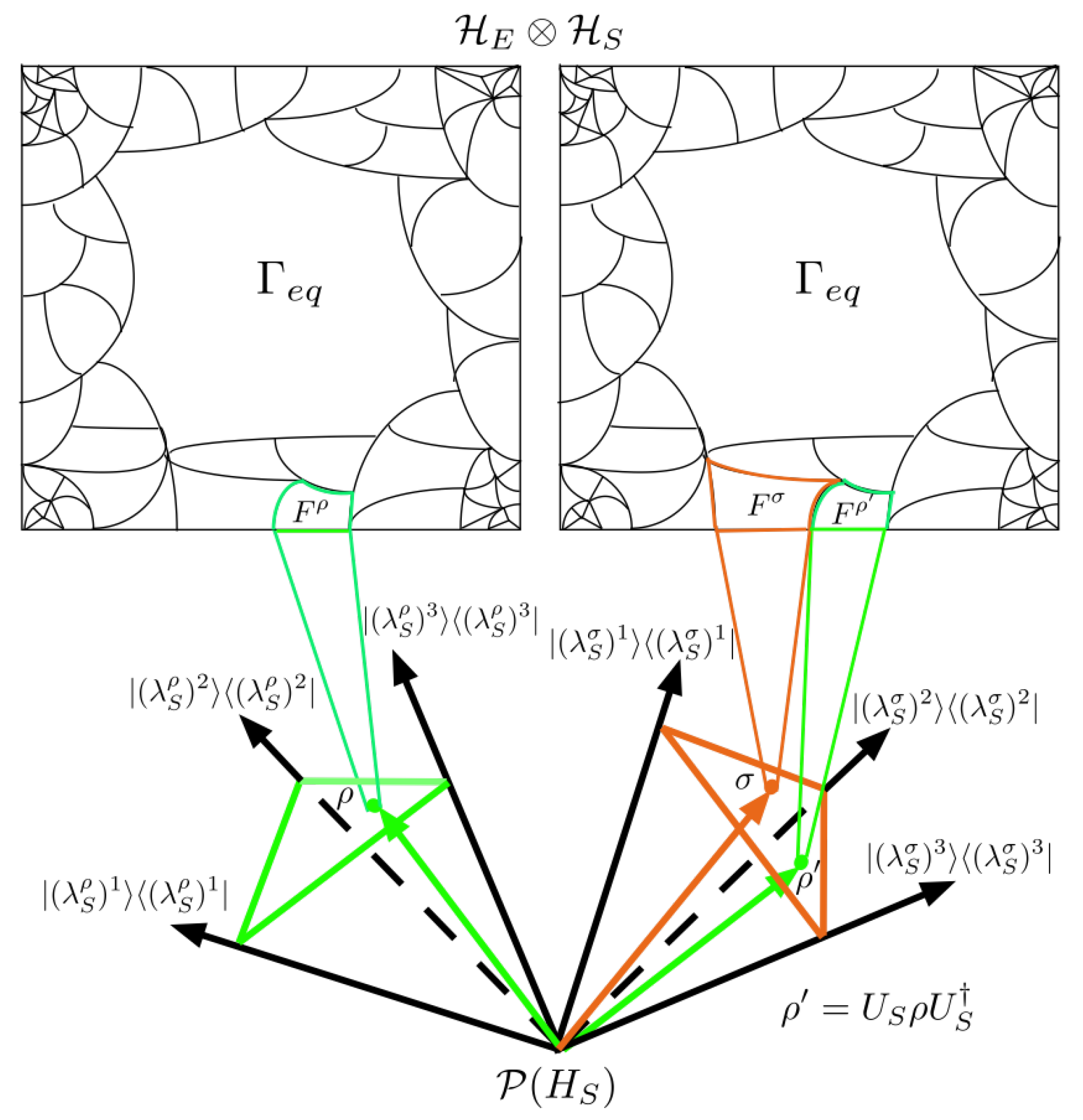
4. Generalizing the Entanglement Coarse-Graining
5. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| CG | Coarse-graining |
| ECG | Entanglement coarse-graining |
| SOI | Surfaces of ignorance |
| S | System |
| E | Environment |
References
- Wilde, M. Quantum Information Theory; Cambridge University Press: Cambridge, UK, 2013. [Google Scholar]
- Deutsch, J. Thermodynamic entropy of a many-body energy eigenstate. New J. Phys. 2010, 12, 075021. [Google Scholar] [CrossRef]
- Santos, L.F.; Polkovnikov, A.; Rigol, M. Weak and strong typicality in quantum systems. Phys. Rev. E 2012, 86, 010102. [Google Scholar] [CrossRef] [PubMed]
- Deutsch, J.; Li, H.; Sharma, A. Microscopic origin of thermodynamic entropy in isolated systems. Phys. Rev. E 2013, 87, 042135. [Google Scholar] [CrossRef]
- Kaufman, A.M.; Tai, E.M.; Lukin, A.; Rispoli, M.; Schittko, R.; Preiss, P.M.; Greiner, M. Quantum thermalization through entanglement in an isolated many-body system. Science 2016, 353, 6301. [Google Scholar] [CrossRef] [PubMed]
- Safranek, D.; Deutsch, J.; Aguirre, A. Quantum coarse-graining entropy and thermalization in closed systems. Phys. Rev. A 2019, 99, 012103. [Google Scholar] [CrossRef]
- Duarte, C.; Carvalho, G.D.; Bernardes, N.K.; Melo, F.d. Emerging dynamics arising from coarse-grained quantum systems. Phys. Rev. A 2017, 96, 032113. [Google Scholar] [CrossRef]
- Kabernik, O. Quantum coarse graining, symmetries, and reducibility of dynamics. Phys. Rev. A 2018, 97, 052130. [Google Scholar] [CrossRef]
- Correia, P.S.; Obando, P.C.; Vallejos, O.R.; de Melo, F. Macro-to-micro quantum mapping and the emergence of nonlinearity. Phys. Rev. A 2021, 103, 052210. [Google Scholar] [CrossRef]
- Correia, P.S.; de Melo, F. Spin-entanglement wave in a coarse-grained optical lattice. Phys. Rev. A 2019, 100, 022334. [Google Scholar] [CrossRef]
- Carvalho, G.D.; Correia, P.S. Decay of quantumness in a measurement process: Action of a coarse-graining channel. Phys. Rev. A 2020, 102, 032217. [Google Scholar] [CrossRef]
- Pineda, C.; Davalos, D.; Viviescas, C.; Rosado, A. Fuzzy measurement and coarse graining in quantum many-body systems. Phys. Rev. A 2021, 104, 042218. [Google Scholar] [CrossRef]
- Uffink, J. Boltzmann’s Work in Statistical Physics. In The Stanford Encyclopedia of Philosophy; Spring 2017 ed.; Zalta, E.N., Ed.; Metaphysics Research Lab, Stanford University: Stanford, CA, USA, 2017. [Google Scholar]
- Goldstein, S.; Huse, D.A.; Lebowitz, J.L.; Tumulka, R. Macroscopic and microscopic thermal equilibrium. Ann. Phys. 2017, 529, 1600301. [Google Scholar] [CrossRef]
- Goldstein, S. Boltzmann’s Approach to Statistical Mechanics; Springer: Berlin/Heidelberg, Germany, 2001; pp. 39–54. [Google Scholar]
- Goldstein, S.; Lebowitz, J.L.; Tumulka, R.; Zanghi, N. Gibbs and Boltzmann Entropy in Classical and Quantum Mechanics; World Scientific Publishing Co.: Singapore, 2020; p. 519. [Google Scholar]
- Goldstein, S.; Lebowitz, J.L.; Tumulka, R.; Nino, Z. Canonical Typicality. Phys. Rev. Lett. 2006, 96, 050403. [Google Scholar] [CrossRef] [PubMed]
- Goldstein, S.; Tumulka, R. On the approach to thermal equilibrium of macroscopic quantum systems. AIP Conf. Proc. 2011, 1332, 155. [Google Scholar]
- Tasaki, H. Typicality of thermal equilibrium and thermalization in isolated macroscopic quantum systems. J. Stat. Phys. 2016, 163, 937–997. [Google Scholar] [CrossRef]
- Popescu, S.; Short, A.J.; Winter, A. Entanglement and the foundations of statistical mechanics. Nat. Phys. 2006, 2, 754–758. [Google Scholar] [CrossRef]
- Boltzmann, L. Vorlesungen über Gastheorie. Leipzi: Barth (Part I 1896, Part II 1898); University of California Press: Berkeley, CA, USA, 1964. [Google Scholar]
- Landford, O.E. Entropy and Equilibrium States in Classical Statistical Mechanics; Springer-Verlag: Berlin/Heidelberg, Germany, 1973; pp. 1–113. [Google Scholar]
- Maruyama, K.; Nori, F.; Vedral, V. Colloquium: The physics of Maxwell’s demon and information. Rev. Mod. Phys. 2009, 81, 1. [Google Scholar] [CrossRef]
- Brillouin, L. Maxwell’s demon cannot operate: Information and entropy: I. J. App. Phys. 1951, 22, 334. [Google Scholar] [CrossRef]
- Schrödinger, E. What Is Life? The Physical Aspect of the Living Cell; Cambridge University Press: Cambridge, UK, 1944. [Google Scholar]
- Brillouin, L. Science and Information Theory, 2nd ed.; Dover Publications: Mineola, NY, USA, 2013. [Google Scholar]
- Jaynes, E.T. Information theory and statistical mechanics. Phys. Rev. 1957, 106, 620–630. [Google Scholar] [CrossRef]
- Zyczkowski, K.; Kus, M. Random unitary matrices. J. Phys. A Math. Gen. 1994, 27, 4235. [Google Scholar] [CrossRef]
- Zyczkowski, K.; Horodecki, P.; Sanpera, A.; Lewenstein, M. Volume of the set of separable states. Phys. Rev. A 1998, 58, 2. [Google Scholar] [CrossRef]
- Bengtsson, I.; Zyczkowski, K. Geometry of Quantum States: An Introduction to Quantum Entanglement, 2nd ed.; Cambridge University Press: Cambridge, UK, 2017. [Google Scholar]
- Jozsa, R. Fidelity for mixed quantum states. J. Mod. Opt. 1994, 41, 2315–2323. [Google Scholar] [CrossRef]
- Hayden, P.; Leung, D.W.; Winter, A. Aspects of generic entanglement. Commun. Math. Phys. 2006, 265, 95–117. [Google Scholar] [CrossRef]
- Lloyd, S. Black Holes, Demons and the Loss of Coherence: How Complex System Get Information, and What They Do with It; Rockefeller University: New York, NY, USA, 1988; Chapter 3. [Google Scholar]
- Deffner, S.; Zurek, H.W. Foundations of statistical mechanics from symmetries of entanglement. New J. Phys. 2016, 18, 063013. [Google Scholar] [CrossRef]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ray, S.; Alsing, P.M.; Cafaro, C.; Jacinto, H.S. A Differential-Geometric Approach to Quantum Ignorance Consistent with Entropic Properties of Statistical Mechanics. Entropy 2023, 25, 788. https://doi.org/10.3390/e25050788
Ray S, Alsing PM, Cafaro C, Jacinto HS. A Differential-Geometric Approach to Quantum Ignorance Consistent with Entropic Properties of Statistical Mechanics. Entropy. 2023; 25(5):788. https://doi.org/10.3390/e25050788
Chicago/Turabian StyleRay, Shannon, Paul M. Alsing, Carlo Cafaro, and H S. Jacinto. 2023. "A Differential-Geometric Approach to Quantum Ignorance Consistent with Entropic Properties of Statistical Mechanics" Entropy 25, no. 5: 788. https://doi.org/10.3390/e25050788
APA StyleRay, S., Alsing, P. M., Cafaro, C., & Jacinto, H. S. (2023). A Differential-Geometric Approach to Quantum Ignorance Consistent with Entropic Properties of Statistical Mechanics. Entropy, 25(5), 788. https://doi.org/10.3390/e25050788







