Fluctuation Theorem for Information Thermodynamics of Quantum Correlated Systems
Abstract
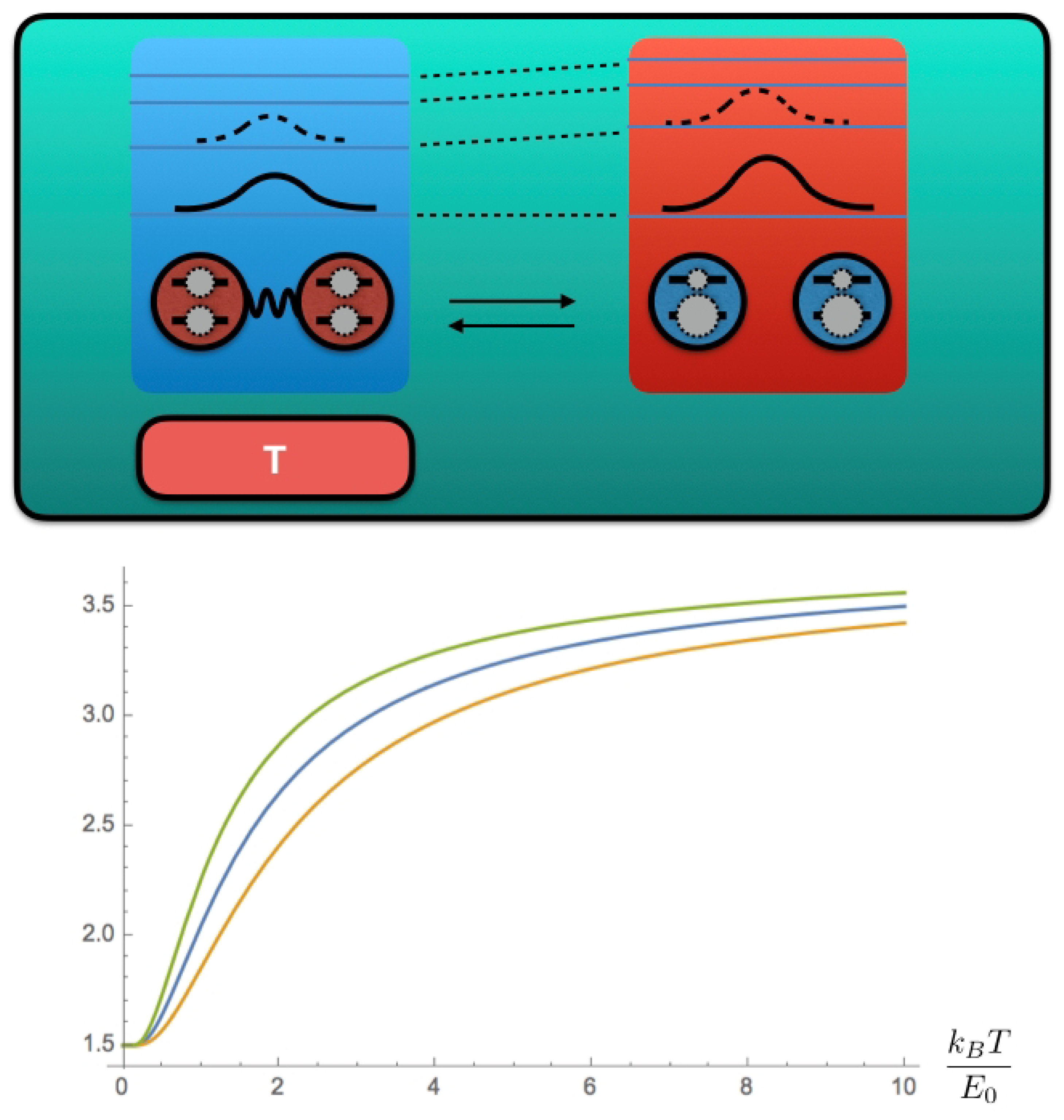
1. Introduction
2. Fluctuation Theorem for Open Bipartite Quantum System
2.1. Deriving the FT in Equation (4)
2.1.1. Detailed FT
2.1.2. Integral FT
2.2. Thermodynamic Inequalities for Heat Transfer and Work
2.2.1. Heat Transfer
2.2.2. Work
2.3. Determining in Experiment
2.3.1. Obtaining and
2.3.2. Obtaining the Transition Probabilities
3. Example
3.1. Local Isothermal Process for Composite Quantum System
3.1.1. Time-Forward Process
3.1.2. Time-Reversed Process
3.2. Heat Transfer
3.3. Work Inequality
4. Summary
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Derivation of Equation (6)
Appendix B. Proof of Equation (11)
Appendix C. Calculation of Relevant Quantities for the Example in Section 3.1
Appendix C.1. Time-Forward Process
Appendix C.2. Time-Reversed Process
References
- Parrondo, J.M.R.; Horowitz, J.M.; Sagawa, T. Thermodynamics of information. Nat. Phys. 2015, 11, 131. [Google Scholar] [CrossRef]
- Goold, J.; Huber, M.; Riera, A.; del Rio, L.; Skrzypczyk, P. The role of quantum information in thermodynamics—A topical review. J. Phys. A Math. Theor. 2016, 49, 143001. [Google Scholar] [CrossRef]
- Talkner, P.; Hänggi, P. Colloquium: Statistical mechanics and thermodynamics at strong coupling: Quantum and classical. Rev. Mod. Phys. 2020, 92, 041002. [Google Scholar] [CrossRef]
- Gemmer, J.; Michel, M.; Mahler, G. Quantum Thermodynamics; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Evans, D.; Cohen, E.; Morriss, G. Probability of second law violations in shearing steady states. Phys. Rev. Lett. 1993, 71, 2401–2404. [Google Scholar] [CrossRef]
- Gallavotti, G.; Cohen, E. Dynamical Ensembles in Nonequilibrium Statistical Mechanics. Phys. Rev. Lett. 1995, 74, 2694–2697. [Google Scholar] [CrossRef]
- Jarzynski, C. Nonequilibrium Equality for Free Energy Differences. Phys. Rev. Lett. 1997, 78, 2690. [Google Scholar] [CrossRef]
- Crooks, G. Entropy production fluctuation theorem and the nonequilibrium work relation for free energy differences. Phys. Rev. E 1999, 60, 2721. [Google Scholar] [CrossRef]
- Tasaki, H. Jarzynski Relations for Quantum Systems and Some Applications. arXiv 2000, arXiv:cond-mat/0009244. [Google Scholar]
- Kurchan, J. A Quantum Fluctuation Theorem. arXiv 2000, arXiv:cond-mat/0007360. [Google Scholar]
- Seifert, U. Entropy Production along a Stochastic Trajectory and an Integral Fluctuation Theorem. Phys. Rev. Lett. 2005, 95, 040602. [Google Scholar] [CrossRef]
- Talkner, P.; Hänggi, P. The Tasaki–Crooks quantum fluctuation theorem. J. Phys. Math. Theor. 2007, 40, F569. [Google Scholar] [CrossRef]
- Crooks, G. On the Jarzynski relation for dissipative quantum dynamics. J. Stat. Mech. Theory Exp. 2008, 2008, P10023. [Google Scholar] [CrossRef]
- Esposito, M.; Harbola, U.; Mukamel, S. Nonequilibrium fluctuations, fluctuation theorems, and counting statistics in quantum systems. Rev. Mod. Phys. 2009, 81, 1665. [Google Scholar] [CrossRef]
- Campisi, M.; Talkner, P.; Hänggi, P. Fluctuation Theorem for Arbitrary Open Quantum Systems. Phys. Rev. Lett. 2009, 102, 210401. [Google Scholar] [CrossRef]
- Campisi, M.; Talkner, P.; Hänggi, P. Fluctuation Theorems for Continuously Monitored Quantum Fluxes. Phys. Rev. Lett. 2010, 105, 140601. [Google Scholar] [CrossRef]
- Campisi, M.; Hänggi, P.; Talkner, P. Colloquium: Quantum fluctuation relations: Foundations and applications. Rev. Mod. Phys. 2011, 83, 771–791. [Google Scholar] [CrossRef]
- Vedral, V. An information-theoretic equality implying the Jarzynski relation. J. Phys. A Math. Theor. 2012, 45, 272001. [Google Scholar] [CrossRef]
- Aberg, J. Fully Quantum Fluctuation Theorems. Phys. Rev. X 2018, 8, 011019. [Google Scholar]
- Alhambra, Á.; Masanes, L.; Oppenheim, J.; Perry, C. Fluctuating Work: From Quantum Thermodynamical Identities to a Second Law Equality. Phys. Rev. X 2016, 6, 041017. [Google Scholar] [CrossRef]
- Allahverdyan, A. Nonequilibrium quantum fluctuations of work. Phys. Rev. E 2014, 90, 032137. [Google Scholar] [CrossRef]
- Talkner, P.; Hänggi, P. Aspects of quantum work. Phys. Rev. E 2016, 93, 022131. [Google Scholar] [CrossRef]
- Dahlsten, O.; Choi, M.; Braun, D.; Garner, A.; Halpern, N.; Vedral, V. Entropic equality for worst-case work at any protocol speed. New J. Phys. 2017, 19, 043013. [Google Scholar] [CrossRef]
- Quan, H.; Dong, H. Quantum Crooks fluctuation theorem and quantum Jarzynski equality in the presence of a reservoir. arXiv 2008, arXiv:0812.4955. [Google Scholar]
- Gong, Z.; Quan, H. Jarzynski equality, Crooks fluctuation theorem, and the fluctuation theorems of heat for arbitrary initial states. Phys. Rev. E 2015, 92, 012131. [Google Scholar] [CrossRef]
- Chetrite, R.; Mallick, K. Quantum Fluctuation Relations for the Lindblad Master Equation. J. Stat. Phys. 2012, 148, 480–501. [Google Scholar] [CrossRef]
- Crooks, G. Quantum operation time reversal. Phys. Rev. A 2008, 77, 034101. [Google Scholar] [CrossRef]
- Kwon, H.; Kim, M.S. Fluctuation Theorems for a Quantum Channel. Phys. Rev. X 2019, 9, 031029. [Google Scholar] [CrossRef]
- Holmes, Z.; Weidt, S.; Jennings, D.; Anders, J.; Mintert, F. Coherent fluctuation relations: From the abstract to the concrete. Quantum 2019, 3, 124. [Google Scholar] [CrossRef]
- Lostaglio, M.; Jennings, D.; Rudolph, T. Description of quantum coherence in thermodynamic processes requires constraints beyond free energy. Nat. Commun. 2015, 6, 6383. [Google Scholar] [CrossRef]
- Morris, B.; Adesso, G. Quantum coherence fluctuation relations. J. Phys. A Math. Theor. 2018, 51, 414007. [Google Scholar] [CrossRef]
- Manzano, G.; Horowitz, J.; Parrondo, J. Nonequilibrium potential and fluctuation theorems for quantum maps. Phys. Rev. E 2015, 92, 032129. [Google Scholar] [CrossRef]
- Esposito, M.; Lindenberg, K.; Broeck, C. Entropy production as correlation between system and reservoir. New J. Phys. 2010, 12, 013013. [Google Scholar] [CrossRef]
- Koski, J.; Maisi, V.; Sagawa, T.; Pekola, J. Experimental Observation of the Role of Mutual Information in the Nonequilibrium Dynamics of a Maxwell Demon. Phys. Rev. Lett. 2014, 113, 030601. [Google Scholar] [CrossRef]
- Chapman, A.; Miyake, A. How an autonomous quantum Maxwell demon can harness correlated information. Phys. Rev. E 2015, 92, 062125. [Google Scholar] [CrossRef]
- Deffner, S.; Jarzynski, C. Information Processing and the Second Law of Thermodynamics: An Inclusive, Hamiltonian Approach. Phys. Rev. X 2013, 3, 041003. [Google Scholar] [CrossRef]
- Horowitz, J. Multipartite information flow for multiple Maxwell demons. J. Stat. Mech. Theory Exp. 2015, 2015, P03006. [Google Scholar] [CrossRef]
- Horowitz, J.; Vaikuntanathan, S. Nonequilibrium detailed fluctuation theorem for repeated discrete feedback. Phys. Rev. E 2010, 82, 061120. [Google Scholar] [CrossRef]
- Strasberg, P.; Schaller, G.; Brandes, T.; Esposito, M. Quantum and Information Thermodynamics: A Unifying Framework Based on Repeated Interactions. Phys. Rev. X 2017, 7, 021003. [Google Scholar] [CrossRef]
- Strasberg, P.; Schaller, G.; Brandes, T.; Jarzynski, C. Second laws for an information driven current through a spin valve. Phys. Rev. E 2014, 90, 062107. [Google Scholar] [CrossRef]
- Horowitz, J.; Sandberg, H. Second-law-like inequalities with information and their interpretations. New J. Phys. 2014, 16, 125007. [Google Scholar] [CrossRef]
- Seifert, U. Stochastic thermodynamics, fluctuation theorems and molecular machines. Rep. Prog. Phys. 2012, 75, 126001. [Google Scholar] [CrossRef]
- Hartich, D.; Barato, A.; Seifert, U. Stochastic thermodynamics of bipartite systems: Transfer entropy inequalities and a Maxwell’s demon interpretation. J. Stat. Mech. Theory Exp. 2014, 2014, P02016. [Google Scholar] [CrossRef]
- Strasberg, P.; Schaller, G.; Brandes, T.; Esposito, M. Thermodynamics of a Physical Model Implementing a Maxwell Demon. Phys. Rev. Lett. 2013, 110, 040601. [Google Scholar] [CrossRef]
- Abreu, D.; Seifert, U. Thermodynamics of Genuine Nonequilibrium States under Feedback Control. Phys. Rev. Lett. 2012, 108, 030601. [Google Scholar] [CrossRef]
- Ptaszyński, K.; Esposito, M. Thermodynamics of Quantum Information Flows. Phys. Rev. Lett. 2019, 122, 150603. [Google Scholar] [CrossRef]
- Horowitz, J.; Esposito, M. Thermodynamics with Continuous Information Flow. Phys. Rev. X 2014, 4, 031015. [Google Scholar] [CrossRef]
- Esposito, M.; Broeck, C. Three Detailed Fluctuation Theorems. Phys. Rev. Lett. 2010, 104, 090601. [Google Scholar] [CrossRef]
- Barato, A.; Seifert, U. Unifying Three Perspectives on Information Processing in Stochastic Thermodynamics. Phys. Rev. Lett. 2014, 112, 090601. [Google Scholar] [CrossRef]
- Iyoda, E.; Kaneko, K.; Sagawa, T. Fluctuation Theorem for Many-Body Pure Quantum States. Phys. Rev. Lett. 2017, 119, 100601. [Google Scholar] [CrossRef]
- Szilard, L. Über die Entropieverminderung in einem thermodynamischen System bei Eingriffen intelligenter Wesen. Z. Phys. 1929, 53, 840–856. [Google Scholar] [CrossRef]
- Leff, H.; Rex, A. Maxwell’s Demon 2 Entropy, Classical and Quantum Information, Computing; CRC Press: Boca Raton, FL, USA, 2002. [Google Scholar]
- Sagawa, T.; Ueda, M. Fluctuation Theorem with Information Exchange: Role of Correlations in Stochastic Thermodynamics. Phys. Rev. Lett. 2012, 109, 180602. [Google Scholar] [CrossRef] [PubMed]
- Sagawa, T.; Ueda, M. Generalized Jarzynski Equality under Nonequilibrium Feedback Control. Phys. Rev. Lett. 2010, 104, 090602. [Google Scholar] [CrossRef] [PubMed]
- Funo, K.; Watanabe, Y.; Ueda, M. Integral quantum fluctuation theorems under measurement and feedback control. Phys. Rev. E 2013, 88, 052121. [Google Scholar] [CrossRef] [PubMed]
- Jevtic, S.; Rudolph, T.; Jennings, D.; Hirono, Y.; Nakayama, S.; Murao, M. Exchange fluctuation theorem for correlated quantum systems. Phys. Rev. E 2015, 92, 042113. [Google Scholar] [CrossRef] [PubMed]
- Manzano, G.; Horowitz, J.; Parrondo, J. Quantum Fluctuation Theorems for Arbitrary Environments: Adiabatic and Nonadiabatic Entropy Production. Phys. Rev. X 2018, 8, 031037. [Google Scholar] [CrossRef]
- Park, J.J.; Nha, H.; Kim, S.W.; Vedral, V. Information fluctuation theorem for an open quantum bipartite system. Phys. Rev. E 2020, 101, 052128. [Google Scholar] [CrossRef]
- Morikuni, Y.; Tasaki, H. Quantum Jarzynski-Sagawa-Ueda Relations. J. Stat. Phys. 2011, 143, 1–10. [Google Scholar] [CrossRef]
- Sagawa, T.; Ueda, M. Role of mutual information in entropy production under information exchanges. New J. Phys. 2013, 15, 125012. [Google Scholar] [CrossRef]
- Kwon, C.; Um, J.; Park, H. Information thermodynamics for a multi-feedback process with time delay. EPL Europhys. Lett. 2017, 117, 10011. [Google Scholar] [CrossRef]
- Ponmurugan, M. Generalized detailed fluctuation theorem under nonequilibrium feedback control. Phys. Rev. E 2010, 82, 031129. [Google Scholar] [CrossRef]
- Talkner, P.; Lutz, E.; Hänggi, P. Fluctuation theorems: Work is not an observable. Phys. Rev. E 2007, 75, 050102. [Google Scholar] [CrossRef] [PubMed]
- Kirkwood, J. Quantum Statistics of Almost Classical Assemblies. Phys. Rev. 1933, 44, 31–37. [Google Scholar] [CrossRef]
- Dirac, P. On the Analogy Between Classical and Quantum Mechanics. Rev. Mod. Phys. 1945, 17, 195–199. [Google Scholar] [CrossRef]
- Barut, A. Distribution Functions for Noncommuting Operators. Phys. Rev. 1957, 108, 565–569. [Google Scholar] [CrossRef]
- Margenau, H.; Hill, R. Correlation between Measurements in Quantum Theory. Prog. Theor. Phys. 1961, 26, 722–738. [Google Scholar] [CrossRef]
- Modi, K.; Paterek, T.; Son, W.; Vedral, V.; Williamson, M. Unified View of Quantum and Classical Correlations. Phys. Rev. Lett. 2010, 104, 080501. [Google Scholar] [CrossRef]
- Nation, P.; Johansson, J.; Blencowe, M.; Nori, F. Colloquium: Stimulating uncertainty: Amplifying the quantum vacuum with superconducting circuits. Rev. Mod. Phys. 2012, 84, 1–24. [Google Scholar] [CrossRef]
- Funo, K.; Murashita, Y.; Ueda, M. Quantum nonequilibrium equalities with absolute irreversibility. New J. Phys. 2015, 17, 075005. [Google Scholar] [CrossRef]
- Becker, T.; Schnell, A.; Thingna, J. Canonically Consistent Quantum Master Equation. Phys. Rev. Lett. 2022, 129, 200403. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Park, J.J.; Nha, H. Fluctuation Theorem for Information Thermodynamics of Quantum Correlated Systems. Entropy 2023, 25, 165. https://doi.org/10.3390/e25010165
Park JJ, Nha H. Fluctuation Theorem for Information Thermodynamics of Quantum Correlated Systems. Entropy. 2023; 25(1):165. https://doi.org/10.3390/e25010165
Chicago/Turabian StylePark, Jung Jun, and Hyunchul Nha. 2023. "Fluctuation Theorem for Information Thermodynamics of Quantum Correlated Systems" Entropy 25, no. 1: 165. https://doi.org/10.3390/e25010165
APA StylePark, J. J., & Nha, H. (2023). Fluctuation Theorem for Information Thermodynamics of Quantum Correlated Systems. Entropy, 25(1), 165. https://doi.org/10.3390/e25010165





