Multipartite Correlations in Quantum Collision Models
Abstract
:1. Introduction
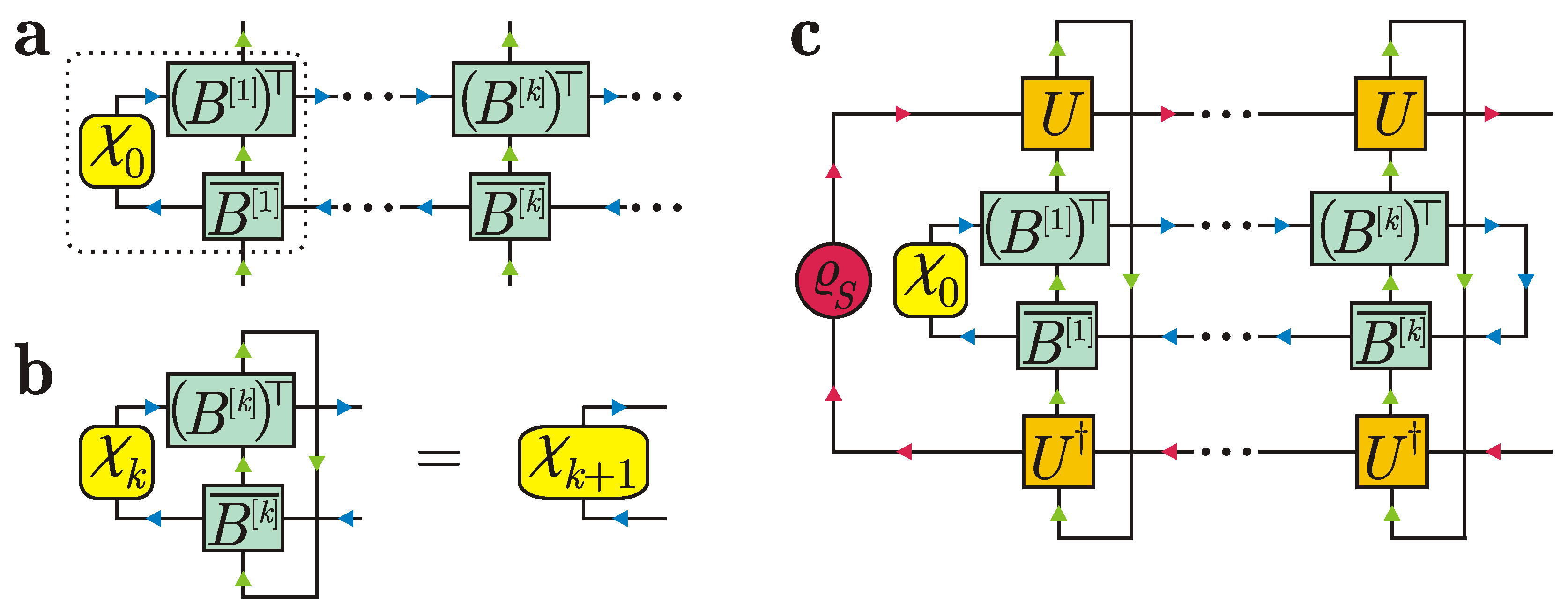
2. Tensor Network Notation
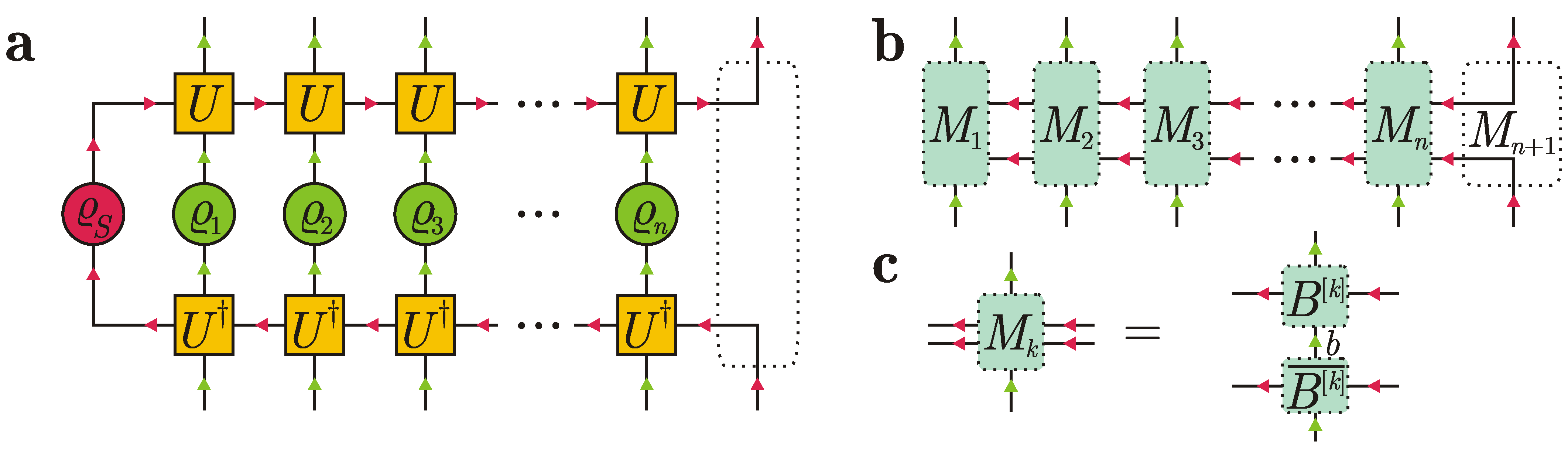
3. Matrix Product State Correlations in the Standard Collision Model
4. Generalization to Mixed States of the System and Ancillas
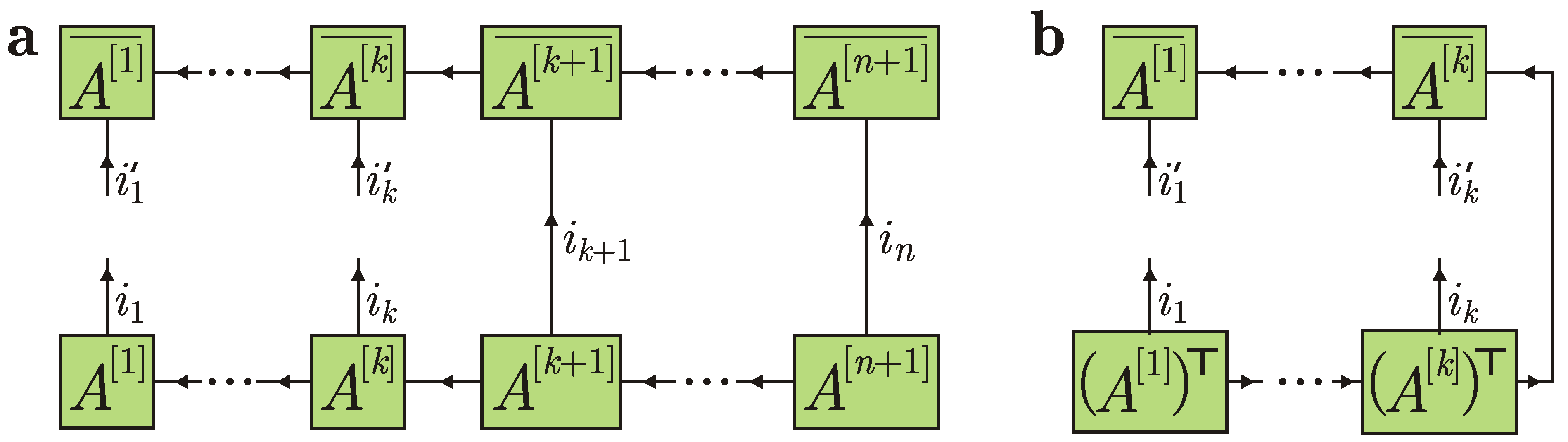
5. Collision Model with a Generally Correlated State of Ancillas
6. Master Equation
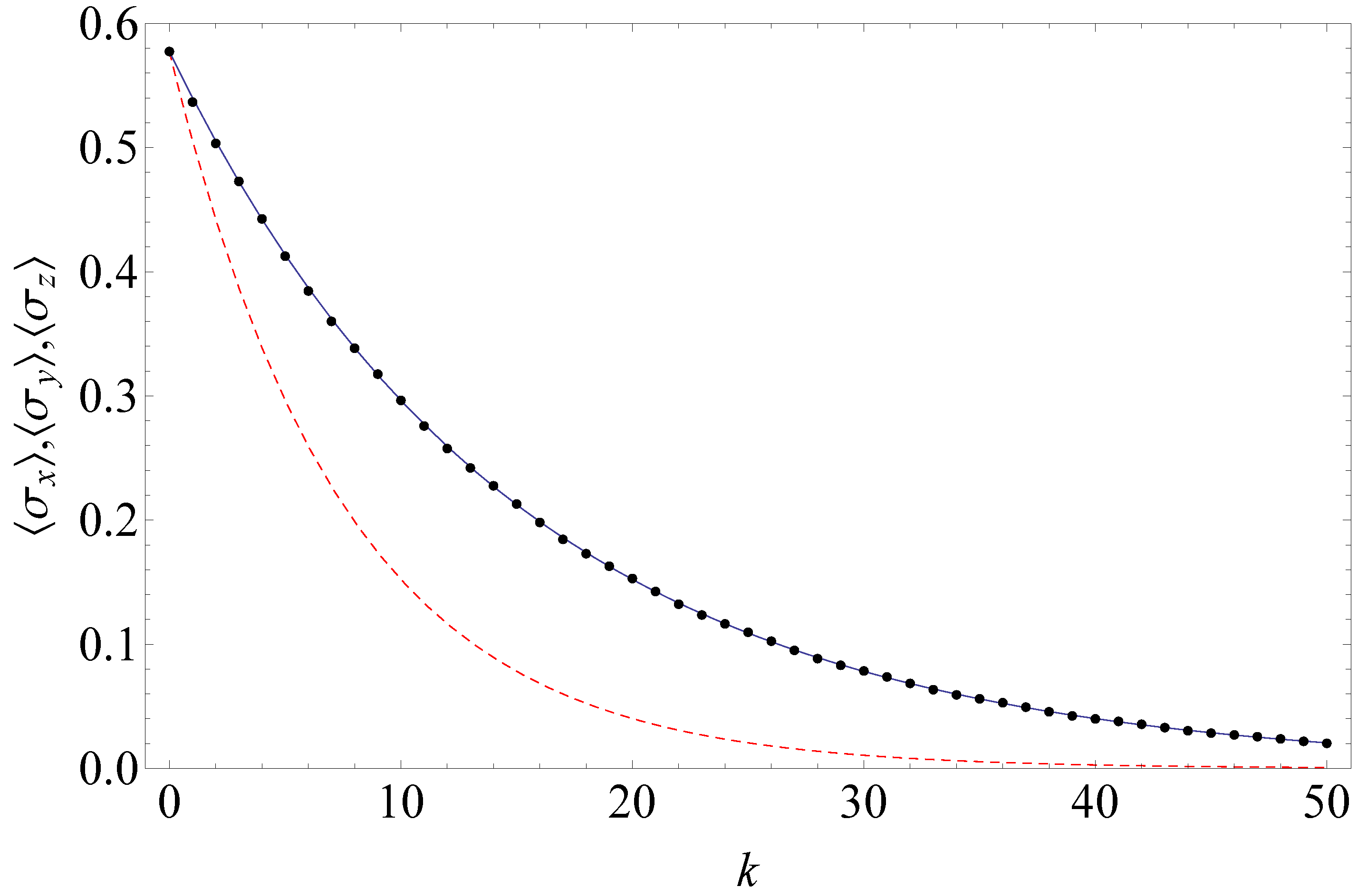
7. Effect of Two-Point Correlations
8. Stroboscopic Limit
9. Effect of Multipoint Correlations in the Higher-Order Stroboscopic Limit
10. Conclusions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rau, J. Relaxation phenomena in spin and harmonic oscillator systems. Phys. Rev. 1963, 129, 1880–1888. [Google Scholar] [CrossRef]
- Nachtergaele, B.; Vershynina, A.; Zagrebnov, V.A. Non-Equilibrium states of a photon cavity pumped by an atomic beam. Ann. Henri Poincaré 2008, 15, 213–262. [Google Scholar] [CrossRef] [Green Version]
- Scarani, V.; Ziman, M.; Štelmachovič, P.; Gisin, N.; Bužek, V. Thermalizing quantum machines: Dissipation and entanglement. Phys. Rev. Lett. 2002, 88, 097905. [Google Scholar] [CrossRef] [Green Version]
- Ziman, M.; Štelmachovič, P.; Bužek, V. Description of quantum dynamics of open systems based on collision-like models. Open Syst. Inf. Dyn. 2005, 12, 81–91. [Google Scholar] [CrossRef] [Green Version]
- Ziman, M.; Bužek, V. All (qubit) decoherences: Complete characterization and physical implementation. Phys. Rev. A 2005, 72, 022110. [Google Scholar] [CrossRef] [Green Version]
- Grimmer, D.; Layden, D.; Mann, R.B.; Martın-Martınez, E. Open dynamics under rapid repeated interaction. Phys. Rev. A 2016, 94, 032126. [Google Scholar] [CrossRef] [Green Version]
- Ziman, M.; Štelmachovič, P.; Bužek, V.; Hillery, M.; Scarani, V.; Gisin, N. Diluting quantum information: An analysis of information transfer in system-reservoir interactions. Phys. Rev. A 2002, 65, 042105. [Google Scholar] [CrossRef] [Green Version]
- Ziman, M.; Bužek, V. Open system dynamics of simple collision models. In Quantum Dynamics and Information; Olkiewicz, R., Cegła, W., Frydryszak, A., Garbaczewski, P., Jakóbczyk, L., Eds.; World Scientific: Singapore, 2011; pp. 199–227. [Google Scholar]
- Karevski, D.; Platini, T. Quantum nonequilibrium steady states induced by repeated interactions. Phys. Rev. Lett. 2009, 102, 207207. [Google Scholar] [CrossRef] [Green Version]
- Román-Ancheyta, R.; Kolář, M.; Guarnieri, G.; Filip, R. Enhanced steady-state coherence via repeated system-bath interactions. Phys. Rev. A 2021, 104, 062209. [Google Scholar] [CrossRef]
- Heineken, D.; Beyer, K.; Luoma, K.; Strunz, W.T. Quantum-memory-enhanced dissipative entanglement creation in nonequilibrium steady states. Phys. Rev. A 2021, 104, 052426. [Google Scholar] [CrossRef]
- Daryanoosh, S.; Baragiola, B.Q.; Guff, T.; Gilchrist, A. Quantum master equations for entangled qubit environments. Phys. Rev. A 2018, 98, 062104. [Google Scholar] [CrossRef] [Green Version]
- Çakmak, B.; Campbell, S.; Vacchini, B.; Müstecaplıoğlu, Ö.E.; Paternostro, M. Robust multipartite entanglement generation via a collision model. Phys. Rev. A 2019, 99, 012319. [Google Scholar] [CrossRef] [Green Version]
- Attal, S.; Pautrat, Y. From repeated to continuous quantum interactions. Ann. Henri Poincaré 2006, 7, 59. [Google Scholar] [CrossRef] [Green Version]
- Attal, S.; Joye, A. Weak coupling and continuous limits for repeated quantum interactions. J. Stat. Phys. 2007, 126, 1241–1283. [Google Scholar] [CrossRef] [Green Version]
- Vargas, R. Repeated interaction quantum systems: Van Hove limits and asymptotic states. J. Stat. Phys. 2008, 133, 491–511. [Google Scholar] [CrossRef] [Green Version]
- Li, L.; Hall, M.J.W.; Wiseman, H.M. Concepts of quantum non-Markovianity: A hierarchy. Phys. Rep. 2018, 759, 1. [Google Scholar] [CrossRef] [Green Version]
- Attal, S.; Petruccione, F.; Sinayskiy, I. Open quantum walks on graphs. Phys. Lett. A 2012, 376, 1545. [Google Scholar] [CrossRef] [Green Version]
- Attal, S.; Petruccione, F.; Sabot, C.; Sinayskiy, I. Open quantum random walks. J. Stat. Phys. 2012, 147, 832. [Google Scholar] [CrossRef] [Green Version]
- Pellegrini, C. Continuous time open quantum random walks and non-Markovian Lindblad master equations. J. Stat. Phys. 2014, 154, 838–865. [Google Scholar] [CrossRef]
- Sinayskiy, I.; Petruccione, F. Microscopic derivation of open quantum walks. Phys. Rev. A 2015, 92, 032105. [Google Scholar] [CrossRef] [Green Version]
- Liu, C.; Balu, R. Steady states of continuous-time open quantum walks. Quantum Inf. Process. 2017, 16, 173. [Google Scholar] [CrossRef]
- Chia, A.; Paterek, T.; Kwek, L.C. Hitting statistics from quantum jumps. Quantum 2017, 1, 19. [Google Scholar] [CrossRef] [Green Version]
- Bruneau, L.; Joye, A.; Merkli, M. Repeated interactions in open quantum systems. J. Math. Phys. 2014, 55, 075204. [Google Scholar] [CrossRef] [Green Version]
- Bruneau, L.; Joye, A.; Merkli, M. Asymptotics of repeated interaction quantum systems. J. Funct. Anal. 2006, 239, 310–344. [Google Scholar] [CrossRef] [Green Version]
- Tamura, H.; Zagrebnov, V.A. Dynamics of an open system for repeated harmonic perturbation. J. Stat. Phys. 2016, 163, 844–867. [Google Scholar] [CrossRef] [Green Version]
- Bruneau, L.; Joye, A.; Merkli, M. Random repeated interaction quantum systems. Commun. Math. Phys. 2008, 284, 553–581. [Google Scholar] [CrossRef] [Green Version]
- Nechita, I.; Pellegrini, C. Random repeated quantum interactions and random invariant states. Probab. Theory Relat. Fields 2012, 152, 299–320. [Google Scholar] [CrossRef] [Green Version]
- Purkayastha, A.; Guarnieri, G.; Campbell, S.; Prior, J.; Goold, J. Periodically refreshed baths to simulate open quantum many-body dynamics. Phys. Rev. B 2021, 104, 045417. [Google Scholar] [CrossRef]
- Cattaneo, M.; De Chiara, G.; Maniscalco, S.; Zambrini, R.; Giorgi, G.L. Collision models can efficiently simulate any multipartite Markovian quantum dynamics. Phys. Rev. Lett. 2021, 126, 130403. [Google Scholar] [CrossRef]
- García-Pérez, G.; Rossi, M.A.C.; Maniscalco, S. IBM Q Experience as a versatile experimental testbed for simulating open quantum systems. NPJ Quantum Inf. 2020, 6, 1. [Google Scholar] [CrossRef] [Green Version]
- Filippov, S.N.; Semin, G.N.; Pechen, A.N. Quantum master equations for a system interacting with a quantum gas in the low-density limit and for the semiclassical collision model. Phys. Rev. A 2020, 101, 012114. [Google Scholar] [CrossRef] [Green Version]
- Kosloff, R. Quantum thermodynamics and open-systems modeling. J. Chem. Phys. 2019, 150, 204105. [Google Scholar] [CrossRef] [PubMed]
- Seah, S.; Nimmrichter, S.; Grimmer, D.; Santos, J.P.; Scarani, V.; Landi, G.T. Collisional quantum thermometry. Phys. Rev. Lett. 2019, 123, 180602. [Google Scholar] [CrossRef] [Green Version]
- Strasberg, P. Repeated interactions and quantum stochastic thermodynamics at strong coupling. Phys. Rev. Lett. 2019, 123, 180604. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Pichler, H.; Zoller, P. Photonic circuits with time delays and quantum feedback. Phys. Rev. Lett. 2016, 116, 093601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Guimond, P.-O.; Pletyukhov, M.; Pichler, H.; Zoller, P. Delayed coherent quantum feedback from a scattering theory and a matrix product state perspective. Quantum Sci. Technol. 2017, 2, 044012. [Google Scholar] [CrossRef] [Green Version]
- Ciccarello, F. Collision models in quantum optics. Quantum Meas. Quantum Metrol. 2017, 4, 53–63. [Google Scholar] [CrossRef] [Green Version]
- Gross, J.A.; Caves, C.M.; Milburn, G.J.; Combes, J. Qubit models of weak continuous measurements: Markovian conditional and open-system dynamics. Quantum Sci. Technol. 2018, 3, 024005. [Google Scholar] [CrossRef] [Green Version]
- Fischer, K.A.; Trivedi, R.; Ramasesh, V.; Siddiqi, I.; Vučković, J. Scattering into one-dimensional waveguides from a coherently-driven quantum-optical system. Quantum 2018, 2, 69. [Google Scholar] [CrossRef]
- Cilluffo, D.; Carollo, A.; Lorenzo, S.; Gross, J.A.; Palma, G.M.; Ciccarello, F. Collisional picture of quantum optics with giant emitters. Phys. Rev. Res. 2020, 2, 043070. [Google Scholar] [CrossRef]
- Carmele, A.; Nemet, N.; Canela, V.; Parkins, S. Pronounced non-Markovian features in multiply excited, multiple emitter waveguide QED: Retardation induced anomalous population trapping. Phys. Rev. Res. 2020, 2, 013238. [Google Scholar] [CrossRef] [Green Version]
- Ferreira, V.S.; Banker, J.; Sipahigil, A.; Matheny, M.H.; Keller, A.J.; Kim, E.; Mirhosseini, M.; Painter, O. Collapse and revival of an artificial atom coupled to a structured photonic reservoir. Phys. Rev. X 2021, 11, 041043. [Google Scholar] [CrossRef]
- Wein, S.C.; Loredo, J.C.; Maffei, M.; Hilaire, P.; Harouri, A.; Somaschi, N.; Lemaître, A.; Sagnes, I.; Lanco, L.; Krebs, O.; et al. Photon-Number Entanglement Generated by Sequential Excitation of a Two-Level Atom. Available online: https://arxiv.org/abs/2106.02049 (accessed on 21 February 2022).
- Maffei, M.; Camati, P.A.; Auffèves, A. Closed-system solution of the 1D atom from collision model. Entropy 2022, 24, 151. [Google Scholar] [CrossRef] [PubMed]
- Gheri, K.M.; Ellinger, K.; Pellizzari, T.; Zoller, P. Photon-wavepackets as flying quantum bits. Fortschr. Phys. 1998, 46, 401–415. [Google Scholar] [CrossRef]
- Baragiola, B.Q.; Cook, R.L.; Brańczyk, A.M.; Combes, J. N-photon wave packets interacting with an arbitrary quantum system. Phys. Rev. A 2012, 86, 013811. [Google Scholar] [CrossRef] [Green Version]
- Dąbrowska, A.M. From a posteriori to a priori solutions for a two-level system interacting with a single-photon wavepacket. J. Opt. Soc. Am. B 2020, 37, 1240–1248. [Google Scholar] [CrossRef]
- Dąbrowska, A.; Chruściński, D.; Chakraborty, S.; Sarbicki, G. Eternally non-Markovian dynamics of a qubit interacting with a single-photon wavepacket. New J. Phys. 2021, 23, 123019. [Google Scholar] [CrossRef]
- Rybár, T.; Filippov, S.N.; Ziman, M.; Bužek, V. Simulation of indivisible qubit channels in collision models. J. Phys. B At. Mol. Opt. Phys. 2012, 45, 154006. [Google Scholar] [CrossRef] [Green Version]
- Filippov, S.N.; Piilo, J.; Maniscalco, S.; Ziman, M. Divisibility of quantum dynamical maps and collision models. Phys. Rev. A 2017, 96, 032111. [Google Scholar] [CrossRef] [Green Version]
- Ciccarello, F.; Palma, G.M.; Giovannetti, V. Collision-model-based approach to non-Markovian quantum dynamics. Phys. Rev. A 2013, 87, 040103. [Google Scholar] [CrossRef]
- Ciccarello, F.; Giovannetti, V. A quantum non-Markovian collision model: Incoherent swap case. Phys. Scr. 2013, T153, 014010. [Google Scholar] [CrossRef] [Green Version]
- Kretschmer, S.; Luoma, K.; Strunz, W.T. Collision model for non-Markovian quantum dynamics. Phys. Rev. A 2016, 94, 012106. [Google Scholar] [CrossRef] [Green Version]
- Campbell, S.; Ciccarello, F.; Palma, G.M.; Vacchini, B. System-environment correlations and Markovian embedding of quantum non-Markovian dynamics. Phys. Rev. A 2018, 98, 012142. [Google Scholar] [CrossRef]
- Lorenzo, S.; Ciccarello, F.; Palma, G.M. Composite quantum collision models. Phys. Rev. A 2017, 96, 032107. [Google Scholar] [CrossRef] [Green Version]
- Pellegrini, C.; Petruccione, F. Non-Markovian quantum repeated interactions and measurements. J. Phys. A Math. Theor. 2009, 42, 425304. [Google Scholar] [CrossRef] [Green Version]
- Cilluffo, D.; Ciccarello, F. Quantum non-Markovian collision models from colored-noise baths. In Advances in Open Systems and Fundamental Tests of Quantum Mechanics, Springer Proceedings in Physics; Vacchini, B., Breuer, H.-P., Bassi, A., Eds.; Springer: Cham, Switzerland, 2019; Volume 237, pp. 29–40. [Google Scholar]
- Taranto, P.; Milz, S.; Pollock, F.A.; Modi, K. Structure of quantum stochastic processes with finite Markov order. Phys. Rev. A 2019, 99, 042108. [Google Scholar] [CrossRef] [Green Version]
- Kretschmann, D.; Werner, R.F. Quantum channels with memory. Phys. Rev. A 2005, 72, 062323. [Google Scholar] [CrossRef] [Green Version]
- Plenio, M.B.; Virmani, S. Spin chains and channels with memory. Phys. Rev. Lett. 2007, 99, 120504. [Google Scholar] [CrossRef] [Green Version]
- Plenio, M.B.; Virmani, S. Many-body physics and the capacity of quantum channels with memory. New J. Phys. 2008, 10, 043032. [Google Scholar] [CrossRef] [Green Version]
- Rybár, T.; Ziman, M. Repeatable quantum memory channels. Phys. Rev. A 2008, 78, 052114. [Google Scholar] [CrossRef] [Green Version]
- Rybár, T.; Ziman, M. Quantum finite-depth memory channels: Case study. Phys. Rev. A 2009, 80, 042306. [Google Scholar] [CrossRef]
- Giovannetti, V.; Palma, G.M. Master equations for correlated quantum channels. Phys. Rev. Lett. 2012, 108, 040401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Giovannetti, V.; Palma, G.M. Master equation for cascade quantum channels: A collisional approach. J. Phys. B At. Mol. Opt. Phys. 2012, 45, 154003. [Google Scholar] [CrossRef]
- Rybár, T.; Ziman, M. Process estimation in the presence of time-invariant memory effects. Phys. Rev. A 2015, 92, 042315. [Google Scholar] [CrossRef] [Green Version]
- Ciccarello, F.; Lorenzo, S.; Giovannetti, V.; Palma, G.M. Quantum collision models: Open system dynamics from repeated interactions. Phys. Rep. 2022, 954, 1–70. [Google Scholar] [CrossRef]
- Campbell, S.; Vacchini, B. Collision models in open system dynamics: A versatile tool for deeper insights? EPL 2021, 133, 60001. [Google Scholar] [CrossRef]
- Affleck, I.; Kennedy, T.; Lieb, E.H.; Tasaki, H. Rigorous results on valence-bond ground states in antiferromagnets. Phys. Rev. Lett. 1987, 59, 799. [Google Scholar] [CrossRef]
- Comar, N.E.; Landi, G.T. Correlations breaking homogenization. Phys. Rev. A 2021, 104, 032217. [Google Scholar] [CrossRef]
- Filippov, S.N.; Luchnikov, I.A. Collisional Open Quantum Dynamics with a Generally Correlated Environment: Exact Solvability in Tensor Networks. Available online: https://arxiv.org/abs/2202.04697 (accessed on 21 February 2022).
- Pérez-García, D.; Verstraete, F.; Wolf, M.M.; Cirac, J.I. Matrix product state representations. Quantum Inf. Comput. 2007, 7, 401. [Google Scholar] [CrossRef]
- Verstraete, F.; Murg, V.; Cirac, J.I. Matrix product states, projected entangled pair states, and variational renormalization group methods for quantum spin systems. Adv. Phys. 2008, 57, 143. [Google Scholar] [CrossRef] [Green Version]
- Schollwöck, U. The density-matrix renormalization group in the age of matrix product states. Ann. Phys. 2011, 326, 96. [Google Scholar] [CrossRef] [Green Version]
- Cirac, J.I.; Pérez-García, D.; Schuch, N.; Verstraete, F. Matrix product states and projected entangled pair states: Concepts, symmetries, theorems. Rev. Mod. Phys. 2021, 93, 045003. [Google Scholar] [CrossRef]
- Orús, R. A practical introduction to tensor networks: Matrix product states and projected entangled pair states. Ann. Phys. 2014, 349, 117–158. [Google Scholar] [CrossRef] [Green Version]
- Orús, R. Tensor networks for complex quantum systems. Nat. Rev. Phys. 2019, 1, 538–550. [Google Scholar] [CrossRef] [Green Version]
- Montangero, S. Introduction to Tensor Network Methods; Springer: New York, NY, USA, 2018. [Google Scholar]
- Vidal, G. Efficient classical simulation of slightly entangled quantum computations. Phys. Rev. Lett. 2003, 91, 147902. [Google Scholar] [CrossRef] [Green Version]
- Luchnikov, I.A.; Ryzhov, A.; Filippov, S.N.; Ouerdane, H. QGOpt: Riemannian optimization for quantum technologies. SciPost Phys. 2021, 10, 079. [Google Scholar] [CrossRef]
- Luchnikov, I.A.; Krechetov, M.E.; Filippov, S.N. Riemannian geometry and automatic differentiation for optimization problems of quantum physics and quantum technologies. New J. Phys. 2021, 23, 073006. [Google Scholar] [CrossRef]
- Verstraete, F.; García-Ripoll, J.J.; Cirac, J.I. Matrix product density operators: Simulation of finite-temperature and dissipative systems. Phys. Rev. Lett. 2004, 93, 207204. [Google Scholar] [CrossRef] [Green Version]
- Zwolak, M.; Vidal, G. Mixed-state dynamics in one-dimensional quantum lattice systems: A time-dependent superoperator renormalization algorithm. Phys. Rev. Lett. 2004, 93, 207205. [Google Scholar] [CrossRef] [Green Version]
- Chen, C.-F.; Kato, K.; Brandão, F.G.S.L. Matrix Product Density Operators: When Do They Have a Local Parent Hamiltonian? Available online: https://arxiv.org/abs/2010.14682 (accessed on 21 February 2022).
- Bondarenko, D. Constructing K-Local Parent Lindbladians for Matrix Product Density Operators. Available online: https://arxiv.org/abs/2110.13134 (accessed on 21 February 2022).
- Wood, C.J.; Biamonte, J.D.; Cory, D.G. Tensor networks and graphical calculus for open quantum systems. Quantum Inf. Comput. 2015, 15, 759–811. [Google Scholar] [CrossRef]
- Dhand, I.; Engelkemeier, M.; Sansoni, L.; Barkhofen, S.; Silberhorn, C.; Plenio, M.B. Proposal for quantum simulation via all-optically-generated tensor network states. Phys. Rev. Lett. 2018, 120, 130501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lubasch, M.; Valido, A.A.; Renema, J.J.; Kolthammer, W.S.; Jaksch, D.; Kim, M.S.; Walmsley, I.; Garcıa-Patrón, R. Tensor network states in time-bin quantum optics. Phys. Rev. A 2018, 97, 062304. [Google Scholar] [CrossRef] [Green Version]
- Istrati, D.; Pilnyak, Y.; Loredo, J.C.; Antón, C.; Somaschi, N.; Hilaire, P.; Ollivier, H.; Esmann, M.; Cohen, L.; Vidro, L.; et al. Sequential generation of linear cluster states from a single photon emitter. Nat. Commun. 2020, 11, 5501. [Google Scholar] [CrossRef] [PubMed]
- Besse, J.-C.; Reuer, K.; Collodo, M.C.; Wulff, A.; Wernli, L.; Copetudo, A.; Malz, D.; Magnard, P.; Akin, A.; Gabureac, M.; et al. Realizing a deterministic source of multipartite-entangled photonic qubits. Nat. Commun. 2020, 11, 4877. [Google Scholar] [CrossRef]
- Tiurev, K.; Appel, M.H.; Mirambell, P.L.; Lauritzen, M.B.; Tiranov, A.; Lodahl, P.; Sørensen, A.S. High-Fidelity Multi-Photon-Entangled Cluster State with Solid-State Quantum Emitters in Photonic Nanostructures. Available online: https://arxiv.org/abs/2007.09295 (accessed on 21 February 2022).
- Wei, Z.-Y.; Malz, D.; González-Tudela, A.; Cirac, J.I. Generation of photonic matrix product states with Rydberg atomic arrays. Phys. Rev. Res. 2021, 3, 023021. [Google Scholar] [CrossRef]
- Dalzell, A.M.; Brandão, F.G.S.L. Locally accurate MPS approximations for ground states of one-dimensional gapped local Hamiltonians. Quantum 2019, 3, 187. [Google Scholar] [CrossRef]
- Pollock, F.A.; Rodríguez-Rosario, C.; Frauenheim, T.; Paternostro, M.; Modi, K. Non-Markovian quantum processes: Complete framework and efficient characterization. Phys. Rev. A 2018, 97, 012127. [Google Scholar] [CrossRef] [Green Version]
- Pollock, F.A.; Rodríguez-Rosario, C.; Frauenheim, T.; Paternostro, M.; Modi, K. Operational Markov condition for quantum processes. Phys. Rev. Lett. 2018, 120, 040405. [Google Scholar] [CrossRef] [Green Version]
- White, G.A.L.; Hill, C.D.; Pollock, F.A.; Hollenberg, L.C.L.; Modi, K. Demonstration of non-Markovian process characterisation and control on a quantum processor. Nat. Commun. 2020, 11, 6301. [Google Scholar] [CrossRef]
- Taranto, P. Memory effects in quantum processes. Int. J. Quantum Inf. 2020, 18, 1941002. [Google Scholar] [CrossRef]
- Luchnikov, I.A.; Vintskevich, S.V.; Ouerdane, H.; Filippov, S.N. Simulation complexity of open quantum dynamics: Connection with tensor networks. Phys. Rev. Lett. 2019, 122, 160401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Luchnikov, I.A.; Vintskevich, S.V.; Grigoriev, D.A.; Filippov, S.N. Machine learning non-Markovian quantum dynamics. Phys. Rev. Lett. 2020, 124, 140502. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chruściński, D.; Maniscalco, S. Degree of non-Markovianity of quantum evolution. Phys. Rev. Lett. 2014, 112, 120404. [Google Scholar] [CrossRef] [Green Version]
- Filippov, S.N.; Glinov, A.N.; Leppäjärvi, L. Phase covariant qubit dynamics and divisibility. Lobachevskii J. Math. 2020, 41, 617–630. [Google Scholar] [CrossRef]
- Breuer, H.-P.; Petruccione, F. The Theory of Open Quantum Systems; Oxford University Press: Oxford, UK, 2002; Chapter 9. [Google Scholar]
- Nakajima, S. On quantum theory of transport phenomena: Steady diffusion. Prog. Theor. Phys. 1958, 20, 948–959. [Google Scholar] [CrossRef] [Green Version]
- Zwanzig, R. Ensemble method in the theory of irreversibility. J. Chem. Phys. 1960, 33, 1338–1341. [Google Scholar] [CrossRef]
- Luchnikov, I.A.; Filippov, S.N. Quantum evolution in the stroboscopic limit of repeated measurements. Phys. Rev. A 2017, 95, 022113. [Google Scholar] [CrossRef] [Green Version]
- Grimaudo, R.; Messina, A.; Sergi, A.; Vitanov, N.V.; Filippov, S.N. Two-qubit entanglement generation through non-Hermitian Hamiltonians induced by repeated measurements on an ancilla. Entropy 2020, 22, 1184. [Google Scholar] [CrossRef]
- Chruściński, D.; Kossakowski, A. Non-Markovian quantum dynamics: Local versus nonlocal. Phys. Rev. Lett. 2010, 104, 070406. [Google Scholar] [CrossRef] [Green Version]
- Smirne, A.; Vacchini, B. Nakajima-Zwanzig versus time-convolutionless master equation for the non-Markovian dynamics of a two-level system. Phys. Rev. A 2010, 82, 022110. [Google Scholar] [CrossRef] [Green Version]
- Filippov, S.N.; Chruściński, D. Time deformations of master equations. Phys. Rev. A 2018, 98, 022123. [Google Scholar] [CrossRef] [Green Version]
- Gorini, V.; Kossakowski, A.; Sudarshan, E.C.G. Completely positive dynamical semigroups of n-level systems. J. Math. Phys. 1976, 17, 821. [Google Scholar] [CrossRef]
- Lindblad, G. On the generators of quantum dynamical semigroups. Comm. Math. Phys. 1976, 48, 119. [Google Scholar] [CrossRef]
- von Waldenfels, W. An approach to the theory of pressure broadening of spectral lines. In Probability and Information Theory II; Behara, M., Krickeberg, K., Wolfowitz, J., Eds.; Springer: Berlin/Heidelberg, Germany, 1973; pp. 19–69. [Google Scholar]
- Hegerfeldt, G.C.; Schulze, H. Noncommutative cumulants for stochastic differential equations and for generalized Dyson series. J. Stat. Phys. 1988, 51, 691–710. [Google Scholar] [CrossRef]
- Pechen, A.N. The multitime correlation functions, free white noise, and the generalized Poisson statistics in the low density limit. J. Math. Phys. 2006, 47, 033507. [Google Scholar] [CrossRef] [Green Version]
- Nosal’, I.A.; Teretenkov, A.E. Exact dynamics of moments and correlation functions for GKSL fermionic equations of Poisson type. Math Notes 2020, 108, 911–915. [Google Scholar] [CrossRef]
- Gherardini, S.; Smirne, A.; Huelga, S.F.; Caruso, F. Transfer-tensor description of memory effects in open-system dynamics and multi-time statistics. Quantum Sci. Technol. 2022, 7, 025005. [Google Scholar] [CrossRef]
- Hübener, R.; Mari, A.; Eisert, J. Wick’s theorem for matrix product states. Phys. Rev. Lett. 2013, 110, 040401. [Google Scholar] [CrossRef] [Green Version]
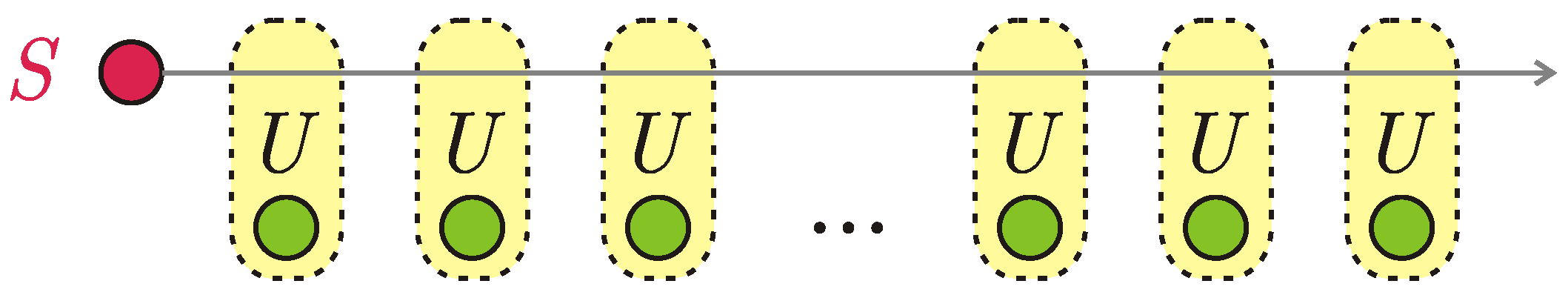

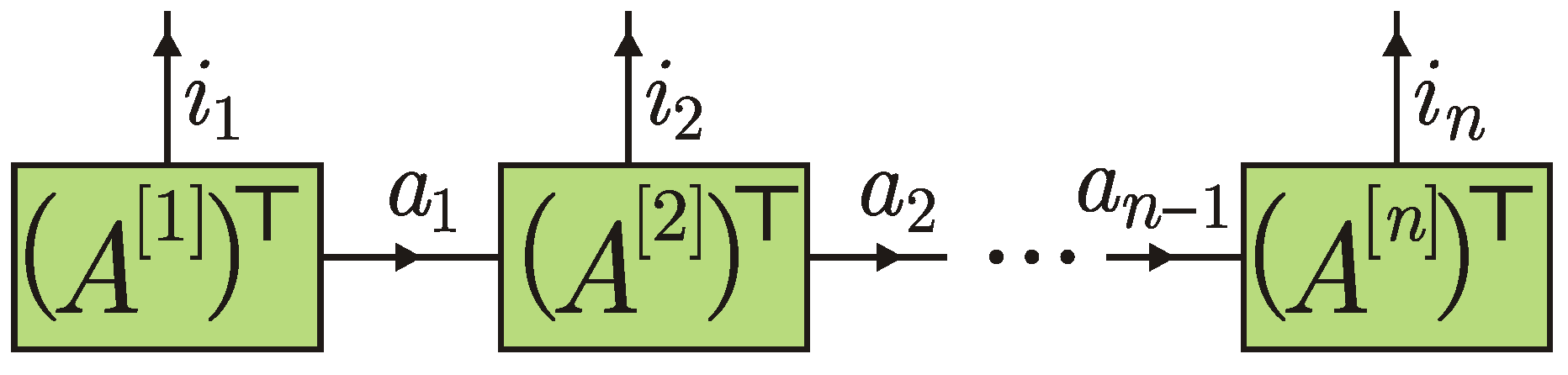



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Filippov, S. Multipartite Correlations in Quantum Collision Models. Entropy 2022, 24, 508. https://doi.org/10.3390/e24040508
Filippov S. Multipartite Correlations in Quantum Collision Models. Entropy. 2022; 24(4):508. https://doi.org/10.3390/e24040508
Chicago/Turabian StyleFilippov, Sergey. 2022. "Multipartite Correlations in Quantum Collision Models" Entropy 24, no. 4: 508. https://doi.org/10.3390/e24040508
APA StyleFilippov, S. (2022). Multipartite Correlations in Quantum Collision Models. Entropy, 24(4), 508. https://doi.org/10.3390/e24040508





