Steady-State Thermodynamics of a Cascaded Collision Model
Abstract
:1. Introduction
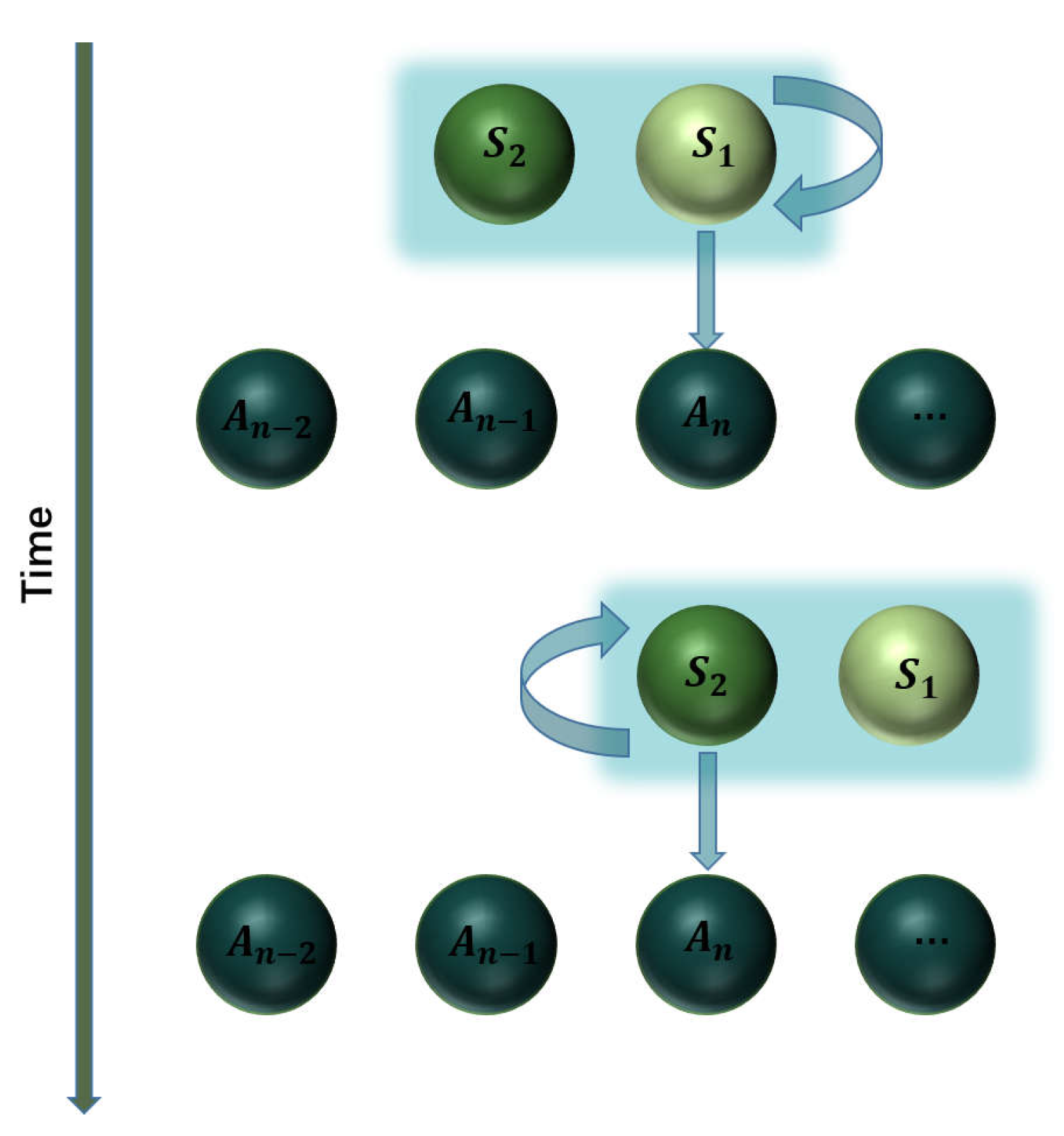
2. The Model and Master Equation
3. Thermodynamic Quantities of the Cascaded Model
4. Demonstration by Two-Level System
4.1. The Interaction of Is Energy-Preserving
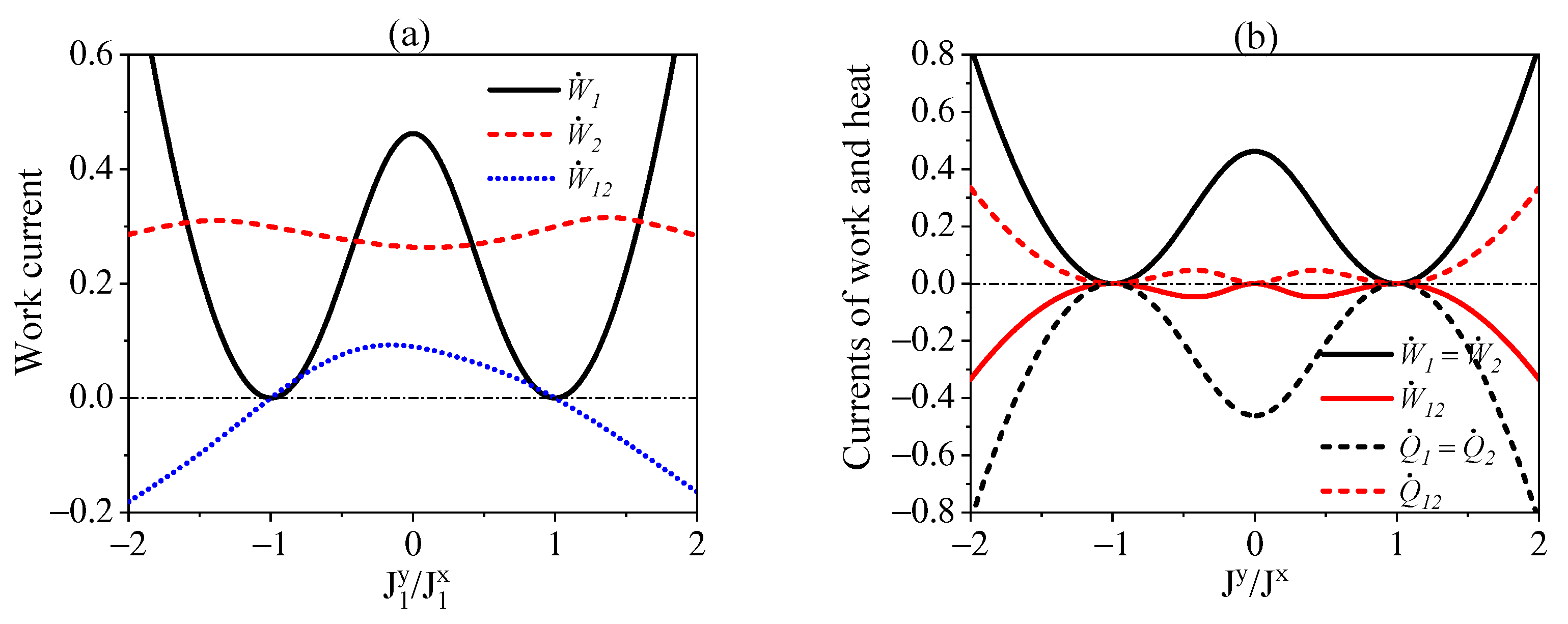
4.2. Both the Interactions of and Are Not Energy-Preserving
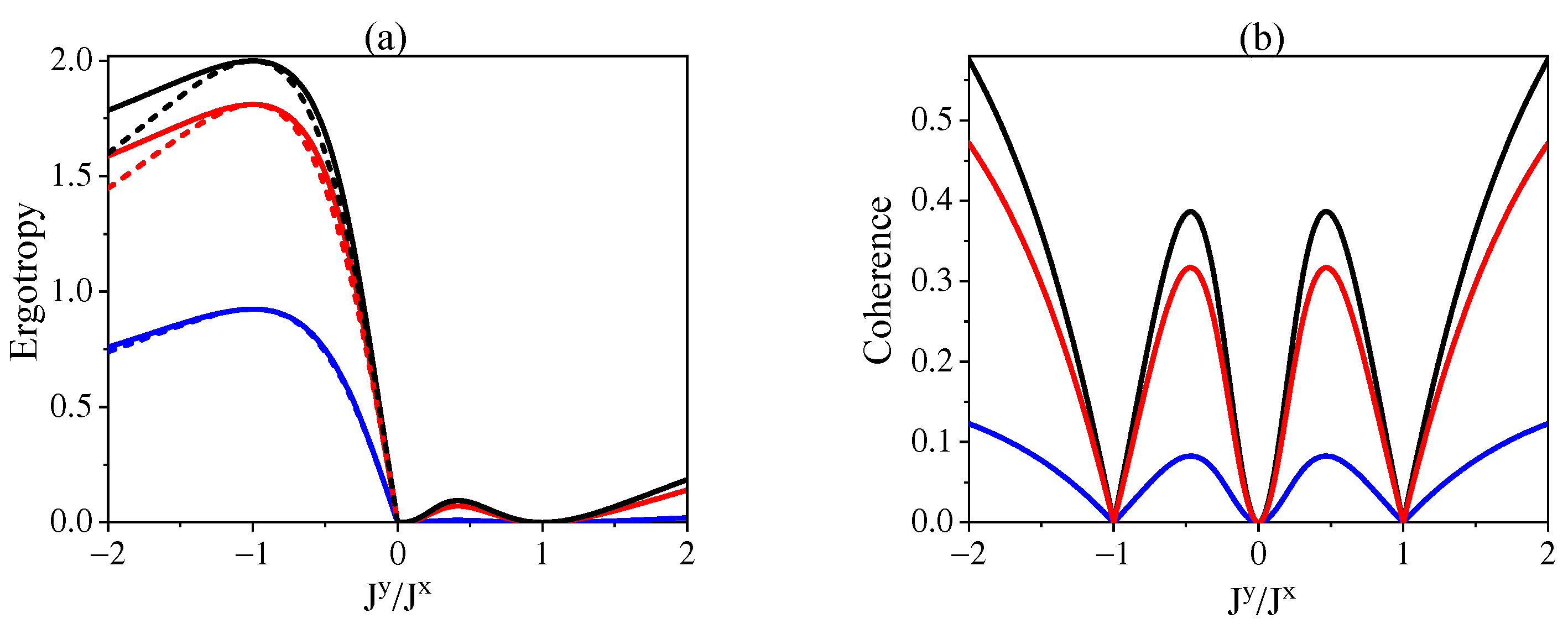
5. The Extractable Work in Cascaded Model
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Gemma, G.; Michel, M.; Mahler, G. Quantum Thermodynamics; Springer: Berlin/Heidelberg, Germany, 2004. [Google Scholar]
- Kosloff, R. Quantum Thermodynamics: A Dynamical Viewpoint. Entropy 2013, 15, 2100. [Google Scholar] [CrossRef] [Green Version]
- Breuer, H.; Petruccione, F. The Theory of Open Quantum Systems; Oxford University Press: Oxford, NY, USA, 2002. [Google Scholar]
- Scully, M.O.; Zubairy, M.S.; Agarwal, G.S.; Walther, H. Extracting Work from a Single Heat Bath via Vanishing Quantum Coherence. Science 2003, 299, 862. [Google Scholar] [CrossRef]
- Türkpençe, D.; Müstecaplıoǧlu, O.E. Quantum fuel with multilevel atomic coherence for ultrahigh specific work in a photonic Carnot engine. Phys. Rev. E 2016, 93, 012145. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Shi, Y.H.; Shi, H.L.; Wang, X.H.; Hu, M.L.; Liu, S.Y.; Yang, W.L.; Fan, H. Quantum coherence in a quantum heat engine. J. Phys. A 2020, 53, 085301. [Google Scholar] [CrossRef] [Green Version]
- Mehta, V.; Johal, R.S. Quantum Otto engine with exchange coupling in the presence of level degeneracy. Phys. Rev. E 2017, 96, 032110. [Google Scholar] [CrossRef] [Green Version]
- Dillenschneider, R.; Lutz, E. Energetics of quantum correlations. EPL (Europhys. Lett.) 2009, 88, 50003. [Google Scholar] [CrossRef] [Green Version]
- Brandner, K.; Bauer, M.; Schmid, M.T.; Seifert, U. Coherence-enhanced efficiency of feedback-driven quantum engines. New J. Phys. 2015, 17, 065006. [Google Scholar] [CrossRef]
- Brunner, N.; Huber, M.; Linden, N.; Popescu, S.; Silva, R.; Skrzypczyk, P. Entanglement enhances cooling in microscopic quantum refrigerators. Phys. Rev. E 2014, 89, 032115. [Google Scholar] [CrossRef] [Green Version]
- Niedenzu, W.; Gelbwaser-Klimovsky, D.; Kofman, A.G.; Kurizki, G. On the operation of machines powered by quantum non-thermal baths. New J. Phys. 2016, 18, 083012. [Google Scholar] [CrossRef] [Green Version]
- Uzdin, R. Coherence-Induced Reversibility and Collective Operation of Quantum Heat Machines via Coherence Recycling. Phys. Rev. Appl. 2016, 6, 024004. [Google Scholar] [CrossRef]
- Doyeux, P.; Leggio, B.; Messina, R.; Antezza, M. Quantum thermal machine acting on a many-body quantum system: Role of correlations in thermodynamic tasks. Phys. Rev. E 2016, 93, 022134. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Latune, C.; Sinayskiy, I.; Petruccione, F. Quantum coherence, many-body correlations, and non-thermal effects for autonomous thermal machines. Sci. Rep. 2019, 9, 3191. [Google Scholar] [CrossRef] [Green Version]
- Åberg, J. Catalytic Coherence. Phys. Rev. Lett. 2014, 113, 150402. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Korzekwa, K.; Lostaglio, M.; Oppenheim, J.; Jennings, D. The extraction of work from quantum coherence. New J. Phys. 2016, 18, 023045. [Google Scholar] [CrossRef]
- Li, H.; Zou, J.; Yu, W.L.; Xu, B.M.; Li, J.G.; Shao, B. Quantum coherence rather than quantum correlations reflect the effects of a reservoir on a system’s work capability. Phys. Rev. E 2014, 89, 052132. [Google Scholar] [CrossRef] [Green Version]
- Liao, J.Q.; Dong, H.; Sun, C.P. Single-particle machine for quantum thermalization. Phys. Rev. A 2010, 81, 052121. [Google Scholar] [CrossRef] [Green Version]
- Manatuly, A.; Niedenzu, W.; Román-Ancheyta, R.; Çakmak, B.; Müstecaplıoğlu, O.E.; Kurizki, G. Collectively enhanced thermalization via multiqubit collisions. Phys. Rev. E 2019, 99, 042145. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Daǧ, C.B.; Niedenzu, W.; Ozaydin, F.; Müstecaplıoǧlu, O.E.; Kurizki, G. Temperature Control in Dissipative Cavities by Entangled Dimers. J. Phys. Chem. C 2019, 123, 4035. [Google Scholar] [CrossRef] [Green Version]
- Karimi, B.; Pekola, J.P. Correlated versus uncorrelated noise acting on a quantum refrigerator. Phys. Rev. B 2017, 96, 115408. [Google Scholar] [CrossRef] [Green Version]
- Manzano, G.; Giorgi, G.L.; Fazio, R.; Zambrini, R. Boosting the performance of small autonomous refrigerators via common environmental effects. New J. Phys. 2019, 21, 123026. [Google Scholar] [CrossRef]
- Wang, C.; Xu, D.; Liu, H.; Gao, X. Thermal rectification and heat amplification in a nonequilibrium V-type three-level system. Phys. Rev. E 2019, 99, 042102. [Google Scholar] [CrossRef] [Green Version]
- Man, Z.X.; Tavakoli, A.; Brask, J.B.; Xia, Y.J. Improving autonomous thermal entanglement generation using a common reservoir. Phys. Scr. 2019, 94, 075101. [Google Scholar] [CrossRef] [Green Version]
- Lorenzo, S.; Farace, A.; Ciccarello, F.; Palma, G.M.; Giovannetti, V. Heat flux and quantum correlations in dissipative cascaded systems. Phys. Rev. A 2015, 91, 022121. [Google Scholar] [CrossRef]
- Giovannetti, V.; Palma, G.M. Master Equations for Correlated Quantum Channels. Phys. Rev. Lett. 2012, 108, 040401. [Google Scholar] [CrossRef] [Green Version]
- Giovannetti, V.; Palma, G.M. Master equation for cascade quantum channels: A collisional approach. J. Phys. B 2012, 45, 154003. [Google Scholar] [CrossRef]
- Lorenzo, S.; McCloskey, R.; Ciccarello, F.; Paternostro, M.; Palma, G.M. Landauer’s Principle in Multipartite Open Quantum System Dynamics. Phys. Rev. Lett. 2015, 115, 120403. [Google Scholar] [CrossRef]
- Man, Z.X.; Xia, Y.J.; An, N.B. Heat fluxes in a two-qubit cascaded system due to coherences of a non-thermal bath. J. Phys. B 2020, 53, 205505. [Google Scholar] [CrossRef]
- Stockburger, J.T.; Motz, T. Thermodynamic deficiencies of some simple lindblad operators. Fortschr. Phys. 2016, 65, 1600067. [Google Scholar] [CrossRef] [Green Version]
- Kołodyński, J.; Brask, J.B.; Perarnau-Llobet, M.; Bylicka, B. Adding dynamical generators in quantum master equations. Phys. Rev. A 2018, 97, 062124. [Google Scholar] [CrossRef] [Green Version]
- Correa, L.A.; Palao, J.P.; Alonso, D. Internal dissipation and heat leaks in quantum thermodynamic cycles. Phys. Rev. E 2015, 92, 032136. [Google Scholar] [CrossRef] [Green Version]
- Hofer, P.P.; Perarnau-Llobet, M.; Miranda, L.D.M.; Haack, G.; Silva, R.; Brask, J.B.; Brunner, N. Markovian master equations for quantum thermal machines: Local versus global approach. New J. Phys. 2017, 19, 123037. [Google Scholar] [CrossRef]
- Mitchison, M.T.; Plenio, M.B. Non-additive dissipation in open quantum networks out of equilibrium. New J. Phys. 2018, 20, 033005. [Google Scholar] [CrossRef] [Green Version]
- Naseem, M.T.; Xuereb, A.; Müstecaplıoğlu, O.E. Thermodynamic consistency of the optomechanical master equation. Phys. Rev. A 2018, 98, 052123. [Google Scholar] [CrossRef] [Green Version]
- Purkayastha, A.; Dhar, A.; Kulkarni, M. Out-of-equilibrium open quantum systems: A comparison of approximate quantum master equation approaches with exact results. Phys. Rev. A 2016, 93, 062114. [Google Scholar] [CrossRef] [Green Version]
- Wichterich, H.; Henrich, M.J.; Breuer, H.P.; Gemmer, J.; Michel, M. Modeling heat transport through completely positive maps. Phys. Rev. E 2007, 76, 031115. [Google Scholar] [CrossRef] [Green Version]
- Cattaneo, M.; Giorgi, G.L.; Maniscalco, S.; Zambrini, R. Local versus global master equation with common and separate baths: Superiority of the global approach in partial secular approximation. New J. Phys. 2019, 21, 113045. [Google Scholar] [CrossRef]
- Scali, S.; Anders, J.; Correa, L.A. Local master equations bypass the secular approximation. arXiv 2009, arXiv:2009.11324. [Google Scholar] [CrossRef]
- Hewgill, A.; De Chiara, G.; Imparato, A. Quantum thermodynamically consistent local master equations. Phys. Rev. Res. 2021, 3, 013165. [Google Scholar] [CrossRef]
- Levy, A.; Kosloff, R. The local approach to quantum transport may violate the second law of thermodynamics. EPL (Europhys. Lett.) 2014, 107, 20004. [Google Scholar] [CrossRef] [Green Version]
- Rau, J. Relaxation Phenomena in Spin and Harmonic Oscillator Systems. Phys. Rev. 1963, 129, 1880. [Google Scholar] [CrossRef]
- Cattaneo, M.; De Chiara, G.; Maniscalco, S.; Zambrini, R.; Giorgi, G.L. Collision Models Can Efficiently Simulate Any Multipartite Markovian Quantum Dynamics. Phys. Rev. Lett. 2021, 126, 130403. [Google Scholar] [CrossRef]
- Scarani, V.; Ziman, M.; Stelmachovic, P.; Gisin, N.; Bužek, V. Thermalizing Quantum Machines: Dissipation and Entanglement. Phys. Rev. Lett. 2002, 88, 097905. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Ziman, M.; Stelmachovic, P.; Bužek, V.; Hillery, M.; Scarani, V.; Gisin, N. Diluting quantum information: An analysis of information transfer in system-reservoir interactions. Phys. Rev. A 2002, 65, 042105. [Google Scholar] [CrossRef] [Green Version]
- Ziman, M.; Bužek, V. All (qubit) decoherences: Complete characterization and physical implementation. Phys. Rev. A 2005, 72, 022110. [Google Scholar] [CrossRef] [Green Version]
- Benenti, G.; Palma, G.M. Reversible and irreversible dynamics of a qubit interacting with a small environment. Phys. Rev. A 2007, 75, 052110. [Google Scholar] [CrossRef] [Green Version]
- Gennaro, G.; Benenti, G.; Palma, G.M. Relaxation due to random collisions with a many-qudit environment. Phys. Rev. A 2009, 79, 022105. [Google Scholar] [CrossRef] [Green Version]
- Campbell, S.; Vacchini, B. Collision models in open system dynamics: A versatile tool for deeper insights? EPL 2021, 133, 60001. [Google Scholar] [CrossRef]
- Ciccarello, F.; Lorenzo, S.; Giovannetti, V.; Palma, G.M. Quantum collision models: Open system dynamics from repeated interactions. Phys. Rep. 2022, 954, 1–70. [Google Scholar] [CrossRef]
- Ciccarello, F.; Palma, G.M.; Giovannetti, V. Collision-model-based approach to non-Markovian quantum dynamics. Phys. Rev. A 2013, 87, 040103. [Google Scholar] [CrossRef]
- Kretschmer, S.; Luoma, K.; Strunz, W.T. Collision model for non-Markovian quantum dynamics. Phys. Rev. A 2016, 94, 012106. [Google Scholar] [CrossRef] [Green Version]
- Lorenzo, S.; Ciccarello, F.; Palma, G.M. Class of exact memory-kernel master equations. Phys. Rev. A 2016, 93, 052111. [Google Scholar] [CrossRef] [Green Version]
- Bernardes, N.K.; Carvalho, A.R.R.; Monken, C.H.; Santos, M.F. Coarse graining a non-Markovian collisional model. Phys. Rev. A 2017, 95, 032117. [Google Scholar] [CrossRef] [Green Version]
- Çakmak, B.; Pezzutto, M.; Paternostro, M.; Müstecaplioğlu, O.E. Non-Markovianity, coherence, and system-environment correlations in a long-range collision model. Phys. Rev. A 2017, 96, 022109. [Google Scholar] [CrossRef] [Green Version]
- Lorenzo, S.; Ciccarello, F.; Palma, G.M. Composite quantum collision models. Phys. Rev. A 2017, 96, 032107. [Google Scholar] [CrossRef] [Green Version]
- Filippov, S.N.; Piilo, J.; Maniscalco, S.; Ziman, M. Divisibility of quantum dynamical maps and collision models. Phys. Rev. A 2017, 96, 032111. [Google Scholar] [CrossRef] [Green Version]
- Mccloskey, R.; Paternostro, M. Non-Markovianity and system-environment correlations in a microscopic collision model. Phys. Rev. A 2014, 89, 052120. [Google Scholar] [CrossRef] [Green Version]
- Bernardes, N.K.; Carvalho, A.R.R.; Monken, C.H.; Santos, M.F. Environmental correlations and Markovian to non-Markovian transitions in collisional models. Phys. Rev. A 2014, 90, 032111. [Google Scholar] [CrossRef] [Green Version]
- Jin, J.; Yu, C.S. Non-Markovianity in the collision model with environmental block. New J. Phys. 2018, 20, 053026. [Google Scholar] [CrossRef]
- Man, Z.X.; Xia, Y.J.; Lo Franco, R. Temperature effects on quantum non-Markovianity via collision models. Phys. Rev. A 2018, 97, 062104. [Google Scholar] [CrossRef] [Green Version]
- Barra, F. The thermodynamic cost of driving quantum systems by their boundaries. Sci. Rep. 2015, 5, 14873. [Google Scholar] [CrossRef] [Green Version]
- Chiara, G.D.; Landi, G.; Hewgill, A.; Reid, B.; Ferraro, A.; Roncaglia, A.J.; Antezza, M. Reconciliation of quantum local master equations with thermodynamics. New J. Phys. 2018, 20, 113024. [Google Scholar] [CrossRef]
- Karevski, D.; Platini, T. Quantum Nonequilibrium Steady States Induced by Repeated Interactions. Phys. Rev. Lett. 2009, 102, 207207. [Google Scholar] [CrossRef] [Green Version]
- Barra, F.; Lledó, C. Stochastic thermodynamics of quantum maps with and without equilibrium. Phys. Rev. E 2017, 96, 052114. [Google Scholar] [CrossRef] [Green Version]
- Cusumano, S.; Cavina, V.; Keck, M.; De Pasquale, A.; Giovannetti, V. Entropy production and asymptotic factorization via thermalization: A collisional model approach. Phys. Rev. A 2018, 98, 032119. [Google Scholar] [CrossRef] [Green Version]
- Seah, S.; Nimmrichter, S.; Scarani, V. Nonequilibrium dynamics with finite-time repeated interactions. Phys. Rev. E 2019, 99, 042103. [Google Scholar] [CrossRef] [Green Version]
- Arısoy, O.; Campbell, S.; Müstecaplıoǧlu, O.E. Thermalization of Finite Many-Body Systems by a Collision Model. Entropy 2019, 21, 1182. [Google Scholar] [CrossRef] [Green Version]
- Landi, G.T.; Novais, E.; de Oliveira, M.J.; Karevski, D. Flux rectification in the quantum XXZ chain. Phys. Rev. E 2014, 90, 042142. [Google Scholar] [CrossRef] [Green Version]
- Seah, S.; Nimmrichter, S.; Grimmer, D.; Santos, J.P.; Scarani, V.; Landi, G.T. Collisional Quantum Thermometry. Phys. Rev. Lett. 2019, 123, 180602. [Google Scholar] [CrossRef] [Green Version]
- Barra, F. Dissipative Charging of a Quantum Battery. Phys. Rev. Lett. 2019, 122, 210601. [Google Scholar] [CrossRef] [Green Version]
- Daǧ, C.B.; Niedenzu, W.; Müstecaplıoǧlu, O.E.; Kurizki, G. Multiatom Quantum Coherences in Micromasers as Fuel for Thermal and Nonthermal Machines. Entropy 2016, 18, 244. [Google Scholar] [CrossRef] [Green Version]
- Hewgill, A.; González, J.O.; Palao, J.P.; Alonso, D.; Ferraro, A.; De Chiara, G. Three-qubit refrigerator with two-body interactions. Phys. Rev. E 2020, 101, 012109. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- De Chiara, G.; Antezza, M. Quantum machines powered by correlated baths. Phys. Rev. Res. 2020, 2, 033315. [Google Scholar] [CrossRef]
- Pezzutto, M.; Paternostro, M.; Omar, Y. Implications of non-Markovian quantum dynamics for the Landauer bound. New J. Phys. 2016, 18, 123018. [Google Scholar] [CrossRef]
- Man, Z.X.; Xia, Y.J.; Lo Franco, R. Validity of the Landauer principle and quantum memory effects via collisional models. Phys. Rev. A 2019, 99, 042106. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Q.; Man, Z.X.; Xia, Y.J. Non-Markovianity and the Landauer principle in composite thermal environments. Phys. Rev. A 2021, 103, 032201. [Google Scholar] [CrossRef]
- Pezzutto, M.; Paternostro, M.; Omar, Y. An out-of-equilibrium non-Markovian quantum heat engine. Quantum Sci. Technol. 2019, 4, 025002. [Google Scholar] [CrossRef] [Green Version]
- Abah, O.; Paternostro, M. Implications of non-Markovian dynamics on information-driven engine. J. Phys. Commun. 2020, 4, 085016. [Google Scholar] [CrossRef]
- Baumgratz, T.; Cramer, M.; Plenio, M.B. Quantifying Coherence. Phys. Rev. Lett. 2014, 113, 140401. [Google Scholar] [CrossRef] [Green Version]
- Allahverdyan, A.E.; Balian, R.; Nieuwenhuizen, T.M. Maximal work extraction from finite quantum systems. EPL (Europhys. Lett.) 2004, 67, 565. [Google Scholar] [CrossRef] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, L.; Man, Z.-X.; Xia, Y.-J. Steady-State Thermodynamics of a Cascaded Collision Model. Entropy 2022, 24, 644. https://doi.org/10.3390/e24050644
Li L, Man Z-X, Xia Y-J. Steady-State Thermodynamics of a Cascaded Collision Model. Entropy. 2022; 24(5):644. https://doi.org/10.3390/e24050644
Chicago/Turabian StyleLi, Lu, Zhong-Xiao Man, and Yun-Jie Xia. 2022. "Steady-State Thermodynamics of a Cascaded Collision Model" Entropy 24, no. 5: 644. https://doi.org/10.3390/e24050644
APA StyleLi, L., Man, Z.-X., & Xia, Y.-J. (2022). Steady-State Thermodynamics of a Cascaded Collision Model. Entropy, 24(5), 644. https://doi.org/10.3390/e24050644





